The Role of Asymmetry on the Resonances of Conjoined Split-Ring Resonators
Abstract
1. Introduction
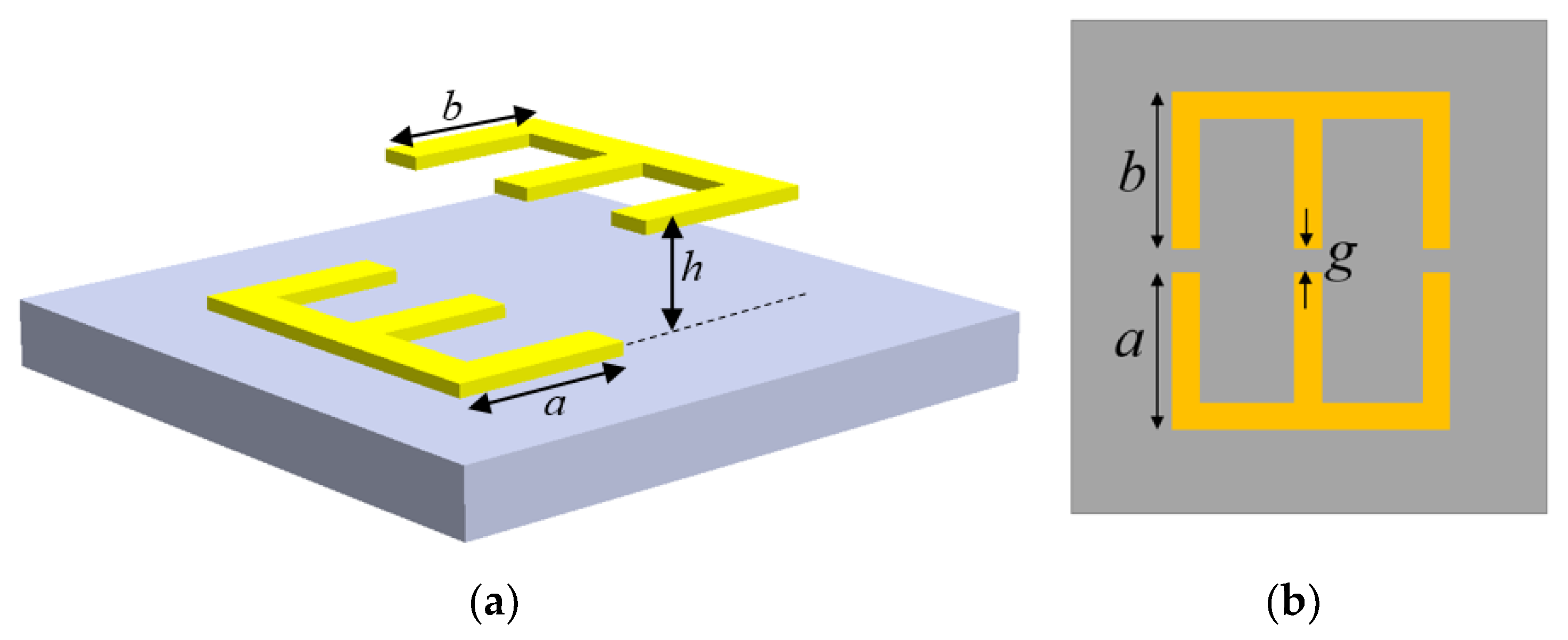
2. Materials and Methods
3. Results and Discussion
3.1. Symmetric Changes in Both Sides
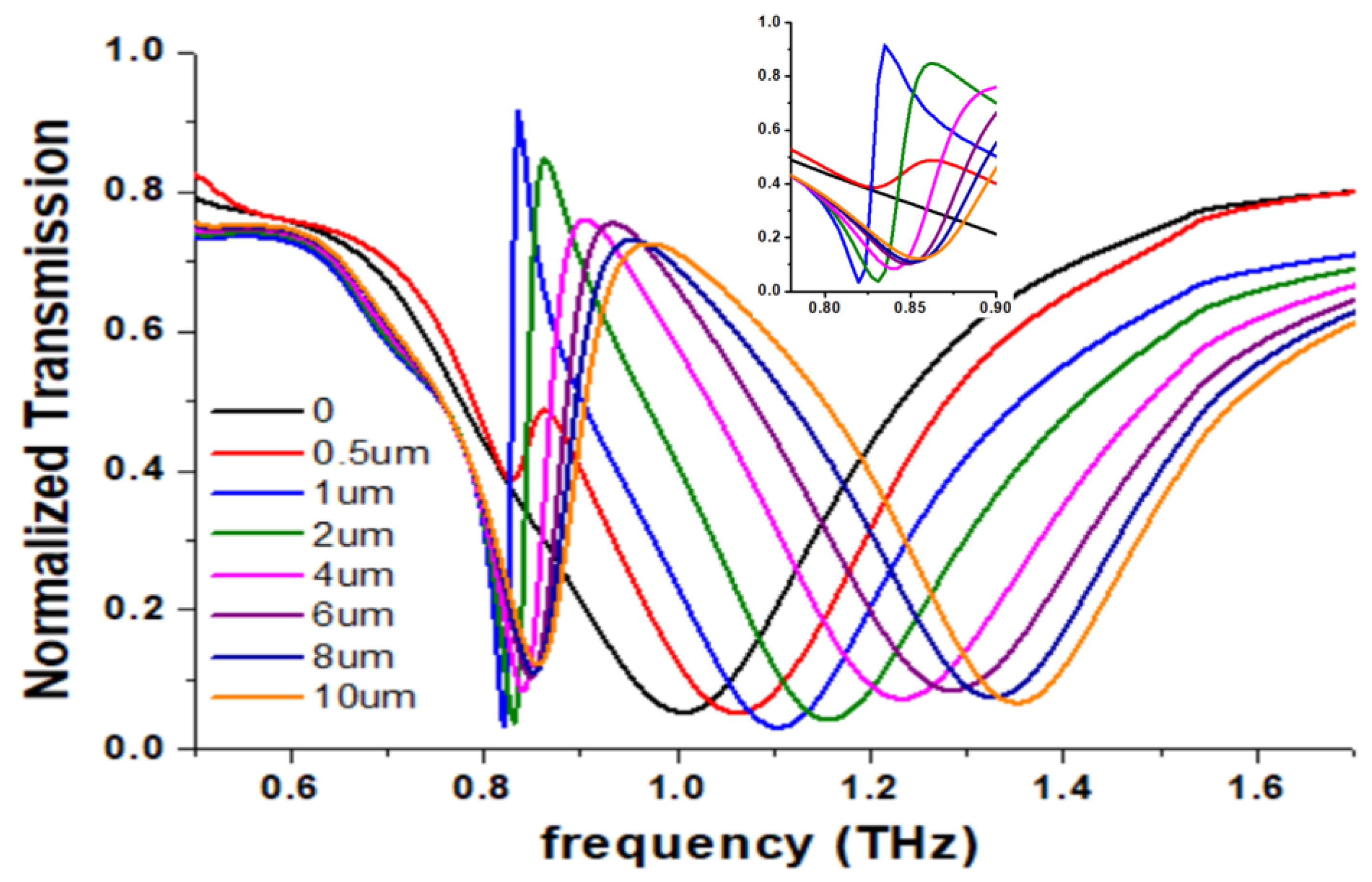
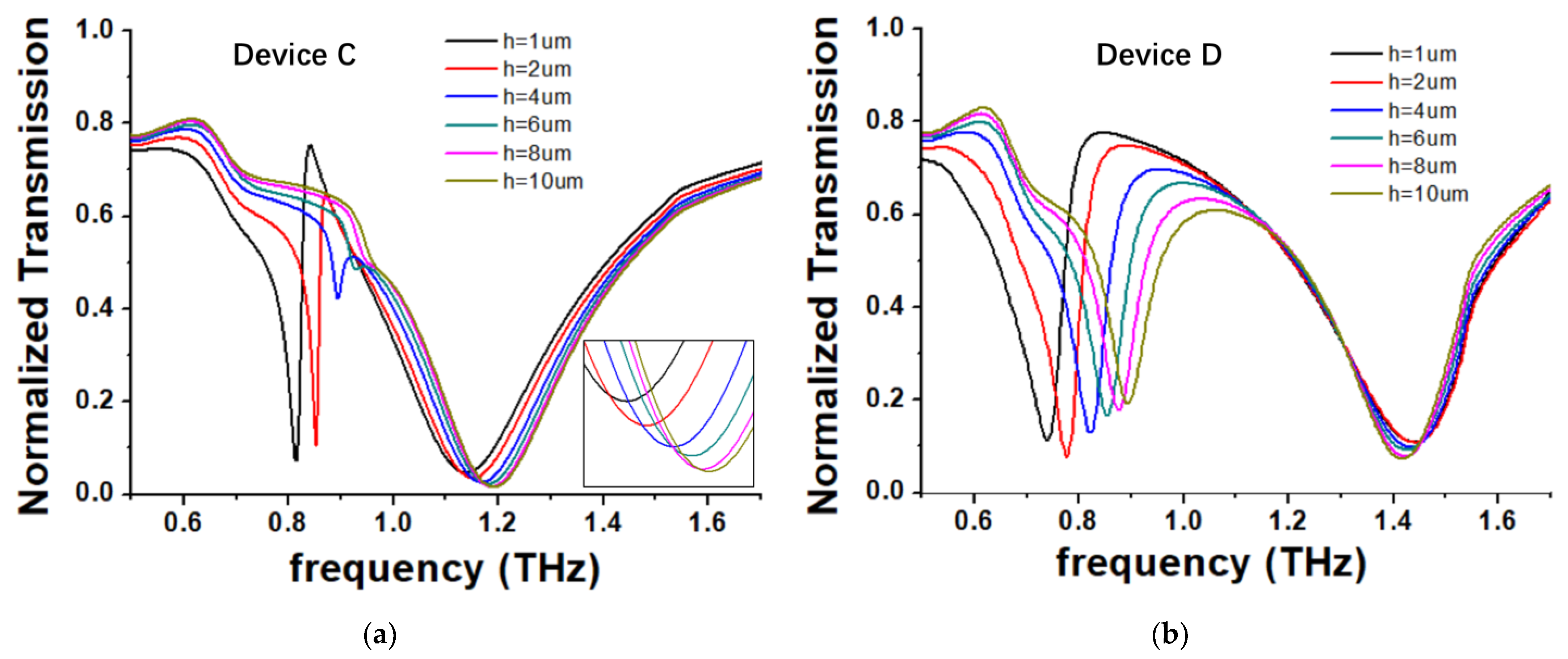
3.2. Asymmetric Changes in Both Sides
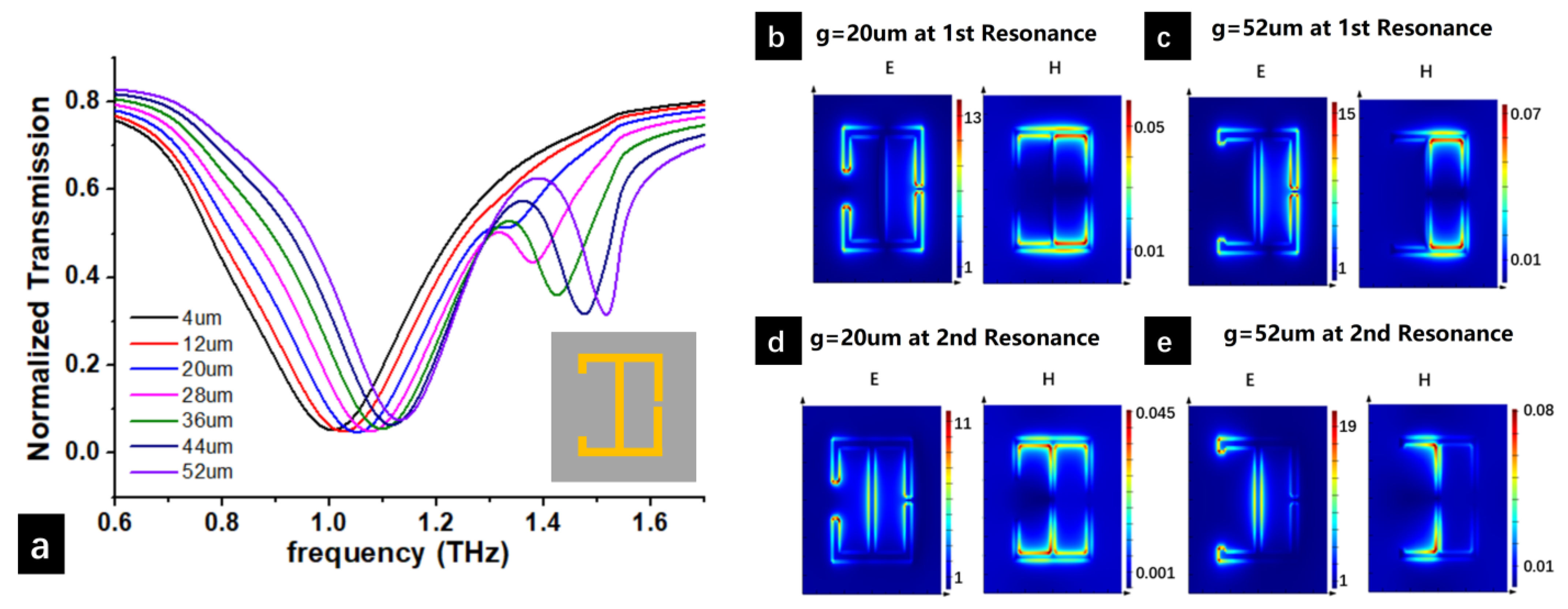
3.3. Spatial Asymmetry in Co-SRR
3.4. Comparison with Previously Reported THz Metamaterial Modulators and Sensors
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Co-SRR | conjoined split-ring resonator |
| THz | terahertz |
| EM | electromagnetic |
| SRR | split-ring resonator |
| SDR | split-disk resonator |
| DSRR | double split-ring resonator |
| MD | modulation depth |
| Q | quality factor |
| EIT | electromagnetic-induced transparency |
| FSR | free spectral range |
| FOM | figure of merit |
| FDTD | finite-difference time-domain |
| PML | perfectly matched layer |
References
- Chen, H.T.; Padilla, W.J.; Zide, J.M.; Gossard, A.C.; Taylor, A.J.; Averitt, R.D. Active terahertz metamaterial devices. Nature 2006, 444, 597–600. [Google Scholar] [CrossRef] [PubMed]
- Choi, M.; Lee, S.H.; Kim, Y.; Kang, S.B.; Shin, J.; Kwak, M.H.; Kang, K.Y.; Lee, Y.H.; Park, N.; Min, B. A terahertz metamaterial with unnaturally high refractive index. Nature 2011, 470, 369–373. [Google Scholar] [CrossRef] [PubMed]
- Lee, D.K.; Kang, J.H.; Kwon, J.; Lee, J.S.; Lee, S.; Woo, D.H.; Kim, J.H.; Song, C.S.; Park, Q.H.; Seo, M. Nano metamaterials for ultrasensitive Terahertz biosensing. Sci. Rep. 2017, 7, 8146. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Niu, J.; Jin, X.; Qian, X.; Xiao, C.; Wang, W. Molecularly Resonant Metamaterials for Broad-Band Electromagnetic Stealth. Adv. Sci. 2023, 10, 2301170. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Zhang, X.; Xu, Q.; Tian, C.; Wang, Q.; Xu, Y.; Li, Y.; Gu, J.; Tian, Z.; Ouyang, C.; et al. High-efficiency dielectric metasurfaces for polarization-dependent terahertz wavefront manipulation. Adv. Opt. Mater. 2018, 6, 1700773. [Google Scholar] [CrossRef]
- Saadeldin, A.S.; Hameed, M.F.O.; Elkaramany, E.M.; Obayya, S.S. Highly sensitive terahertz metamaterial sensor. IEEE Sens. J. 2019, 19, 7993–7999. [Google Scholar] [CrossRef]
- Yuan, G.; Rogers, K.S.; Rogers, E.T.; Zheludev, N.I. Far-field superoscillatory metamaterial superlens. Phys. Rev. Appl. 2019, 11, 064016. [Google Scholar] [CrossRef]
- Alrayes, N.; Hussein, M.I. Metamaterial-based sensor design using split ring resonator and Hilbert fractal for biomedical application. Sens. Bio-Sens. Res. 2021, 31, 100395. [Google Scholar] [CrossRef]
- Xiong, Z.; Shang, L.; Yang, J.; Chen, L.; Guo, J.; Liu, Q.; Danso, S.A.; Li, G. Terahertz sensor with resonance enhancement based on square split-ring resonators. IEEE Access 2021, 9, 59211–59221. [Google Scholar] [CrossRef]
- Han, J.; Lakhtakia, A. Semiconductor split-ring resonators for thermally tunable terahertz metamaterials. J. Mod. Optic. 2009, 56, 554–557. [Google Scholar] [CrossRef]
- Wu, P.J.; Tsai, W.C.; Yang, C.S. Electrically tunable graphene-based multi-band terahertz metamaterial filters. Opt. Express 2022, 31, 469–478. [Google Scholar] [CrossRef]
- Feng, H.; Zhang, Z.; Zhang, J.; Fang, D.; Wang, J.; Liu, C.; Wu, T.; Wang, G.; Wang, L.; Ran, L.; et al. Tunable dual-broadband terahertz absorber with vanadium dioxide metamaterial. Nanomaterials 2022, 12, 1731. [Google Scholar] [CrossRef] [PubMed]
- Lai, W.H.; Li, B.; Fu, S.H.; Lin, Y.S. Tunable MEMS-based terahertz metamaterial for pressure sensing application. Micromachines 2023, 14, 169. [Google Scholar] [CrossRef] [PubMed]
- Özer, Z.; Akdoğan, V.; Wang, L.; Karaaslan, M. Graphene-based tunable metamaterial absorber for terahertz sensing applications. Plasmonics 2024, 19, 1–11. [Google Scholar] [CrossRef]
- Zhang, K.; Dong, S.; Wu, X.; Yu, K.; Liu, Y. Graphene-based tunable broadband metamaterial absorber for terahertz waves. Opt. Laser Technol. 2025, 180, 111490. [Google Scholar] [CrossRef]
- Lan, F.; Wang, L.; Zeng, H.; Liang, S.; Song, T.; Liu, W.; Mazumder, P.; Yang, Z.; Zhang, Y.; Mittleman, D.M. Real-time programmable metasurface for terahertz multifunctional wave front engineering. Light Sci. Appl. 2023, 12, 191. [Google Scholar] [CrossRef] [PubMed]
- Zheng, D.; Lin, Y.S. Tunable Dual-Split-Disk Resonator with Electromagnetically Induced Transparency Characteristic. Adv. Mater. Technol-Us. 2020, 5, 2000584. [Google Scholar] [CrossRef]
- Manjappa, M.; Srivastava, Y.K.; Cong, L.; Al-Naib, I.; Singh, R. Active photoswitching of sharp Fano resonances in THz metadevices. Adv. Mater. 2016, 29, 1603355. [Google Scholar] [CrossRef]
- Li, Q.; Gupta, M.; Zhang, X.; Wang, S.; Chen, T.; Singh, R.; Han, J.; Zhang, W. Active control of asymmetric Fano resonances with graphene–silicon-integrated terahertz metamaterials. Adv. Mater. Technol. 2020, 5, 1900840. [Google Scholar] [CrossRef]
- Lin, Y.S.; Liao, S.; Liu, X.; Tong, Y.; Xu, Z.; Xu, R.; Yao, D.; Yu, Y. Tunable terahertz metamaterial by using three-dimensional double split-ring resonators. Opt. Laser Technol. 2019, 112, 215–221. [Google Scholar] [CrossRef]
- Saleh, G.; Ateeq, I.S.; Al-Naib, I. Glucose level sensing using single asymmetric split ring resonator. Sensors 2021, 21, 2945. [Google Scholar] [CrossRef] [PubMed]
- Urbonis, D.; Ragulis, P.; Šlekas, G.; Kamarauskas, A.; Seliuta, D.; Kancleris, Ž. Symmetric and asymmetric Fano resonances in a broken axial symmetry metasurface of split ring resonators. J. Appl. Phys. 2023, 134, 133102. [Google Scholar] [CrossRef]
- Wang, X.; Liang, T.; Li, G.; Shen, J.; Zhang, B. Fano resonance and sensing application based on terahertz asymmetric split-ring metasurfaces. J. Alloy. Compd. 2024, 976, 173130. [Google Scholar] [CrossRef]
- Shih, K.; Pitchappa, P.; Manjappa, M.; Ho, C.P.; Singh, R.; Lee, C. Microfluidic metamaterial sensor: Selective trapping and remote sensing of microparticles. J. Appl. Phys. 2017, 121, 023102. [Google Scholar] [CrossRef]
- Zhu, M.; Lee, C. A design of terahertz broadband filters and its effect in eliminating asymmetric characteristics in device structures. J. Light. Technol. 2015, 33, 3280–3285. [Google Scholar] [CrossRef]
- Soukoulis, C.M.; Kafesaki, M.; Economou, E.N. Negative-Index Materials: New Frontiers in Optics. Adv. Mater. 2006, 18, 1941–1952. [Google Scholar] [CrossRef]
- Lin, Y.S.; Qian, Y.; Ma, F.; Liu, Z.; Kropelnicki, P.; Lee, C. Development of stress-induced curved actuators for a tunable THz filter based on double split-ring resonators. Appl. Phys. Lett. 2013, 102, 111908. [Google Scholar] [CrossRef]
- Motamedi, M.E.; Southwell, W.H.; Gunning, W.J. Antireflection surfaces in silicon using binary optics technology. Appl. Opt. 1992, 31, 4371–4376. [Google Scholar] [CrossRef] [PubMed]
- Linden, S.; Enkrich, C.; Wegener, M.; Zhou, J.; Koschny, T.; Soukoulis, C.M. Magnetic response of metamaterials at 100 terahertz. Science 2004, 306, 1351–1353. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Lin, Y.S. Design of tunable terahertz metamaterial sensor with single-and dual-resonance characteristic. Nanomaterials 2021, 11, 2212. [Google Scholar] [CrossRef]
- Pitchappa, P.; Kumar, A.; Singh, R.; Wang, N. Electromechanically tunable frequency-agile metamaterial bandpass filters for terahertz waves. Adv. Opt. Mater. 2022, 10, 2101544. [Google Scholar] [CrossRef]
- Guo, R.; Duan, S.; He, Z.; Liang, X.; Niu, Z.; He, M.; Jiang, Y.; Wu, J.; Ji, L.; Jin, B.; et al. Josephson Plasmon Resonance in Tl2Ba2CaCu2O8 High-Temperature Superconductor Tunable Terahertz Metamaterials. Adv. Funct. Mater. 2021, 31, 2106891. [Google Scholar] [CrossRef]
- Li, C.; Li, W.; Duan, S.; Wu, J.; Chen, B.; Yang, S.; Su, R.; Jiang, C.; Zhang, C.; Jin, B.; et al. Electrically tunable electromagnetically induced transparency in superconducting terahertz metamaterials. Appl. Phys. Lett. 2021, 119, 052602. [Google Scholar] [CrossRef]
- Jiang, M.; Hu, F.; Zhang, L.; Quan, B.; Xu, W.; Du, H.; Xie, D.; Chen, Y. Electrically triggered VO 2 reconfigurable metasurface for amplitude and phase modulation of terahertz wave. J. Light. Technol. 2021, 39, 3488–3494. [Google Scholar] [CrossRef]
- Sun, H.; Hu, Y.; Tang, Y.; You, J.; Zhou, J.; Liu, H.; Zheng, X. Ultrafast polarization-dependent all-optical switching of germanium-based metaphotonic devices. Photon. Res. 2020, 8, 263–270. [Google Scholar] [CrossRef]
- He, W.; Tong, M.; Xu, Z.; Hu, Y.; Cheng, X.A.; Jiang, T. Ultrafast all-optical terahertz modulation based on an inverse-designed metasurface. Photon. Res. 2021, 9, 1099–1108. [Google Scholar] [CrossRef]
- Chen, X.; Tian, Z.; Lu, Y.; Xu, Y.; Zhang, X.; Ouyang, C.; Gu, J.; Han, J.; Zhang, W. Electrically tunable perfect terahertz absorber based on a graphene salisbury screen hybrid metasurface. Adv. Opt. Mater. 2020, 8, 1900660. [Google Scholar] [CrossRef]
- Xu, R.; Xu, X.; Yang, B.R.; Gui, X.; Qin, Z.; Lin, Y.S. Actively logical modulation of MEMS-based terahertz metamaterial. Photon. Res. 2021, 9, 1409–1415. [Google Scholar] [CrossRef]
- Chen, T.; Zhang, D.; Huang, F.; Li, Z.; Hu, F. Design of a terahertz metamaterial sensor based on split ring resonator nested square ring resonator. Mater. Res. Express 2020, 7, 095802. [Google Scholar] [CrossRef]
- Li, F.; He, K.; Tang, T.; Mao, Y.; Wang, R.; Li, C.; Shen, J. The terahertz metamaterials for sensitive biosensors in the detection of ethanol solutions. Opt. Commun. 2020, 475, 126287. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Sarkar, R.; Sharma, N.K.; Bhowmik, B.K.; Ahmad, A.; Kumar, G. Multiband transparency effect induced by toroidal excitation in a strongly coupled planar terahertz metamaterial. Sci. Rep. 2021, 11, 19186. [Google Scholar] [CrossRef] [PubMed]

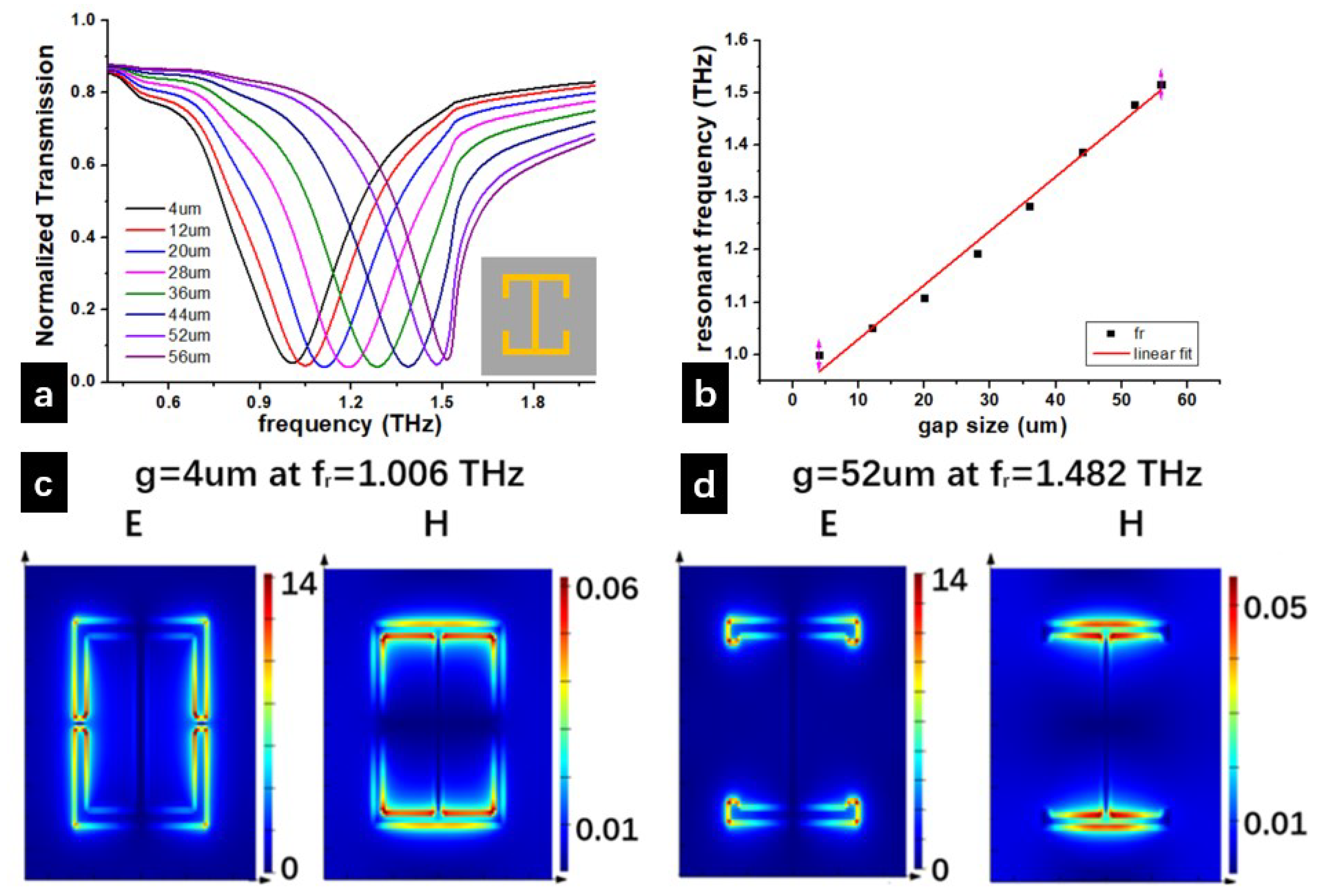
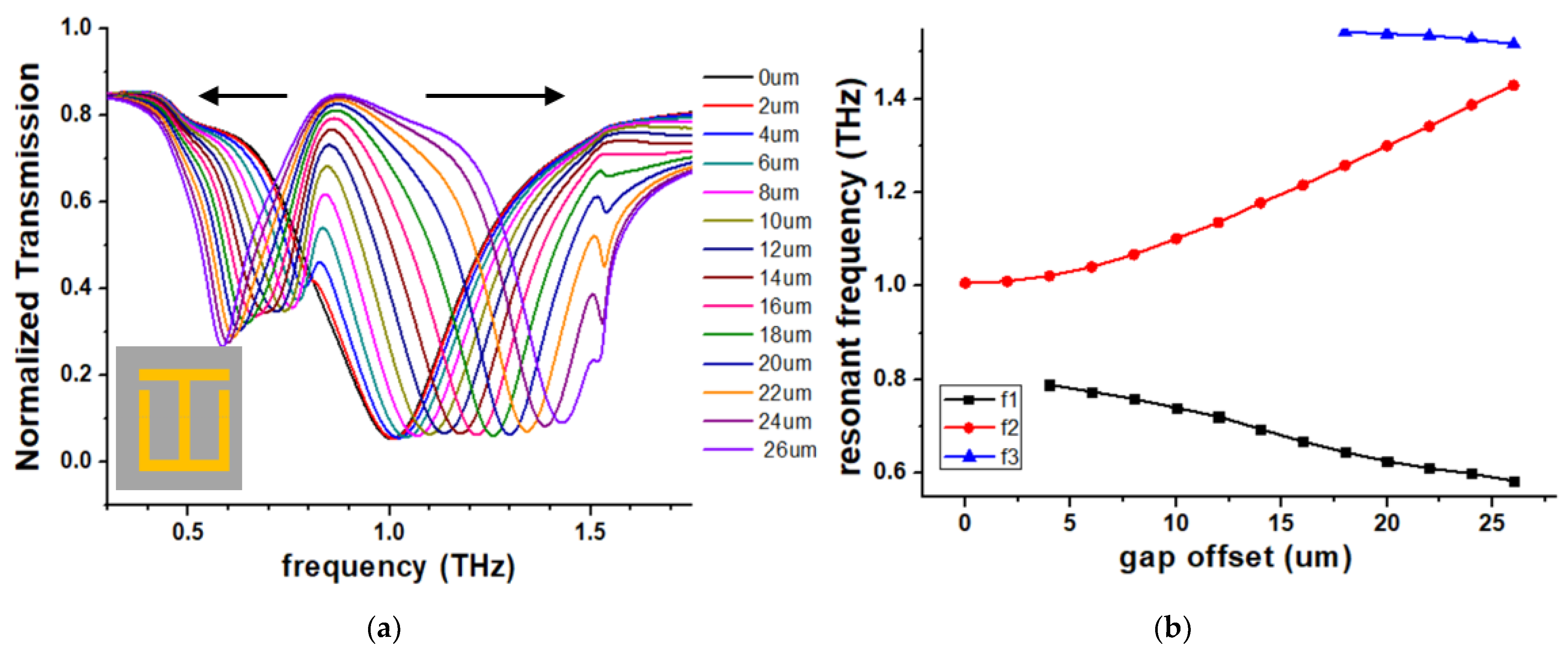
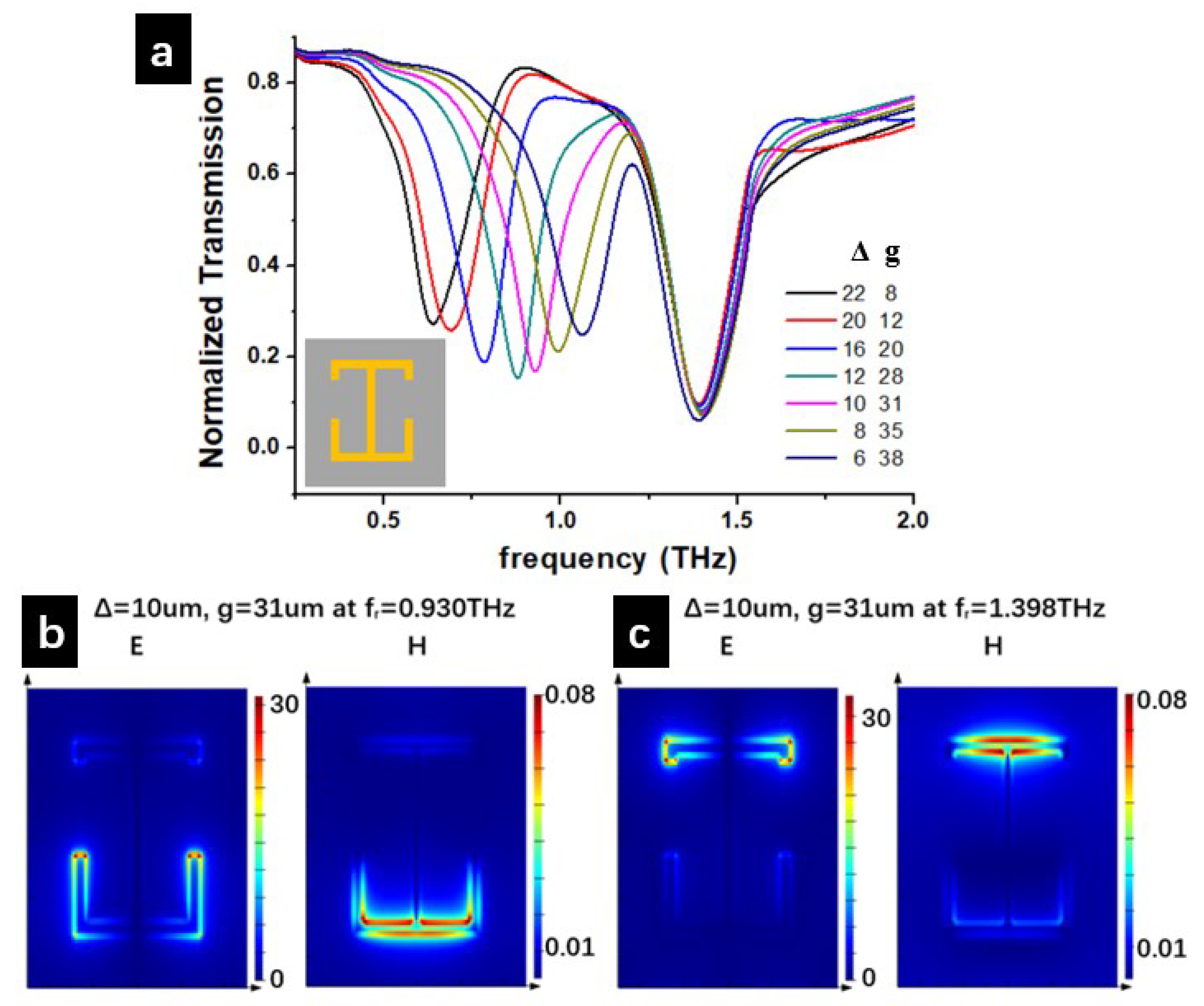

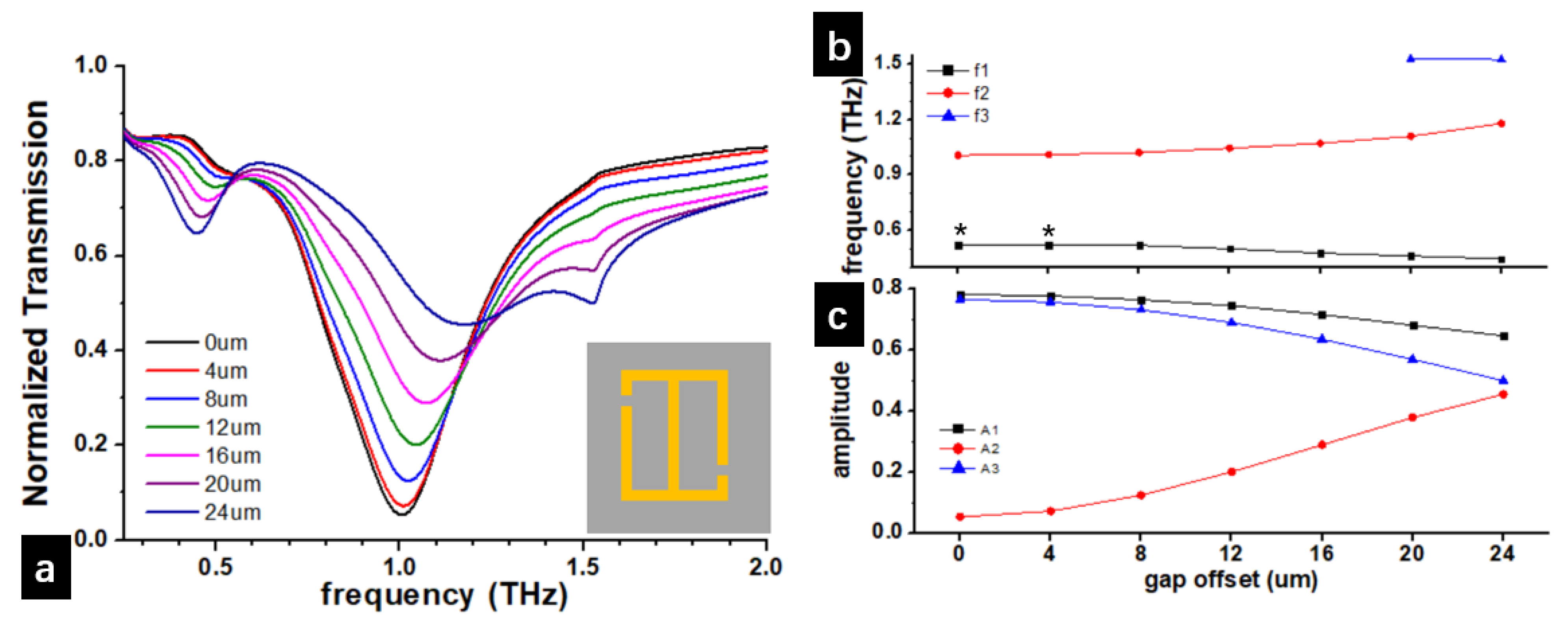


| Δ | g | a | b |
|---|---|---|---|
| 22 | 8 | 50 | 6 |
| 20 | 12 | 46 | 6 |
| 16 | 20 | 38 | 6 |
| 12 | 28 | 30 | 6 |
| 10 | 31 | 26.5 | 6.5 |
| 8 | 35 | 22.5 | 6.5 |
| 6 | 38 | 19 | 7 |
| Resonant Dips | EIT Peak | |||
|---|---|---|---|---|
| h (μm) | 1 | 2 | 2 | 1 |
| b (μm) | 30 | 30 | 42 | 30 |
| frequency (THz) | 0.820 | 0.831 | 0.852 | 0.835 |
| Q | 22.8 | 15.98 | 44.8 | 10.3 |
| FOM | 22.0 | 15.38 | 40.1 | 9.4 |
| References | [17] | [20] | [31] | [32] | [33] | Our Work |
|---|---|---|---|---|---|---|
| Frequency shift (GHz) | 200 | 400 | 318 | 108 | 109 | 510 1 |
| FSR tuning range (GHz) | 240 | 550 | - | - | - | 613 2 |
| References | [17] | [34] | [35] | [36] | [37] | [38] | Our Work |
|---|---|---|---|---|---|---|---|
| MD (%) | 75.6 | 71 | 45 | 50 | 25 | 23 | 93.4 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, M.; Wang, X.; Zhang, L.; Geng, J.; Chen, J. The Role of Asymmetry on the Resonances of Conjoined Split-Ring Resonators. Symmetry 2025, 17, 332. https://doi.org/10.3390/sym17030332
Zhu M, Wang X, Zhang L, Geng J, Chen J. The Role of Asymmetry on the Resonances of Conjoined Split-Ring Resonators. Symmetry. 2025; 17(3):332. https://doi.org/10.3390/sym17030332
Chicago/Turabian StyleZhu, Mei, Xitao Wang, Lian Zhang, Jiguo Geng, and Jun Chen. 2025. "The Role of Asymmetry on the Resonances of Conjoined Split-Ring Resonators" Symmetry 17, no. 3: 332. https://doi.org/10.3390/sym17030332
APA StyleZhu, M., Wang, X., Zhang, L., Geng, J., & Chen, J. (2025). The Role of Asymmetry on the Resonances of Conjoined Split-Ring Resonators. Symmetry, 17(3), 332. https://doi.org/10.3390/sym17030332





