Blood Damage Analysis within the FDA Benchmark Nozzle Geometry at Laminar Conditions: Prediction Sensitivities to Software and Non-Newtonian Viscosity Models
Abstract
1. Introduction
1.1. Flow Symmetry within the FDA Benchmark Nozzle in the Laminar Regime (Re < 2000)
1.2. Newtonian vs. Non-Newtonian Viscosity Model Effects in the Laminar Regime (Re < 2000)
1.3. Non-Newtonian Simulations of the FDA Nozzle
1.4. Prediction Sensitivity to Software
2. Materials and Methods
3. Results
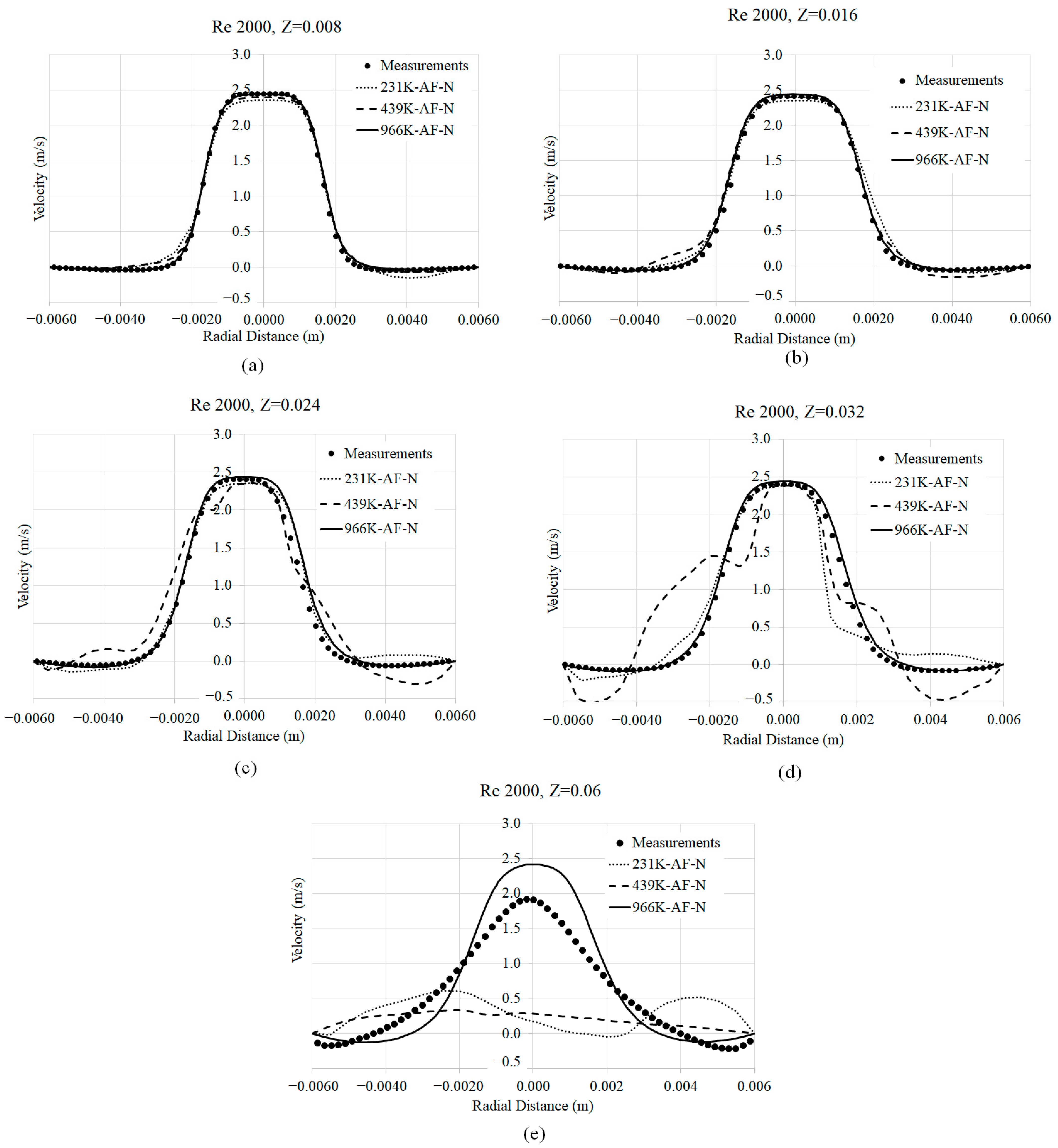
3.1. Mesh Convergence
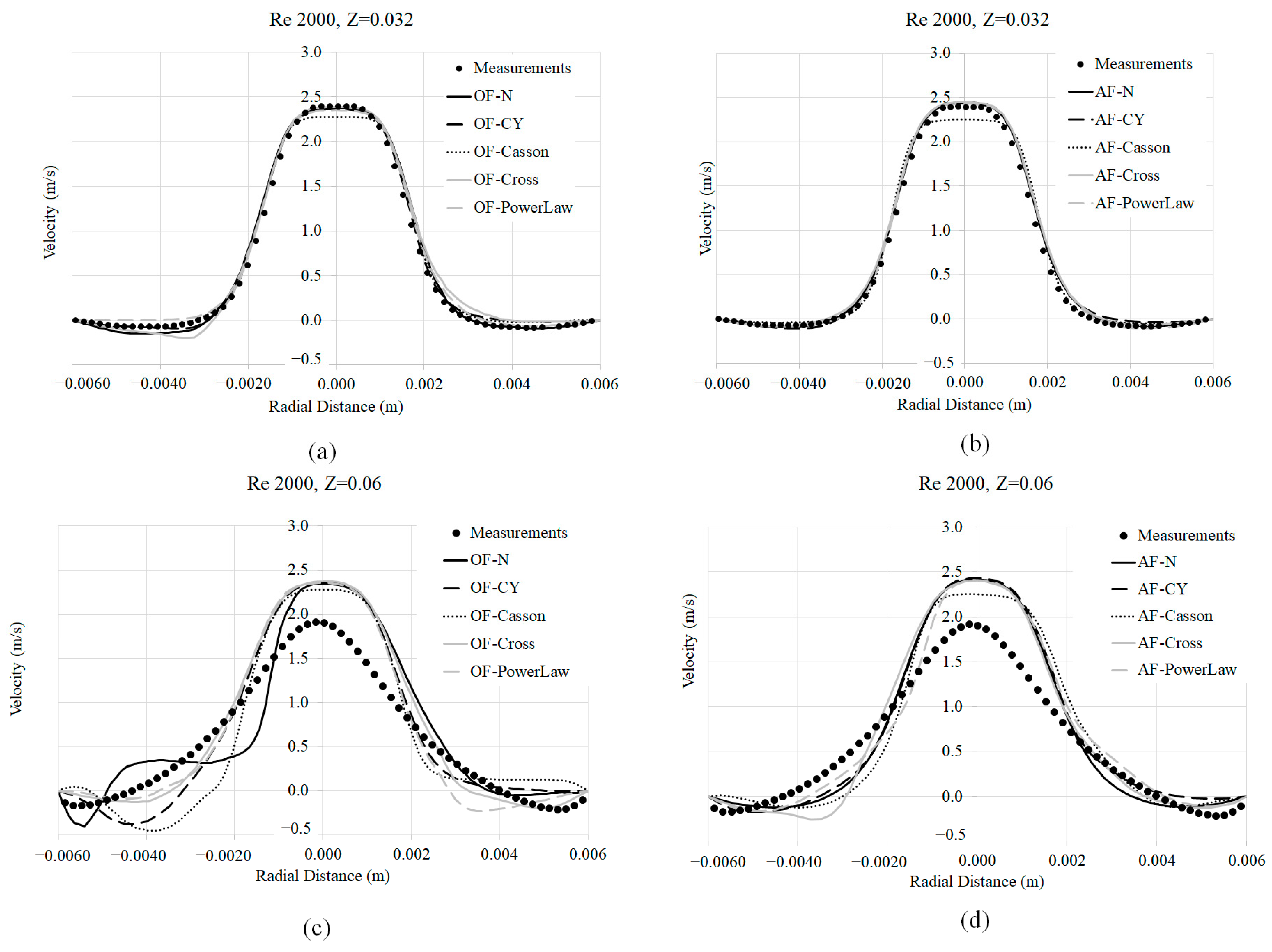
3.2. Axial Velocity Prediction Sensitivity to Viscosity Models
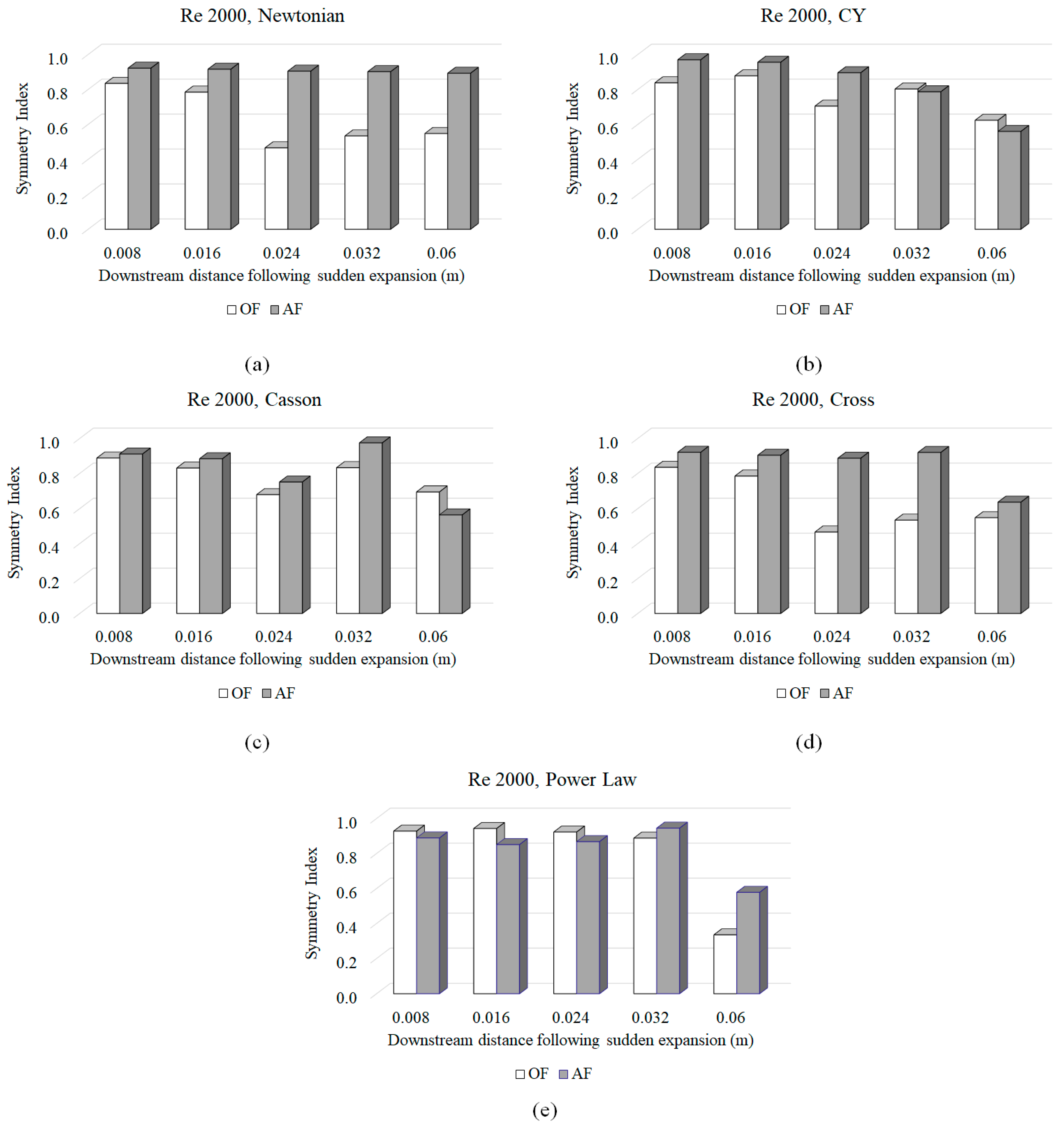
3.3. Symmetry Index (SI)
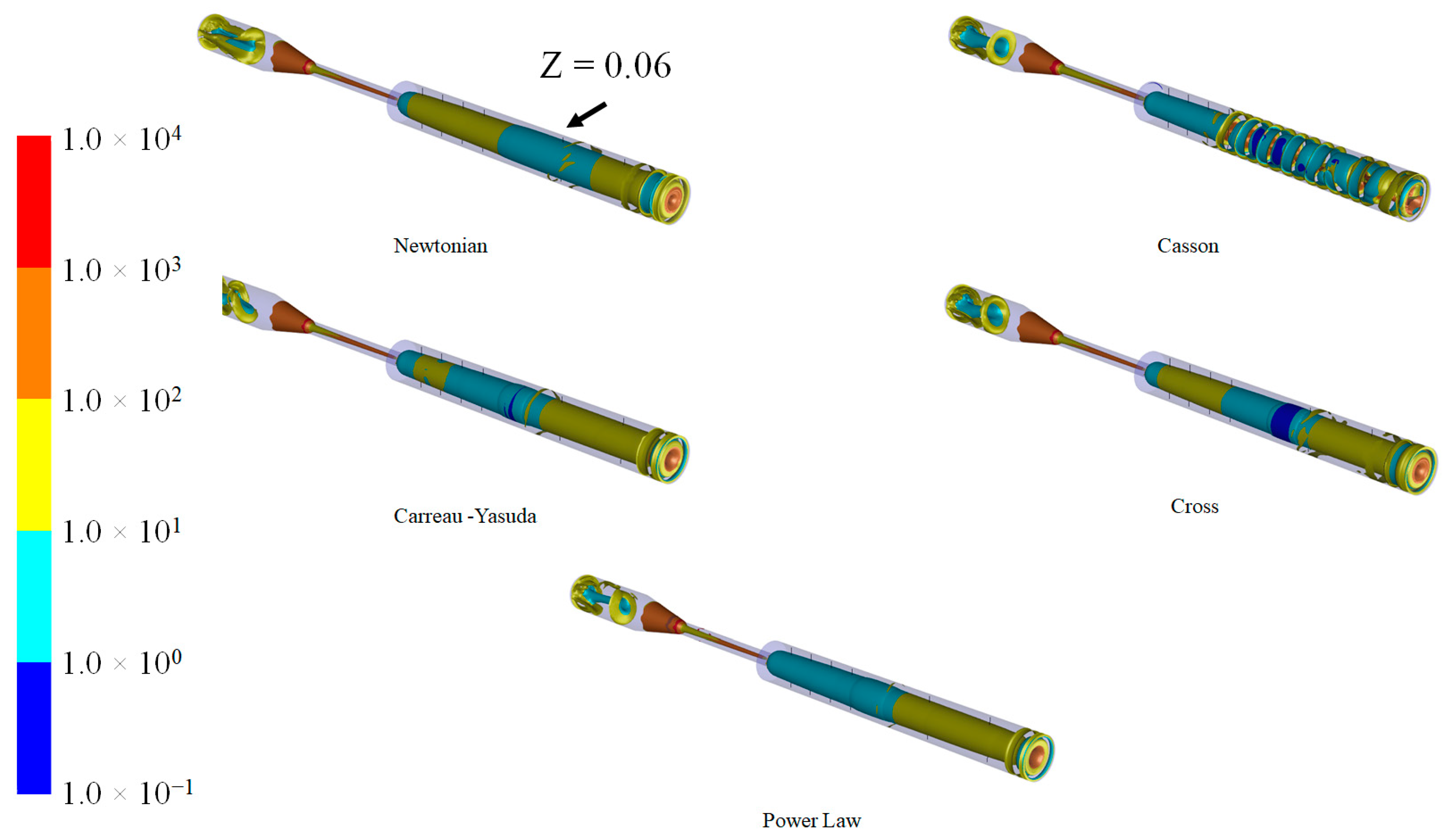
3.4. Q-Criterion and Vortex Shape Identification
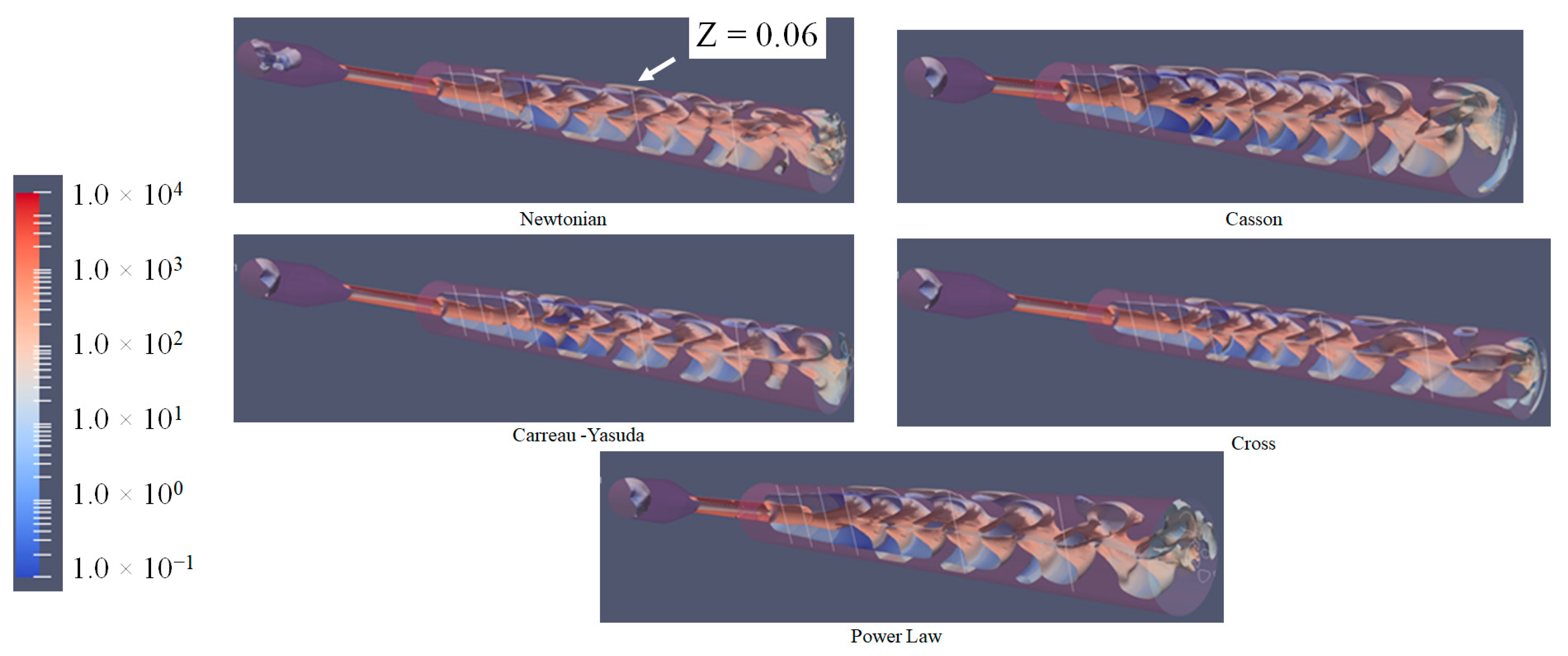
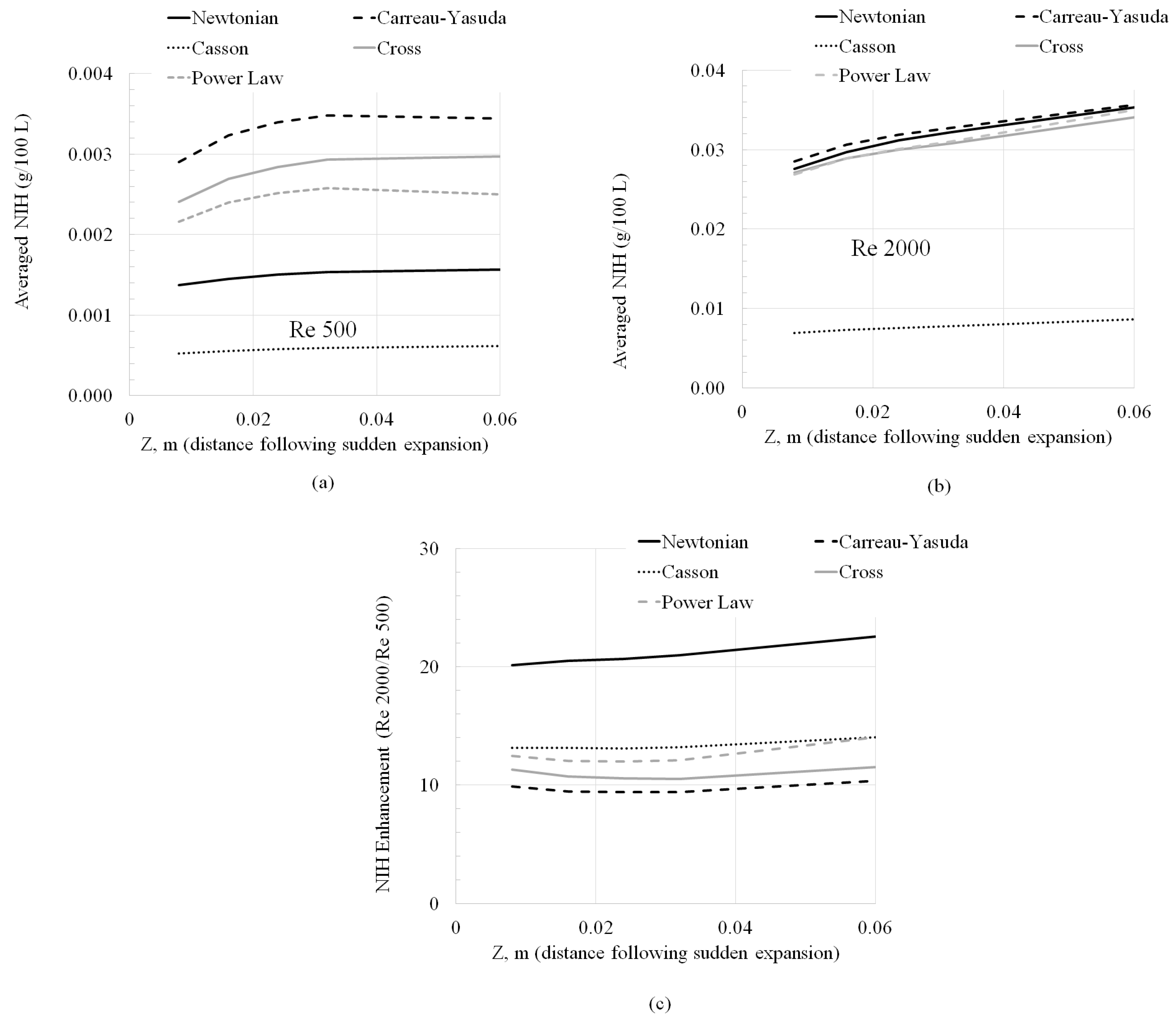
3.5. Normalized Index of Hemolysis (NIH) Evaluation
3.6. Mathematical Component
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bergersen, A.W.; Mortensen, M.; Valen-Sendstad, K. The FDA nozzle benchmark: “In theory there is no difference between theory and practice but in practice there is”. Int. J. Numer. Methods Biomed. Eng. 2019, 35, 3150. [Google Scholar] [CrossRef] [PubMed]
- Bhushan, S.; Walters, D.K.; Burgreen, G.W. Laminar, turbulent, and transitional simulations in benchmark cases with cardiovascular device features. Cardiovasc. Eng. Technol. 2013, 4, 408–426. [Google Scholar] [CrossRef]
- Chabannes, V.; Prud’Homme, C.; Szopos, M.; Tarabay, R. High-order finite element simulations for fluid dynamics validated by experimental data from the FDA benchmark nozzle model. arXiv 2017, arXiv:1701.02179. [Google Scholar]
- Delorme, Y.T.; Anupindi, K.; Frankel, S.H. Large eddy simulation of FDA’s idealized medical device. Cardiovasc. Eng. Technol. 2013, 4, 392–407. [Google Scholar] [CrossRef]
- Fehn, N.; Wall, W.A.; Kronbichler, M. Modern discontinuous Galerkin methods for the simulation of transitional and turbulent flows in biomedical engineering: A comprehensive LES study of the FDA benchmark nozzle model. Int. J. Numer. Methods Biomed. Eng. 2019, 35, 3228. [Google Scholar] [CrossRef]
- Huang, F.; Noël, R.; Berg, P.; Hosseini, S.A. Simulation of the FDA nozzle benchmark: A lattice Boltzmann study. Comput. Methods Programs Biomed. 2022, 221, 106863. [Google Scholar] [CrossRef]
- Jain, K. Efficacy of the FDA nozzle benchmark and the lattice Boltzmann method for the analysis of biomedical flows in the transitional regime. Med. Biol. Eng. Comput. 2020, 58, 1817–1830. [Google Scholar] [CrossRef]
- Manchester, E.L.; Xu, X.Y. The effect of turbulence on transitional flow in the FDA’s benchmark nozzle model using large-eddy simulation. Int. J. Numer. Methods Biomed. Eng. 2020, 36, 3389. [Google Scholar] [CrossRef]
- Nicoud, F.; Chnafa, C.; Siguenza, J.; Zmijanovic, V.; Mendez, S. Large-eddy simulation of turbulence in cardiovascular flows. In Biomedical Technology: Modeling, Experiments, and Simulation; Springer: Berlin/Heidelberg, Germany, 2018; pp. 147–167. [Google Scholar]
- Passerini, T.; Quaini, A.; Veneziani, A.; Wood, N.B. Validation of an open-source framework for the simulation of blood flow in rigid and deformable vessels. Int. J. Numer. Methods Biomed. Eng. 2013, 29, 1192–1213. [Google Scholar] [CrossRef]
- Pewowaruk, R.; Li, Y.; Rowinski, D.; Roldán-Alzate, A. Solution adaptive refinement of cut-cell Cartesian meshes can improve FDA nozzle computational fluid dynamics efficiency. Int. J. Numer. Methods Biomed. Eng. 2021, 37, 3432. [Google Scholar] [CrossRef]
- Sánchez Abad, N.; Vinuesa, R.; Schlatter, P.; Andersson, M.; Karlsson, M. Simulation strategies for the Food and Drug Administration nozzle using Nek5000. AIP Adv. 2020, 10, 2. [Google Scholar] [CrossRef]
- Stewart, S.F.; Paterson, E.G.; Burgreen, G.W.; Hariharan, P.; Reddy, V.; Day, S.W. Assessment of CFD performance in simulations of an idealized medical device: Results of FDA’s first computational interlaboratory study. Cardiovasc. Eng. Technol. 2013, 4, 374–391. [Google Scholar] [CrossRef]
- Stewart, S.F.; Paterson, E.G.; Burgreen, G.W.; Hariharan, P.; Reddy, V.; Day, S.W. Results of FDA’s first interlaboratory computational study of a nozzle with a sudden contraction and conical diffuser. Cardiovasc. Eng. Technol. 2012, 3, 139–160. [Google Scholar] [CrossRef]
- Stiehm, M.; Wüstenhagen, C.; Siewert, S.; Grabow, N.; Schmitz, K.P. Numerical simulation of pulsatile flow through a coronary nozzle model based on FDA’s benchmark geometry. Curr. Dir. Biomed. Eng. 2017, 3, 775–778. [Google Scholar] [CrossRef]
- Taylor, C.A.; Steinman, D.A.; Simon, H.A.; Valen-Sendstad, K.; Bergersen, A.W.; Loth, F. Predicting the quality of the FDA’s nozzle benchmark. J. Biomech. 2016, 49, 2245–2251. [Google Scholar]
- Zmijanovic, V.; Löhner, R.; Indrakanti, S.S. Large eddy simulation of transitional and turbulent flow in the FDA nozzle model. J. Biomech. Eng. 2017, 139, 041003. [Google Scholar]
- Qiao, Y.; Luo, K. Computational prediction of thrombosis in FDA’s benchmark nozzle. Front. Physiol. 2022, 13, 867613. [Google Scholar] [CrossRef]
- Tobin, N.; Manning, K.B. Large-eddy simulations of flow in the FDA benchmark nozzle geometry to predict hemolysis. Cardiovasc. Eng. Technol. 2020, 11, 254–267. [Google Scholar] [CrossRef]
- Sobey, I.J.; Drazin, P.G. Bifurcations of two-dimensional channel flows. J. Fluid Mech. 1986, 171, 263–287. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Fluent, Version 2023 R2; ANSYS Inc.: Canonsburg, PA, USA, 2023; Available online: https://www.ansys.com/products/fluids/ansys-fluent (accessed on 1 January 2024).
- The OpenFOAM Foundation. OpenFOAM, Version 9; The OpenFOAM Foundation: London, UK, 2021; Available online: www.openfoam.org (accessed on 1 January 2024).
- Biswas, D.; Casey, D.M.; Crowder, D.C.; Steinman, D.A.; Yun, Y.H.; Loth, F. Characterization of transition to turbulence for blood in a straight pipe under steady flow conditions. I. Biomech Eng. 2016, 138, 071001. [Google Scholar] [CrossRef]
- Cebral, J.R.; Castro, M.A.; Appanaboyina, S.; Putman, C.M.; Millan, D.; Frangi, A.F. Efficient pipeline for image-based patient-specific analysis of cerebral aneurysm hemodynamics: Technique and sensitivity. IEEE Trans. Med. Imaging 2005, 24, 457–467. [Google Scholar] [CrossRef]
- Costa, R.P.; Simplice Talla Nwotchouang, B.; Yao, J.; Biswas, D.; Casey, D.; McKenzie, R.; Steinman, D.A.; Loth, F. Transition to turbulence downstream of a stenosis for whole blood and a Newtonian analog under steady flow conditions. J. Biomech. Eng. 2022, 144, 031008. [Google Scholar] [CrossRef] [PubMed]
- Haley, A.L.; Valen-Sendstad, K.; Steinman, D.A. On delayed transition to turbulence in an eccentric stenosis model for clean vs. noisy high-fidelity CFD. J. Biomech. 2021, 125, 110588. [Google Scholar] [CrossRef] [PubMed]
- Johnston, B.M.; Johnston, P.R.; Corney, S.; Kilpatrick, D. Non-Newtonian blood flow in human right coronary arteries: Steady-state simulations. J. Biomech. 2004, 37, 709–720. [Google Scholar] [CrossRef] [PubMed]
- Khan, M.O.; Valen-Sendstad, K.; Steinman, D.A. Direct numerical simulation of laminar-turbulent transition in a non-axisymmetric stenosis model for Newtonian vs. shear-thinning non-Newtonian rheologies. Flow Turbul. Combust. 2019, 102, 43–72. [Google Scholar] [CrossRef]
- Lee, S.W.; Steinman, D.A. On the relative importance of rheology for image-based CFD models of the carotid bifurcation. J. Biomech. Eng. 2007, 129, 273–278. [Google Scholar] [CrossRef]
- Razavi, A.; Shirani, E.; Sadeghi, M.R. Numerical simulation of blood pulsatile flow in a stenosed carotid artery using different rheological models. J. Biomech. 2011, 44, 2021–2030. [Google Scholar] [CrossRef]
- Good, B.C. The effects of non-Newtonian blood modeling and pulsatility on hemodynamics in the Food and Drug Administration’s benchmark nozzle model. Biorheology 2023, 59, 1–18. [Google Scholar] [CrossRef]
- Hussein, B.K.; Al-Azawy, M.G.; Al-Waaly, A.A.; Hamza, Z.A. Evaluation of turbulence and non-Newtonian blood rheology models through FDA nozzle. In Proceedings of the 2021 International Conference on Advance of Sustainable Engineering and its Application (ICASEA), Wasit, Iraq, 27–28 October 2021; IEEE: Toulouse, France, 2021; pp. 1–5. [Google Scholar]
- Trias, M.; Arbona, A.; Massó, J.; Miñano, B.; Bona, C. FDA’s nozzle numerical simulation challenge: Non-Newtonian fluid effects and blood damage. PLoS ONE 2014, 9, e92638. [Google Scholar] [CrossRef]
- Zakaria, M.S.; Zainudin, S.H.; Abdullah, H.; Yuan, C.S.; Abd Latif, M.J.; Osman, K. CFD simulation of non-Newtonian effect on hemodynamics characteristics of blood flow through benchmark nozzle. J. Adv. Res. Fluid Mech. Therm. Sci. 2019, 64, 117–125. [Google Scholar]
- Berg, P.; Janiga, G.; Thévenin, D. CFD Challenge: Solutions using the commercial solver Fluent and the open-source solver OpenFOAM. In Proceedings of the Summer Bioengineering Conference, Fajardo, PR, USA, 20–23 June 2012; American Society of Mechanical Engineers: New York, NY, USA, 2012; Volume 44809, pp. 115–116. [Google Scholar]
- Lysenko, D.A.; Ertesvåg, I.S.; Rian, K.E. Modeling of turbulent separated flows using OpenFOAM. Comput. Fluids 2013, 80, 408–422. [Google Scholar] [CrossRef]
- Robertson, E.; Choudhury, V.; Bhushan, S.; Walters, D.K. Validation of OpenFOAM numerical methods and turbulence models for incompressible bluff body flows. Comput. Fluids 2015, 123, 122–145. [Google Scholar] [CrossRef]
- Jones, D.A. CFD RANS Simulations on a Generic Conventional Scale Model Submarine: Comparison between Fluent and OpenFOAM; Technical Note; Defence Science and Technology Organisation, Maritime Division: Fishermans Bend, VIC, Australia, 2015; Available online: https://apps.dtic.mil/sti/pdfs/ADA622263.pdf (accessed on 26 March 2024).
- Greifzu, F.; Kratzsch, C.; Forgber, T.; Lindner, F.; Schwarze, R. Assessment of particle-tracking models for dispersed particle-laden flows implemented in OpenFOAM and ANSYS Fluent. Eng. Appl. Comput. Fluid Mech. 2015, 10, 30–43. [Google Scholar] [CrossRef]
- Dresar, P.; Duhovnik, J. A hybrid RANS-LES computational fluid dynamics simulation of an FDA medical device benchmark. Mechanics 2019, 25, 291–298. [Google Scholar] [CrossRef]
- Jarrell, J.A.; Sytsma, B.J.; Wilson, L.H.; Pan, F.L.; Lau, K.H.; Kirby, G.T.; Lievano, A.A.; Pawell, R.S. Numerical optimization of microfluidic vortex shedding for genome editing T cells with Cas9. Sci. Rep. 2021, 11, 11818. [Google Scholar] [CrossRef] [PubMed]
- Menon, P.G.; Teslovich, N.; Chen, C.Y.; Undar, A.; Pekkan, K. Characterization of neonatal aortic cannula jet flow regimes for improved cardiopulmonary bypass. J. Biomech. 2013, 46, 362–372. [Google Scholar] [CrossRef]
- Mancini, V.; Bergersen, A.W.; Valen-Sendstad, K.; Segers, P. Computed poststenotic flow instabilities correlate phenotypically with vibrations measured using laser Doppler vibrometry: Perspectives for a promising in vivo device for early detection of moderate and severe carotid stenosis. J. Biomech. Eng. 2020, 142, 091007. [Google Scholar] [CrossRef]
- Ozturk Papavassiliou, D.V.; O’Rear, E.A. An approach for assessing turbulent flow damage to blood in medical devices. J. Biomech. Eng. 2017, 139, 011008. [Google Scholar]
- Sonntag, S.J.; Lipinski, E.; Neidlin, M.; Hugenroth, K.; Benkowski, R.; Motomura, T.; Kaufmann, T.A.S. Virtual fitting and hemodynamic simulation of the EVAHEART 2 left ventricular assist device and double-cuff tipless inflow cannula. ASAIO J. 2019, 65, 698–706. [Google Scholar] [CrossRef]
- Kopernik, M. Modeling of blood thrombosis at microscopic and mesoscopic scales. Comput. Assist. Methods Eng. Sci. 2019, 25, 21–45. [Google Scholar]








| Authors | Publication Year | Summary |
|---|---|---|
| Bergersen et al. [1] | 2013 | In this study, at a Reynolds number (Re) of 500, simulation predictions remained stable despite numerical noise introduced at the inlet to simulate experimental uncertainties. However, at a Reynolds number of 3500, such inlet perturbations influenced the location of vortex breakdown |
| Bhushan et al. [2] | 2013 | In this study, the use of turbulence models at Reynolds number (Re) 500 led to excessive diffusion, which resulted in lower centerline velocities compared to experimental data. However, transition-sensitive unsteady RANS turbulence models can deliver accurate predictions across the entire Reynolds number range if the turbulence intensity levels at the inlet are correctly specified. |
| Chabannes et al. [3] | 2017 | In this study, at Re 500, solution accuracies were assessed using mesh refinement studies. At Re 2000, the jet breakdown occurred downstream of the experimentally measured location. |
| Delorme et al. [4] | 2013 | LES and Immersed Boundary Method were employed. Predictions agreed with the experimental results for the mean velocities at Re = 500, 2000 and 3500. While Re = 500 stayed symmetric throughout, coherent vortices, as identified by the λ2 criterion, were observed close to the 0.032 m mark. |
| Fehn et al. [5] | 2019 | Flow remains laminar throughout at Re 500. At Re 2000 transition can be induced if the simulation parameters can be tweaked. Simulations were carried out using a high-order discontinuous Galerkin discretization technique. |
| Huang et al. [6] | 2022 | No jet breakdown after sudden expansion at Re 500 for unsteady simulations carried out with the Lattice Boltzmann Method. Symmetric solutions and zero centerline shear stresses persist downstream of the sudden expansion. |
| Jain [7] | 2020 | High resolution (HR) and Extreme spatial resolution (XR) of 40 µm; 20 µm is necessary for accurate results in Lattice Boltzmann Method-based simulations. Jet breakdown for the Re 2000 case occurs between 0.06 m and 0.08 m downstream of the sudden expansion. At low resolutions, the jet breaks down between 0.024 m and 0.032 m. |
| Manchester et al. [8] | 2020 | Using LES at Re 2000 using the OpenFOAM solver, laminar quantities were predicted throughout the model. In the jet breakdown region (0.06 m downstream of sudden expansion), where maximum Reynolds stresses occur, Reynolds shear stresses showed excellent agreement with measurements. |
| Nicoud et al. [9] | 2018 | LES simulations were carried out with a fourth-order accurate solver. Inlet perturbations did not induce turbulence in the laminar case (Re 500), whereas improved axial velocity predictions were seen at Re 2000. |
| Passerini et al. [10] | 2013 | Transient finite element simulations were carried out. At Re 500, the flow is axially symmetric throughout. At Re 2000, simulations predicted a jet breakdown location further downstream of the experimental and the differences were attributed to the fidelity of the numerical integration in the solver. |
| Pewowaruk et al. [11] | 2021 | Adaptive Mesh Refinement (AMR) study was carried out in a cut-cell representation of the device. Velocity contours show that symmetric profiles persist downstream of the sudden expansion at Re 500. |
| Sanchez, A. et al. [12] | 2020 | Used a high-order Spectral Element Method to carry out the simulations. At Re 500, no flow transition was observed. At Re 2000, flow did not transition to turbulence. However, by increasing the length of the domain downstream of the expansion and by incorporating some fluctuations at the inlet, a transition was eventually observed. |
| Stewart et al. [13] | 2012 | Re 500–6500 simulated. Turbulence models (k-epsilon, k-omega, and shear stress transport models) were employed in all Re > 500 simulations. Turbulence models were unable to accurately predict velocities and shear stresses in the recirculation zones downstream of the sudden expansion. |
| Stewart et al. [14] | 2012 | Summarized the results from several CFD groups. Results showed modest agreement in global and local flow behaviors. All CFD data sets contained wide degrees of velocity variation in comparison to the experiment and with each other at Re 2000 and between 0.032 m and 0.06 m downstream of the sudden expansion section. |
| Stiehm et al. [15] | 2017 | Pulsatile (time-dependent) boundary condition simulations were performed on a scaled-down (1/3rd scale) of the original FDA geometry at Re 500. Replacing the time-dependent boundary condition with an appropriate time-average boundary condition to employ in steady-state simulations was deemed to be sufficient if the interest is on obtaining time-averaged values. |
| Taylor et al. [16] | 2016 | Large variations in the LDV and PIV measurements of axial velocity at 0.06 m downstream of the sudden expansion due to instabilities for the Re 2000 case. The highest turbulence intensity and shear stresses were seen during jet breakdown between 0.032 m and 0.06 m downstream of the sudden expansion. |
| Zmijanovic et al. [17] | 2017 | LES simulations showed that Re 500 remains laminar throughout with no breakdown in symmetry. Results indicate a considerable impact of numerical aspects on the prediction of the location of the transition to turbulence for Re 3500. |
| Qiao et al. [18] | 2022 | Thrombus formation investigated at different Re. An inverse relationship between thrombosis and hemolysis was observed with thrombosis formation accelerated at lower Re. |
| Tobin et al. [19] | 2020 | The objective of this study is to utilize existing models to account for turbulence’s chaotic nature and the interplay of fluctuating velocities and eddies within the flow. To validate these adaptations, the study compared model predictions with experimental data gathered from previous research. |
| Authors | Publication Year | Summary |
|---|---|---|
| Biswas et al. [23] | 2016 | Two mathematically defined methods, based on the velocity profile shape change and turbulent kinetic energy (TKE), were used to detect the transition to turbulence experimentally. Overall, the critical Re for turbulence onset was delayed by 20% (2316 vs. 2871) for blood compared to the Newtonian fluid. |
| Cebral et al. [24] | 2005 | Non-Newtonian viscosity models, like the Casson model, produce smaller velocity gradients due to higher local viscosity in low-flow regions. Despite this, the overall flow patterns and mean wall shear stress remain largely unchanged. The most significant factor influencing intra-aneurysmal flow patterns is the geometry of the aneurysm and its connections. |
| Costa et al. [25] | 2022 | Normalized turbulent kinetic energy (TKE) was employed to establish the critical Reynolds number for each fluid. The study found a 19% delay in TT for whole blood compared to the Newtonian fluid. |
| Haley et al. [26] | 2021 | Delays in TT between “noisy” non-Newtonian and Newtonian results were studied in an eccentric geometry. Introducing noise did not eliminate this distinction. However, as Re increased, the flow profiles under both rheologies started to converge towards similar outcomes. |
| Johnston et al. [27] | 2004 | While the Newtonian model of blood viscosity provides a good approximation in regions of mid-range to high shear, it is advisable to use the Generalized Power Law (non-Newtonian) model. This model converges to the Newtonian model in high shear ranges but offers a better approximation of wall shear stress at low shear rates. |
| Khan et al. [28] | 2019 | Blood exhibits a delayed TT compared to Newtonian fluids. However, defining a critical Reynolds number for non-Newtonian fluids accurately remains challenging in complex geometries. This difficulty is compounded by factors beyond shear thinning, including the potential influence of viscoelastic properties. |
| Lee, S.W. et al. [29] | 2007 | Prediction sensitivities to non-Newtonian models were in different geometries and flow rates. Using the Carreau–Yasuda model, it was observed that shear-thinning has a minimal effect on carotid bifurcation hemodynamics. In contrast, high shear rates, inlet boundary conditions, and geometric factors exerted more significant influences. |
| Razavi, A. et al. [30] | 2011 | In this study, flow conditions in a stenosed artery for various viscosity models, including Newtonian and six non-Newtonian models (power law, generalized power law, Carreau, Carreau–Yasuda, modified Casson, and Walburn–Schneck) were compared. The findings indicate that the power law model exhibits greater variation in wall shear stress and velocity values compared to other models. In contrast, the modified Casson and generalized power law models show a closer approximation to Newtonian behavior. |
| Authors | Publication Year | Summary |
|---|---|---|
| Good [31] | 2023 | Model differences were most evident at Re = 500. The non-Newtonian model predicted blunter upstream velocity profiles, and a greater pressure drop, with minimal differences observed at higher Reynolds numbers. |
| Hussein et al. [32] | 2021 | At Re 500, TKE and turbulent viscosity ratio were lower with Carreau in comparison to the Newtonian model. Pressure profiles were also different, attributed to larger recirculation zones at lower Re. Both differences diminished between the viscosity models at higher Re. |
| Trias, et al. [33] | 2014 | Axial velocity in the downstream region is inversely proportional to viscosity, with the highest velocity in the Newtonian model, followed by Carreau–Yasuda and then Casson. Velocity differences are within 10%. Viscosity models do not significantly affect the pressure drop. Non-Newtonian effects, while not greatly impacting velocity or pressure drop, are important for assessing blood damage, as they predict higher wall shear stresses and greater blood damage. |
| Zakaria et al. [34] | 2019 | The Newtonian model exhibits higher centerline velocities due to its constant viscosity assumption, which should be higher at lower shear rates. It also shows a higher pressure drop, likely due to more vortices. Using the lambda-2 criterion, chaotic and unstable vortices were observed with the Newtonian model. Overall, the Newtonian model produced more severe hemodynamic properties. |
| Authors | Publication Year | Summary |
|---|---|---|
| Berg et al. [35] | 2012 | Centerline pressure predictions between Ansys Fluent and OpenFOAM show a high level of agreement, with differences being less than 1.03%. This indicates that both software packages provide reliable and consistent results for predicting centerline pressures in CFD simulations. |
| Lysenko et al. [36] | 2013 | In simulations of turbulent flow (Reynolds number = 3900) over a circular cylinder, AF and OF showed differences in predicting small, secondary vortices at the rear of the cylinder. These discrepancies underscore the importance of turbulence modeling and numerical methods in accurately capturing flow features and phenomena in CFD simulations. |
| Robertson et al. [37] | 2015 | In flow simulations around a sphere at Reynolds number 104, OF exhibits secondary circulations near the sphere’s separation point. Variations in predictions between OF and other solvers primarily stem from differences in turbulence source term modeling rather than numerical methods. For simulations involving a backward-facing step, OF shows greater sensitivity to grid resolution. However, both OF and other solvers agree well on integral and mean flow variables, demonstrating reliable performance in predicting overall flow characteristics despite localized differences. |
| Jones et al. [38] | 2015 | Accurate CFD simulations are crucial for submarine support. The authors compared Fluent to commonly utilized OpenFOAM. Simulations on a submarine model showed up to 15% differences in drag coefficients between the two codes. Some discrepancies were resolved, but significant differences remain. This report details efforts to address these differences and suggests further work to ensure confidence in both codes. |
| Greifzu et al. [39] | 2015 | This study investigates two benchmark problems for turbulent dispersed particle-laden flow using CFD programs OpenFOAM and Ansys Fluent. The Lagrangian–Eulerian point-particle models for RANS simulations are compared in steady state and transient modes against experimental data. The results from both programs align well with experimental data. Dispersed phase results show Ansys Fluent slightly under-predicting and OpenFOAM slightly overestimating particle dispersion. |
| Authors | Date of Publication | Summary |
|---|---|---|
| Dresar et al. [40] | 2019 | RANS, SAS, DES and LES turbulence models were compared. While RANS and SAS did not show vortices, their predictions were insensitive to boundary mesh size. |
| Jarrell et al. [41] | 2021 | Intracellular delivery of functional macromolecules, such as DNA and RNA, across the cell microfluidic chips CFD simulations highlighted key hydrodynamic features that enhance vortex shedding and promote intracellular delivery of functional macromolecules such as DNA and RNA across cell microfluidic chips. |
| Menon et al. [42] | 2013 | The study visualized vortical structures in simulated aortic cannulation configurations using the Q-criterion to identify coherent vortex patterns in the jet wake. It compared two configurations: one arbitrarily oriented, leading to complex vortex structures and high hemolysis near the transverse aortic arch, and another based on surgeon sketches, showing simpler vortex patterns and lower hemolysis near the descending aorta. The findings highlight the importance of optimizing cannulation parameters for better hemodynamic outcomes during surgery. |
| Mancini et al. [43] | 2020 | LDA, along with Q-criterion, was employed to assess stenosis in vivo. Receiver operating characteristic analyses of power spectra revealed that the most relevant frequency bands for the detection of moderate (56–76%) and severe (86–96%) stenoses were 80–200 Hz and 0–40 Hz, respectively. |
| Ozturk et al. [44] | 2017 | The study found that smaller eddies, specifically those with diameters up to about 10 μm (Kolmogorov scale eddies), are significantly associated with hemolysis. There is a clear correlation between hemolysis and the total surface area occupied by these smaller eddies. However, no such relationship was observed for larger eddies. This suggests that predicting the distribution of Kolmogorov scale eddies could help evaluate the susceptibility of medical device designs to hemolysis. Adjusting the design to increase the size of Kolmogorov scales may be beneficial in reducing the risk of hemolysis associated with these smaller turbulent structures. |
| Sonntag et al. [45] | 2019 | Intraventricular vortices were identified and visualized using the Q criterion in various studies. These vortices are linked to ventricular health. |
| Type | Viscosity Model | Constants |
|---|---|---|
| Carreau–Yasuda (CY) | ||
| Casson | ||
| Cross | ||
| Power Law | n = 0.7755 λ = 0.01467 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krishnamoorthy, G.; Gholizadeh, N. Blood Damage Analysis within the FDA Benchmark Nozzle Geometry at Laminar Conditions: Prediction Sensitivities to Software and Non-Newtonian Viscosity Models. Symmetry 2024, 16, 1165. https://doi.org/10.3390/sym16091165
Krishnamoorthy G, Gholizadeh N. Blood Damage Analysis within the FDA Benchmark Nozzle Geometry at Laminar Conditions: Prediction Sensitivities to Software and Non-Newtonian Viscosity Models. Symmetry. 2024; 16(9):1165. https://doi.org/10.3390/sym16091165
Chicago/Turabian StyleKrishnamoorthy, Gautham, and Nasim Gholizadeh. 2024. "Blood Damage Analysis within the FDA Benchmark Nozzle Geometry at Laminar Conditions: Prediction Sensitivities to Software and Non-Newtonian Viscosity Models" Symmetry 16, no. 9: 1165. https://doi.org/10.3390/sym16091165
APA StyleKrishnamoorthy, G., & Gholizadeh, N. (2024). Blood Damage Analysis within the FDA Benchmark Nozzle Geometry at Laminar Conditions: Prediction Sensitivities to Software and Non-Newtonian Viscosity Models. Symmetry, 16(9), 1165. https://doi.org/10.3390/sym16091165






