Correspondence Analysis for Assessing Departures from Perfect Symmetry Using the Cressie–Read Family of Divergence Statistics
Abstract
1. Introduction
2. Test of Perfect Symmetry and the Cressie–Read Family of Divergence Statistics
2.1. Notation
2.2. Testing Departures from a Hypothesised
2.3. Testing Departures from Complete Independence
2.4. Testing for Departures from Perfect Symmetry
2.5. A Second-Order Approximation
3. Correspondence Analysis and Perfect Symmetry
3.1. The Divergence Residual
3.2. Is the Matrix of Divergence Residuals Skew-Symmetric?
3.3. Singular Value Decomposition and the Divergence Residual
3.4. The Principal Inertia Values
3.5. Principal Coordinates
3.6. On the Total Inertia and the Origin
4. Example 1: Artificial Data
4.1. The Data
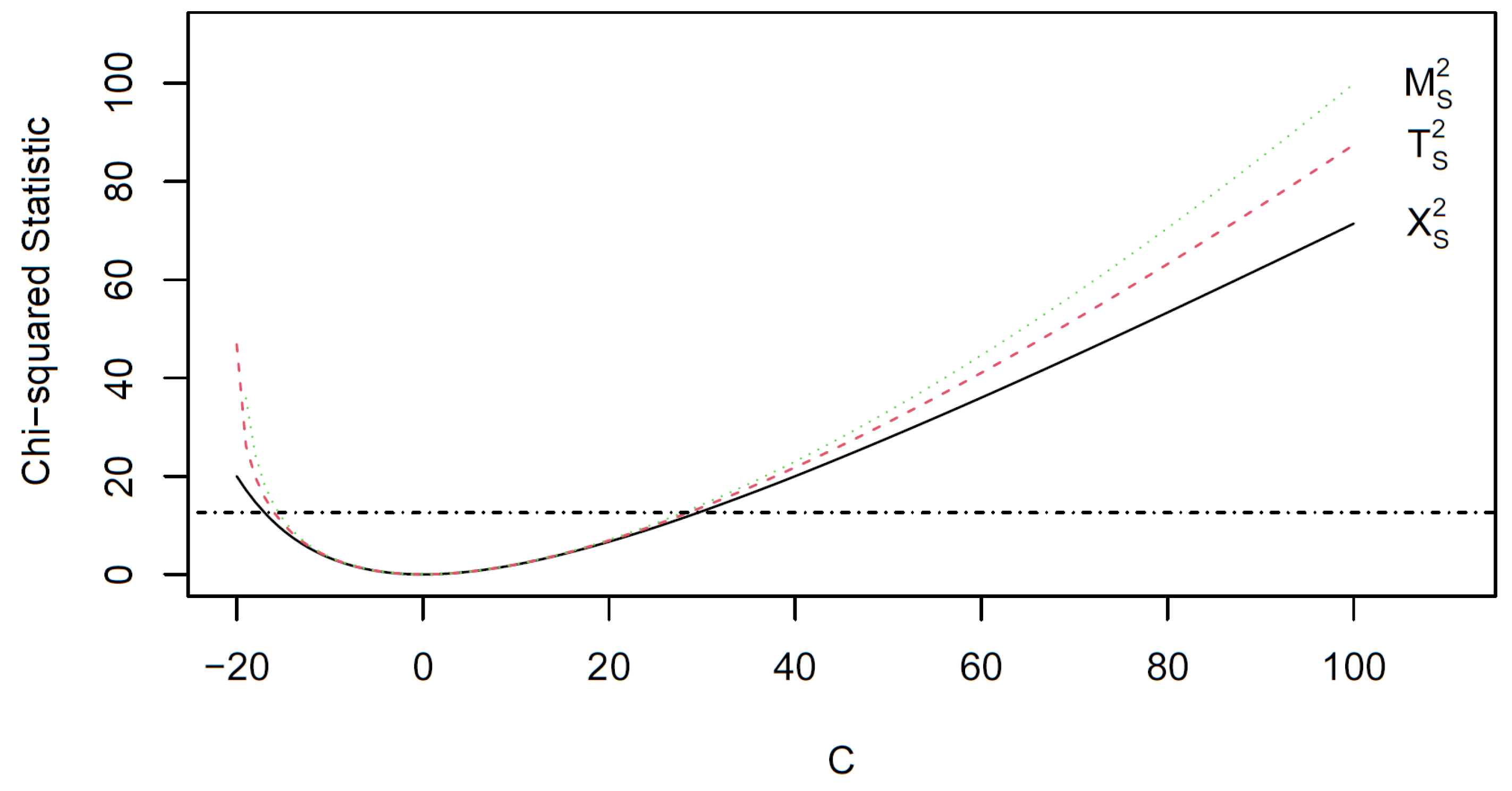
4.2. The Family of Divergence Statistics
4.3. On the Departure from Perfect Symmetry
4.4. Features of Correspondence Analysis and Symmetry
4.4.1. The Matrix of Divergence Residuals
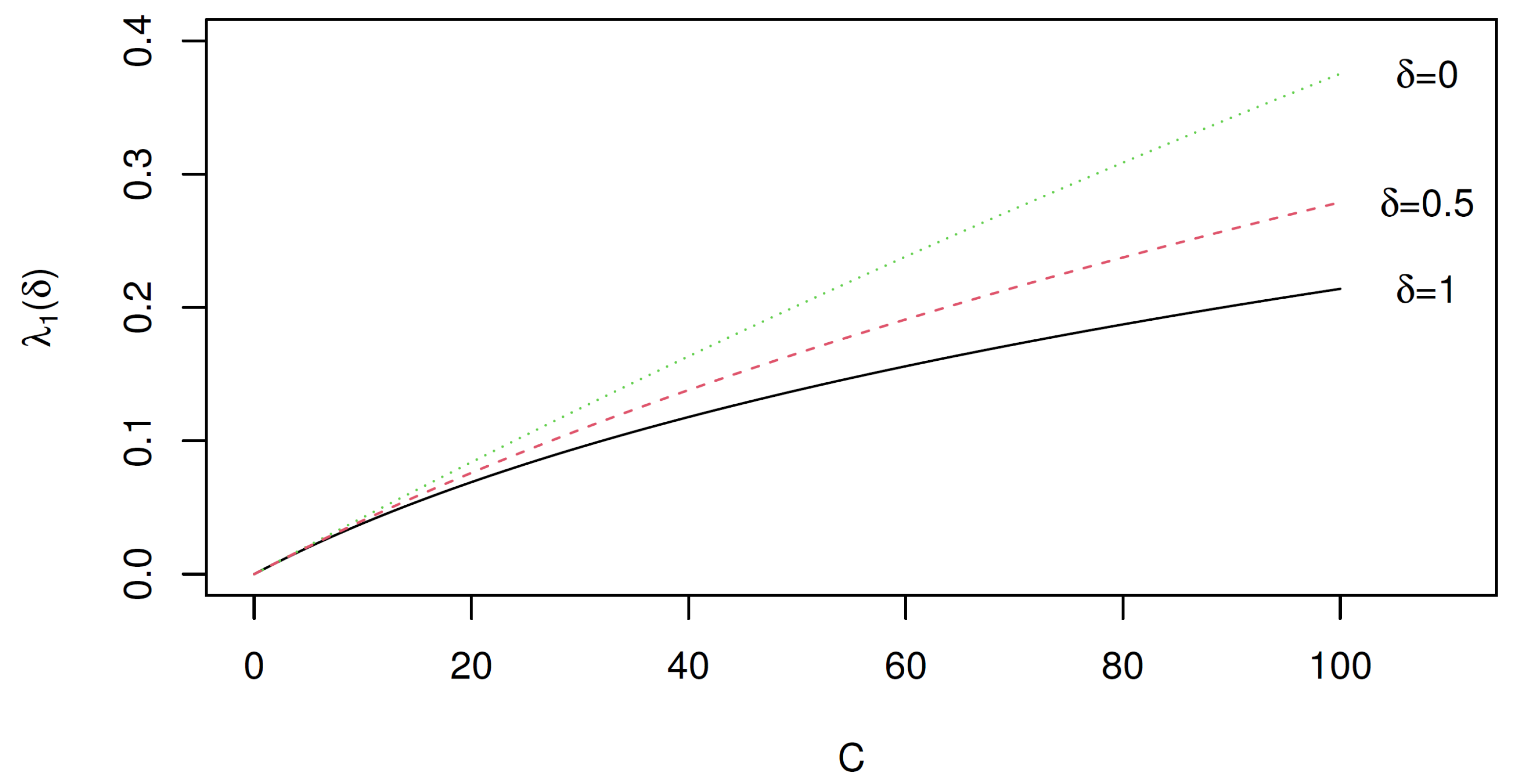
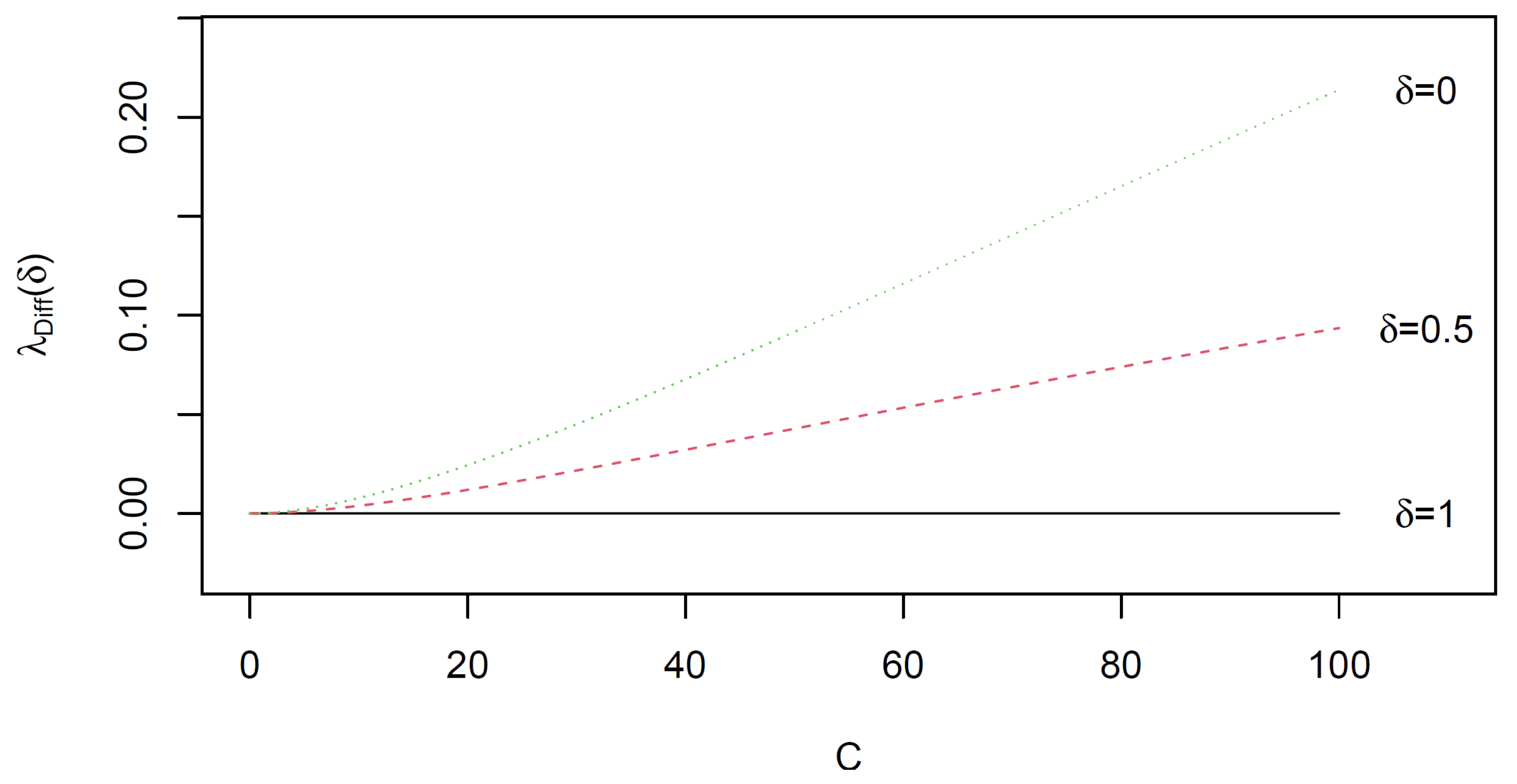
4.4.2. The Singular Values
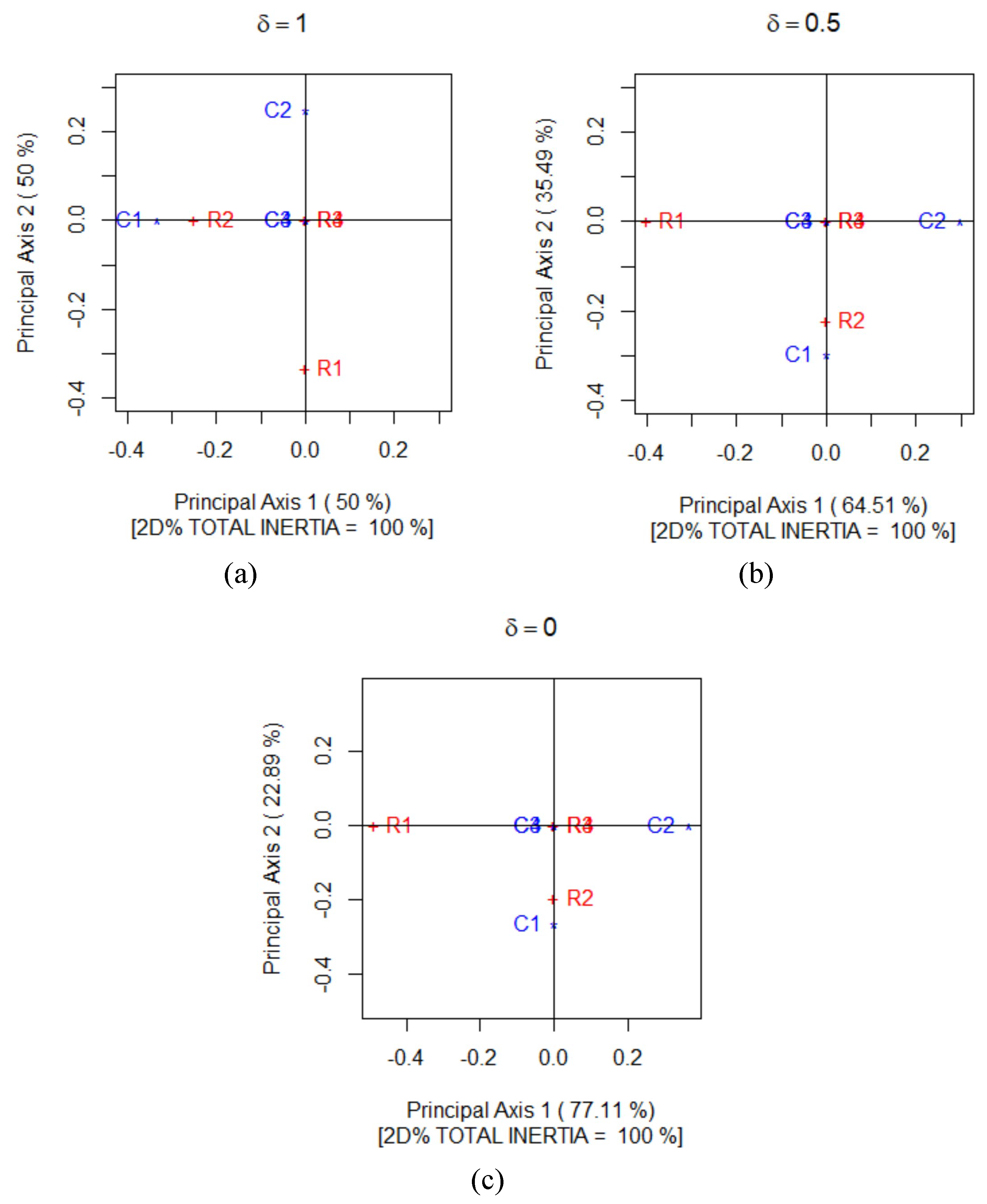
4.4.3. Principal Coordinates
4.5. The Correspondence Plots
5. Example 2: Pre- and Post-Courtship Behaviour of Bitterlings
5.1. The Data
5.2. Test of the Departure from Perfect Symmetry
5.3. On the Divergence Residuals
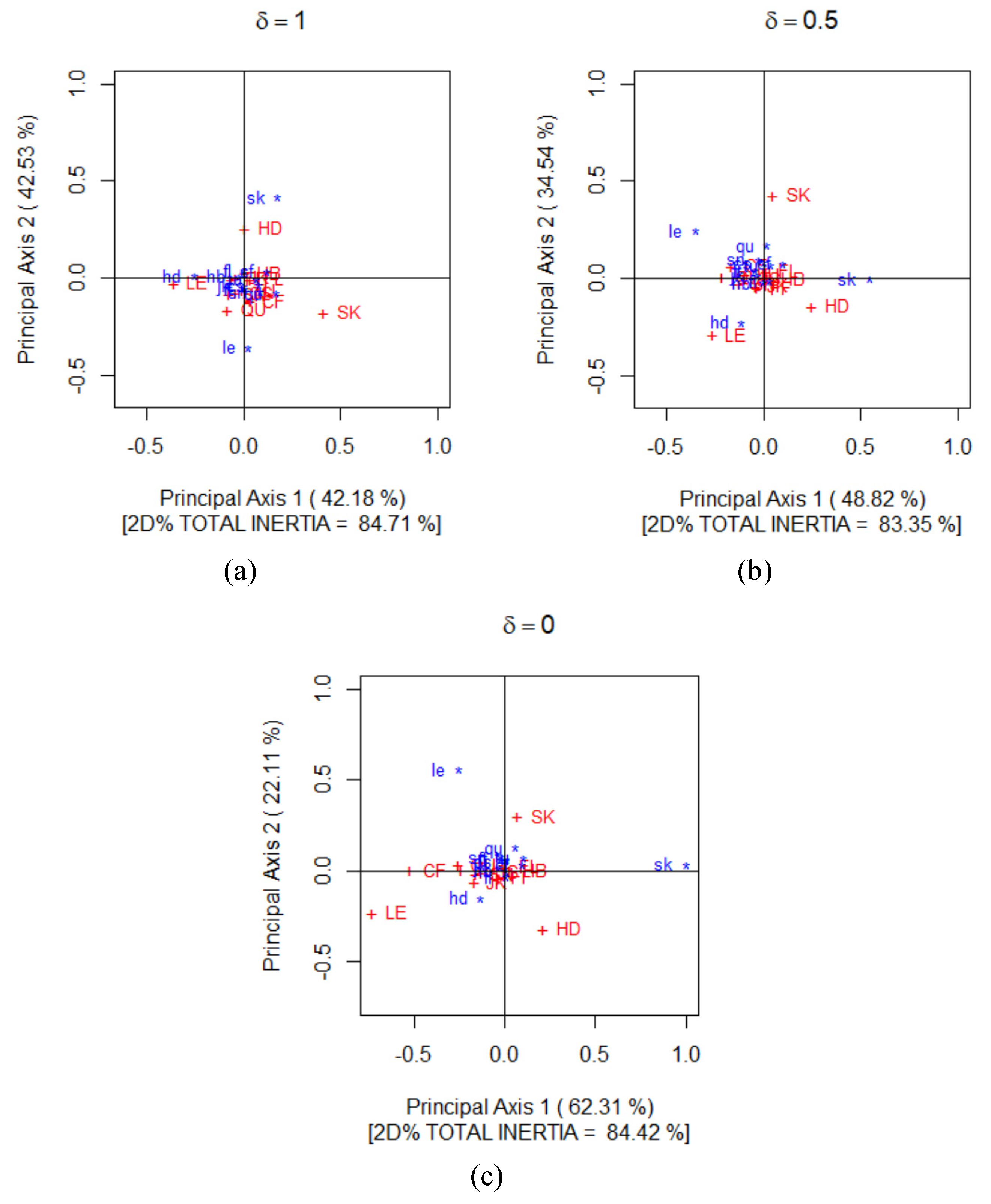
5.4. Visualising the Departures from Perfect Symmetry
6. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Beh, E.J.; Lombardo, R. Correspondence Analysis: Theory, Practice and New Strategies; Wiley: Chichester, UK, 2014. [Google Scholar]
- Greenacre, M.J. Theory and Applications of Correspondence Analysis; Academic Press: London, UK, 1984. [Google Scholar]
- Lebart, L.; Morineau, A.; Warwick, K.M. Multivariate Descriptive Statistical Analysis: Correspondence Analysis and Related Techniques for Large Matrices; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Beh, E.J.; Lombardo, R. Visualising departures from symmetry and Bowker’s X2 statistic. Symmetry 2022, 14, 1103. [Google Scholar] [CrossRef]
- Greenacre, M. Correspondence analysis of square asymmetric matrices. J. R. Stat. Soc. Ser. C Appl. Stat. 2000, 49, 297–310. [Google Scholar] [CrossRef]
- Bove, G. Asymmetric multidimensional scaling and correspondence analysis for square tables. Stat. Appl. 1992, 4, 587–598. [Google Scholar]
- Constantine, A.G.; Gower, J.C. Graphical representation of asymmetry. Appl. Stat. 1978, 27, 297–304. [Google Scholar] [CrossRef]
- Gower, J.C. The analysis of asymmetry and orthogonality. In Recent Developments in Statistics; Barra, J.R., Brodeau, F., Romer, G., van Cutsem, B., Eds.; North-Holland: Amsterdam, The Netherlands, 1977; pp. 109–123. [Google Scholar]
- Bowker, A.H. A test for symmetry in contingency tables. J. Am. Stat. Assoc. 1948, 43, 572–598. [Google Scholar] [CrossRef] [PubMed]
- Cressie, N.A.C.; Read, T.R.C. Multinomial goodness-of-fit tests. J. R. Stat. Soc. Ser. B Methodol. 1984, 46, 440–464. [Google Scholar] [CrossRef]
- Beh, E.J.; Lombardo, R. Correspondence analysis and the Cressie-Read family of divergence statistics. Int. Stat. Rev. 2024, 92, 17–42. [Google Scholar] [CrossRef]
- Greenacre, M. Power transformations in correspondence analysis. Comput. Stat. Data Anal. 2009, 53, 3107–3116. [Google Scholar] [CrossRef]
- Greenacre, M. Log-ratio analysis is a limiting case of correspondence analysis. Math. Geosci. 2010, 42, 129–134. [Google Scholar] [CrossRef]
- Cuadras, C.M.; Cuadras, D. A parametric approach to correspondence analysis. Linear Algebra Its Appl. 2006, 417, 64–74. [Google Scholar] [CrossRef]
- Cuadras, C.M.; Cuadras, D.; Greenacre, M.J. A comparison of different methods of representing categorical data. Commun. Stat.-Simul. Comput. 2006, 35, 447–459. [Google Scholar] [CrossRef]
- Neyman, J. Contributions to the theory of the χ2 test. In Proceedings of the Berkeley Symposium on Mathematical Statistics and Probability; Neyman, J., Ed.; Statistical Laboratory of the University of California: Berkeley, CA, USA, 1949; pp. 239–273. [Google Scholar]
- Freeman, M.F.; Tukey, J.W. Transformations related to the angular and square root. Ann. Math. Stat. 1950, 21, 607–611. [Google Scholar] [CrossRef]
- Wiepkema, P.R. An ethological analysis of the reproductive behaviour of the bitterling (Rhodeus amarus Bloch). Arch. NÉerlandaises Zool. 1961, 14, 103–199. [Google Scholar] [CrossRef]
- Beh, E.J.; Lombardo, R.; Alberti, G. Correspondence analysis and the Freeman-Tukey statistic: A study of archaeological data. Comput. Stat. Data Anal. 2018, 128, 73–86. [Google Scholar] [CrossRef]
- Agresti, A. Categorical Data Analysis, 3rd ed.; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Anderson, E.B. The Statistical Analysis of Categorical Data; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Tomizawa, S.; Seo, T.; Yamamoto, H. Power-divergence-type measure of departure from symmetry for square contingency tables that have nominal categories. J. Appl. Stat. 1998, 25, 387–398. [Google Scholar] [CrossRef]
- Ando, S.; Hoshi, H.; Ishii, A.; Tomizawa, S. A generalized two-dimensional index to measure the degree of deviation from double symmetry in square contingency tables. Symmetry 2021, 13, 2067. [Google Scholar] [CrossRef]
- Altun, G.; Saraçbaşi, T. Determination of model fitting with power-divergence-type measure of departure from symmetry for sparse and non-sparse contingency tables. Commun. Stat.-Simul. Comput. 2022, 51, 4087–4111. [Google Scholar] [CrossRef]
- Bishop, Y.M.; Fienberg, S.E.; Holland, P.W. Discrete Multivariate Analysis: Theory and Practice; MIT Press: Cambridge, MA, USA, 1975. [Google Scholar]
- Haberman, S.J. Analysis of Qualitative Data, Volume 2: New Developments; Academic Press: New York, NY, USA, 1979. [Google Scholar]
- Ireland, C.T.; Ku, H.H.; Kullback, S. Symmetry and marginal homogeneity of an r × r contingency table. J. Am. Stat. Assoc. 1969, 64, 1323–1341. [Google Scholar] [CrossRef]
- Ward, R.C.; Gray, L.J. Eigensystem computation for skew-symmetric matrices and a class of symmetric matrices. ACM Trans. Math. Softw. 1978, 4, 278–285. [Google Scholar] [CrossRef]
- Beh, E.J. Elliptical confidence regions for simple correspondence analysis. J. Stat. Plan. Inference 2010, 140, 2582–2588. [Google Scholar] [CrossRef]
- Beh, E.J.; Lombardo, R. Confidence regions and approximate p-values for classical and non symmetric correspondence analysis. Commun. Stat.-Theory Methods 2015, 44, 95–114. [Google Scholar] [CrossRef]
- Alzahrani, A.; Beh, E.J.; Stojanovski, E. Confidence regions for simple correspondence analysis using the Cressie-Read family of divergence statistics. Electron. J. Appl. Stat. Anal. 2023, 16, 423–448. [Google Scholar]
- van der Heijden, P.G.M.; de Vries, H.; van Hooff, J.A.R.A.M. Correspondence analysis of transition matrices, with special attention to missing entries and asymmetry. Anim. Behav. 1990, 40, 49–64. [Google Scholar] [CrossRef]
- Csisźar, I. Information-type measures of difference of probability distributions and indirect observations. Stud. Sci. Math. Hung. 1967, 2, 229–318. [Google Scholar]
- Kharazmi, O.; Contreras-Reyes, J.E. Deng-Fisher information measure and its extensions: Application to Conway’s Game of Life. Chaos Solitons Fractals 2023, 174, 113871. [Google Scholar] [CrossRef]

| Columns | |||||
|---|---|---|---|---|---|
| Rows | C1 | C2 | C3 | C4 | Total |
| R1 | 10 | 20 | 30 | 40 | 100 |
| R2 | 20 + C | 50 | 60 | 70 | 200 + C |
| R3 | 30 | 60 | 20 | 40 | 150 |
| R4 | 40 | 70 | 40 | 80 | 230 |
| Total | 100 + C | 200 | 150 | 230 | 680 + C |
| Pre-Courtship Behaviour | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Post- | jk | tu | hb | cs | fl | qu | le | hd | sk | sn | cf | ff | Total |
| JK | 654 | 128 | 172 | 56 | 27 | 25 | 1 | 28 | 0 | 46 | 14 | 18 | 1169 |
| TU | 101 | 132 | 62 | 27 | 5 | 1 | 1 | 11 | 0 | 8 | 5 | 9 | 362 |
| HB | 171 | 62 | 197 | 130 | 0 | 25 | 0 | 50 | 14 | 18 | 14 | 12 | 693 |
| CS | 60 | 22 | 152 | 135 | 0 | 8 | 0 | 43 | 16 | 15 | 12 | 4 | 467 |
| FL | 19 | 2 | 0 | 0 | 419 | 19 | 0 | 2 | 0 | 17 | 5 | 11 | 494 |
| QU | 36 | 1 | 18 | 5 | 12 | 789 | 119 | 295 | 26 | 70 | 1 | 14 | 1386 |
| LE | 4 | 0 | 0 | 0 | 0 | 57 | 167 | 73 | 0 | 8 | 0 | 0 | 309 |
| HD | 22 | 9 | 40 | 37 | 5 | 245 | 7 | 171 | 287 | 53 | 8 | 13 | 897 |
| SK | 3 | 2 | 7 | 38 | 0 | 120 | 8 | 134 | 19 | 28 | 4 | 9 | 363 |
| SN | 42 | 2 | 17 | 16 | 20 | 70 | 11 | 67 | 9 | 225 | 12 | 12 | 503 |
| CF | 18 | 3 | 10 | 13 | 6 | 5 | 0 | 8 | 0 | 24 | 97 | 9 | 193 |
| FF | 27 | 3 | 6 | 5 | 10 | 13 | 0 | 18 | 0 | 10 | 8 | 29 | 129 |
| Total | 1157 | 366 | 681 | 462 | 504 | 1377 | 314 | 900 | 371 | 522 | 180 | 131 | 6965 |
| Pre-Courtship Behaviour | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Post- | jk | tu | hb | cs | fl | qu | le | hd | sk | sn | cf | ff | |
| 1 | 0.015 | <0.001 | −0.003 | 0.010 | −0.012 | −0.011 | 0.007 | −0.015 | 0.004 | −0.006 | −0.011 | ||
| JK | 1/2 | 0.015 | <0.001 | −0.003 | 0.010 | −0.013 | −0.014 | 0.007 | −0.027 | 0.004 | −0.006 | −0.012 | |
| 0 | 0.014 | <0.001 | −0.003 | 0.009 | −0.013 | −0.017 | 0.007 | −0.074 | 0.004 | −0.006 | −0.013 | ||
| 1 | −0.015 | 0 | 0.006 | 0.010 | 0 | 0.008 | 0.004 | −0.012 | 0.016 | 0.006 | 0.015 | ||
| TU | 1/2 | −0.016 | 0 | 0.006 | 0.009 | 0 | 0.007 | 0.004 | −0.022 | 0.014 | 0.006 | 0.013 | |
| 0 | −0.016 | 0 | 0.006 | 0.008 | 0 | 0.006 | 0.004 | −0.056 | 0.013 | 0.005 | 0.012 | ||
| 1 | <0.001 | 0 | −0.011 | 0 | 0.009 | 0 | 0.009 | 0.013 | 0.001 | 0.007 | 0.012 | ||
| HB | 1/2 | <0.001 | 0 | −0.011 | 0 | 0.009 | 0 | 0.009 | 0.012 | 0.001 | 0.007 | 0.011 | |
| 0 | <0.001 | 0 | −0.012 | 0 | 0.008 | 0 | 0.008 | 0.011 | 0.001 | 0.006 | 0.010 | ||
| 1 | 0.003 | −0.006 | 0.011 | 0 | 0.007 | 0 | 0.006 | −0.025 | −0.002 | −0.002 | −0.003 | ||
| CS | 1/2 | 0.003 | −0.006 | 0.011 | 0 | 0.007 | 0 | 0.006 | −0.029 | −0.002 | −0.002 | −0.003 | |
| 0 | 0.003 | −0.006 | 0.011 | 0 | 0.006 | 0 | 0.005 | −0.033 | −0.002 | −0.002 | −0.003 | ||
| 1 | −0.010 | −0.010 | 0 | 0 | 0.011 | 0 | −0.010 | 0 | −0.004 | −0.003 | 0.002 | ||
| FL | 1/2 | −0.010 | −0.011 | 0 | 0 | 0.010 | 0 | −0.011 | 0 | −0.004 | −0.003 | 0.002 | |
| 0 | −0.011 | −0.013 | 0 | 0 | 0.010 | 0 | −0.013 | 0 | −0.004 | −0.003 | 0.002 | ||
| 1 | 0.012 | 0 | −0.009 | −0.007 | −0.011 | 0.040 | 0.018 | −0.066 | 0 | −0.014 | 0.002 | ||
| QU | 1/2 | 0.011 | 0 | −0.009 | −0.008 | −0.011 | 0.037 | 0.018 | −0.083 | 0 | −0.017 | 0.002 | |
| 0 | 0.011 | 0 | −0.010 | −0.008 | −0.012 | 0.034 | 0.017 | −0.106 | 0 | −0.023 | 0.002 | ||
| 1 | 0.011 | −0.008 | 0 | 0 | 0 | −0.040 | 0.063 | −0.024 | −0.006 | 0 | 0 | ||
| LE | 1/2 | 0.010 | −0.015 | 0 | 0 | 0 | −0.044 | 0.053 | −0.046 | −0.006 | 0 | 0 | |
| 0 | 0.009 | −0.034 | 0 | 0 | 0 | −0.049 | 0.046 | −0.144 | −0.006 | 0 | 0 | ||
| 1 | −0.007 | −0.004 | −0.009 | −0.006 | 0.010 | −0.018 | −0.063 | 0.063 | −0.011 | 0 | −0.008 | ||
| HD | 1/2 | −0.007 | −0.004 | −0.009 | −0.006 | 0.009 | −0.019 | −0.088 | 0.058 | −0.011 | 0 | −0.008 | |
| 0 | −0.008 | −0.004 | −0.009 | −0.006 | 0.008 | −0.019 | −0.132 | 0.054 | −0.012 | 0 | −0.008 | ||
| 1 | 0.015 | 0.012 | −0.013 | 0.025 | 0 | 0.066 | 0.024 | −0.063 | 0.026 | 0.017 | 0 | ||
| SK | 1/2 | 0.012 | 0.010 | −0.014 | 0.023 | 0 | 0.058 | 0.020 | −0.070 | 0.024 | 0.014 | 0 | |
| 0 | 0.010 | 0.008 | −0.016 | 0.021 | 0 | 0.051 | 0.017 | −0.079 | 0.021 | 0.012 | 0 | ||
| 1 | −0.004 | −0.016 | −0.001 | 0.002 | 0.004 | 0 | 0.006 | 0.011 | −0.026 | −0.017 | 0.004 | ||
| SN | 1/2 | −0.004 | −0.020 | −0.001 | 0.002 | 0.004 | 0 | 0.006 | 0.011 | −0.031 | −0.019 | 0.004 | |
| 0 | −0.004 | −0.024 | −0.001 | 0.001 | 0.004 | 0 | 0.005 | 0.010 | −0.037 | −0.021 | 0.003 | ||
| 1 | 0.006 | −0.006 | −0.007 | 0.002 | 0.003 | 0.014 | 0 | 0 | −0.017 | 0.017 | 0.002 | ||
| CF | 1/2 | 0.006 | −0.006 | −0.007 | 0.002 | 0.002 | 0.012 | 0 | 0 | −0.032 | 0.016 | 0.002 | |
| 0 | 0.006 | −0.007 | −0.008 | 0.002 | 0.002 | 0.011 | 0 | 0 | −0.090 | 0.015 | 0.002 | ||
| 1 | 0.011 | −0.015 | −0.012 | 0.003 | −0.002 | −0.002 | 0 | 0.008 | 0 | −0.004 | −0.002 | ||
| FF | 1/2 | 0.011 | −0.017 | −0.013 | 0.003 | −0.002 | −0.002 | 0 | 0.007 | 0 | −0.004 | −0.002 | |
| 0 | 0.010 | −0.020 | −0.015 | 0.003 | −0.002 | −0.002 | 0 | 0.007 | 0 | −0.004 | −0.002 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Beh, E.J.; Lombardo, R. Correspondence Analysis for Assessing Departures from Perfect Symmetry Using the Cressie–Read Family of Divergence Statistics. Symmetry 2024, 16, 830. https://doi.org/10.3390/sym16070830
Beh EJ, Lombardo R. Correspondence Analysis for Assessing Departures from Perfect Symmetry Using the Cressie–Read Family of Divergence Statistics. Symmetry. 2024; 16(7):830. https://doi.org/10.3390/sym16070830
Chicago/Turabian StyleBeh, Eric J., and Rosaria Lombardo. 2024. "Correspondence Analysis for Assessing Departures from Perfect Symmetry Using the Cressie–Read Family of Divergence Statistics" Symmetry 16, no. 7: 830. https://doi.org/10.3390/sym16070830
APA StyleBeh, E. J., & Lombardo, R. (2024). Correspondence Analysis for Assessing Departures from Perfect Symmetry Using the Cressie–Read Family of Divergence Statistics. Symmetry, 16(7), 830. https://doi.org/10.3390/sym16070830






