Numerical Approach Based on the Haar Wavelet Collocation Method for Solving a Coupled System with the Caputo–Fabrizio Fractional Derivative
Abstract
1. Introduction
2. Basic Knowledge
2.1. Caputo–Fabrizio Fractional Integral and Derivative
2.2. Haar Wavelet Basis
3. Haar Wavelet Approximation Method
3.1. Method of Solution
3.2. Analysis of Error Estimations
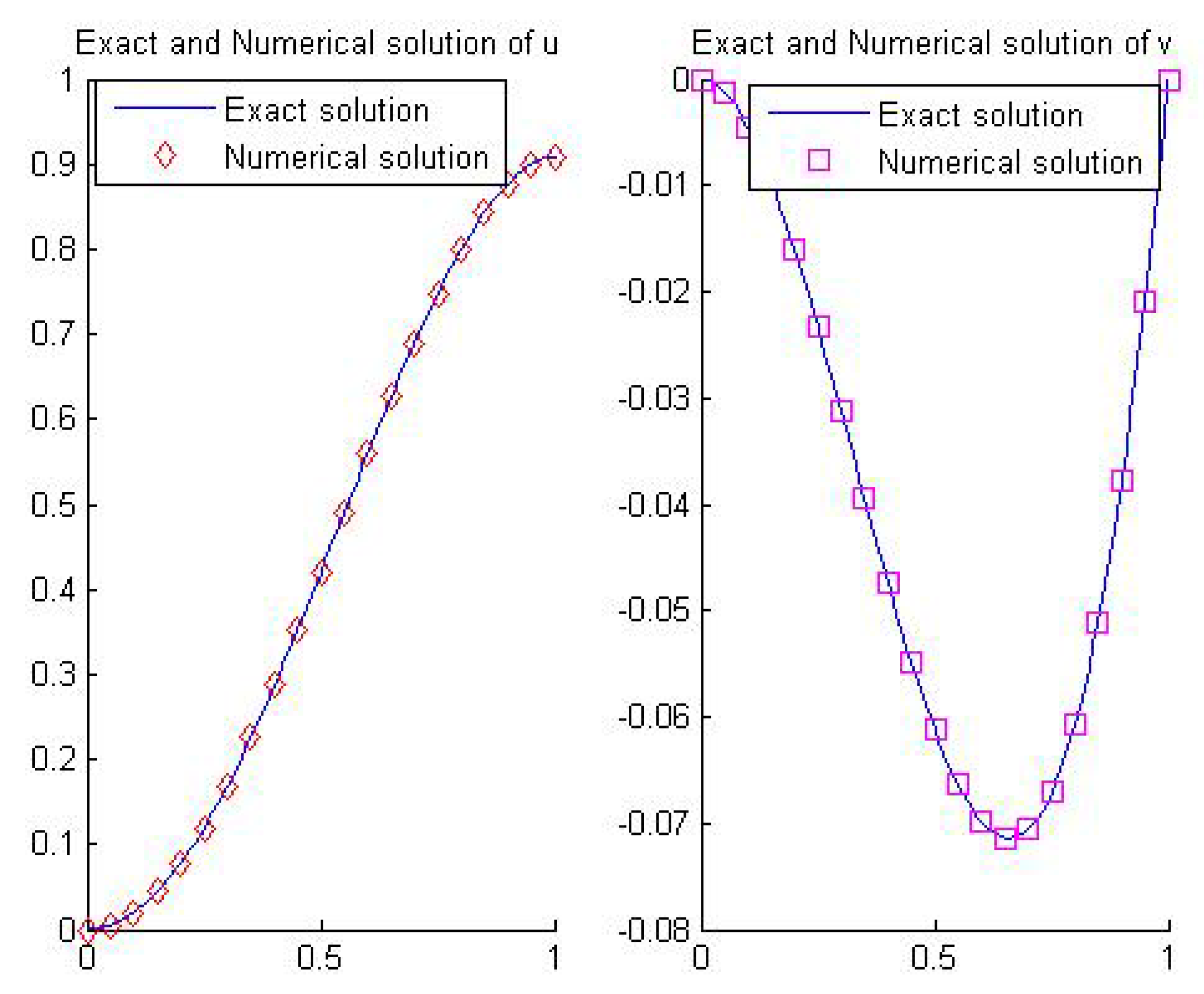
4. Numerical Examples
5. Conclusions
- It can decrease computational efforts and is suitable in terms of computation costs and the convergence rate.
- It is suitable for the analysis of the dynamical system with fractional derivatives.
- The convergence properties of this class of methods are very helpful.
- The error analysis of this method is characterized by a good convergence rate.
- Unlike the collocation method, other methods have several limitations in their applications to unlimited classes of singular problems.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dehda, B.; Ahmed, A.A.; Yazid, F.; Djeradi, F.S. Numerical solution of a class of Caputo–Fabrizio derivative problem using Haar wavelet collocation method. J. Appl. Math. Comput. 2023, 69, 2761–2774. [Google Scholar] [CrossRef]
- Qureshi, S.; Rangaig, N.A.; Baleanu, D. New Numerical Aspects of Caputo–Fabrizio Fractional Derivative Operator. Mathematics 2019, 7, 374. [Google Scholar] [CrossRef]
- Bekkouche, M.M.; Mansouri, I.; Ahmed, A.A. Numerical solution of fractional boundary value problem with Caputo–Fabrizio and its fractional integral. J. Appl. Math. Comput. 2022, 68, 4305–4316. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. A New Definition of Fractional Derivative without Singular Kernel. Progr. Fract. Differ. Appl. 2015, 1, 73–85. [Google Scholar]
- Losada, J.; Nieto, J.J. Properties of a New Fractional Derivative without Singular Kernel. Progr. Fract. Differ. Appl. 2015, 1, 87–92. [Google Scholar]
- Mansouri, I.; Bekkouche, M.M.; Ahmed, A.A. Numerical Solution of A Fractional Coupled System with The Caputo–Fabrizio Fractional Derivative. J. Appl. Math. Computat. Mech. 2023, 22, 46–56. [Google Scholar] [CrossRef]
- Shiralashetti, S.C.; Harishkumar, E. Haar wavelet matrices for the numerical solution of system of ordinary differential equations. Malaya J. Mat. 2020, 1, 144–147. [Google Scholar]
- Heydari, M.; Avazzadeh, Z.; Hosseinzadeh, N. Haar Wavelet Method for Solving High-Order Differential Equations with Multi-Point Boundary Conditions. J. Appl. Comput. Mech. 2022, 8, 528–544. [Google Scholar]
- Islam, S.; Aziz, I.; Ahmad, M. Numerical solution of two-dimensional elliptic PDEs with nonlocal boundary conditions. Comput. Math. Appl. 2015, 69, 180–205. [Google Scholar] [CrossRef]
- Wichailukkana, N.; Novaprateep, B.; Boonyasiriwat, C. A convergence analysis of the numerical solution of boundary value problems by using two-dimensional Haar wavelets. Sci. Asia 2016, 42, 346–355. [Google Scholar] [CrossRef]
- Aziz, I.; Islam, S.; Khan, F. A new method based on Haar wavelet for the numerical solution of two-dimensional nonlinear integral equations. J. Comput. Appl. Math. 2014, 272, 70–80. [Google Scholar] [CrossRef]
- Babolian, E.; Shahsavaran, A. Numerical solution of nonlinear Fredholm integral equations of the second kind using Haar wavelets. J. Comput. Appl. Math. 2009, 225, 87–95. [Google Scholar] [CrossRef]
- Fallahpour, M.; Khodabin, M.; Maleknejad, K. Theoretical Error Analysis of Solution for Two-Dimensional Stochastic Volterra Integral Equations by Haar Wavelet. Int. J. Appl. Comput. Math. 2019, 5, 152. [Google Scholar] [CrossRef]
- Singh, I.; Kumar, S. Haar wavelet method for some nonlinear Volterra integral equations of the first kind. J. Comput. Appl. Math. 2016, 292, 541–552. [Google Scholar] [CrossRef]
- Alrabaiah, H.; Ahmad, I.; Amin, R.; Shah, K. A numerical method for fractional variable order pantograph differential equations based on Haar wavelet. Eng. Comput. 2022, 38, 2655–2668. [Google Scholar] [CrossRef]
- Al-Shimmary, A.; Radhi, S.K.; Hussain, A.K. Haar wavelet method for solving coupled system of fractional order partial differential equations. Indones. J. Electr. Eng. Comp. Sci. 2021, 21, 1444–1454. [Google Scholar] [CrossRef]
- Amin, R.; Shah, K.; Asif, M.; Khan, I.; Ullah, F. An efficient algorithm for numerical solution of fractional integro-differential equations via Haar wavelet. J. Comput. Appl. Math. 2020, 381, 113028. [Google Scholar] [CrossRef]
- Laique, Z. Imran, A. Numerical solution of fractional partial differential equations via Haar wavelet. Numer. Meth. Part. Diff. Equ. 2020, 38, 222–242. [Google Scholar]
- Shah, F.A.; Abass, R. Haar Wavelet Operational Matrix Method for the Numerical Solution of Fractional Order Differential Equations. Nonl. Eng. 2015, 4, 203–213. [Google Scholar] [CrossRef]
- Shiralashetti, S.C.; Deshi, A.B. An efficient Haar wavelet collocation method for the numerical solution of multi-term fractional differential equations. Nonl. Dyn. 2016, 83, 293–303. [Google Scholar] [CrossRef]
- Urrehman, M.; Khan, R.A. A numerical method for solving boundary value problems for fractional differential equations. Appl. Math. Model. 2012, 36, 894–907. [Google Scholar]
- Azzaoui, B.; Tellab, B.; Zennir, K. Positive solutions for integral nonlinear boundary value problem in fractional Sobolev spaces. Math. Meth. Appl. Sci. 2023, 46, 3115–3131. [Google Scholar] [CrossRef]
- Naimi, A.; Tellab, B. Zennir, K. Existence and stability results of a nonlinear fractional integro-differential equation with integral boundary conditions. Kragujevac J. Math. 2022, 46, 685–699. [Google Scholar]
- Wang, L.; Wang, Z.; Qian, Z. A meshfree method for inverse wave propagation using collocation and radial basis functions. Comp. Meth. Appl. Mech. Eng. 2017, 322, 311–350. [Google Scholar] [CrossRef]
- Wang, L.; Liu, Y.; Zhou, Y.; Yang, F. Static and dynamic analysis of thin functionally graded shell with in-plane material inhomogeneity. Int. J. Mech. Sci. 2021, 193, 106165. [Google Scholar] [CrossRef]
- Wang, L.; Chen, J.S. Subdomain radial basis collocation method for fracture mechanics. Int. J. Nume. Meth. Eng. 2010, 83, 851–876. [Google Scholar] [CrossRef]



| J | M | of Proposed Method | of ADM | ||
|---|---|---|---|---|---|
| 0 | 1 | 2 | |||
| 1 | 2 | 4 | |||
| 2 | 4 | 8 | |||
| 3 | 8 | 16 | |||
| 4 | 16 | 32 |
| J | M | of Proposed Method | of ADM | ||
|---|---|---|---|---|---|
| 0 | 1 | 2 | |||
| 1 | 2 | 4 | |||
| 2 | 4 | 8 | |||
| 3 | 8 | 16 | |||
| 4 | 16 | 32 |
| J | M | of Proposed Method | of ADM | ||
|---|---|---|---|---|---|
| 0 | 1 | 2 | |||
| 1 | 2 | 4 | |||
| 2 | 4 | 8 | |||
| 3 | 8 | 16 | |||
| 4 | 16 | 32 |
| J | M | of Proposed Method | of ADM | ||
|---|---|---|---|---|---|
| 0 | 1 | 2 | |||
| 1 | 2 | 4 | |||
| 2 | 4 | 8 | |||
| 3 | 8 | 16 | |||
| 4 | 16 | 32 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dehda, B.; Yazid, F.; Djeradi, F.S.; Zennir, K.; Bouhali, K.; Radwan, T. Numerical Approach Based on the Haar Wavelet Collocation Method for Solving a Coupled System with the Caputo–Fabrizio Fractional Derivative. Symmetry 2024, 16, 713. https://doi.org/10.3390/sym16060713
Dehda B, Yazid F, Djeradi FS, Zennir K, Bouhali K, Radwan T. Numerical Approach Based on the Haar Wavelet Collocation Method for Solving a Coupled System with the Caputo–Fabrizio Fractional Derivative. Symmetry. 2024; 16(6):713. https://doi.org/10.3390/sym16060713
Chicago/Turabian StyleDehda, Bachir, Fares Yazid, Fatima Siham Djeradi, Khaled Zennir, Keltoum Bouhali, and Taha Radwan. 2024. "Numerical Approach Based on the Haar Wavelet Collocation Method for Solving a Coupled System with the Caputo–Fabrizio Fractional Derivative" Symmetry 16, no. 6: 713. https://doi.org/10.3390/sym16060713
APA StyleDehda, B., Yazid, F., Djeradi, F. S., Zennir, K., Bouhali, K., & Radwan, T. (2024). Numerical Approach Based on the Haar Wavelet Collocation Method for Solving a Coupled System with the Caputo–Fabrizio Fractional Derivative. Symmetry, 16(6), 713. https://doi.org/10.3390/sym16060713







