A Deterministic and Stochastic Fractional-Order ILSR Rumor Propagation Model Incorporating Media Reports and a Nonlinear Inhibition Mechanism
Abstract
1. Introduction
2. Numerous Concepts Associated with the Field of Fractional Calculus
- 1.
- and exhibit continuity throughout .
- 2.
- for all , with and consisting of a pair of positive variables.
3. Description of the Model
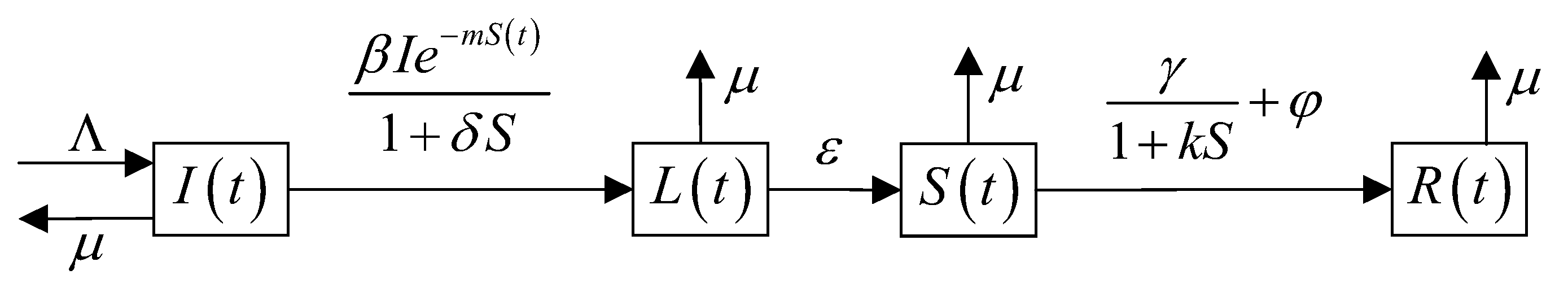
- (i)
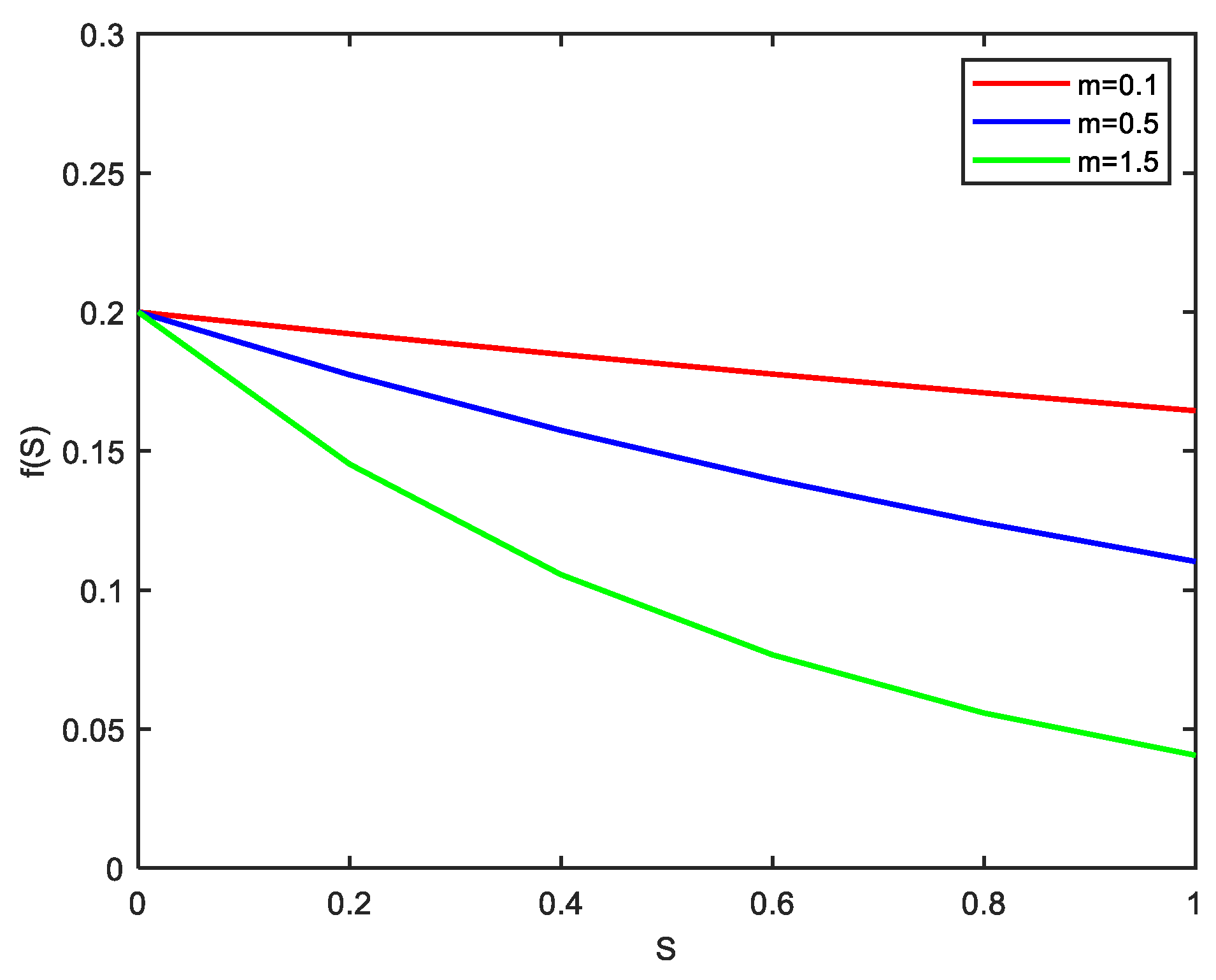
- When an Ignorant has contact with a Latent, the Ignorant is affected and becomes a Latent with the possibility of , where denotes the rumor contact rate, denotes the saturation constant, and implies the level of the media reports. Obviously, as . The timely popularization of scientific knowledge and countering rumors through media reports can help control rumors to a certain extent. The influence of media reports on communication is not inherently decisive, Ref. [15]. The transmission rate is influenced by media reports in Figure 1.
- (ii)
- Some Latents may tend to become Spreaders with a transfer rate and the parameter represents the recovery rate of Spreaders when they are exposed to the impacts of the forgetting mechanism.
- (iii)
- represents a saturated treatment function that represents the nonlinear inhibition mechanism, where and . means the highest level of inhibition measure for the Spreaders group.
- (iv)
- We make the assumption that the rate of immigration is , while the rate of emigration is . All the parameters of the model are assumed to possess constant and positive values.
4. Analysis of the Model
5. Fractional Optimal Control of the Model
6. ILSR Rumor Model with Fractional Stochastic
7. Sensitivity Analysis and Numerical Simulation
7.1. Sensitivity Analysis
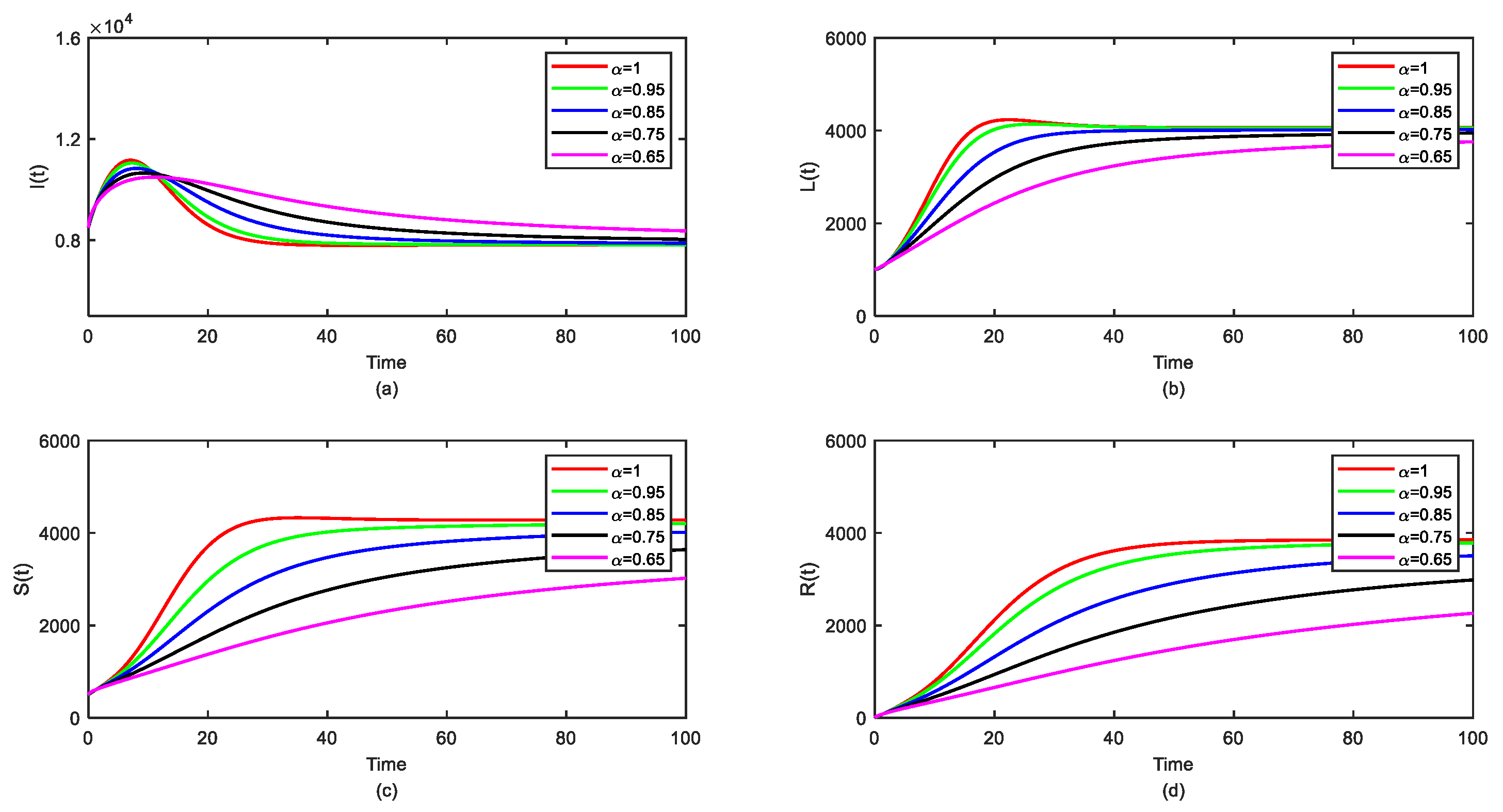
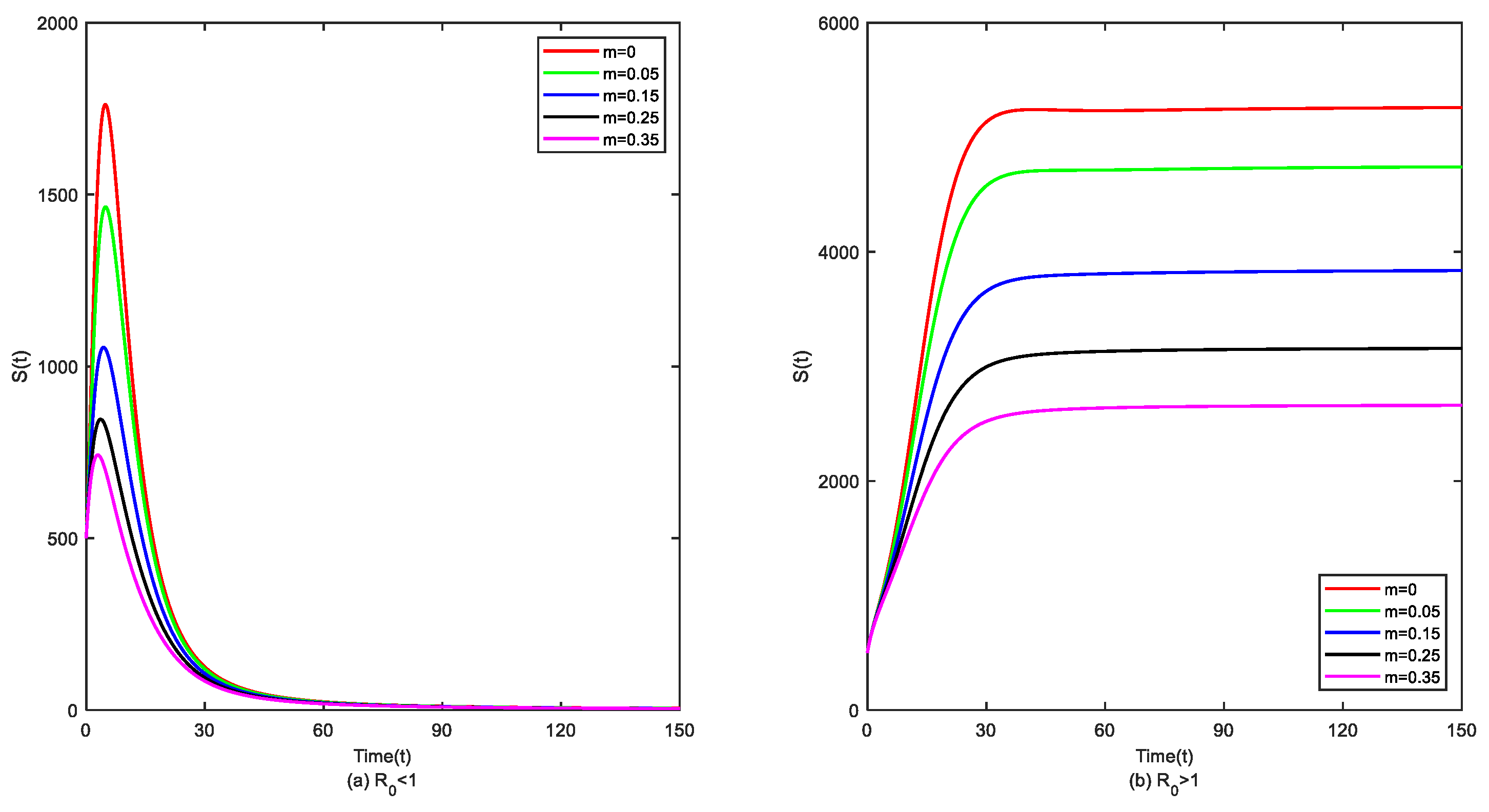
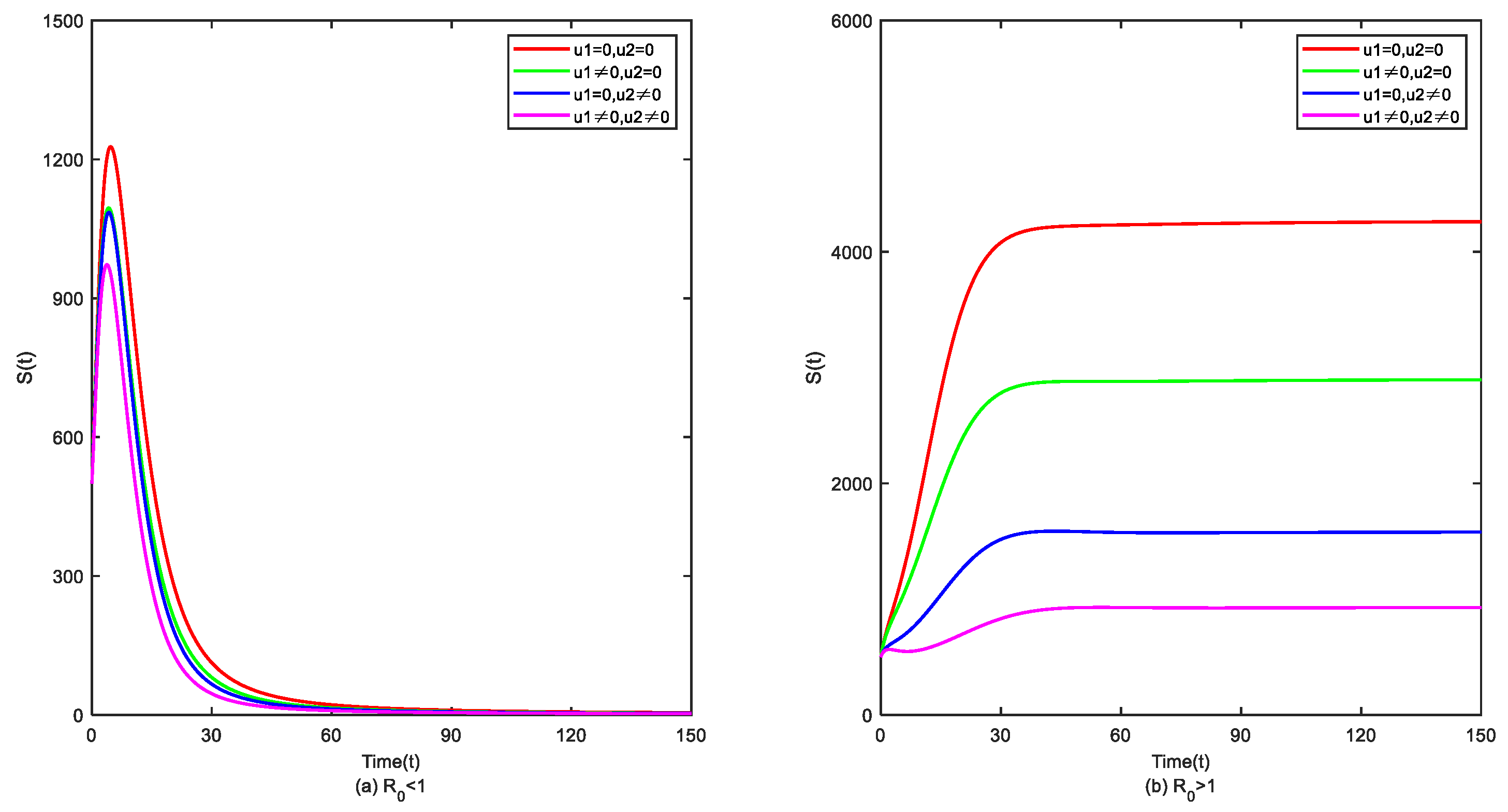
7.2. Numerical Simulation
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bodaghi, A.; Goliaei, S.; Salehi, M. The number of followings as an influential factor in rumor spreading. Appl. Math. Comput 2019, 357, 167–184. [Google Scholar] [CrossRef]
- Luo, P.; Wang, C.; Guo, F.; Luo, L. Factors affecting individual online rumor sharing behavior in the COVID-19 pandemic. Comput. Hum. Behav. 2021, 125, 106968. [Google Scholar] [CrossRef] [PubMed]
- Daley, D.J.; Kendall, D.G. Epidemics and rumours. Nature 1964, 204, 1118. [Google Scholar] [CrossRef] [PubMed]
- Maki, D.P.; Thompson, M. Mathematical Models and Applications: With Emphasis on the Social, Life, and Management Sciences; Prentice-Hall: Upper Saddle River, NJ, USA, 1973; ISBN 9780135616703. [Google Scholar]
- Zanette, D.H. Dynamics of rumor propagation on small-world networks. Phys. Rev. E 2002, 65, 041908. [Google Scholar] [CrossRef] [PubMed]
- Xia, L.-L.; Jiang, G.-P.; Song, B.; Song, Y.-R. Rumor spreading model considering hesitating mechanism in complex social networks. Phys. A Stat. Mech. Its Appl. 2015, 437, 295–303. [Google Scholar] [CrossRef]
- Wenkai, C.; Zhang, H.; Georgescu, P.; Li, T.; Zhang, B. Taming obstinate spreaders: The dynamics of a rumor spreading model incorporating inhibiting mechanisms and attitude adjustment. Comput. Appl. Math. 2021, 40, 125. [Google Scholar] [CrossRef]
- Capasso, V.; Serio, G. A generalization of the Kermack-McKendrick deterministic epidemic model. Math. Biosci. 1978, 42, 43–61. [Google Scholar] [CrossRef]
- Laarabi, H.; Rachik, M.; Kaddar, A. Optimal control of an epidemic model with a saturated incidence rate. Nonlinear Anal. Model. Control 2012, 17, 448–459. [Google Scholar] [CrossRef]
- Zhu, L.; Zhao, H. Dynamical behaviours and control measures of rumour-spreading model with consideration of network topology. Int. J. Syst. Sci. 2017, 48, 2064–2078. [Google Scholar] [CrossRef]
- Zhu, L.; Huang, X.; Liu, Y.; Zhang, Z. Spatiotemporal dynamics analysis and optimal control method for an SI reaction-diffusion propagation model. J. Math. Anal. Appl. 2021, 493, 124539. [Google Scholar] [CrossRef]
- Luo, X.; Jiang, H.; Chen, S.; Li, J. Stability and optimal control for delayed rumor-spreading model with nonlinear incidence over heterogeneous networks. Chin. Phys. B 2023, 32, 058702. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhu, J.; Din, A.; Ma, X. Dynamics of a stochastic epidemic-like rumor propagation model with generalized nonlinear incidence and time delay. Phys. Scr. 2023, 98, 045232. [Google Scholar] [CrossRef]
- Cui, J.; Sun, Y.; Zhu, H. The impact of media on the control of infectious diseases. J. Dyn. Differ. Equ. 2008, 20, 31–53. [Google Scholar] [CrossRef] [PubMed]
- Sahu, G.P.; Dhar, J. Dynamics of an SEQIHRS epidemic model with media coverage, quarantine and isolation in a community with pre-existing immunity. J. Math. Anal. Appl. 2015, 421, 1651–1672. [Google Scholar] [CrossRef] [PubMed]
- Huo, L.; Dong, Y. Analyzing the dynamics of a stochastic rumor propagation model incorporating media coverage. Math. Methods Appl. Sci. 2020, 43, 6903–6920. [Google Scholar] [CrossRef]
- Huo, L.-A.; Dong, Y.-F.; Lin, T.-T. Dynamics of a stochastic rumor propagation model incorporating media coverage and driven by Lévy noise. Chin. Phys. B 2021, 30, 080201. [Google Scholar] [CrossRef]
- Dai, L.; Liu, X.; Chen, Y. Global dynamics of a fractional-order SIS epidemic model with media coverage. Nonlinear Dyn. 2023, 111, 19513–19526. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhao, L. Stability analysis and optimal control of rumor spreading model under media coverage considering time delay and pulse vaccination. Chaos Solitons Fractals 2022, 157, 111931. [Google Scholar] [CrossRef]
- Pan, W.; Yan, W.; Hu, Y.; He, R.; Wu, L. Dynamic analysis of a SIDRW rumor propagation model considering the effect of media reports and rumor refuters. Nonlinear Dyn. 2023, 111, 3925–3936. [Google Scholar] [CrossRef]
- Guo, H.; Yan, X. Dynamic modeling and simulation of rumor propagation based on the double refutation mechanism. Inf. Sci. 2023, 630, 385–402. [Google Scholar] [CrossRef]
- Guo, H.; Yan, X.; Niu, Y.; Zhang, J. Dynamic analysis of rumor propagation model with media report and time delay on social networks. J. Appl. Math. Comput. 2023, 69, 2473–2502. [Google Scholar] [CrossRef]
- Zhu, L.-H.; Li, L. Dynamic analysis of rumor-spread-delaying model based on rumor-refuting mechanism. Acta Phys. Sin. 2020, 69, 020501. [Google Scholar] [CrossRef]
- Wang, J.; Jiang, H.; Hu, C.; Yu, Z.; Li, J. Stability and Hopf bifurcation analysis of multi-lingual rumor spreading model with nonlinear inhibition mechanism. Chaos Solitons Fractals 2021, 153, 111464. [Google Scholar] [CrossRef]
- Yuan, T.; Guan, G.; Shen, S.; Zhu, L. Stability analysis and optimal control of epidemic-like transmission model with nonlinear inhibition mechanism and time delay in both homogeneous and heterogeneous networks. J. Math. Anal. Appl. 2023, 526, 127273. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, Y.; Ye, Y. Complex dynamics and control strategies of SEIR heterogeneous network model with saturated treatment. Phys. A Stat. Mech. Its Appl. 2022, 608, 128287. [Google Scholar] [CrossRef]
- Zhu, L.; Liu, W.; Zhang, Z. Delay differential equations modeling of rumor propagation in both homogeneous and heterogeneous networks with a forced silence function. Appl. Math. Comput. 2020, 370, 124925. [Google Scholar] [CrossRef]
- Zhu, H.; Zhang, X.; An, Q. Global stability of a rumor spreading model with discontinuous control strategies. Phys. A Stat. Mech. Its Appl. 2022, 606, 128157. [Google Scholar] [CrossRef]
- Yu, S.; Yu, Z.; Jiang, H.; Mei, X.; Li, J. The spread and control of rumors in a multilingual environment. Nonlinear Dyn. 2020, 100, 2933–2951. [Google Scholar] [CrossRef]
- Ding, L.; Hu, P.; Guan, Z.-H.; Li, T. An efficient hybrid control strategy for restraining rumor spreading. IEEE Trans. Syst. Man Cybern. Syst. 2020, 51, 6779–6791. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, Y. Rumor spreading model with noise interference in complex social networks. Phys. A Stat. Mech. Its Appl. 2017, 469, 750–760. [Google Scholar] [CrossRef]
- Tong, X.; Jiang, H.; Qiu, J.; Luo, X.; Chen, S. Dynamic analysis of the IFCD rumor propagation model under stochastic disturbance on heterogeneous networks. Chaos Solitons Fractals 2023, 173, 113637. [Google Scholar] [CrossRef]
- Ghosh, M.; Das, P.; Das, P. A comparative study of deterministic and stochastic dynamics of rumor propagation model with counter-rumor spreader. Nonlinear Dyn. 2023, 111, 16875–16894. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, J. A rumor spreading model considering the cumulative effects of memory. Discret. Dyn. Nat. Soc. 2014, 2014, 204395. [Google Scholar] [CrossRef]
- Saeedian, M.; Khalighi, M.; Azimi-Tafreshi, N.; Jafari, G.; Ausloos, M. Memory effects on epidemic evolution: The susceptible-infected-recovered epidemic model. Phys. Rev. E 2017, 95, 22409. [Google Scholar] [CrossRef] [PubMed]
- González-Parra, G.; Arenas, A.J.; Chen-Charpentier, B.M. A fractional order epidemic model for the simulation of outbreaks of influenza A (H1N1). Math. Methods Appl. Sci. 2014, 37, 2218–2226. [Google Scholar] [CrossRef]
- Alzahrani, F.; Razzaq, O.A.; Rehman, D.U.; Khan, N.A.; Alshomrani, A.S.; Ullah, M.Z. Repercussions of unreported populace on disease dynamics and its optimal control through system of fractional order delay differential equations. Chaos Solitons Fractals 2022, 158, 111997. [Google Scholar] [CrossRef]
- Kheiri, H.; Jafari, M. Stability analysis of a fractional order model for the HIV/AIDS epidemic in a patchy environment. J. Comput. Appl. Math. 2019, 346, 323–339. [Google Scholar] [CrossRef]
- Ali, H.M.; Ameen, I.G. Optimal control strategies of a fractional order model for Zika virus infection involving various transmissions. Chaos Solitons Fractals 2021, 146, 110864. [Google Scholar] [CrossRef]
- Huo, J.; Zhao, H. Dynamical analysis of a fractional SIR model with birth and death on heterogeneous complex networks. Phys. A Stat. Mech. Its Appl. 2016, 448, 41–56. [Google Scholar] [CrossRef]
- Alzahrani, E.; Khan, M. Modeling the dynamics of Hepatitis E with optimal control. Chaos Solitons Fractals 2018, 116, 287–301. [Google Scholar] [CrossRef]
- Cui, X.; Xue, D.; Pan, F. Dynamic analysis and optimal control for a fractional-order delayed SIR epidemic model with saturated treatment. Eur. Phys. J. Plus 2022, 137, 586. [Google Scholar] [CrossRef]
- Singh, J. A new analysis for fractional rumor spreading dynamical model in a social network with Mittag-Leffler law. Chaos Interdiscip. J. Nonlinear Sci. 2019, 29, 013137. [Google Scholar] [CrossRef] [PubMed]
- Ye, M.; Li, J.; Han, X.; Jiang, H. Global analysis of a fractional-order reaction-diffusion rumor-spreading model in multi-lingual environment. Eur. Phys. J. Plus 2023, 138, 102. [Google Scholar] [CrossRef]
- Lakshmikantham, V.; Vatsala, A.S. Basic theory of fractional differential equations. Nonlinear Anal. Theory Methods Appl. 2008, 69, 2677–2682. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Lin, W. Global existence theory and chaos control of fractional differential equations. J. Math. Anal. Appl. 2007, 332, 709–726. [Google Scholar] [CrossRef]
- Diethelm, K. The mean value theorems and a Nagumo-type uniqueness theorem for Caputo’s fractional calculus. Fract. Calc. Appl. Anal. 2012, 15, 304–313. [Google Scholar] [CrossRef]
- Matignon, D. Stability results for fractional differential equations with applications to control processing. Comput. Eng. Syst. Appl. 1996, 2, 963–968. [Google Scholar]
- Gallegos, J.A.; Duarte-Mermoud, M.A. Robustness and convergence of fractional systems and their applications to adaptive schemes. Fract. Calc. Appl. Anal. 2017, 20, 895–913. [Google Scholar] [CrossRef]
- Yang, A.; Huang, X.; Cai, X.; Zhu, X.; Lu, L. ILSR rumor spreading model with degree in complex network. Phys. A Stat. Mech. Its Appl. 2019, 531, 121807. [Google Scholar] [CrossRef]
- Xia, Y.; Jiang, H.; Yu, Z. Global dynamics of ILSR rumor spreading model with general nonlinear spreading rate in multi-lingual environment. Chaos Solitons Fractals 2022, 154, 111698. [Google Scholar] [CrossRef]
- Li, H.-L.; Zhang, L.; Hu, C.; Jiang, Y.-L.; Teng, Z. Dynamical analysis of a fractional-order predator-prey model incorporating a prey refuge. J. Appl. Math. Comput. 2017, 54, 435–449. [Google Scholar] [CrossRef]
- Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef] [PubMed]
- Bourafa, S.; Abdelouahab, M.; Moussaoui, A. On some extended Routh–Hurwitz conditions for fractional-order autonomous systems of order α∈(0, 2) and their applications to some population dynamic models. Chaos Solitons Fractals 2020, 133, 109623. [Google Scholar] [CrossRef]
- Hespanha, J.P. Uniform stability of switched linear systems: Extensions of LaSalle’s invariance principle. IEEE Trans. Autom. Control 2004, 49, 470–482. [Google Scholar] [CrossRef]
- Rishel, R.W. An extended Pontryagin principle for control systems whose control laws contain measures. J. Soc. Ind. Appl. Math. Ser. A Control 1965, 3, 191–205. [Google Scholar] [CrossRef]
- Zhang, N.; Jia, G. W-symmetries of backward stochastic differential equations, preservation of simple symmetries and Kozlov’s theory. Commun. Nonlinear Sci. Numer. Simul. 2021, 93, 105527. [Google Scholar] [CrossRef]
- Roshan, T.; Ghosh, S.; Chauhan, R.P.; Kumar, S. A robust study on fractional order HIV/AIDS model by using numerical methods. Eng. Comput. 2023, 40, 1545–1569. [Google Scholar] [CrossRef]
- Majee, S.; Jana, S.; Das, D.K.; Kar, T. Global dynamics of a fractional-order HFMD model incorporating optimal treatment and stochastic stability. Chaos Solitons Fractals 2022, 161, 112291. [Google Scholar] [CrossRef]
- Data, Z. Mathematical Models and Applications: With Emphasis on the Social, Life, and Management Sciences. 2023. Available online: https://efzhiweidatacom/event/e6b93ad10916233c10051487/trend (accessed on 23 April 2023).





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, X.; Zhu, W. A Deterministic and Stochastic Fractional-Order ILSR Rumor Propagation Model Incorporating Media Reports and a Nonlinear Inhibition Mechanism. Symmetry 2024, 16, 602. https://doi.org/10.3390/sym16050602
Yue X, Zhu W. A Deterministic and Stochastic Fractional-Order ILSR Rumor Propagation Model Incorporating Media Reports and a Nonlinear Inhibition Mechanism. Symmetry. 2024; 16(5):602. https://doi.org/10.3390/sym16050602
Chicago/Turabian StyleYue, Xuefeng, and Weiwei Zhu. 2024. "A Deterministic and Stochastic Fractional-Order ILSR Rumor Propagation Model Incorporating Media Reports and a Nonlinear Inhibition Mechanism" Symmetry 16, no. 5: 602. https://doi.org/10.3390/sym16050602
APA StyleYue, X., & Zhu, W. (2024). A Deterministic and Stochastic Fractional-Order ILSR Rumor Propagation Model Incorporating Media Reports and a Nonlinear Inhibition Mechanism. Symmetry, 16(5), 602. https://doi.org/10.3390/sym16050602





