Indeterminate Stieltjes Moment Problem: Entropy Convergence
Abstract
1. Introduction
- improve the proof of convergence in entropy provided by [1], Section 3, when the Stieltjes moment problem is determinate, listing through the use of the limit parabolic regions described below all the possible cases that may arise;
- state and prove an analogous entropy convergence result in the case of the indeterminate Stieltjes moment problem. That extension is not immediate and additional considerations of MaxEnt distribution properties are required.
- Consider the so-called Stieltjes class ([6], Section 3) with a center at and perturbation function h defined as follows:where , is a continuous probability density function, that is, , where F is the distribution function of some random variable X and is a non-identically zero continuous function, satisfying the condition , for all . Then, is an infinite family of densities all sharing the same moment sequence as and obviously requires knowledge of both functions and h. The latter satisfies the infinite relations , con . Usually, both and h are chosen by trial and error to satisfy (1). It is well-known that convergence in entropy implies convergence almost everywhere. Consequently, it makes sense to assume since it is distinguishable (hence, identifiable) from all densities in . Recently, h has been obtained with a precise theoretical procedure by [7], Thm. 1.2, starting from . Therefore, through the combination of the proposal to assume with the theoretical procedure of López-Garcia for the calculation of h, a complete theoretical program for the construction of Stieltjes classes solely using the given moment sequence is outlined.The originality of our study lies in the fact that, within the class of densities, we identify a particular one, , with the highest entropy . This density is the limit of a sequence of MaxEnt densities with an increasing number of moments coinciding with the given one.
- In the so-called Method of Moments, [8], Section 6, p. 540–541, proved the following.Theorem 1 ([9]). Let and F be probability distributions supported on with finite moments of all orders, which we denote by and , respectively. If F is the only distribution with the moments and if for , then the sequence converges in distribution to F; that isat any point of continuity of .The uniqueness of is assumed to be not only sufficient for the validity of the relation (2) (at any point of continuity) but also necessary. Theorem 7 below leads us to conjecture that the assumed moment problem determinacy condition, designed to guarantee convergence in distribution, could be sufficient but not necessary. This topic will be investigated in future research.
2. Moment Problem: Existence and Determinacy Conditions
3. Limit Parabolic Regions
- 1.
- Hamburger caseSuppose is a moment sequence for which the moments are kept fixed while the moments and are left to be continuously varying. Setting and , the whole moment sequence can be written as and the numbers x and y (i.e., the moments preceding ) cannot be arbitrary.Following [15], we set and consider the moments:where, as before, are fixed and and can vary continuously. For each M, the relation defines a closed convex region as follows:which is bounded by a proper parabola with horizontal axis and vertex in the right-half plane. Since, for , the Hankel matrices are positive semidefinite, the regions are nested (i.e., ) and the element of interest is their intersection, , called a limit parabolic region. One possibility is that is a proper closed region in the right-half plane such that is bounded by a proper parabola and contains the initially given moments The alternative is that degenerates, becoming just a ray.Theorem 5 ([15], Thm 1). Let be a Hamburger moment sequence for the measure ν. Then, ν is indeterminate if and only if the point is an interior point of the limit parabolic region .
- 2.
- Stieltjes caseSuppose that is a Stieltjes moment sequence. In this case, we develop a procedure similar to that in the Hamburger case but with some specific features. For doing this, we rely essentially on two known results. First, we recall that every Stieltjes sequence can also be considered a Hamburger sequence; hence, the existence of the limit parabolic region is assured and defined by the two relations and , in which we use and . Second, , a ray, or is the intersection of proper limit parabolic regions in the half plain . Taking into account Theorem 5, the following conclusion can be drawn.Theorem 6 ([15], Thm 2). The positive definite Stieltjes moment sequence generates an indeterminate measure ν if and only if two conditions are satisfied:
- (i)
- the point is an interior point of the limit parabolic region ;
- (ii)
- .
4. Stieltjes Moment Problem
4.1. Maxent Method
4.2. Limit Parabolic Regions for S-Det or S-Indet
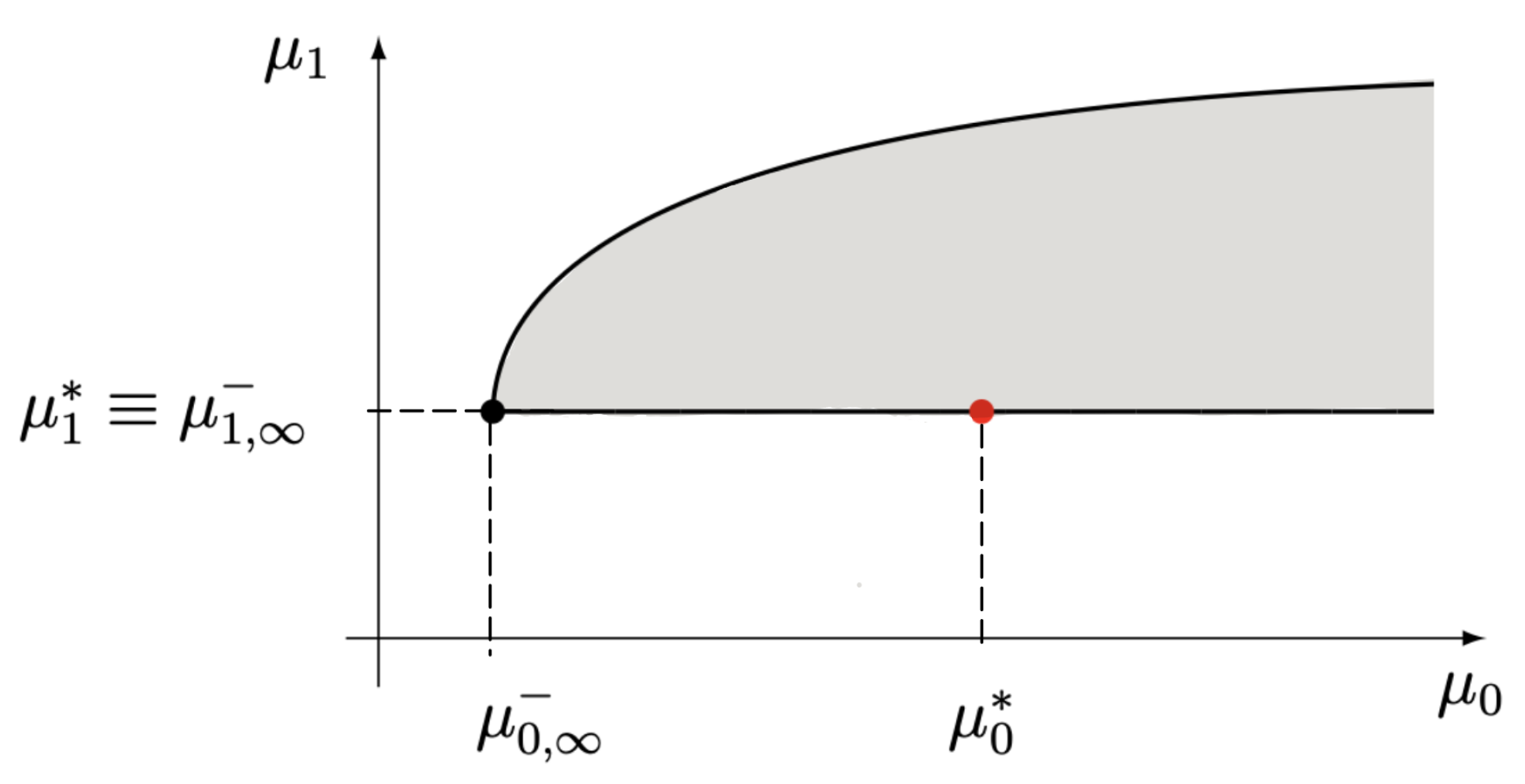
- Case 1:
- is S-det with H-indet (see [15], Corollary, p. 417). Combining Theorems 3 and 4, it follows thatequivalently, and both from (6) and (see Figure 1).Note that both here and in the following graphs, the shaded area represents the parabolic region and the red dot indicates the fixed value of the point . Ref. [17], in a corollary on p. 481, states that if a measure is determinate in the Stieltjes sense and indeterminate in the Hamburger sense, then is a Nevanlinna-extremal measure and, in particular, is discrete with a positive mass at the origin. As a consequence, the S-det case is disregarded. In contrast, the H-indet case where infinitely many densities occur, will be investigated in Lemma (1).
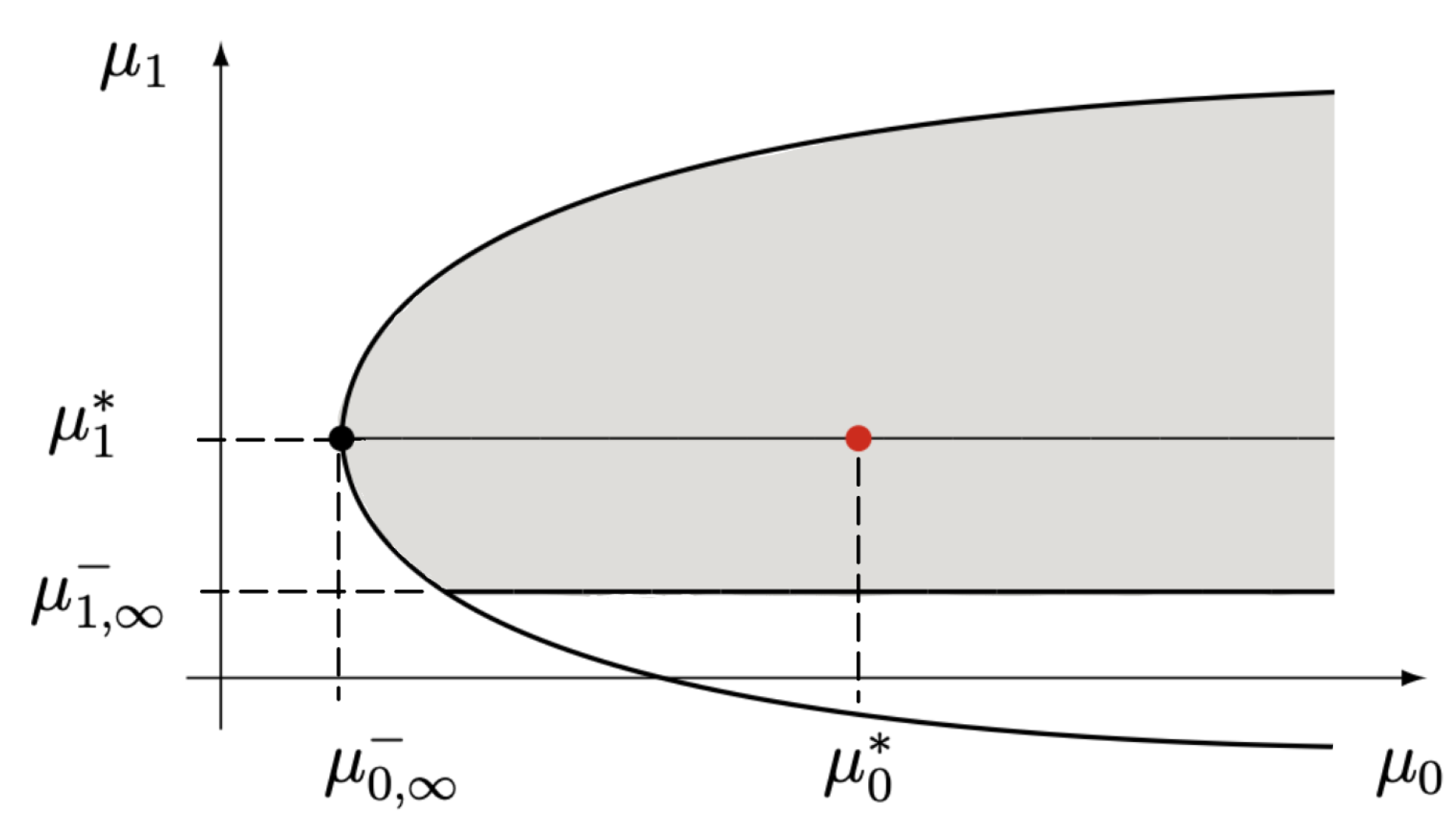
- Case 2:
- is S-indet with H-indet.There are infinitely many densities with support and . Combining Theorems 3 and 4, both inequalities and follow; equivalently, from Theorem 6, the point is inside the limit parabolic (see Figure 2).Entropy convergence in both cases S-indet and H-indet will be investigated below.
- Case 3.
- is S-det with H-det.For a given moment sequence, S-det means that there is only one measure with support compatible with it. Here, we take the opportunity to review and refine the proof previously given by [1], Section 3. Combining Theorems 3 and 4 and their geometric meaning, we find that there are four possible limit parabolic regions, all of which are feasible. More specifically, we have the following:
- (a)
- the two limit parabolic regions degenerate into different rays, both arising fromso that and fromrespectively. Hence, or occur;
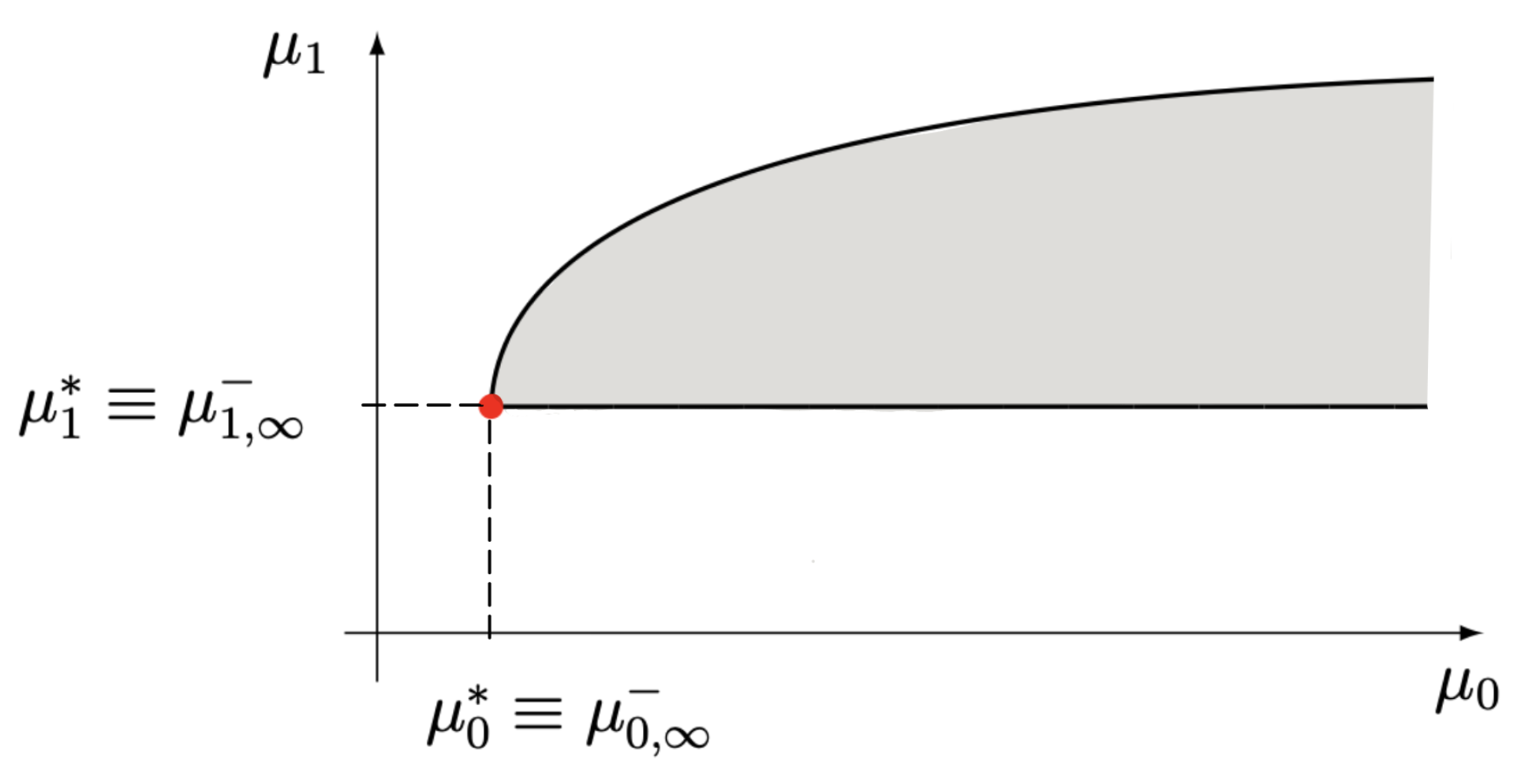
- (b)
- a non-degenerate limit parabolic region arising from Case 1. Accordingly, we have S-det with H-indet after removing the mass at the origin, so that both(see Figure 3). In agreement with the results for S-det attained in Case 1, the measure is once more purely discrete with no mass at the origin and then it has to be disregarded;
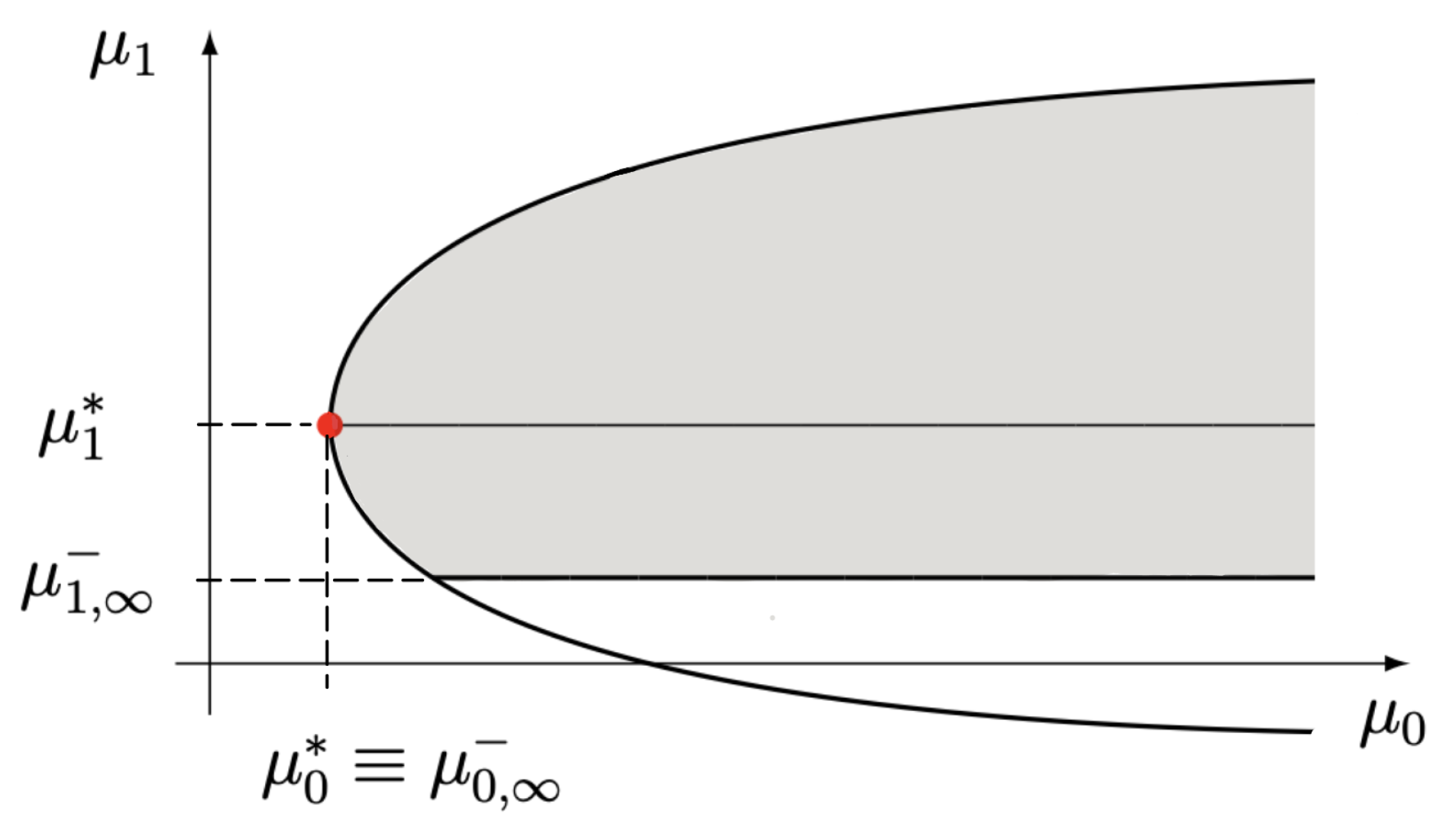
- (c)
- a non-degenerate limit parabolic region arising from the conditionsso that the corresponding distribution has zero mass at the origin. As a consequence and hold, so that the point is on the boundary of the limit parabolic region (see Figure 4).The nature (probability mass or density) of the unique solution can now be investigated by adding a mass at the origin and renormalizing. The moments sequence amounts to . In such a case, the moment problem becomes S-indet with H-indet (Figure 2), with maximum mass concentration at the origin. As is known, in an indeterminate Hamburger problem the maximum mass concentration at the origin just coincides with , so that the initial solution is transformed into an extremal solution of the S-indet with H-indet problem. Since there exists only a discrete extremal solution of the moment problem for which this maximum is attained [8], Thm. 2.13, p. 60; [11], Corollary 7.17, p. 159), the original determinate Stieltjes solution is purely discrete with no mass at the origin, in accordance with with [18] p. 94. Thus, it has to be disregarded.
4.3. Convergence in Entropy of the Maxent Approximation in the S-Indet Case
- 1.
- Let be the unique measure with support and density f having the given moment sequence . Consider the finite set , with . If are kept fixed while varies continuously, the function is monotonic increasing and concave (to ensure the monotonicity of , if needed, the interval could be replaced with , leaving the procedure unchanged). As , so that corresponds to a purely discrete distribution with a finite number of mass points ([8], p. 5, Thm. 1.2). Then, as the differential entropy of a discrete distribution can be considered to be ([4], p 247-9), holds. On the other hand, as , holds. Then, since is a continuous function of , there exists a value of (say ) such that . As , from (and then too) it follows that . From here, , which proves the entropy convergence in the H-det case.
- 2.
- From Theorem 6, in the S-indet case, the point is an interior point of the limit parabolic region such that . In accordance with the above item in this list, is monotonic increasing over withAs we have , both scenarios are admissible as follows:
- (i)
- (strictly);
- (ii)
Following the procedure outlined in the first item of this list, no conclusion about entropy convergence can be drawn, so an alternative approach must be sought.
4.3.1. Densities Supported on
- (i)
- Consider a given moment set , the corresponding MaxEnt density having moments , , whereand the additional new moment setNow, let be the MaxEnt density constrained by (18) and let be the sequence of its moments in analogy with (17). The following are the reasons for introducing :
- 1.
- the densities and coincide, as satisfies the first moments too, so that has its Lagrange multipliers ;
- 2.
- gives rise to a measure , which is S-det, since it admits a moment-generating function (which is a sufficient condition to guarantee moment problem determinacy, the so-called Cramér criterion) for each . Indeed, from (14), as , , with ;
- 3.
- related to the sequence , there are the numbers , which are the unique solution of the equation , whether or , where in the matrix , is replaced with , as outlined in Section 2. Further, for each K holds. As K increases, the sequence is monotonic increasing since the moment sequence coincides with the one associated with . The latter density, as said previously, gives rise to a determinate moment problem so that, as , , in accordance with the conclusions drawn above from Case 3. in Section 4.2.
In conclusion, with finite and fixed M and arbitrary K, MaxEnt density constrained by fulfils the two following properties:That is, the distribution corresponding to has the same entropy as the distribution having density so that the supplementary K moments do not vary the entropy but, at the same time, they push towards as K increases, forcing the moment problem to be determinate. This is a crucial condition for the convergence in entropy. - (ii)
- From (19) with arbitrary K, the two sequences and fulfill the relationshipsince the moment sequence does not introduce additional information content. In conclusion, taking into account (19) and using the moment sequence , which directly stems from , we are led to the S-det case. Indeed, as , and the unique f (in the S-det case) and and the unique (in the S-indet case) share the same amount of information provided by the two different moment sequences and , respectively. From this it follows that and . Accordingly, from here on, the procedure is carried out in a manner more or less similar to that described by [1], Thm. 1, with the only difference being that f, , , and are here replaced with , , , and , respectively. Combining the previous arguments, we can state the followingThe introduction of the sequence (17), and therefore of in place of , has the twofold aim of (i) not altering the entropy of and (ii) permitting the relationship , forcing the moment problem to be determinate and bringing us back to the previously solved case S-det. Foe the previously formulated admissible scenarios (strictly) or , a precise answer is found.Hence, Theorem 7 is proved. □
4.3.2. Densities Supported on
- (a)
- the solutions associated with and those of each are in one–one correspondence;
- (b)
- if the density solves the problem, solves the one and vice versa. Specifically, to the densities and correspond the densities and , with the obvious equalities and ;
- (c)
- for any indeterminate Stieltjes problem, there is always a unique , so that the sequence can be the following:
- (i)
- an indeterminate Stieltjes moment sequence for each ;
- (ii)
- a determinate Stieltjes moment sequence (but, of course, with the H-indet problem) for , giving rise to the so-called Friedrichs’s solution;
- (iii)
- not a Stieltjes moment sequence for .
5. Conclusions
- an improvement of an earlier proof of convergence in entropy provided by the authors when the Stieltjes moment problem is determinate;
- the statement and proof of an analogous entropy convergence result in the case of an indeterminate Stieltjes moment problem.
- criteria for the determinacy/indeterminacy of the Stieltjes moment problem with entropic techniques;
- an analogous entropy convergence criterion concerning the case of the indeterminate Hamburger problem;
- a criterion for the determinacy/indeterminacy of the Hamburger moment problem involving entropic techniques.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Milev, M.; Tagliani, A. Convergence of finite moment approximations in Hamburger and Stieltjes problems. Stat. Prob. Lett. 2017, 120, 114–117. [Google Scholar] [CrossRef]
- Frontini, M.; Tagliani, A. Entropy-convergence in Stieltjes and Hamburger moment problem. Appl. Math. Comput. 1997, 88, 39–51. [Google Scholar] [CrossRef]
- Jaynes, E.T. Information theory and statistical mechanics. Phys. Rev. 1957, 106, 620. [Google Scholar] [CrossRef]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory; Wiley-Interscience: Hoboken, NJ, USA, 2006. [Google Scholar]
- Novi Inverardi, P.L.; Tagliani, A. Probability Distributions Approximation via Fractional Moments and Maximum Entropy: Theoretical and Computational Aspects. Axioms 2023, 13, 28. [Google Scholar] [CrossRef]
- Stoyanov, J. Stieltjes Classes for Moment-Indeterminate Probability Distributions. J. Appl. Prob. 2004, 41, 281–294. [Google Scholar] [CrossRef]
- López-García, M. Krein condition and the Hilbert transform. Electron. Commun. Probab. 2020, 25, 71. [Google Scholar]
- Shohat, J.A.; Tamarkin, J.D. The Problem of Moments, Reprint of the Original 1943 Edition; Mathematical Surveys; American Mathematical Society: Providence, RI, USA, 1970; Volume 1. [Google Scholar]
- Fréchet, M.; Shohat, J.A. A Proof of the Generalized Second-Limit Theorem in the Theory of Probability. Trans. Am. Math. Soc. 1931, 33, 533–543. [Google Scholar] [CrossRef]
- Akhiezer, N.I. The Classical Moment Problem and Some Related Questions in Analysis; Oliver and Boyd: Edinburgh, UK, 1965. [Google Scholar]
- Schmüdgen, K. The Moment Problem; Graduate Texts in Mathematics; Springer: Berlin/Heidelberg, Germany, 2017; Volume 277. [Google Scholar]
- Olteanu, O. Symmetry and asymmetry in moment, functional equations and optimization problems. Symmetry 2023, 15, 1471. [Google Scholar] [CrossRef]
- Stoyanov, J. Krein condition in probabilistic moment problems. Bernoulli 2000, 6, 939–949. [Google Scholar] [CrossRef]
- Berg, C.; Christensen, J.P.R. Density questions in the classical theory of moments. Ann. l’Institut Fourier 1981, 31, 99–114. [Google Scholar] [CrossRef]
- Merkes, E.P.; Wetzel, M. A geometric characterization of indeterminate moment sequences. Pacific J. Math. 1976, 65, 409–419. [Google Scholar] [CrossRef][Green Version]
- Kesavan, H.K.; Kapur, J.N. Entropy Optimization Principles with Applications; Academic Press: London, UK, 1992. [Google Scholar]
- Chihara, T.S. On Indeterminate Hamburger Moment Problems. Pacific J. Math. 1968, 27, 475–484. [Google Scholar] [CrossRef]
- Heyde, C.C. Some remarks on the moment problem (I). Quart. J. Math. 1963, 14, 91–96. [Google Scholar] [CrossRef]
- Simon, B. The Classical Moment Problem as a Self-Adjoint Finite Difference Operator. Adv. Math. 1998, 137, 82–203. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Novi Inverardi, P.L.; Tagliani, A. Indeterminate Stieltjes Moment Problem: Entropy Convergence. Symmetry 2024, 16, 313. https://doi.org/10.3390/sym16030313
Novi Inverardi PL, Tagliani A. Indeterminate Stieltjes Moment Problem: Entropy Convergence. Symmetry. 2024; 16(3):313. https://doi.org/10.3390/sym16030313
Chicago/Turabian StyleNovi Inverardi, Pier Luigi, and Aldo Tagliani. 2024. "Indeterminate Stieltjes Moment Problem: Entropy Convergence" Symmetry 16, no. 3: 313. https://doi.org/10.3390/sym16030313
APA StyleNovi Inverardi, P. L., & Tagliani, A. (2024). Indeterminate Stieltjes Moment Problem: Entropy Convergence. Symmetry, 16(3), 313. https://doi.org/10.3390/sym16030313





