Nonlinear Dynamic Analysis of a Curved Sandwich Beam with a Time-Dependent Viscoelastic Core Using the Generalized Differential Quadrature Method (GDQM)
Abstract
1. Introduction
2. Theory and Formulations
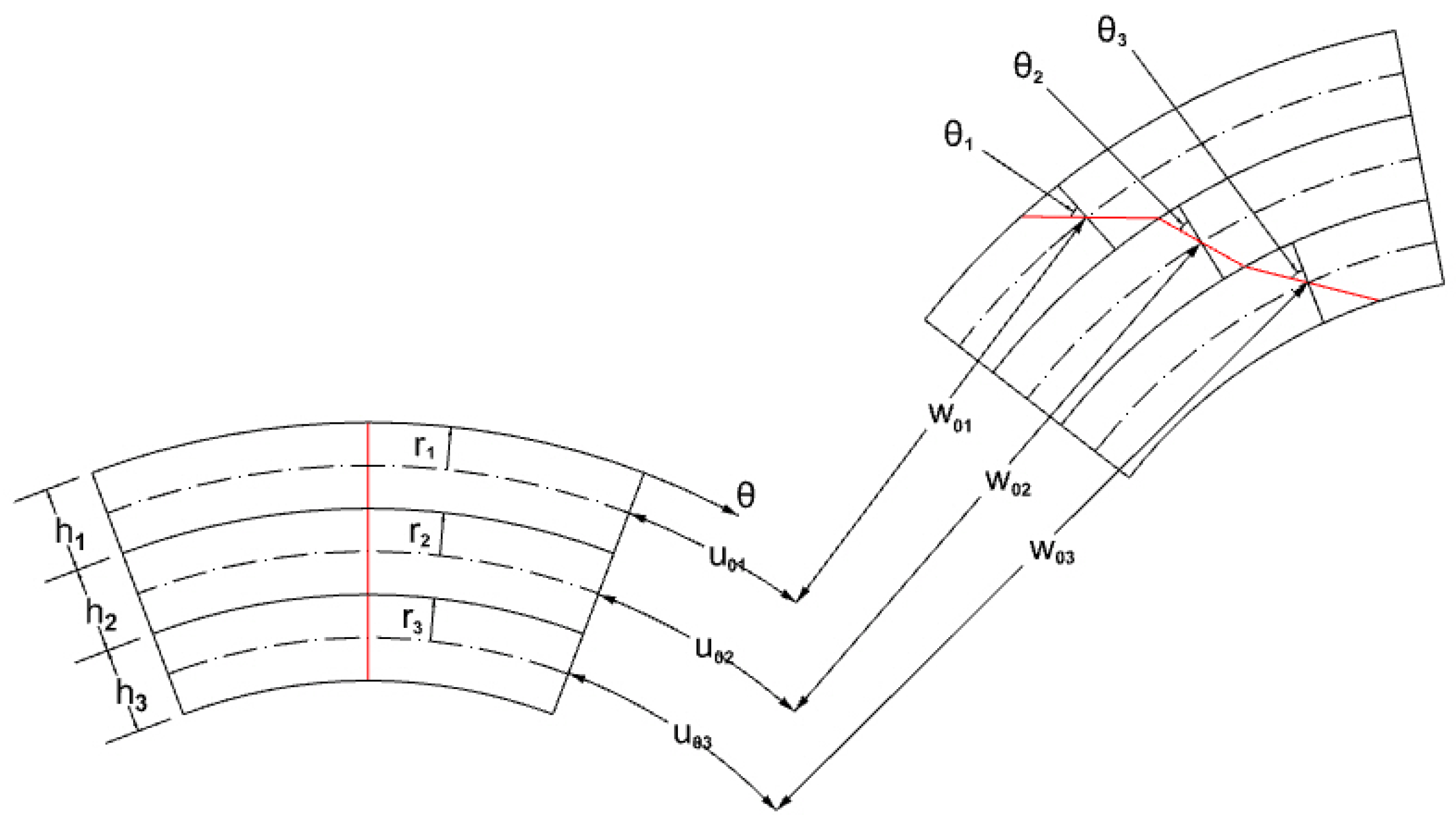
2.1. Kinematics, Constitutive, and Equilibrium Equations
- The connection between layers is simply perfect, so delamination does not occur.
- First-order shear deformation theory is used.
- Face layers are assumed to be incompressible, unlike the core layer.
- Since the deformations are not small, Von Karman-type nonlinear strain terms are included.
2.2. Generalized Differential Quadrature Method
2.3. Time Integration, Linearization of the Equation of Motion, and Viscoelasticity
2.3.1. Newmark-Beta Method and Newton–Raphson Method with GDQM
2.3.2. Viscoelasticity
3. Validation Studies
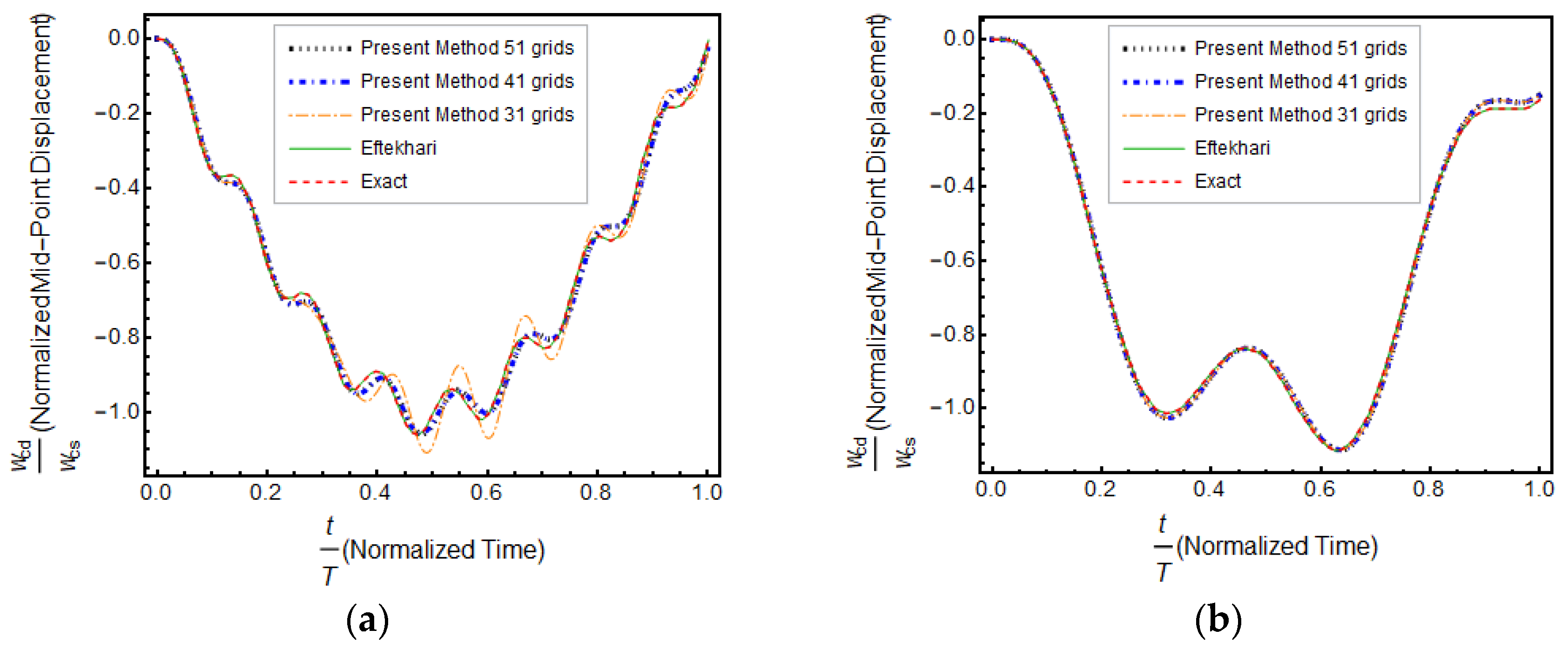
3.1. Example 1
3.2. Example 2
3.3. Example 3
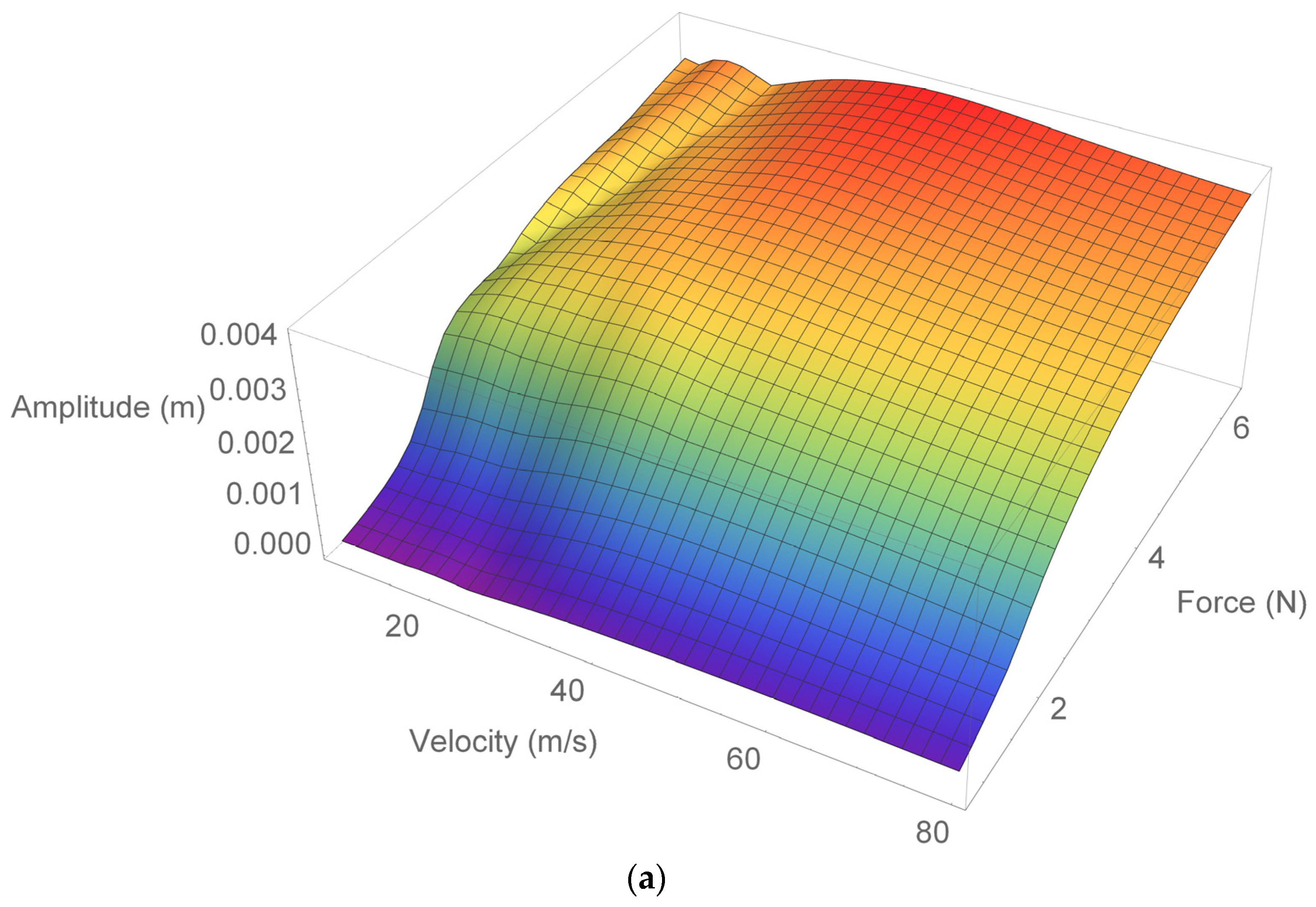
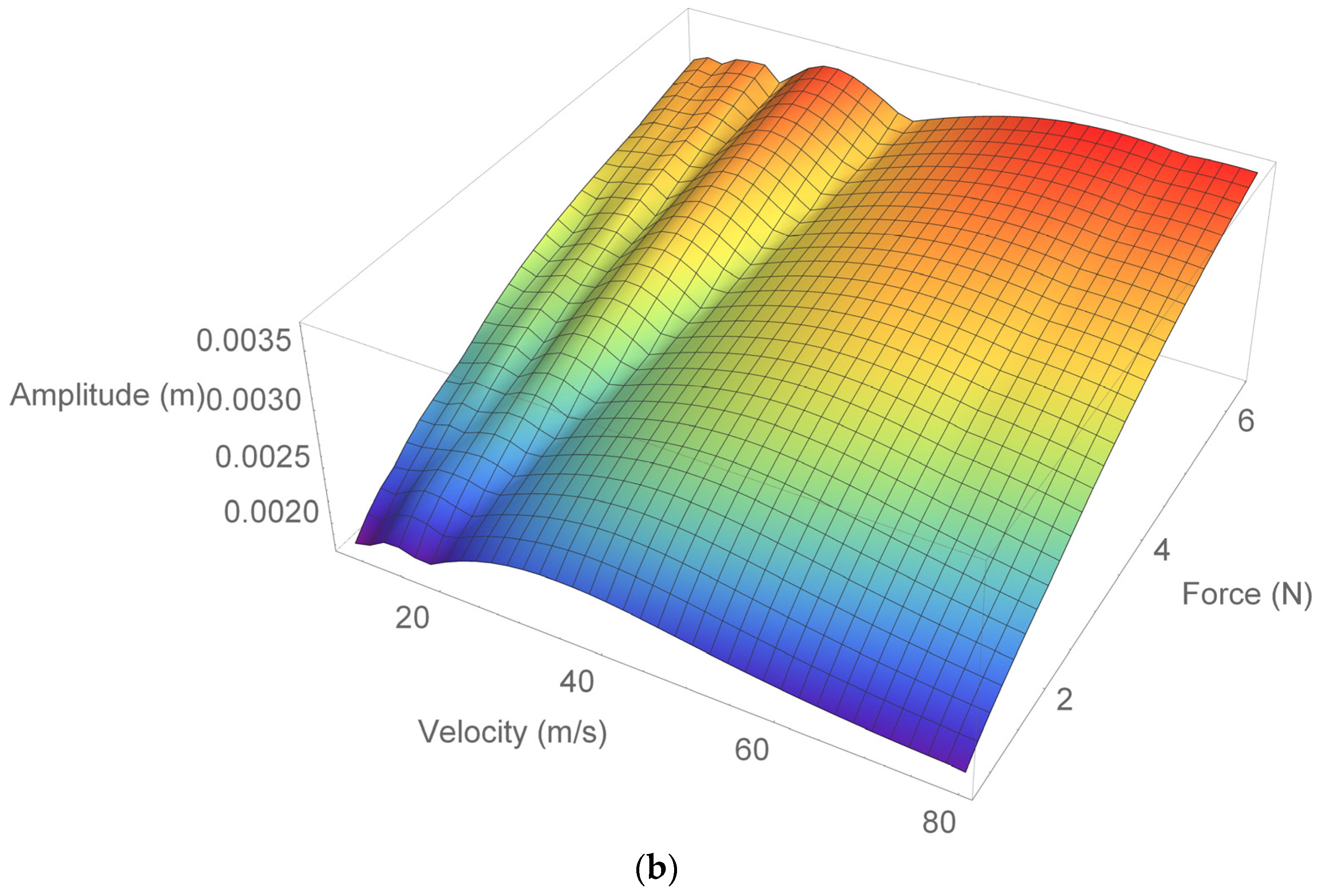
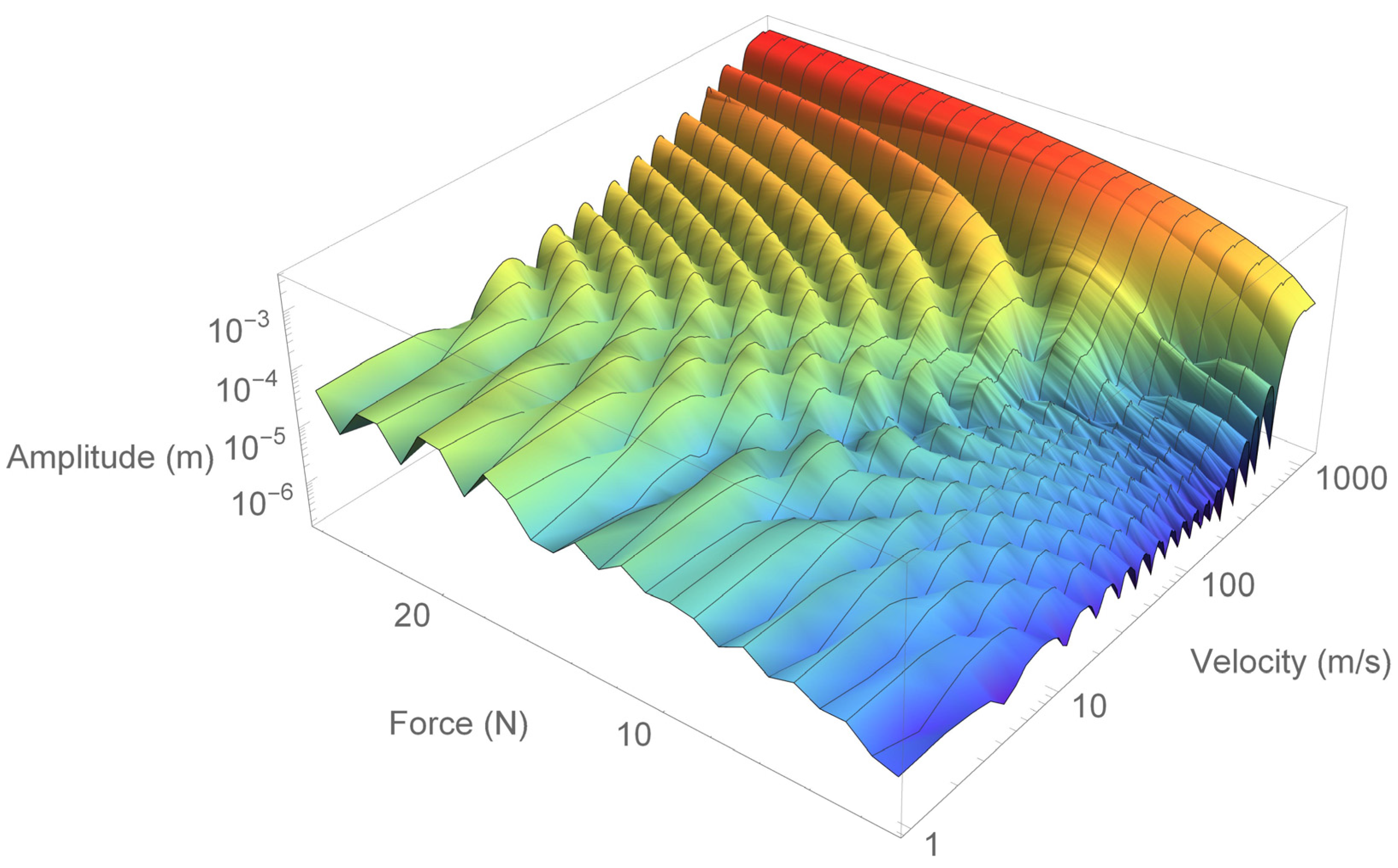
4. Case Studies
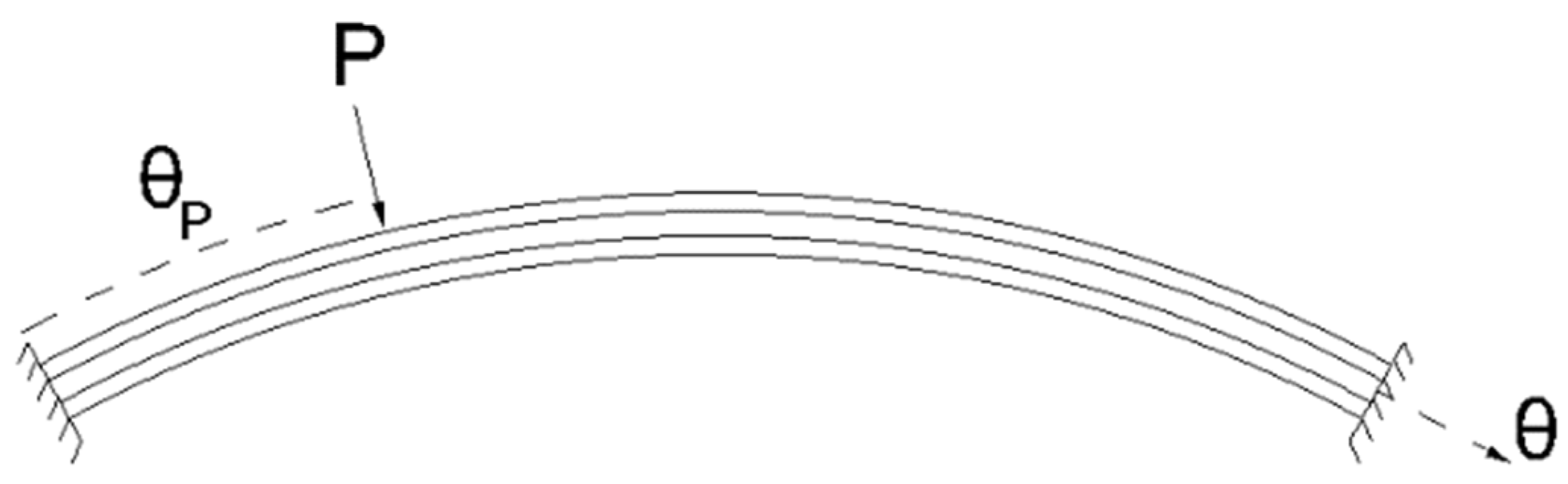
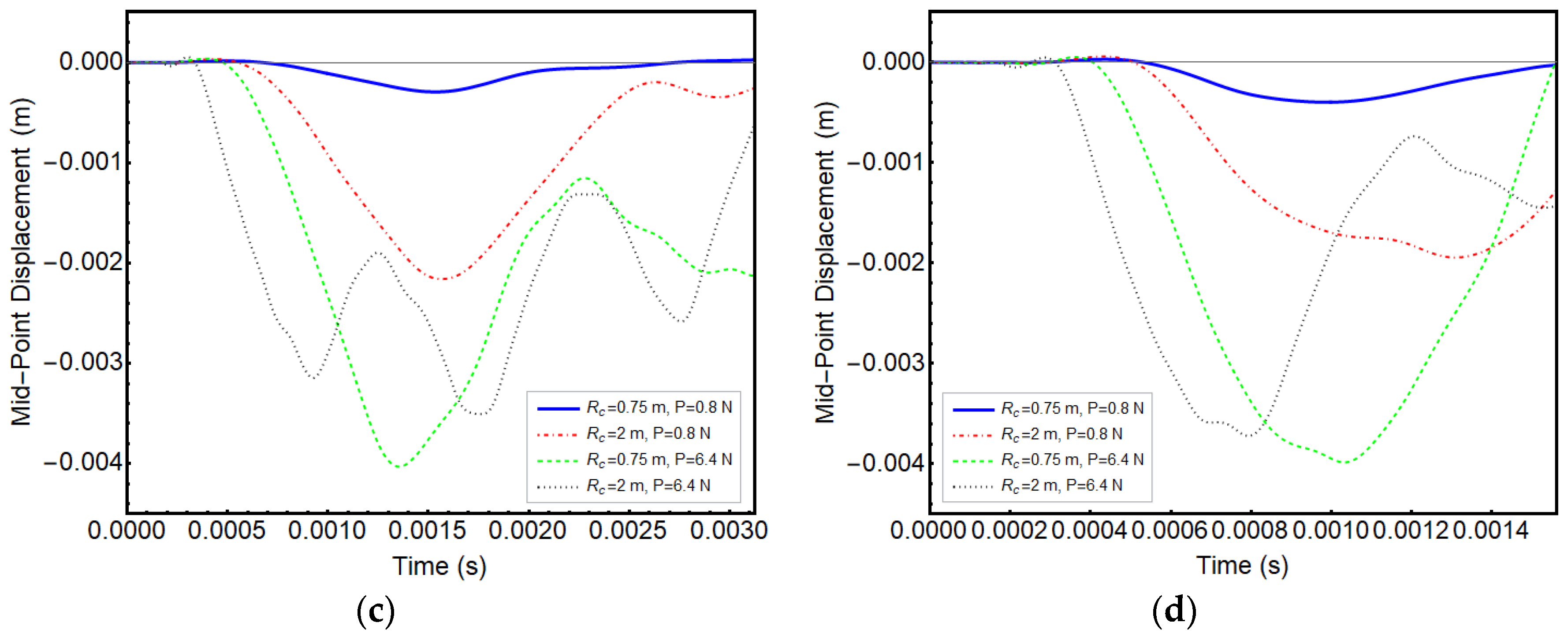
4.1. Sandwich Beam Subjected to Moving Load (Forced Vibration)
4.2. Sandwich Beam Subjected to Moving Load (Free Vibration)
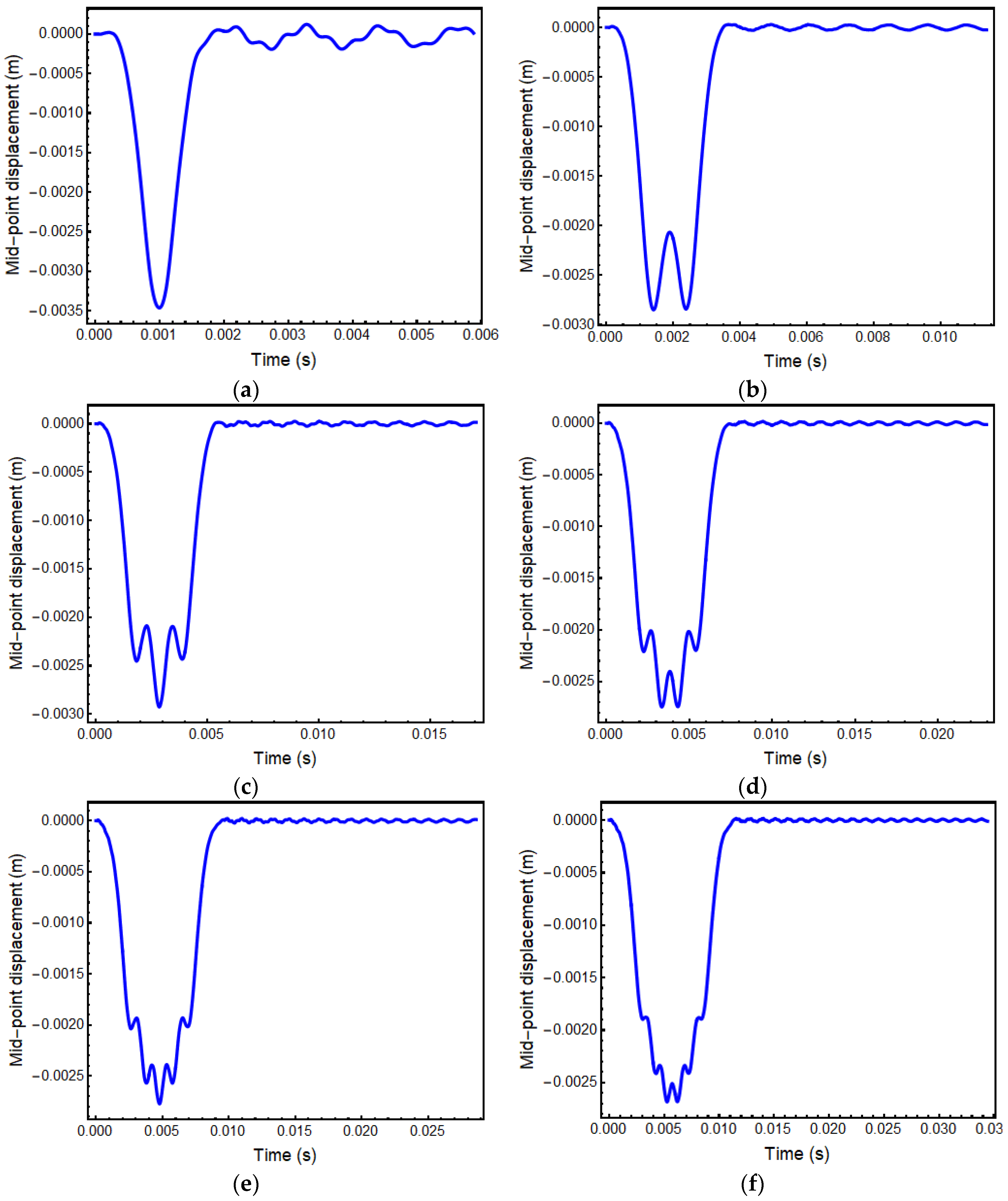
4.3. Sandwich Beam Subjected to Multiple Moving Loads (Forced Vibration)
5. Conclusions
- Increasing the radius of curvature does not cause an increase in mid-point displacement for all cases within the forced vibration.
- Resonance and cancellation velocity values change with the load amplitude due to the nonlinear effects.
- The load travels with the nth cancellation velocity, causing n number of oscillations during the forced vibration, in which the time history of the mid-point is symmetrical.
- The resonance velocity for the beam is not the same for the free vibration condition after leaving a single moving load and the forced vibration condition with multiple moving loads.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Singh, G.; Sharma, A.K.; Venkateswara Rao, G. Large-amplitude free vibrations of beams—A discussion on various formulations and assumptions. J. Sound Vib. 1990, 142, 77–85. [Google Scholar] [CrossRef]
- Gafsi, W.; Najar, F.; Choura, S.; El-Borgi, S. Confinement of Vibrations in Variable-Geometry Nonlinear Flexible Beam. Shock Vib. 2014, 2014, 687340. [Google Scholar] [CrossRef]
- Bert, C.W.; Malik, M. Differential Quadrature Method in Computational Mechanics: A Review. Appl. Mech. Rev. 1996, 49, 1–28. [Google Scholar] [CrossRef]
- Jang, S.K.; Bert, C.W.; Striz, A.G. Application of differential quadrature to static analysis of structural components. Int. J. Numer. Meth. Eng. 1989, 28, 561–577. [Google Scholar] [CrossRef]
- Feng, Y.; Bert, C.W. Application of the quadrature method to flexural vibration analysis of a geometrically nonlinear beam. Nonlinear Dyn. 1992, 3, 13–18. [Google Scholar] [CrossRef]
- Karami, G.; Malekzadeh, P. A new differential quadrature methodology for beam analysis and the associated differential quadrature element method. Comput. Methods Appl. Mech. Eng. 2002, 191, 3509–3526. [Google Scholar] [CrossRef]
- Zhong, H.; Guo, Q. Nonlinear Vibration Analysis of Timoshenko Beams Using the Differential Quadrature Method. Nonlinear Dyn. 2003, 32, 223–234. [Google Scholar] [CrossRef]
- Ghasemi, A.R.; Mohandes, M. The effect of finite strain on the nonlinear free vibration of a unidirectional composite Timoshenko beam using GDQM. Adv. Aircr. Spacecr. Sci. 2016, 3, 379–397. [Google Scholar] [CrossRef]
- Mahmoud, A.A.; Esmaeel, R.A.; Nassar, M.M. Application of the generalized differential quadrature method to the free vibrations of delaminated beam plates. Eng. Mech. 2007, 14, 431–441. [Google Scholar]
- Eftekhari, S.A. A Differential Quadrature Procedure with Regularization of the Dirac-delta Function for Numerical Solution of Moving Load Problem. Lat. Am. J. Solids Struct. 2015, 12, 1241–1265. [Google Scholar] [CrossRef]
- Eftekhari, S.A. A simple and accurate mixed Ritz-DQM formulation for free vibration of rectangular plates involving free corners. Ain Shams Eng. J. 2016, 7, 777–790. [Google Scholar] [CrossRef][Green Version]
- Eftekhari, S.A. A simple and systematic approach for implementing boundary conditions in the differential quadrature free and forced vibration analysis of beams and rectangular plates. J. Solid Mech. 2015, 7.4, 7.4,374–399. [Google Scholar]
- Eftekhari, S.A. A differential quadrature procedure for linear and nonlinear steady state vibrations of infinite beams traversed by a moving point load. Meccanica 2016, 51, 2417–2434. [Google Scholar] [CrossRef]
- Yang, Y.-B.; Wu, C.-M.; Yau, J.-D. Dynamic response of a horizontally curved beam subjected to vertical and horizontal moving loads. J. Sound Vib. 2001, 242, 519–537. [Google Scholar] [CrossRef]
- Hajianmaleki, M.; Qatu, M.S. Static and vibration analyses of thick, generally laminated deep curved beams with different boundary conditions. Compos. Part B Eng. 2012, 43, 1767–1775. [Google Scholar] [CrossRef]
- Poojary, J.; Roy, S.K. In-plane vibration of curved beams subjected to moving loads using finite element method. J. Phys. Conf. Ser. 2019, 1240, 012048. [Google Scholar] [CrossRef]
- Kurtaran, H. Large displacement static and transient analysis of functionally graded deep curved beams with generalized differential quadrature method. Compos. Struct. 2015, 131, 821–831. [Google Scholar] [CrossRef]
- Kurtaran, H. Geometrically nonlinear transient analysis of thick deep composite curved beams with generalized differential quadrature method. Compos. Struct. 2015, 128, 241–250. [Google Scholar] [CrossRef]
- Nikkhoo, A. Application of differential quadrature method to investigate dynamics of a curved beam structure acted upon by a moving concentrated load. Indian J. Sci. Technol. 2012, 5, 8. [Google Scholar] [CrossRef]
- Kang, K.; Bert, C.W.; Striz, A.G. Vibration Analysis of Horizontally Curved Beams with Warping Using DQM. J. Struct. Eng. 1996, 122, 657–662. [Google Scholar] [CrossRef]
- Akgün, G.; Kurtaran, H. Geometrically nonlinear transient analysis of laminated composite super-elliptic shell structures with generalized differential quadrature method. Int. J. Non-Linear Mech. 2018, 105, 221–241. [Google Scholar] [CrossRef]
- Malik, M.; Bert, C.W. Implementing multiple boundary conditions in the dq solution of higher-order pdes: Application to free vibration of plates. Int. J. Numer. Meth. Eng. 1996, 39, 1237–1258. [Google Scholar] [CrossRef]
- Fung, T.C. Stability and accuracy of differential quadrature method in solving dynamic problems. Comput. Methods Appl. Mech. Eng. 2002, 191, 1311–1331. [Google Scholar] [CrossRef]
- Bacciocchi, M.; Eisenberger, M.; Fantuzzi, N.; Tornabene, F.; Viola, E. Vibration analysis of variable thickness plates and shells by the Generalized Differential Quadrature method. Compos. Struct. 2016, 156, 218–237. [Google Scholar] [CrossRef]
- Soares da Costa Azevêdo, A.; Soares, A.S.C. Dynamic analysis of a viscoelastic Timoshenko beam. In Proceedings of the 24th ABCM International Congress of Mechanicl Engineering, ABCM, Curitiba, Brazil, 15 February 2017. [Google Scholar]
- Chen, W.-H.; Lin, T.-C. Dynamic analysis of viscoelastic structures using incremental finite element method. Eng. Struct. 1982, 4, 271–276. [Google Scholar] [CrossRef]
- Shafei, E.; Faroughi, S.; Rabczuk, T. Nonlinear transient vibration of viscoelastic plates: A NURBS-based isogeometric HSDT approach. Comput. Math. Appl. 2021, 84, 1–15. [Google Scholar] [CrossRef]
- Tolpekina, T.; Pyckhout-Hintzen, W.; Persson, B.N.J. Linear and Nonlinear Viscoelastic Modulus of Rubber. Lubricants 2019, 7, 22. [Google Scholar] [CrossRef]
- Arikoglu, A. A new fractional derivative model for linearly viscoelastic materials and parameter identification via genetic algorithms. Rheol. Acta 2014, 53, 219–233. [Google Scholar] [CrossRef]
- Demir, O.; Balkan, D.; Peker, R.C.; Metin, M.; Arikoglu, A. Vibration analysis of curved composite sandwich beams with viscoelastic core by using differential quadrature method. J. Sandw. Struct. Mater. 2020, 22, 743–770. [Google Scholar] [CrossRef]
- Arikoglu, A.; Ozkol, I. Vibration Analysis of Composite Sandwich Plates by the Generalized Differential Quadrature Method. AIAA J. 2012, 50, 620–630. [Google Scholar] [CrossRef]
- Taskin, M.; Arikoglu, A.; Demir, O. Vibration and Damping Analysis of Sandwich Cylindrical Shells by the GDQM. AIAA J. 2019, 57, 3040–3051. [Google Scholar] [CrossRef]
- Chopra, A.K. Dynamics of Structures: Theory and Applications to Earthquake Engineering; Prentice Hall: Upper Saddle River, NJ, USA, 2015; ISBN 978-0-273-77424-2. [Google Scholar]
- Wang, X. Differential Quadrature and Differential Quadrature Based Element Methods: Theory and Applications; Elsevier Science: Amsterdam, The Netherlands, 2015; ISBN 978-0-12-803081-3. [Google Scholar]
- Oller, S. Nonlinear Dynamics of Structures; Lecture Notes on Numerical Methods in Engineering and Sciences; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 978-3-319-05193-2. [Google Scholar]








| Length | Density | ||
| Width × Thickness | Dirac-delta Coefficient | 0.005 | |
| Young’s Modulus | Time Intervals | 200 |
| Length | Creep Decay Exponent | 15.7 | |
| Width × Thickness | Density | ||
| Radius of Curvature | ST Poisson’s Ratio | 0.25 | |
| ST Shear Modulus | Distributed Load | 0.01 | |
| LT Shear Modulus | Timestep | 4 × 10−5 |
| Beam Arc Length | Width × Thickness | ||
| Radius of Curvature | Timestep | 4 × 10−6 | |
| Young’s Modulus (Faces) | Density (Faces) | ||
| Poisson’s Ratio (Faces) | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Serveren, M.M.; Demir, O.; Arikoglu, A. Nonlinear Dynamic Analysis of a Curved Sandwich Beam with a Time-Dependent Viscoelastic Core Using the Generalized Differential Quadrature Method (GDQM). Symmetry 2024, 16, 238. https://doi.org/10.3390/sym16020238
Serveren MM, Demir O, Arikoglu A. Nonlinear Dynamic Analysis of a Curved Sandwich Beam with a Time-Dependent Viscoelastic Core Using the Generalized Differential Quadrature Method (GDQM). Symmetry. 2024; 16(2):238. https://doi.org/10.3390/sym16020238
Chicago/Turabian StyleServeren, Mehmet Mert, Ozgur Demir, and Aytac Arikoglu. 2024. "Nonlinear Dynamic Analysis of a Curved Sandwich Beam with a Time-Dependent Viscoelastic Core Using the Generalized Differential Quadrature Method (GDQM)" Symmetry 16, no. 2: 238. https://doi.org/10.3390/sym16020238
APA StyleServeren, M. M., Demir, O., & Arikoglu, A. (2024). Nonlinear Dynamic Analysis of a Curved Sandwich Beam with a Time-Dependent Viscoelastic Core Using the Generalized Differential Quadrature Method (GDQM). Symmetry, 16(2), 238. https://doi.org/10.3390/sym16020238





