1. Nuclear Clustering and Symmetries
Since the beginning of the introduction of the nuclear shell model, it has been known that symmetries play a fundamental role in nuclei [
1,
2]. These symmetries relate to the spin and orbital angular momentum of the nucleus and the approximate isospin symmetry, which involves neutrons and protons. However, there are other symmetries that involve how nucleons organize themselves inside nuclei, associated with the phenomenon of nuclear clustering [
3,
4,
5].
The nucleus is often depicted as a homogeneous distribution of protons and neutrons. However, details of the nucleon–nucleon interaction, mediated by the strong nuclear force, mean that the structure of the nucleus is far from this simple picture. At a macroscopic level, the impact of the strong interaction can be felt through the binding energies of nuclei. Binding energies provide a determination of the amount of energy required to separate the nucleons bound inside the nucleus to their free, unbound counterparts. As such, this quantity is a direct measure of the average force nucleons experience within the nucleus. This has been parameterized through the semi-empirical mass formula [
6], which has the following form:
where
N and
Z represent the numbers of neutrons and protons, and
A = N + Z. The five terms correspond to different features of a nucleus, as represented by a liquid drop of nuclear matter. In order, the terms correspond to a volume energy (
), associated with the interactions felt by the
A nucleons and accounting for the saturation of the nuclear force (i.e., nucleons only interact with those in close proximity). From this, correction terms associated with the deficit of nucleons at the surface (
, nucleons at the surface have ~50% fewer nucleons to interact with), the Coulomb repulsion of protons (
), and the imbalance of protons and neutrons (
) are subtracted, and then finally, a pairing term
is added.
The first three terms reveal nothing surprising in the nature of the strong and electromagnetic interaction; however, the last two do. There is an energy associated with the asymmetry in the numbers of protons and neutrons, with the term vanishing when . This indicates that a nucleus has the highest binding when neutrons and protons reside in identical quantum levels, or orbits. The last term is the pairing term, , which is positive when both neutron and proton numbers are even, zero when only one of N or Z are even, and negative when both are odd. This can be traced to pairs of protons/neutrons with their spins anti-aligned in counter-propagating orbits with their angular momentum, spin, and orbital angular momentum coupled to zero.
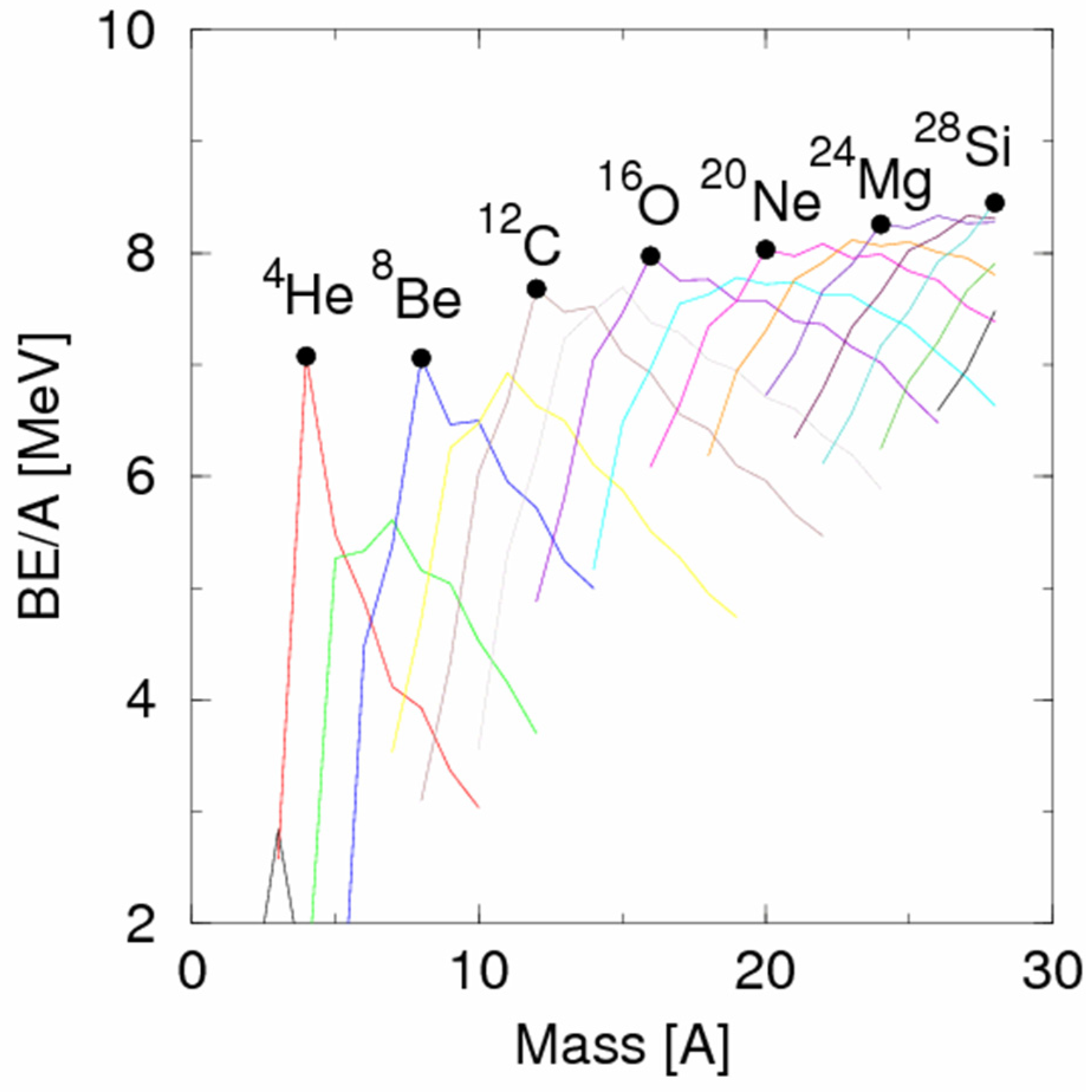
These details impact nuclear structure and impose both correlations in momentum and physical space, and are most noticeable in light nuclear systems where the energy associated with these correlations are of a similar scale to the volume energy. This is seen in
Figure 1, where nuclei with
N = Z and even proton and neutron numbers are most tightly bound, which is most obvious in the case of the
4He nucleus. In fact, the binding of
4He is so large that the
8Be nucleus finds itself unbound to decay into two
4He nuclei. This is a feature that is important in limiting the rate of helium burning in the red giant phase of stars and subsequently the rate of formation of
12C [
7]. As nuclei such as
8Be,
12C,
16O,… can be decomposed into α-particles,
4He nuclei subunits invite the idea that these nuclei might be described in terms of α-particle clusters.
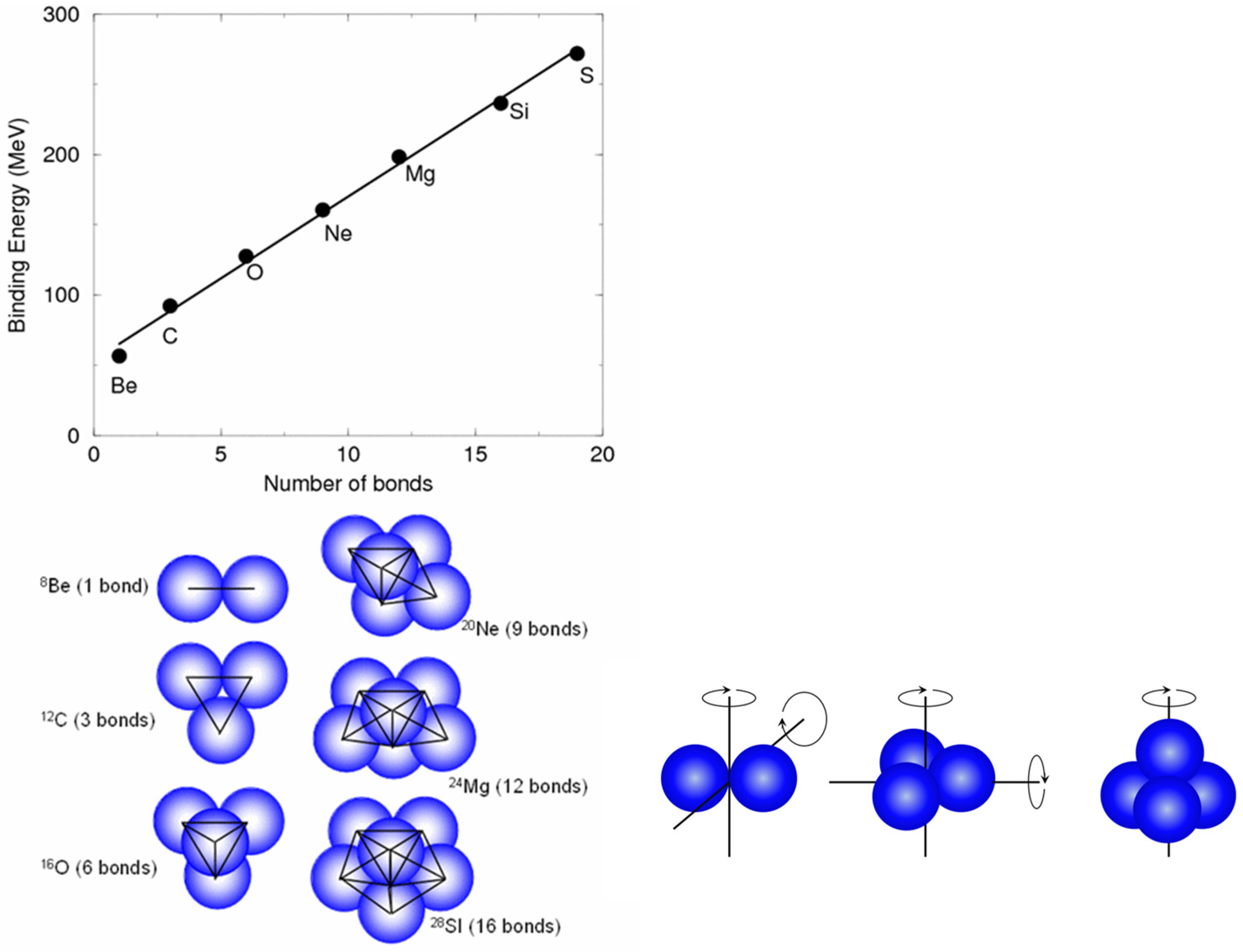
The question arises as to whether these clusters arise only in the momentum correlations associated with the manifestation of the nuclear strong interaction or if it is appropriate to consider these clusters to be spatial arrangements of α-particles. The first real glimpse of this was found in the study of Hafstadt and Teller [
8], as shown in
Figure 2. This illustrated how the binding energies could be understood in terms of the number of interactions between α-particles and an α–α interaction energy. This invites a view of these nuclei in which the α-particle structure plays a significant role and that the momentum correlations associated with the nucleon–nucleon interaction, in turn, induces spatial arrangements of the α-particles. The other important feature of the α-particle is the very high energy of the first excited state, which lies at ~20 MeV. Compared with other nuclei, this is very high and attests to the strength of the correlations and the inertness of the α-particle. As such, once formed within the nuclear environment, one can expect the α-particle to have a significant lifetime. The structures shown in
Figure 2 have particular symmetries that then invite an interpretation of their excited states in terms of collective rotations.
In the case of
8Be, there are two identical axes around which the rotations may occur, and the equation for the rotational energy is [
8]:
where
is the moment of inertia of two touching α-particles. This produces a set of quantum states
,… up to a maximum angular momentum that the system can sustain. In the case of the
8Be nucleus, there are four particles in p-orbitals (
l = 1); then, the maximum total angular momentum that can be generated is
For
12C, there are two different symmetry axes. The first has a three-fold rotational symmetry (perpendicular to the plane of the triangle) and the second has a two-fold symmetry (in the plane of the triangle). The second of these corresponds to a rotation of the two α-particles in the base of the triangle, i.e., the moment of inertia is given by
. This symmetry is designated
. The rotations around the three-fold symmetry axis are labelled by the quantum number
K, and
can take values of
… Collective rotations are labelled by the
and
J values and the rotational energy is given by [
8]:
For = , the rotations will be around an axis that lies in the plane of the three α-particles (in fact, passing through the center of one α- particle and between the other two), generating a series of states The in the denominator in the second term arises from the moment of a triangle of three touching spheres around the triangle center being approximately equal to twice the moment of inertia of two touching spheres. The next set of rotations are associated with the rotation around an axis perpendicular to the plane of the triangle, with each α-particle having one unit of angular momentum (, giving L = 3 × 1ℏ and =. Rotations around this axis and that parallel to the plane combine to give a series of states The next set of collective states then corresponds to each α-particle, with = 2ℏ and corresponding to L = 3 × 2ℏ.
For the tetrahedral arrangement of clusters in
16O, there is one common symmetry axis and the rotational energies are given by:
Here, the relevant symmetry is Td.
A more contemporary description of these symmetries is found in the algebraic cluster model, which accounts for both the rotational and vibrational symmetries of these nuclei and has been performed for the
12C and
16O systems [
10,
11]. These cluster symmetries assume that the α-particles are boson-like and that the internal structure can be neglected. However, one should recognize that the full wavefunction of the system needs to be fully antisymmetrized, given the fermionic nucleon components. This process of antisymmetrization precludes certain states that would appear otherwise, as demonstrated in Refs. [
12,
13].
Up to this point, the symmetries that arise from the point and rotational symmetries grow from an assumption of a crystalline arrangement of clusters. However, a successful description of nuclei should recognize that the individual nucleons move in a mean field formed by the average interaction that a nucleon experiences within the nucleus. This is typically represented by a Woods–Saxon potential with an additional spin–orbit interaction to give the nuclear shell model solutions of the Schrödinger equation. Here, the nucleons are then associated with standing wave solutions for the given potential. It is then not immediately obvious how these might map onto those realized from the α-particle geometric arrangements.
It is possible to realize analytic solutions of the deformed harmonic oscillator, which illustrate the connection. It is clear that the deformed harmonic oscillator potential is only an approximation to more realistic nuclear potentials, but that the conclusions reached in the following are robust and map to more complex nuclear models [
14]. The solutions of the deformed harmonic oscillator are given by:
where
are the characteristic angular frequencies in the three Cartesian coordinate directions, and
are the associated oscillator quanta. Here,
and for axial symmetry
, the nuclear/oscillator deformation parameter is then given by:
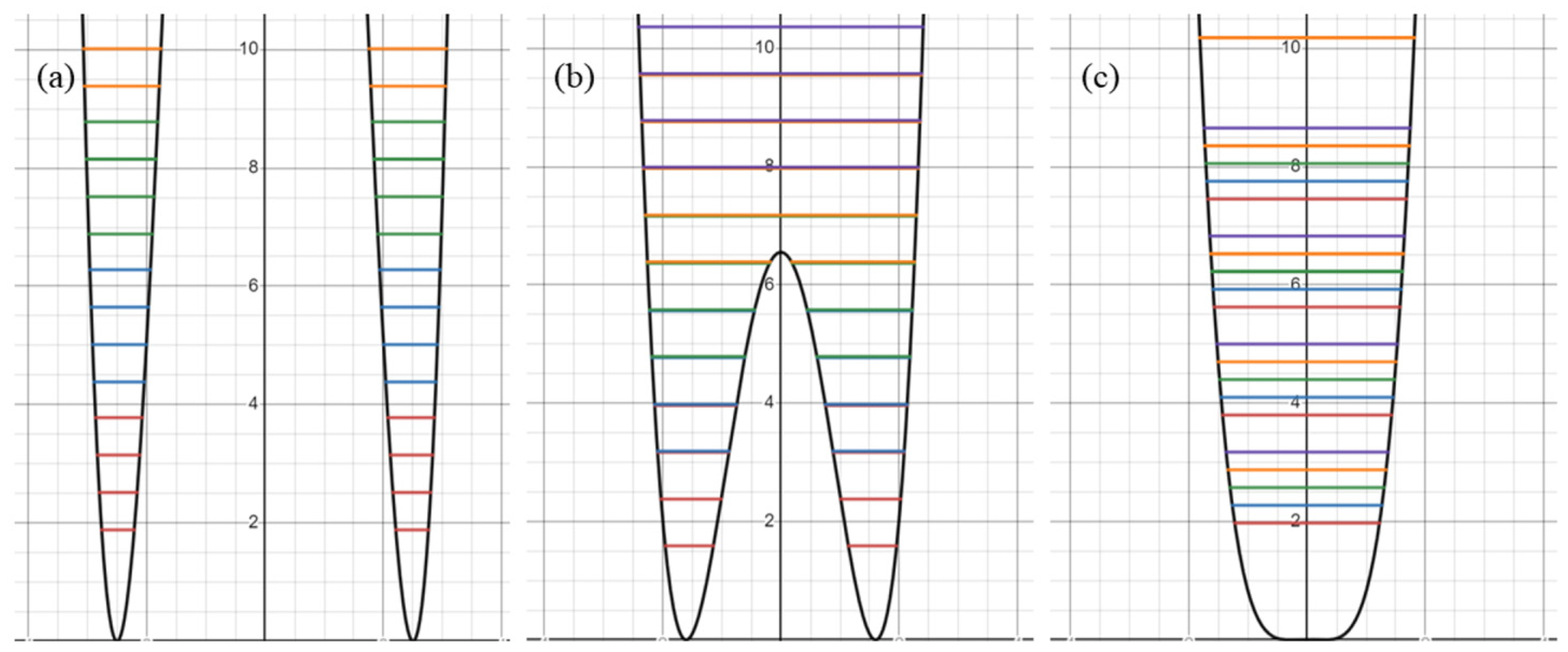
The energy levels of the deformed harmonic oscillator are illustrated in
Figure 3. In nuclei, the presence of a shell structure, or equivalent regions of high-density states, is associated with stability. For example, spherical nuclei such as
4He,
16O, and
40Ca have high binding energies and comparatively high excitation energies for the first excited state. These are associated with magic proton and neutron numbers 2, 8, and 20.
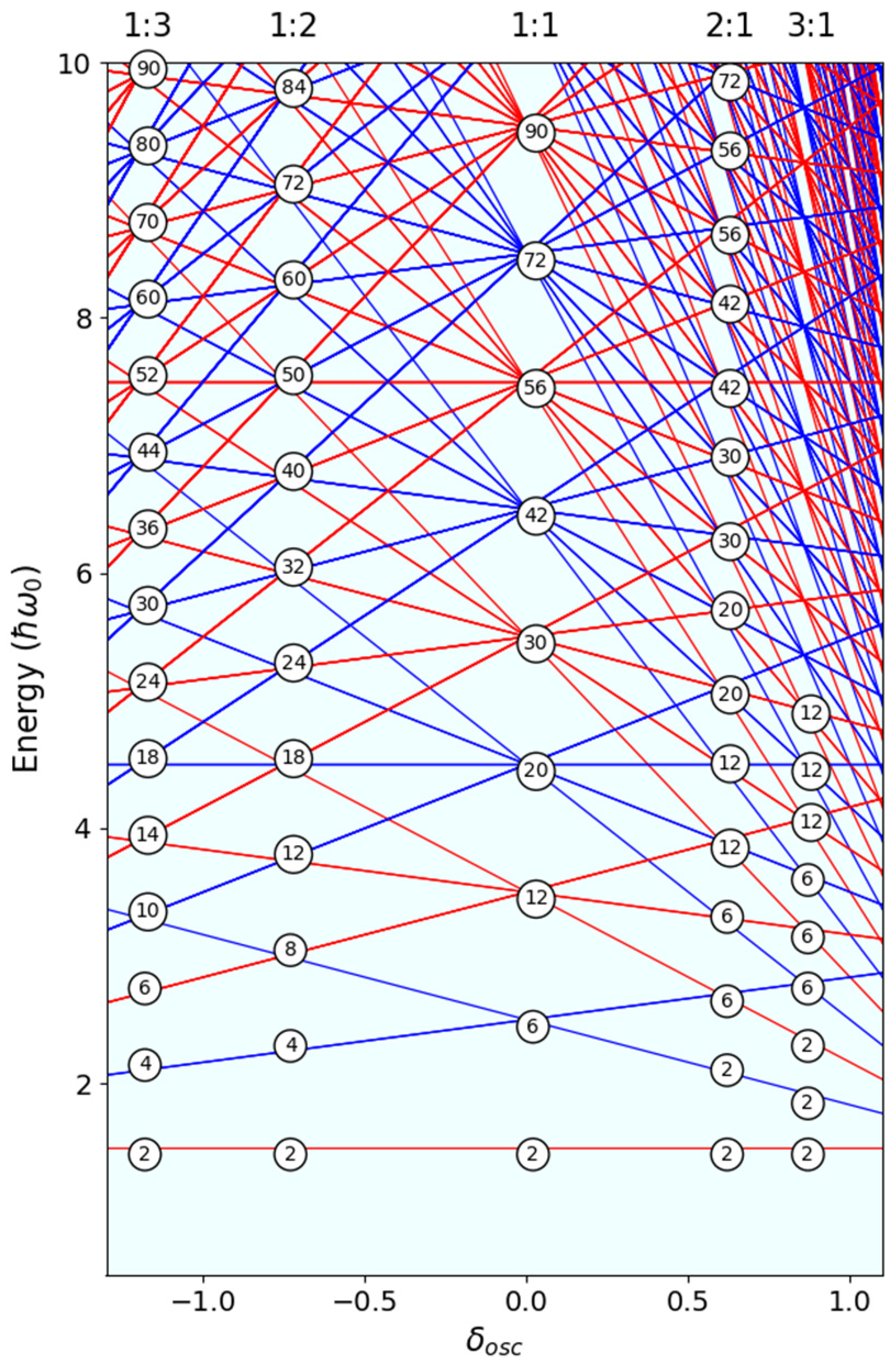
Figure 3 illustrates that at high levels of degeneracy, shell gaps appear in the deformed energy level scheme at both prolate
and oblate
deformations associated with integer ratios of axial deformation parameters/frequencies. This produces new sets of magic numbers particular to that deformation. As demonstrated in Ref. [
15], these new magic numbers can be represented by a single sequence of numbers, 2, 6, 12, 20, 30,…, as illustrated in
Figure 4.
This sequence is that found for the spherical solutions; 1:1 is found to be repeated at each deformation of the system with a slightly different set of systematics either side of sphericity. For n:1 prolate deformations, the sequence of numbers is stacked such that all of the 2s appear first, then the 6s, etc., …, whereas on the oblate side at a deformation of 1:n, the sequences linked to the prolate n:1 deformations are repeated n times. There is thus an underlying symmetry that can be traced back to the spherical harmonic oscillator.
The interpretation of this symmetry is as follows: For a 2:1 prolate deformation, the shell structure and degeneracy is represented by filling two harmonic oscillators, and at
n:1, there are
n harmonic oscillators. Crucially, from a geometric perspective, these oscillators would all be aligned along the deformation axis. Thus, from a cluster perspective, the interpretation would be
n clusters aligned along a common axis. In its simplest form, this would reproduce the α+α clustering associated with
8Be. For the 1:2 oblate deformation, it is seen that the sequence of degeneracies is created by combining two 2:1 prolate sequences, but with one offset by 1
. As discussed in Ref. [
15], this sequence can reproduce the structure of oblate, deformed, clustered nuclei such as
12C and
28Si and the associated
D3h structure of
12C. The
Td structure of
16O corresponds to the spherical 1:1 deformation with an α-particle stacked on top of the 3α, triangular,
D3h structure of
12C.
The assembly of the individual clusters into the composite system, such that the resulting structures respect the Pauli exclusion principle, can be described by the Harvey model [
16]. The methodology extending from 2 to
n centers is explained in Ref. [
17]. The Harvey model is a method for combining the oscillator quanta from a multi-center system into a single center. It ensures that the Pauli exclusion principle is observed when levels from multi-centers are combined. For a two center system with levels labelled by
, merged along the
-axis, then the resulting levels are associated by the
quantum numbers 2
and 2
. Extending to
N centers, the merged levels have
quantum numbers
N,
, …
N These principles preserve the number of internal nodes of the wavefunctions being combined in merging from
N centers to one center.
In summary, both from a perspective of considering light, alpha-conjugate nuclei to be constructed from arrangements of α-particles arranged into geometric structures and that of solutions of the Schrödinger equation associated with the deformed harmonic oscillator, clustering is evident in the structure of light nuclei. These geometric structures have characteristic point symmetries that then, in turn, are associated with collective rotational behavior, which appear as rotational bands. These observations tally well with the available experimental evidence, e.g., as demonstrated in the case of the rotations of
12C [
10].
3. Results
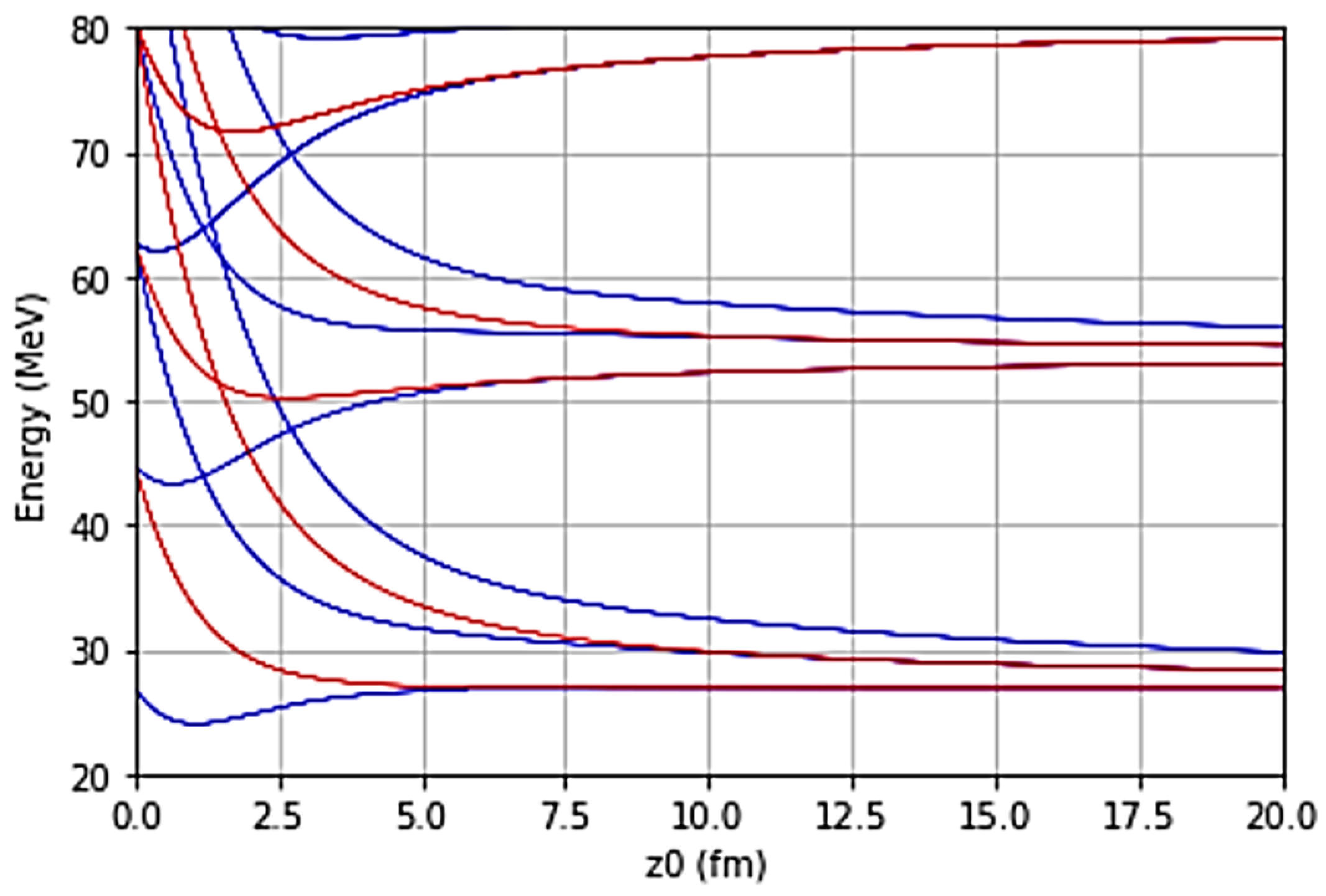
The solutions of the DCHO are shown in
Figure 6 and
Figure 7 for the
values and the associated energy levels. There are some simple rules.
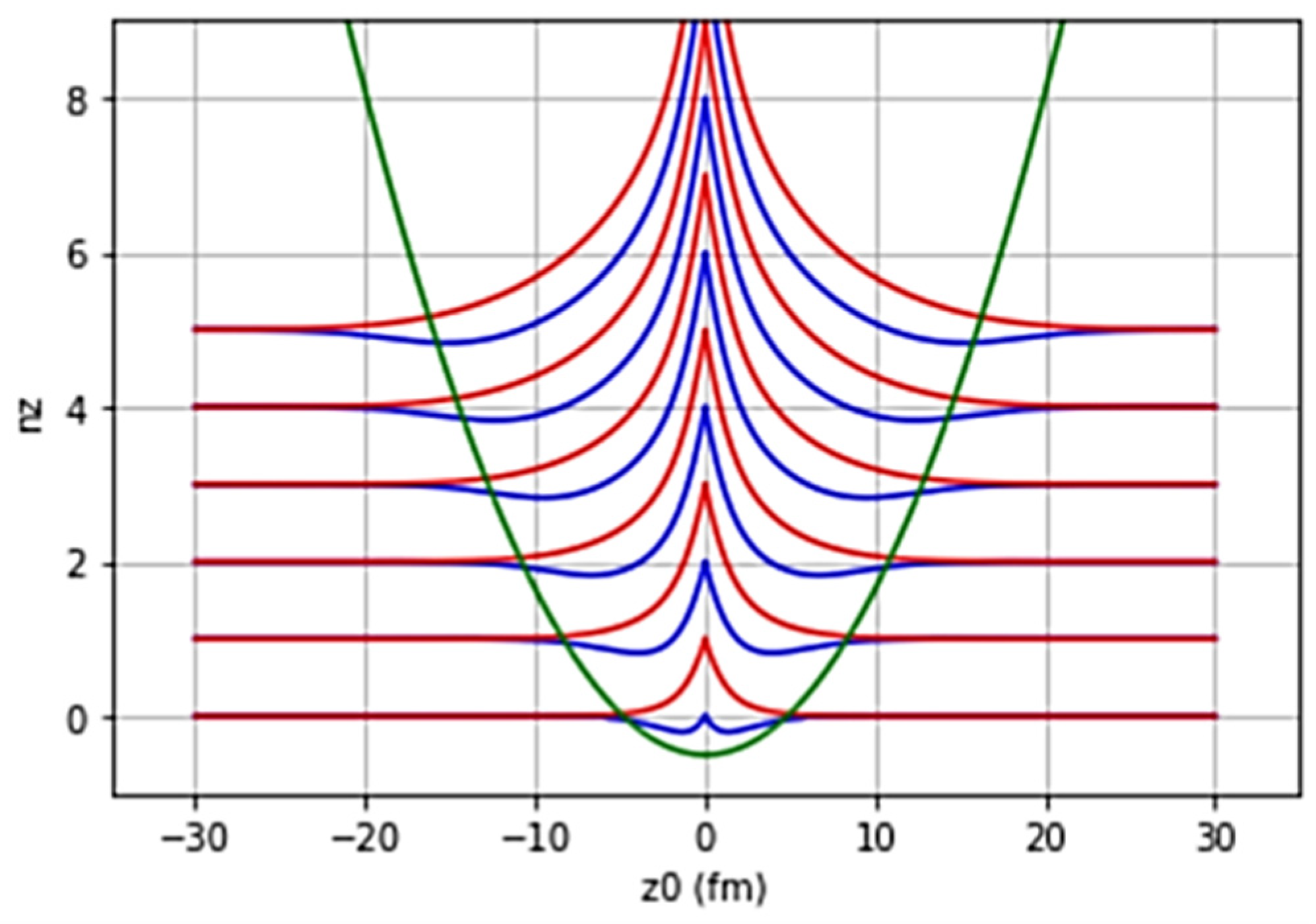
The solutions for the DCHO demonstrate some guiding principles of the evolution of two harmonic oscillator potentials as they evolve from infinite separation to zero separation. As is shown in
Figure 6, there is a change in the
values at infinite separation to
and
at zero separation. This evolution reflects the change in the wavefunctions such that the final single center wavefunction preserves the number of nodes in the system and introduces an additional node according to the linear combinations:
Thus, in
Figure 6, the merging of the potentials from the negative and positive
z-direction results in protons and neutrons either following the blue line or red line from either side, but not both blue and red together. The green line in
Figure 6 illustrates the approximate variation in the location at which levels in the separate HO potentials overlap and the system changes from two separate HO potentials to solutions of the double-center harmonic oscillator.
The rules of the DCHO are encoded in the Harvey rules [
16]. These are illustrated in
Figure 8 for the fusion of two
12C nuclei.
The
12C nucleus in its ground state can be represented by the HO configuration
(0,0,0)
4, (0,0,1)
4, and (0,1,0)
4, which is associated with a triangular structure orientated in the
y–z plane. Different orientations of the 3α structure can be created by populating the (1,0,0), (0,1,0), and (0,0,1) levels with different pairs. As is shown in
Figure 8, when these nuclei merge, following solutions of the DCHO and as depicted by the Harvey scheme, different final
24Mg structures are produced. The interesting observation is that the final structure that is produced, as represented by the DHO densities, retains the symmetries of the original arrangements of the
12C clusters. For example, the left-hand side of
Figure 8 shows the merger of two
12C nuclei with all α-particles in the
y–z plane and this results in a
24Mg cluster structure in which 6α-particles are arranged, where two 3α triangles are clear. Different orientations give different cluster structures with the central example corresponding to an α +
16O + α structure and the right-hand image would be a compact
24Mg structure associated with an arrangement similar to the
24Mg ground state.
Another thing to note is that for different orientations, the circles that represent 2p+2n (α-particle) combinations, are promoted to orbits of different energies associated with the different orientations. In other words, the potential energy associated with different orientations is different, with that of the more compact structure being lowest and the planar structure being the highest (the α +
16O + α structure is intermediate). This can be thought of as a Pauli repulsion effect, which would add to the Coulomb repulsion associated with the like proton charges in the two
12C nuclei. Thus, the formation of the compact structure would proceed at lower energies and the planar structure at higher energies, and the difference in barriers would drive re-orientation of the
12C nuclei in the collision process. This has been demonstrated, for example, in coupled-reaction-channel calculations by Boztosun and Rae [
26].
The calculations in
Figure 8 are performed with the DHO in the limit of zero separation of the two
12C nuclei. However, using the DCHO and the two-center wavefunctions, it is possible to calculate the evolution of the densities from the point where the two potentials begin to merge, through the point of closest contact and the formation of the intermediate structure to the point where the two nuclei move apart. In these calculations there is full consideration of the incident kinetic energy and the repulsive effect of the Pauli repulsion as particles trace out orbitals that climb in energy and the repulsive effect of the Coulomb repulsion. This is shown in
Figure 9. Here, it is assumed that in the DCHO calculations, the line joining the two
12C nuclei remains as the
z-axis.
It is observed in these calculations that the 3α structure of 12C and the 6α structure of 24Mg is preserved, and the symmetries described earlier in this paper not only affect the static properties of nuclei, but also the dynamical ones as well.