Modeling Pollutant Diffusion in the Ground Using Conformable Fractional Derivative in Spherical Coordinates with Complete Symmetry
Abstract
1. Introduction
2. Materials and Methods
2.1. Diffusion Equation in Spherical Coordinates with Conformal Fractional Time-Derivative
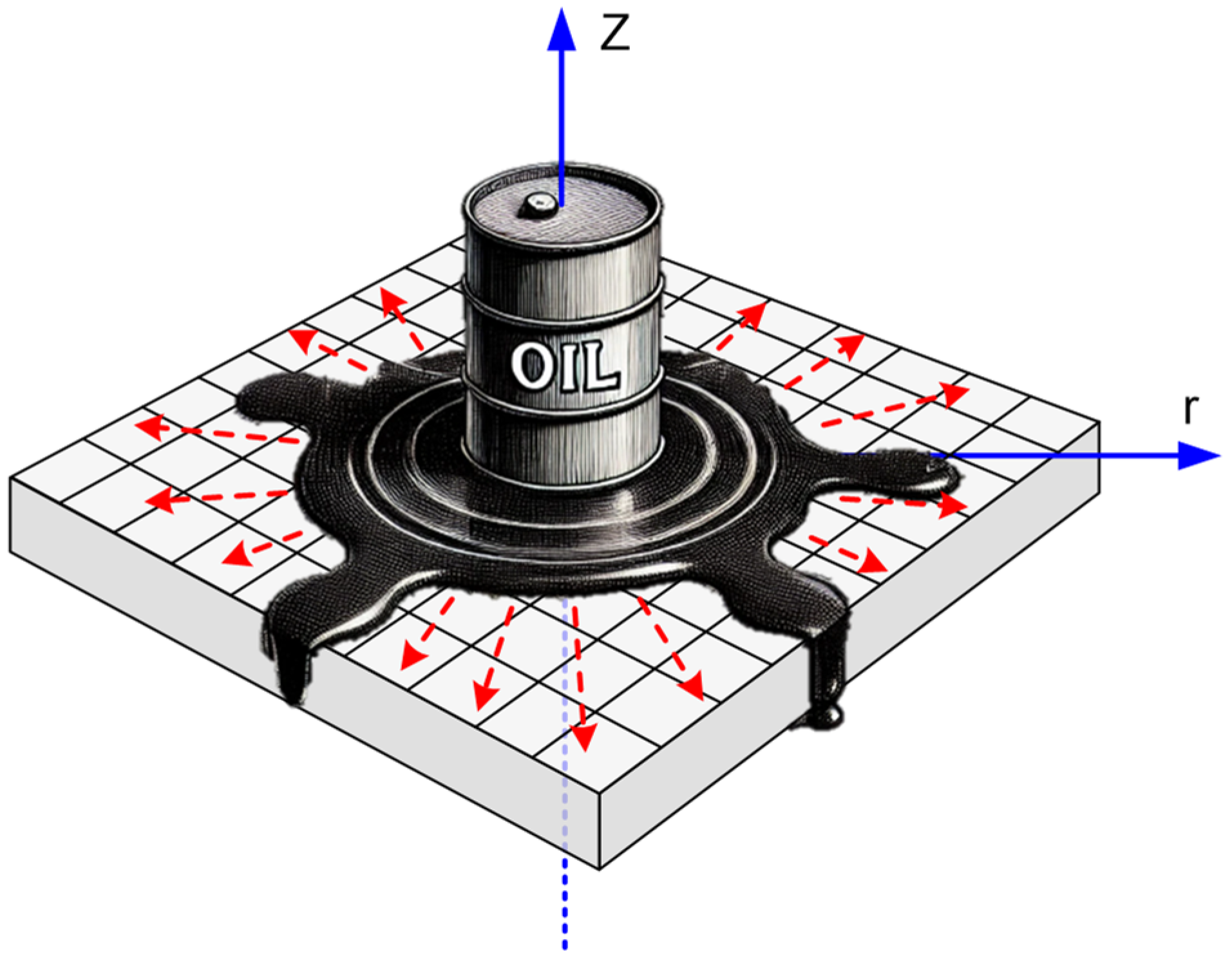
2.2. Diffusion of the Spilled Pollutant Problem and Derivation of Its Solution
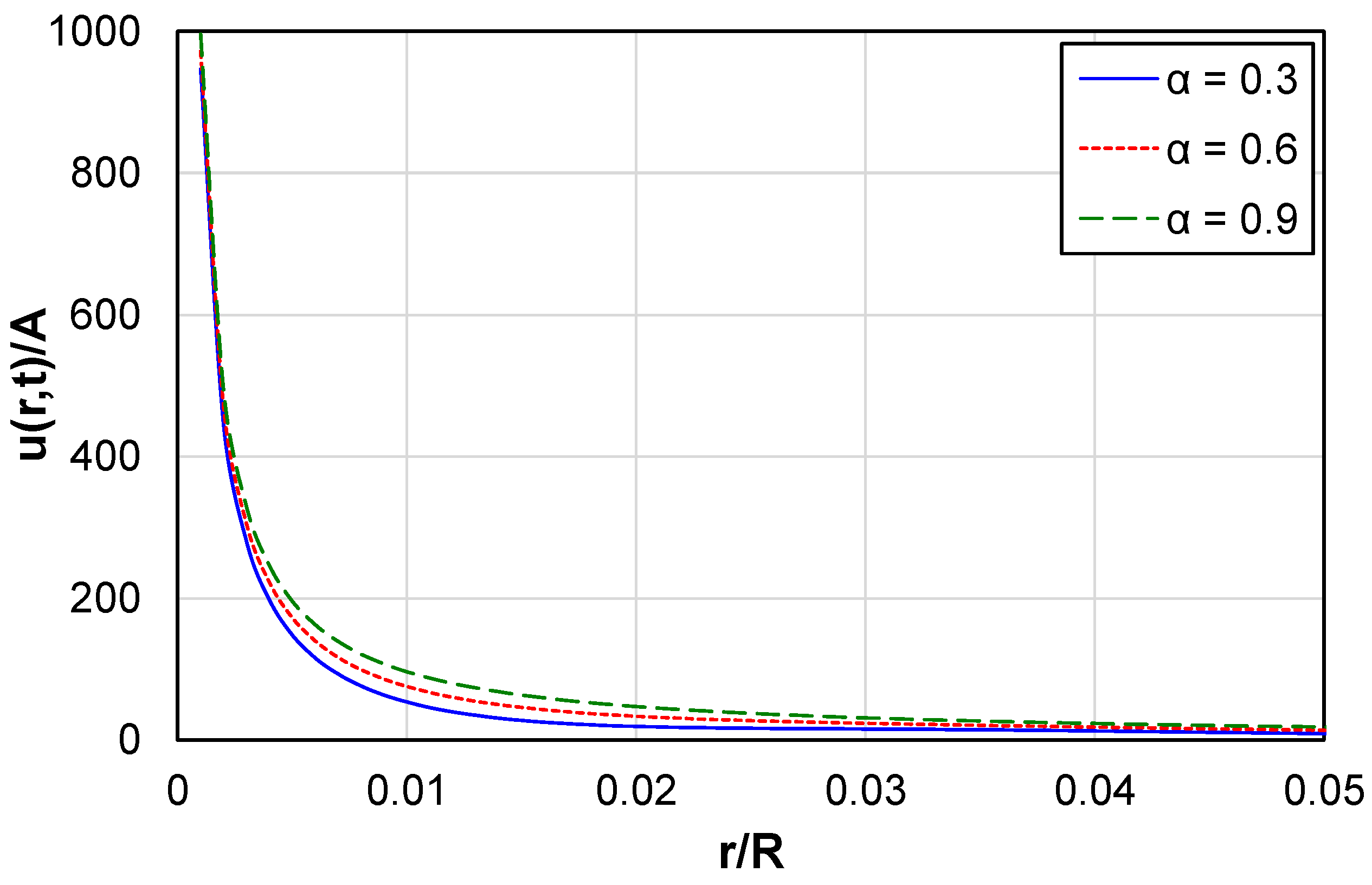
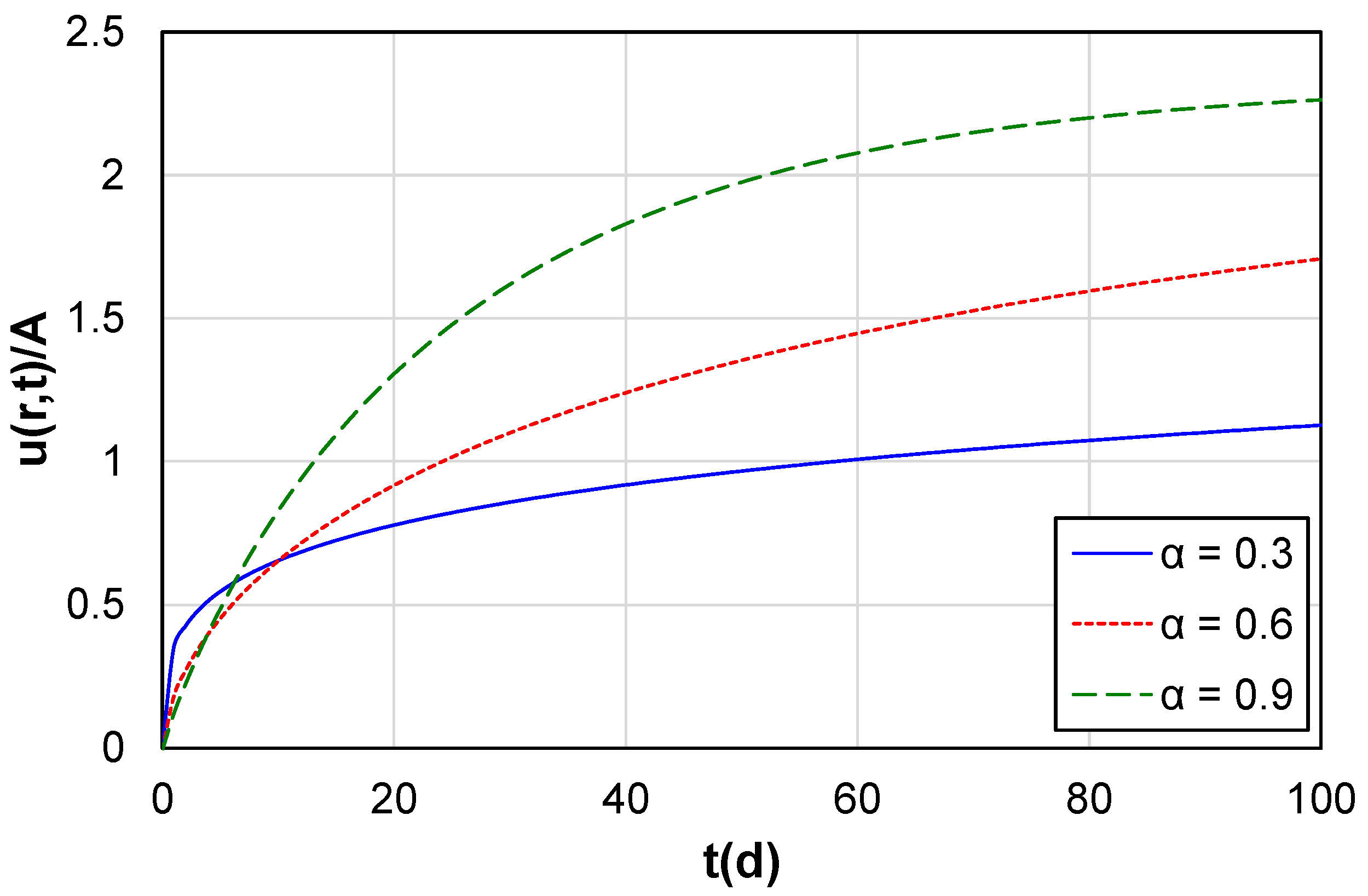
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations, 1st ed.; Wiley: Hoboken, NJ, USA, 1993. [Google Scholar]
- Ross, B. The development of fractional calculus 1695–1900. Hist. Math. 1977, 4, 75–89. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications, 1st ed.; Elsevier: Amsterdam, The Nederlands, 1998. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Derivatives and Integrals: Theory and Applications; Gordon and Breach Science Publishers: Philadelphia, PA, USA, 1993. [Google Scholar]
- Yang, X.J. General Fractional Derivatives: Theory, Methods and Applications, 1st ed.; Chapman and Hall/CRC: Boca Raton, FL, USA, 2019. [Google Scholar]
- Su, N. Fractional Calculus for Hydrology, Soil Science and Geomechanics: An Introduction to Applications, 1st ed.; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Babiarz, A.; Czornik, A.; Klamka, J.; Niezabitowski, M. Theory and applications of non-integer order systems. In Proceedings of the 8th Conference on Non-integer Order Calculus and Its Applications, Zakopane, Poland, 20–21 September 2016. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, 1st ed.; Elsevier: Amsterdam, The Nederlands, 2006. [Google Scholar]
- Desai, C.S.; Zaman, M. Advanced Geotechnical Engineering: Soil-Structure Interaction Using Computer and Material Models; Taylor & Francis: London, UK, 2013. [Google Scholar]
- Langlands, T.A.; Henry, B.I. The accuracy and stability of an implicit solution method for the fractional diffusion equation. J. Comput. Phys. 2005, 205, 719–736. [Google Scholar] [CrossRef]
- Li, C.; Zeng, F. Numerical Methods for Fractional Calculus, 1st ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Tadjeran, C.; Meerschaert, M.M. A second-order accurate numerical method for the two-dimensional fractional diffusion equation. J. Comput. Phys. 2007, 220, 813–823. [Google Scholar] [CrossRef]
- Aslefallah, M.; Rostamy, D.; Hosseinkhani, K. Solving time-fractional differential diffusion equation by theta-method. Int. J. Adv. Appl. Math. Mech. 2014, 2, 1–8. [Google Scholar]
- Gong, C.; Bao, W.; Tang, G.; Jiang, Y.; Liu, J. A parallel algorithm for the two-dimensional time fractional diffusion equation with implicit difference method. Sci. World J. 2014, 2014, 219580. [Google Scholar] [CrossRef]
- Pandey, P.; Kumar, S.; Gomez-Aguilar, J.F. Numerical solution of the time fractional reaction-advection-diffusion equation in porous media. J. Appl. Comput. Mech. 2022, 8, 84–96. [Google Scholar] [CrossRef]
- Li, L.; Jiang, Z.; Yin, Z. Compact finite-difference method for 2D time-fractional convection-diffusion equation of groundwater pollution problems. Comput. Appl. Math. 2020, 39, 142. [Google Scholar] [CrossRef]
- Atangana, A.; Kilicman, A. Analytical solutions of the space-time fractional derivative of advection dispersion equation. Math. Probl. Eng. 2013, 2013, 853127. [Google Scholar] [CrossRef]
- Khalil, R.; Al Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Abdeljawad, T. On conformable fractional calculus. J. Comput. Appl. Math. 2015, 279, 57–66. [Google Scholar] [CrossRef]
- Teodoro, G.S.; Machado, J.T.; De Oliveira, E.C. A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 2019, 388, 195–208. [Google Scholar] [CrossRef]
- Zhao, D.; Luo, M. General conformable fractional derivative and its physical interpretation. Calcolo 2017, 54, 903–917. [Google Scholar] [CrossRef]
- Abu Hammad, I.; Khalil, R. Fractional fourier series with applications. Am. J. Comput. Appl. Math. 2014, 4, 187–191. [Google Scholar] [CrossRef]
- Hammad, M.A.; Khalil, R. Legendre fractional differential equation and legender fractional polynomials. Int. J. Appl. Math. Res. 2014, 3, 214–219. [Google Scholar] [CrossRef]
- Tajadodi, H.; Khan, Z.A.; Irshad, A.R.; Gomez-Aguilar, J.F.; Khan, A.; Khan, H. Exact solutions of conformable fractional differential equations. Results Phys. 2021, 22, 103916. [Google Scholar] [CrossRef]
- Patel, H.; Patel, T.; Pandit, D. An efficient technique for solving fractional-order diffusion equations arising in oil pollution. J. Ocean Eng. Sci. 2023, 8, 217–225. [Google Scholar] [CrossRef]
- Bouaouid, M.; Hilal, K.; Melliani, S. Nonlocal telegraph equation in frame of the conformable time-fractional derivative. Adv. Math. Phys. 2019, 2019, 7528937. [Google Scholar] [CrossRef]
- Ortega, A.; Rosales, J.J. Newton’s law of cooling with fractional conformable derivative. Rev. Mex. Fís. 2018, 64, 172–175. [Google Scholar] [CrossRef]
- Bayrak, M.A.; Demir, A.; Ozbilge, E. A novel approach for the solution of fractional diffusion problems with conformable derivative. Numer. Meth. Part Differ. Equ. 2023, 39, 1870–1887. [Google Scholar] [CrossRef]
- Chaudhary, M.; Kumar, R.; Singh, M.K. Fractional convection-dispersion equation with conformable derivative approach. Chaos Solitons Fractals 2020, 141, 110426. [Google Scholar] [CrossRef]
- Zhou, H.; Yang, S.; Zhang, S. Conformable derivative approach to anomalous diffusion. Physica A 2018, 491, 1001–1013. [Google Scholar] [CrossRef]
- Sabaté, J.; Vinas, M.; Solanas, A. Laboratory-scale bioremediation experiments on hydrocarbon-contaminated soils. Int. Biodeterior. Biodegrad. 2004, 54, 19–25. [Google Scholar] [CrossRef]
- Reible, D.; Demnerova, K. Innovative Approaches to the On-Site Assessment and Remediation of Contaminated Sites, 15th ed.; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Blazek, J. Computational Fluid Dynamics: Principles and Applications, 3rd ed.; Elsevier: Amsterdam, The Nederlands, 2015. [Google Scholar]
- He, J.H. Approximate analytical solution for seepage flow with fractional derivatives in porous media. Comput. Meth. Appl. Mech. Eng. 1998, 167, 57–68. [Google Scholar] [CrossRef]
- Ordu, S.; Ordu, E.; Mutlu, R. Axisymmetric spilled pollutant analysis in steady-state using finite difference method. Fresenius Environ. Bull. 2022, 31, 9587–9592. [Google Scholar]
- Ordu, S.; Ordu, E.; Mutlu, R. Seepage analysis from a long-polluted river in steady-state using finite difference method. Adiyaman Univ. J. Eng. Sci. 2023, 10, 1–14. (In Turkish) [Google Scholar] [CrossRef]
- Salehi, Y.; Darvishi, M.T.; Schiesser, W.E. Numerical solution of space fractional diffusion equation by the method of lines and splines. Appl. Math. Comput. 2018, 336, 465–480. [Google Scholar] [CrossRef]
- Wang, Y.M.; Wang, T. A compact locally one-dimensional method for fractional diffusion-wave equations. J. Appl. Math. Comput. 2015, 49, 41–67. [Google Scholar] [CrossRef]
- Zhang, K. Existence results for a generalization of the time-fractional diffusion equation with variable coefficients. Bound. Value Probl. 2019, 2019, 10. [Google Scholar] [CrossRef]
- Zhuang, P.; Liu, F. Finite difference approximation for two-dimensional time fractional diffusion equation. J. Algorithms Comput. Technol. 2007, 1, 1–16. [Google Scholar] [CrossRef]
- Murio, D.A. Implicit finite difference approximation for time fractional diffusion equations. Comput. Math. Appl. 2008, 56, 1138–1145. [Google Scholar] [CrossRef]
- Baleanu, D.; Agheli, B.; Al Qurashi, M.M. Fractional advection differential equation within Caputo and Caputo–Fabrizio derivatives. Adv. Mech. Eng. 2016, 8, 1687814016683305. [Google Scholar] [CrossRef]
- Bohaienko, V.; Bulavatsky, V. Mathematical modeling of solutes migration under the conditions of groundwater filtration by the model with the k-Caputo fractional derivative. Fractal Fract. 2018, 2, 28. [Google Scholar] [CrossRef]
- Gulkaç, V. A method of finding source function for inverse diffusion problem with time-fractional derivative. Adv. Math. Phys. 2016, 2016, 6470949. [Google Scholar] [CrossRef]
- Bildik, N.; Deniz, S. A new fractional analysis on the polluted lakes system. Chaos Solitons Fractals 2019, 122, 17–24. [Google Scholar] [CrossRef]
- Yao, L.; Ren, L.; Gong, G. Time-fractional model of chloride diffusion in concrete: Analysis using meshless method. Adv. Mater. Sci. Eng. 2020, 2020, 4171689. [Google Scholar] [CrossRef]
- Mirza, I.A.; Akram, M.S.; Shah, N.A.; Akhtar, S.; Muneer, M. Study of one-dimensional contaminant transport in soils using fractional calculus. Math. Meth. Appl. Sci. 2021, 44, 6839–6856. [Google Scholar] [CrossRef]
- Atangana, A.; Bildik, N. The use of fractional order derivative to predict the groundwater flow. Math. Probl. Eng. 2013, 2013, 543026. [Google Scholar] [CrossRef]
- MacCluer, C.R. Boundary Value Problems and Orthogonal Expansions: Physical Problems from a Sobolev Viewpoint, 1st ed.; IEEE Press: Piscataway, NJ, USA, 1994. [Google Scholar]
- Wong, J.S. On the generalized Emden–Fowler equation. J. Korean Soc. Ind. Appl. Math. 1975, 17, 339–360. [Google Scholar] [CrossRef]
- Emden, R. Gaskugeln, Anwendungen der Mechanischen Warmen-Theorie auf Kosmologie und Meteorologische Probleme, 1st ed.; Bibliotheca Teubneriana: Berlin, Germany, 1907. [Google Scholar]
- Lucena, L.; Da Silva, L.; Evangelista, L.; Lenzi, M.; Rossato, R.; Lenzi, E. Solutions for a fractional diffusion equation with spherical symmetry using green function approach. Chem. Phys. 2008, 344, 90–94. [Google Scholar] [CrossRef]
- Carleson, L. On convergence and growth of partial sums of Fourier series. Acta Math. 1966, 116, 135–157. [Google Scholar] [CrossRef]
- Dirichlet, G.L. Sur la convergence des series trigonometriques qui servent à represénter une fonction arbitraire entre des limites donnees. J. Reine Angew. Math. 1829, 4, 157–169. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, M.; Mert Coskun, O.; Ordu, S.; Mutlu, R. Modeling Pollutant Diffusion in the Ground Using Conformable Fractional Derivative in Spherical Coordinates with Complete Symmetry. Symmetry 2024, 16, 1358. https://doi.org/10.3390/sym16101358
Kim M, Mert Coskun O, Ordu S, Mutlu R. Modeling Pollutant Diffusion in the Ground Using Conformable Fractional Derivative in Spherical Coordinates with Complete Symmetry. Symmetry. 2024; 16(10):1358. https://doi.org/10.3390/sym16101358
Chicago/Turabian StyleKim, Mintae, Oya Mert Coskun, Seyma Ordu, and Resat Mutlu. 2024. "Modeling Pollutant Diffusion in the Ground Using Conformable Fractional Derivative in Spherical Coordinates with Complete Symmetry" Symmetry 16, no. 10: 1358. https://doi.org/10.3390/sym16101358
APA StyleKim, M., Mert Coskun, O., Ordu, S., & Mutlu, R. (2024). Modeling Pollutant Diffusion in the Ground Using Conformable Fractional Derivative in Spherical Coordinates with Complete Symmetry. Symmetry, 16(10), 1358. https://doi.org/10.3390/sym16101358




