Calogero-like Model without Rearrangement Symmetry
Abstract
1. Introduction
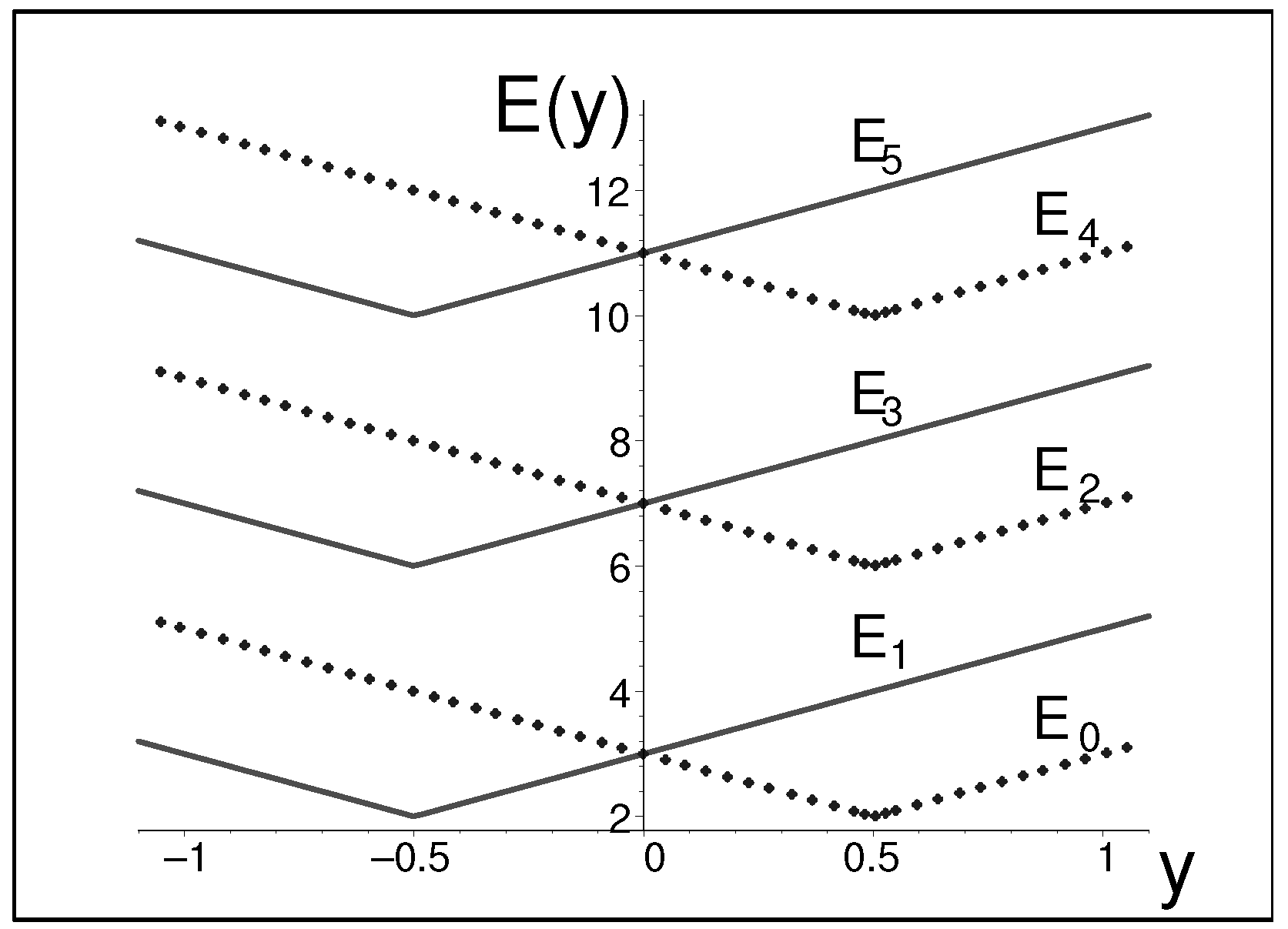
2. Spectral Degeneracy and Its Unfolding at A = 2
2.1. Singularity in the Origin
2.2. Asymmetric Barrier
3. Spectral Degeneracy and its Unfolding at A = 3
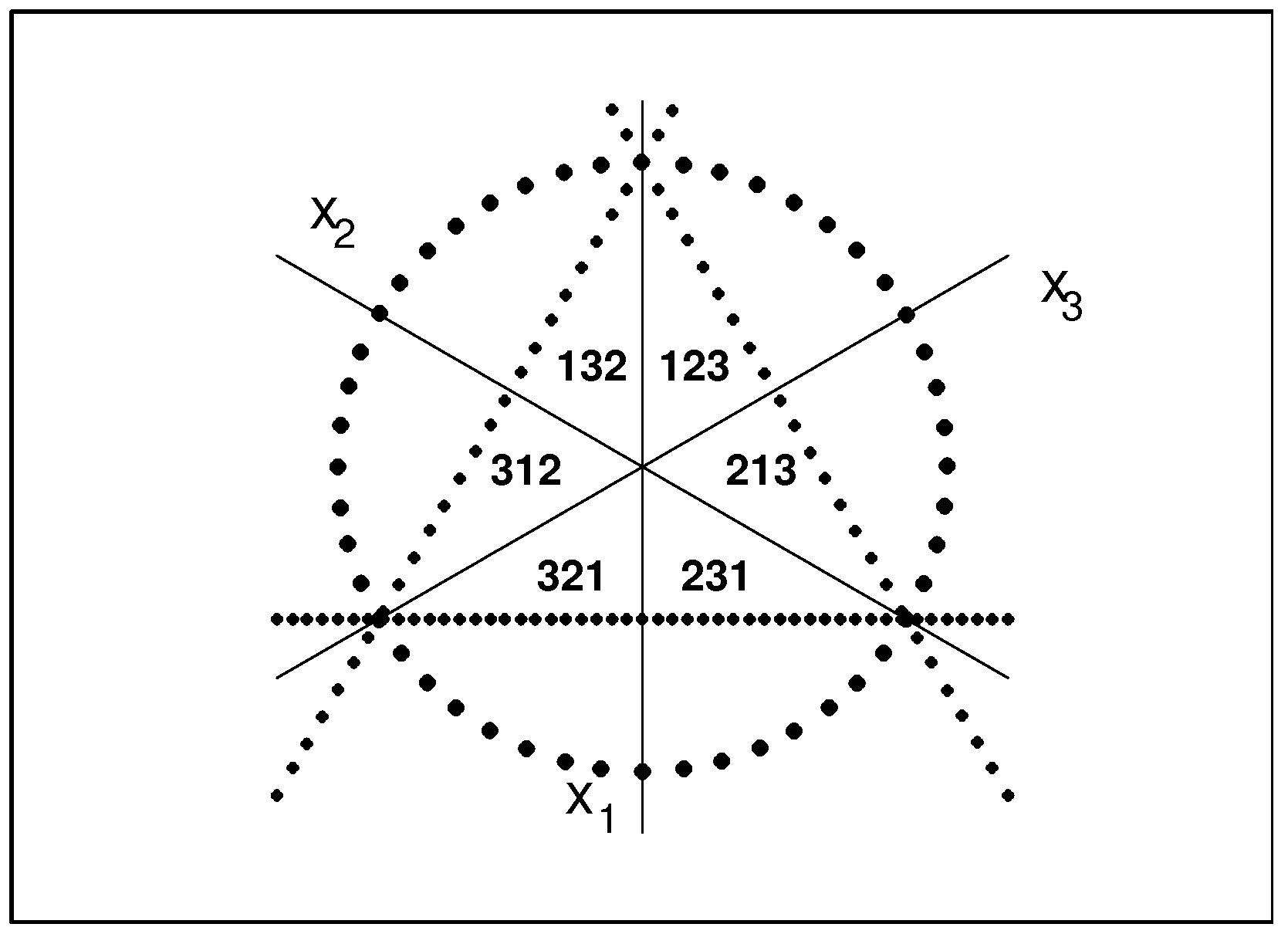
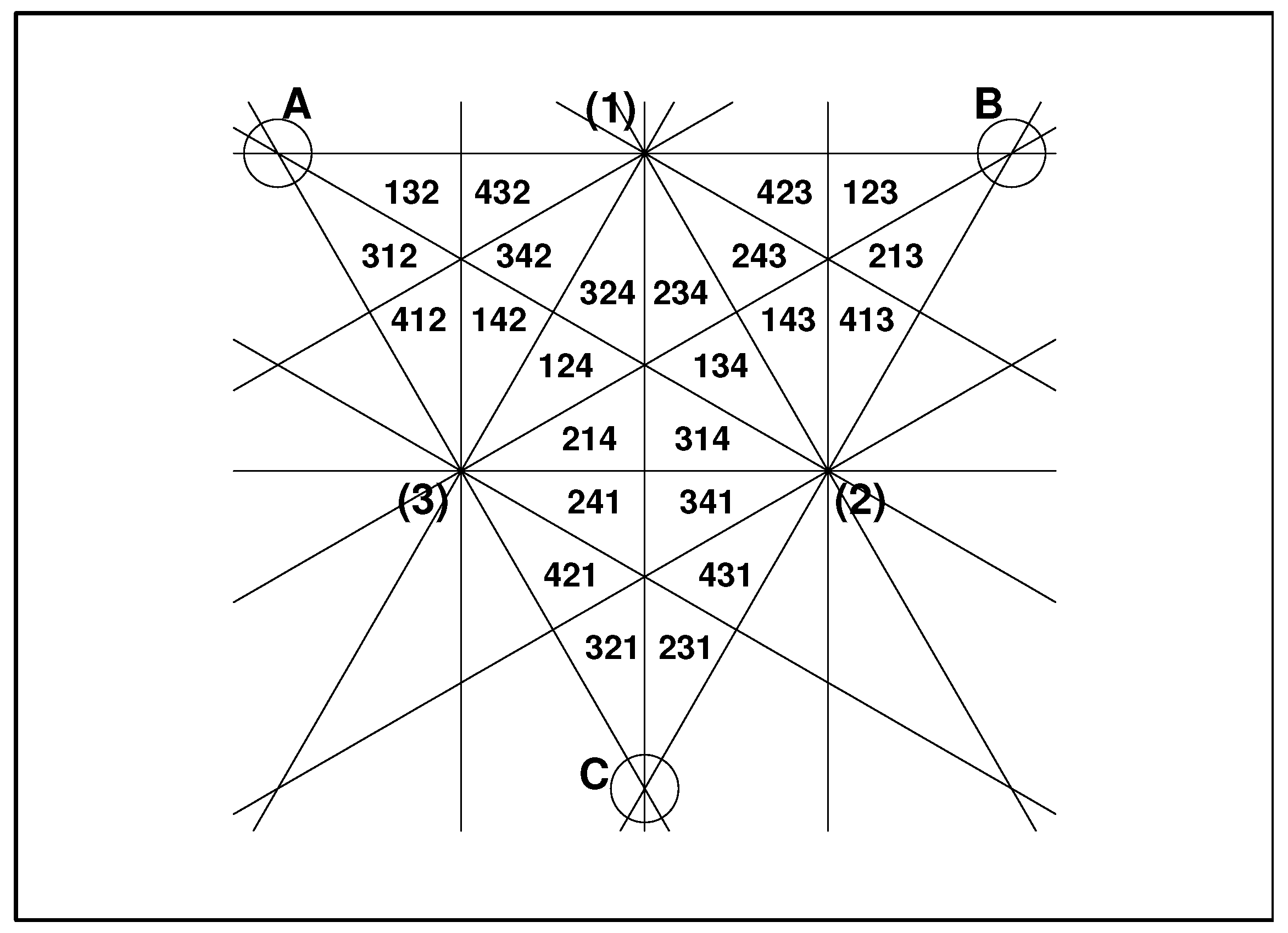
3.1. Weyl Chambers
3.2. Nonequal Barriers vs. Loss of Solvability
3.3. Reinstallation of Solvability: Asymmetric Barriers
4. Spectral Degeneracy and Its Unfolding Beyond A = 3
4.1. Exact Solvability
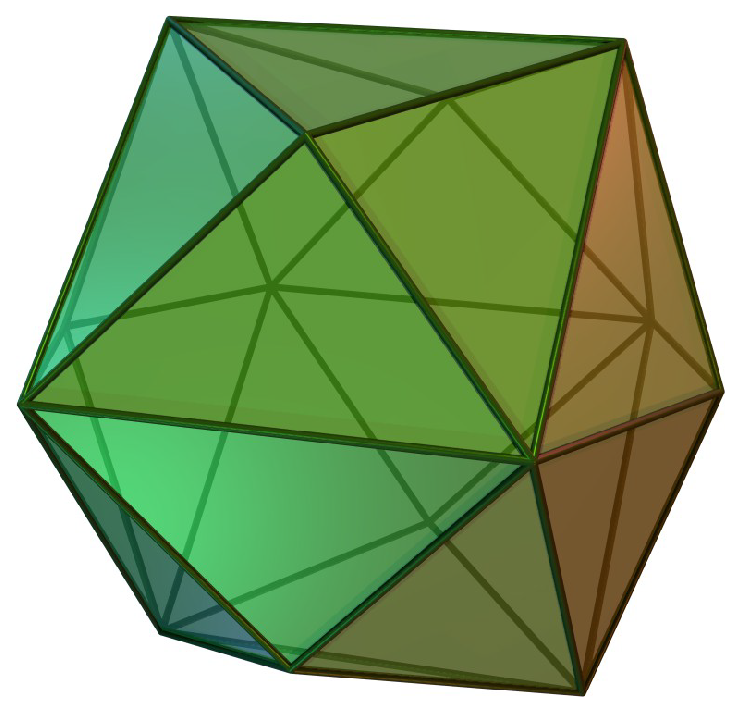
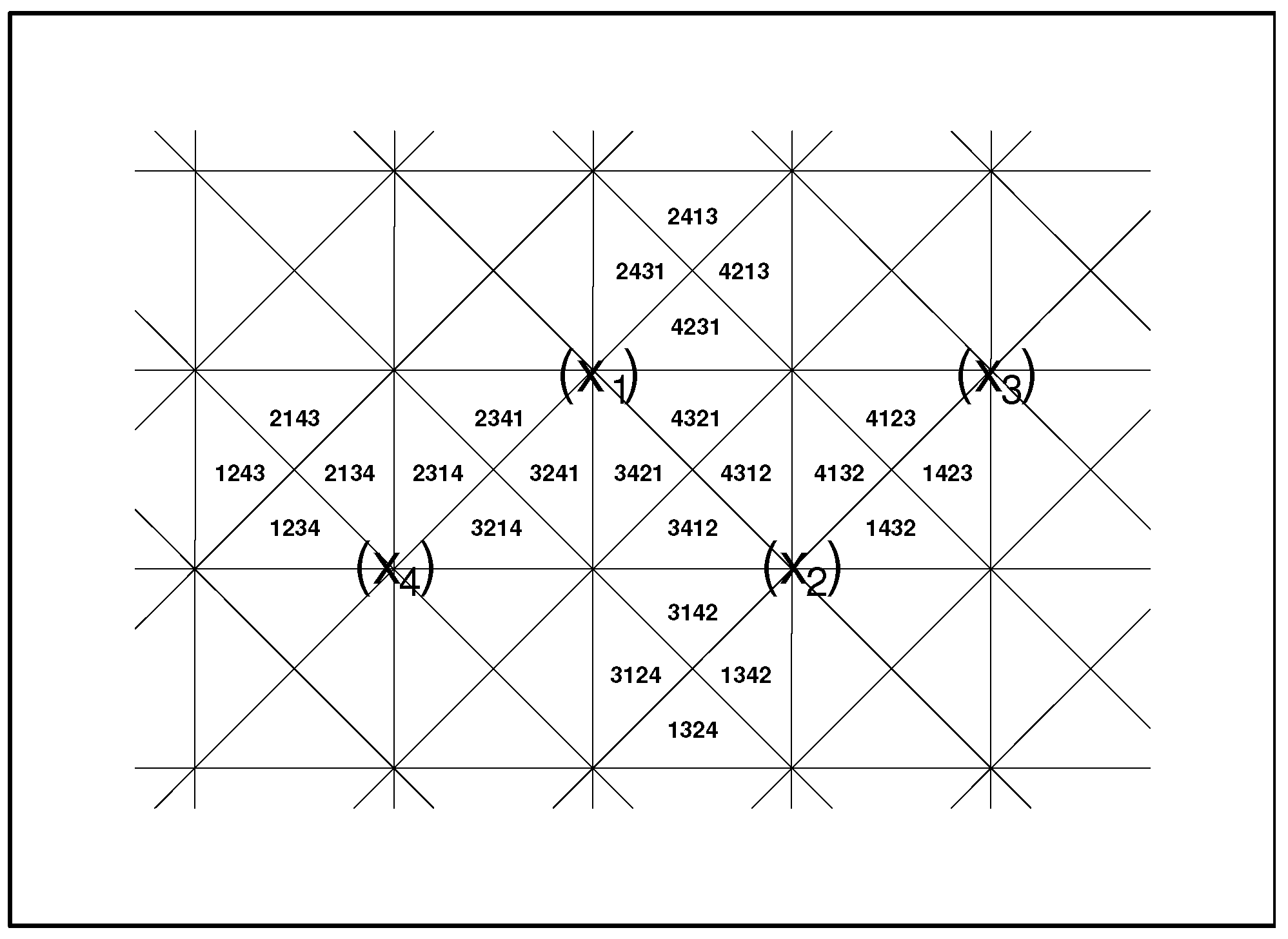
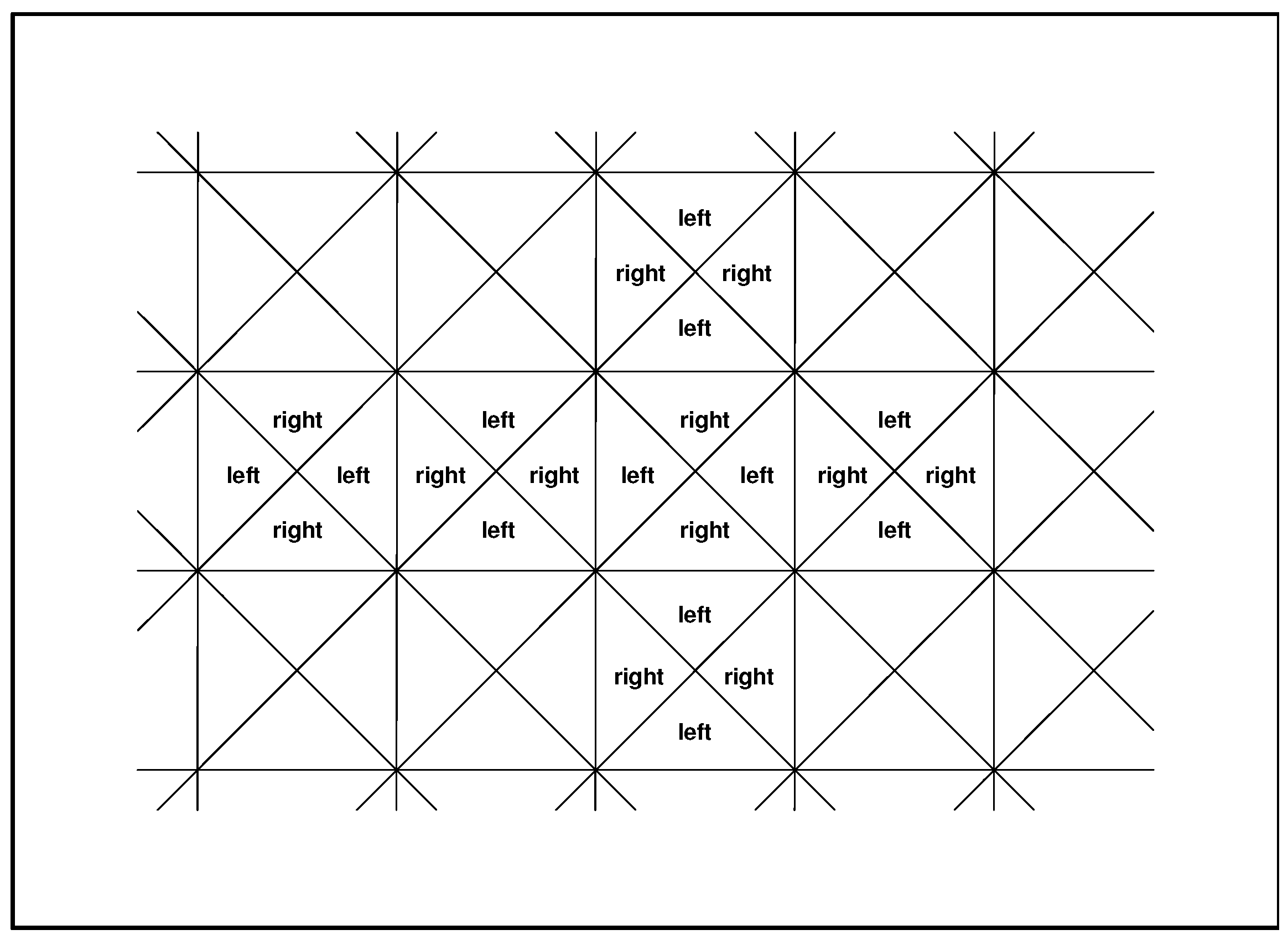
4.2. Weyl-Chamber Boundaries and Their Cartography at A = 4
4.2.1. Colorings at
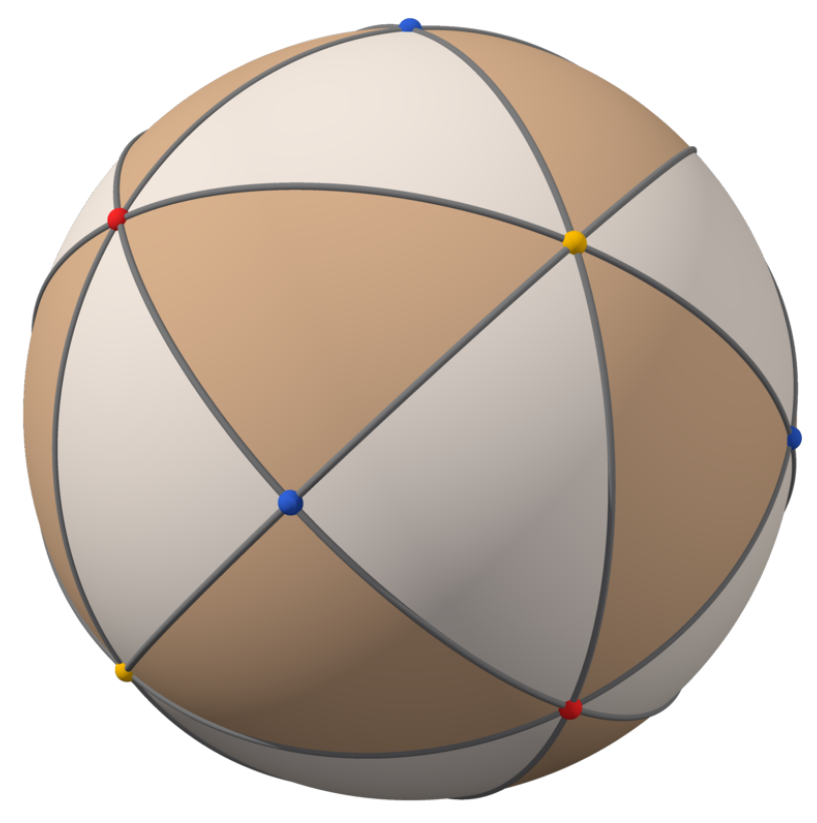
4.2.2. Projection on a Central Sphere
4.2.3. Projections on a Central Cube
4.2.4. Numbering by Permutations
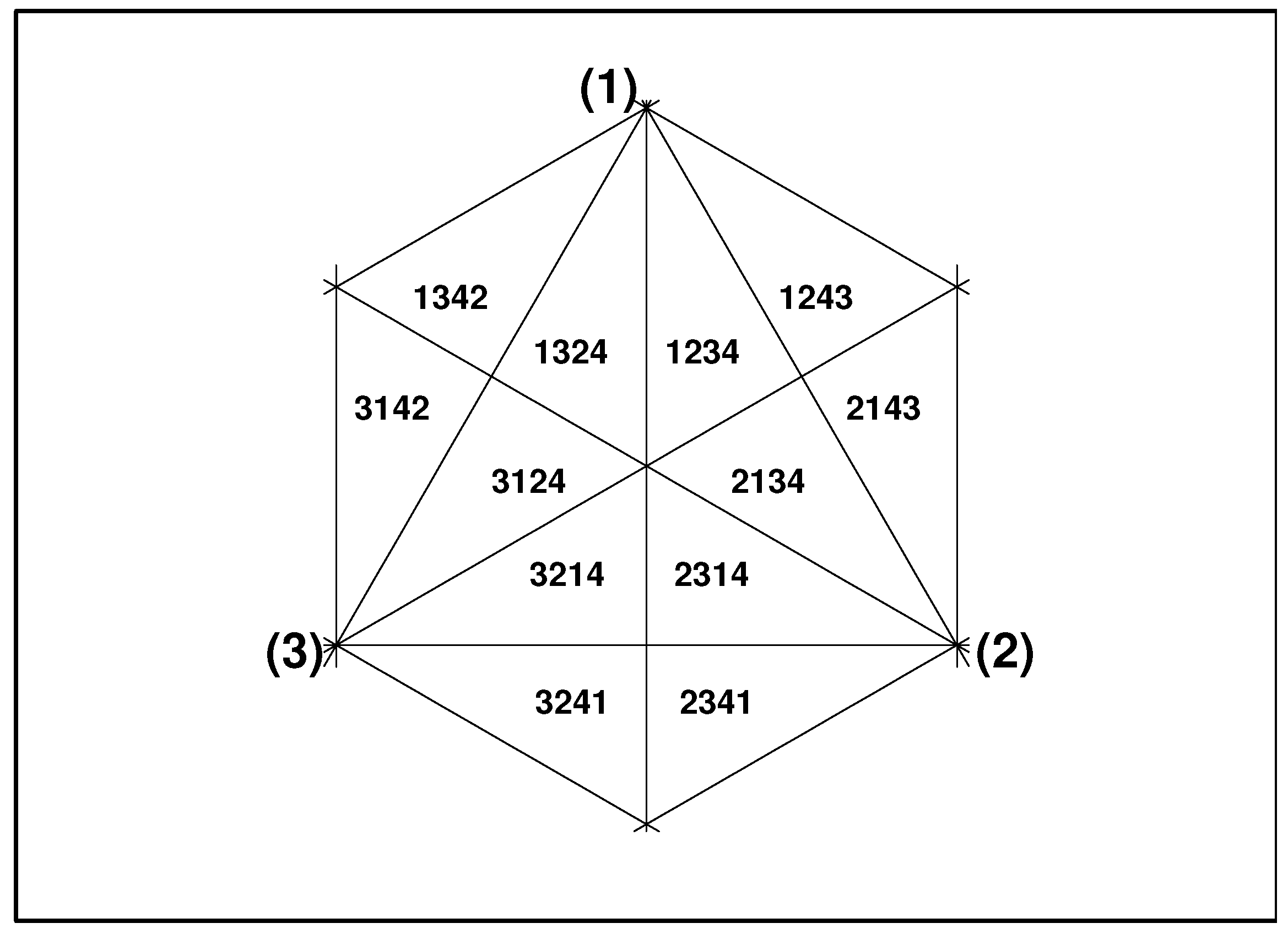
4.3. Alternative Cartography at A = 4
4.3.1. Projections of the Set of Weyl Chambers on a Tetrahedron
4.3.2. An Ultimate Classification of the Barriers
5. Summary
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. The Occurrence of Asymmetric Impenetrable Barriers in Relativistic Quantum Mechanics
References
- Olshanetsky, M.A.; Perelomov, A.M. Quantum completely integrable systems connected with semi-simple Lie algebras. Lett. Math. Phys. 1977, 2, 7–13. [Google Scholar] [CrossRef]
- Olshanetsky, M.A.; Perelomov, A.M. Quantum integrable systems related to Lie algebras. Phys. Rept. 1983, 94, 313–404. [Google Scholar] [CrossRef]
- Turbiner, A. Hidden algebra of the N-body Calogero problem. Phys. Lett. B 1994, 320, 281–286. [Google Scholar] [CrossRef]
- Calogero, F. Solution of a three-body problem in one dimension. J. Math. Phys. 1969, 10, 2191–2196. [Google Scholar] [CrossRef]
- Calogero, F. Ground state of a one-dimensional N-body problem. J. Math. Phys. 1969, 10, 2197–2200. [Google Scholar] [CrossRef]
- Calogero, F. Solution of the one-dimensional N-body problem with quadratic and/or inversely quadratic pair potentials. J. Math. Phys. 1971, 12, 419–436. [Google Scholar] [CrossRef]
- Van Diejen, J.F.; Vinet, L. (Eds.) Calogero-Moser- Sutherland Models (CRM Series in Mathematical Physics); Springer: New York, NY, USA, 2000. [Google Scholar]
- Rühl, W.; Turbiner, A.V. Exact solvability of the Calogero and Sutherland models. Mod. Phys. Lett. A 1995, 10, 2213–2222. [Google Scholar] [CrossRef]
- Sutherland, B. Beautiful Models: 70 Years of Exactly Solved Quantum Many-Body Problems; World Scientific: Singapore, 2004. [Google Scholar]
- Polychronakos, A.P. Physics and mathematics of Calogero particles. J. Phys. A Math. Gen. 2006, 39, 12793. [Google Scholar] [CrossRef]
- Calogero, F. Calogero-Moser system. Scholarpedia 2008, 3, 7216. [Google Scholar] [CrossRef][Green Version]
- Ghosh, P.K.; Khare, A.; Sivakumar, M. Supersymmetry, shape invariance, and solvability of AN-1 and BCN Calogero-Sutherland model. Phys. Rev. A 1998, 58, 821. [Google Scholar] [CrossRef]
- Khastgir, S.P.; Pocklington, A.J.; Sasaki, R. Quantum Calogero-Moser Models: Integrability for all Root Systems. J. Phys. A Math. Gen. 2000, 33, 9033–9064. [Google Scholar] [CrossRef][Green Version]
- Boreskov, K.G.; Turbiner, A.V.; Vieyra, J.C.L. Solvability of the Hamiltonians related to exceptional root spaces: Rational case. Commun. Math. Phys. 2005, 260, 17–44. [Google Scholar] [CrossRef]
- Dunkl, C.F.; Hu, Y. Orthogonal Polynomials of Several Variables; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Van Diejen, J.F. Confluent hypergeometric orthogonal polynomials related to the rational quantum Calogero system with harmonic confinement. Commun. Math. Phys. 1997, 188, 467–497. [Google Scholar] [CrossRef]
- Chung, W.S.; Hassanabadi, H. One-dimensional quantum mechanics with Dunkl derivative. Mod. Phys. Lett. A 2019, 34, 1950190. [Google Scholar] [CrossRef]
- Sedaghatnia, P.; Hassanabadi, H.; Junker, G.; Kříž, J.; Hassanabadi, S.; Chung, W.S. Investigation of the generalised Wigner-Dunkl harmonic oscillator and its coherent states. Ann. Phys. 2023, 458, 169445. [Google Scholar] [CrossRef]
- Scholtz, F.G.; Geyer, H.B.; Hahne, F.J.W. Quasi-Hermitian Operators in Quantum Mechanics and the Variational Principle. Ann. Phys. 1992, 213, 74–101. [Google Scholar] [CrossRef]
- Znojil, M.; Tater, M. Complex Calogero model with real energies. J. Phys. A Math. Gen. 2001, 34, 1793–1803. [Google Scholar] [CrossRef]
- Znojil, M.; Tater, M. Exactly solvable three-body Calogero-type model with translucent two-body barriers. Phys. Lett. A 2001, 284, 225–230. [Google Scholar] [CrossRef]
- Ghosh, P.K.; Gupta, K.S. On the real spectra of Calogero model with complex coupling. Phys. Lett. A 2004, 323, 29–33. [Google Scholar] [CrossRef]
- Assis, P.E.G.; Fring, A. From real fields to complex Calogero particles. J. Phys. A Math. Gen. 2009, 42, 425206. [Google Scholar] [CrossRef]
- Fring, A.; Smith, M. Antilinear deformations of Coxeter groups, an application to Calogero models. J. Phys. A Math. Gen. 2010, 43, 325201. [Google Scholar] [CrossRef]
- Bagarello, F.; Gazeau, J.-P.; Szafraniec, F.; Znojil, M. (Eds.) Non-Selfadjoint Operators in Quantum Physics: Mathematical Aspects; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Znojil, M. PT symmetric harmonic oscillators. Phys. Lett. A 1999, 259, 220–223. [Google Scholar] [CrossRef]
- Jakubsky, V. PT-symmetric Calogero-type model. Czechosl. J. Phys. 2004, 54, 67–69. [Google Scholar] [CrossRef]
- Brihaye, Y.; Nininahazwe, A. On PT symmetric extensions of the Calogero model. Int. J. Mod. Phys. A 2004, 19, 4391–4400. [Google Scholar] [CrossRef]
- Fring, A.; Znojil, M. PT-symmetric deformations of Calogero models. J. Phys. A Math. Gen. 2008, 41, 194010. [Google Scholar] [CrossRef]
- Fring, A. PT-symmetric deformations of integrable models. Phil. Trans. Roy. Soc. Lond. A 2013, 371, 20120046. [Google Scholar] [CrossRef] [PubMed]
- Correa, F.; Lechtenfeld, O. Algebraic integrability of PT-deformed Calogero models. J. Phys. Conf. Ser. 2021, 2038, 012007. [Google Scholar] [CrossRef]
- Jakubsky, V.; Znojil, M.; Luis, E.A.; Kleefeld, F. Trigonometric identities, angular Schrödinger equations and a new family of solvable models. Phys. Lett. A 2005, 334, 154–159. [Google Scholar] [CrossRef]
- Hakobyan, T.; Nersessian, A.; Yeghikyan, V. The cuboctahedric Higgs oscillator from the rational Calogero model. J. Phys. A Math. Theor. 2009, 42, 205206. [Google Scholar] [CrossRef]
- Feigin, M.V. Intertwining relations for the spherical parts of generalized Calogero operators. Theor. Math. Phys. 2003, 135, 497–509. [Google Scholar]
- Hakobyan, T.; Lechtenfeld, O.; Nersessian, A. The spherical sector of the Calogero model as a reduced matrix model. Nucl. Phys. B 2012, 858, 250–266. [Google Scholar] [CrossRef][Green Version]
- Feigin, M.; Lechtenfeldl, O.; Polychronakos, A. The quantum angular Calogero-Moser model. J. High Energy Phys. 2013, 1307, 162. [Google Scholar] [CrossRef]
- Correa, F.; Lechtenfeld, O. The tetrahexahedric angular Calogero model. J. High Energy Phys. 2015, 1510, 191. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics: Non-Relativistic Theory; Pergamon: New York, NY, USA, 1977. [Google Scholar]
- Lapointe, L.; Vinet, L. Exact Operator Solution of the Calogero-Sutherland Model. Commun. Math. Phys. 1996, 178, 425–452. [Google Scholar] [CrossRef]
- Znojil, M. Comment on conditionally exactly soluble class of quantum potentials. Phys. Rev. A 2000, 61, 066101. [Google Scholar] [CrossRef]
- Ishkhanyan, A.M. Conditionally exactly solvable Dirac potential, including x1/3 pseudoscalar interaction. In Proceedings of the International Conference of Analytic and Algebraic Methods in Physics XX (FNSPE), Prague, Czech Republic, 28–31 August 2023. [Google Scholar]
- Ishkhanyan, A.M.; Kreinov, V.P. Exact solution of the 1D Dirac equaiton for a pseudoscalar interaction potential with the inverse-square-root variation law. Sci. Rep. 2023, 13, 13482. [Google Scholar] [CrossRef]
- Ishkhanyan, A.M.; Kreinov, V.P. Conditionally exactly solvable Dirac potential, including x1/3 pseudoscalar interaction. Phys. Scr. 2023, 98, 075229. [Google Scholar] [CrossRef]
- Flügge, S. Practical Quantum Mechanics I; Springer: Berlin/Heidelberg, Germany, 1971. [Google Scholar]
- Post, G.; Turbiner, A.V. Quasi-solvability of Calogero-Sutherland model. Russ. J. Math. Phys. 1995, 3, 113. [Google Scholar]
- Turbiner, A.V. Quasi-Exactly Solvable Hamiltonians related to Root Spaces. J. Nonlin. Math. Phys. 2005, 12 (Suppl. S1), 660–675. [Google Scholar] [CrossRef]
- Brink, L.; Turbiner, A.V.; Wyllard, N. Hidden algebras of the (super) Calogero and Sutherland models. J. Mathe. Phys. 1998, 39, 1285–1315. [Google Scholar] [CrossRef]
- Boreskov, K.G.; Vieyra, J.C.L.; Turbiner, A.V. Solvability of the F4 integrable system. Int. J. Mod. Phys. A 2001, 16, 4769–4801. [Google Scholar] [CrossRef]
- Humphreys, J.E. Reflection Groups and Coxeter Groups; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Coxeter, H.S.M. Regular Polytopes, 3rd ed.; Dover Publications: New York, NY, USA, 1973. [Google Scholar]
- Available online: https://commons.wikimedia.org/wiki/File:Disdyakis_6_spherical.png (accessed on 27 November 2023).
- Available online: https://en.wikipedia.org/wiki/File:Tetrakishexahedron.jpg (accessed on 27 November 2023).
- Available online: https://commons.wikimedia.org/wiki/File:Disdyakis_6_in_rhombic_6.png (accessed on 27 November 2023).
- Brink, L.; Hansson, T.H.; Vasiliev, M. Explicit solution to the N body Calogero problem. Phys. Lett. B 1992, 286, 109–111. [Google Scholar] [CrossRef]

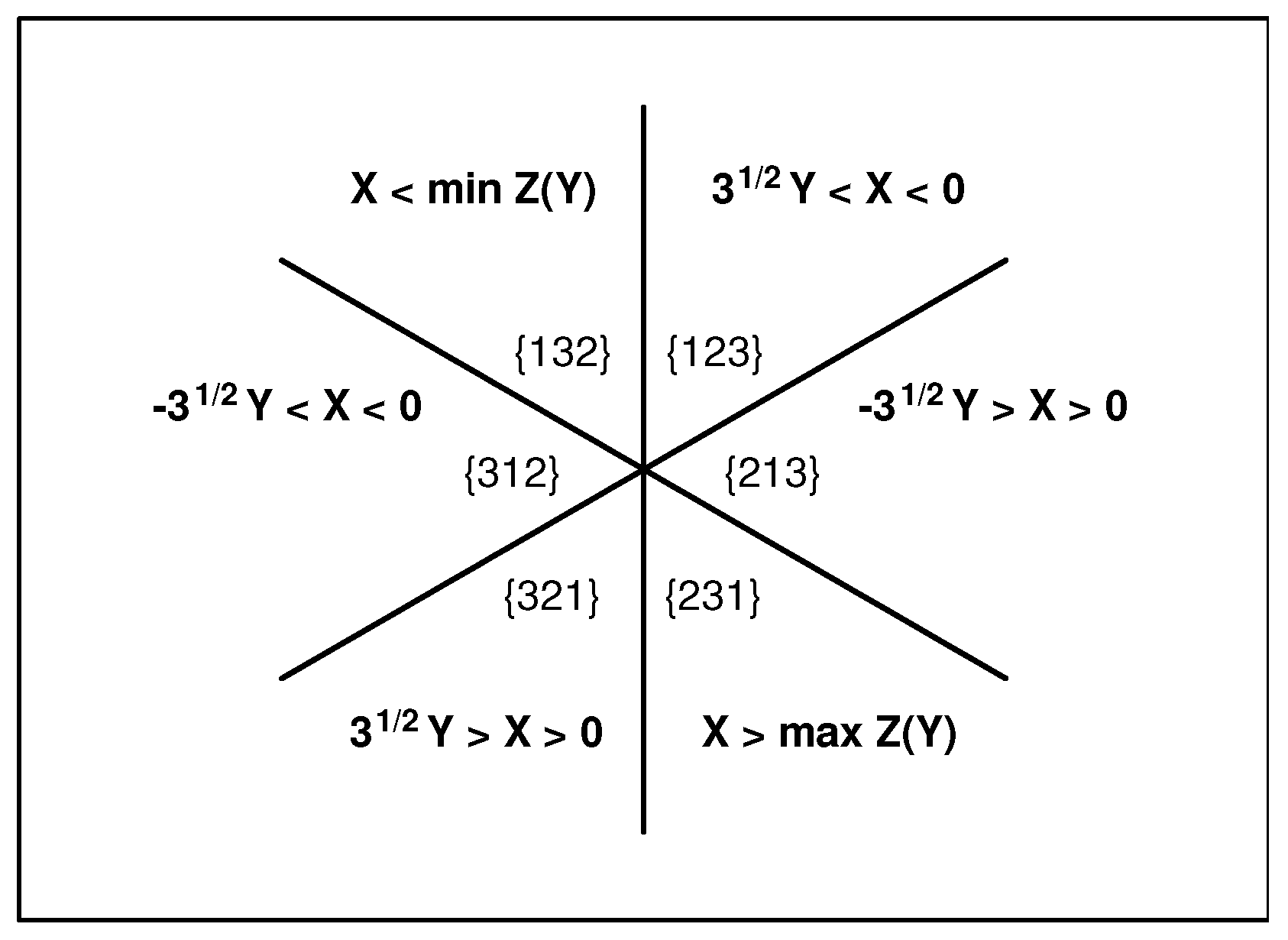

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Znojil, M. Calogero-like Model without Rearrangement Symmetry. Symmetry 2024, 16, 27. https://doi.org/10.3390/sym16010027
Znojil M. Calogero-like Model without Rearrangement Symmetry. Symmetry. 2024; 16(1):27. https://doi.org/10.3390/sym16010027
Chicago/Turabian StyleZnojil, Miloslav. 2024. "Calogero-like Model without Rearrangement Symmetry" Symmetry 16, no. 1: 27. https://doi.org/10.3390/sym16010027
APA StyleZnojil, M. (2024). Calogero-like Model without Rearrangement Symmetry. Symmetry, 16(1), 27. https://doi.org/10.3390/sym16010027





