Explicit Formulas for the Deformation of Chiral Porous Circular Beams in Gradient Thermoelasticity

Abstract

Chirality and porosity are characteristic properties of nanostructured materials. Their effects on the mechanical behaviour of structural elements, such as shells, plates and beams, cannot be disregarded. In this paper, we study the thermoelastic deformation of a chiral porous circular beam loaded with an axial force and torque. The beam is also under the action of a constant temperature field. The analytical solution is obtained using the results established in a paper recently published by the Author within the context of the strain gradient theory proposed by Papanicopolous. In the constitutive equations, the chirality is introduced by a material constant parameter and the porosity is described by means of a scalar function. Displacements, microdilatation function, and stress and strain fields are expressed in explicit form and in terms of engineering constants. Explicit formulas of the stiffness of chiral porous circular beams are presented and the effects of right and left chirality are discussed.

1. Introduction

In general, the term chiral, is used to describe an object that is non-superposable on its mirror image. In continuum mechanics, a material whose properties are not invariant to inversion is called chiral. Ordinary materials, as structural and building materials, do not exhibit chirality and this property has been ignored in mechanics for a long time. In recent years, the great scientific and technical achievements in the creation of nanomaterials and nanotechnology have driven interest in the research of novel phenomena and properties of materials whose internal structure has nanoscale dimensions. Chirality is a characteristic property of nanomaterials and its effects on the behaviour of structural elements (shells, plates, beams, etc.) are not negligible. For a brief historical sketch on nanomaterials and nanotechnology, see Guz and Rushchitskii [1] and the references therein. For an overview of chirality in mechanics, see Lakes [2].

Various mathematical theories have been considered to introduce chirality in the mechanical behaviour of materials [3,4,5,6]. Among the microcontinuum theories of elasticity, the Cosserat theory has attracted increasing interest for describing microstructural effects due to chirality of the microstructure [7,8,9]. An extended micropolar theory was ideated to study the chiral effects that arise from coupling between stretching deformations and the microrotation [10,11,12,13]. In Cosserat models, chirality introduces three additional parameters for a total of nine material parameters. An alternative approach is provided by the strain gradient theory, which has been shown to be effective in investigating problems related to size effects and nanotechnology [14,15]. In [16], Papanicolopulos adapted the Mindlin–Eshel strain gradient theory for centrosymmetric materials [17] for the case of noncentrosymmetric solids. In contrast with the Cosserat model, in the strain gradient theory the chiral behaviour is controlled by a single material parameter and the sign of parameter allows for distinguishing between right and left chirality.

Porosity is another important feature of materials with nanostructures. Nanotubes, nanospheres, and other nanoparticles possess a high porosity because of their internal cavities [18,19].

There are a number of theories that describe the mechanical properties of materials with single and multiple porosity (see, e.g., [20,21,22] and the references therein). Microcontinuum field theories provide suitable mathematical models to investigate the behaviour of porous media [23,24]. For instance, if in the microstretch theory the rigid microrotation of the material particles is absent, we obtain the theory of materials with voids proposed by Cowin and Nunziato [25]. In both theories, the variation in void volume is described by an independent kinematical variable named the microdilatation function. The Cowin–Nunziato theory has been subject to intensive study and a great number of contributions regarding the fundamentals and applications have been published [26,27,28,29,30,31,32,33].

This paper is concerned with the strain gradient theory of porous thermoelastic materials. The theory is constructed adding the second-order partial derivative of the displacement and the first order derivative of the microdilatation function in the classical set of independent constitutive variables [34,35]. We applied this theory to study the thermoelastic deformation of a chiral porous circular cylinder.

The problems of the elastic deformation of generalized models of beams have been largely investigated [36,37,38,39,40,41]. For recent theoretical results on the theory of nanobeams, see [42,43,44,45]. The method of solution is well known and consists of reducing the 3D problem in the solution of two-dimensional problems. Usually, the solution depends on the cross section and cannot be expressed in explicit form, so we have only a qualitative description of the deformation. In the case of chiral beams, in contrast with the classical elasticity, an axial force produces torsion and torque produces extension. These effects are not typical of chirality and have been observed in some nonlinear phenomena, termed the poynting effect [46,47,48]. Possible correlations between the poynting effect and chirality have not yet been investigated.

In a recent article, De Cicco and Iesan [49] solved the general problem of the thermoelastic deformation of chiral porous cylinders. In this paper, we used the results established in [49] to obtain explicit formulas for the case of a cylinder with a circular cross-section and loaded with an axial force, torque, and constant temperature field. As the general solution presented in [49] was quite complex and could be difficult to use, the purpose of this study was to express the relations for the displacement, microdilatation function, and stress and strain fields in a convenient form, avoiding the use exceedingly complicated and tedious calculations used by researchers in the field. We obtained a clear qualitative and quantitative description of the deformation, which made it possible to distinguish the effects of right and left chirality. Using suitable notations, we introduced engineering constants and expressed the solution in terms of the Young-type modulus and Poisson-type ratio. The results were of practical use and would be useful for testing mechanical properties, such as the stiffness and strength of materials with nanostructures.

The paper is structured as follows. First, we present the basic equations of chiral porous elastic solids and formulate the problem of thermoelastic deformation of the right circular cylinder. Then, we establish the solution with the help of two-dimensional problems. The solutions of special cases, such as chiral (non porous) cylinder and porous (non chiral) cylinder, are derived. Using appropriate notations the displacements, microdilatation function, and stress and strain fields are expressed in terms of engineering constants. The results and the effects of right and left chirality are discussed.

2. Preliminaries

In this section, we formulate the equilibrium problem of a porous chiral cylinder subjected to mechanical forces and a variation in temperature. The cylinder is supposed to be homogeneous and isotropic. We denote the lateral boundary by $\Pi$, the terminal cross-sections by ${\Sigma }_{\alpha }(\alpha =1,2)$, a generic cross-section by $\Sigma$, the boundary of ${\Sigma }_{\alpha }$ by ${\Gamma }_{\alpha }$, the boundary of $\Sigma$ by $\Gamma$, and the length of the cylinders by h. We chose a system of rectangular axes, such that $x_3-axis$ is parallel to the generator of the cylinder and the $x_{1}O{x}_2-plane$ contains the basis ${\Sigma }_1$ at $x_3=0$. Let $\mathbf{F}$ = $(F_1,F_2,F_3)$ and $\mathbf{M}$ = $(M_1,M_2,M_3)$ be the prescribed vector representing the resultant force and the resultant moment about O of the tractions acting on ${\Sigma }_1$. On ${\Sigma }_2$, there are tractions applied so as to satisfy the equilibrium conditions of the cylinder. We assume a thermal field $\mathbf{T}$ that is independent of the axial coordinate $x_3$. The cylinder is supposed to be free of lateral loading. Let $u_i$ be the components of the displacement vector and $\phi$ is the microdilatation function. The equilibrium problem of the cylinder consists of finding the functions $u_i$ and $\phi$, satisfying the following systems of equations:

geometric equations

$$e_{ij}=\frac{1}{2}(u_{i,j}+u_{j,i}),{\kappa }_{ijk}=u_{k,ij}$$

(1)

where $e_{ij}$ is the strain tensor and ${\kappa }_{ijk}$ is the strain gradient tensor.

constitutive equations

$$\begin{array}{cc}\hfill {\tau }_{ij}& =\lambda e_{rr}{\delta }_{ij}+2\mu e_{ij}+d\phi {\delta }_{ij}+f({\epsilon }_{ikm}{\kappa }_{jkm}+{\epsilon }_{jkm}{\kappa }_{ikm})-bT{\delta }_{ij},\hfill \\ \hfill {\mu }_{ijk}& =\frac{1}{2}{\alpha }_1({\kappa }_{rri}{\delta }_{jk}+2{\kappa }_{krr}{\delta }_{ij}+{\kappa }_{rrj}{\delta }_{ik})+{\alpha }_2({\kappa }_{irr}{\delta }_{jk}+{\kappa }_{jrr}{\delta }_{ik})\hfill \\ & +2{\alpha }_3{\kappa }_{rrk}{\delta }_{ij}+{\beta }_1{\delta }_{ij}{\phi }_{,k}+{\beta }_2({\delta }_{ik}{\phi }_{,j}+{\delta }_{jk}{\phi }_{,i})\hfill \\ & +2{\alpha }_4{\kappa }_{ijk}+{\alpha }_5({\kappa }_{kji}+{\kappa }_{kij})+f({\epsilon }_{iks}{e}_{js}+{\epsilon }_{jks}{e}_{is}),\hfill \\ \hfill {\sigma }_i& ={\beta }_1{\kappa }_{rri}+2{\beta }_2{\kappa }_{irr}+a_0{\phi }_{,i},g=d{e}_{rr}+\xi \phi -\beta T,\hfill \end{array}$$

(2)

where ${\tau }_{ij}$ is the stress tensor; ${\mu }_{ijk}$ is the dipolar stress tensor; ${\sigma }_i$ is the equilibrated stress vector; g is the intrinsic body force; T is the temperature; ${\delta }_{ij}$ is the Kronecker delta; ${\epsilon }_{ijk}$ is the alternating symbol; $\lambda ,$$\mu$ and b are the constitutive constants of the classical theory of elasticity; ${\alpha }_i(i=1,2,\dots ,5)$ and ${\beta }_j(j=1,2)$ are the constitutive constants associated with the gradient terms; $d$, $a_0$, $\xi$, and $\beta$ are constitutive constants linked to porosity; and f is a constant associated with the chiral behaviour.

equilibrium equations

$${\tau }_{ji,j}-{\mu }_{kji,kj}=0,{\sigma }_{j,j}-g=0.$$

(3)

The following boundary conditions

$$P_i=0,R_i=0,Q_i=0,{\sigma }_{\alpha }{n}_{\alpha }=0$$

(4)

must be satisfied on the lateral surface $\Pi$. Here, $(n_1,n_2,0)$ are the direction cosines of the exterior normal to $\Pi$ and

$$\begin{array}{cc}\hfill & P_i={\tau }_{3i}+2{\mu }_{\alpha 3i,\alpha }+{\mu }_{33i,3},R_i={\mu }_{33i}\mathrm{on}{\Sigma }_1,\hfill \\ \hfill & Q_i=-2{\mu }_{\alpha 3i}{n}_{\alpha },\mathrm{on}{\Gamma }_1.\hfill \end{array}$$

(5)

Further,

$$\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}{P}_{\alpha }da+{\int }_{{\Gamma }_1}{Q}_{\alpha }ds=F_{\alpha },\hfill \end{array}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}$$

(6)

$$\begin{array}{cc}& {\int }_{{\Sigma }_1}{P}_{3}da+{\int }_{{\Gamma }_1}{Q}_{3}ds=F_3,\hfill \end{array}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}$$

(7)

$$\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}(x_{\alpha }{P}_3+R_{\alpha })da+{\int }_{{\Gamma }_1}{x}_{\alpha }{Q}_{3}ds={\epsilon }_{\beta \alpha 3}{M}_{\beta },\hfill \end{array}$$

(8)

$$\begin{array}{cc}& {\int }_{{\Sigma }_1}{\epsilon }_{\alpha \beta 3}{x}_{\alpha }{P}_{\beta }da+{\int }_{{\Gamma }_1}{\epsilon }_{\alpha \beta 3}{x}_{\alpha }{Q}_{\beta }ds=M_3\hfill \end{array}$$

(9)

have to be satisfied on the end ${\Sigma }_1$.

3. Circular Cylinder

We consider a porous chiral circular cylinder of radius a subjected to the following external data

$$\mathbf{F}=(0,0,F_3),\mathbf{M}=(0,0,M_3),T=T^{*}$$

(10)

where $F_3$, $M_3$, and $T^{*}$ are the prescribed constants. The solutions of the problem under discussion have the form

$$\begin{array}{cc}\hfill u_{\alpha }& ={\epsilon }_{3\beta \alpha }{a}_2{x}_{\beta }{x}_3+a_1{u}_{\alpha }^{\left(1\right)}+a_2{u}_{\alpha }^{\left(2\right)}+w_{\alpha },\hfill \\ \hfill u_3& =a_1{x}_3+a_1{u}_3^{\left(1\right)}+a_2{u}_3^{\left(2\right)}+w_3,\hfill \\ \hfill \phi & =a_1{\phi }^{\left(1\right)}+a_2{\phi }^{\left(2\right)}+\psi ,\hfill \end{array}$$

(11)

where $u_i^{\left(\rho \right)}$, ${\phi }^{\left(\rho \right)}$, $w_1$, and $\psi$ are unknown functions, which are independent of $x_3$, and $a_1$ and $a_2$ are unknown constants. The functions $u_i^{\left(\rho \right)}$ and ${\phi }^{\left(\rho \right)}$ are the solution of two isotermal plane strain problems, denoted by $A^{\left(\rho \right)}$, and the functions $w_i$ and $\psi$ are the solution of a nonisothermal plane strain problem denoted by $A^{\left(0\right)}$. For brevity, the solutions of the problems $A^{\left(0\right)}$ and $A^{\left(\rho \right)}$ are provided directly omitting the calculations. We obtain

$$\begin{array}{cc}\hfill u_1^{\left(1\right)}& =c_1{x}_1,u_2^{\left(1\right)}=c_1{x}_2,u_3^{\left(1\right)}=0,{\phi }^{\left(1\right)}=c_2,\hfill \end{array}$$

(12)

$$\begin{array}{cc}\hfill u_1^{\left(2\right)}& =h_1{x}_1,u_2^{\left(2\right)}=h_1{x}_2,u_3^{\left(2\right)}=0,{\phi }^{\left(2\right)}=h_2,\hfill \end{array}$$

(13)

$$\begin{array}{cc}\hfill w_1& =s_1{x}_1,w_2=s_1{x}_2,w_3=0,\psi =s_2,\hfill \end{array}\hspace{1em}$$

(14)

where

$$\begin{array}{cc}\hfill c_1& =\frac{\lambda \xi -d^2}{D},c_2=\frac{2\mu d}{D},h_1=-2f\xi /D,h_2=4df/D\hfill \\ \hfill s_1& =\frac{\beta d-b\xi }{D}{T}^{*},s_2=\frac{2[db-\beta (\lambda +\mu \left)\right]}{D}{T}^{*}\hfill \\ \hfill D& =2[d^2-\xi (\lambda +\mu )].\hfill \end{array}$$

(15)

The strain and stress tensors associated with $(u_i^{\left(1\right)},{\phi }^{\left(1\right)})$ are provided by

$$\begin{array}{cc}\hfill e_{11}^{\left(1\right)}& =e_{22}^{\left(1\right)}=c_1,e_{12}^{\left(1\right)}=e_{13}^{\left(1\right)}=e_{23}^{\left(1\right)}=e_{33}^{\left(1\right)}=0,{\kappa }_{ijk}^{\left(1\right)}=0,\hfill \\ \hfill {\tau }_{11}^{\left(1\right)}& ={\tau }_{22}^{\left(1\right)}=2(\mu +\lambda )c_1+d{c}_2,{\tau }_{12}^{\left(1\right)}={\tau }_{13}^{\left(1\right)}={\tau }_{23}^{\left(1\right)}=0,\hfill \\ \hfill {\mu }_{\alpha \beta \gamma }^{\left(1\right)}& ={\mu }_{\alpha \beta 3}^{\left(1\right)}=0,{\sigma }_{\alpha }^{\left(1\right)}=0,g^{\left(1\right)}=2c_{1}d+c_2\hfill \end{array}$$

(16)

From relations (13) we obtain

$$\begin{array}{cc}\hfill e_{11}^{\left(2\right)}& =e_{22}^{\left(2\right)}=h,e_{12}^{\left(2\right)}=e_{13}^{\left(2\right)}=e_{23}^{\left(2\right)}=e_{33}^{\left(2\right)}=0,{\kappa }_{ijk}^{\left(2\right)}=0,\hfill \\ \hfill {\tau }_{11}^{\left(2\right)}& ={\tau }_{22}^{\left(2\right)}=2(\mu +\lambda )h_1+d{h}_2,{\tau }_{12}^{\left(2\right)}={\tau }_{13}^{\left(2\right)}={\tau }_{23}^{\left(2\right)}=0,\hfill \\ \hfill {\mu }_{\alpha \beta \gamma }^{\left(2\right)}& ={\mu }_{\alpha \beta 3}^{\left(2\right)}=0,{\sigma }_{\alpha }^{\left(2\right)}=0,g^{\left(2\right)}=2h_{1}d+h_2.\hfill \end{array}$$

(17)

The relations (14) provide

$$\begin{array}{cc}\hfill e_{11}^{\left(0\right)}& =e_{22}^{\left(0\right)}=s_1,e_{12}^{\left(0\right)}=e_{13}^{\left(0\right)}=e_{23}^{\left(0\right)}=e_{33}^{\left(0\right)}=0,{\kappa }_{ijk}^{\left(0\right)}=0,\hfill \\ \hfill {\tau }_{11}^{\left(0\right)}& ={\tau }_{22}^{\left(0\right)}=2(\mu +\lambda )s_1+d{s}_2-b{T}^{*},{\tau }_{12}^{\left(0\right)}={\tau }_{13}^{\left(0\right)}={\tau }_{23}^{\left(0\right)}=0,\hfill \\ \hfill {\mu }_{\alpha \beta \gamma }^{\left(0\right)}& ={\mu }_{\alpha \beta 3}^{\left(0\right)}=0,{\sigma }_{\alpha }^{\left(0\right)}=0,g^{\left(0\right)}=2s_{1}d+s_2-\beta T^{*}\hfill \end{array}$$

(18)

We have to determine the constants $a_1$ and $a_2$. In view of external data (10), the boundary conditions (6)–(9) became

$$\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}{P}_{\alpha }da+{\int }_{{\Gamma }_1}{Q}_{\alpha }ds=0,\hfill \end{array}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}$$

(19)

$$\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}{P}_{3}da+{\int }_{{\Gamma }_1}{Q}_{3}ds=F_3,\hfill \end{array}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}$$

(20)

$$\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}(x_{\alpha }{P}_3+R_{\alpha })da+{\int }_{{\Gamma }_1}{x}_{\alpha }{Q}_{3}ds=0,\hfill \end{array}$$

(21)

$$\hspace{1em}\begin{array}{cc}\hfill & {\int }_{{\Sigma }_1}{\epsilon }_{\alpha \beta 3}{x}_{\alpha }{P}_{\beta }da+{\int }_{{\Gamma }_1}{\epsilon }_{a\beta 3}{x}_{\alpha }{Q}_{\beta }ds=M_3.\hfill \end{array}$$

(22)

The conditions (19) and (21) in view of Equations (5), (16)–(18) are identically satisfied. Conditions (20) and (22) reduce to the following system

$$\begin{array}{cc}\hfill D_{11}{a}_1+D_{12}{a}_2& =-(F_3+F_3^{*}),\hfill \\ \hfill D_{21}{a}_1+D_{22}{a}_2& =-M_3\hfill \end{array}$$

(23)

where

$$\begin{array}{cc}\hfill D_{11}& =2\pi a^2\mu \frac{3d^2-\xi (2\mu +3\lambda )}{D},\hfill \\ \hfill D_{12}& =D_{21}=4\pi a^{2}f\frac{3d^2-\xi (2\mu +3\lambda )}{D},\hfill \\ \hfill D_{22}& =\mu \frac{\pi a^4}{2}+4(2{\alpha }_4-{\alpha }_5+\frac{2\xi f^2}{D})\pi a^2.\hfill \\ \hfill F_3^{*}& =-2\mu \pi a^2{s}_1.\hfill \end{array}$$

(24)

System (26) uniquely determines the constants $a_1$ and $a_2$. We have

$$\begin{array}{cc}\hfill a_1& =[-(F_3+F_3^{*})D_{22}-M_3{D}_{12}]/D^{*}\hfill \\ \hfill a_2& =[(F_3+F_3^{*})D_{12}-M_3{D}_{11}]/D^{*}\hfill \end{array}$$

(25)

where

$$D^{*}=D_{11}{D}_{22}-D_{12}^2.$$

(26)

The solution (11) can be rewritten in the form

$$\begin{array}{cc}\hfill u_1& =\frac{1}{D^{*}}\{-F_3[D_{12}{x}_2{x}_3+(c_1{D}_{22}-h_1{D}_{12})x_1]+M_3[D_{11}{x}_2{x}_3+(c_1{D}_{12}-h_1{D}_{11})x_1]\hfill \\ & +{\gamma }_1{T}^{*}[D_{12}{x}_2{x}_3+(c_1{D}_{22}-h_1{D}_{12}+\frac{D^{*}}{2\mu \pi a^2})x_1]\},\hfill \\ \hfill u_2& =\frac{1}{D^{*}}\{-F_3[-D_{12}{x}_1{x}_3+(c_1{D}_{22}-h_1{D}_{12})x_2]+M_3[-D_{11}{x}_1{x}_3+(c_1{D}_{12}-h_1{D}_{11})x_2]\hfill \\ \hfill & +{\gamma }_1{T}^{*}[-D_{12}{x}_1{x}_3+(c_1{D}_{22}-h_1{D}_{12}+\frac{D^{*}}{2\mu \pi a^2})x_2]\},\hfill \\ \hfill u_3& =\frac{1}{D^{*}}(-F_3{D}_{22}+M_3{D}_{12}+{\gamma }_1{T}^{*}{D}_{22})x_3\hfill \\ \hfill \phi & =\frac{1}{D^{*}}\{-F_3(c_2{D}_{22}-h_2{D}_{12})+M_3(c_2{D}_{12}-h_2{D}_{11})+T^{*}[{\gamma }_1(c_2{D}_{22}-h_2{D}_{12})+{\gamma }_2{D}^{*}]\hfill \end{array}$$

(27)

where

$${\gamma }_1=2\mu \pi a^2\frac{\beta d-b\xi }{D},{\gamma }_2=\frac{2[db-\beta (\lambda +\mu \left)\right]}{D}.$$

(28)

From the general solution (27), we derive the solutions of some special cases.

• chiral circular cylinder ($b=0,\beta =0$)

It follows from (15) and (28)

$$c_2=0,h_2=0,{\gamma }_2=0$$

(29)

so that $\phi =0$. From (27) and (29), we obtain

$$\begin{array}{cc}\hfill u_1& =F_3\{-\frac{2f}{\mu k_1}{x}_2{x}_3+[\frac{\lambda }{2\mu (2\mu +3\lambda )A}+\frac{2f^2}{{\mu }^2{k}_1}]x_1\}+M_3(\frac{1}{k_1}{x}_2{x}_3-\frac{f}{\mu k_1}{x}_1)\hfill \\ \hfill & +T^{*}b\{\frac{2fA}{(\mu +\lambda )k_1}{x}_2{x}_3+[\frac{1}{2\mu +3\lambda }-\frac{2f^{2}A}{\mu (\mu +\lambda )k_1}]x_1\}\hfill \\ \hfill u_2& =F_3\{\frac{2f}{\mu k_1}{x}_1{x}_3+[\frac{\lambda }{2\mu (2\mu +3\lambda )A}+\frac{2f^2}{{\mu }^2{k}_1}]x_2\}-M_3(\frac{1}{k_1}{x}_1{x}_3+\frac{f}{\mu k_1}{x}_2)\hfill \\ \hfill & +T^{*}b\{-\frac{2fA}{(\mu +\lambda )k_1}{x}_1{x}_3+[\frac{1}{2\mu +3\lambda }-\frac{2f^{2}A}{\mu (\mu +\lambda )k_1}]x_2\}\hfill \\ \hfill u_3& =\{-F_3[\frac{\mu +\lambda }{\mu (2\mu +3\lambda )A}+\frac{4f^2}{{\mu }^2{k}_1}]+M_3\frac{2f}{\mu k_1}+T^{*}b[\frac{1}{2\mu +3\lambda }+\frac{4f^{2}A}{\mu (\mu +\lambda )k_1}]\}{x}_3\hfill \end{array}$$

(30)

where $A=\pi a^2$, $I_0=\pi a^4/2$ and

$$k_1=\mu I_0+4(2{\alpha }_4-{\alpha }_5)A-\frac{12f^{2}A}{\mu }.$$

(31)

• porous circular cylinder($f=0$)

When the material is achiral, f is equal to zero. Moreover, we put the constitutive constants associated with the strain gradient equal to zero. These assumptions imply

$$\begin{array}{cc}\hfill D_{12}& =0,D_{22}=\mu I_0,D^{*}=D_{11}{D}_{22}{h}_1=h_2=0.\hfill \end{array}$$

(32)

Introducing Equations (32) into (27), we have the solution of an achiral circular cylinder in the theory of materials with voids.

$$\begin{array}{cc}\hfill u_1& =-\frac{F_3{c}_1}{D_{11}}{x}_1+\frac{M_3}{D_{22}}{x}_2{x}_3+T^{*}\frac{{\gamma }_1}{D_{11}}{x}_1\hfill \\ \hfill u_2& =-\frac{F_3{c}_1}{D_{11}}{x}_2-\frac{M_3}{D_{22}}{x}_1{x}_3+T^{*}\frac{{\gamma }_1}{D_{11}}{x}_2\hfill \\ \hfill u_3& =(-F_3+T^{*}{\gamma }_1)\frac{1}{D_{11}}{x}_3\hfill \\ \hfill \phi & =-\frac{F_3{c}_2}{D_{11}}+T^{*}(\frac{{\gamma }_1{c}_2}{D_{11}}+{\gamma }_2)\hfill \end{array}$$

(33)

where we used the relation

$$\frac{1}{D_{11}}=\frac{c_1}{D_{11}}+\frac{1}{2\mu \pi a^2}$$

(34)

4. Engineering Material Constants

In this section, solution (27), (30), and (33) are rewritten in terms of technical constants.

• porous achiral circular cylinder

We assume that the internal energy density is a positive definite form. This assumption implies that

$$\begin{array}{c}\hfill \mu >0,\xi >0,a_0>0,2\mu +3\lambda >0,\xi (2\mu +3\lambda )>3d^2\end{array}$$

(35)

We introduce the notations

$$E^{*}=\frac{\mu (2\mu +3{\lambda }^{*})}{\mu +{\lambda }^{*}},{\nu }^{*}=\frac{{\lambda }^{*}}{2(\mu +{\lambda }^{*})},$$

(36)

where

$${\lambda }^{*}=\lambda -d^2/\xi .$$

(37)

Of course

$${\lambda }^{*}<\lambda ,E^{*}<E,2\mu +3{\lambda }^{*}>0.$$

(38)

The elastic constants $E^{*}$ and ${\nu }^{*}$ are the counterpart of Young modulus and Poisson ratio in the theory of elastic materials with voids. The inverse relations of (36) are provided by

$$2\mu =\frac{E^{*}}{1+{\nu }^{*}},{\lambda }^{*}=\frac{{\nu }^{*}{E}^{*}}{(1+{\nu }^{*})(1-2{\nu }^{*})},2\mu +3{\lambda }^{*}=\frac{E^{*}}{1-2{\nu }^{*}}.$$

(39)

With the help of (36) and (39), we obtain

$$\begin{array}{cc}\hfill c_1& =-{\nu }^{*},c_2=-\frac{d}{\xi }(1-2{\nu }^{*}),\hfill \\ \hfill {\gamma }_1& =b^{*}(1-2{\nu }^{*})A,{\gamma }_2=\frac{2b^0(1+{\nu }^{*})(1-2{\nu }^{*})}{\xi E^{*}},\hfill \\ \hfill D_{11}& =A{E}^{*},D_{22}=I_0\frac{E^{*}}{2(1+{\nu }^{*})},D=-\frac{\xi E^{*}}{(1+{\nu }^{*})(1-2{\nu }^{*})},\hfill \end{array}$$

(40)

where

$$b^{*}=b-\frac{\beta d}{\xi },b^0=\beta (\lambda +\mu )-db=\beta (\mu +{\lambda }^{*})-b^{*}d.$$

(41)

Taking into account (40) and (41), relations (33) became

$$\begin{array}{cc}\hfill u_1& =\frac{1}{E^{*}}[\frac{F_3{\nu }^{*}}{A}{x}_1+\frac{2M_3(1+{\nu }^{*})}{I_0}{x}_2{x}_3+T^{*}{b}^{*}(1-2{\nu }^{*})x_1],\hfill \\ \hfill u_2& =\frac{1}{E^{*}}[\frac{F_3{\nu }^{*}}{A}{x}_2-\frac{2M_3(1+{\nu }^{*})}{I_0}{x}_1{x}_3+T^{*}{b}^{*}(1-2{\nu }^{*})x_2],\hfill \\ \hfill u_3& =\frac{1}{E^{*}}[-\frac{F_3}{A}+T^{*}{b}^{*}(1-2{\nu }^{*})]x_3,\hfill \\ \hfill \phi & =\frac{1-2{\nu }^{*}}{E^{*}\xi }[\frac{F_{3}d}{A}-(3b^{*}d-\frac{\beta E^{*}}{1-2{\nu }^{*}})T^{*}].\hfill \end{array}$$

(42)

From (1) and (42), the strain tensor is provided by

$$\begin{array}{cc}\hfill e_{11}& =e_{22}=\frac{1}{E^{*}}[\frac{F_3{\nu }^{*}}{A}+T^{*}{b}^{*}(1-2{\nu }^{*})],\hfill \\ \hfill e_{12}& =0,e_{33}=\frac{1}{E^{*}}[-\frac{F_3}{A}+T^{*}{b}^{*}(1-2{\nu }^{*})],\hfill \\ \hfill e_{13}& =\frac{M_3(1+{\nu }^{*})}{E^{*}{I}_0}{x}_2,e_{23}=-\frac{M_3(1+{\nu }^{*})}{E^{*}{I}_0}{x}_1\hfill \end{array}$$

(43)

From the constitutive Equations (2) and (43), we obtain

$$\begin{array}{cc}\hfill t_{11}& =t_{22}=t_{12}=0,t_{33}=-\frac{F_3}{A},\hfill \\ \hfill t_{13}& =\frac{M_3}{I_0}{x}_2,t_{23}=-\frac{M_3}{I_0}{x}_1\hfill \\ \hfill {\sigma }_1& ={\sigma }_2={\sigma }_3=0,g=0\hfill \end{array}$$

(44)

It should be mentioned that if in the Equation (42), we replace $E^{*}$ with E, ${\nu }^{*}$ with $\nu$, $b^{*}$ with b, and put $\phi =0$, we obtain the solution of the analogous problem in the classical theory of elasticity.

• chiral circular cylinder

Together with (31) and (45), the relations (30) yield

$$\begin{array}{cc}\hfill u_1& =F_3[-\frac{2}{d_1{k}_1}{x}_2{x}_3+(\frac{\nu }{EA}+\frac{2}{d_1^2{k}_1})x_1]+M_3(\frac{1}{k_1}{x}_2{x}_3-\frac{1}{d_1{k}_1}{x}_1)\hfill \\ \hfill & +T^{*}bA[\frac{2}{d_2{k}_1}{x}_2{x}_3+(\frac{1-2\nu }{EA}-\frac{2}{d_1{d}_2{k}_1})x_1]\hfill \\ \hfill u_2& =F_3[\frac{2}{d_1{k}_1}{x}_1{x}_3+(\frac{\nu }{EA}+\frac{2}{d_1^2{k}_1})x_2]-M_3(\frac{1}{k_1}{x}_1{x}_3+\frac{1}{d_1{k}_1}{x}_2)\hfill \\ \hfill & +T^{*}bA[-\frac{2}{d_2{k}_1}{x}_1{x}_3+(\frac{1-2\nu }{EA}-\frac{2}{d_1{d}_2{k}_1})x_2]\hfill \\ \hfill u_3& =[-F_3(\frac{1}{EA}+\frac{4}{d_1^2{k}_1})+M_3\frac{2}{d_1{k}_1}+T^{*}b(\frac{1-2\nu }{E}+\frac{4A}{d_1{d}_2{k}_1})]x_3,\hfill \end{array}$$

(45)

where

$$d_1=\frac{E}{2(1+\nu )f},d_2=\frac{E}{2(1+\nu )(1-2\nu )f}.$$

(46)

The strain tensor is provided by

$$\begin{array}{cc}\hfill e_{11}& =e_{22}=F_3\left(\frac{\nu }{EA}+\frac{2}{d_1^2{k}_1}\right)-M_3\frac{2}{d_1{k}_1}+T^{*}b\left(\frac{1-2\nu }{E}-\frac{2A}{d_1{d}_2{k}_1}\right),\hfill \\ \hfill e_{33}& =-F_3\left(\frac{1}{EA}+\frac{4}{d_1^2{k}_1}\right)+M_3\frac{2}{d_1{k}_1}+T^{*}b\left(\frac{1-2\nu }{E}+\frac{4A}{d_1{d}_2{k}_1}\right),e_{12}=0,\hfill \\ \hfill e_{13}& =\left(-\frac{F_3}{d_1}+\frac{M_3}{2}-\frac{T^{*}bA}{d_2}\right)\frac{x_2}{k_1},e_{23}=\left(\frac{F_3}{d_1}-\frac{M_3}{2}+\frac{T^{*}bA}{d_2}\right)\frac{x_1}{k_1}\hfill \end{array}$$

(47)

The components of the stress tensor are

$$\begin{array}{cc}\hfill t_{11}& =t_{22}=\frac{2f}{k_1}(\frac{2F_3}{d_1}-M_3-\frac{2b{T}^{*}A}{d_2}),\hfill \\ \hfill t_{33}& =-\left(\frac{F_3}{A}+2t_{11}\right)\hfill \\ \hfill t_{13}& =\{\frac{E}{2(1+\nu )}{M}_3+2f[-F_3+T^{*}bA(1-2\nu )]\}\frac{x_2}{k_1}{t}_{12}=0,\hfill \\ \hfill t_{23}& =\{-\frac{E}{2(1+\nu )}{M}_3-2f[-F_3+T^{*}bA(1-2\nu )]\}\frac{x_1}{k_1}\hfill \end{array}$$

(48)

• porous chiral circular cylinder

From (24), (26), and (40), we obtain

$$\begin{array}{cc}\hfill \frac{c_1{D}_{22}-h_1{D}_{12}}{D^{*}}& =-(\frac{{\nu }^{*}}{E^{*}A}+\frac{2}{{\widehat{d}}_1^2{k}_1}),\frac{c_1{D}_{12}-h_1{D}_{11}}{D^{*}}=-\frac{1}{{\widehat{d}}_1{k}_1},\hfill \\ \hfill \frac{c_2{D}_{12}-h_2{D}_{11}}{D^{*}}& =0,\frac{c_2{D}_{22}-h_2{D}_{12}}{D^{*}}=-\frac{(1-2{\nu }^{*})d}{\xi E^{*}A},\hfill \\ \hfill {\gamma }_1\left(\frac{c_1{D}_{22}-h_1{D}_{12}}{D^{*}}+\frac{1}{2\mu A}\right)& =b^{*}\left(\frac{1-2{\nu }^{*}}{E^{*}}-\frac{2A}{d_1{d}_2{k}_1}\right)\hfill \\ \hfill {\gamma }_1\left(\frac{c_2{D}_{22}-h_2{D}_{12}}{D^{*}}\right)+{\gamma }_2& =-\frac{3(1-2{\nu }^{*})b^{*}d}{\xi E^{*}}+\frac{\beta }{\xi }\hfill \end{array}$$

(49)

where

$${\widehat{d}}_1=\frac{E^{*}}{2(1+{\nu }^{*})f},{\widehat{d}}_2=\frac{E^{*}}{2(1+{\nu }^{*})(1-2{\nu }^{*})f}.$$

(50)

Substituting (49) into (27), we obtain

$$\begin{array}{cc}\hfill u_1& =F_3\left[-\frac{2}{{\widehat{d}}_1{k}_1}{x}_2{x}_3+(\frac{{\nu }^{*}}{E^{*}A}+\frac{2}{{\widehat{d}}_1^2{k}_1})x_1\right]+M_3\left(\frac{1}{k_1}{x}_2{x}_3-\frac{1}{{\widehat{d}}_1{k}_1}{x}_1\right)\hfill \\ \hfill & +T^{*}{b}^{*}A\left[\frac{2}{{\widehat{d}}_2{k}_1}{x}_2{x}_3+\left(\frac{1-2{\nu }^{*}}{E^{*}A}-\frac{2}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right)x_1\right]\hfill \\ \hfill u_2& =F_3\left[\frac{2}{{\widehat{d}}_1{k}_1}{x}_1{x}_3+\left(\frac{{\nu }^{*}}{E^{*}A}+\frac{2}{{\widehat{d}}_1^2{k}_1}\right)x_2\right]-M_3\left(\frac{1}{k_1}{x}_1{x}_3+\frac{1}{{\widehat{d}}_1{k}_1}{x}_2\right)\hfill \\ \hfill & +T^{*}{b}^{*}A\left[-\frac{2}{{\widehat{d}}_2{k}_1}{x}_1{x}_3+\left(\frac{1-2{\nu }^{*}}{E^{*}A}-\frac{2}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right)x_2\right]\hfill \\ \hfill u_3& =\left[-F_3\left(\frac{1}{E^{*}A}+\frac{4}{{\widehat{d}}_1^2{k}_1}\right)+\frac{2M_3}{{\widehat{d}}_1{k}_1}+T^{*}{b}^{*}A\left(\frac{1-2{\nu }^{*}}{E^{*}A}+\frac{4}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right)\right]x_3,\hfill \\ \hfill \phi & =\frac{1-2{\nu }^{*}}{E^{*}\xi }\left[\frac{F_{3}d}{A}+T^{*}\left(-3b^{*}d+\frac{\beta E^{*}}{1-2{\nu }^{*}}\right)\right].\hfill \end{array}$$

(51)

The strain tensor is easily determined from (51)

$$\begin{array}{cc}\hfill e_{11}& =e_{22}=F_3\left(\frac{{\nu }^{*}}{E^{*}A}+\frac{2}{{\widehat{d}}_1^2{k}_1}\right)-\frac{M_3}{{\widehat{d}}_1{k}_1}+T^{*}{b}^{*}A\left(\frac{1-2{\nu }^{*}}{E^{*}A}-\frac{2}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right),\hfill \\ \hfill e_{33}& =-F_3\left(\frac{1}{E^{*}A}+\frac{4}{{\widehat{d}}_1^2{k}_1}\right)+\frac{2M_3}{{\widehat{d}}_1{k}_1}+T^{*}{b}^{*}A\left(\frac{1-2{\nu }^{*}}{E^{*}A}+\frac{4}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right),e_{12}=0,\hfill \\ \hfill e_{13}& =\frac{1}{k_1}\left(-\frac{F_3}{{\widehat{d}}_1}+\frac{M_3}{2}+\frac{T^{*}{b}^{*}A}{{\widehat{d}}_2}\right)x_2,e_{23}=-\frac{1}{k_1}\left(-\frac{F_3}{{\widehat{d}}_1}+\frac{M_3}{2}+\frac{T^{*}{b}^{*}A}{{\widehat{d}}_2}\right)x_1.\hfill \end{array}$$

(52)

The stress tensor is provided by

$$\begin{array}{cc}\hfill t_{11}& =t_{22}=\frac{2f}{k_1}\left(\frac{2}{{\widehat{d}}_1}-M_3-\frac{2}{{\widehat{d}}_2}\right),\hfill \\ \hfill t_{33}& =-\frac{F_3}{A}-\frac{4f}{k_1}\left(\frac{2F_3}{{\widehat{d}}_1}-M_3+\frac{T^{*}{b}^{*}A}{{\widehat{d}}_2}\right)\hfill \\ \hfill t_{13}& {\displaystyle =\left\{{\displaystyle \frac{E^{*}}{2(1-{\nu }^{*})}{M}_3+2f\left[-\frac{F_3}{{\widehat{d}}_1}+T^{*}{b}^{*}A(1-2\nu )\right]}\right\}\frac{x_2}{k_1}}\hfill \\ \hfill t_{23}& {\displaystyle =\left\{{\displaystyle \frac{E^{*}}{2(1-{\nu }^{*})}{M}_3-2f\left[-\frac{F_3}{{\widehat{d}}_1}+T^{*}{b}^{*}A(1-2\nu )\right]}\right\}\frac{x_1}{k_1}}\hfill \\ \hfill t_{12}& =0{\sigma }_i=0,g=0\hfill \end{array}$$

(53)

5. Extensional and Torsional Rigidities

In [17], Mindlin and Eshel presented three forms for the potential energy-density in the case of centrosymmetric and isotropic materials, and derived the relations connecting the three forms. In the context of non-centrosymmetric strain gradient theory, Papanicolopulos [16] modified the forms II and III by adding a term that introduced chirality in the material behaviour. In this paper, we considered form I of the strain gradient theory. The energy-density function is provided by

$$\begin{array}{cc}\hfill W& {\displaystyle =\frac{1}{2}\lambda e_{ij}{e}_{ij}+\mu e_{ij}+{\alpha }_1{\kappa }_{iik}{\kappa }_{kjj}+{\alpha }_2{\kappa }_{ijj}{\kappa }_{ikk}+{\alpha }_3{\kappa }_{iik}{\kappa }_{jjk}+{\alpha }_4{\kappa }_{ijk}{\kappa }_{ijk}}\hfill \\ \hfill & +{\alpha }_5{\kappa }_{ijk}{\kappa }_{kji}+2f{ϵ}_{ijk}{\kappa }_{ijk}{e}_{jl}.\hfill \end{array}$$

(54)

Necessary and sufficient conditions for positive definiteness of W are

$$\begin{array}{cc}\hfill \mu & >0,2\mu +3\lambda >0,2{\alpha }_4-{\alpha }_5>0,{\alpha }_4+{\alpha }_5>0,\hfill \\ \hfill \frac{5}{3}({\alpha }_1+{\alpha }_2+{\alpha }_3)+{\alpha }_4+{\alpha }_5& >02(-2{\alpha }_1+{\alpha }_2+4{\alpha }_3)+3(2{\alpha }_4-{\alpha }_5)>0,\hfill \\ \hfill \frac{5}{6}{(-{\alpha }_1+2{\alpha }_2-4{\alpha }_3)}^2& <[2(-2{\alpha }_1+{\alpha }_2+4{\alpha }_3)+3(2{\alpha }_4-{\alpha }_5)][\frac{5}{3}({\alpha }_1+{\alpha }_2+{\alpha }_3)+{\alpha }_4+{\alpha }_5]\hfill \\ \hfill 12f^2& <\mu (2{\alpha }_4-{\alpha }_5).\hfill \end{array}$$

(55)

The quantity $k_1$ in Equation (31) is the torsional rigidity of the cylinder. It consists of three terms; the first term is the usual torsional rigidity in classical elasticity, the second term depends by the strain gradient theory, and the third negative term is due to the chirality of the material. From the last disequality of (55), it immediately follows that $k_1>0$. In contrast with the classical theory of elasticity, each mechanical or thermal action produces extension, torsion, and a deformation in the plane of the cross section of the cylinder. As consequence, in the case under discussion, we have three torsional rigidities and three extensional rigidities.

Table 1. Rigidities of the chiral circular cylinder.

	Torsional Rigidity	Extensional Rigidity
$F_3$	${\displaystyle \frac{1}{2}{d}_1{k}_1}$	${\left({\displaystyle \frac{1}{EA}+\frac{4}{d_1^2{k}_1}}\right)}^{-1}$
$M_3$	$k_1$	${\displaystyle \frac{1}{2}{d}_1{k}_1}$
$T^{*}$	${\displaystyle \frac{d_2{k}_1}{2bA}}$	${\displaystyle \frac{1}{b}{\left(\frac{1-2\nu }{E}+\frac{4A}{d_1{d}_2{k}_1}\right)}^{-1}}$

and

Table 2. Rigidities of chiral porous circular cylinder.

	Torsional Rigidity	Extensional Rigidity
$F_3$	${\displaystyle \frac{1}{2}{\widehat{d}}_1{k}_1}$	${\left({\displaystyle \frac{1}{E^{*}A}+\frac{4}{{\widehat{d}}_1^2{k}_1}}\right)}^{-1}$
$M_3$	$k_1$	${\displaystyle \frac{1}{2}{\widehat{d}}_1{k}_1}$
$T^{*}$	${\displaystyle \frac{{\widehat{d}}_2{k}_1}{2bA}}$	${\displaystyle \frac{1}{b}{\left(\frac{1-2{\nu }^{*}}{E^{*}}+\frac{4A}{{\widehat{d}}_1{\widehat{d}}_2{k}_1}\right)}^{-1}}$

show the rigidities corresponding to the forces $F_3$ and $M_3$, and to the temperature $T^{*}$.

The axial force $F_3$ rotates the cross-section by an angle

$${\displaystyle \theta \left(x_3\right)=\frac{4(1+\nu )f}{E{k}_1}{F}_3{x}_3,}$$

(56)

in the case of a chiral cylinder, and by an angle

$${\displaystyle \theta \left(x_3\right)=\frac{4(1+{\nu }^{*})f}{E^{*}{k}_1}{F}_3{x}_3,}$$

(57)

in the case of a porous chiral cylinder. The sign of $\theta$ depends on the sign of the product $F_{3}f$. If $F_{3}f>0$, the angle $\theta$ is positive (counterclockwise), otherwise if $F_{3}f<0$, $\theta$ is negative (clockwise), Figure 1a,b.

As a dual effect, the cylinder lengthens or shortens when twisted. If $M_{3}f>0$, the cylinder undergoes an elongation and a contraction of the cross-section. Vice versa, if $M_{3}f<0$, the torsion induces a shortening of the cylinder and a dilatation of the cross section, Figure 2a,b.

The effects of chirality vanish when $f=0$. In the case of a combined action of the axial force $F_3$ and the torque $M_3$, the angle of torsion $\theta$ is provided by

$${\displaystyle \theta \left(x_3\right)=\left({\displaystyle \frac{2F_3}{d_1}-M_3}\right)\frac{x_3}{k_1}.}$$

(58)

Immediately, we see that if $M_3$ is related to $F_3$, by the following relation

$${\displaystyle M_3=\frac{4(1+\nu )}{E}f{F}_3\left({\displaystyle M_3=\frac{4(1+{\nu }^{*})}{E^{*}}f{F}_3}\right),}$$

(59)

the angle $\theta$ is equal to 0 and the torsional effect produced by $F_3$ vanishes. Moreover, we denote by $\Delta l$ the variation in length of the axis of the cylinder. If relation (59) holds, from (45), we obtain

$${\displaystyle \Delta l=-\frac{F_{3}l}{EA}\left({\displaystyle \Delta l=-\frac{F_{3}l}{E^{*}A}}\right),}$$

(60)

and the extensional effect produced by $M_3$ disappears.

6. Conclusions

The results presented in this paper can be summarized as follows:

• We present the basic equations of the strain gradient theory of chiral porous thermoelastic solids and formulate the equilibrium problem of a homogeneous and isotropic circular cylinder subjected to a prescribed axial force and a torque acting on its bases. The cylinder is also under tha action of a constant temperature field.
• The analytical solution is determined through the help of two-dimensional problems. The solutions of a chiral (non porous) cylinder and a porous (non chiral) cylinder are derived as special cases.
• With the introduction of suitable notations, we define engineering constants, such as Young-type modulus and Poisson-type ratio, for chiral porous materials. Explicit formulas for the displacements, microdilatation function, stresses, and strain are written in terms of such engineering constants.
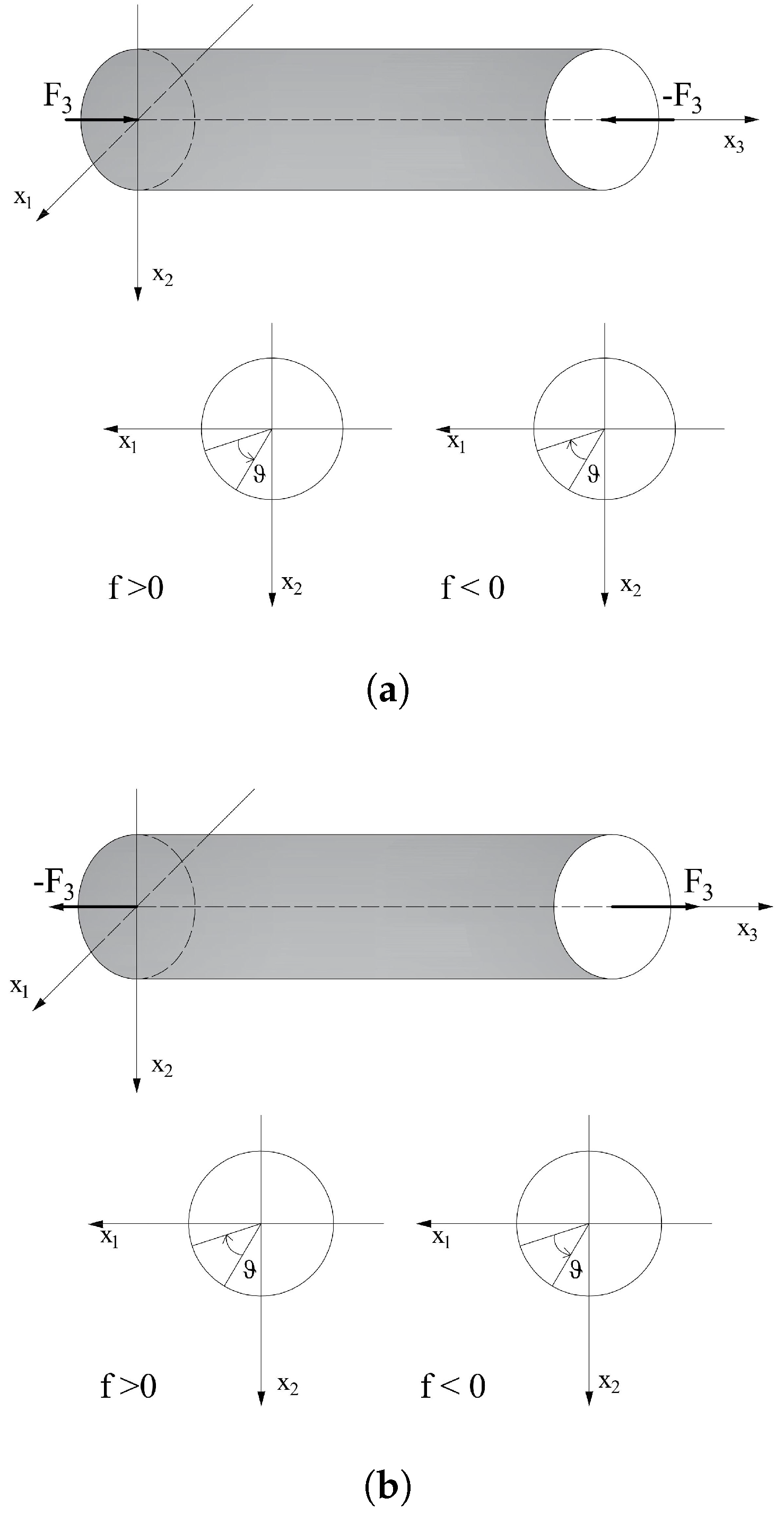
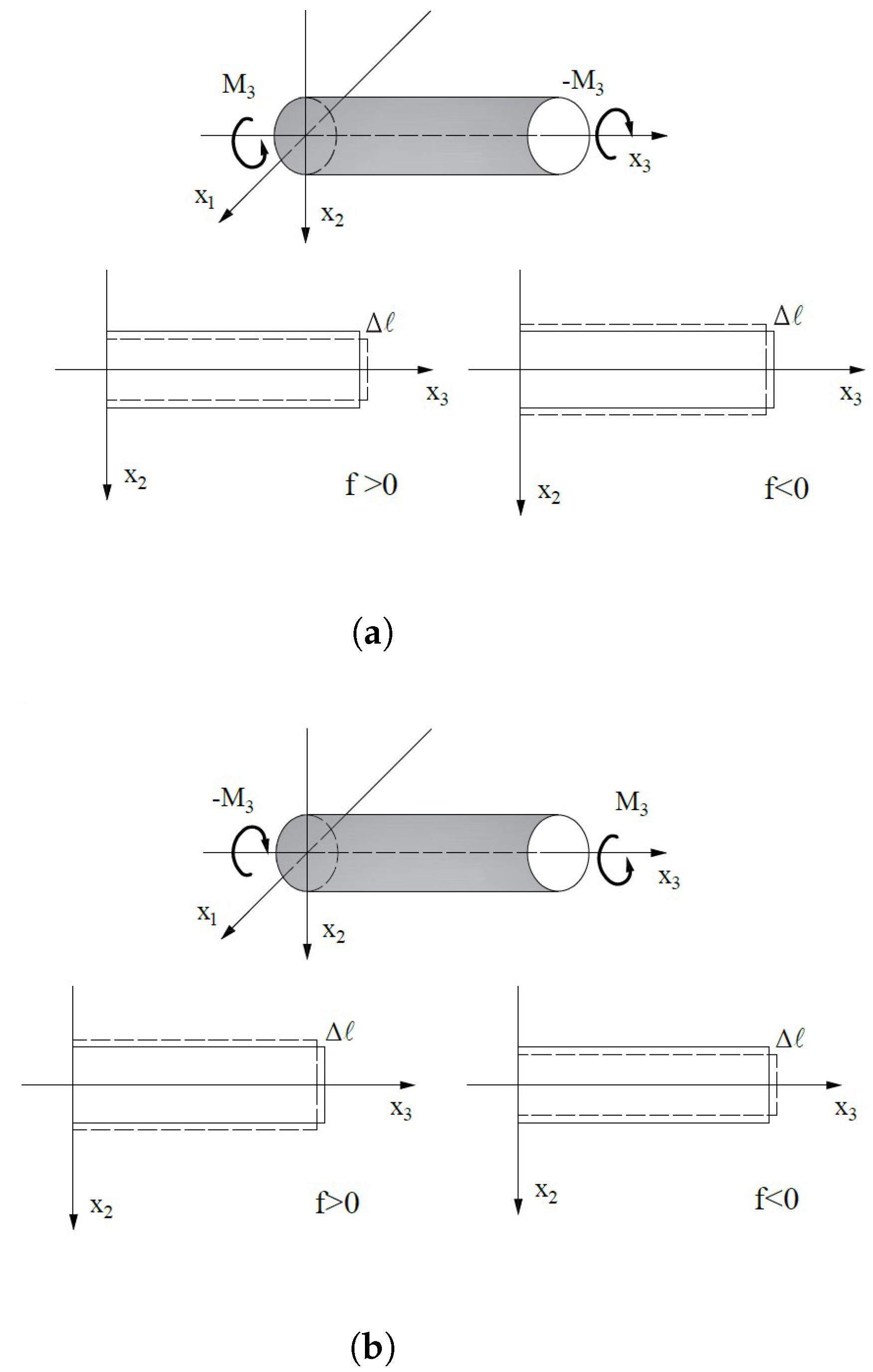
• The chirality is introduced in the constitutive equations by a material constant f. The sign of f may be positive or negative. We show that the cylinder is twisted by the axial force $F_3$ and the rotation will be counterclockwise if $F_{3}f>0$ or clockwise if $F_{3}f<0$. In addition, the torque $M_3$ produces extension. The cylinder lengthens or shortens depending on $M_{3}f>0$ or $M_{3}f<0$, respectively. Furthermore the cylinder is twisted by the variation in temperature $T^{*}$ and the sign of the product $f{T}^{*}$ discriminates between the two directions of the rotation.
• In contrast with the classical theory of elasticity, the rigidity in the torsion of a chiral beam is measured by three moduli. Similarly, we use three moduli for the extensional rigidity. These moduli are computed and are presented in Table 1 and Table 2.
• On the basis of the results presented in the paper, a possible next step will be to investigate the elastic deformation of a chiral porous circular beam under the action of a bending moment and a shear force. Bending and shear stiffness can be derived from the solutions of these problems.
• We show that the bending does not occur in a chiral circular cylinder under uniaxial force, although it is predicted by the solution for cylinders with an arbitrary cross-section. It might be interesting to solve the problem of a cylinder with a non circular section to study the coupling of deformation modes using extension-bending.
• Other important mechanical properties of chiral materials such as the ultimate tensile strength, elongation at rupture, fatigue properties, and so on need to be determined in various research areas, depending on the theoretical and experimental approaches used.