Monomiality and a New Family of Hermite Polynomials
Abstract
1. Introduction
- (a)
- (b)
- (c)
- It is possible to univocally define a polynomial set such that:then it follows that
- (d)
- (e)
- and the polynomials are said quasi-monomials.
2. Quasi-Hermite and Appéll Sequences
- (i)
- From property , we writewhich provides, from property ,
- (ii)
- and finally
- (iii)
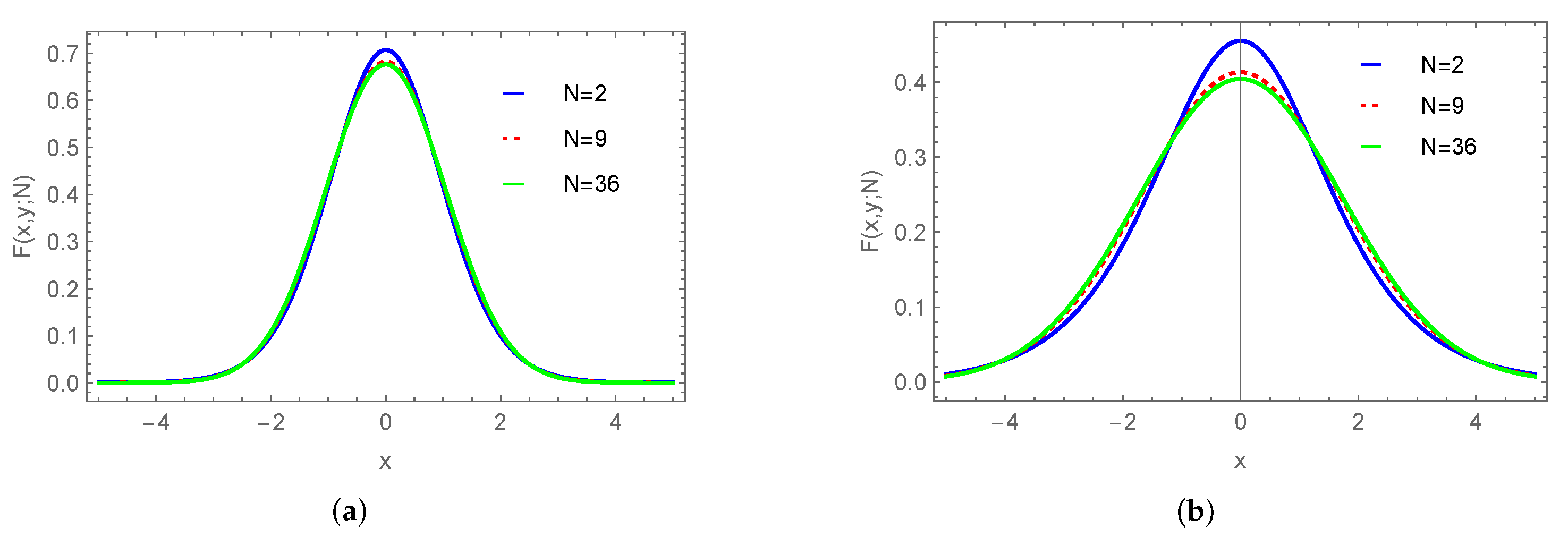
3. Multivariable QHP
4. Final Comments
- 1.
- 2.
- We use the two variable Hermite-generating functions (we have ) [38] to write
- 3.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wigner, E.P. Application of Group Theory to the Special Functions of Mathematical Physics; Lecture Notes; Princeton University: Princeton, NJ, USA, 1955. [Google Scholar]
- Vilenkin, N.J. Special Functions and the Theory of Group Representation; American Mathematical Society: Providence, RI, USA, 1968. [Google Scholar]
- Cartan, E. Sur la détermination d’un système orthogonal complet dans un espace de riemann symétrique clos. Rend. Circ. Matem. Palermo 1929, 53, 217–252. [Google Scholar] [CrossRef]
- Heisenberg, W. Erinnerungen and die Zeit der Entwicklung der Quantenmechanik. In Theoretical Physics in the Twentieth Century; A Memorial Volum to Wolfgang Pauli; Interscience: New York, NY, USA, 1960. [Google Scholar]
- Weyl, H. The Theory of Groups and Quantum Mechanics; Dover: New York, NY, USA, 1950. [Google Scholar]
- Wolf, K.B. The Heisenberg-Weyl ring in Quantum Mechanics. In Group Theory and Its Applications; Loebl, E.M., Ed.; Academic Press: New York, NY, USA, 1975. [Google Scholar]
- Thangavelu, S. Lectures on Hermite and Laguerre Expansions; Mathematical Notes; Princeton Univ. Press: Princeton, NJ, USA, 1993; Volume 42. [Google Scholar]
- Thangavelu, S. Harmonic Analysis on the Heisenberg Group; Birkhauser: Boston, MA, USA, 1998. [Google Scholar]
- Dattoli, G.; Torre, A.; Mazzacurati, G. An Alternative point of view to the theory of Fractional Fourier Transform. IMA J. Appl. Math. 1998, 60, 215–224. [Google Scholar] [CrossRef]
- Kim, Y.S.; Noz, M.E. Phase Space Picture of Quantum Mechanics: Group Theoretical Approach; World Scientific Lecture Notes in Physics; World Scientific: Singapore, 1991. [Google Scholar]
- Bogolubov, N.N.; Bogolubov, N.N., Jr. Introduction to Quantum Statistical Mechanics; World Scientific: Singapore, 1982. [Google Scholar] [CrossRef]
- Wilcox, R.M. Exponential Operators and Parameter Differentiation in Quantum Physics. J. Math. Phys. 1967, 8, 962–982. [Google Scholar] [CrossRef]
- Talman, J.D. Special Functions, a Group Theoretic Approach; Benjamin: New York, NY, USA, 1968. [Google Scholar]
- Eriksen, E. Properties of Higher-Order Commutator Products and the Baker-Campbell-Hausdorf formula. J. Math. Phys. 1968, 9, 790–796. [Google Scholar] [CrossRef]
- Dattoli, G.; Gallardo, J.C.; Torre, A. An algebraic view to the operatorial ordering and its applications to optics. Riv. Del Nuovo Cim. 1988, 11, 1–79. [Google Scholar] [CrossRef]
- Dattoli, G.; Loreto, V.; Mari, C.; Richetta, M.; Torre, A. Biunitary Transformations and Ordinary differential Equations. Nuovo Cim. 1991, 106B, 1357–1374. [Google Scholar] [CrossRef]
- Celeghini, E.; Gadella, M.; Dell’Olmo, M.A. Generalized Heisenberg-Weyl Groups and Hermite Functions. Symmetry 2021, 13, 1060. [Google Scholar] [CrossRef]
- Celeghini, E.; Gadella, M.; Dell’Olmo, M.A. Symmetry Groups, Quantum Mechanics and Generalized Hermite Functions. Mathematics 2022, 10, 1448. [Google Scholar] [CrossRef]
- Dattoli, G.; Levi, D.; Winternitz, P. Heisenberg algebra, umbral calculus and orthogonal polynomials. J. Math. Phys. 2008, 49, 053509. [Google Scholar] [CrossRef]
- Dattoli, G.; Torre, A.; Mazzacurati, G. Quasi Monomials and Isospectral Problems. Nuovo Cim. 1997, 112B, 133–138. [Google Scholar]
- Smirnov, Y.; Turbiner, A. Lie algebraic discretization of differential equations. Mod. Phys. Lett. A 1995, 10, 1795–1802. [Google Scholar] [CrossRef]
- Licciardi, S.; Dattoli, G. Guide to the Umbral Calculus, a Different Mathematical Language; World Scientific: Singapore, 2022. [Google Scholar]
- Roman, S.; Rota, G.C. The umbral calculus. Adv. Math. 1978, 27, 95–188. [Google Scholar] [CrossRef]
- Roman, S. The Umbral Calculus; Academic: New York, NY, USA, 1984. [Google Scholar]
- Rota, G.C. Finite Operator Calculus; Academic: New York, NY, USA, 1975. [Google Scholar]
- Di Bucchianico, A.; Loeb, D. A Selected Survey of Umbral Calculus. Electron. J. Comb. 2000, 2, 28. [Google Scholar]
- Appéll, P.; Kampé de Fériét, J. Fonctions Hypergeometriques and Hyperspheriques. Polynomes d’Hermite; Gauthiers-Villars: Paris, France, 1926. [Google Scholar]
- Bell, E.T. The History of Blissard’s Symbolic Method, with a Sketch of its Inventor’s Life. Am. Math. Mon. 1938, 45, 414–421. [Google Scholar] [CrossRef]
- Dattoli, G. Generalized polynomials, operational identities and their applications. J. Comput. Appl. Math. 2000, 118, 111–123. [Google Scholar] [CrossRef]
- Steffensen, J.F. Interpolation; The Williams & Wilkins Company: Baltimore, MD, USA, 1927. [Google Scholar]
- Steffensen, J.F. On the definition of the central factorial. J. Inst. Actuar. 1933, 64, 165–168. [Google Scholar] [CrossRef]
- Steffensen, J.F. The Poweroid, an Extension of the Mathematical Notion of Power. Acta Math. 1941, 73, 333–366. [Google Scholar] [CrossRef]
- Dowker, J.S. Poweroids revisited—An old symbolic approach. arXiv 2013, arXiv:1307.3150. [Google Scholar]
- Boole, G. Calculus of Finite Differences, 2nd ed.; MacMillan: Cambridge, MA, USA, 1872. [Google Scholar]
- Jordan, C. Calculus of Finite Differences, 3rd ed.; AMS Chelsea: New York, NY, USA, 1965. [Google Scholar]
- Dattoli, G. Hermite-Bessel and Laguerre-Bessel functions: A by-product of the monomiality principle. In Advanced Special Functions and Applications, Proceedings of the Melfi School on Advanced Topics in Mathematics and Physics, Melfi, Italy, 9–12 May 1999; Cocolicchio, D., Dattoli, G., Srivastava, H.M., Eds.; Aracne Editrice: Rome, Italy, 2000; pp. 147–164. [Google Scholar]
- Dattoli, G. Laguerre and generalized Hermite polynomials: The point of view of the operational method. Int. Trans. Spec. Funct. 2004, 15, 93–99. [Google Scholar] [CrossRef]
- Babusci, D.; Dattoli, G.; Licciardi, S.; Sabia, E. Mathematical Methods for Physics; World Scientific: Singapore, 2019. [Google Scholar]
- Abramovitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables, 9th ed.; Dover: New York, NY, USA, 1972. [Google Scholar]
- Dattoli, G.; Ottaviani, P.L.; Torre, A.; Vazquez, L. Evolution operator equations: Integration with algebraic and finitedifference methods. applications to physical problems in classical and quantum mechanics and quantum field theory. Riv. Nuovo Cim. 1997, 20, 3–133. [Google Scholar] [CrossRef]
- Louisell, W.H. Quantum Statistical Properties of Radiation; John Wiley and Sons: Hoboken, NJ, USA, 1973; Chapter 2. [Google Scholar]
- Sheffer, I.M. Some Properties of Polynomial Sets of Type Zero. Duke Math. J. 1939, 5, 590–622. [Google Scholar] [CrossRef]
- Boas, R.P.; Buck, R.C. Polynomial Expansions of Analytic Functions; Ergebnisse der Mathematik und ihrer Grenzgebiete. Neue Folge, 19; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1958. [Google Scholar]
- Andrews, L.C. Special Functions For Engeneers and Applied Mathematicians; Mc Millan: New York, NY, USA, 1985. [Google Scholar]
- Dattoli, G.; Germano, B.; Martinelli, M.R.; Ricci, P.E. Sheffer and Non-Sheffer Polynomial Families. Int. J. Math. Math. Sci. 2012, 2012, 323725. [Google Scholar] [CrossRef]
- Dattoli, G.; Zhukovsky, K. Appél Polynomial Series Expansions. Intern. Mathem. Forum 2010, 5, 649–662. [Google Scholar]
- Dattoli, G.; Khan, S.; Ricci, P.E. On Crofton-Glaisher type relations and derivation of generating functions for Hermite polynomials including the multi-index case. Int. Transf. Spec. Funct. 2008, 19, 1–9. [Google Scholar] [CrossRef]
- Dattoli, G.; Germano, B.; Ricci, P.E. Comments on monomiality, ordinary polynomials and associated bi-orthogonal functions. Appl. Math. Comput. 2004, 154, 219–227. [Google Scholar] [CrossRef]
- Dattoli, G.; Germano, B.; Ricci, P.E. Hermite polynomials with more than two variables and associated bi-orthogonal functions. Integr. Transf. Spec. Funct. 2009, 20, 17–22. [Google Scholar] [CrossRef]
- Appell, P. Sur une classe de polynômes. Ann. Sci. Éc. Norm. Supéer 1880, 9, 119–144. [Google Scholar] [CrossRef]
- Costabile, F.A.; Gualtieri, M.I.; Napoli, A. Towards the Centenary of Sheffer Polynomial Sequences: Old and Recent Results. Mathematics 2022, 10, 4435. [Google Scholar] [CrossRef]
- Dattoli, G.; Migliorati, M.; Srivastava, H. Sheffer polynomials, monomiality principle, algebraic methods and the theory of classical polynomials. Math. Comput. Model. 2007, 45, 1033–1041. [Google Scholar] [CrossRef]
- Dattoli, G.; Arena, A.; Ricci, P.E. The Laguerrian Derivative and Wright functions. Math. Comput. Model. 2004, 40, 877–881. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dattoli, G.; Licciardi, S. Monomiality and a New Family of Hermite Polynomials. Symmetry 2023, 15, 1254. https://doi.org/10.3390/sym15061254
Dattoli G, Licciardi S. Monomiality and a New Family of Hermite Polynomials. Symmetry. 2023; 15(6):1254. https://doi.org/10.3390/sym15061254
Chicago/Turabian StyleDattoli, Giuseppe, and Silvia Licciardi. 2023. "Monomiality and a New Family of Hermite Polynomials" Symmetry 15, no. 6: 1254. https://doi.org/10.3390/sym15061254
APA StyleDattoli, G., & Licciardi, S. (2023). Monomiality and a New Family of Hermite Polynomials. Symmetry, 15(6), 1254. https://doi.org/10.3390/sym15061254





