New Comparison Theorems to Investigate the Asymptotic Behavior of Even-Order Neutral Differential Equations

Abstract

Based on a comparison with first-order equations, we obtain new criteria for investigating the asymptotic behavior of a class of differential equations with neutral arguments. In this work, we consider the non-canonical case for an even-order equation. We concentrate on the requirements for excluding positive solutions, as the method used considers the symmetry between the positive and negative solutions of the studied equation. The results obtained do not require some restrictions that were necessary to apply previous relevant results in the literature.

1. Introduction

Differential equations (DEs) have been widely used in both pure and applied mathematics since they were first introduced in the middle of the 17th century. New connections between the various branches of mathematics, beneficial interactions with practical domains, and reformulations of fundamental problems and theories in diverse sciences have all led to a vast variety of new models and issues.

DEs can be used to simulate almost any physical, technological, or biological activity, including astronomical motion, the construction of bridges, and interactions between neurons. Most models that represent real phenomena and applications cannot have closed-form solutions. The available options in this case include finding approximate solutions or studying the qualitative properties of the solutions of these models, which include stability, symmetry, oscillation, periodicity, and others.

A type of functional differential equation known as “neutral differential equations (NDEs)” occurs when the highest derivative of the unknown function appears on the solution both with and without delay. NDEs are used to simulate a wide range of phenomena in many applied sciences, see [1].

The various differential models that have been proposed in various applied sciences have served as a great source of inspiration for research into the qualitative theory of DEs. According to this approach, the oscillation theory of DEs has made huge strides in recent decades, see [2,3,4,5]. Numerous authors have examined the oscillation of even-order differential equations and various methods for developing oscillatory criteria for these equations [6,7,8,9].

This study aims to create new conditions to investigate the asymptotic behavior of the even-order NDE

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }+\phi \left(s\right)x\left(\upsilon \left(s\right)\right)=0,$$

(1)

where $s\ge s_0,$ $n\ge 4$ are even integers, $w\left(s\right):=x\left(s\right)+\rho \left(s\right)x\left(\delta \left(s\right)\right),$ $a,$ $\rho \in {\mathbf{C}}^1\left(\left[s_0,\infty \right)\right),$ and $\delta ,$ $\upsilon ,$ $\phi \in \mathbf{C}\left(\left[s_0,\infty \right)\right)$. Furthermore, we suppose that $a^{\prime }\left(s\right)>0,$ $0<\rho \left(s\right)\le {\rho }_0<1,$ $\phi \ge 0,$ $\phi$ does not vanish eventually, $\delta \left(s\right)\le s,$ $\upsilon \left(s\right)\le s$, ${\upsilon }^{\prime }\left(s\right)\ge 0$, ${lim}_{s\to \infty }\delta \left(s\right)={lim}_{s\to \infty }\upsilon \left(s\right)=\infty$, and

$${\int }_{s_0}^{\infty }\frac{1}{a\left(\kappa \right)}\mathrm{d}\kappa <\infty .$$

For a solution to (1), we select a function $x\in \mathbf{C}\left(\left[s_x,\infty \right)\right),$ $s_x\ge s_0$, which has the properties $w\in {\mathbf{C}}^{\left(n-1\right)}\left(\left[s_x,\infty \right)\right)$ and $a{w}^{\left(n-1\right)}\in {\mathbf{C}}^1\left(\left[s_x,\infty \right)\right)$, and x satisfies (1) on $\left[s_x,\infty \right).$ We consider only those solutions to Equation (1) that will not vanish eventually. If a solution x of (1) is eventually positive or negative, then it is said to be non-oscillatory; otherwise, it is said to be oscillatory.

The oscillation theory, which has lately seen major growth and development, covers the study of oscillation for delay, neutral, mixed, and damping ordinary, fractional, and partial DEs. Second-order delay DEs have received the majority of attention in the literature, notably in the non-canonical case, see, for example, [10,11,12,13,14,15,16]. Recently, Bohner et al. [17] presented improved criteria for testing the oscillation of solutions of non-canonical second-order advanced differential equations.

In the non-canonical case, even-order delay DEs have gained more attention than neutral equations, see, for example, [6,7,18,19].

Li and Rogovchenko [20] considered the NDE

$${\left(a\left(s\right){\left(w^{\left(n-1\right)}\left(s\right)\right)}^{\alpha }\right)}^{\prime }+\phi \left(s\right)x^{\gamma }\left(\upsilon \left(s\right)\right)=0,$$

(2)

where $\alpha$ and $\gamma$ are ratios of odd positive integers. They obtained the oscillation criteria for Equation (2) by using comparison techniques and assuming three unknown functions that satisfy certain conditions. Moreover, the results in [20] required the following restrictions:

$${\delta }^{\prime }\left(s\right)\ge {\delta }_{*}>0\mathrm{and}\delta \circ \upsilon =\upsilon \circ \delta$$

(3)

Recently, Moaaz et al. [21] studied the asymptotic behavior of solutions to the NDE (1).

Theorem 1 

(Theorem 2.1 in [21]). Suppose that

$${\int }_{s_0}^{\infty }{\int }_l^{\infty }{\left(h-s\right)}^{n-3}\left(\frac{1}{a\left(h\right)}{\int }_{s_1}^h\phi \left(\kappa \right)\mathrm{d}\kappa \right)\mathrm{d}h\mathrm{d}l=\infty .$$

(4)

If there is an ${ϵ}_1\in \left(0,1\right)$ such that the delay DE

$${\psi }^{\prime }\left(s\right)+\frac{{ϵ}_1}{\left(n-1\right)!a\left(\upsilon \left(s\right)\right)}\phi \left(s\right)\left(1-\rho \left(\upsilon \left(s\right)\right)\right){\left[\upsilon \left(s\right)\right]}^{n-1}\psi \left(\upsilon \left(s\right)\right)=0$$

is oscillatory and the condition

$$\underset{s\to \infty }{limsup}{\int }_{s_0}^s\left(\frac{{ϵ}_1}{\left(n-2\right)!}{\upsilon }^{n-2}\left(\kappa \right)\phi \left(\kappa \right)\left(1-\rho \left(\upsilon \left(\kappa \right)\right)\right)\varphi \left(\kappa \right)-\frac{1}{4a\left(\kappa \right)\varphi \left(\kappa \right)}\right)\mathrm{d}\kappa =\infty$$

holds for some ${ϵ}_1\in \left(0,1\right)$, then all solutions of Equation (1) oscillate or converge to zero, where

$$\varphi \left(s\right)={\int }_s^{\infty }{a}^{-1}\left(\kappa \right)\mathrm{d}\kappa .$$

We provide helpful lemmas that will be applied throughout the results in the sections that follow.

Lemma 1

([22]). Suppose that $\psi \in {\mathbf{C}}^{m+1}\left(\left[s_0,\infty \right)\right),$ ${\psi }^{\left(j\right)}>0,$ for $j=0,1,...,m$ , and ${\psi }^{\left(m+1\right)}\le 0.$ Then, $\psi \left(s\right)\ge \frac{ϵs}{m}{\psi }^{\prime }\left(s\right),$ for all $ϵ\in \left(0,1\right)$.

Lemma 2

([4]). Suppose that $\psi \in {\mathbf{C}}^m(\left[s_0,\infty \right),\left(0,\infty \right))$, ${\psi }^{\left(m\right)}$ does not vanish eventually, and ${\psi }^{\left(m\right)}$ is of fixed sign. If ${\psi }^{\left(m-1\right)}{\psi }^{\left(m\right)}\le 0$ and${lim}_{s\to \infty }\psi \left(s\right)\ne 0,$ then, eventually,

$$\psi \left(s\right)\ge \frac{ϵ}{\left(m-1\right)!}{s}^{m-1}\left|{\psi }^{\left(m-1\right)}\left(s\right)\right|,$$

for every $ϵ\in (0,1).$

Lemma 3

(Lemma 1.2 in [23]). Suppose that ${\lambda }_1\ge 0$ and ${\lambda }_2>0$. Then,

$${\lambda }_1\psi -{\lambda }_2{\psi }^{\left(\alpha +1\right)/\alpha }\le \frac{{\alpha }^{\alpha }}{{\left(\alpha +1\right)}^{\alpha +1}}\frac{{\lambda }_1^{\alpha +1}}{{\lambda }_2^{\alpha }}.$$

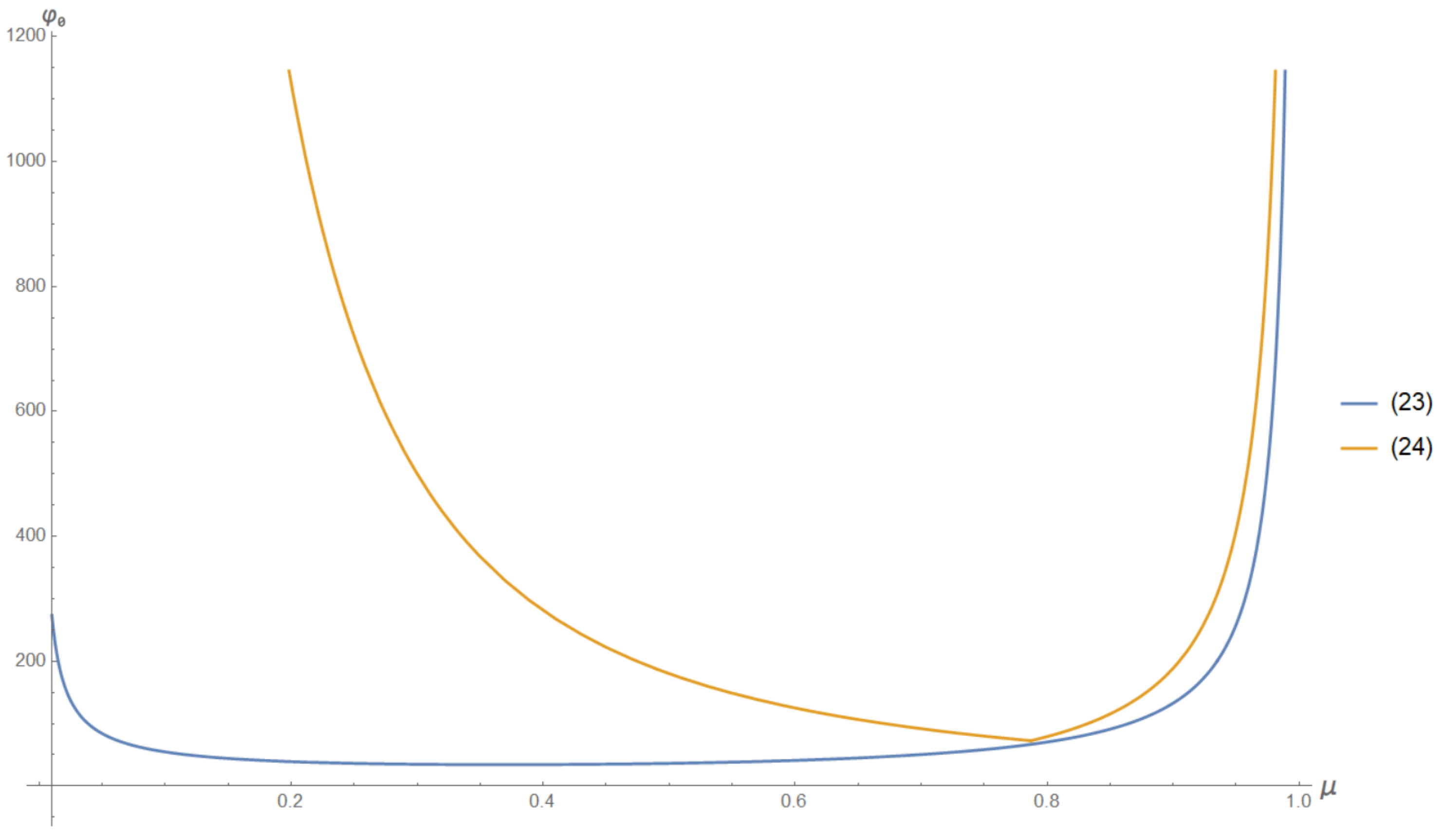
2. Main Results

For any eventually positive solution x to Equation (1), we find that the corresponding function w has one of the following cases, based on Lemma 1.1 in [22]:

Case 1

$w,$$w^{\prime },$ and $w^{\left(n-1\right)}$ are positive and $w^{\left(n\right)}$ is nonpositive;

Case 2

$w,$$w^{\prime },$ and $w^{\left(n-2\right)}$ are positive and $w^{\left(n-1\right)}$ is negative;

Case 3

${\left(-1\right)}^k{w}^{\left(k\right)}$ is positive for all $k=0,1,2,...,n-1.$

For ease, the symbol $S_i$ indicates the category of eventually positive solutions whose corresponding function satisfies case (i) for $i=1,2,3$. Moreover, we define

$$f^{\left[0\right]}\left(s\right):=s,f^{\left[m\right]}\left(s\right)=f\left(f^{\left[m-1\right]}\left(s\right)\right),\mathrm{for}m=1,2,...$$

and

$${\varphi }_0\left(s\right):={\int }_s^{\infty }\frac{1}{a\left(\kappa \right)}\mathrm{d}\kappa ,{\varphi }_j\left(s\right):={\int }_s^{\infty }{\varphi }_{j-1}\left(\kappa \right)\mathrm{d}\kappa ,\mathrm{for}j=1,...,n-2.$$

Lemma 4 

(Lemma 1 in [24]). Suppose that x is an eventually positive solution of (1). Then, eventually,

$$x\left(s\right)>\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}w\left({\delta }^{\left[2r\right]}\left(s\right)\right)-w\left({\delta }^{\left[2r+1\right]}\left(s\right)\right)\right],$$

(5)

for any integer $m\ge 0$.

Lemma 5. 

Suppose that $x\in S_1\cup S_2$. Then,

$$x\left(s\right)>\tilde{\rho }\left(s;m\right)w\left(s\right),$$

where

$$\tilde{\rho }\left(s;m\right):=\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}-1\right]{\left[\frac{{\delta }^{\left[2r\right]}\left(s\right)}{s}\right]}^{\left(n-1\right)/ϵ}.$$

Proof. 

Assume that $x\in S_1\cup S_2$. Then, assume that there is an integer $\ell \in \left[1,n-1\right]$ such that $w^{\left(\ell +1\right)}$ is the first nonpositive derivative of w. Using Lemma 1 with $\psi =w$ and $m=\ell$, we obtain $w\left(s\right)\ge \frac{ϵ}{\ell }s{w}^{\prime }\left(s\right)$ for all $ϵ\in \left(0,1\right)$. Thus,

$$\frac{\mathrm{d}}{\mathrm{d}s}\left(\frac{w}{s^{\ell /ϵ}}\right)=\frac{\ell }{ϵs^{\ell /ϵ+1}}\left[\frac{ϵ}{\ell }s{w}^{\prime }-w\right]\le 0.$$

Using this property with the fact that $w^{\prime }\left(s\right)>0$, we have that

$$w\left({\delta }^{\left[2r+1\right]}\left(s\right)\right)\le w\left({\delta }^{\left[2r\right]}\left(s\right)\right)$$

and

$$w\left({\delta }^{\left[2r\right]}\left(s\right)\right)\ge {\left[\frac{{\delta }^{\left[2r\right]}\left(s\right)}{s}\right]}^{\ell /ϵ}w\left(s\right)\ge {\left[\frac{{\delta }^{\left[2r\right]}\left(s\right)}{s}\right]}^{\left(n-1\right)/ϵ}w\left(s\right).$$

Hence, it follows from Lemma 4 that

$$x\left(s\right)>w\left(s\right)\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}-1\right]{\left[\frac{{\delta }^{\left[2r\right]}\left(s\right)}{s}\right]}^{\left(n-1\right)/ϵ}.$$

The proof is now complete. □

Lemma 6. 

Suppose that x is an eventually positive solution of (1),

$$\underset{s\to \infty }{liminf}{\int }_{\upsilon \left(s\right)}^s{Q}_1\left(\kappa \right)\mathrm{d}\kappa >\frac{1}{\mathrm{e}},$$

(6)

and

$$\underset{s\to \infty }{liminf}{\int }_{\upsilon \left(s\right)}^s{Q}_2\left(\kappa \right)\mathrm{d}\kappa >\frac{1}{\mathrm{e}},$$

(7)

where

$$Q_1\left(s\right):=\frac{1}{\left(n-1\right)!a\left(\upsilon \left(s\right)\right)}{\left[\upsilon \left(s\right)\right]}^{n-1}\phi \left(s\right)\tilde{\rho }\left(\upsilon \left(s\right);m\right)$$

and

$$Q_2\left(s\right):=\frac{1}{\left(n-2\right)!a\left(\upsilon \left(s\right)\right)}{\int }_{s_1}^{\upsilon \left(s\right)}\phi \left(\kappa \right)\tilde{\rho }\left(\upsilon \left(\kappa \right);m\right){\left[\upsilon \left(\kappa \right)\right]}^{n-2}\mathrm{d}\kappa .$$

Then, $x\in S_3$.

Proof. 

Suppose the contrary, i.e., that $x\in S_1\cup S_2$. From Lemma 5, we have that $x\left(s\right)>\tilde{\rho }\left(s;m\right)w\left(s\right)$. Thus, from (1), we arrive at

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le -\phi \left(s\right)\tilde{\rho }\left(\upsilon \left(s\right);m\right)w\left(\upsilon \left(s\right)\right).$$

(8)

Assume that $x\in S_1$. Using Lemma 2 with $\psi =w$ and $m=n$, we obtain

$$w\left(s\right)\ge \frac{ϵ}{\left(n-1\right)!}{s}^{n-1}{w}^{\left(n-1\right)}\left(s\right),$$

which with (8), gives

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }+\frac{ϵ}{\left(n-1\right)!}{\left[\upsilon \left(s\right)\right]}^{n-1}\phi \left(s\right)\tilde{\rho }\left(\upsilon \left(s\right);m\right)w^{\left(n-1\right)}\left(\upsilon \left(s\right)\right)\le 0.$$

Setting $V\left(s\right):=a\left(s\right)w^{\left(n-1\right)}\left(s\right)>0$, we obtain

$$V^{\prime }\left(s\right)+ϵQ_1\left(s\right)V\left(\upsilon \left(s\right)\right)\le 0.$$

(9)

Now, we have that V is a positive solution of (9). It follows from [25] (Theorem 1), that the equation

$$V^{\prime }\left(s\right)+ϵQ_1\left(s\right)V\left(\upsilon \left(s\right)\right)=0$$

(10)

has also a positive solution. Although, Theorem 2 in [26] asserts that condition (6) ensures the oscillation of Equation (10), which is a contradiction.

Assume that $x\in S_2$. Using Lemma 2 with $\psi =w$ and $m=n-1$, we obtain, for all $ϵ\in \left(0,1\right)$,

$$w\left(s\right)\ge \frac{ϵ}{\left(n-2\right)!}{s}^{n-2}{w}^{\left(n-2\right)}\left(s\right).$$

(11)

Integrating (8) from $s_1$ to s, we have

$$a\left(s\right)w^{\left(n-1\right)}\left(s\right)\le a\left(s_1\right)w^{\left(n-1\right)}\left(s_1\right)-{\int }_{s_1}^s\phi \left(\kappa \right)\tilde{\rho }\left(\upsilon \left(\kappa \right);m\right)w\left(\upsilon \left(\kappa \right)\right)\mathrm{d}\kappa ,$$

which with (11) gives

$$a\left(s\right)w^{\left(n-1\right)}\left(s\right)\le -\frac{ϵ}{\left(n-2\right)!}{\int }_{s_1}^s\phi \left(\kappa \right)\tilde{\rho }\left(\upsilon \left(\kappa \right);m\right){\left[\upsilon \left(\kappa \right)\right]}^{n-2}{w}^{\left(n-2\right)}\left(\upsilon \left(\kappa \right)\right)\mathrm{d}\kappa .$$

Since $w^{\left(n-1\right)}\left(s\right)<0$, we have that $w^{\left(n-2\right)}\left(\upsilon \left(\kappa \right)\right)\ge w^{\left(n-2\right)}\left(\upsilon \left(s\right)\right)$, and so

$$a\left(s\right)w^{\left(n-1\right)}\left(s\right)\le -\frac{ϵ}{\left(n-2\right)!}{w}^{\left(n-2\right)}\left(\upsilon \left(s\right)\right){\int }_{s_1}^s\phi \left(\kappa \right)\tilde{\rho }\left(\upsilon \left(\kappa \right);m\right){\left[\upsilon \left(\kappa \right)\right]}^{n-2}\mathrm{d}\kappa ,$$

or

$$w^{\left(n-1\right)}\left(\upsilon \left(s\right)\right)\le -\frac{ϵ}{\left(n-2\right)!a\left(\upsilon \left(s\right)\right)}{w}^{\left(n-2\right)}\left({\upsilon }^{\left[2\right]}\left(s\right)\right){\int }_{s_1}^{\upsilon \left(s\right)}\phi \left(\kappa \right)\tilde{\rho }\left(\upsilon \left(\kappa \right);m\right){\left[\upsilon \left(\kappa \right)\right]}^{n-2}\mathrm{d}\kappa$$

Setting $U\left(s\right):=w^{\left(n-2\right)}\left(\upsilon \left(s\right)\right)>0$, we obtain

$$U^{\prime }\left(s\right)+ϵQ_2\left(s\right)U\left(\upsilon \left(s\right)\right)\le 0.$$

(12)

Now, we have that U is a positive solution of (12). It follows from [25] (Theorem 1) that the equation

$$U^{\prime }\left(s\right)+ϵQ_2\left(s\right)U\left(\upsilon \left(s\right)\right)=0$$

(13)

also has a positive solution. Although, Theorem 2 in [26] asserts that condition (7) ensures the oscillation of Equation (13), which is a contradiction.

Therefore, $x\in S_3$. The proof is now complete. □

2.1. Criteria for Convergence of Non-Oscillatory Solutions to Zero

Theorem 2. 

Suppose that (6) and (7) hold. If

$${\int }_{s_0}^{\infty }{\int }_h^{\infty }\frac{{\left(l-s\right)}^{n-3}}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l\mathrm{d}h=\infty ,$$

(14)

then all solutions of Equation (1) oscillate or converge to zero.

Proof. 

Assume the contrary, i.e., that x is an eventually positive solution of (1). From Lemma 6, we have $x\in S_3$. Since $w\left(s\right)>0$ and $w^{\prime }\left(s\right)<0$, we have that ${lim}_{s\to \infty }w\left(s\right)=c\ge 0$. Assume that $c>0$. Then, there is a $s_1\ge s_0$ such that $c-ϵ<w\left(s\right)<c+ϵ$ for all $s\ge s_1$ and $ϵ>0$. By choosing $ϵ<\frac{1-{\rho }_0}{1+{\rho }_0}c$, we get that

$$x\left(s\right)>w\left(s\right)-{\rho }_{0}w\left(\delta \left(s\right)\right)>\left(1-{\rho }_0\right)c-\left(1+{\rho }_0\right)ϵ>Lc,$$

where $L=\frac{\left(1-{\rho }_0\right)c-\left(1+{\rho }_0\right)ϵ}{c}>0$. Hence, (1) becomes

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le -Lc\phi \left(s\right).$$

(15)

Integrating (15) from $s_1$ to s, we get

$$a\left(s\right)w^{\left(n-1\right)}\left(s\right)\le a\left(s_1\right)w^{\left(n-1\right)}\left(s_1\right)-Lc{\int }_{s_1}^s\phi \left(\kappa \right)\mathrm{d}\kappa ,$$

and so

$$w^{\left(n-1\right)}\left(s\right)\le -\frac{Lc}{a\left(s\right)}{\int }_{s_1}^s\phi \left(\kappa \right)\mathrm{d}\kappa$$

(16)

Integrating (16) twice from s to ∞, we get

$$w^{\left(n-2\right)}\left(s\right)\ge Lc{\int }_s^{\infty }\frac{1}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l,$$

and

$$\begin{array}{ccc}\hfill w^{\left(n-3\right)}\left(s\right)& \le & -Lc{\int }_s^{\infty }{\int }_h^{\infty }\frac{1}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l\mathrm{d}h\hfill \\ & =& -Lc{\int }_s^{\infty }\frac{\left(l-s\right)}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l.\hfill \end{array}$$

(17)

Integrating (17) $n-4$ times from s to ∞, we obtain

$$w^{\prime }\left(s\right)\le -Lc{\int }_s^{\infty }\frac{{\left(l-s\right)}^{n-3}}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l.$$

Integrating this inequality from $s_1$ to ∞, we obtain

$$w\left(s_1\right)\ge Lc{\int }_{s_1}^{\infty }{\int }_h^{\infty }\frac{{\left(l-s\right)}^{n-3}}{a\left(l\right)}{\int }_{s_1}^l\phi \left(\kappa \right)\mathrm{d}\kappa \mathrm{d}l\mathrm{d}h,$$

which contradicts (14). Then, $c=0$ and hence ${lim}_{s\to \infty }x\left(s\right)=0$. The proof is now complete. □

In the following theorem, we prove that the nonoscillatory solutions of Equation (1) converge to zero without using an additional condition such as condition (14) in Theorem 2.

Theorem 3. 

Suppose that

$$\underset{s\to \infty }{liminf}{\int }_{\upsilon \left(s\right)}^s{\tilde{Q}}_1\left(\kappa \right)\mathrm{d}\kappa >\frac{1}{\mathrm{e}}$$

(18)

and

$$\underset{s\to \infty }{liminf}{\int }_{\upsilon \left(s\right)}^s{\tilde{Q}}_2\left(\kappa \right)\mathrm{d}\kappa >\frac{1}{\mathrm{e}},$$

(19)

where

$${\tilde{Q}}_1\left(s\right):=\frac{1}{\left(n-1\right)!a\left(\upsilon \left(s\right)\right)}{\left[\upsilon \left(s\right)\right]}^{n-1}{Q}_3\left(s\right),$$

$${\tilde{Q}}_2\left(s\right):=\frac{1}{\left(n-2\right)!a\left(s\right)}{\int }_{s_1}^s{\left[\upsilon \left(\kappa \right)\right]}^{n-2}{Q}_3\left(s\right)\mathrm{d}\kappa ,$$

and

$$Q_3\left(s\right):=\phi \left(s\right)\left(1-\rho \left(\upsilon \left(s\right)\right)\frac{{\varphi }_{n-2}\left(\delta \left(\upsilon \left(s\right)\right)\right)}{{\varphi }_{n-2}\left(\upsilon \left(s\right)\right)}\right).$$

Then, all solutions of Equation (1) oscillate or converge to zero.

Proof. 

Assume the contrary, i.e., that x is an eventually positive solution of (1) and ${lim}_{s\to \infty }x\left(s\right)\ne 0$. From the fact that $w^{\prime }$ is of fixed sign, we have that w is increasing or decreasing.

Assume that w is increasing. Since ${\varphi }_{n-2}^{\prime }\left(s\right)\le 0$, we get that ${\varphi }_{n-2}\left(\delta \left(s\right)\right)\ge {\varphi }_{n-2}\left(s\right)$ and

$$1-\rho \left(s\right)\ge 1-\rho \left(s\right)\frac{{\varphi }_{n-2}\left(\delta \left(s\right)\right)}{{\varphi }_{n-2}\left(s\right)}.$$

Thus,

$$x\left(s\right)>\left(1-\rho \left(s\right)\frac{{\varphi }_{n-2}\left(\delta \left(s\right)\right)}{{\varphi }_{n-2}\left(s\right)}\right)w\left(s\right).$$

(20)

Assume that w is decreasing. Then, ${\left(-1\right)}^k{w}^{\left(k\right)}$ are positive for all $k=0,1,2,...,n-1$. Using the fact that ${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le 0$, we obtain

$$-w^{\left(n-2\right)}\left(s\right)\le {\int }_s^{\infty }\frac{a\left(\kappa \right)w^{\left(n-1\right)}\left(\kappa \right)}{a\left(\kappa \right)}\mathrm{d}\kappa \le {\varphi }_0\left(s\right)a\left(s\right)w^{\left(n-1\right)}\left(s\right).$$

Then, ${\left(w^{\left(n-2\right)}/{\varphi }_0\right)}^{\prime }\ge 0$, and so

$$-w^{\left(n-3\right)}\left(s\right)\ge {\int }_s^{\infty }\frac{w^{\left(n-2\right)}\left(\kappa \right)}{{\varphi }_0\left(\kappa \right)}{\varphi }_0\left(\kappa \right)\mathrm{d}\kappa \ge \frac{{\varphi }_1\left(s\right)}{{\varphi }_0\left(s\right)}{w}^{\left(n-2\right)}\left(s\right).$$

By repeating this procedure, we arrive at ${\left(w/{\varphi }_{n-2}\right)}^{\prime }\ge 0$. Using this property, we get that (20) holds. Therefore, Equation (1) becomes

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le -Q_3\left(s\right)w\left(\upsilon \left(s\right)\right).$$

(21)

Now, we classify the positive solutions of Equation (1) into the following only two categories:

(C1)

w and $w^{\left(n-1\right)}$ are positive and $w^{\left(n\right)}$ is non-positive;

(C2)

w and $w^{\left(n-2\right)}$ are positive and $w^{\left(n-1\right)}$ is negative.

By following the same approach as in Lemma 6 and using inequality (21) instead of (8), we get the required result.

The proof is now complete. □

Example 1. 

Consider the NDE

$${\left(s^4{\left[x\left(s\right)+{\rho }_{0}x\left(\lambda s\right)\right]}^{‴}\right)}^{\prime }+{\phi }_{0}x\left(\mu s\right)=0,$$

(22)

where ${\rho }_0\in \left(0,1\right),$ $\lambda ,$ $\mu \in \left(0,1\right],$ and ${\phi }_0>0$. We find ${\delta }^{\left[2r\right]}\left(s\right)={\lambda }^{2r}s,$

$$\tilde{\rho }\left(s;m\right)=\left[\frac{1}{{\rho }_0}-1\right]\sum _{r=0}^m{\rho }_0^{2r+1}{\lambda }^{6r/ϵ}:={\tilde{\rho }}_0,$$

$$Q_1\left(s\right)=\frac{{\tilde{\rho }}_0{\phi }_0}{6\mu }\frac{1}{s},$$

and

$$Q_2\left(s\right)=\frac{{\phi }_0{\tilde{\rho }}_0\mu }{6}\frac{1}{s}.$$

It is easy to verify that (14) is satisfied. Conditions (6) and (7) reduce to

$$\frac{{\tilde{\rho }}_0{\phi }_0}{6\mu }ln\frac{1}{\mu }>\frac{1}{\mathrm{e}}$$

and

$$\frac{{\phi }_0{\tilde{\rho }}_0\mu }{6}ln\frac{1}{\mu }>\frac{1}{\mathrm{e}},$$

respectively. Therefore, using Theorem 2, all solutions of Equation (22) oscillate or converge to zero if

$${\phi }_0>\frac{6}{\mathrm{e}{\tilde{\rho }}_0\mu ln\left(1/\mu \right)}.$$

(23)

Remark 1. 

By applying Theorem 1, we obtain that all solutions of Equation (22) oscillate or converge to zero if

$${\phi }_0>max\left\{\frac{6\mu }{\mathrm{e}\left(1-{\rho }_0\right)ln\left(1/\mu \right)},\frac{9}{2{\mu }^2\left(1-{\rho }_0\right)}\right\}.$$

(24)

Consider the following special case of (22):

$${\left(s^4{\left[x\left(s\right)+\frac{9}{10}x\left(\frac{9s}{10}\right)\right]}^{‴}\right)}^{\prime }+{\phi }_{0}x\left(\mu s\right)=0.$$

If $\mu =0.5$, then conditions (23) and (24) reduce to ${\phi }_0>36.532$ and ${\phi }_0>180.0$, respectively. Figure 1 shows the minimum values of the parameter ${\phi }_0$ for all values of $\mu \in \left(0,1\right)$ for conditions (23) and (24). Thus, our results improve the results in [21].

2.2. Oscillation Criteria for All Solutions

In the next section, we present criteria that test the oscillation of all solutions of the considered equation. For this, we need the following constraint:

$$\frac{1}{\rho \left(s\right)}>\frac{{\varphi }_{n-2}\left(\delta \left(s\right)\right)}{{\varphi }_{n-2}\left(s\right)}$$

Lemma 7 

(Lemma 2, Lemma 3 in [27]). Suppose that $x\in S_3$. Then,

$${\left(-1\right)}^{i+1}{w}^{\left(i\right)}\left(s\right)\le \left[a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right]{\varphi }_{n-i-2}\left(s\right)$$

and

$${\left(-1\right)}^i{\left(\frac{w^{\left(i\right)}\left(s\right)}{{\varphi }_{n-i-2}\left(s\right)}\right)}^{\prime }\ge 0$$

eventually for $i=0,1,...,n-2$.

Lemma 8. 

Suppose that $x\in S_3$. Then,

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le -\phi \left(s\right)\widehat{\rho }\left(\upsilon \left(s\right);m\right)w\left(\upsilon \left(s\right)\right),$$

(25)

where

$$\widehat{\rho }\left(s;m\right):=\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}-\frac{{\varphi }_{n-2}\left({\delta }^{\left[2r+1\right]}\left(s\right)\right)}{{\varphi }_{n-2}\left({\delta }^{\left[2r\right]}\left(s\right)\right)}\right].$$

Proof. 

It follows from Lemma 4 that (5) holds. From Lemma 7, we have $w/{\varphi }_{n-2}\left(s\right)$ is increasing, and so

$$w\left({\delta }^{\left[2r+1\right]}\right)\le \frac{{\varphi }_{n-2}\left({\delta }^{\left[2r+1\right]}\right)}{{\varphi }_{n-2}\left({\delta }^{\left[2r\right]}\right)}w\left({\delta }^{\left[2r\right]}\right).$$

Thus, (5) becomes

$$x\left(s\right)>\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}-\frac{{\varphi }_{n-2}\left({\delta }^{\left[2r+1\right]}\left(s\right)\right)}{{\varphi }_{n-2}\left({\delta }^{\left[2r\right]}\left(s\right)\right)}\right]w\left({\delta }^{\left[2r\right]}\left(s\right)\right).$$

Since z is decreasing, we obtain

$$x\left(s\right)>w\left(s\right)\sum _{r=0}^m\left(\prod _{l=0}^{2r}\rho \left({\delta }^{\left[l\right]}\left(s\right)\right)\right)\left[\frac{1}{\rho \left({\delta }^{\left[2r\right]}\left(s\right)\right)}-\frac{{\varphi }_{n-2}\left({\delta }^{\left[2r+1\right]}\left(s\right)\right)}{{\varphi }_{n-2}\left({\delta }^{\left[2r\right]}\left(s\right)\right)}\right],$$

which with Equation (1) gives

$${\left(a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right)}^{\prime }\le -\phi \left(s\right)\widehat{\rho }\left(\upsilon \left(s\right);m\right)w\left(\upsilon \left(s\right)\right).$$

The proof is now complete. □

Theorem 4. 

Suppose that (6) and (7) hold. If

$$\underset{s\to \infty }{liminf}{\int }_{\upsilon \left(s\right)}^s\left[{\varphi }_{n-3}\left(u\right){\int }_{s_1}^u\phi \left(\kappa \right)\widehat{\rho }\left(\upsilon \left(\kappa \right);m\right)\mathrm{d}\kappa \right]\mathrm{d}u>\frac{1}{\mathrm{e}},$$

(26)

then all solutions of Equation (1) are oscillatory.

Proof. 

Assume the contrary, i.e., that x is an eventually positive solution of (1). From Lemma 6, we have $x\in S_3$. Using Lemma 8, we get (25). Integrating (25) from $s_1$ to s, we arrive at

$$\begin{array}{ccc}\hfill a\left(s\right)w^{\left(n-1\right)}\left(s\right)& \le & -{\int }_{s_1}^s\phi \left(s\right)\widehat{\rho }\left(\upsilon \left(\kappa \right);m\right)w\left(\upsilon \left(\kappa \right)\right)\mathrm{d}\kappa \hfill \\ & \le & -w\left(\upsilon \left(s\right)\right){\int }_{s_1}^s\phi \left(\kappa \right)\widehat{\rho }\left(\upsilon \left(\kappa \right);m\right)\mathrm{d}\kappa .\hfill \end{array}$$

It follows from Lemma 7 that $w^{\prime }\left(s\right)\le \left[a\left(s\right)w^{\left(n-1\right)}\left(s\right)\right]{\varphi }_{n-3}\left(s\right)$, and so

$$w^{\prime }\left(s\right)+w\left(\upsilon \left(s\right)\right){\varphi }_{n-3}\left(s\right){\int }_{s_1}^s\phi \left(\kappa \right)\widehat{\rho }\left(\upsilon \left(\kappa \right);m\right)\mathrm{d}\kappa \le 0.$$

(27)

Therefore, w is a positive solution of (27). It follows from [25] (Theorem 1), that the equation

$$w^{\prime }\left(s\right)+w\left(\upsilon \left(s\right)\right){\varphi }_{n-3}\left(s\right){\int }_{s_1}^s\phi \left(\kappa \right)\widehat{\rho }\left(\upsilon \left(\kappa \right);m\right)\mathrm{d}\kappa =0$$

(28)

also has a positive solution. Although, Theorem 2 in [26] asserts that condition (26) ensures the oscillation of Equation (28), which is a contradiction.

The proof is now complete. □

Example 2. 

Consider NDE (22), where ${\rho }_0<\lambda$. We find ${\varphi }_0\left(s\right)=1/\left(3s^3\right)$, ${\varphi }_1\left(s\right)=1/\left(6s^2\right)$, ${\varphi }_1\left(s\right)=1/\left(12s\right)$, and

$$\widehat{\rho }\left(s;m\right):=\left[1-\frac{{\rho }_0}{\lambda }\right]\sum _{r=0}^m{\rho }_0^{2r}={\widehat{\rho }}_0.$$

Condition (26) reduces to

$$\frac{1}{6}{\widehat{\rho }}_0{\phi }_{0}ln\frac{1}{\mu }>\frac{1}{\mathrm{e}}.$$

(29)

Using Theorem 4, all solutions of Equation (22) are oscillatory if

$${\phi }_0>max\left\{\frac{6}{\mathrm{e}{\tilde{\rho }}_0\mu ln\left(1/\mu \right)},\frac{6}{\mathrm{e}{\widehat{\rho }}_{0}ln\left(1/\mu \right)}\right\}.$$

Consider the following special case of (22):

$${\left(s^4{\left[x\left(s\right)+\frac{7}{8}x\left(\frac{9s}{10}\right)\right]}^{‴}\right)}^{\prime }+{\phi }_{0}x\left(\frac{s}{2}\right)=0$$

(30)

and

$${\left(s^4{\left[x\left(s\right)+\frac{7}{8}x\left(\frac{9s}{10}\right)\right]}^{‴}\right)}^{\prime }+{\phi }_{0}x\left(\frac{7s}{10}\right)=0.$$

(31)

All solutions of Equations (30) and (31) are oscillatory if ${\phi }_0>55.136$ (condition (23)) and ${\phi }_0>30.243$ (condition (29)), respectively.

3. Conclusions

In this work, the asymptotic behavior of solutions to even-order neutral differential equations in the non-canonical case is studied. We obtained a new relationship between the solution and its corresponding function. We then used this new relationship to derive criteria that ensure that all non-oscillatory solutions converge to zero. The new criteria do not require additional restrictions to delay functions (as in (3)). Furthermore, Theorem 3 improves Theorem 1, as it does not require verification of the extra condition (4).