Guided Wave-Based Damage Detection of Square Steel Tubes Utilizing Structure Symmetry
Abstract
1. Introduction
2. Propagation Characteristics and Symmetry Analysis of Guided Waves in Square Steel Tube
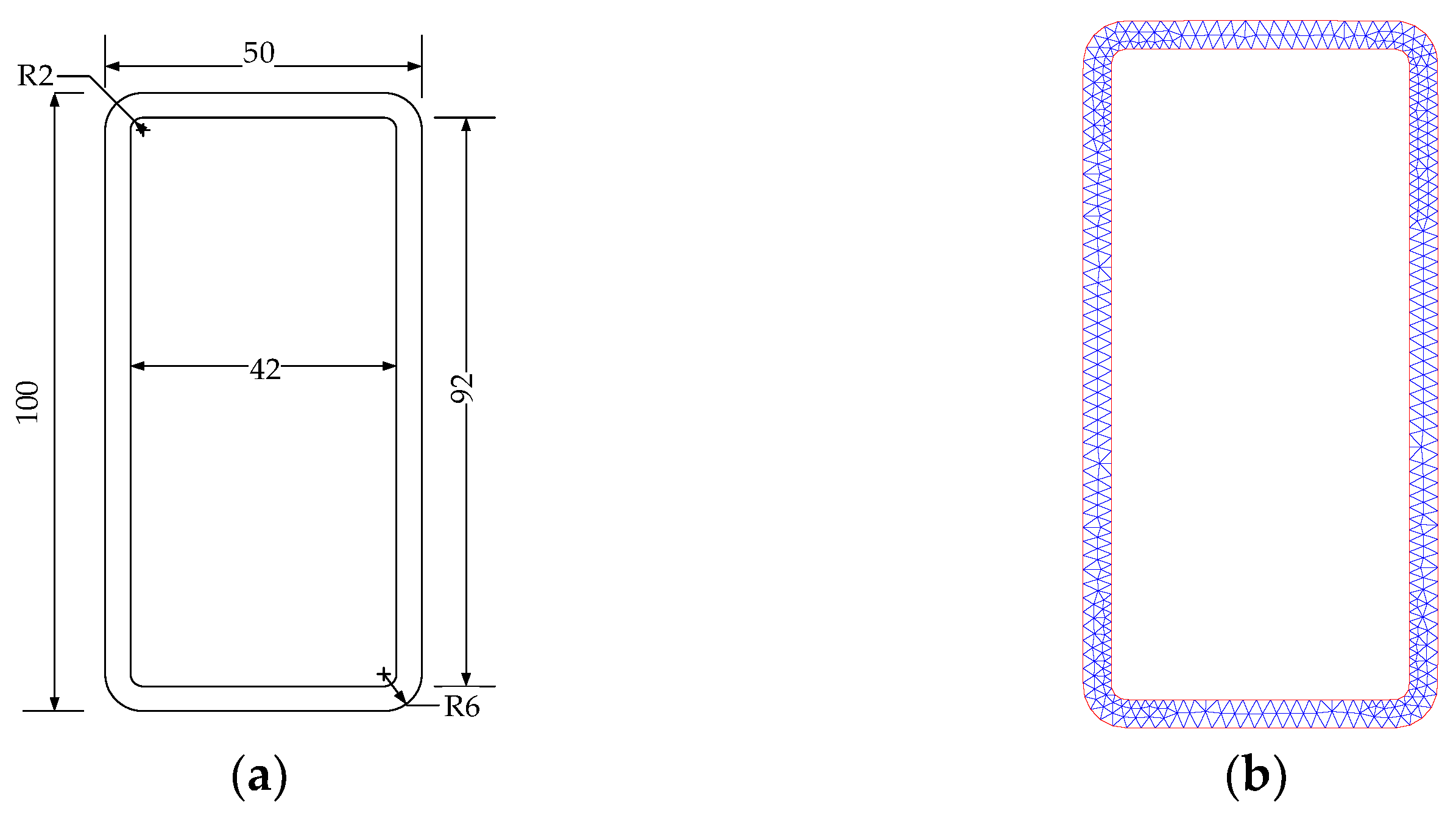
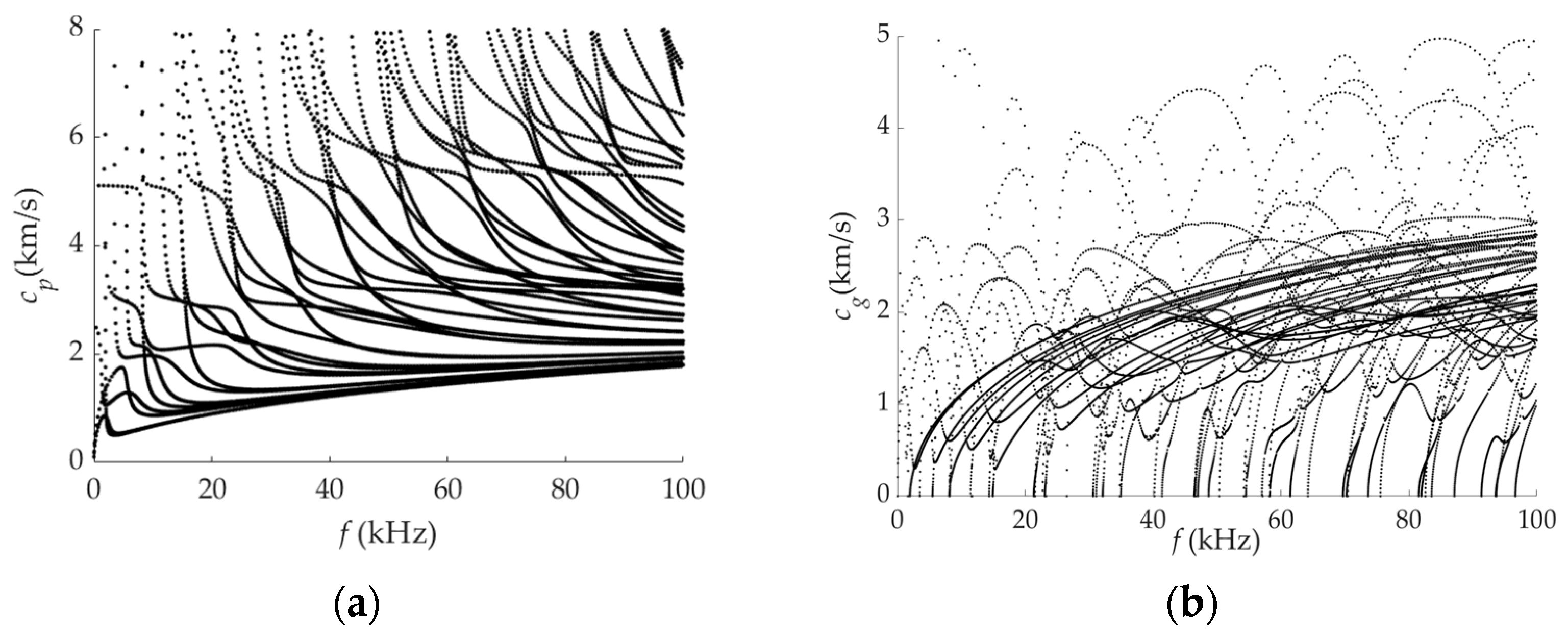
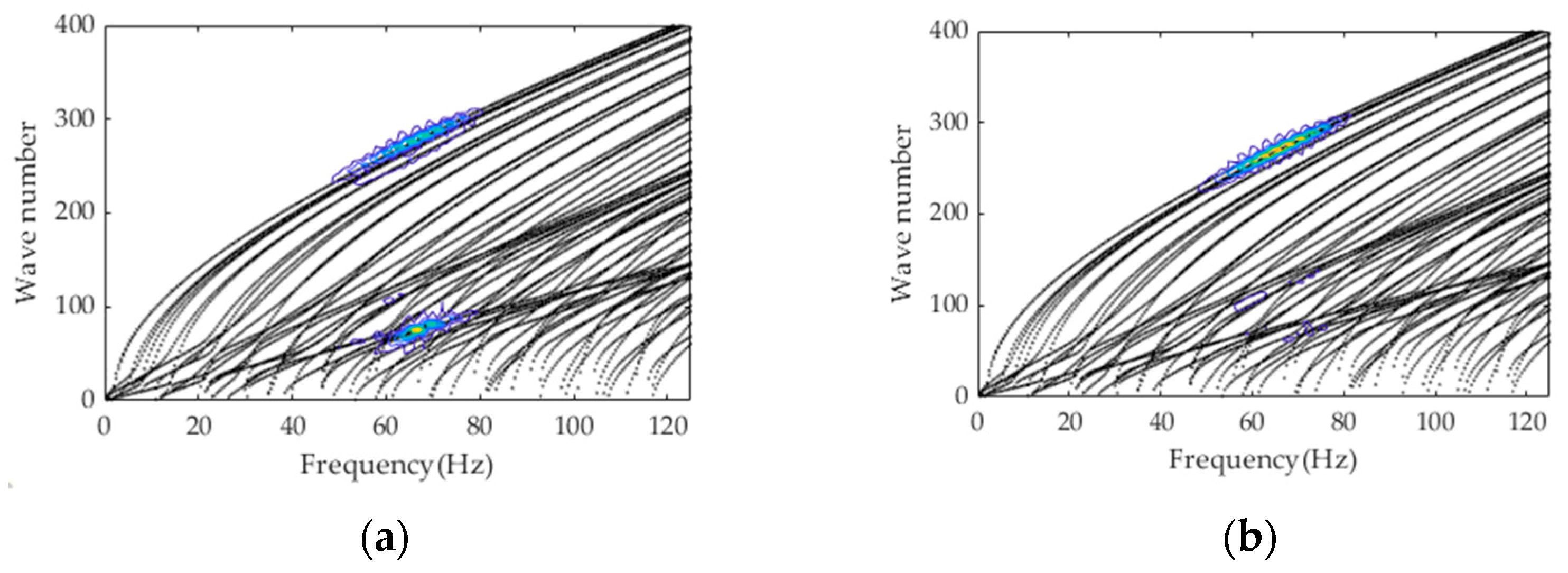
2.1. Dispersion Characteristics Analysis Based on SAFE Method
2.2. Symmetry Analysis for Guided Waves in Square Steel Tube
2.3. The Procedure of the Damage Detection Method
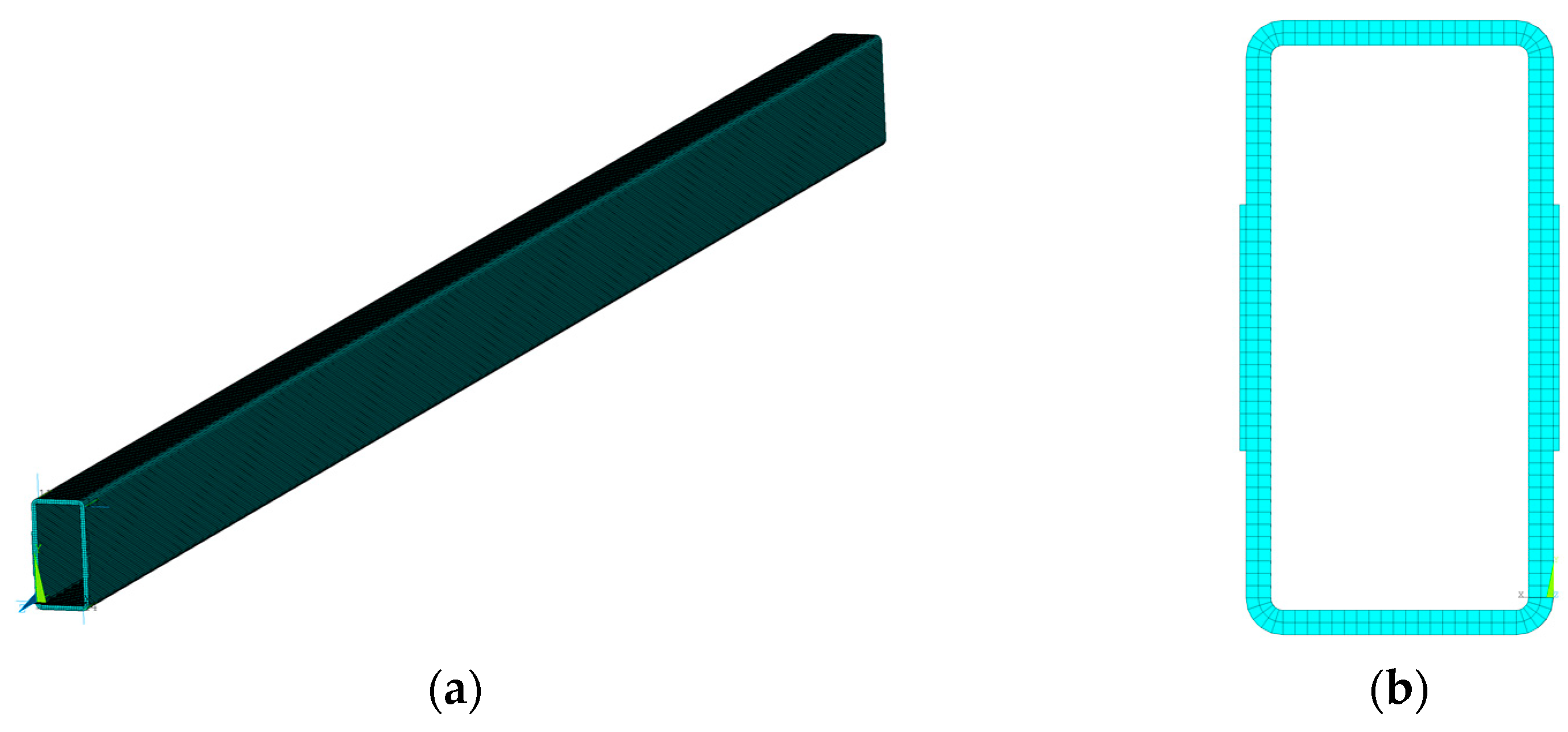
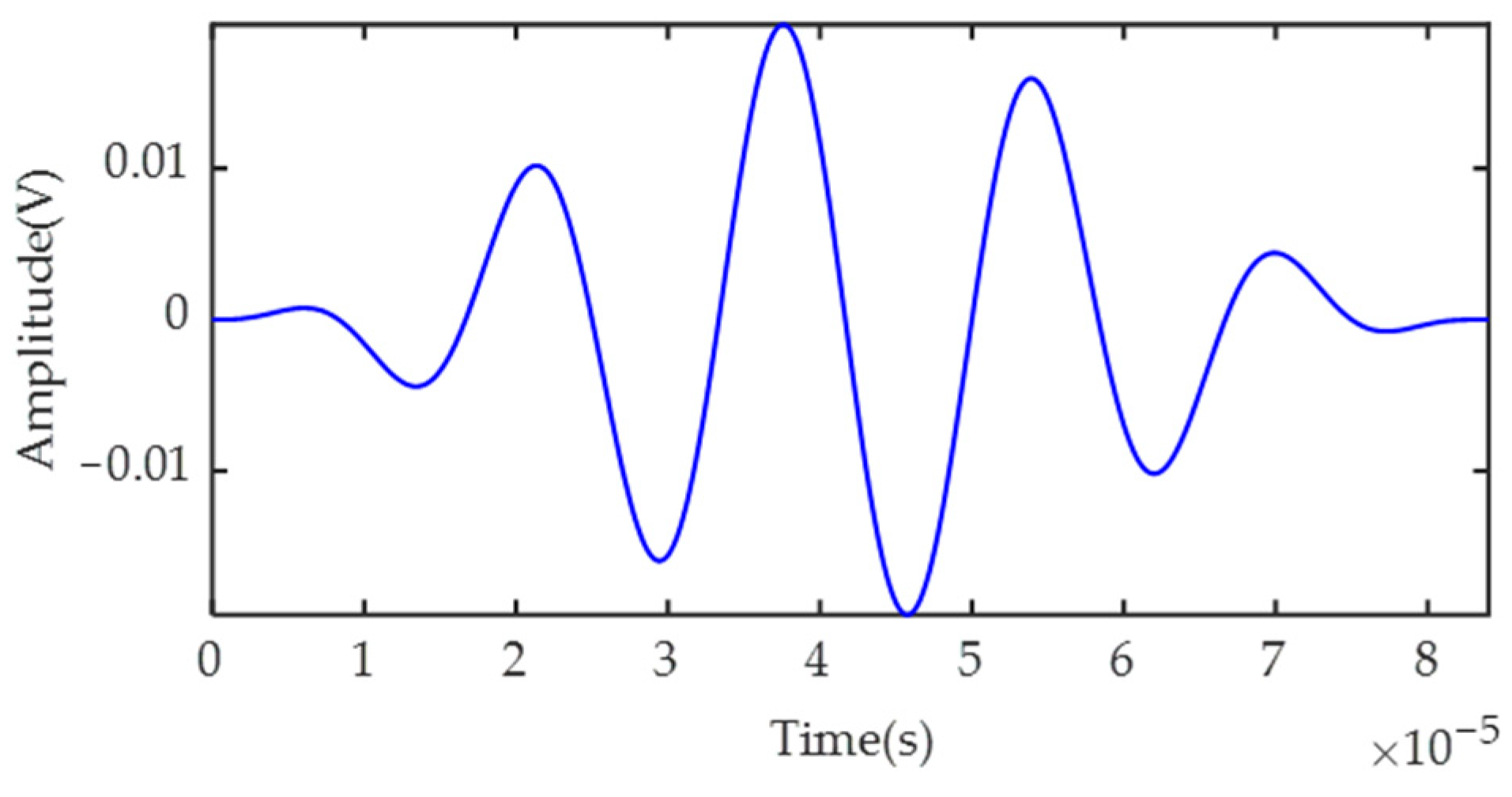
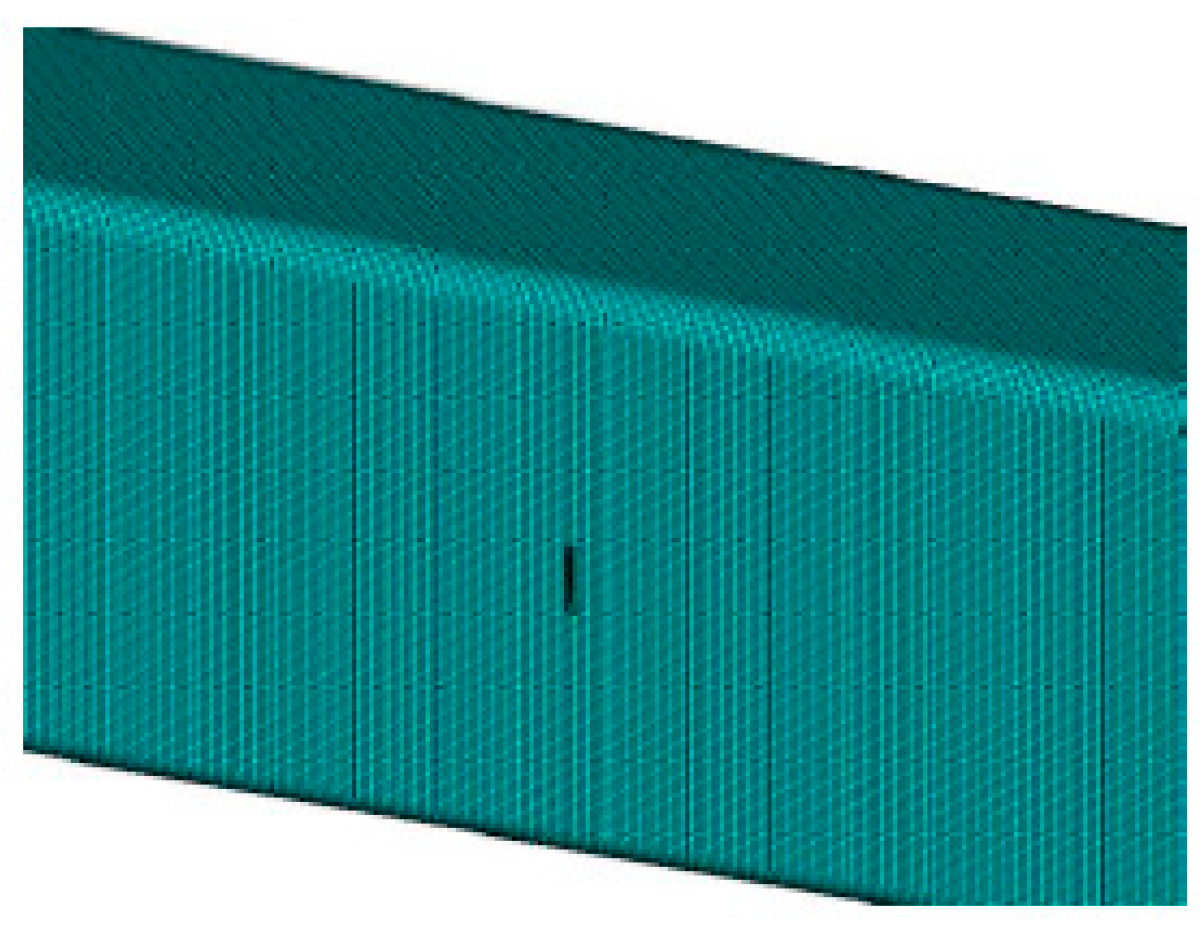
3. Numerical Simulation
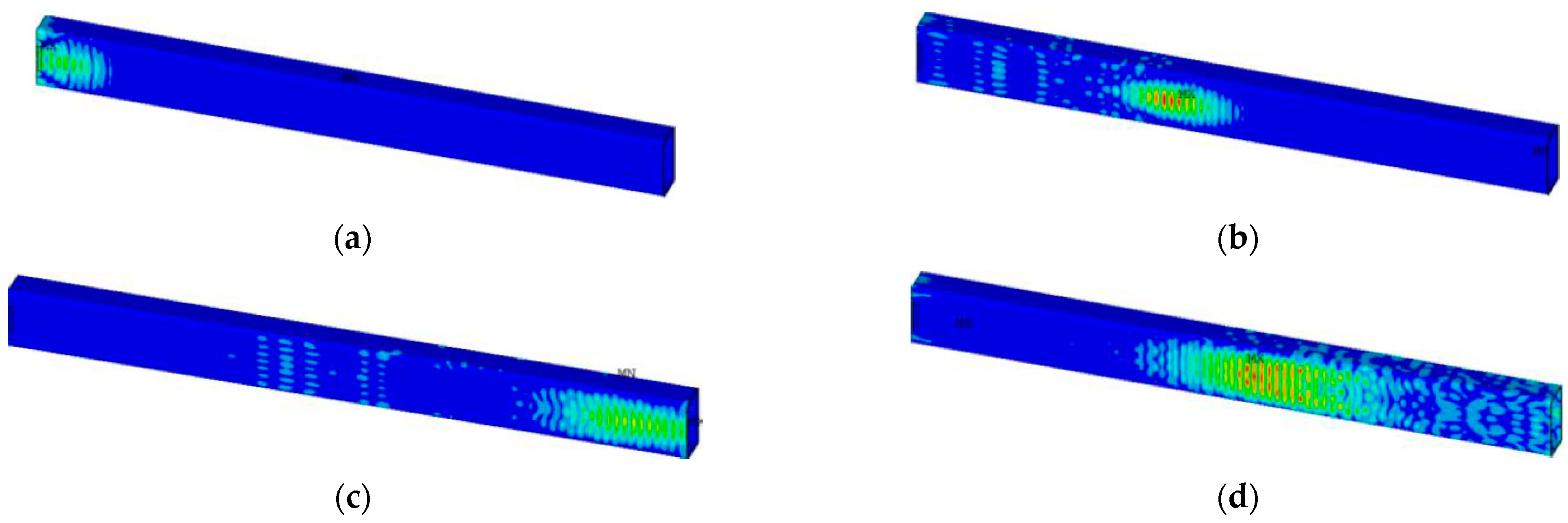
3.1. Propagation Characteristic of Guided Waves in Square Steel Tube
3.2. Damage Detection
4. Experimental Investigations
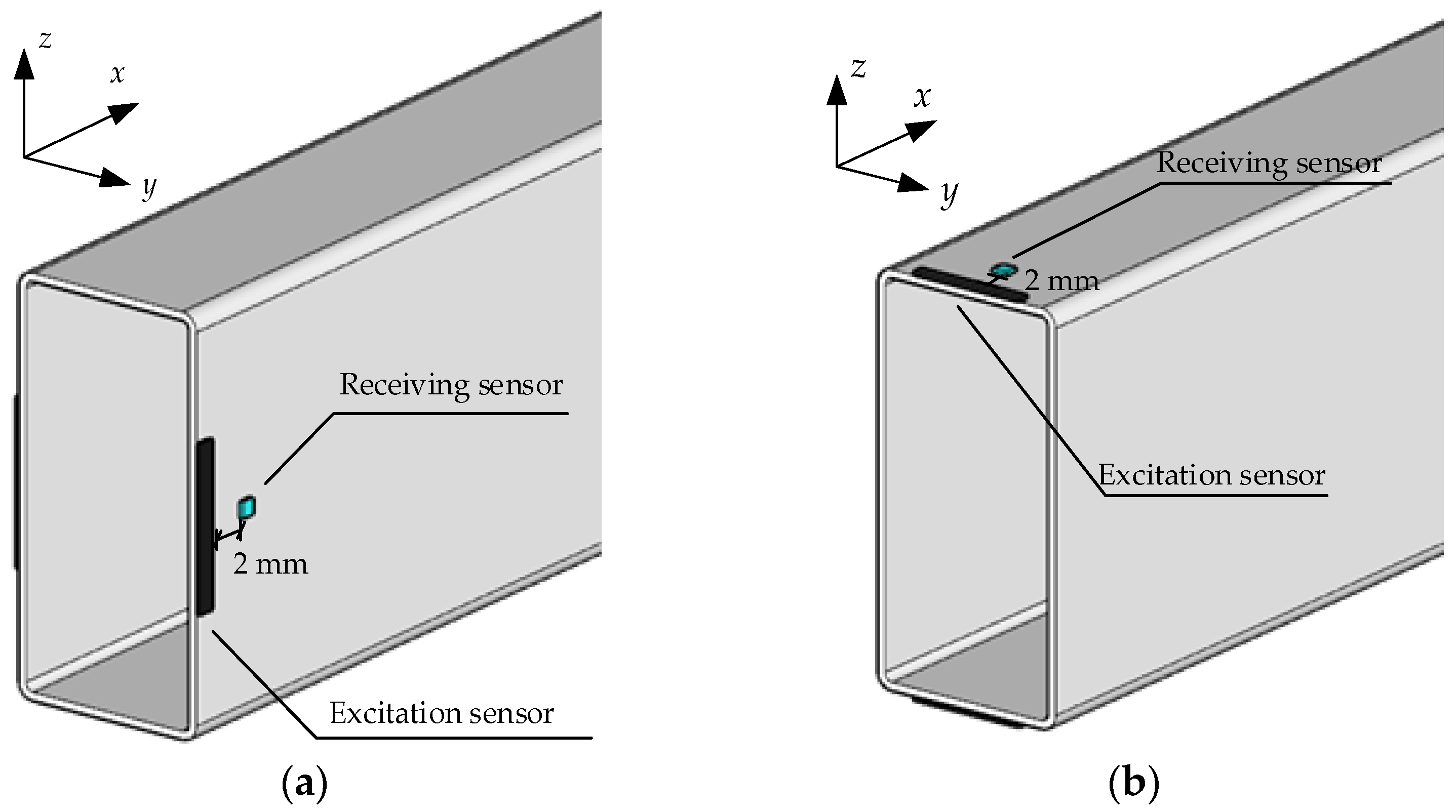
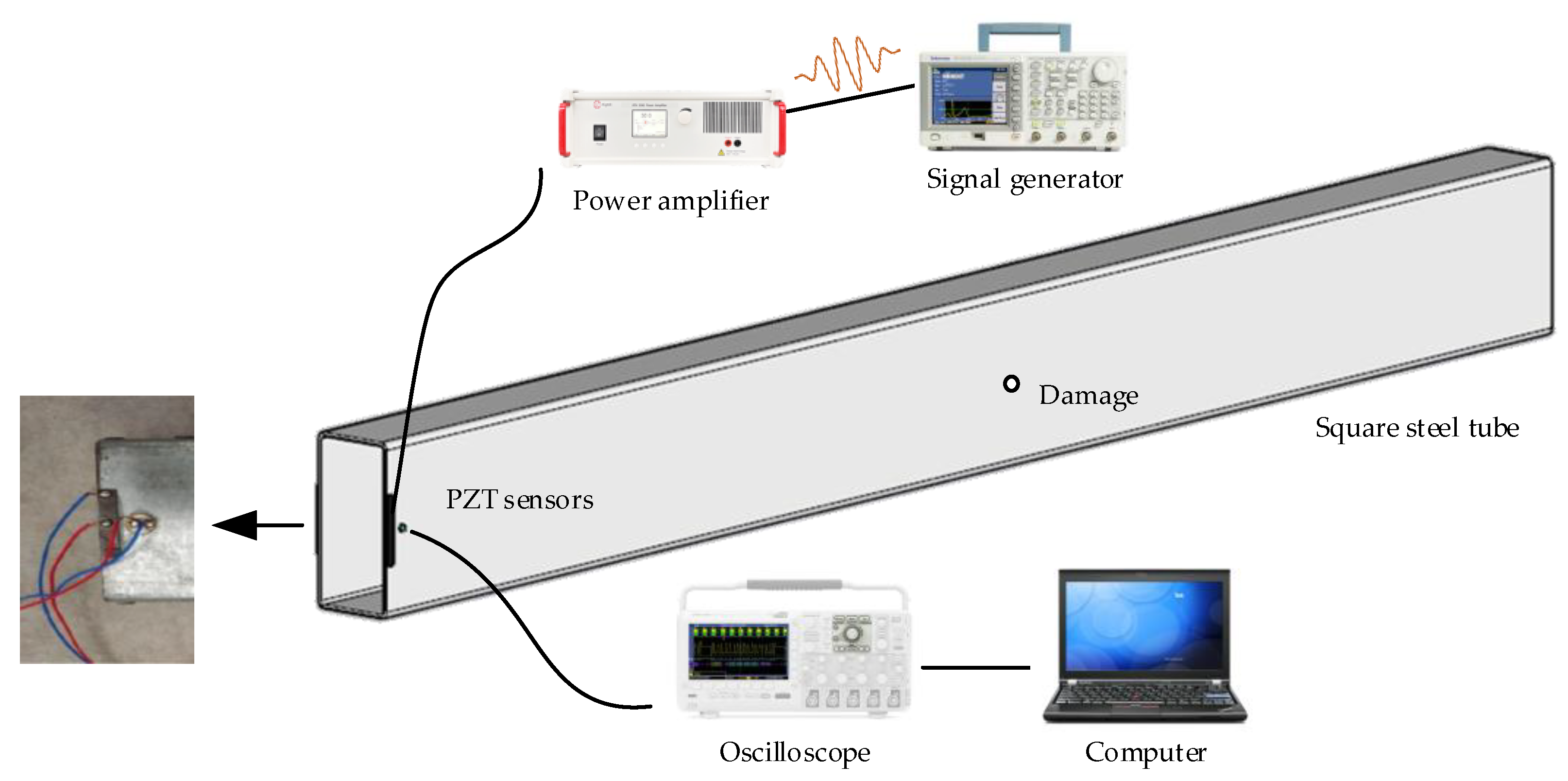
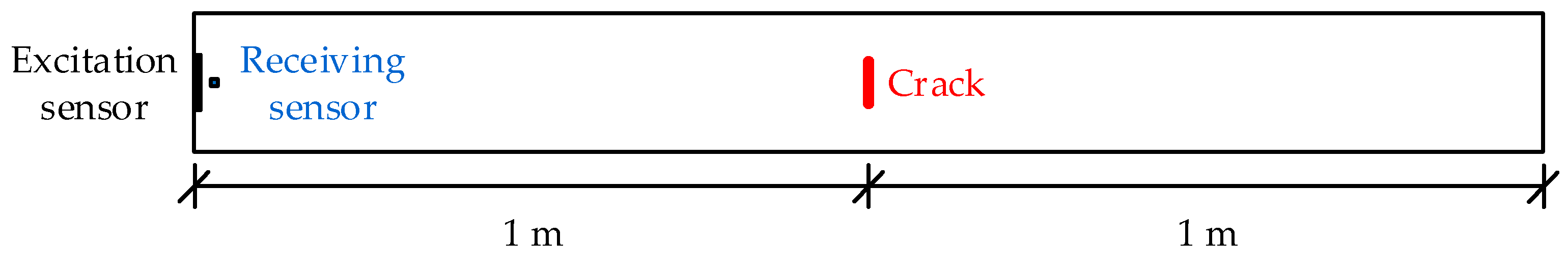
4.1. Experimental Setup
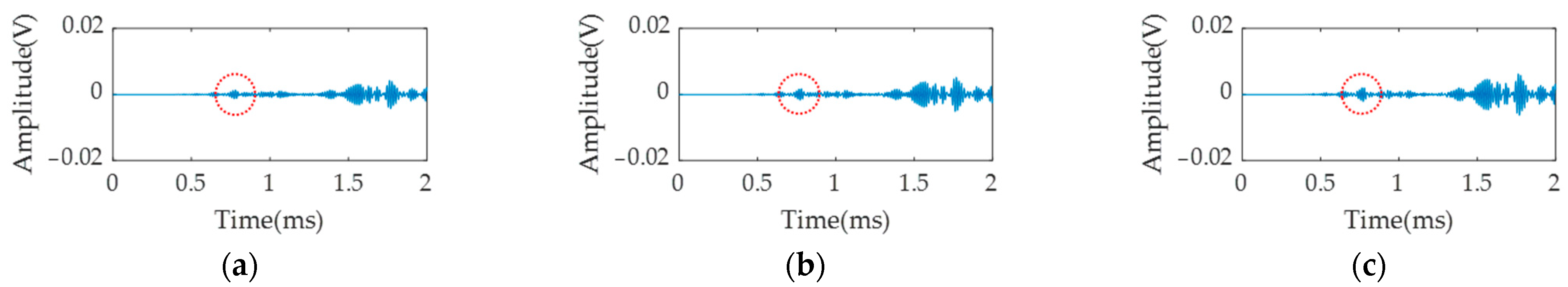
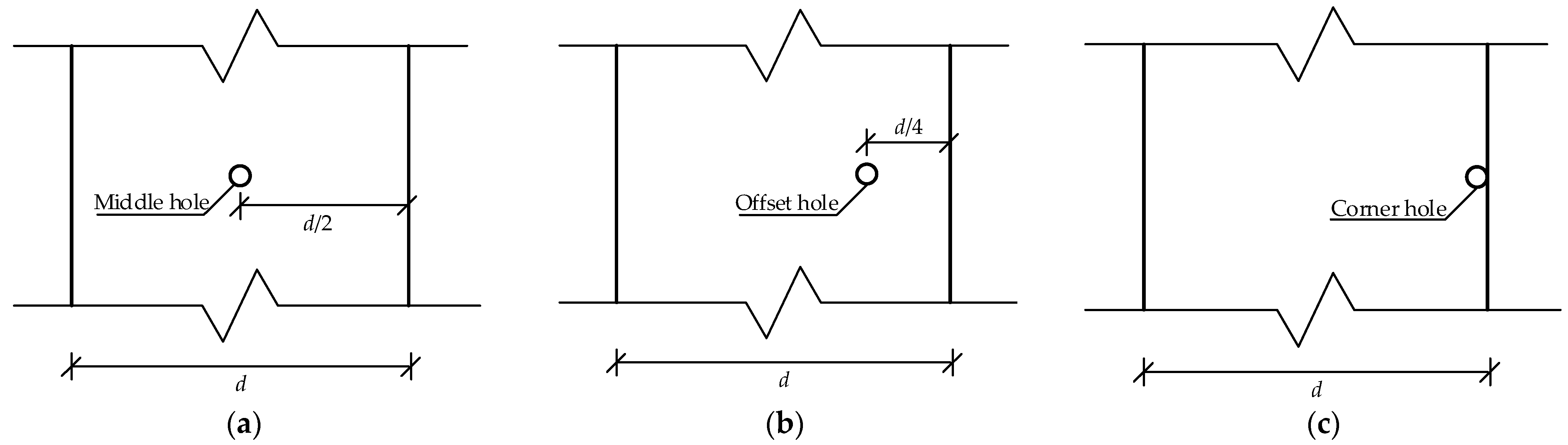
4.2. Inspection of Different Shape Damage
4.3. Damage Localization
4.4. Further Discussion on Corner Damage and Multiple Damages
5. Conclusions
- The SAFE method was used to solve the dispersion characteristics of the square steel tube, and the first, second, fifth and sixth order modes were selected as the desired modes for inspecting the damage status of long and short edges. Through finite element simulation and NME analysis, it was verified that by using structure symmetry, the influence of the dispersion effect and boundary limit can be reduced effectively, and thus clutter can be eliminated.
- The method to identify the damage of square steel tube based on section symmetry was proposed and verified by numerical simulation and laboratory experiments. The test results show that for cracks of different sizes and middle and offset hole damage in long and short edges, the symmetrical damage difference signals will appear as obvious damage wave packets at the damage locations.
- Damage location can be determined by the group wave velocity of desired modes and the appearance time of the damage wave packet. The calculation results show that the identification errors of crack, middle hole and offset hole damage are no more than 9.11%, indicating high positioning accuracy.
- For corner hole damage, the initial scheme had a poor identification effect, but it can be improved by changing the position of the receiving piezoelectric plate. However, for multi damages, the proposed method still has a good identification effect.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wan, X.; Liu, M.R.; Zhang, X.H.; Fan, H.W.; Tse, P.W.; Dong, M.; Wang, X.; Wei, H.H.; Xu, C.H.; Ma, H.W. The Use of Ultrasonic Guided Waves for the Inspection of Square Tube Structures: Dispersion Analysis and Numerical and Experimental Studies. Struct. Health Monit. 2021, 20, 58–73. [Google Scholar] [CrossRef]
- He, J.Z.; Yuan, F.G. Damage Identification for Composite Structures Using a Cross-Correlation Reverse-Time Migration Technique. Struct. Health Monit.-Int. J. 2015, 14, 558–570. [Google Scholar] [CrossRef]
- He, J.Z.; Leckey, C.A.C.; Leser, P.E.; Leser, W.P. Multi-Mode Reverse Time Migration Damage Imaging Using Ultrasonic Guided Waves. Ultrasonics 2019, 94, 319–331. [Google Scholar] [CrossRef] [PubMed]
- He, J.Z.; Rocha, D.C.; Sava, P. Guided Wave Tomography Based on Least-Squares Reverse-Time Migration. Struct. Health Monit.-Int. J. 2019, 19, 1237–1249. [Google Scholar] [CrossRef]
- Zhou, W.S.; Li, H.; Yuan, F.G. Fundamental Understanding of Wave Generation and Reception Using d36 Type Piezoelectric Transducers. Ultrasonics 2015, 57, 135–143. [Google Scholar] [CrossRef]
- Golub, M.V.; Doroshenko, O.V.; Arsenov, M.A.; Bareiko, I.A.; Eremin, A.A. Identification of Material Properties of Elastic Plate Using Guided Waves Based on the Matrix Pencil Method and Laser Doppler Vibrometry. Symmetry 2022, 14, 1077. [Google Scholar] [CrossRef]
- Yaylaci, M. Simulate of Edge and an Internal Crack Problem and Estimation of Stress Intensity Factor Through Finite Element Method. Adv. Nano Res. 2022, 12, 405–414. [Google Scholar]
- Vinh, P.V.; Avcar, M.; Belarbi, M.O.; Tounsi, A.; Huy, L.Q. A New Higher-Order Mixed Four-Node Quadrilateral Finite Element for Static Bending Analysis of Functionally Graded Plates. Structures 2023, 47, 1595–1612. [Google Scholar] [CrossRef]
- Sobhani, E. Improvement of Vibrational Characteristics of Multipurpose Structures (Plate and Shells) Used in Aerospace Components by Deploying Graphene Oxide Powders (GOPs) in a Matrix as a Nano-Reinforcement: A Comprehensive Study. Eng. Anal. Bound. Elem. 2023, 146, 598–635. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Ersoy, H.; Civalek, O. Solution of Moore–Gibson–Thompson Equation of an Unbounded Medium with a Cylindrical Hole. Mathematics 2021, 9, 1536. [Google Scholar] [CrossRef]
- Miao, H.C.; Huan, Q.; Wang, Q.Z.; Li, F.X. Excitation and Reception of Single Torsional Wave T(0,1) Mode in Pipes Using Face-Shear d24 Piezoelectric Ring Array. Smart Mater. Struct. 2017, 26, 025021. [Google Scholar] [CrossRef]
- Zhang, H.; Du, Y.H.; Tang, J.H.; Kang, G.Z.; Miao, H.C. Circumferential SH Wave Piezoelectric Transducer System for Monitoring Corrosion-Like Defect in Large-Diameter Pipes. Sensors 2020, 20, 460. [Google Scholar] [CrossRef] [PubMed]
- Dabak, A. Data-Driven Semi-Supervised and Supervised Learning Algorithms for Health Monitoring of Pipes. Mech. Syst. Signal Process. 2019, 131, 524–537. [Google Scholar]
- Yeung, C.; Ng, C.T. Time-Domain Spectral Finite Element Method for Analysis of Torsional Guided Waves Scattering and Mode Conversion by Cracks in Pipes. Mech. Syst. Signal Process. 2019, 128, 305–317. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, S.; Wang, Q.; Zhao, W.; Huang, S.L. Development of a Helical Lamb Wave Electromagnetic Acoustic Transducer for Pipeline Inspection. IEEE Sens. J. 2020, 20, 9715–9723. [Google Scholar] [CrossRef]
- Guan, R.Q.; Lu, Y.; Wang, K.; Su, Z.Q. Fatigue Crack Detection in Pipes with Multiple Mode Nonlinear Guided Waves. Struct. Health Monit.-Int. J. 2019, 18, 180–192. [Google Scholar] [CrossRef]
- El Najjar, J.; Mustapha, S. Condition Assessment of Timber Utility Poles Using Ultrasonic Guided Waves. Constr. Build. Mater. 2020, 272, 121902. [Google Scholar] [CrossRef]
- Majhi, S.; Mukherjee, A.; George, N.V.; Uy, B. Corrosion Detection in Steel Bar: A Time-Frequency Approach. NDT E Int. 2019, 107, 102150. [Google Scholar] [CrossRef]
- Sun, K.; Meng, G.; Li, F.; Ye, L.; Lu, Y. Damage Identification in Thick Steel Beam Based on Guided Ultrasonic Waves. J. Intell. Mater. Syst. Struct. 2010, 21, 225–232. [Google Scholar] [CrossRef]
- Song, G.; Li, H.; Gajic, B.; Zhou, W.; Chen, P.; Gu, H. Wind Turbine Blade Health Monitoring with Piezoceramic-Based Wireless Sensor Network. Int. J. Smart Nano Mater. 2013, 4, 150–166. [Google Scholar] [CrossRef]
- Rose, J.L.; Ditri, J.J.; Pilarski, A.; Rajana, K.; Carr, F. A Guided Wave Inspection Technique for Nuclear Steam Generator Tubing. NDT E Int. 1994, 27, 307–310. [Google Scholar] [CrossRef]
- Shin, H.J.; Rose, J.L. Guided Waves by Axisymmetric and Non-Axisymmetric Surface Loading on Hollow Cylinders. Ultrasonics 1999, 37, 355–363. [Google Scholar] [CrossRef] [PubMed]
- Geetha, G.K.; Gopalakrishnan, S.; Hanagud, S. Laser Doppler Imaging of Delamination in a Composite T-Joint with Remotely Located Ultrasonic Actuators. Compos. Struct. 2016, 147, 197–210. [Google Scholar] [CrossRef]
- Serey, V.; Quaegebeur, N.; Renier, M.; Micheau, P.; Masson, P.; Castaings, M. Selective Generation of Ultrasonic Guided Waves for Damage Detection in Rectangular Bars. Struct. Health Monit.-Int. J. 2020, 20, 1156–1168. [Google Scholar] [CrossRef]
- Zhang, J.Q.; Wu, Z.J.; Yang, Z.Y.; Liu, K.H.; Zhou, K.; Zheng, Y.B. Excitation of Guided Wave Modes in Arbitrary Cross-Section Structures by Applied Surface Tractions. Smart Mater. Struct. 2020, 29, 065010. [Google Scholar] [CrossRef]
- Tu, J.Q.; Tang, Z.F.; Yun, C.B.; Wu, J.; Xu, X. Guided Wave-Based Damage Assessment on Welded Steel I-Beam Under Ambient Temperature Variations. Struct. Control Health Monit. 2021, 28, e2696. [Google Scholar] [CrossRef]
- Ditri, J.J.; Rose, J.L. Excitation of Guided Elastic Wave Modes in Hollow Cylinders by Applied Surface Tractions. J. Appl. Phys. 1992, 72, 2589–2597. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M. Full-Scale Experimental and Numerical Investigations on the Modal Parameters of a Single-Span Steel-Frame Footbridge. Symmetry 2019, 11, 404. [Google Scholar] [CrossRef]
- Yang, Y.; Yang, L.; Yao, G. Post-Processing of High Formwork Monitoring Data Based on the Back Propagation Neural Networks Model and the Autoregressive-Moving-Average Model. Symmetry 2021, 13, 1543. [Google Scholar] [CrossRef]
- Yao, G.; Sun, Y.J.; Wong, M.P.; Lv, X.N. A Real-Time Detection Method for Concrete Surface Cracks Based on Improved YOLOv4. Symmetry 2021, 13, 1716. [Google Scholar] [CrossRef]
- Hayashi, T.; Rose, J.L. Guided Wave Simulation and Visualization by A Semi-Analytical Finite Element Method. Mater. Eval. 2003, 61, 75–79. [Google Scholar]
- Hayashi, T.; Kawashima, K.; Sun, Z.Q.; Rose, J.L. Analysis of Flexural Mode Focusing by a Semi-Analytical Finite Element Method. J. Acoust. Soc. Am. 2003, 113, 1241–1248. [Google Scholar] [CrossRef] [PubMed]
- Hayashi, T.; Song, W.J.; Rose, J.L. Guided Wave Dispersion Curves for a Bar with an Arbitrary Cross-Section, a Rod and Rail Example. Ultrasonics 2004, 41, 175–183. [Google Scholar] [CrossRef] [PubMed]
- Xie, J.; Ding, W.; Zou, W.; Wang, T.; Yang, J. Defect Detection inside a Rail Head by Ultrasonic Guided Waves. Symmetry 2022, 14, 2566. [Google Scholar] [CrossRef]








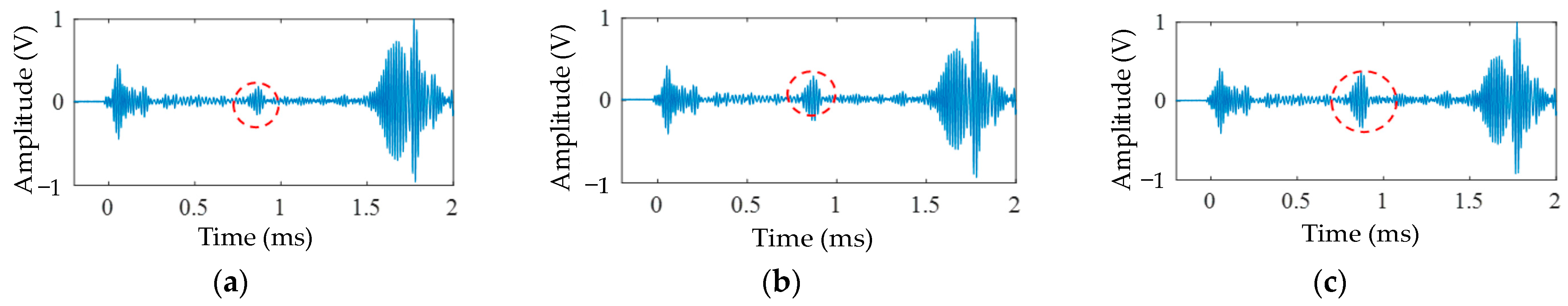
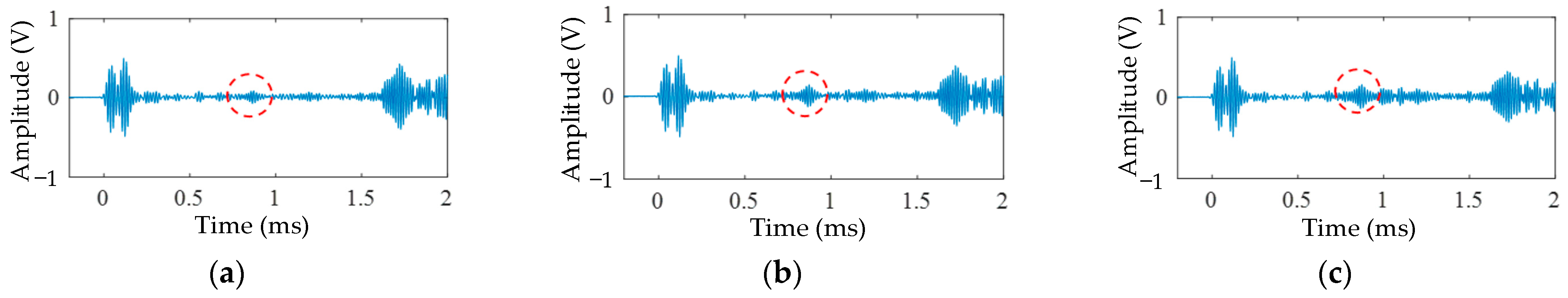
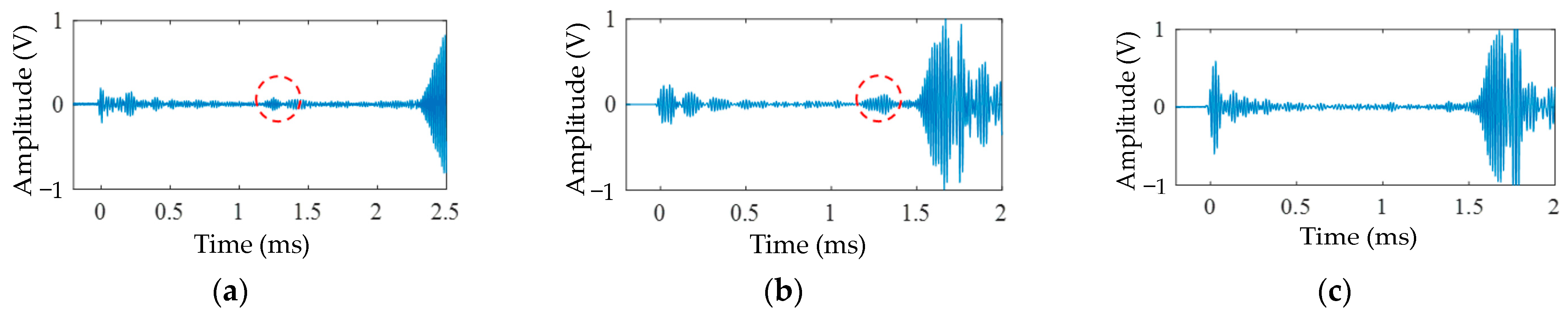




| Case No. | Crack Length (cm) | Position | Tube Length (m) | Defect Location (m) |
|---|---|---|---|---|
| 1 | 2 | Long edge | 2 | 1 |
| 2 | Short edge | 2 | 1 | |
| 3 | 3 | Long edge | 2 | 1 |
| 4 | Short edge | 2 | 1 | |
| 5 | 4 | Long edge | 2 | 1 |
| 6 | Short edge | 2 | 1 |
| Case No. | Damage Location | Position | Tube Length (m) | Defect Location (m) |
|---|---|---|---|---|
| 7 | Middle hole | Long edge | 3 | 1.5 |
| 8 | Short edge | 3 | 2 | |
| 9 | Offset hole | Long edge | 2 | 1.5 |
| 10 | Short edge | 2 | 1 | |
| 11 | Corner hole | Long edge | 2 | 1.5 |
| 12 | Short edge | 2 | 1 |
| Case No. | Time Difference (s) | Tube Length (m) | Identified Defect Location (m) | Actual Defect Location (m) | Inspection Error |
|---|---|---|---|---|---|
| 1 | 868 | 2 | 1.09 | 1 | 8.98% |
| 2 | 843 | 2 | 1.015 | 1 | 1.51% |
| 3 | 869 | 2 | 1.091 | 1 | 9.11% |
| 4 | 844 | 2 | 1.016 | 1 | 1.63% |
| 5 | 868 | 2 | 1.09 | 1 | 8.98% |
| 6 | 844 | 2 | 1.016 | 1 | 1.63% |
| Case No. | Time Difference (s) | Tube Length (m) | Identified Defect Location (m) | Actual Defect Location (m) | Inspection Error |
|---|---|---|---|---|---|
| 7 | 1232 | 3 | 1.543 | 1.5 | 2.84% |
| 8 | 1642 | 3 | 1.968 | 2 | 1.62% |
| 9 | 1258 | 2 | 1.575 | 1.5 | 5.00% |
| 10 | 837 | 2 | 1.008 | 1 | 0.79% |
| 11 | 1383 | 2 | 1.731 | 1.5 | 15.37% |
| 12 | 974 | 2 | 1.171 | 1 | 17.13% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, T.; Zhou, W.; Yu, L. Guided Wave-Based Damage Detection of Square Steel Tubes Utilizing Structure Symmetry. Symmetry 2023, 15, 805. https://doi.org/10.3390/sym15040805
Yang T, Zhou W, Yu L. Guided Wave-Based Damage Detection of Square Steel Tubes Utilizing Structure Symmetry. Symmetry. 2023; 15(4):805. https://doi.org/10.3390/sym15040805
Chicago/Turabian StyleYang, Tingting, Wensong Zhou, and Lei Yu. 2023. "Guided Wave-Based Damage Detection of Square Steel Tubes Utilizing Structure Symmetry" Symmetry 15, no. 4: 805. https://doi.org/10.3390/sym15040805
APA StyleYang, T., Zhou, W., & Yu, L. (2023). Guided Wave-Based Damage Detection of Square Steel Tubes Utilizing Structure Symmetry. Symmetry, 15(4), 805. https://doi.org/10.3390/sym15040805








