The Geometry of the Inextensible Flows of Timelike Curves according to the Quasi-Frame in Minkowski Space
Abstract
1. Introduction
2. Basic Geometric Concepts of Timelike Curves in Minkowski Space
- Let X and Y be spacelike vectors ; if X and Y span a timelike vector subspace, then , and there is a unique positive real number θ such thatwhere the real number θ is called the Lorentz timelike angle between X and Y.
- Let X and Y be spacelike vectors in that span a spacelike vector subspace; then, there is a unique real number , such thatwhere the real number θ is called the Lorentz spacelike angle between X and Y.
- Let X and Y be future pointing (past pointing) timelike vectors in ; then, there is a unique nonnegative real number θ, such thatwhere the real number θ is called the Lorentz timelike angle between X and Y.
- Let X be a spacelike vector and Y a future pointing timelike vector in ; then, there is a unique nonnegative real number , such thatwhere the real number θ is called the Lorentz timelike angle between X and Y.
2.1. Frenet Frame for Timelike Curves in
- ,, , , and
- , and .
- where k and τ are the curvature and torsion for the timelike curve, respectively.
2.2. Quasi-Frame for Timelike Curves in
3. Main Results and Discussion
Time Evolution Equations for the Quasi-Timelike Curves (QTIC) in
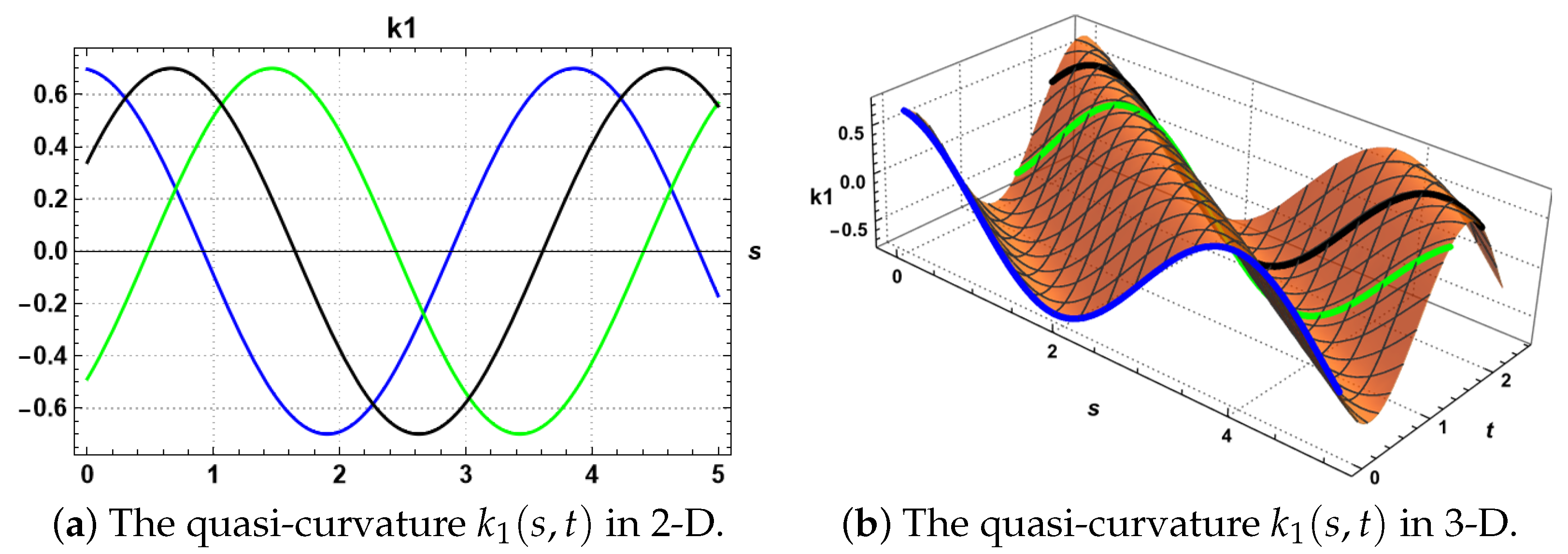
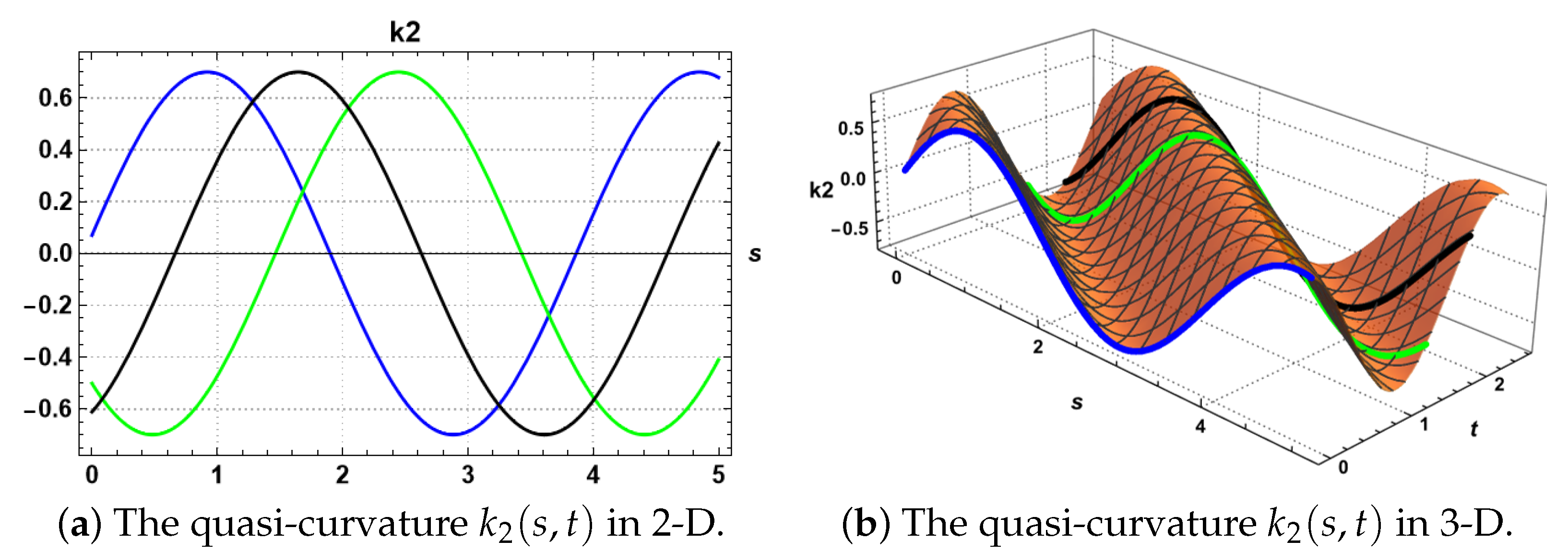
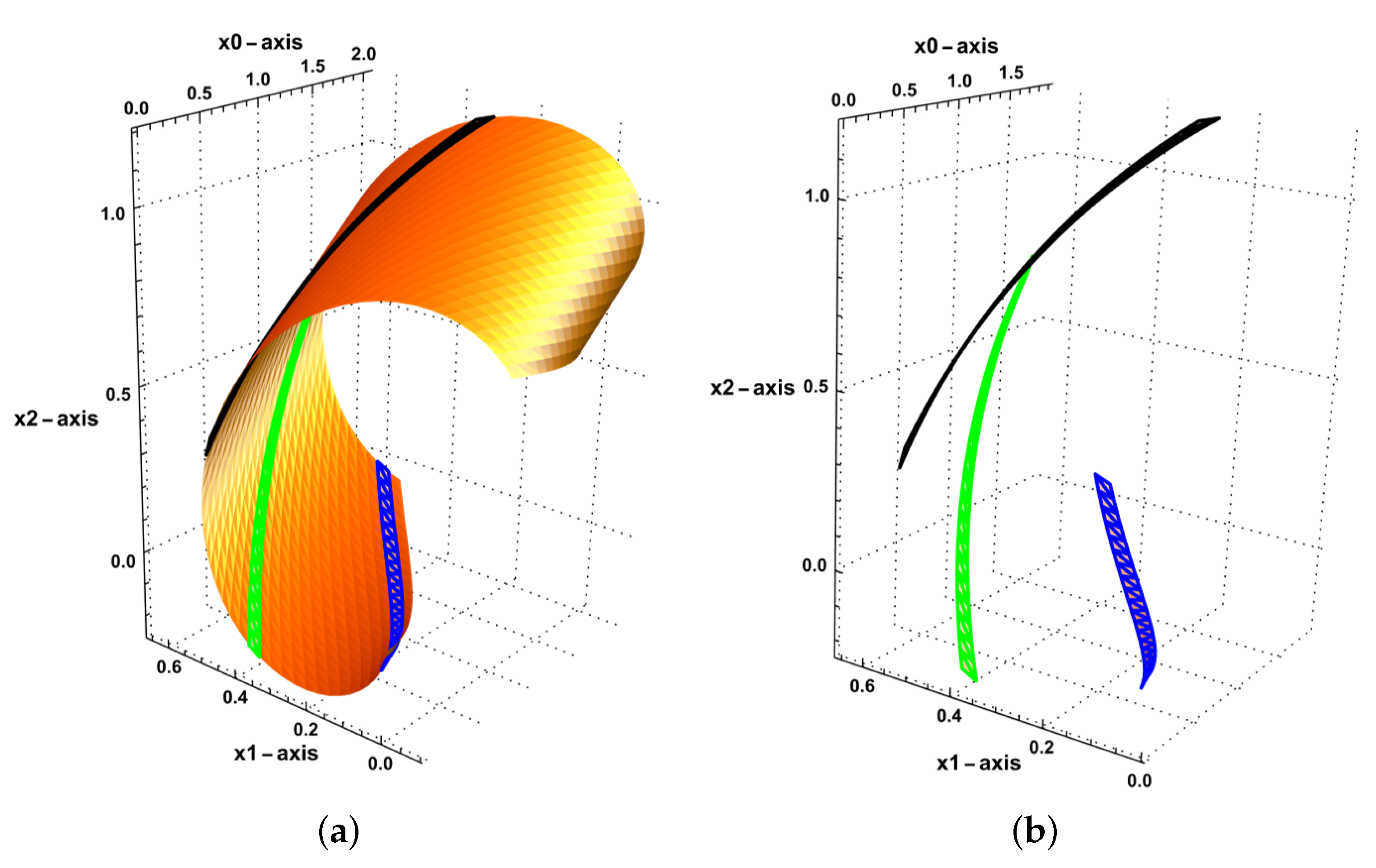
4. An Application for the Motion of the QTIC by Velocity Fields
5. The Flows of the QTIC via the Acceleration Fields
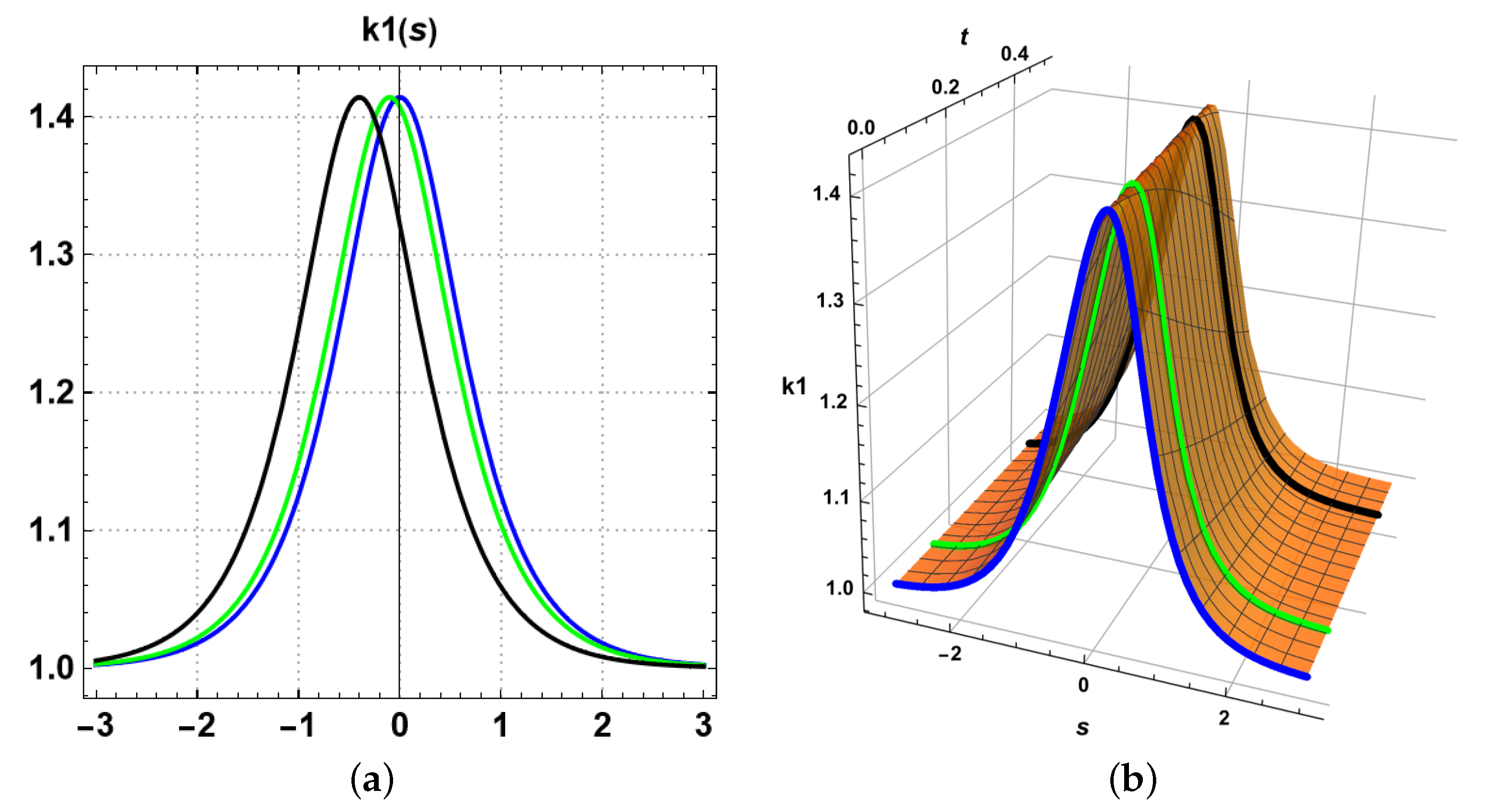
An Application for the Motion of the QTIC by the Acceleration Fields
6. Discussion
7. Conclusions
- 1.
- We studied the motion of the QTIC by the velocity fields , and , with the equation of motion , where , and represented the velocity functions in the direction of the q- frame .
- 2.
- The time evolution equations (TEEs) for the q-frame of the QTIC in Minkowski space were derived, and the TEEs for the quasi-curvatures and were obtained as a system of PDEs (Theorem 2).
- 3.
- 4.
- We studied the motion of the QTIC described by the acceleration fields with the equation of motion .
- 5.
- 6.
- Through the given applications, we presented the description of the graphs, which indicated the flows of the quasi-timelike curves and their first and second quasi-curvatures.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| (2-D) | Two dimensions. |
| (3-D) | Three dimensions. |
| IFC | Inextensible flows of curves. |
| PDE(s) | Partial Differential Equation(s) |
| q-frame | Quasi-frame. |
| QTIC | Quasi-timelike curve. |
| TEEs | Time evolution equations. |
References
- Nakayama, K.; Wadati, M. Motion of curves in the plane. J. Phys. Soc. JPN 1993, 62, 473–479. [Google Scholar] [CrossRef]
- Nakayama, K.; Segur, H.; Wadati, M. Integrability and the motion of curves. Phys. Rev. Lett. 1992, 69, 2603–2606. [Google Scholar] [CrossRef] [PubMed]
- Abdel-All, N.H.; Hussien, R.A.; Youssef, T. Evolution of curves via the velocities of the moving frame. J. Math. Comput. Sci. 2012, 2, 1170–1185. [Google Scholar]
- Doliwa, A.; Santini, P. An elementary geometric characterization of the integrable motions of a curve. Phys. Lett. A 1994, 185, 373–384. [Google Scholar] [CrossRef]
- Kwon, D.Y.; Park, F.C. Evolution of inelastic plane curves. Appl. Math. Lett. 1999, 12, 115–119. [Google Scholar] [CrossRef]
- Hasimoto, H. A soliton on a vortex filament. J. Fluid Mech. 1972, 52, 477–485. [Google Scholar] [CrossRef]
- Kızıltuğ, S. On characterization of inextensible flows of curves according to type-2 Bishop Frame in . Math. Comput. Appl. 2014, 19, 69–77. [Google Scholar]
- Yıldız, O.G.; Ersoy, S.; Masal, M. A Note on inextensible flows of curves on oriented Surface. CUBO A Math. J. 2014, 16, 11–19. [Google Scholar] [CrossRef]
- Abdel All, N.; Mohamed, S.; Al-Dossary, M. Evolution of generalized space curve as a function of its local geometry. Appl. Math. 2014, 5, 2381–2392. [Google Scholar] [CrossRef]
- Hussien, R.; Gaber, S. Generated surfaces via inextensible flows of curves in . J. Appl. Math. 2016, 2016, 1–8. [Google Scholar] [CrossRef]
- Selçuk, B. A new method for inextensible flows of adjoint curves in space. AIP Con. Proc. 2019, 2116, 240003. [Google Scholar]
- Gaber, S. New models of normal motions of the inextensible curves according to type-1 Bishop frame in . Int. J. Geom. Methods Mod. Phys. 2021, 18, 2150009. [Google Scholar]
- Elshenhab, A.M.; Moaaz, O.; Dassios, I.; Elsharkawy, A. Motion along a Space Curve with a Quasi-Frame in Euclidean 3-Space: Acceleration and Jerk. Symmetry 2022, 14, 1610. [Google Scholar] [CrossRef]
- Ding, Q.; Wang, W.; Wang, Y. A motion of spacelike curves in the Minkowski 3-space and the KdV equation. Phys. Lett. A 2010, 374, 3201–3205. [Google Scholar] [CrossRef]
- Yıldız Ö., G.; Tosun, M. A note on inextensible flows of curves in . Int. Electron. J. Geom. 2013, 6, 118–124. [Google Scholar]
- Gaber, S. Binormal motions of inextensible curves in de-sitter space . J. Egypt. Math. Soc. 2017, 25, 313–318. [Google Scholar]
- Yılmaz, S.; Turgut, M. A new version of Bishop frame and an application to spherical images. J.Math. Anal. Appl. 2010, 371, 764–776. [Google Scholar] [CrossRef]
- Korpinar, T.; Turgut, M. A new method for inextensible flows of timelike curves in 4-dimensional LP-Sasakian manifolds. Asian-Eur. J. Math. 2015, 8, 1550073. [Google Scholar] [CrossRef]
- Aslan, M.C.; Unluturk, Y. The evaluation of the conditions for the non-null curves to be Inextensible in Lorentzian 6-Space. Kyungpook Math. J. 2021, 61, 805–812. [Google Scholar] [CrossRef]
- Suleyman, C.; Ozturk, E.; Ozturk, U. Motions of curves in the Pseudo-Galilean Space . Math. Probl. Eng. 2015, 2015. [Google Scholar] [CrossRef]
- Oztekin, H.; Bozok, H.G. Inextensible flows of curves in the Equiform Geometry of the pseudo-Galilean space . WMS J. App. Eng. Math. 2016, 6, 175–184. [Google Scholar]
- Bozok, H.G. Inextensible flows of curves according to Sabban frame in Galilean space . J. Univers. Math. 2019, 2, 75–81. [Google Scholar] [CrossRef]
- Bozok, H.G.; Sertkol, I.N. A note on inextensible flows of curves with fermi-walker derivative in Galilean space . Honam Math. J. 2020, 42, 769–780. [Google Scholar] [CrossRef]
- Sorour, A.H. A study of the inextensible flows of tube-like surfaces associated with focal curves in Galilean 3-space . Stud. Univ. Babeş-Bolyai Math. 2017, 62, 341–352. [Google Scholar] [CrossRef]
- López, R.l. Differential geometry of curves and surfaces in Lorentz-Minkowski space. Int. Electron. J. Geom. 2014, 7, 44–107. [Google Scholar] [CrossRef]
- Fu, Y.; Wang, X.S. Classification of timelike constant slope Surfaces in 3-Dimensional Minkowski spaces. Results Math. 2013, 63, 1095–1108. [Google Scholar] [CrossRef]
- Yavuz, A. A Dynamical approach to position vector of timelike Curve by Vectorial Momentum, Torque and Tangential Dual Curve. J. Nonlinear Math. Phys. 2022, 29, 818–842. [Google Scholar] [CrossRef]
- Ekici, C.; Göksel, M.; Mustafa, D. Smarandache curves according to q-Frame in Minkowski 3-Space. Conf. Proc. Sci. Technol. 2019, 2, 110–118. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaber, S.; Sorour, A.H.
The Geometry of the Inextensible Flows of Timelike Curves according to the Quasi-Frame in Minkowski Space
Gaber S, Sorour AH.
The Geometry of the Inextensible Flows of Timelike Curves according to the Quasi-Frame in Minkowski Space
Gaber, Samah, and Adel H. Sorour.
2023. "The Geometry of the Inextensible Flows of Timelike Curves according to the Quasi-Frame in Minkowski Space
Gaber, S., & Sorour, A. H.
(2023). The Geometry of the Inextensible Flows of Timelike Curves according to the Quasi-Frame in Minkowski Space




