A Comprehensive Review of Non-Newtonian Nanofluid Heat Transfer
Abstract
1. Introduction
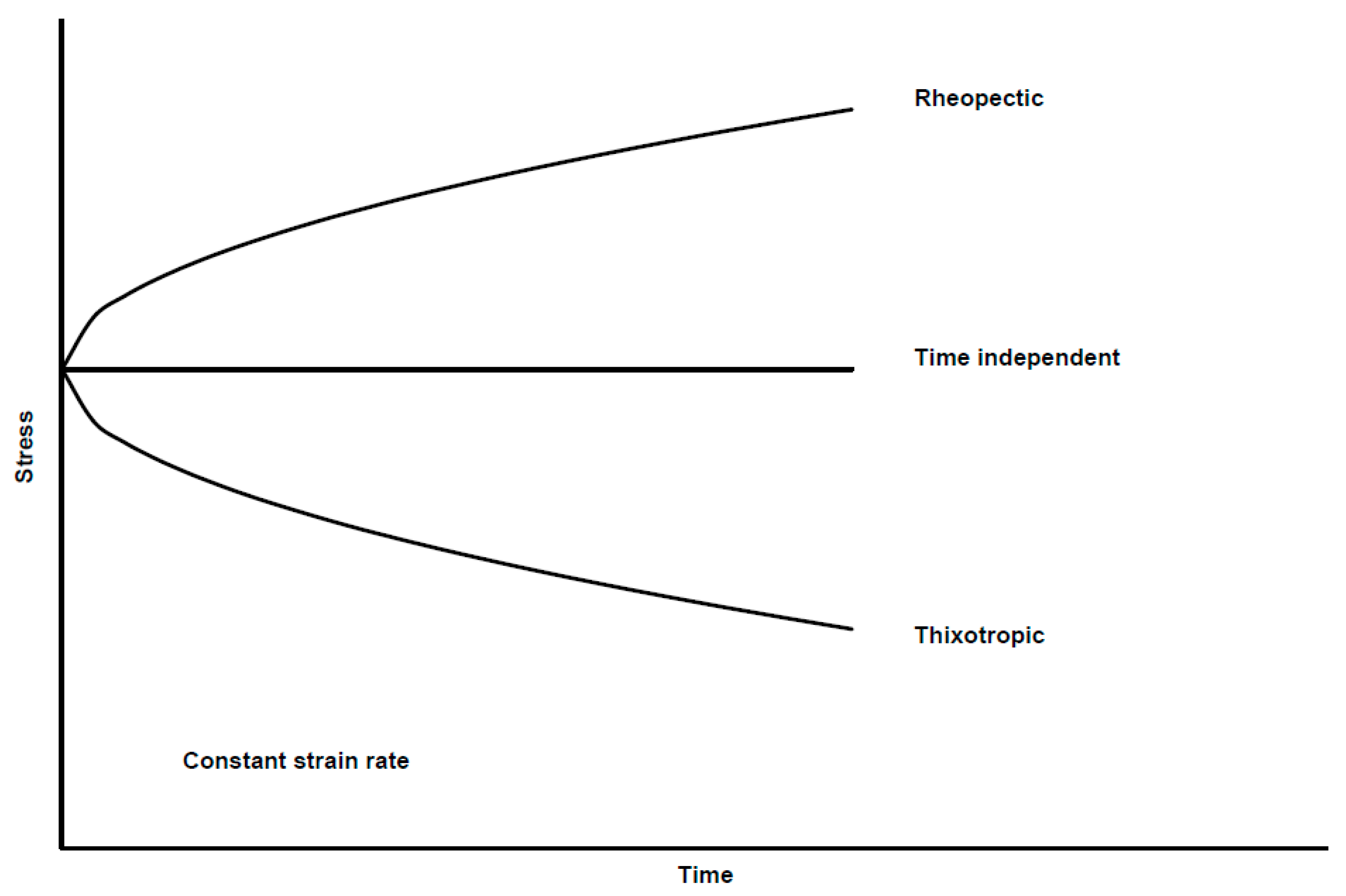
2. Non-Newtonian Nanofluid Heat Transfer
3. Statistics and Results
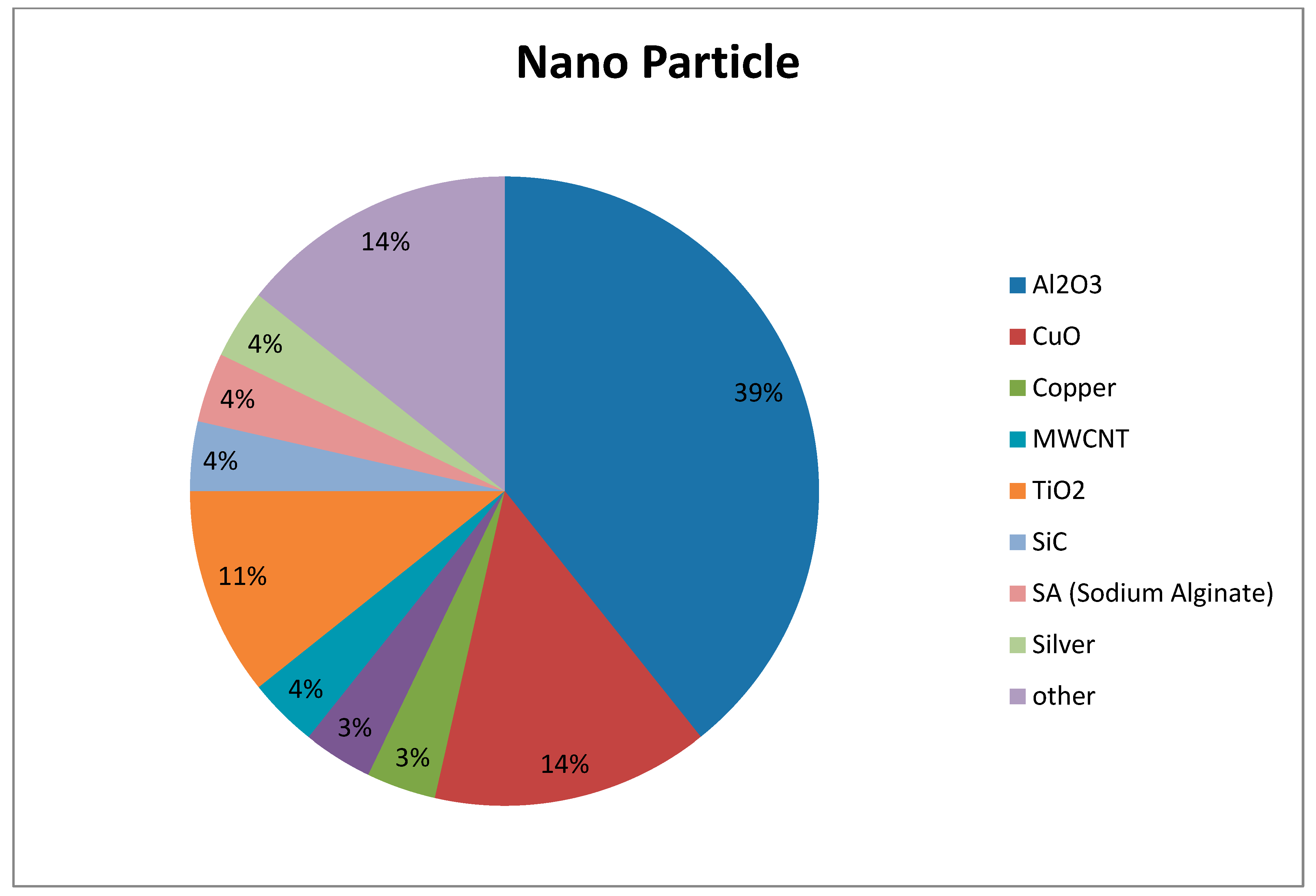
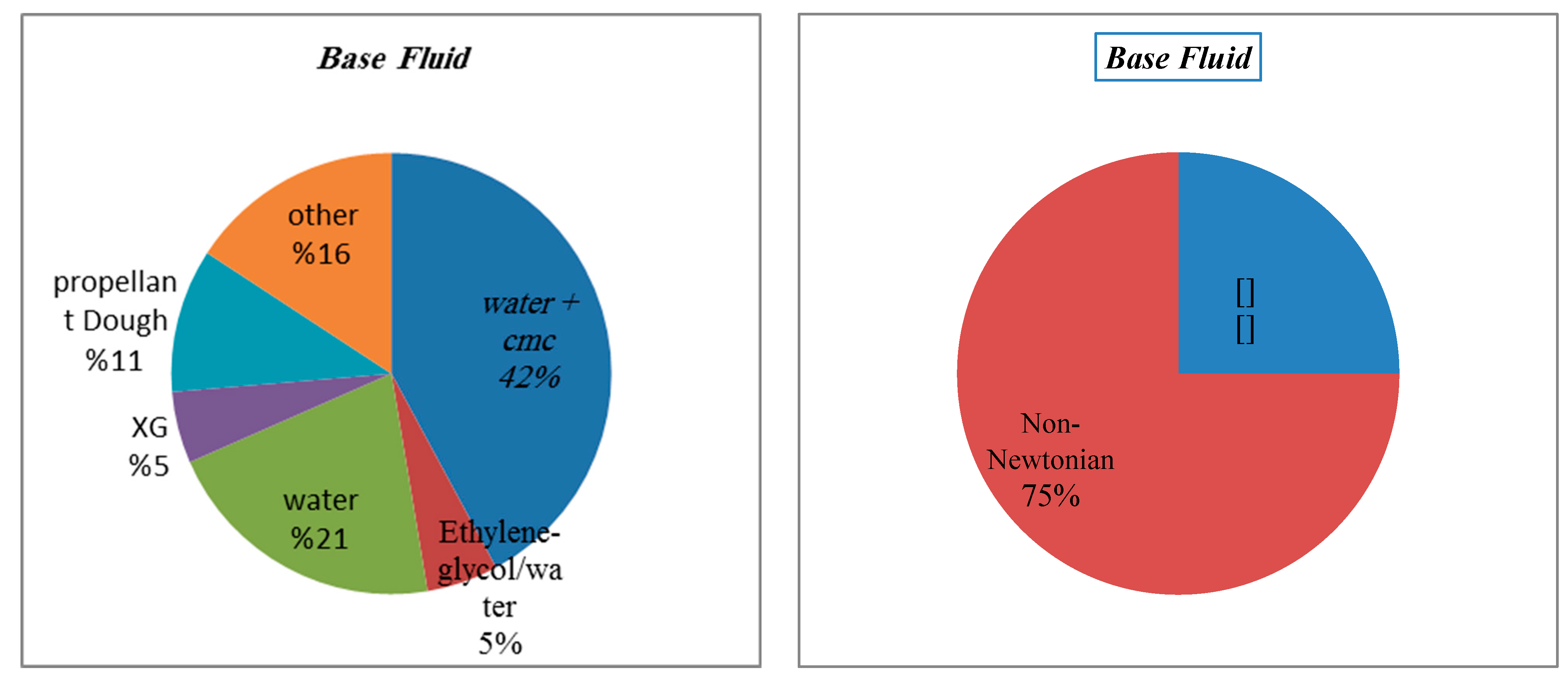
3.1. Which Nanoparticles Were Used the Most?
3.2. Which BaseFluidswere Used the Most?
3.3. Which Non-Newtonian Models Were Used the Most?
3.4. Which Numerical/Analytical Methods Were Used the Most?
4. Conclusions
- Al2O3was the most frequently used nanoparticle.
- 25% of nanofluids modeled as non-Newtonian have a Newtonian base fluid.
- The FVM numerical method is the most widely applied method.
- The water CMC base fluid is further investigated compared with other base fluids.
- The power-law model has been the most frequently applied non-Newtonian modeling of nanofluid heat transfer.
- In all non-Newtonian models, when nanofluid volume fraction increases, the forced convection heat transfer will be increased.
- Increasing non-Newtonian parameters, such as power-law index, Maxwell parameter, etc., leads to a decrease in heat transfer.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chhabra, R.; Richardson, J. Non-Newtonian Fluid. Behaviour 2008, 1–55. [Google Scholar] [CrossRef]
- Chhabra, R.P. Non-Newtonian Fluids: An Introduction. In Rheology of Complex Fluids; Springer: New York, NY, USA, 2010; pp. 3–34. [Google Scholar] [CrossRef]
- Chhabra, R.; Richardson, J. Non-Newtonian flow in the process industries: Fundamentals and engineering applications. In Non-Newtonian Flow Process Industries; Butterworth-Heinemann: Oxford, UK, 1999; pp. 73–161. [Google Scholar] [CrossRef]
- Yapici, K.; Osturk, O.; Uludag, Y. Dependency of nanofluid rheology on particle size and concentration of various metal oxide nanoparticles. Braz. J. Chem. Eng. 2018, 35, 575–586. [Google Scholar] [CrossRef]
- Pek, Y.S.; Wan, A.C.A.; Shekaran, A.; Zhuo, L.; Ying, J.Y. A thixotropic nanocomposite gel for three-dimensional cell culture. Nat. Nanotechnol. 2008, 3, 671–675. [Google Scholar] [CrossRef] [PubMed]
- Ghica, M.V.; Hîrjău, M.; Lupuleasa, D.; Dinu-Pîrvu, C.-E. Flow and Thixotropic Parameters for Rheological Characterization of Hydrogels. Molecules 2016, 21, 786. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Li, W.; Gong, X. Thixotropy of MR shear-thickening fluids. Smart Mater. Struct. 2010, 19, 125012. [Google Scholar] [CrossRef]
- Naderi, A.; Lindström, T. A comparative study of the rheological properties of three different nanofibrillated cellulose systems. Nord. Pulp Pap. Res. J. 2016, 31, 354–363. [Google Scholar] [CrossRef]
- Wang, G.; Nie, Q.; Zang, C.; Zhang, B.; Zhu, Q.; Luo, G.; Wang, S. Self-Assembled ThermoresponsiveNanogels Prepared by Reverse Micelle → Positive Micelle Method for Ophthalmic Delivery of Muscone, a Poorly Water-Soluble Drug. J. Pharm. Sci. 2016, 105, 2752–2759. [Google Scholar] [CrossRef]
- Das, M.R.; Paria, S. Rheological Behavior of Clay Water Slurry in the Presence of Cationic Surfactants and Electrolytes. Ph.D. Thesis, National Institute of Technology, Rourkela, India, 2013. [Google Scholar]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Siavashi, M.; Taylor, R.A.; Niazmand, H.; et al. Recent advances in modeling and simulation of nanofluid flows-Part I: Fundamentals and theory. Phys. Rep. 2019, 790, 1–48. [Google Scholar] [CrossRef]
- Agi, A.; Junin, R.; Gbadamosi, A. Mechanism governing nanoparticle flow behaviour in porous media: Insight for enhanced oil recovery applications. Int. Nano Lett. 2018, 8, 49–77. [Google Scholar] [CrossRef]
- Okonkwo, E.C.; Wole-Osho, I.; Almanassra, I.W.; Abdullatif, Y.M.; Al-Ansari, T. An updated review of nanofluids in various heat transfer devices. J. Therm. Anal. Calorim. 2020, 145, 2817–2872. [Google Scholar] [CrossRef]
- Gbadeyan, J.; Titiloye, E.; Adeosun, A. Effect of variable thermal conductivity and viscosity on Casson nanofluid flow with convective heating and velocity slip. Heliyon 2020, 6, e03076. [Google Scholar] [CrossRef] [PubMed]
- Sivashanmugam, P. Application of Nanofluids in Heat Transfer. In An Overview of Heat Transfer; INTECH Publications: Reijka, Croatia, 2012. [Google Scholar] [CrossRef]
- Morrison, N.F.; Harlen, O.G. Inkjet printing of non-Newtonian fluids. Int. Conf. Digit. Print. Technol. 2011, 2011, 360–364. [Google Scholar]
- Travnicek, P.; Krcalova, E.; Vitez, T. Rheopectic behavior of rendered fat. Pol. J. Environ. Stud. 2013, 22, 1499–1504. [Google Scholar]
- Quanji, Z. Thixotropic Behavior of Cement-Based Materials: Effect of Clay and Cement Types; Iowa State University: Ames, IA, USA, 2010; p. 126. [Google Scholar]
- Bambang, N.; Ikhsan, M.; Tensiska; Sukri, N. Mahani Rheological Properties of Honey and its Application on Honey Flow Simulation through Vertical Tube. IOP Conf. Series: Earth Environ. Sci. 2019, 334, 012041. [Google Scholar] [CrossRef]
- Irgens, F. Rheology and Non-Newtonian Fluids; Springer International Publishing: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Barman, P.C.; Kairi, R.R.; Das, A.; Islam, R. An Overview of Non-Newtonian Fluid. Int. J. Appl. Sci. Eng. 2016, 4, 97. [Google Scholar] [CrossRef]
- Green, R.G. A Fundamental Study of the Flow of Dilatant Fluids. Ph.D. Thesis, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 1966. [Google Scholar]
- Anon. Non-Newtonian Fluids: Introduction and Guide To Classification and Characteristics. Eng. Sci. Data Unit Data Items 1997, 1982, 97034. [Google Scholar]
- Worldwide, M.I. A Basic Introduction to Rheology. Whitepaper. 2016, pp. 1–19. Available online: https://cdn.technologynetworks.com/TN/Resources/PDF/WP160620BasicIntroRheology.pdf (accessed on 21 December 2022).
- Pinto, F.; Meo, M. Design and Manufacturing of a Novel Shear Thickening Fluid Composite (STFC) with Enhanced out-of-Plane Properties and Damage Suppression. Appl. Compos. Mater. 2017, 24, 643–660. [Google Scholar] [CrossRef]
- Hussain, S.; Shoeibi, S.; Armaghani, T. Impact of magnetic field and entropy generation of Casson fluid on double diffusive natural convection in staggered cavity. Int. Commun. Heat Mass Transf. 2021, 127, 105520. [Google Scholar] [CrossRef]
- Garg, J.; Poudel, B.; Chiesa, M.; Gordon, J.B.; Ma, J.J.; Wang, J.B.; Ren, Z.F.; Kang, Y.T.; Ohtani, H.; Nanda, J.; et al. Enhanced thermal conductivity and viscosity of copper nanoparticles in ethylene glycol nanofluid. J. Appl. Phys. 2008, 103, 074301. [Google Scholar] [CrossRef]
- Sahu, R.; Somashekhar, S.; Manivannan, P. Investigation on Copper Nanofluid Obtained through Micro Electrical Discharge Machining for Dispersion Stability and Thermal Conductivity. Procedia Eng. 2013, 64, 946–955. [Google Scholar] [CrossRef]
- Minea, A.A. A Review on Electrical Conductivity of Nanoparticle-Enhanced Fluids. Nanomaterials 2019, 9, 1592. [Google Scholar] [CrossRef] [PubMed]
- Seminara, G.; Lanzoni, S.; Pittaluga, M.; Solari, L.; Balmforth, N.; Provenzale, A. Geomorphological Fluid Mechanics. Lect. Notes Phys. 2001, 582, 319–338. [Google Scholar] [CrossRef]
- Aghakhani, S.; Pordanjani, A.H.; Karimipour, A.; Abdollahi, A.; Afrand, M. Numerical investigation of heat transfer in a power-law non-Newtonian fluid in a C-Shaped cavity with magnetic field effect using finite difference lattice Boltzmann method. Comput. Fluids 2018, 176, 51–67. [Google Scholar] [CrossRef]
- Rashidi, S.; Karimi, N.; Mahian, O.; Esfahani, J.A. A concise review on the role of nanoparticles upon the productivity of solar desalination systems. J. Therm. Anal. Calorim. 2019, 135, 1145–1159. [Google Scholar] [CrossRef]
- Wang, N.; Maleki, A.; AlhuyiNazari, M.; Tlili, I.; SafdariShadloo, M. Thermal Conductivity Modeling of Nanofluids Contain MgO Particles by Employing Different Approaches. Symmetry 2020, 12, 206. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Shafeeurrahaman, M. Mixed convection flow of nanofluid in a vertical channel with hall and ion-slip effects. Front. Heat Mass Transf. 2017, 8, 950–959. [Google Scholar] [CrossRef]
- Venkateswarlu, B.; Narayana, P.V.S. Cu-Al2O3/H2O hybrid nanofluid flow past a porous stretching sheet due to temperatue-dependent viscosity and viscous dissipation. Heat Transf. 2021, 50, 432–449. [Google Scholar] [CrossRef]
- Raju, A.; Ojjela, O. Effects of the induced magnetic field, thermophoresis, and Brownian motion on mixed convective Jeffrey nanofluid flow through a porous channel. Eng. Rep. 2019, 1, e12053. [Google Scholar] [CrossRef]
- Narayana, P.S.; Venkateswarlu, B.; Venkataramana, S. Thermal Radiation and Heat Source Effects on a MHD Nanofluid Past a Vertical Plate in a Rotating System with Porous Medium. Heat Transf. 2015, 44, 1–19. [Google Scholar] [CrossRef]
- Bubbico, R.; Celata, G.P.; D’Annibale, F.; Mazzarotta, B.; Menale, C. Comparison of the heat transfer efficiency of nanofluids. Chem. Eng. Trans. 2015, 43, 703–708. [Google Scholar] [CrossRef]
- Malvandi, A.; Hedayati, F.; Ganji, D.D. Fluid Flow and Heat Transfer of Nanofluids over a Flat Plate with Conjugate Heat Transfer. Chall. Nano Micro Scale Sci. Technol. 2014, 2, 108–117. [Google Scholar] [CrossRef]
- Narayana, P.V.S.; Tarakaramu, N.; Babu, D.H. Influence of chemical reaction on MHD couple stress nanoliquid flow over a bidirectional stretched sheet. Int. J. Ambient. Energy 2022, 43, 4928–4938. [Google Scholar] [CrossRef]
- Al Shdaifat, M.Y.; Zulkifli, R.; Sopian, K.; Salih, A.A. Thermal and Hydraulic Performance of CuO/Water Nanofluids: A Review. Micromachines 2020, 11, 416. [Google Scholar] [CrossRef]
- Saleem, S.; Rafiq, H.; Al-Qahtani, A.; El-Aziz, M.A.; Malik, M.Y.; Animasaun, I.L. Magneto Jeffrey Nanofluid Bioconvection over a Rotating Vertical Cone due to Gyrotactic Microorganism. Math. Probl. Eng. 2019, 2019, 1–11. [Google Scholar] [CrossRef]
- Shahzad, F.; Sagheer, M.; Hussain, S. Numerical simulation of magnetohydrodynamic Jeffrey nanofluid flow and heat transfer over a stretching sheet considering Joule heating and viscous dissipation. AIP Adv. 2018, 8, 065316. [Google Scholar] [CrossRef]
- Narayana, P.V.S.; Tarakaramu, N.; Sarojamma, G.; Animasaun, I.L. Numerical Simulation of Nonlinear Thermal Radiation on the 3D Flow of a Couple Stress Casson Nanofluid Due to a Stretching Sheet. J. Therm. Sci. Eng. Appl. 2021, 13, 021028. [Google Scholar] [CrossRef]
- Tarakaramu, N.; Narayana, P.S. Chemical Reaction Effects on Bio-Convection Nanofluid flow between two Parallel Plates in Rotating System with Variable Viscosity: A Numerical Study. J. Appl. Comput. Mech. 2019, 5, 791–803. [Google Scholar] [CrossRef]
- Naidu, K.K.; Babu, D.H.; Reddy, S.H.; Narayana, P.V.S. Radiation and Partial Slip Effects on Magnetohydrodynamic Jeffrey Nanofluid Containing Gyrotactic Microorganisms Over a Stretching Surface. J. Therm. Sci. Eng. Appl. 2021, 13, 031011. [Google Scholar] [CrossRef]
- Hussain, S.; Tayebi, T.; Armaghani, T.; Rashad, A.M.; Nabwey, H.A. Conjugate natural convection of non-Newtonian hybrid nanofluid in wavy-shaped enclosure. Appl. Math. Mech. 2022, 43, 447–466. [Google Scholar] [CrossRef]
- Hussain, S.; Pour, M.; Jamal, M.; Armaghani, T. MHD Mixed Convection and Entropy Analysis of Non-Newtonian Hybrid Nanofluid in a Novel Wavy Elbow-Shaped Cavity with a Quarter Circle Hot Block and a Rotating Cylinder. Exp. Tech. 2022, 1–20. [Google Scholar] [CrossRef]
- Gupta, S.; Gupta, S. MHD three dimensional flow of Oldroyd-B nanofluid over a bidirectional stretching sheet: DTM-Padé Solution. Nonlinear Eng. 2019, 8, 744–754. [Google Scholar] [CrossRef]
- Ibrahim, W.; Sisay, G.; Gamachu, D. Mixed convection flow of Oldroyd-B nano fluid with Cattaneo-Christov heat and mass flux model with third order slip. AIP Adv. 2019, 9, 125023. [Google Scholar] [CrossRef]
- Awad, F.G.; Ahamed, S.M.S.; Sibanda, P.; Khumalo, M. The Effect of Thermophoresis on Unsteady Oldroyd-B Nanofluid Flow over Stretching Surface. PLOS ONE 2015, 10, e0135914. [Google Scholar] [CrossRef] [PubMed]
- Sulaiman, M.; Ali, A.; Islam, S. Heat and Mass Transfer in Three-Dimensional Flow of an Oldroyd-B Nanofluid with Gyrotactic Micro-Organisms. Math. Probl. Eng. 2018, 2018, 1–15. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Alharbi, S.O.; Thounthong, P.; Nisar, K.S. Numerical Solution of Casson Nanofluid Flow Over a Non-linear Inclined Surface with Soret and Dufour Effects by Keller-Box Method. Front. Phys. 2019, 7, 1–13. [Google Scholar] [CrossRef]
- Shah, Z.; Kumam, P.; Deebani, W. Radiative MHD Casson Nanofluid Flow with Activation energy and chemical reaction over past nonlinearly stretching surface through Entropy generation. Sci. Rep. 2020, 10, 4402. [Google Scholar] [CrossRef]
- Oyelakin, I.S.; Mondal, S.; Sibanda, P. Unsteady Casson nanofluid flow over a stretching sheet with thermal radiation, convective and slip boundary conditions. Alex. Eng. J. 2016, 55, 1025–1035. [Google Scholar] [CrossRef]
- Alzahrani, A.K.; Sivasankaran, S.; Bhuvaneswari, M. Numerical Simulation on Convection and Thermal Radiation of Casson Fluid in an Enclosure with Entropy Generation. Entropy 2020, 22, 229. [Google Scholar] [CrossRef]
- Cabaleiro, D.; Pastoriza-Gallego, M.J.; Gracia-Fernández, C.; Piñeiro, M.M.; Lugo, L. Rheological and volumetric properties of TiO2-ethylene glycol nanofluids. Nanoscale Res. Lett. 2013, 8, 286. [Google Scholar] [CrossRef]
- Pötschke, P.; Fornes, T.; Paul, D. Rheological behavior of multiwalled carbon nanotube/polycarbonate composites. Polymer 2002, 43, 3247–3255. [Google Scholar] [CrossRef]
- Phuoc, T.X.; Massoudi, M.; Chen, R.-H. Viscosity and thermal conductivity of nanofluids containing multi-walled carbon nanotubes stabilized by chitosan. Int. J. Therm. Sci. 2011, 50, 12–18. [Google Scholar] [CrossRef]
- Aladag, B.; Halelfadl, S.; Doner, N.; Maré, T.; Duret, S.; Estellé, P. Experimental investigations of the viscosity of nanofluids at low temperatures. Appl. Energy 2012, 97, 876–880. [Google Scholar] [CrossRef]
- Tamjid, E.; Guenther, B.H. Rheology and colloidal structure of silver nanoparticles dispersed in diethylene glycol. Powder Technol. 2010, 197, 49–53. [Google Scholar] [CrossRef]
- Moghaddam, M.B.; Goharshadi, E.K.; Entezari, M.H.; Nancarrow, P. Preparation, characterization, and rheological properties of graphene–glycerol nanofluids. Chem. Eng. J. 2013, 231, 365–372. [Google Scholar] [CrossRef]
- Aziz, T.; Mahomed, F.M. Applications of Group Theoretical Methods to Non-Newtonian Fluid Flow Models: Survey of Results. Math. Probl. Eng. 2017, 2017, 1–43. [Google Scholar] [CrossRef]
- Pawlek, R.A.; Tien, C. Laminar heat transfer to non-Newtonian fluids in the entrance region of a circular conduit. Can. J. Chem. Eng. 1964, 42, 222–227. [Google Scholar] [CrossRef]
- Malek, J. Introduction to non-Newtonian fluid mechanics. Von Karman Inst. Fluid Dyn. Non-Newton. Flows 2012, 57, 11–34. [Google Scholar]
- Yang, L.; Du, K. A comprehensive review on the natural, forced, and mixed convection of non-Newtonian fluids (nanofluids) inside different cavities. J. Therm. Anal. Calorim. 2020, 140, 2033–2054. [Google Scholar] [CrossRef]
- Sochi, T. Pore-Scale Modeling of Non-Newtonian Flow in Porous Media. arXiv 2010, arXiv:1011.076. [Google Scholar]
- Robertson, A.M. Lecture Notes on Non-Newtonian Fluids Part I: Inelastic Fluids. University of Pittsburgh Press: Pittsburgh, PA, USA, 2005. [Google Scholar]
- Sochi, T. Modeling the Flow of a Bautista-Manero Fluid in Porous Media. arXiv 2009, arXiv:0905.1960,. [Google Scholar] [CrossRef]
- Maleki, H.; Safaei, M.R.; Togun, H.; Dahari, M. Heat transfer and fluid flow of pseudo-plastic nanofluid over a moving permeable plate with viscous dissipation and heat absorption/generation. J. Therm. Anal. Calorim. 2019, 135, 1643–1654. [Google Scholar] [CrossRef]
- Ibrahim, W.; Negera, M. Melting and viscous dissipation effect on upper-convected Maxwell and Williamson nanofluid. Eng. Rep. 2020, 2, e12159. [Google Scholar] [CrossRef]
- Arani, A.A.A.; Sheikhzadeh, G.A.; Arani, A.G. Study of Fluid Flow and Heat Transfer of AL2O3-Water as a Non-Newtonian Nanofluid through Lid-Driven Enclosure. Chall. Nano Micro Scale Sci. Technol. 2014, 2, 118–131. [Google Scholar] [CrossRef]
- Naseem, F.; Shafiq, A.; Zhao, L.; Naseem, A. MHD biconvective flow of Powell Eyring nanofluid over stretched surface. AIP Adv. 2017, 7, 065013. [Google Scholar] [CrossRef]
- Kamran, A.; Hussain, S.; Sagheer, M.; Akmal, N. A numerical study of magnetohydrodynamics flow in Casson nanofluid combined with Joule heating and slip boundary conditions. Results Phys. 2017, 7, 3037–3048. [Google Scholar] [CrossRef]
- Sulochana, C.; Ashwinkumar, G. Carreau Model for Liquid Thin Film Flow of Dissipative Magnetic-Nanofluids over a Stretching Sheet. Int. J. Hybrid Inf. Technol. 2017, 10, 239–254. [Google Scholar] [CrossRef]
- Eldabe, N.; Abou-Zeid, M. Homotopy perturbation method for MHD pulsatile non-Newtonian nanofluid flow with heat transfer through a non-Darcy porous medium. J. Egypt. Math. Soc. 2017, 25, 375–381. [Google Scholar] [CrossRef]
- Hayat, T.; Aziz, A.; Muhammad, T.; Alsaedi, A. A revised model for Jeffrey nanofluid subject to convective condition and heat generation/absorption. PLOS ONE 2017, 12, e0172518. [Google Scholar] [CrossRef]
- Aziz, A.; Shams, M. Entropy generation in MHD Maxwell nanofluid flow with variable thermal conductivity, thermal radiation, slip conditions, and heat source. AIP Adv. 2020, 10, 015038. [Google Scholar] [CrossRef]
- Hashemi, S.; Shokouhmand, H. Study on the Vertical Wall Heat Transfer by Numerical Simulation of Non-Newtonion (Herschel-Bulkley Model) Nanofluid and Changes. J. Mater. Environ. Sci. 2018, 9, 1418–1430. [Google Scholar]
- Ellahi, R.; Aziz, S.; Zeeshan, A. Non-Newtonian nanofluid flow through a porous medium between two coaxial cylinders with heat transfer and variable viscosity. J. Porous Media 2013, 16, 205–216. [Google Scholar] [CrossRef]
- Pahlevaninejad, N.; Rahimi, M.; Gorzin, M. Thermal and hydrodynamic analysis of non-Newtonian nanofluid in wavy microchannel. J. Therm. Anal. Calorim. 2020, 143, 811–825. [Google Scholar] [CrossRef]
- Maghsoudi, P.; Shahriari, G.; Rasam, H.; Sadeghi, S. Flow and natural convection heat transfer characteristics of non-Newtonian nanofluid flow bounded by two infinite vertical flat plates in presence of magnetic field and thermal radiation using Galerkin method. J. Central South Univ. 2019, 26, 1294–1305. [Google Scholar] [CrossRef]
- Yang, L.; Du, K.; Zhang, Z. Heat transfer and flow optimization of a novel sinusoidal minitube filled with non-Newtonian SiC/EG-water nanofluids. Int. J. Mech. Sci. 2020, 168, 105310. [Google Scholar] [CrossRef]
- Singh, A.K.; Kishore, N. Laminar Mixed Convection of Non-Newtonian Nanofluids Flowing Vertically Upward Across a Confined Circular Cylinder. J. Therm. Sci. Eng. Appl. 2018, 10, 041012. [Google Scholar] [CrossRef]
- Rao, A.S.; Sainath, S.; Rajendra, P.; Ramu, G. Mathematical Modelling of Hydromagnetic Casson non-Newtonian Nanofluid Convection Slip Flow from an Isothermal Sphere. Nonlinear Eng. 2019, 8, 645–660. [Google Scholar] [CrossRef]
- Rajkotwala, A.; Banerjee, J. Influence of rheological behavior of nanofluid on heat transfer. WSEAS Trans. Heat Mass Transf. 2013, 8, 67–81. [Google Scholar]
- Mahdy, A.; Chamkha, A.J. Heat transfer and fluid flow of a non-Newtonian nanofluid over an unsteady contracting cylinder employing Buongiorno’s model. Int. J. Numer. Methods Heat Fluid Flow 2015, 25, 703–723. [Google Scholar] [CrossRef]
- Agbaje, T.; Mondal, S.; Motsa, S.; Sibanda, P. A numerical study of unsteady non-Newtonian Powell-Eyring nanofluid flow over a shrinking sheet with heat generation and thermal radiation. Alex. Eng. J. 2017, 56, 81–91. [Google Scholar] [CrossRef]
- Akinshilo, A.; Adingwupu, A.; Olofinkua, J. Further Evaluation of Squeezing Flow and Heat Transfer of non-Newtonian Fluid with Nanoparticles Conveyed through Vertical Parallel Plates. J. Mech. Eng. 2019, 3, 15–24. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Bhatti, M.M. The study of non-Newtonian nanofluid with hall and ion slip effects on peristaltically induced motion in a non-uniform channel. RSC Adv. 2018, 8, 7904–7915. [Google Scholar] [CrossRef] [PubMed]
- Loenko, D.S.; Shenoy, A.; Sheremet, M.A. Natural Convection of Non-Newtonian Power-Law Fluid in a Square Cavity with a Heat-Generating Element. Energies 2019, 12, 2149. [Google Scholar] [CrossRef]
- Ling, Z.; He, Z.; Xu, T.; Fang, X.; Gao, X.; Zhang, Z. Experimental and Numerical Investigation on Non-Newtonian Nanofluids Flowing in Shell Side of Helical Baffled Heat Exchanger Combined with Elliptic Tubes. Appl. Sci. 2017, 7, 48. [Google Scholar] [CrossRef]
- Shamsi, M.R.; Akbari, O.A.; Marzban, A.; Toghraie, D.; Mashayekhi, R. Increasing heat transfer of non-Newtonian nanofluid in rectangular microchannel with triangular ribs. Phys. E: Low-dimensional Syst. Nanostructures 2017, 93, 167–178. [Google Scholar] [CrossRef]
- Hojjat, M. Modeling heat transfer of non-Newtonian nanofluids using hybrid ANN-Metaheuristic optimization algorithm. J. Part. Sci. Technol. 2017, 3, 233–241. [Google Scholar] [CrossRef]
- Jahanbakhshi, A.; Nadooshan, A.A. Numerical study of non-Newtonian fluid convection by power law model in a square enclosure with central heating source. Modares Mech. Eng. 2018, 17, 343–352. [Google Scholar]
- Zhang, Z.; Xie, Y.; Zhang, D.; Xie, G. Flow Characteristic and Heat Transfer for Non-Newtonian Nanofluid in Rectangular Microchannels with Teardrop Dimples/Protrusions. Open Phys. 2017, 15, 197–206. [Google Scholar] [CrossRef]
- Asl, M.S.; Toghraie, D.; Azimian, A.R. Numerical simulation of convective heat transfer in a turbulant non-Newtonian nanofluid flow through a horizontal circular tube. J. Model. Eng. 2018, 16, 113–120. (In Persian) [Google Scholar]
- Nabwey, H.A. A Hybrid Methodology to Extract Decision Rules of Heat and Mass Transfer of the Flow of a Non-Newtonian Nanofluid Towards a Vertical Stretching Surface. J. Nanofluids 2020, 9, 121–127. [Google Scholar] [CrossRef]
- Sajadifar, S.A.; Karimipour, A.; Toghraie, D. Fluid flow and heat transfer of non-Newtonian nanofluid in a microtube considering slip velocity and temperature jump boundary conditions. Eur. J. Mech.-B/Fluids 2017, 61, 25–32. [Google Scholar] [CrossRef]
- Akbari, O.A.; Toghraie, D.; Karimipour, A.; Marzban, A.; Ahmadi, G.R. The effect of velocity and dimension of solid nanoparticles on heat transfer in non-Newtonian nanofluid. Phys. E: Low-dimensional Syst. Nanostructures 2017, 86, 68–75. [Google Scholar] [CrossRef]
- Ternik, P.; Rudolf, R.; Žunič, Z. Heat-transfer characteristics of a non-newtonian Au nanofluid in a cubical enclosure with differentially heated side walls. Mater. Tehnol. 2015, 49, 87–93. [Google Scholar]
- Zhang, Y.; Zhang, M.; Bai, Y. Unsteady flow and heat transfer of power-law nanofluid thin film over a stretching sheet with variable magnetic field and power-law velocity slip effect. J. Taiwan Inst. Chem. Eng. 2017, 70, 104–110. [Google Scholar] [CrossRef]



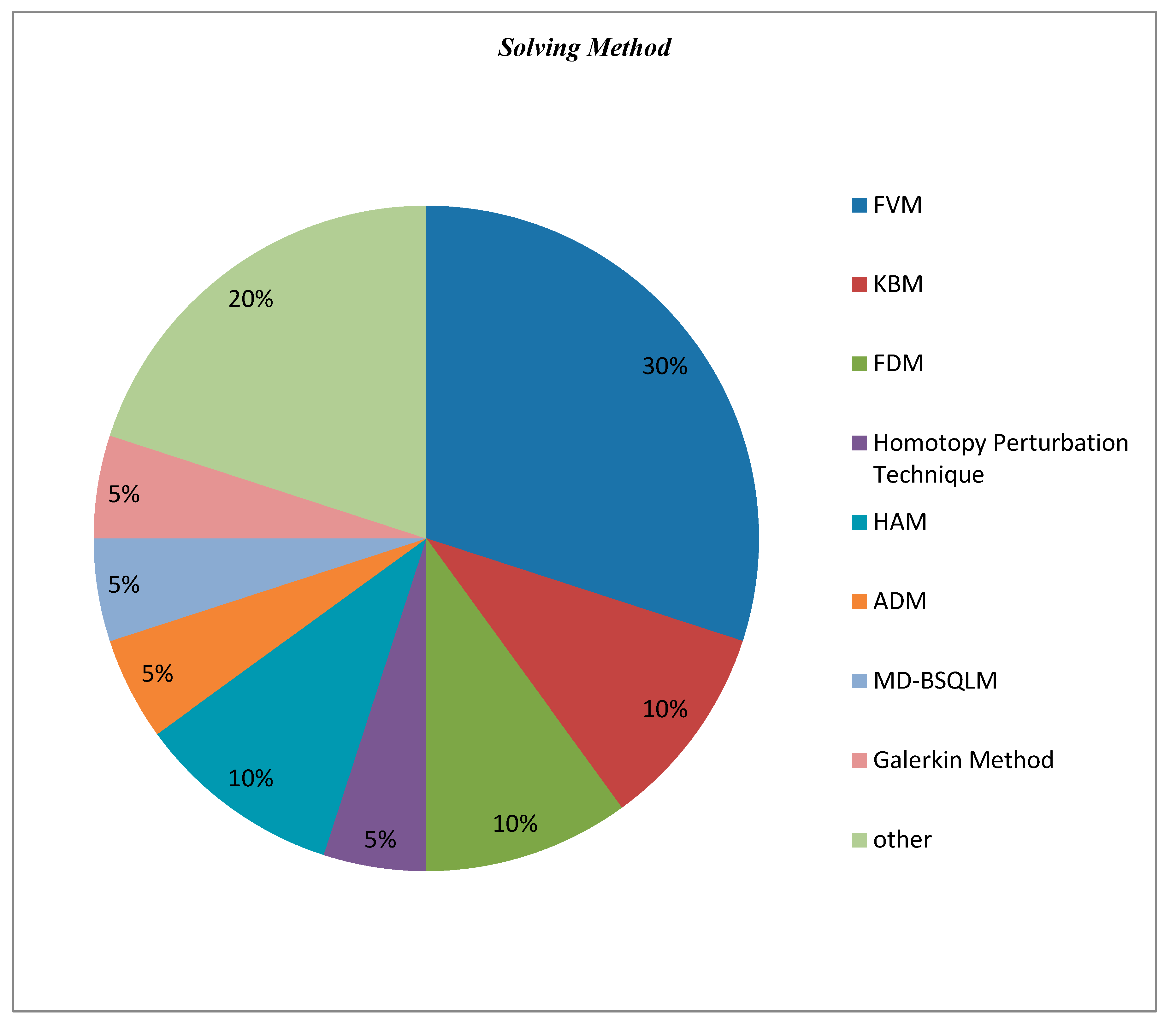
| Model Name | Applicability | |
|---|---|---|
| 1 | PowerLaw [63] (Ostwald de Waele) | The most popular model |
| 2 | Carreau [3] | At extra high and extra low shear rates |
| 3 | Ellis [64] | for low shear rates, it would be better to use the Ellis model |
| 4 | Cross [65] | at low and high shear rates |
| 5 | Bingham plastic [66] | Shear-Thickening or Dilatant Viscoelastic fluid |
| 6 | Herschel–Bulkley [67] | Derivation from Bingham for nonlinear fluid in |
| 7 | Casson [68] | Best model for foodstuffs and biological materials: blood and other fluids in food industrial yoghurt, chocolate and…. |
| 8 | Maxwell Model [47] | viscoelastic |
| 9 | Jeffreys Model [46] | viscoelastic |
| 10 | Upper Convected Maxwell (UCM) Model [63] | viscoelastic |
| 11 | Oldroyd-B Model [67] | second simplest nonlinear viscoelastic model very popular model |
| 12 | Bautista–Manero Model [69] | viscoelasticity and the Fredrickson’s kinetic equation |
| 13 | Godfrey Model [67] | time dependence of thixotropic fluids |
| Ref | Geometry/Problem Description | Remarks and Methodology | Variable Parameters | Highlight Results | Type of Non-Newtonian Nano fluid |
|---|---|---|---|---|---|
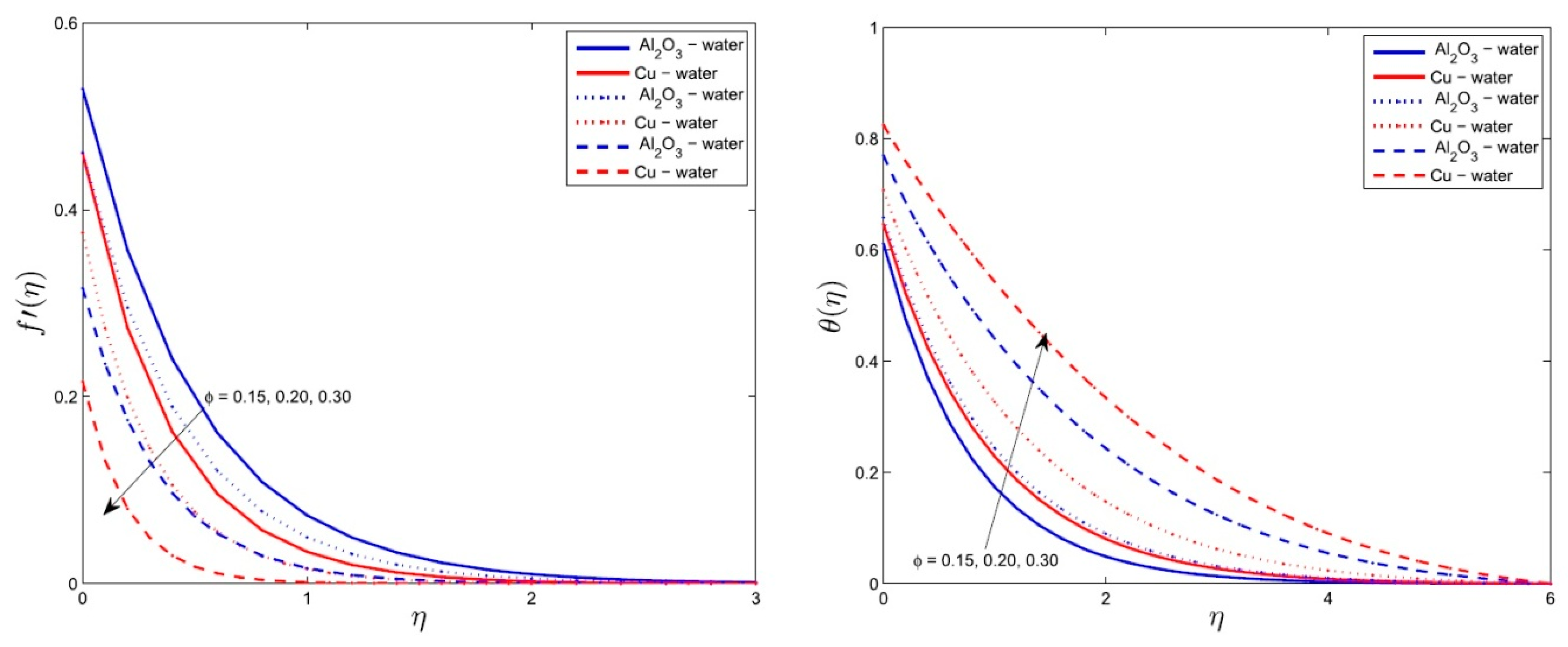
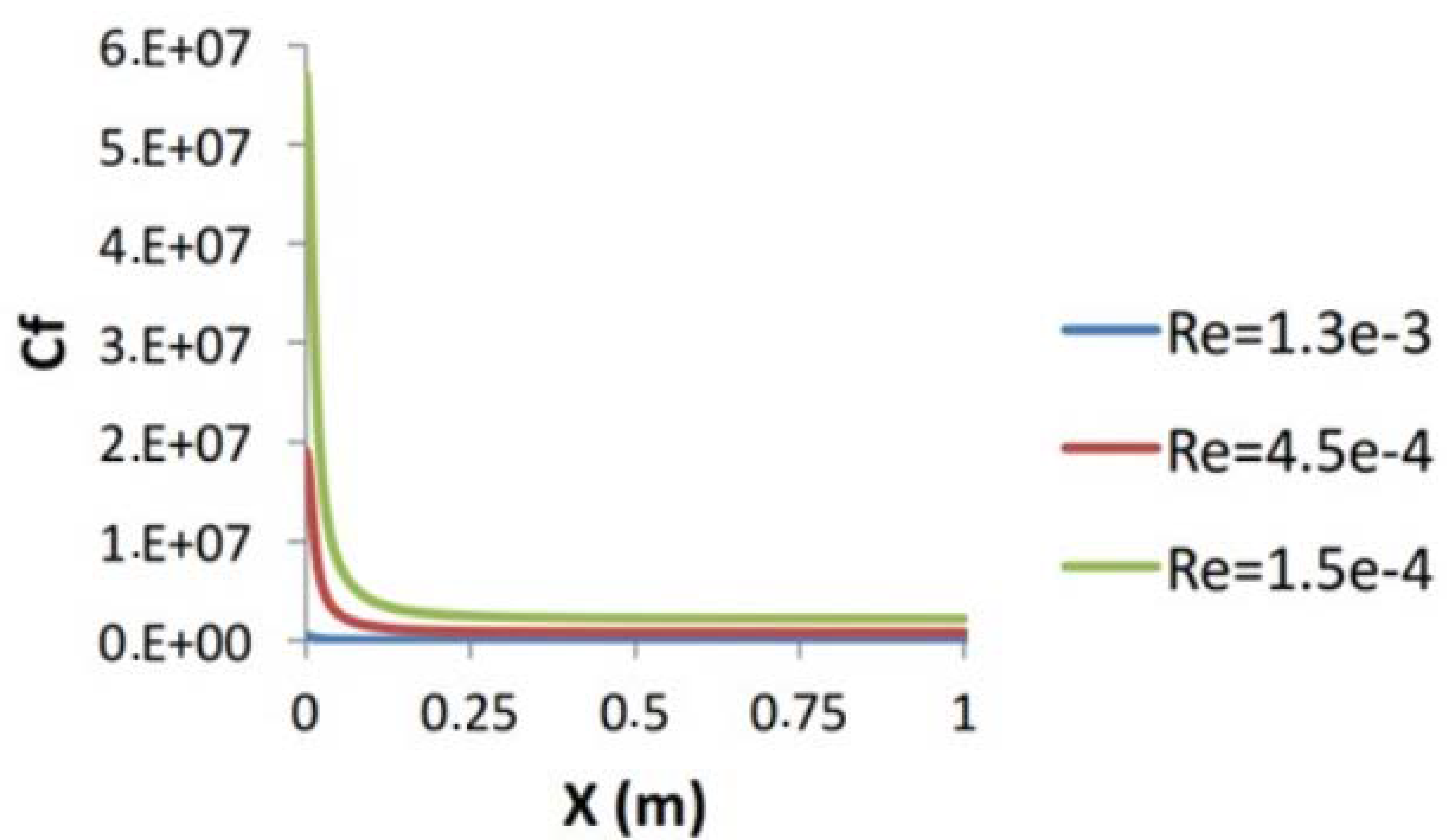
| Hashemi and shokouhmand [79] | 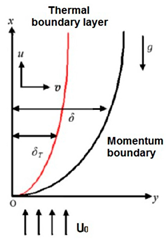 | Numerical FLUENT Laminar | Re = 1.3,1.4,4.5 * 10−4 Φw=1–5% | Nuave ↥ via Φ = 4 and ↧ by more than 4. Brinkman↑Nu↑ | Al2O3 Propellant Dough (35/45) Herschel-Bulkley (model) |
| Ellahi et al. [80] | Non-Newtonian nanofluid flow through a porous media between 2 coaxial cylinder | HAM (Homotopy analysis method) Porous medium | R = 1.0–2.0 Nb = Nt = 1.0–4.0 | Nanoconcentration ↥ Nt and thermophoresis is the opposite of Brownian motion for thermal boundary. | Blood modified Darcy’s law model |
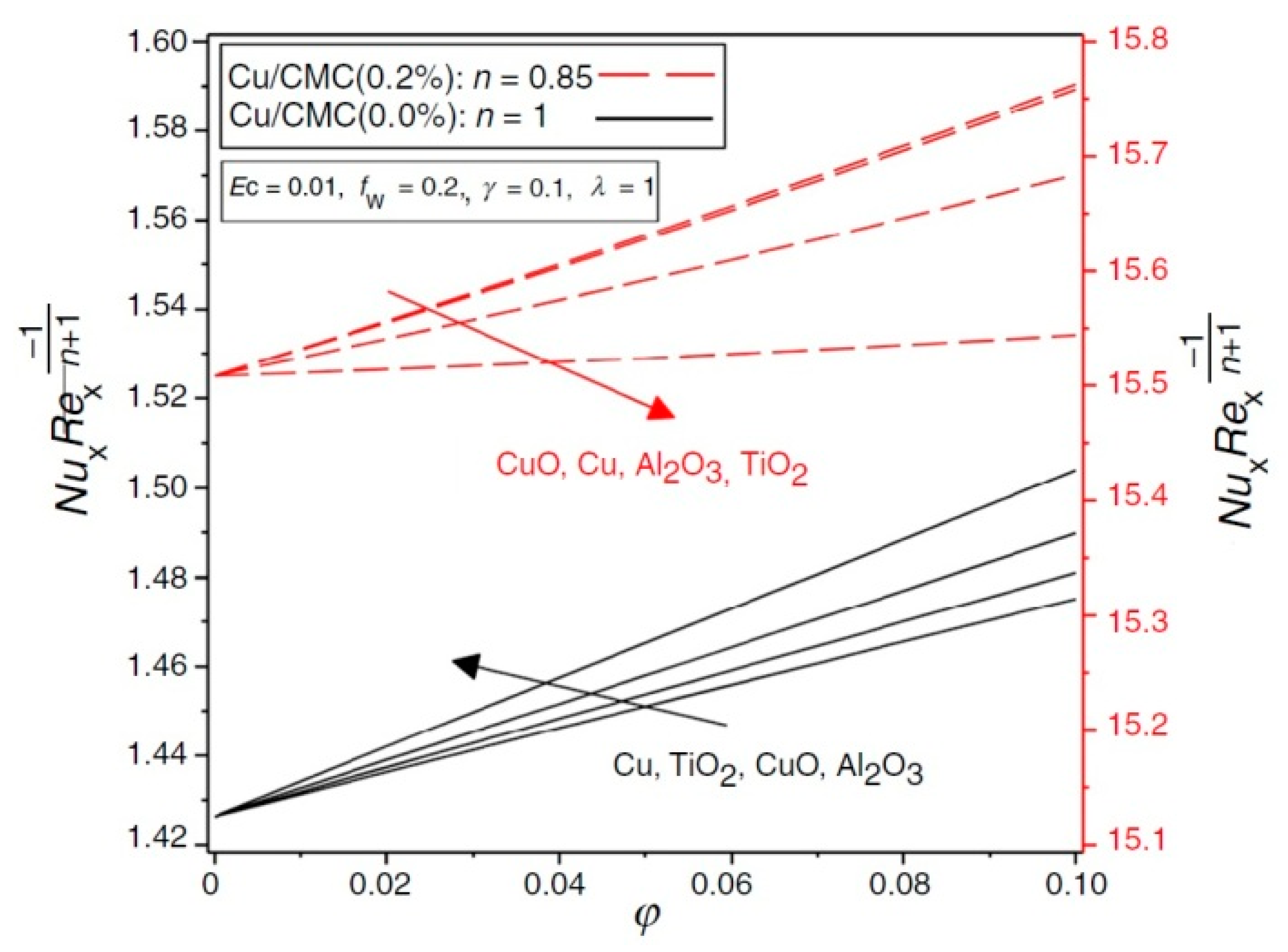
| Pahlevaninejad et al. [81] | wavy microchannel | FVM Fluent constant heat flux | Re = 5–300 φ = 0.005–0.050 | volume fraction → Nuave nanoparticle diameter→ Nuave ↧ Reand volume fraction→ Pressure drop | Water + CMC (non-Newtonian base flow), Al2O3, (nano particles) power-law |
| Maghsoudi et al. [82] | Two infinite vertical flat plates +magnetic field + thermal radiation | Galerkin method (GM) Natural Convective Magnetic field Thermal Radiation | Ha = 0–100 Ec =2–92 φ = 0.005–0.010 N = 0–100 (Radiation dimensionless parameter) | Magnetic parametervelocity ↧ skin friction ↧ Nu ↧ non-Newtonian viscosityNu ↧ and skin friction ↧ Ecskin friction ↧ Nu | Water CuO Comment: (non-Newtonian model is not mentioned) |
| Yang et al. [83] | sinusoidal minitube | FVM Sinusoidal-wavy Mini-tube | Re = 5000–15,000 θ = 0–180 particle size= 16–90nm | water thermal properties better than EG/water. EG/water @ dnp = 90 nm the maximum mean Nu | ethylene-glycol/water (Base fluid) Silicon-Carbide (Nano particle) power-law |
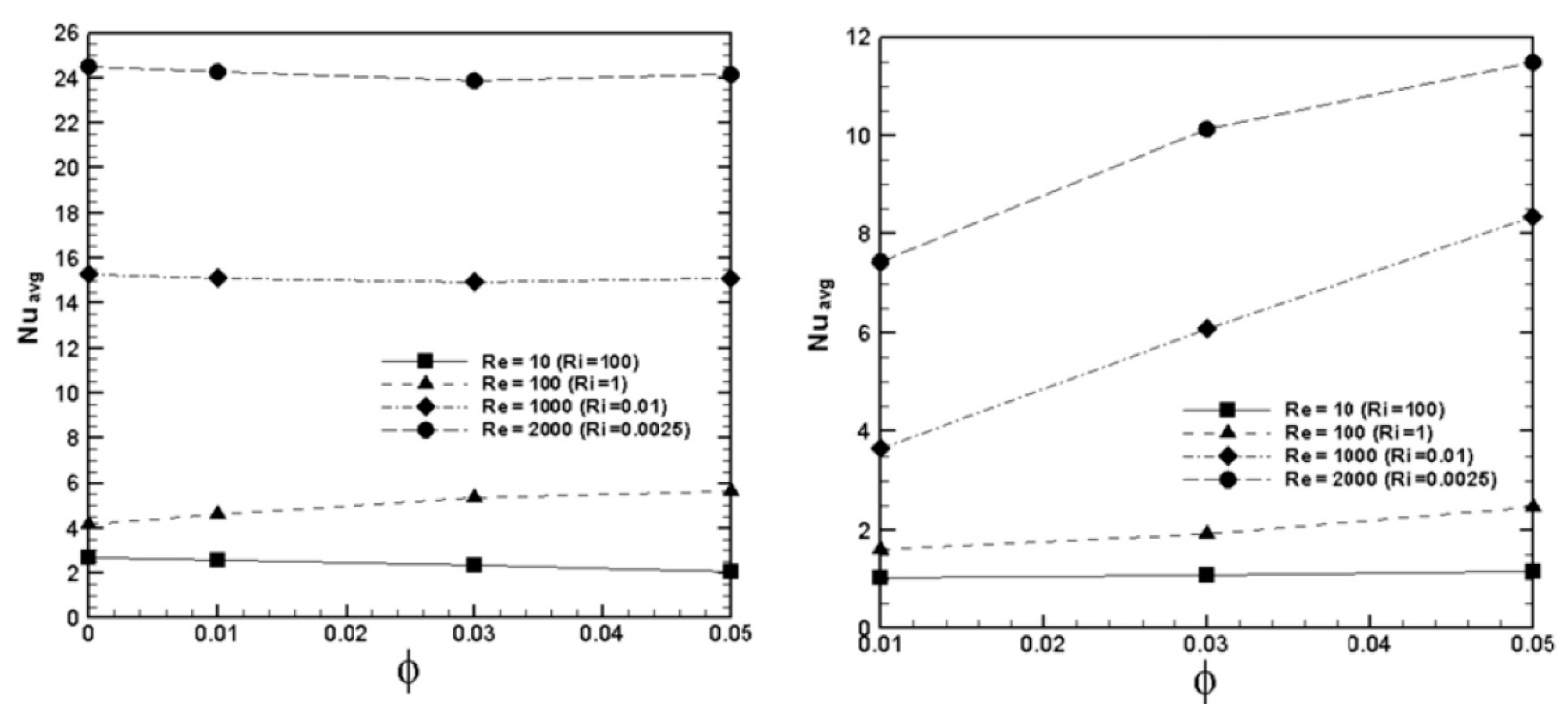
| Singah and Kishore [84] | Non-Newtonian Nanofluids Flowing Vertically Upward Across a Confined Circular Cylinder | FVM with Fluent Laminar Mixed | Re = 1–40 φ = 0.005–0.045 Ri = 0–40 λ = 0.0625–0.5 (confinement ratio of circular cylinder) | Reheat transfer φheat transfer Riheat transfer Kheat transfer At φ >0.005 and Ri < 2Nuave ↧ with ↥ Ri | shear-thinning power-law (TYPE OF NANOFLUID IS NOT MENTIONED) |
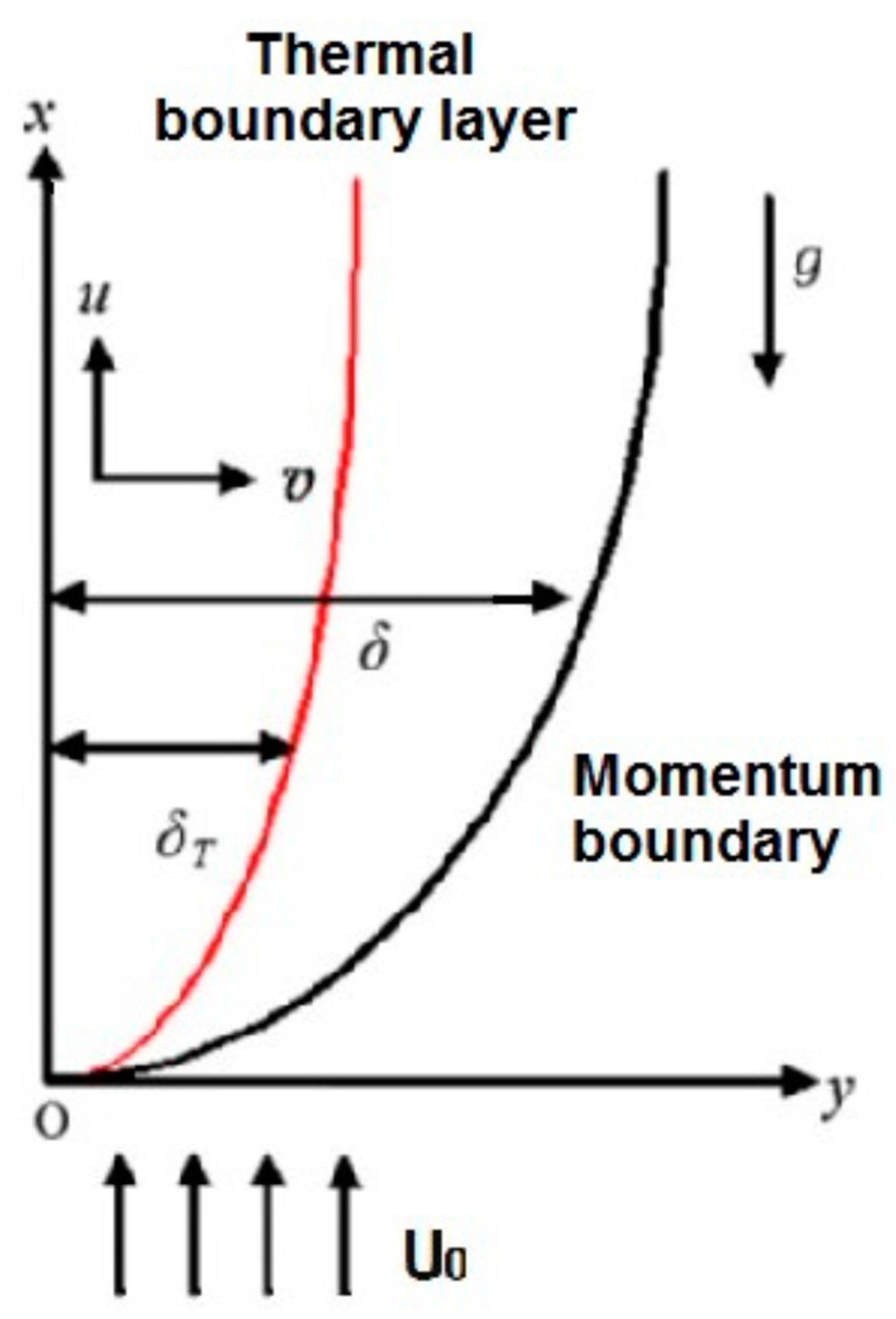
| Rao et al. [85] | 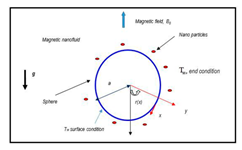 | KBM (MHD) | ζ = 0–90 Nt = 0.02–0.2 St = 0.5–3.0 Nb = 0.01–0.4 Le = 5–50 Pr = 7–100 | ↥ (Casson) parameter decelerates the flow and also ↧ thermal and nanoparticle concentration boundary layer thickness. ↥ thermal slip strongly ↧ velocities, temperatures and nano-particle concentrations. | Casson viscoplastic model |
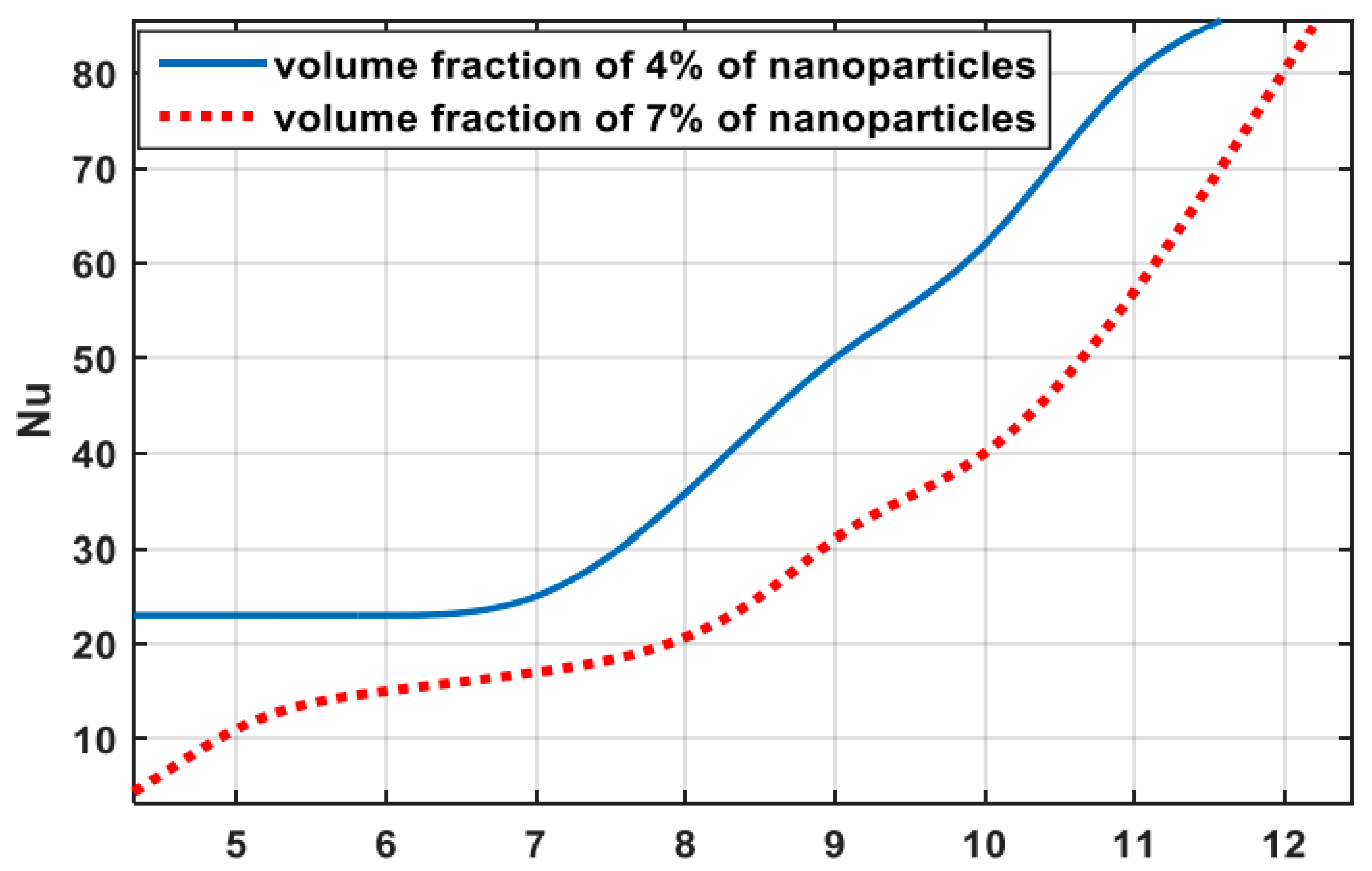
| Rajkotwala and Banerjee [86] | 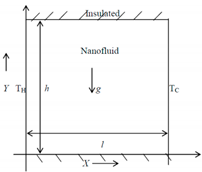 | FVM Natural | Ra = 104–106 φ = 0.05%–5% | ↥ φ, ↧ heat transfer for non-Newtonian models and ↥ for Newtonian model. | Water Copper Ostwald–de Waele model |
| Mahdy and Chamkha [87] | non-Newtonian nanofluid over an unsteady contracting cylinder | Numerical MATLAB Buongiorno Unsteady | Nt = 0.3–1.0, fw = (−0.2, 0.2), β = 0.4–5.0 Le = 1.0–50 Pr = 0.3–20 | The heat and mass transfer rates ↧ via ↥ unsteadiness parameters and Brownian motion. | Casson fluid model |
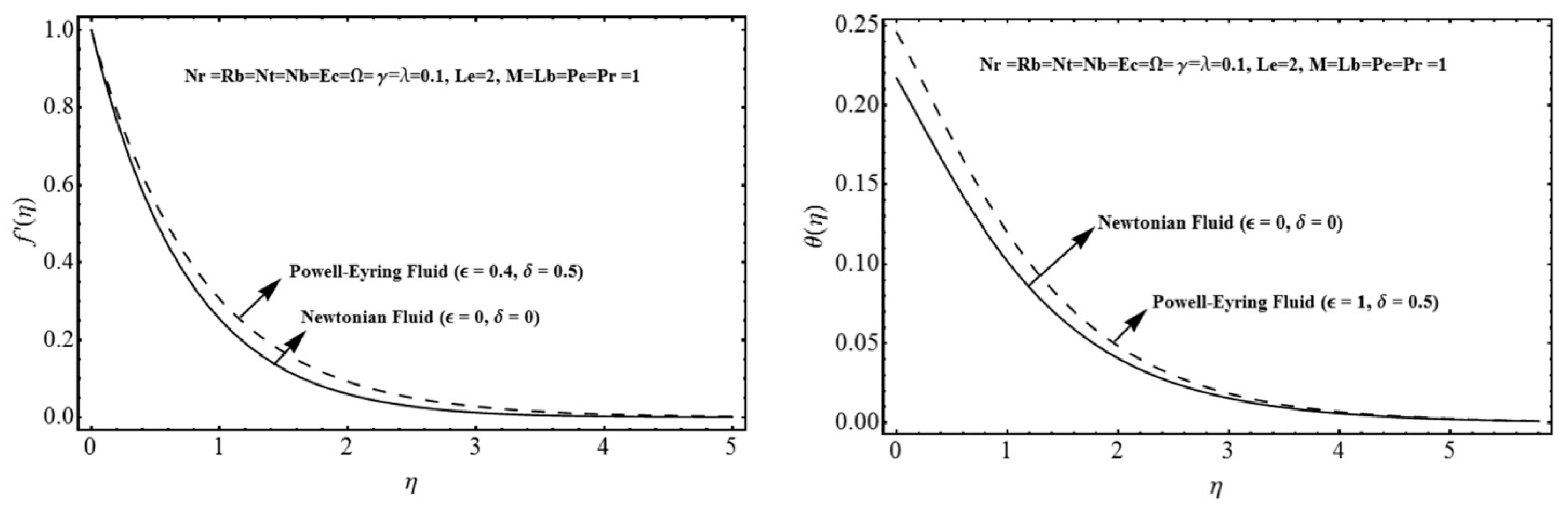
| Agbajeet al. [88] | 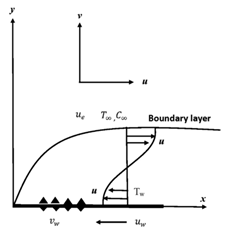 | (MD-BSQLM) METHOD Heat Generation, Thermal Radiation | Pr = 0.3–7.0; Fw= −1.0–1.0 Nb = 0.3–0.9 | ε and δ momentum boundary-layer thickness and The heat generationand thermal radiation enhancetemperature and thermal boundary-layer thickness | Powell-Eyring, |
| Akinshilo et al. [89] | 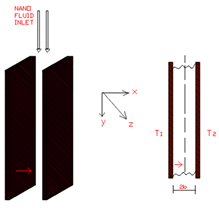 | Adomian decomposition method (ADM) | δ = Ec = 0.5–1, pr = 0.6–2.0 φ = 0.01%–0.05% | Silver provides ↥ heat transfer rates with ↥ thermal conductivity on the other hand ↧ temperature distribution compared with Alumina. | water Sodium Alginate (SA) silver Ag and Al2O3 |
| Abdelsalam and Bhattic [90] | 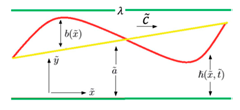 | Homotopy Perturbation Technique Non-uniform Channel Peristaltic | Nb = 0.5–1.5 Nt = 0.5–2.0 Β = 0.1–3.0 M = 0.0–0.8 | ↥ Brownian parameter thermal conductivity will ↥ , but ↧ in concentration profile. and without magnet, the pressure ↥ attains the highest value, and the friction force attains the ↧ value | Blood power-law |
| Loenko et al. [91] | 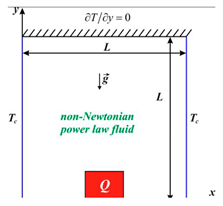 | FDM Natural Convection Heat-Generating | Ra = 104–106 N = 0.8–1.4 K = 1–1000 | ↥ Ra the average Nusselt ↥ . | Power-Law |
| Ling et al. [92] |  | (FVM) FLUENT k-ε model Elliptic tube Experimental | H = 35–78 φ = 0.2%–1% Re =200–800 Nu = 26–50 Eu = 14–23 | By adding nanoparticles heat transfer ↥ and for better heat transfer for xgbase fluid and heat transfer ↥ by MWCNTs. | xanthan gum (XG) (Base Fluid) MWCNT (Nano particles) power-law |
| Shamsi et al. [93] | Rectangular microchannel with triangular ribs | Laminar flow Microchannel Triangular ribs | 5 < Re < 300 φ = 0%–2% L1 = 4 Nuave=0–30 Θ = 30–60 | ↥ nanoparticles lead to ↥ heat transfer ↧ diameter of nanoparticles lead to ↥ heat transfer angle of 30° lead to max Nusselt number and the min pressure drop. | Water Carboxy methyl cellulose (CMC) Al2O3 Power-law |
| Hojjat [94] | 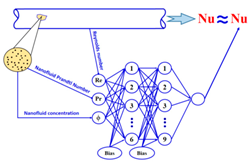 | An optimal artificial neural network (ANN) turbulent regime | Pe = 190,000–350,000 Nu = 90–230 39 <Pr< 71 | ANN predicts the Nusselt number of nanofluids better than the previously correlation. The maxand average absolute relative deviations of the ANN are almost half and one fifth of the values predicted by the correlation in the literature | Al2O3 TiO2 CuO |
| JahanbakhshinandNadooshan [95] | square enclosure with central heating source | FDM Natural Convection Heat Generation | Ra = 104–106 φ = 0.05%–5% n = 0.75–1.4 | Ra for the start of natural convection in the square enclosure is ↧ by ↥ the power law indexstrongernatural convection causes ↥ Nusselt. | Power-Law |
| SHARIFI ASL et al. [96] |  | (CFD) TURBULENT FLOW | Nu = 100–180 φ = 0. 5%–1.5% | ↥ heat transfer coefficient and Nusselt number using non-Newtonian nanofluid. with direct relationship with the volume fraction of the nanoparticles and the Re. | carboxymethyl cellulose (CMC) power-law A12O3 |
| Zhang, et al. [97] |  | FLUENT steady-state laminar flow Microchannel Heat sink | D = 0.2–0.3 V = 1.41–8.69 Vfr = 0.5%–3.5%. Re < 450 | Nu/Nu0 ↥ as inlet velocity ↥ . Al2O3-water nanofluids have superior heat transfer performance than water flowing insmoothmicrochannel | Al2O3 Water power-law shear-thinning model |
| Hussain et al. [98] | Conjugate natural convection +hybrid nanofluid + wavy-shaped enclosure | Galerkin-based finite element method | φ = 1% | The best overall heat transfer rate is achieved at AR = 0.4 for the pseudo-plastic fluid (n=0.6), at AR = 0.5 for the Newtonian fluid (n=1), and at AR = 0.6 for dilatant fluid (n ˃1). | Ag-MgO |
| Sajadifar et al. [99] | Microtube considering slip velocity +temperature jump boundary conditions | FVM non-uniform Forced convection | Φ = 0.0%–5% Re = 0–1600 Nu= 3–12 | ↥ slip coefficient causes ↥ Nu at ↥ Re which meant averaged Nusselt number ↥ due to slip flow regime. | CMC–aluminum oxide under the slip flow |
| Akbari et al. [100] | two-dimensional microchannel with hydrodynamic and temperature fixed boundary conditions | FVM Laminar forced flow | 10 ≤ Re ≤ 1000 Φ = 0.0%–0.5% T = 300–330 Pe = 80–2000 | ↥ volume fraction of the solid nanofluid lead to ↥ in heat transfer rate, Nu and pressure loss. | carboxy methyl cellulose (CMC) power-law |
| Ternik et al. [101] | 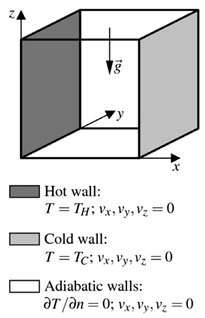 | FVM Natural Convection | 101< Ra< 106 0% <φ < 10% | At a fixed value of the base-fluid Rabf, the nanofluid Ra nf ↧ with the volume fraction of the nanoparticles. Nu ↥ with the ↥ values of the Rabf | carboxymethyl cellulose (CMC) based gold (Au) Al2O3, Cu, TiO power-law |
| Zhang et al. [102] | stretching sheet with variable magnetic field and power-law velocity slip effect | Numerical DTM–NIM | S (unsteadiness) = 0.8–1.4 Pr = 0.1–5.0 M (Hartmann) = 0.1–2 N (power law index) = 0.76–1.0 | Hartmann number ↑ velocity ↥ CuO–EVA nanofluid has better enhancement on heat transfer than TiO2/Al2O3–EVA. | Al2O3 CuO TiO2 EVA (Base fluid) Power law |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nabwey, H.A.; Rahbar, F.; Armaghani, T.; Rashad, A.M.; Chamkha, A.J. A Comprehensive Review of Non-Newtonian Nanofluid Heat Transfer. Symmetry 2023, 15, 362. https://doi.org/10.3390/sym15020362
Nabwey HA, Rahbar F, Armaghani T, Rashad AM, Chamkha AJ. A Comprehensive Review of Non-Newtonian Nanofluid Heat Transfer. Symmetry. 2023; 15(2):362. https://doi.org/10.3390/sym15020362
Chicago/Turabian StyleNabwey, Hossam A., Farhad Rahbar, Taher Armaghani, Ahmed. M. Rashad, and Ali J. Chamkha. 2023. "A Comprehensive Review of Non-Newtonian Nanofluid Heat Transfer" Symmetry 15, no. 2: 362. https://doi.org/10.3390/sym15020362
APA StyleNabwey, H. A., Rahbar, F., Armaghani, T., Rashad, A. M., & Chamkha, A. J. (2023). A Comprehensive Review of Non-Newtonian Nanofluid Heat Transfer. Symmetry, 15(2), 362. https://doi.org/10.3390/sym15020362









