The Fuzzy Bit
Abstract
:1. Introduction
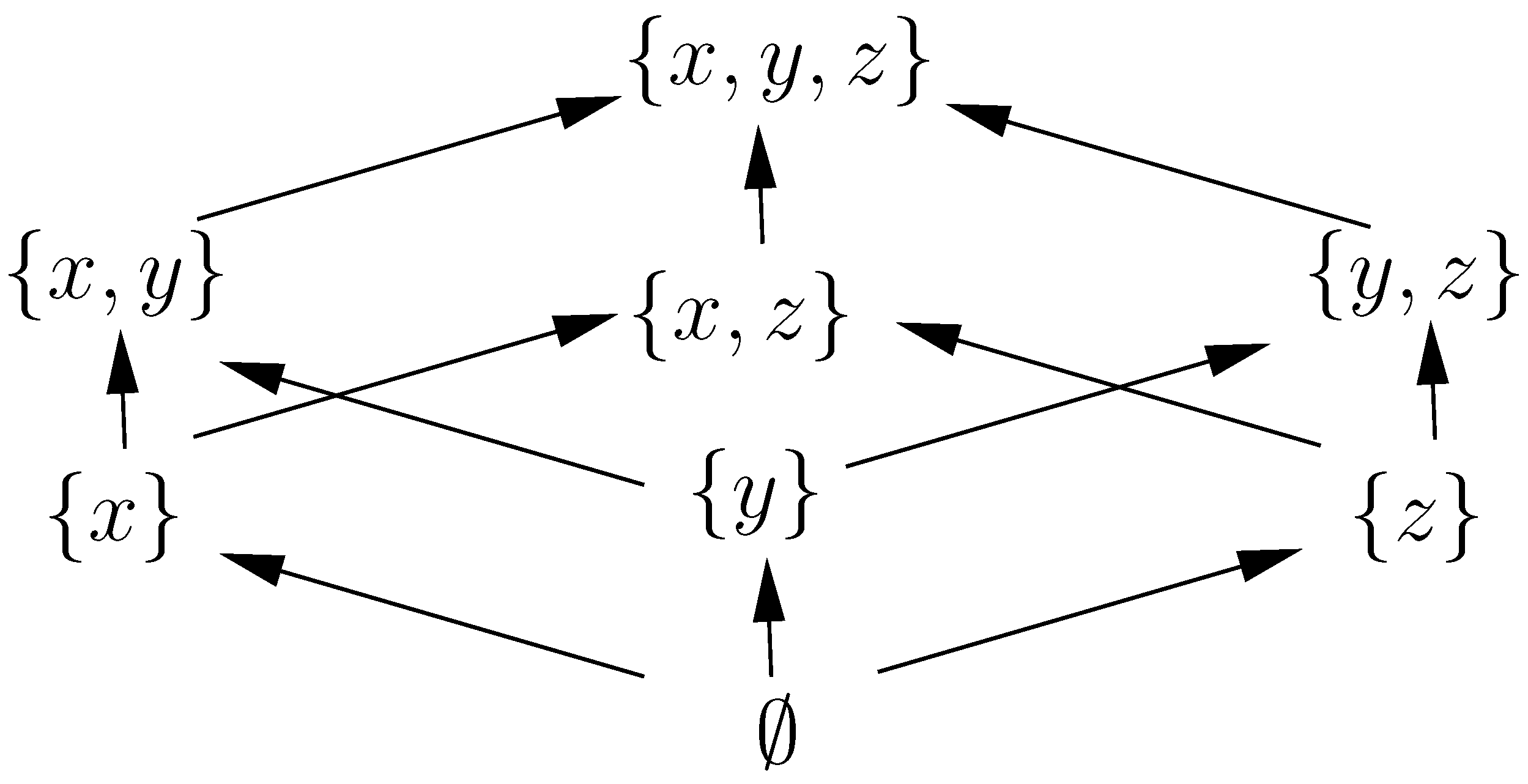
2. Logics
- Reflexivity: for all ;
- Transitivity: for all such that and then ;
- Antisymmetry: if are such that and then .
- for all .
- If is such that , then .
- for all .
- If is such that , then .
- 0 and 1 exist, ,
- for any finite subset , and exist,
- ⊥ is bijective
- (order reversing property)
- (involutivity)
- and (complementarity).
- for any sequence of pairwise orthogonal elements of L.
- ,
- if are such that then, ,
- if is a sequence of pairwise disjoint Borel sets, then .
3. The Mackey Axioms and the Ma̧czyński Theorem
- L is a logic with respect to the natural order of real functions, with complementation , .
- Each observable A determines a unique L-valued measure defined asThe family is surjective
- Each state determines a unique probability measure defined asThe family is ordering.
- For each , and
4. Quantum Mechanics
5. The Ma̧czyński Experimental Functions
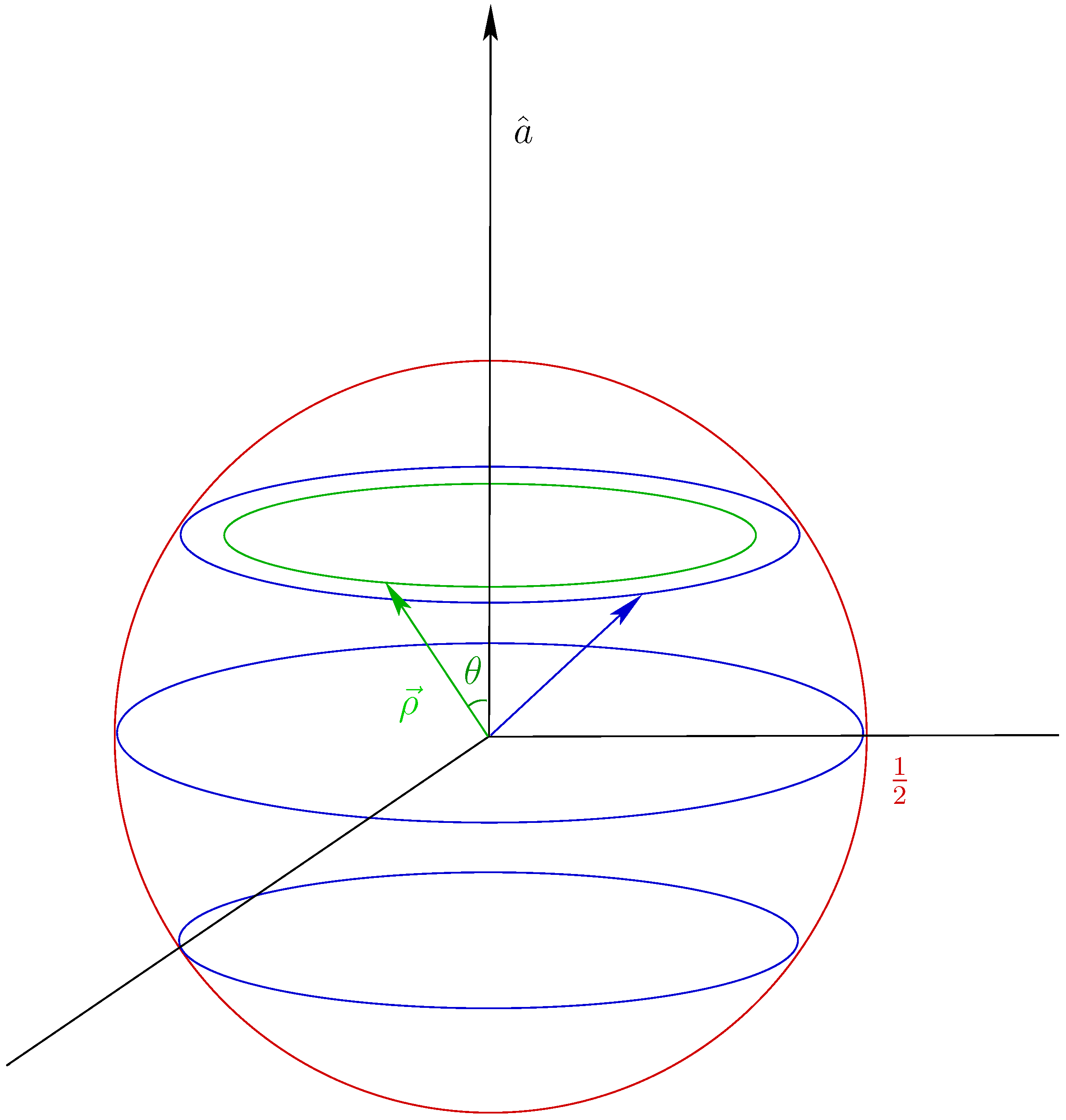
5.1. The Qubit
- Borel sets such that ;
- Borel sets such that and ;
- Borel sets such that and ;
- Borel sets such that .
- ,
- ,
- ,
- .
5.2. Two Qubits Entangled
- ;
- ;
- ;
- If is an eigenvalue of A with eigenvectors , where a runs over the multiplicity of , and is an eigenvalue of B with eigenvectors , b running on the multiplicity of , then is an eigenvalue of and are eigenvectors of .
- Sets that do not contain any eigenvalue,
- Sets that contain only one eigenvalue,
- Sets that contain only the two eigenvalues of the same subsystem,
- Sets that contain only two eigenvalues, one of each subsystem,
- Sets that contain only three eigenvalues,
- Sets containing the four eigenvalues.
- For type 1 we just have ,
- Type 2 corresponds to a projector in one subsystem times a projector over the eigenspace of () in the other. There are four posible combinations but the result is always 0,
- Type 3 corresponds to ,
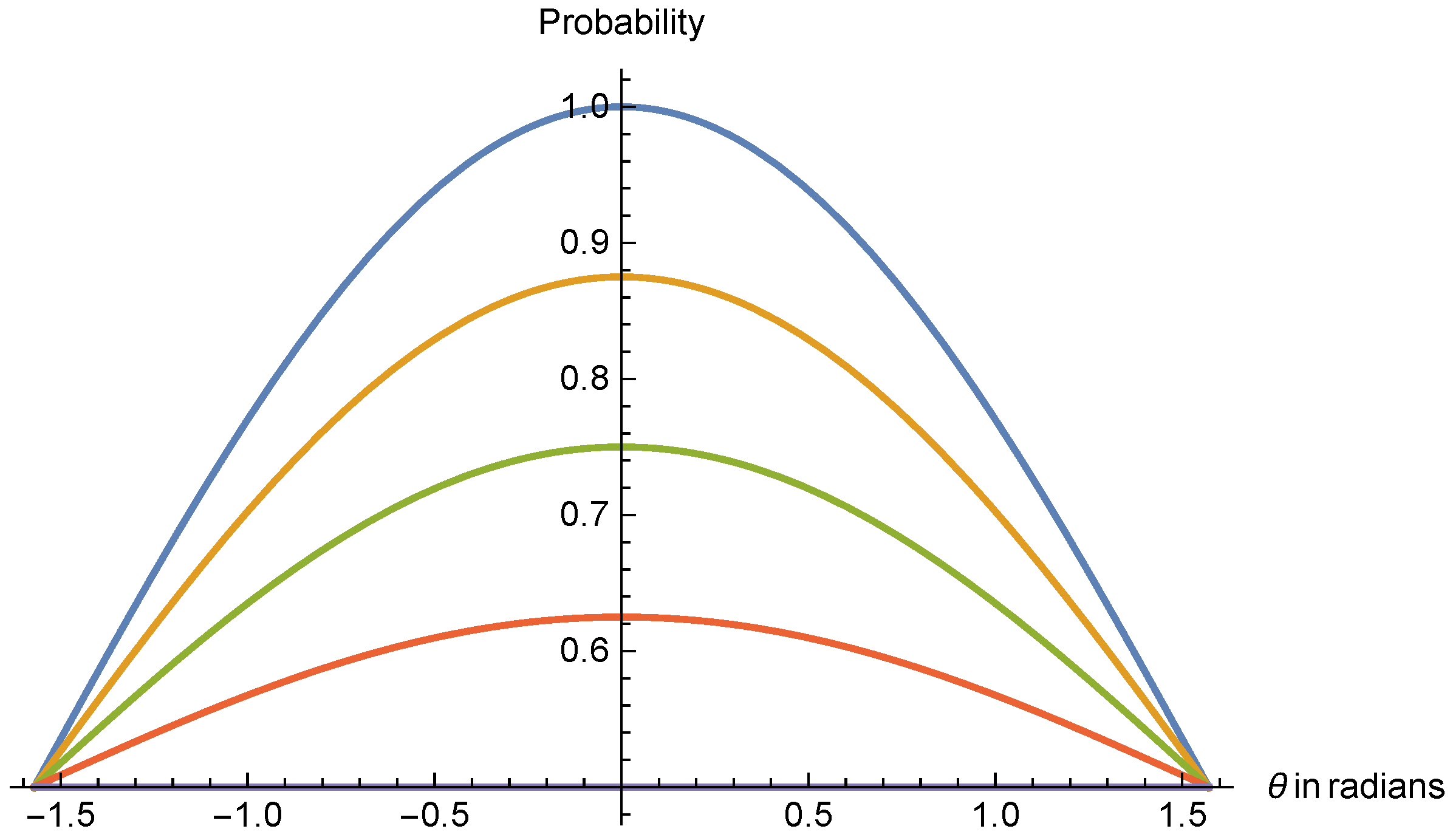
- For type 4, let us consider , the projector onto the subspace of eigenvectors in that correspond to the eigenvalues , that is, we are considering a Borel subset E that contains only and where, according to (10), we have:We denote as , the respective unit vectors for and . The experimental functions on an arbitrary density matrix (14) can be explicitly computed as:After some algebra we getThis can be read as the probability for the observables A and B getting the values and , respectively. The observable gets the value .Unlike for the qubit, it is not straightforward to check here that , but since this is a probability, according to the Hilbert space machinery, the inequalities must be satisfied. At the end of the section we will check it for pure states. These inequalities should be related to the positivity conditions for the matrix , obtained, in the formalism of the Bloch matrix, in Ref. [13].To get the rest of the experimental functions for type 3, we only have to change in the projectors for to obtain and for to obtain .
- Type 5 are projectors of the form or . The experimental function of, say, reproduces the result of (11)
- Type 6 is just the identity.
Pure States
5.3. The ‘Nested’ Qutrit
Unitary Transformations
6. The Fuzzy Set Picture
- if an only if .
- if an only if .
- The bold union is defined as
- The bold intersection is defined as
- The complementation of is
- One defines the empty set ∅ by , and its complement is itself with ,
- and are weakly disjoint if .
- ∅ belongs to the family .
- If belongs to , so does its complement .
- Given a countable family of fuzzy subsets inside which is pairwise weakly disjoint, for , then belongs to .
- If then .
6.1. The Fuzzy Bit
6.2. The Entangled Fuzzy Bits
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mackey, G.W. The Mathematical Foundations of Quantum Mechanics; W. A. Benjamin Inc.: New York, NY, USA, 1963. [Google Scholar]
- Varadarajan, V.S. Geometry of Quantum Theory, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Beltrametti, E.G.; Cassinelli, G. The Logic of Quantum Mechanics; Adison-Wesley: Boston, MA, USA, 1981. [Google Scholar]
- Łukasiewicz, J. Logical Foundations of Probability Theory; Akademien der Wissenschaften Schweiz: Kraków, Poland, 1913. [Google Scholar]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Giles, R. Łukasiewicz logic and fuzzy set theory. Int. J. -Man-Mach. Stud. 1976, 67, 313–327. [Google Scholar] [CrossRef]
- Ospanov, Y.A.; Orazbayev, B.B.; Orazbayeva, K.N.; Gancarczyk, T.; Shaikhanova, A.K. Control of Fuzzy Technological Objects Based on Mathematical Models. In Proceedings of the 16th International Conference on Control, Automation and Systems (ICCAS), Gyeongju, Republic of Korea, 16–19 October 2016; pp. 1487–1493. [Google Scholar]
- Pykacz, J. Fuzzy quantum logics and infinite-valued Łukasiewicz logic. Int. J. Theor. Phys. 1994, 33, 1403–1416. [Google Scholar] [CrossRef]
- Pykacz, J. Quantum Physics, Fuzzy Sets and Logic; Springer Briefs Series; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Ma̧czyński, M.J. The orthogonality postulate in axiomatic Quantum Mechanics. Int. J. Theor. Phys. 1973, 8, 353–360. [Google Scholar] [CrossRef]
- Mączyński, M.J. Functional properties of quantum logics. Int. J. Theor. Phys. 1974, 11, 149–156. [Google Scholar] [CrossRef]
- Byrd, M.S.; Khaneja, N. Characterization of the positivity of the density matrix in terms of the coherence vector representation. Phys. Rev. A 2003, 68, 062322. [Google Scholar] [CrossRef]
- Gamel, O. Entangled Bloch spheres: Bloch matrix and two qubit state space. Phys. Rev. A 2016, 93, 062320. [Google Scholar] [CrossRef]
- Belousov, Y.; Man’ko, V.I.; Migliore, A.; Sergi, A.; Messina, A. Symmetry-induced emergence of a pseudo-qutrit in the dipolar coupling of two qubits. Entropy 2022, 24, 223. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Vala, J.; Sastry, S.; Birgitta Whaley, K. Geometric theory of non local two-qubit operations. Phyiscal Rev. A 2003, 67, 042313. [Google Scholar] [CrossRef]
- Birkhoff, G.; Von Neumann, J. The Logic of Quantum Mechanics; The Annals of Mathematics; Springer: Cham, Switzerland, 1936; Volume 37, pp. 823–843. [Google Scholar]
- Gleason, A.M. Measures on the closed subspaces of a Hilbert space. J. Math. Mech. 1957, 6, 885–893. [Google Scholar] [CrossRef]
- Graphic/Calculation Made with the Help of MATHEMATICA; Version 13.3; Wolfram Research, Inc.: Champaign, IL, USA, 2023.
- Helgason, S. Differential Geometry, Lie Groups, and Symmetric Spaces; Academic Press: San Diego, CA, USA, 2001. [Google Scholar]
- Zawirski, Z. Relationship between many valued logic and the calculus of probabilities. Pr. Kom. Filoz. Pozn. Tow. Przyj. Nauk. 1934, 4, 155–240. (In Polish) [Google Scholar]
- Chiara, M.L.D.; Giuntini, R.; Greechie, R. Reasoning in Quantum Theory. Sharp and Unsharp Logics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Kreinovich, V.; Kohout, L.J.; Kim, E. Square root of ‘not’: A major difference between fuzzy and quantum logics. Int. J. Gen. Syst. 2008, 40, 1–5. [Google Scholar]
- Meyer, J.J. Fisher information in noisy intermediate-scale quantum applications. Quantum 2021, 5, 539. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aldana, M.; Lledó, M.A. The Fuzzy Bit. Symmetry 2023, 15, 2103. https://doi.org/10.3390/sym15122103
Aldana M, Lledó MA. The Fuzzy Bit. Symmetry. 2023; 15(12):2103. https://doi.org/10.3390/sym15122103
Chicago/Turabian StyleAldana, Milagrosa, and María Antonia Lledó. 2023. "The Fuzzy Bit" Symmetry 15, no. 12: 2103. https://doi.org/10.3390/sym15122103
APA StyleAldana, M., & Lledó, M. A. (2023). The Fuzzy Bit. Symmetry, 15(12), 2103. https://doi.org/10.3390/sym15122103





