The Invariants of Dual Parallel Equidistant Ruled Surfaces
Abstract
1. Introduction
2. Preliminaries
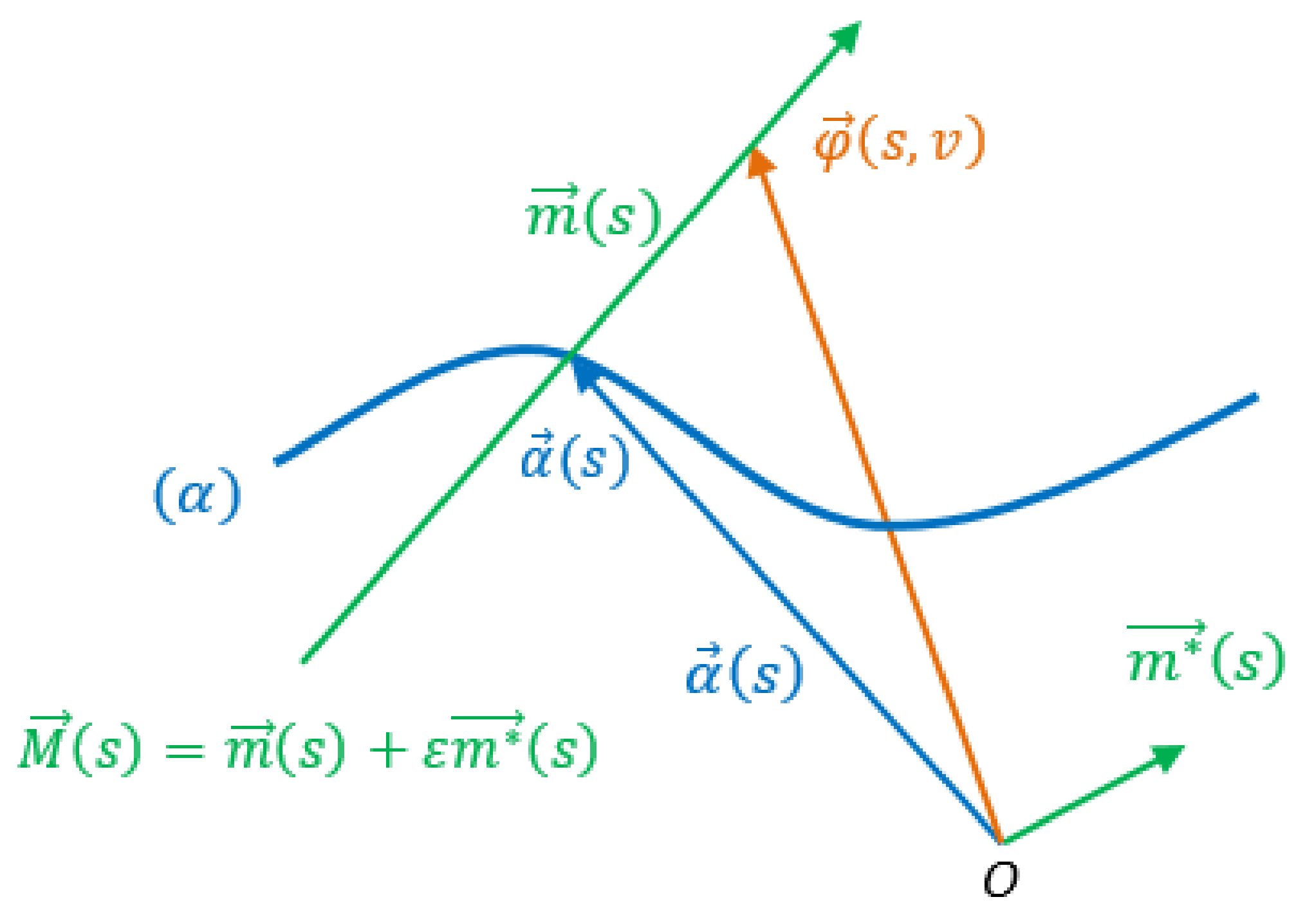
3. The Integral Invariants of DPERS
3.1. The Relationships between the Integral Invariants of the Closed Ruled Surfaces Formed by the Dual Tangent Vectors of DPERS
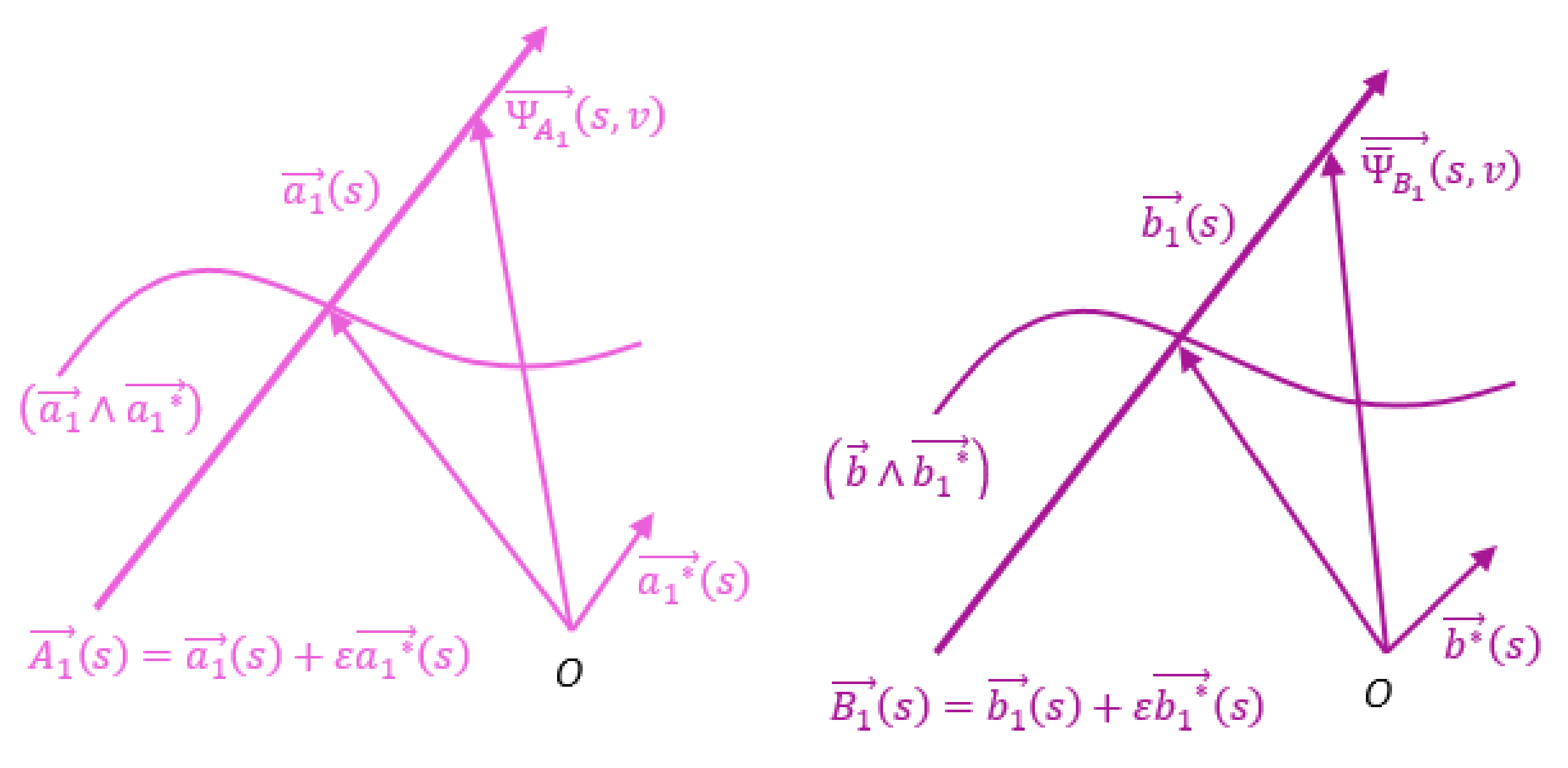
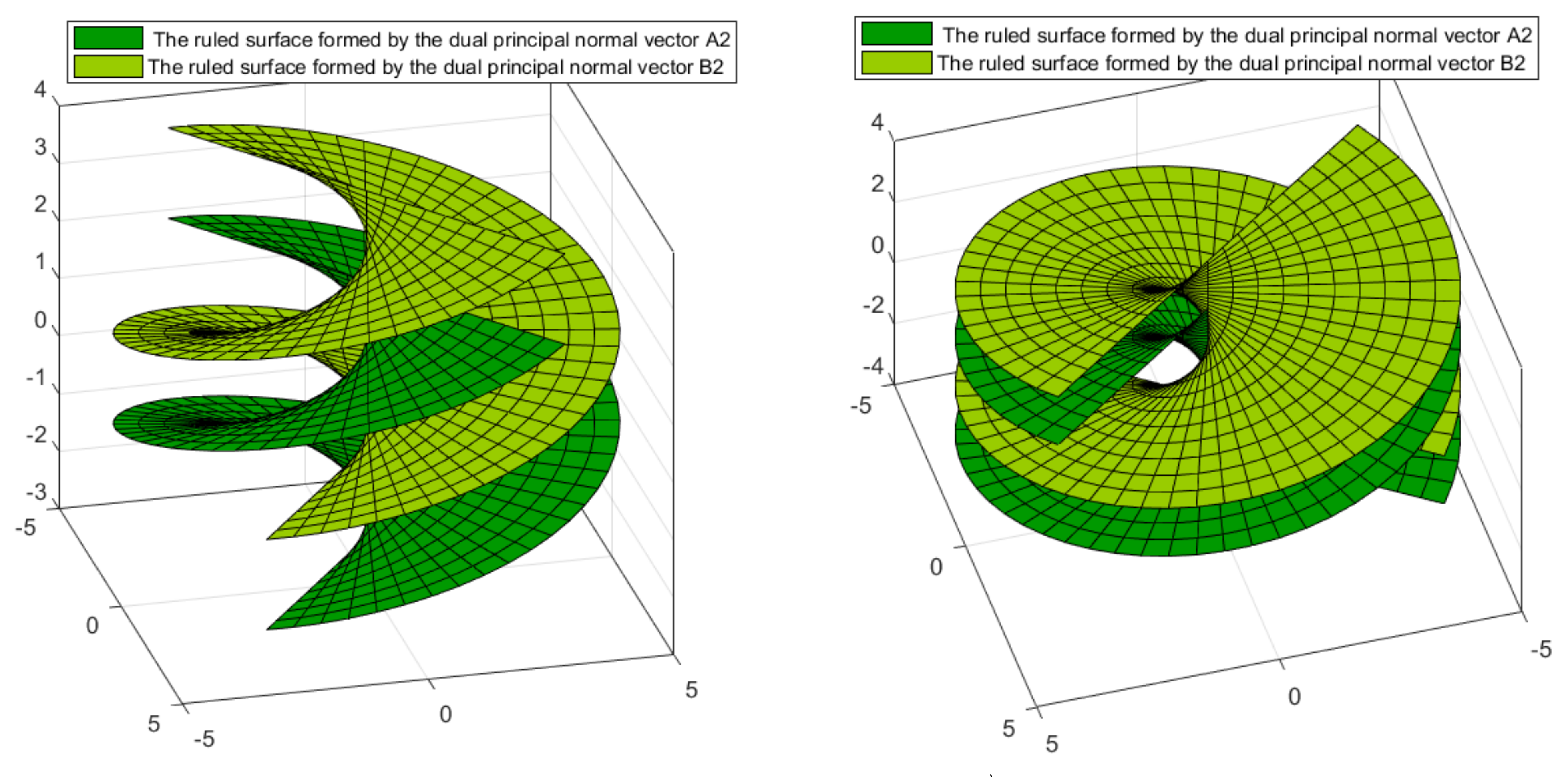
3.2. The Relationships between the Integral Invariants of the Closed Ruled Surfaces Formed by the Dual Principal Normal Vectors of DPERS
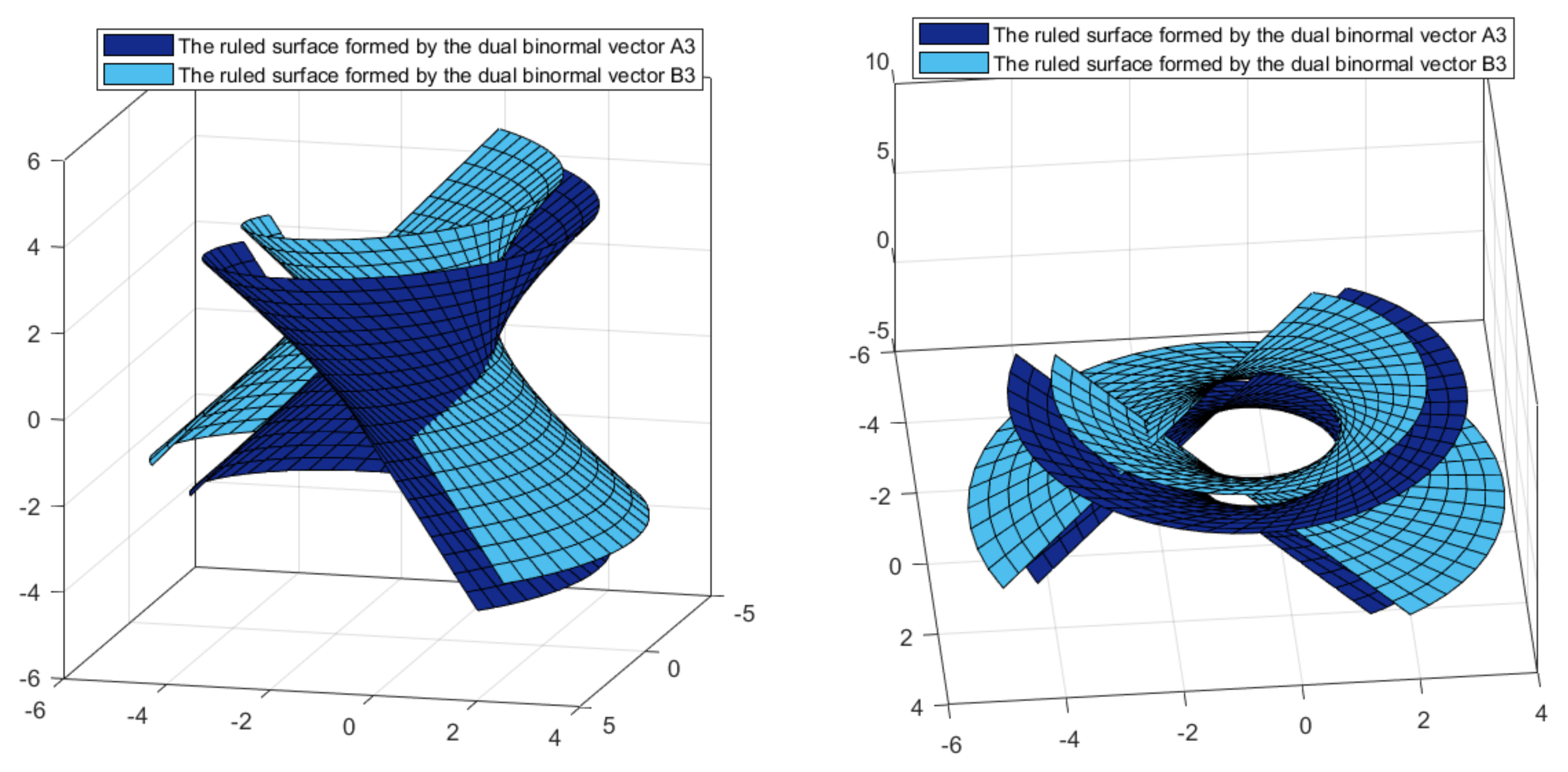
3.3. The Relationships between the Integral Invariants of the Closed Ruled Surfaces Formed by the Dual Binormal Vectors of DPERS
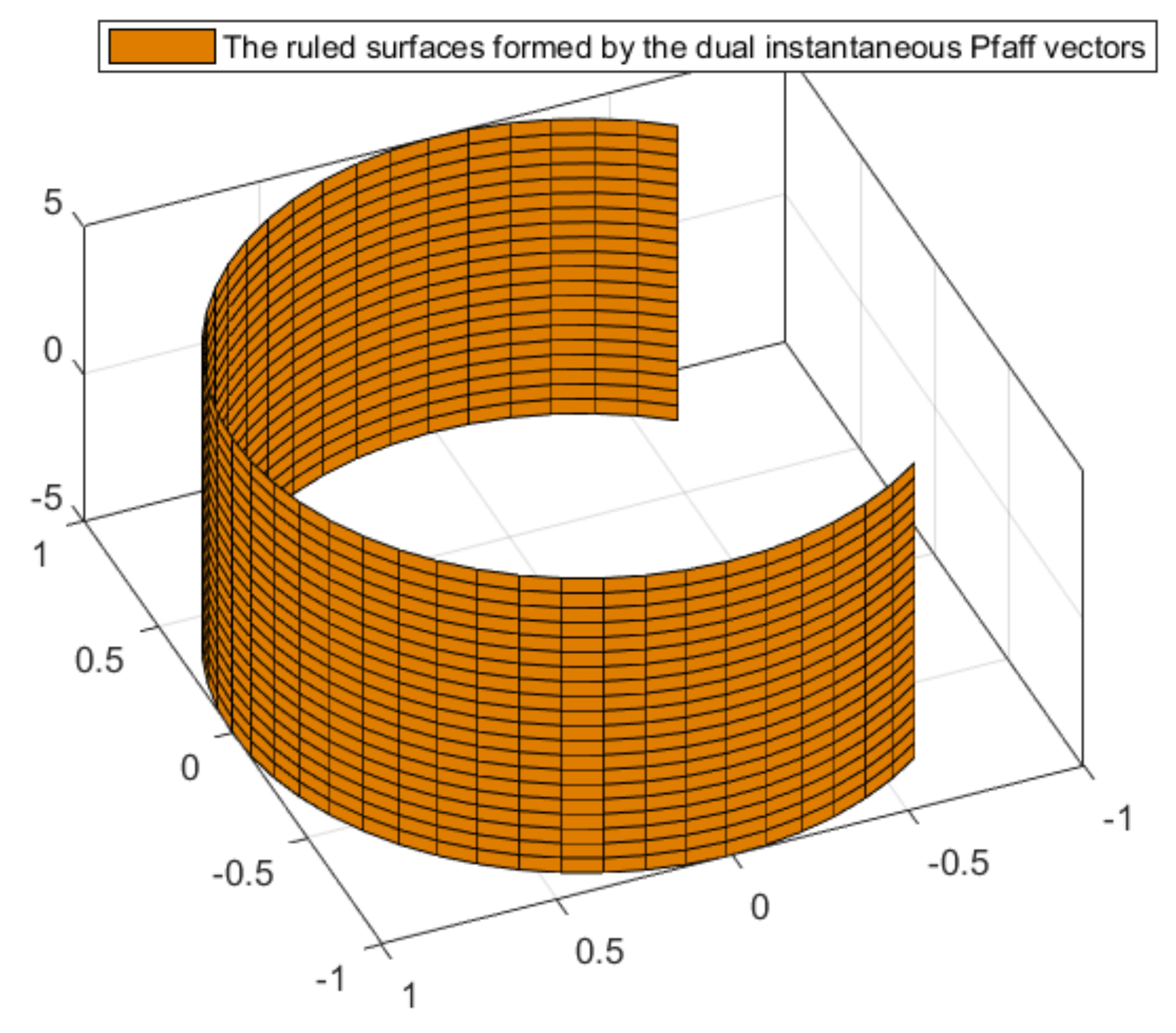
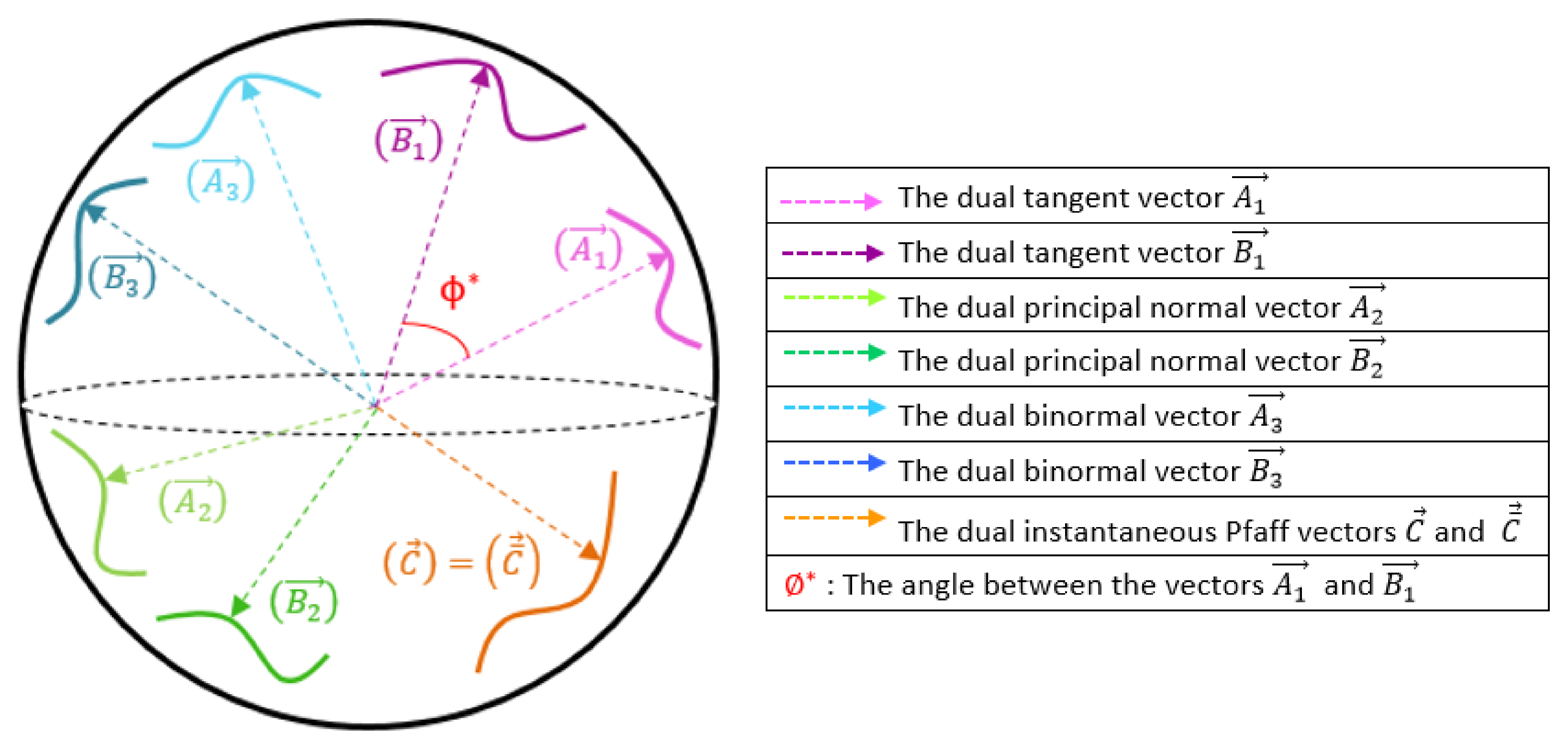
3.4. The Relationships between the Integral Invariants of the Closed Ruled Surfaces Formed by the Dual Instantaneous Pfaff Vectors of DPERS
4. The Gaussian Curvatures of DPERS
4.1. The Relationship between the Gaussian Curvatures of the Ruled Surfaces Formed by the Dual Tangent Vectors of DPERS
4.2. The Relationship between the Gaussian Curvatures of the Ruled Surfaces Formed by the Dual Principal Normal Vectors of DPERS
4.3. The Relationship between the Gaussian Curvatures of the Ruled Surfaces Formed by the Dual Binormal Vectors of DPERS
4.4. The Relationship between the Gaussian Curvatures of the Ruled Surfaces Formed by the Dual Instantaneous Pfaff Vectors of DPERS
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hacisalioglu, H.H. Differential Geometry-II; Ankara University, Faculty of Science Publications: Ankara, Turkey, 1994; pp. 203–286. [Google Scholar]
- Ozdemir, M. Quaternions and Geometry; Altin Nokta Press: İzmir, Turkey, 2020; pp. 150–182. [Google Scholar]
- Sabuncuoglu, A. Differential Geometry; Nobel Press: Ankara, Turkey, 2006. [Google Scholar]
- Fenchel, W. On the Differential Geometry of Closed Space Curves. Bull. Am. Math. Soc. 1951, 57, 44–54. [Google Scholar] [CrossRef]
- Senatalar, M. Differential Geometry (Curves and Surfaces Theory); Istanbul State Engineering and Architecture Academy Publications: Istanbul, Turkey, 1978; pp. 153–170. [Google Scholar]
- Hagemann, M.; Klawitter, D.; Lordick, D. Force Driven Ruled Surfaces. J. Geom. Graph. 2013, 17, 193–204. [Google Scholar]
- Ali, A.T.; Abdel Aziz, H.S.; Sorour, A.H. Ruled surfaces generated by some special curves in Euclidean 3-Space. J. Egypt. Math. Soc. 2013, 21, 285–294. [Google Scholar] [CrossRef]
- Schaaf, A.; Ravani, B. Geometric Continuity of Ruled Surfaces. Comput. Aided Geom. Des. 1998, 15, 289–310. [Google Scholar] [CrossRef]
- Hacisalihoglu, H.H. On the pitch of a closed ruled surfaces. Mech. Mach. Theory 1972, 7, 291–305. [Google Scholar] [CrossRef]
- Senyurt, S.; Gur Mazlum, S.; Grilli, G. Gaussian curvatures of parallel ruled surfaces. Appl. Math. Sci. 2020, 14, 171–183. [Google Scholar] [CrossRef]
- Valeontis, I. Parallel P-Äquidistante Regelflachen Manuscripta. Mathematics 1986, 54, 391–404. [Google Scholar]
- Bektaş, M. On characterizations of general helices for ruled surfaces in the pseudo-Galilean space -(Part-I). J. Math. Kyoto Univ. 2004, 44, 523–528. [Google Scholar]
- Masal, M.; Kuruoglu, N. Some Characteristic Properties of the Parallel P-Equidistant Ruled Surfaces in The Euclidean Space. Pure Appl. Math. Sci. 1999, 50, 35–42. [Google Scholar]
- Masal, M.; Kuruoglu, N. Some Characteristic Properties of the Shape Operators of Parallel p-Equidistant Ruled Surfaces. Bull. Pure Appl. Sci. 2000, 19, 361–364. [Google Scholar]
- Masal, M.; Kuruoglu, N. Spacelike parallel pi-equidistant ruled surfaces in the Minkowski 3-space R13. Algebr. Groups Geom. 2005, 22, 13–24. [Google Scholar]
- Li, Y.; Şenyurt, S.; Özduran, A.; Canlı, D. The Characterizations of Parallel q-Equidistant Ruled Surfaces. Symmetry 2022, 14, 1879. [Google Scholar] [CrossRef]
- As, E.; Senyurt, S. Some Characteristic Properties of Parallel z-Equidistant Ruled Surfaces. Hindawi Publ. Corp. Math. Probl. Eng. 2013, 2013, 587289. [Google Scholar]
- Sarioglugil, A.; Senyurt, S.; Kuruoglu, N. On the Integral Invariants of the Closed Ruled Surfaces Generated by a Parallel p-Equidistant Dual Centroit Curve in the Line Space. Hadron. J. 2011, 34, 34–47. [Google Scholar]
- Senyurt, S. Integral Invariants of Parallel P-Equidistant Ruled Surfaces Which Are Generated by Instantaneous Pfaff Vector. Ordu Univ. Sci. Technol. J. 2012, 2, 13–22. [Google Scholar]
- Clifford, W.K. Preliminary sketch of biquaternions. Proc. Lond. Math. Soc. 1873, 1, 381–395. [Google Scholar] [CrossRef]
- Study, E. Geometrie der Dynamen; Verlag Teubner: Leipzig, Germany, 1903. [Google Scholar]
- Blaschke, W. Differential Geometry Courses; Istanbul University Publications: Istanbul, Turkey, 1949; pp. 332–345. [Google Scholar]
- Hacisalihoglu, H.H. The Motion Geometry and Quaternions Theory; Gazi University, Faculty of Science and Literature Publications: Ankara, Turkey, 1983; pp. 3–55. [Google Scholar]
- Hacisalihoglu, H.H. Acceleration Axes in Spatian Kinematics I. Commun. Fac. Sci. Univ. Ank. Ser. Math. Stat. 1971, 20, 1–15. [Google Scholar]
- Muller, H.R. Kinematics Courses; Ankara University Press: Ankara, Turkey, 1963; pp. 240–251. [Google Scholar]
- Bilici, M. On the Invariants of Ruled Surfaces Generated by the Dual Involute Frenet Trihedron. Commun. Fac. Sci. Univ. Ank. Ser. A1 Math. Stat. 2017, 66, 62–70. [Google Scholar]
- Oral, S.; Kazaz, M. Characterizations for Slant Ruled Surfaces in Dual Space. Iran. J. Sci. Technol. Trans. A Sci. 2017, 41, 191–197. [Google Scholar] [CrossRef]
- Güven, İ.A.; Kaya, S.; Hacisalihoğlu, H.H. On closed ruled surfaces concerned with dual Frenet and Bishop frames. J. Dyn. Syst. Geom. Theor. 2011, 9, 67–74. [Google Scholar] [CrossRef]
- Saracoglu, S.; Yayli, Y. Ruled Surfaces and Dual Spherical Curves. Acta Univ. Apulensis 2012, 20, 337–354. [Google Scholar]
- Gursoy, O. The dual angle of the closed ruled surfaces. Mech. Mach. Theory 1990, 25, 131–140. [Google Scholar] [CrossRef]
- Sahiner, B.; Kazaz, M.; Ugurlu, H.H. A Dual Method to Study Motion of a Robot End-Effector. J. Inform. Math. Sci. 2018, 10, 247–259. [Google Scholar] [CrossRef]
- Culmer, P.R.; Jackson, A.E.; Makower, S.; Richardson, R.; Cozens, J.A.; Levesley, M.C.; Bhakta, B.B. A control strategy for upper limb robotic rehabilitation with a dual robot system. IEEE/ASME Trans. Mechatron. 2009, 15, 575–585. [Google Scholar] [CrossRef]
- Gu, Y.L.; Luh, J. Dual-number transformation and its applications to robotics. IEEE J. Robot. Autom. 1987, 3, 615–623. [Google Scholar]
- Bayro-Corrochano, E.; Kähler, D. Motor algebra approach for computing the kinematics of robot manipulators. J. Robot. Syst. 2000, 17, 495–516. [Google Scholar] [CrossRef]
- Özgür, E.; Mezouar, Y. Kinematic modeling and control of a robot arm using unit dual quaternions. Robot. Auton. Syst. 2016, 77, 66–73. [Google Scholar] [CrossRef]
- Yayli, Y.; Çalişkan, A.; Uğurlu, H.H. The E. Study maps of circles on dual hyperbolic and Lorentzian unit spheres and . Math. Proc. R. Ir. Acad. 2002, 102A, 37–47. [Google Scholar] [CrossRef]
- Şenyurt, S.; Gür, S. Timelike–spacelike involute–evolute curve couple on dual Lorentzian space. J. Math. Comput. Sci. 2012, 2, 1808–1823. [Google Scholar]
- Bektas, Ö.; Senyurt, S. On Some Characterizations of Ruled Surface of a Closed Timelike Curve in Dual Lorentzian Space. Adv. Appl. Clifford Algebr. 2012, 22, 939–953. [Google Scholar] [CrossRef]
- Li, Y.L.; Alluhaibi, N.; Abdel-Baky, R.A. One-parameter Lorentzian dual spherical movements and invariants of the axodes. Symmetry 2022, 14, 1930. [Google Scholar] [CrossRef]
- Gür, S.; Şenyurt, S. Spacelike–timelike involute–evolute curve couple on dual Lorentzian space. J. Math. Comput. Sci. 2013, 3, 1054–1075. [Google Scholar]
- Gür Mazlum, S.; Şenyurt, S.; Grilli, L. The Dual Expression of Parallel Equidistant Ruled Surfaces in Euclidean 3-Space. Symmetry 2022, 14, 1062. [Google Scholar] [CrossRef]
- Li, Y.; Dey, S.; Pahan, S.; Ali, A. Geometry of conformal η-Ricci solitons and conformal η-Ricci almost solitons on Paracontact geometry. Open Math. 2022, 20, 1–20. [Google Scholar] [CrossRef]
- Li, Y.; Ali, A.; Mofarreh, F.; Alluhaibi, N. Homology groups in warped product submanifolds in hyperbolic spaces. J. Math. 2021, 2021, 8554738. [Google Scholar] [CrossRef]
- Yüzbaşı, Z.K.; Bektaş, M.; Yılmaz, M.Y. Compact Totally Real Minimal Submanifolds in a Bochner-Kaehler Manifold. Univers J. Math. Appl. 2018, 1, 254–257. [Google Scholar]
- Yılmaz, M.Y.; Bektaş, M. Curvature Inequalities between a Hessian Manifold with Constant Curvature and its Submanifolds. Math. Sci. Appl. -Notes 2017, 5, 27–33. [Google Scholar] [CrossRef]
- Li, Y.; Gür Mazlum, S.; Şenyurt, S. The Darboux Trihedrons of Timelike Surfaces in the Lorentzian 3-Space. Int. J. Geom. Methods Mod. Phys. 2023, 2350030, 1–35. [Google Scholar] [CrossRef]
- Chen, Z.; Li, Y.; Sarkar, S.; Dey, S.; Bhattacharyya, A. Ricci Soliton and Certain Related Metrics on a Three-Dimensional Trans-Sasakian Manifold. Universe 2022, 8, 595. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gür Mazlum, S.; Şenyurt, S.; Grilli, L. The Invariants of Dual Parallel Equidistant Ruled Surfaces. Symmetry 2023, 15, 206. https://doi.org/10.3390/sym15010206
Gür Mazlum S, Şenyurt S, Grilli L. The Invariants of Dual Parallel Equidistant Ruled Surfaces. Symmetry. 2023; 15(1):206. https://doi.org/10.3390/sym15010206
Chicago/Turabian StyleGür Mazlum, Sümeyye, Süleyman Şenyurt, and Luca Grilli. 2023. "The Invariants of Dual Parallel Equidistant Ruled Surfaces" Symmetry 15, no. 1: 206. https://doi.org/10.3390/sym15010206
APA StyleGür Mazlum, S., Şenyurt, S., & Grilli, L. (2023). The Invariants of Dual Parallel Equidistant Ruled Surfaces. Symmetry, 15(1), 206. https://doi.org/10.3390/sym15010206






