Two New Rb3Sc(IO3)6 Polytypes in Proposed Nonlinear Optical Family A3M(IO3)6 (A = K, Rb; M = Sc, In): Topology–Symmetry Analysis, Order–Disorder and Structure–Properties Relation
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
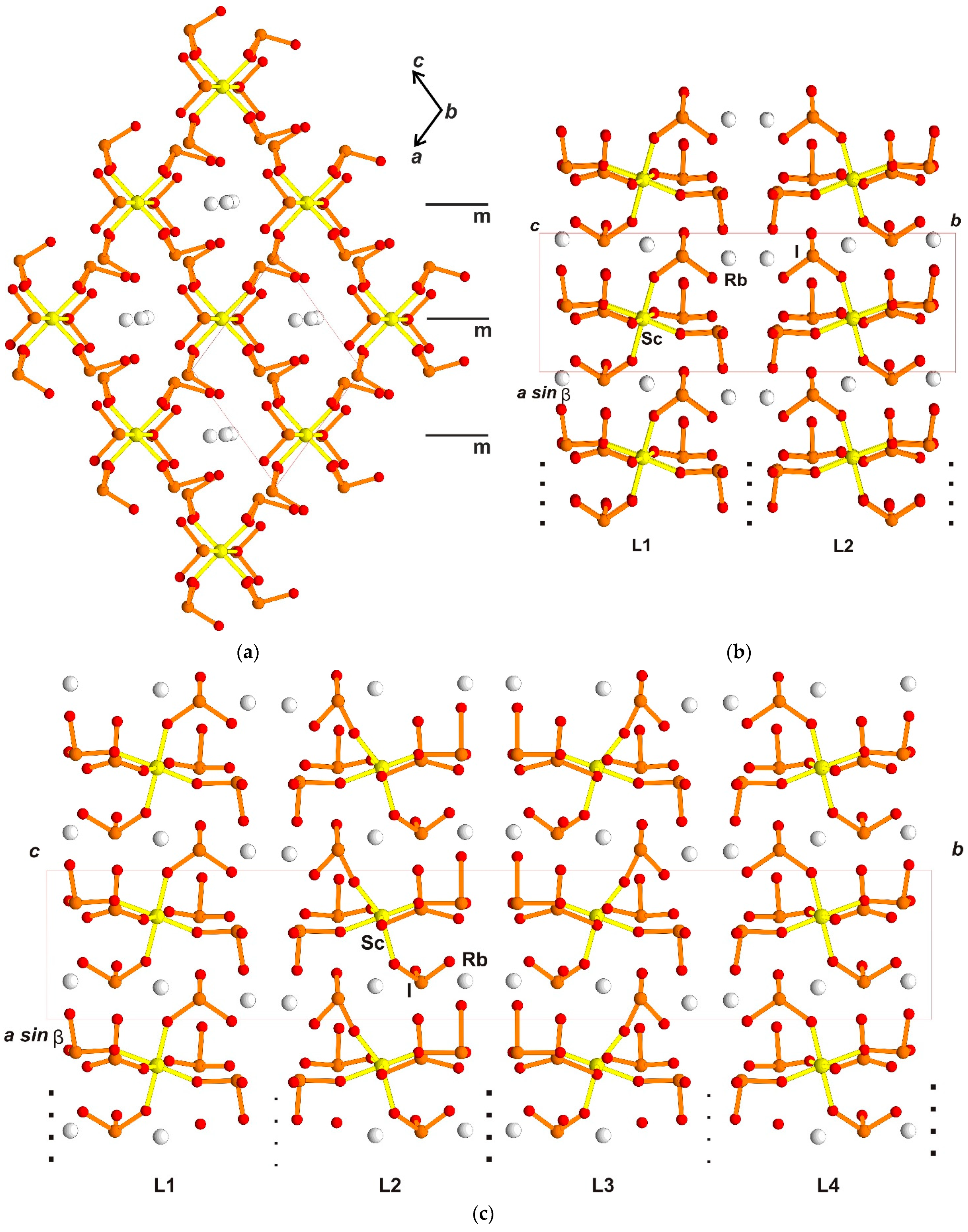
3.1. Rb3Sc(IO3)6 Iodate Structures
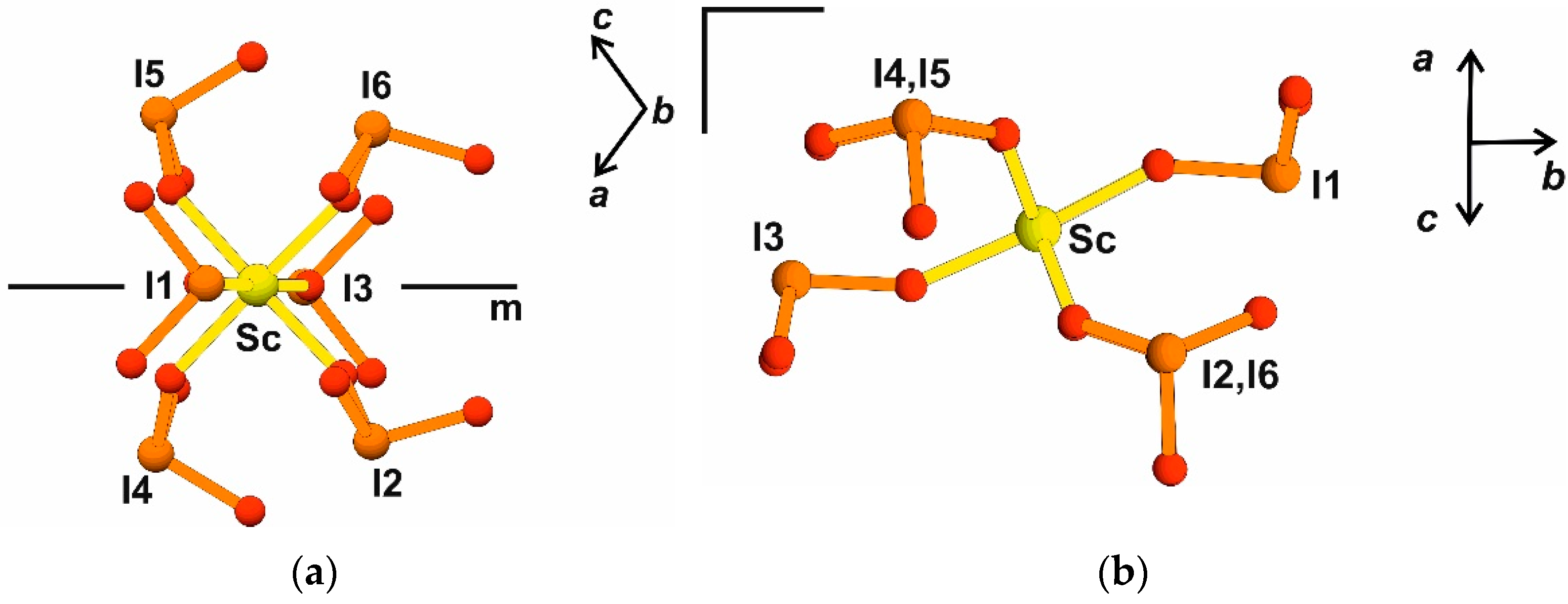
3.1.1. Crystal Structure Rb3Sc(IO3)6, Experiment 1, b = 20 Å
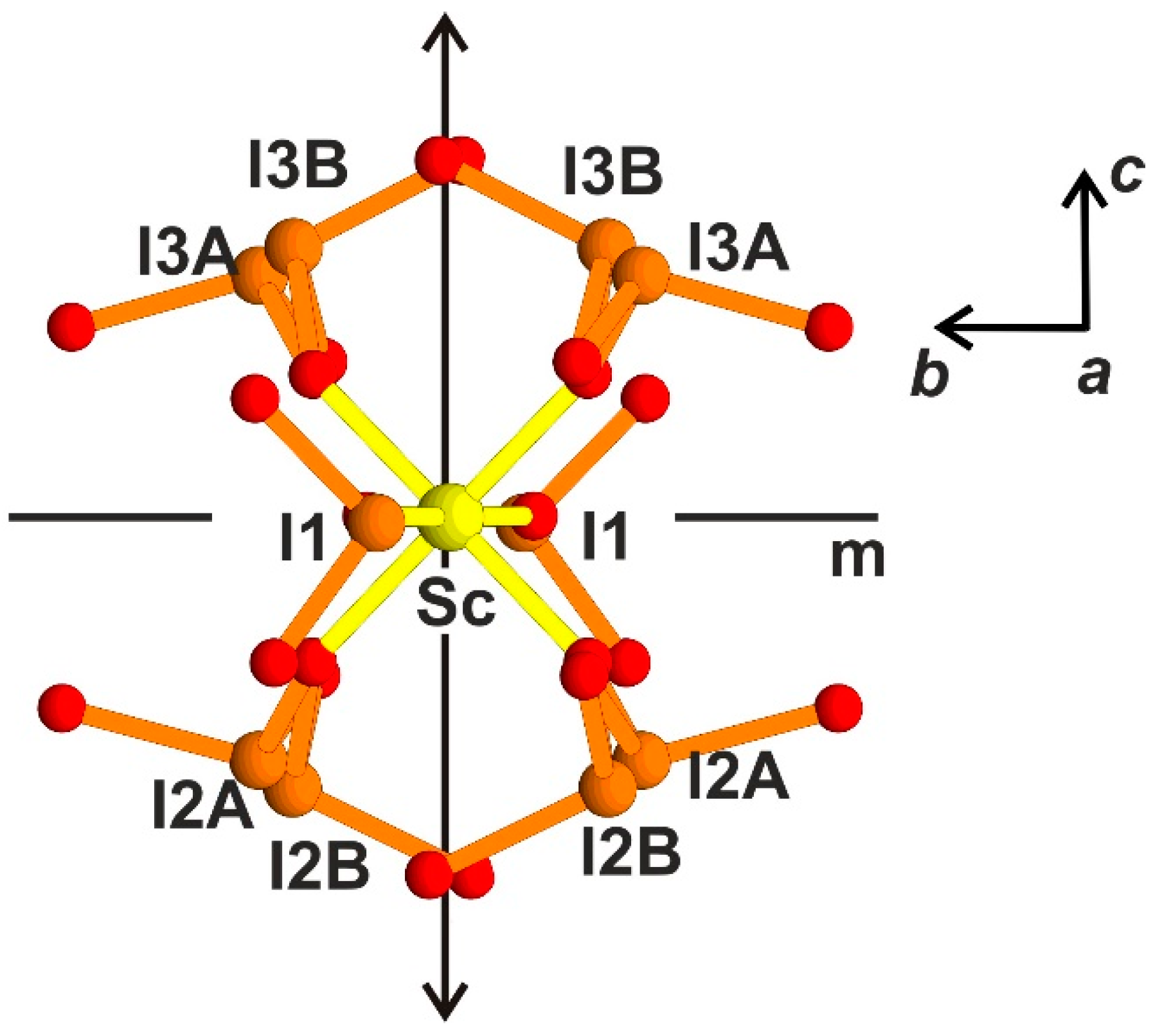
3.1.2. Crystal Structure Rb3Sc(IO3), Experiment 1, b = 40 Å
3.1.3. Crystal Structure Rb3Sc(IO3), Experiment 2, b = 40 Å
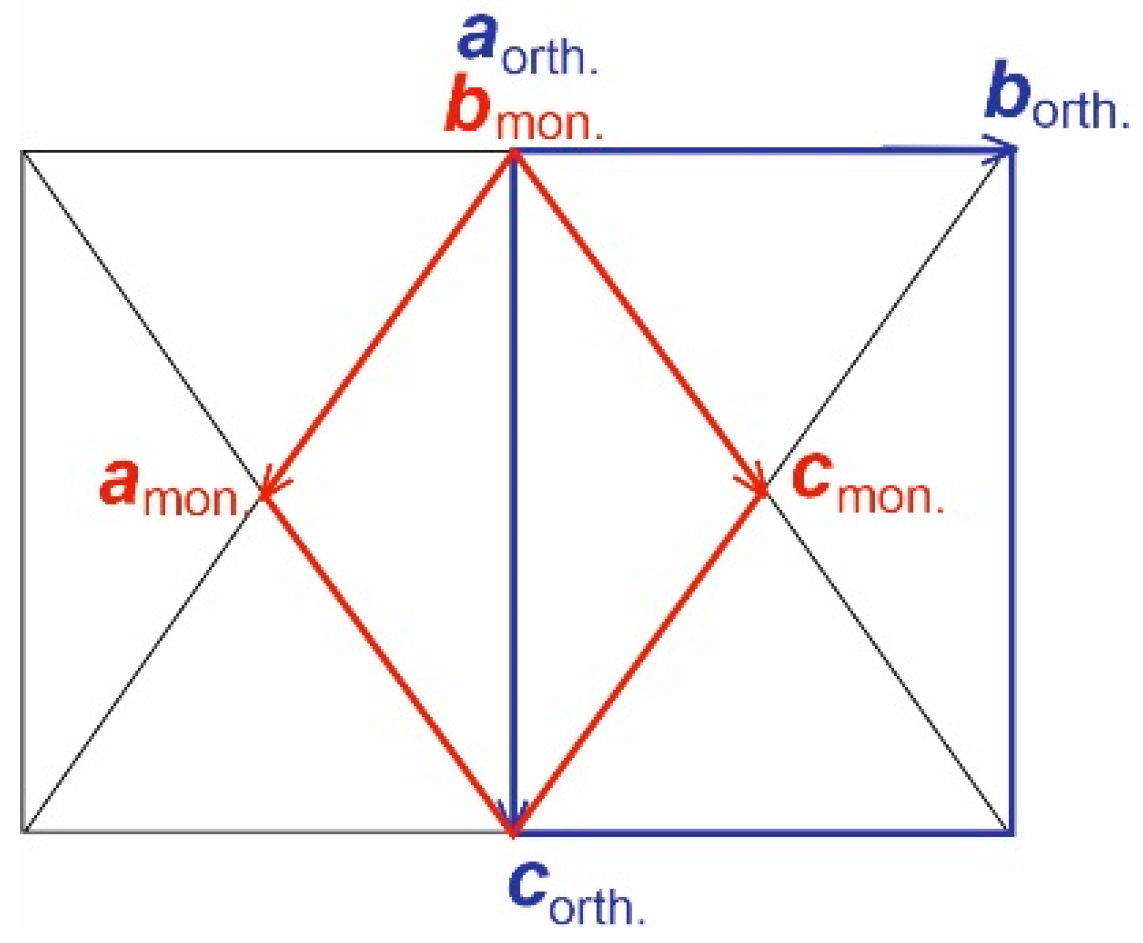
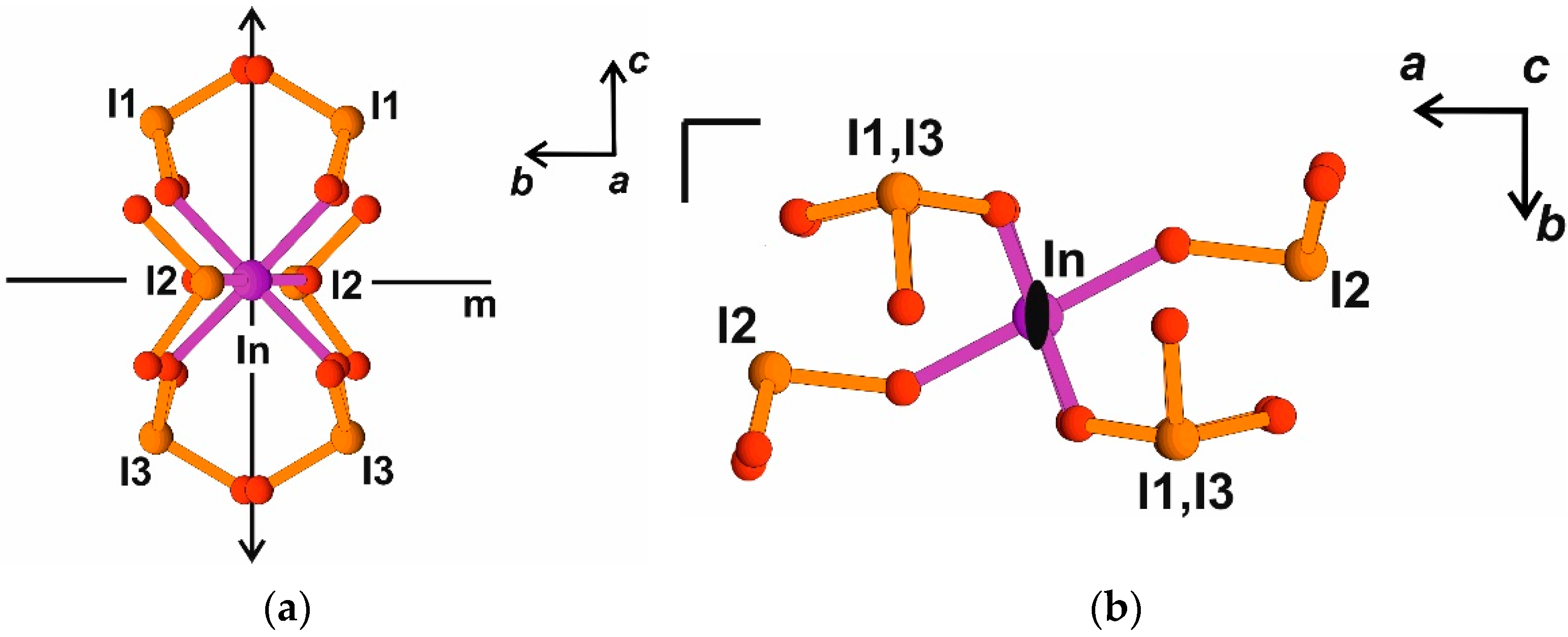
3.2. Comparison of New Monoclinic Rb3Sc(IO3)6 Polytypes with Each Other and with the Orthorhombic K3In(IO3)6 and K3Sc(IO3)6 Using Topology–Symmetry Analysis; Structure–Properties Relation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nguyen, S.D.; Yeon, J.; Kim, S.H.; Halasyamani, P.S. BiO(IO3): A New Polar Iodate that Exhibits an Aurivillius-Type (Bi2O2)2+ Layer and a Large SHG Response. J. Am. Chem. Soc. 2011, 133, 12422–12425. [Google Scholar] [CrossRef]
- Mao, J.-G.; Sun, C.-F.; Yang, B.-P. Structures and properties of functional metal iodates. Sci. China Chem. 2011, 54, 911–922. [Google Scholar] [CrossRef]
- Hu, C.-L.; Mao, J.-G. Recent advances on second-order NLO/materials based on metal iodates. Coord. Chem. Rev. 2015, 288, 1–17. [Google Scholar] [CrossRef]
- Mao, F.-F.; Hu, C.-L.; Chen, J.; Wu, B.-L.; Mao, J.-G. HBa2.5(IO3)6(I2O5) and HBa(IO3)(I4O11): Explorations of second-order nonlinear optical materials in the alkali-earth polyiodate system. Inorg. Chem. 2019, 58, 3982–3989. [Google Scholar] [CrossRef]
- Dornberger-Schiff, K. Grundzuege einer Theory der OD-strukturen aus Schichten. Abh. Deutsch. Akad. Wiss. Berlin 1964, 3, 89. [Google Scholar]
- Reutova, O.; Belokoneva, E.; Volkov, A.; Dimitrova, O. Structure-properties relation in two iodate families studied by topology-symmetry analysis of OD theory. Symmetry 2021, 13, 1477. [Google Scholar] [CrossRef]
- WinXPow; Stoe&CIE GmbH: Darmstadt, Germany, 2002.
- Kurtz, S.K.; Perry, T.T. A Powder Technique for the Evaluation of Nonlinear Optical Materials. J. Appl. Phys. 1968, 39, 3798–3813. [Google Scholar] [CrossRef]
- Beskorovaynaya, D.A.; Deyneko, D.V.; Baryshnikova, O.V.; Stefanovich, S.Y.; Lazoryak, B.I. Optical Non-Linearity Tuning in Ca8-xPbxMBi(VO4)7 Whitlockite-Type Systems. J. Alloys Compd. 2016, 674, 323–330. [Google Scholar] [CrossRef]
- Liu, X.; Li, G.; Hu, Y.; Yang, M.; Kong, X.; Shi, Z.; Feng, S. Hydrothermal synthesis and crystal structure of polar and nonpolar compounds in indium iodate family. Cryst. Growth Des. 2008, 8, 2453–2457. [Google Scholar] [CrossRef]
- Vagourdi, E.M.; Zhang, W.; Denisova, K.; Lemmens, P.; Halasyamani, P.S.; Johnsson, M. Synthesis and characterization of two new second harmonic generation active iodates: K3Sc(IO3)6 and KSc(IO3)3Cl. ACS Omega 2020, 5, 5235–5240. [Google Scholar] [CrossRef] [PubMed]
- Agilent Technologies. CrysAlisPro Software System, version 1.171.3735; Agilent Technologies UK Ltd.: Oxford, UK, 2014. [Google Scholar]
- Sheldrick, G.M. SHELXL-97, a Program for Crystal Structure Refinement; SHELXS-97, a Program for Automatic Solution of Crystal Structures; University of Goettingen: Goettingen, Germany, 1997. [Google Scholar]
- CrysAlisPro, version 1.171.3946; Rigaku Oxford Diffraction: Oxford, UK, 2018.
- Dowty, E. Atoms 3.2—A Computer Program for Displaying Atomic Structures; Shape Software: Kingpost, TN, USA, 1995; p. 37663. [Google Scholar]
- Chang, H.Y.; Kim, S.H.; Ok, K.M.; Halasyamani, P.S. Polar or nonpolar? A+ cation polarity control in A2Ti(IO3)6 (A = Li, Na, K, Rb, Cs, Tl). J. Am. Chem. Soc. 2009, 131, 6865–6873. [Google Scholar] [CrossRef] [PubMed]




| Formula | Rb3Sc(IO3)6 | Rb3Sc(IO3)6 |
|---|---|---|
| Formula weight | 1350.77 | 1350.77 |
| Crystal system | monoclinic | monoclinic |
| Space group, Z | Pc, 2 | Pc, 4 |
| a, Å | 7.1147(1) | 7.1166(2) |
| b, Å, β, ° | 20.1463 (3), 107.620(2) | 40.3039 (8), 107.598(3) |
| c, Å | 7.0991(1) | 7.1009(2) |
| V, Å3 | 969.81(3) | 1941.43(8) |
| Dx, Γ/cM3 | 4.626 | 4.621 |
| μ, MM −1 | 17.493 | 17.477 |
| Wavelength, Å | 0.71073 | 0.71073 |
| Θ range/degree | 2.973–30.615 | 3.00–30.77 |
| Refl. collected/unique/R int. | 15,521/5453/0.0528 | 31,125/10,905/0.0647 |
| Completeness to Θ, % | 0.94 | 0.94 |
| Parameters | 254 | 256 |
| GOF (S) | 1.059 | 1.261 |
| Rall, Rgt, Rwgt | 0.0367, 0.0358, 0.0826 | 0.1158, 0.0966, 0.2203 |
| Δρmin/Δρmax, э/Å3 | −3.117/2.684 | −9.283/7.767 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reutova, O.; Belokoneva, E.; Volkov, A.; Dimitrova, O.; Stefanovich, S. Two New Rb3Sc(IO3)6 Polytypes in Proposed Nonlinear Optical Family A3M(IO3)6 (A = K, Rb; M = Sc, In): Topology–Symmetry Analysis, Order–Disorder and Structure–Properties Relation. Symmetry 2022, 14, 1699. https://doi.org/10.3390/sym14081699
Reutova O, Belokoneva E, Volkov A, Dimitrova O, Stefanovich S. Two New Rb3Sc(IO3)6 Polytypes in Proposed Nonlinear Optical Family A3M(IO3)6 (A = K, Rb; M = Sc, In): Topology–Symmetry Analysis, Order–Disorder and Structure–Properties Relation. Symmetry. 2022; 14(8):1699. https://doi.org/10.3390/sym14081699
Chicago/Turabian StyleReutova, Olga, Elena Belokoneva, Anatoly Volkov, Olga Dimitrova, and Sergey Stefanovich. 2022. "Two New Rb3Sc(IO3)6 Polytypes in Proposed Nonlinear Optical Family A3M(IO3)6 (A = K, Rb; M = Sc, In): Topology–Symmetry Analysis, Order–Disorder and Structure–Properties Relation" Symmetry 14, no. 8: 1699. https://doi.org/10.3390/sym14081699
APA StyleReutova, O., Belokoneva, E., Volkov, A., Dimitrova, O., & Stefanovich, S. (2022). Two New Rb3Sc(IO3)6 Polytypes in Proposed Nonlinear Optical Family A3M(IO3)6 (A = K, Rb; M = Sc, In): Topology–Symmetry Analysis, Order–Disorder and Structure–Properties Relation. Symmetry, 14(8), 1699. https://doi.org/10.3390/sym14081699





