Numerical Solution of Radiative and Viscous Dissipative Fluid Flow along an Oscillating Vertical Plate

Abstract

A numerical analysis of an unsteady radiative and viscous dissipative fluid flow across a semi-infinite oscillating vertical plate with a constant temperature and mass diffusion is examined here. The fluid under consideration is optically thin gray, emitting, heat-retaining, and non-dispersing. A finite-difference approach known as the Crank−Nicolson strategy was applied to find the solution to the limitless governing equations. Applications of such engineering problems can be found in fields such as aerospace, solar power, the cooling of nuclear reactors, and chemical and mechanical engineering. Based on the computational solutions, the impacts of distinct physical parameters, such as velocity, concentration, temperature, skin friction, Nusselt number, and Sherwood number profiles, are portrayed pictorially and imparted. The numerical solution of the velocity profile developed in this study fits extremely well with what was previously published and validated. Furthermore, we discovered that plate oscillation, radiation, and viscous dissipation parameters surprisingly influence the flow pattern.

1. Introduction

Free convection or natural convection is caused by density differences due to temperature variations in the fluid. Free convection flow has several practical applications in agriculture, oceanography, and industry. Due to its extensive range of applications, several articles have been published on the temperamental natural convection progression of a viscid incompressible fluid alongside a semi-boundless upward plate. Soundalgekar [1] has demonstrated that the free convection impacts on the stream over an upward swaying plate can be deduced. He also established that when the plate cools quickly, the velocity of the stream increases, and when the plate heats quickly, the velocity of the stream drops. The transient free convection stream with intermittent temperature variation of a boundless upward plate has been addressed by Das et al. [2].

Scientists have been fascinated by the aftermath of radiative heat and mass transfer due to their general applications in aerospace, solar power, cooling nuclear reactors, and synthetic materials. Using the Rosseland transition model, Hossain and Takhar [3] examined the radiation consequences of heterogeneous convection on an upward plate with a uniform surface temperature. Muthucumaraswamy [4] analyzed the radiative effects of an erratic shear convective stream over a boundless upward swaying plate with a varying temperature and mass dispersion. By the Laplace transform method, he obtained an exact solution to the non-dimensional governing equations. Muthucumaraswamy and Vijayalakshmi [5] investigated the effect of mass exchange and heat transfer across an oscillating upward plate with varying temperatures. The numerical solution provided by Raptis [6] describes the convectional oscillatory flow and mass exchange for a fluid with a thin optical layer lying above a porous plate within the sight of radiation. Prasad et al. [7] examined the impact of thermal radiation on a natural convective viscous incompressible stream travelling over a semi-boundless upward plate with constant mass and heat flux.

Fluid flows periodically illustrate symmetricity under certain conditions. However, such a symmetric flow is not always attained if circumstances change. Because of their importance and relevance in flow control and heat transfer enhancement, the breaks of flow symmetry have been explored theoretically, experimentally, and numerically in fluid dynamics and thermal engineering. The conversion of energy from one form to another is called dissipation in fluid dynamics. The amount of dissipation is high in regions with large gradients as well as in regions with high levels of turbulence. A temperature variation may affect the stream characteristics due to the liquid thickness based on the viscous dissipation’s liquid property. Viscous dissipation is a significant factor in geophysical streams and food processing. A semi-limitless vertical isothermal plate by Gebhart [8] was the first to demonstrate the significance of viscous dissipation in free convection streams. According to Mahajan and Gebhart [9], viscous heating dissipation impacts natural convection. Furthermore, they showed that viscous dissipation effects are negligible compared to pressure effects for all Prandtl numbers (Pr). Soundalgekar et al. [10] illustrated the effect of an erratic shear convection stream of a viscous dissipative fluid along a semi-boundless isothermal plate. Muthucumaraswamy and Saravanan [11] examined the impacts of radiation-induced waves on streams passing through a swaying semi-boundless upward plate with invariant mass and varying temperatures. Mondal et al. [12] developed a numerical study of radiated and chemical reactions associated with the fluid flow across a porous medium with viscous dissemination. The motion of an impenetrable viscous fluid over a permeable upward plate beneath the significance of radiation and viscous dissemination was studied by Das et al. [13]. Dharmendar Reddy and Srinivasa Rao [14] investigated the influence of viscous dissipation in the presence of a heat source/sink on an unsteady, two-dimensional laminar convective boundary layer flow of a viscous, incompressible, chemically reactive fluid along a semi-infinite vertical plate with suction. They applied the finite element technique (FEM) for solving the governing boundary layer equations. Hemamalini and Suresh Kumar [15] examined the impacts of viscous dispersion and warm radiation on a swaying upward plate in a penetrable medium.

Regardless, no expert has suggested that a numerical study be conducted of a transient free convective stream passing through a semi-limitless swaying upward plate in the existence of heat radiation and viscous dissemination. This study employs an implicit finite-difference approach to examine an upward oscillating semi-infinite plate with a stable mass and temperature distribution considering the thermal radiation and viscous dissipation effects.

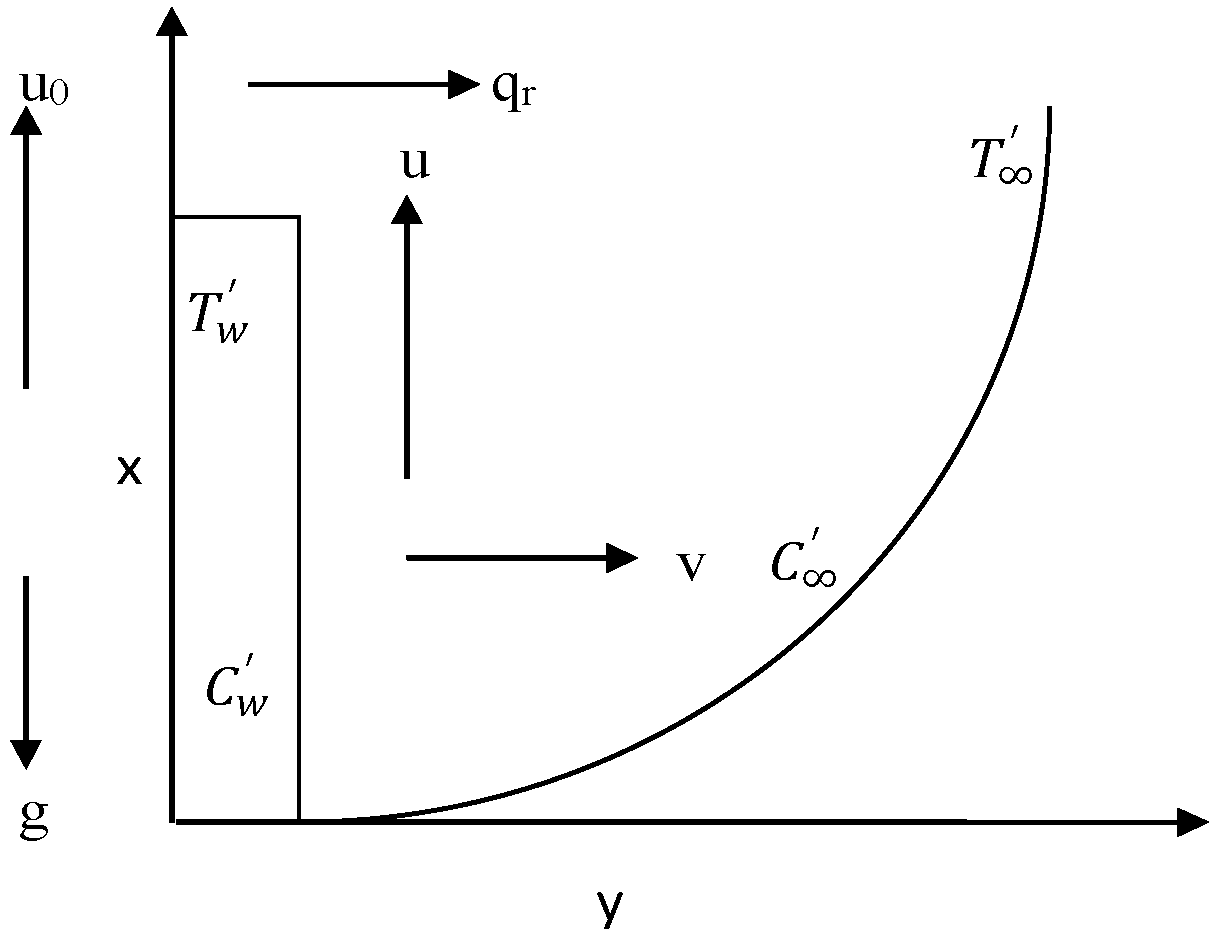
2. Mathematical Analysis

We propose to inspect the unstable laminar convective flow of an incompressible viscid liquid along an oscillating semi-boundless upward plate beneath the prominence of warm radiation and viscous dissemination. In this case, the plate is considered to be vertically aligned along the x-$axis$, with the y-$axis$ being normal to the plate as shown in Figure 1. The concept of symmetry is quite apparent in the boundary layer theory. Here, the boundary is treated as a wall boundary, and the x-$axis$ is considered as a line of symmetry. Both the liquid and plate are at an identical concentration ${\mathcal{C}}_{\infty }^{\prime }$ and temperature ${\mathcal{T}}_{\infty }^{\prime }$ at the beginning. The motion begins at the moment $t^{\prime }>0,$ and the plate sways in its distinguished notch with velocity $u_{0}cos{\omega }^{\prime }{t}^{\prime }$ while the concentration and temperature of the plate are simultaneously uplifted to ${\mathcal{C}}_w^{\prime }$ and ${\mathcal{T}}_w^{\prime }$, respectively. For the energy equation, viscous dissipation is taken into account. According to the above assumptions, the boundary layer equations for continuity, momentum, energy, and mass diffusion are presented below with the customary Boussinesq’s approximation (Gebart and Pera [16]):

$$u_x+u_y=0$$

(1)

$$u_{t^{\prime }}+u{u}_x+v{u}_y=g\beta \left({\mathcal{T}}^{\prime }-{\mathcal{T}}_{\infty }^{\prime }\right)+g{\beta }^{\ast }\left({\mathcal{C}}^{\prime }-{\mathcal{C}}_{\infty }^{\prime }\right)+\upsilon u_{yy}$$

(2)

$${\mathcal{T}}_{t^{\prime }}^{\prime }+u{\mathcal{T}}_x^{\prime }+v{\mathcal{T}}_y^{\prime }=\frac{k}{\rho C_p}{\mathcal{T}}_{yy}^{\prime }-\frac{1}{\rho C_p}{\left(q_r\right)}_y+\frac{\upsilon }{\rho C_p}{\left(u_y\right)}^2$$

(3)

$${\mathcal{C}}_{t^{\prime }}^{\prime }+u{\mathcal{C}}_x^{\prime }+v{\mathcal{C}}_y^{\prime }=D{\mathcal{C}}_{yy}^{\prime }$$

(4)

where $u \mathrm{and} v$ are velocity factors in the $x \mathrm{and} y$ path, respectively, $t^{\prime }$—time, $g$—acceleration due to gravity, ${\beta }^{*}\mathrm{and} \beta$—coefficient of volumetric concentration and thermal expansion, respectively, $k$—thermal conductivity, $\upsilon$—kinematic viscosity, $\rho$—fluid density, $\mu$—coefficient of viscosity, $C_p$—specific heat at constant pressure, ${\mathcal{C}}^{\prime }$—species concentration in the fluid, ${\mathcal{T}}^{\prime }$—temperature of the fluid near the plate, and ${\mathcal{C}}_{\infty }^{\prime }$ and ${\mathcal{T}}_{\infty }^{\prime }$—concentration and temperature at the free stream.

The associated boundary premises are as follows:

$$\begin{array}{l}{t}^{\prime }\le 0: u=0, v=0, {\mathcal{T}}^{\prime }={\mathcal{T}}_{\infty }^{\prime }, {\mathcal{C}}^{\prime }={\mathcal{C}}_{\infty }^{\prime }\\ t^{\prime }>0: u=u_0\cos {\omega }^{\prime }{t}^{\prime }, v=0, {\mathcal{T}}^{\prime }={\mathcal{T}}_w^{\prime }, {\mathcal{C}}^{\prime }={\mathcal{C}}_w^{\prime } at y=0\\ u=0, {\mathcal{T}}^{\prime }={\mathcal{T}}_{\infty }^{\prime }, {\mathcal{C}}^{\prime }={\mathcal{C}}_{\infty }^{\prime } at x=0\\ u\to 0, {\mathcal{T}}^{\prime }\to {\mathcal{T}}_{\infty }^{\prime }, {\mathcal{C}}^{\prime }\to {\mathcal{C}}_{\infty }^{\prime } as y\to \infty \end{array}\}$$

(5)

where $u_0$—velocity of the plate, ${\omega }^{\prime }{t}^{\prime }$—phase angle, and ${\mathcal{C}}_w^{\prime } \mathrm{and} {\mathcal{T}}_w^{\prime }$—concentration and temperature near the wall.

By employing the Rosseland approximation [17], the radiative heat flux $q_r$ is given by the following:

$${\left(q_r\right)}_y=-4a^{\ast }{\sigma }_s\left({{\mathcal{T}}_{\infty }^{\prime }}^4-{{\mathcal{T}}^{\prime }}^4\right)$$

(6)

where $a^{*}$—absorption constant, and ${\sigma }_s$—Stefan−Boltzmann constant.

It should be noted that by using the Rosseland approximation, the present analysis is limited to optically thick fluids. The refractive index of the gas medium is constant. The local thermal radiation intensity is due to radiation emanating from proximate locations in the vicinity of which emission and scattering are comparable to the location of interest. The energy transfer depends on conditions only in the area adjacent to the plate regime, i.e., the boundary layer regime. If the temperature differences within the flow are sufficiently small, then Equation (6) can be linearized by expanding ${{\mathcal{T}}^{\prime }}^4$ in a Taylor series about ${\mathcal{T}}_{\infty }^{\prime }$ and ignoring the terms of higher order, which results in the following:

$${{\mathcal{T}}^{\prime }}^4\cong 4{{\mathcal{T}}_{\infty }^{\prime }}^3{\mathcal{T}}^{\prime }-3{{\mathcal{T}}_{\infty }^{\prime }}^4$$

(7)

Applying Equations (6) and (7), in Equation (3), we arrive at the following:

$${\mathcal{T}}_{t^{\prime }}^{\prime }+u{\mathcal{T}}_x^{\prime }+v{\mathcal{T}}_y^{\prime }=\frac{k}{\rho C_p}\left({\mathcal{T}}_{yy}^{\prime }\right)+\frac{16a^{\ast }{\sigma }_s{{\mathcal{T}}_{\infty }^{\prime }}^3\left({\mathcal{T}}_{\infty }^{\prime }-{\mathcal{T}}^{\prime }\right)}{\rho C_p}+\frac{\upsilon }{\rho C_p}{\left(u_y\right)}^2$$

(8)

The following boundless quantities are identified by using the boundary layer equations and conditions:

$$\begin{array}{l}X=\frac{x{u}_0}{\upsilon }, Y=\frac{y{u}_0}{\upsilon }, U=\frac{u}{u_0}, V=\frac{v}{u_0}, t=\frac{t^{\prime }{u}_0^2}{\upsilon }, \omega =\frac{{\omega }^{\prime }\upsilon }{u_0^2},\\ \mathcal{T}=\frac{{\mathcal{T}}^{\prime }-{\mathcal{T}}_{\infty }^{\prime }}{{\mathcal{T}}_w^{\prime }-{\mathcal{T}}_{\infty }^{\prime }}, \mathcal{C}=\frac{{\mathcal{C}}^{\prime }-{\mathcal{C}}_{\infty }^{\prime }}{{\mathcal{C}}_w^{\prime }-{\mathcal{C}}_{\infty }^{\prime }}, Gr=\frac{\upsilon g\beta \left({\mathcal{T}}_w^{\prime }-{\mathcal{T}}_{\infty }^{\prime }\right)}{u_0^3}, Gc=\frac{\upsilon g{\beta }^{\ast }\left({\mathcal{C}}_w^{\prime }-{\mathcal{C}}_{\infty }^{\prime }\right)}{u_0^3},\\ \mathrm{Pr}=\frac{\upsilon }{\alpha }, Sc=\frac{\upsilon }{D}, Ec=\frac{u_0^2}{C_p\left({\mathcal{T}}_w^{\prime }-{\mathcal{T}}_{\infty }^{\prime }\right)}, R=\frac{16a^{\ast }{\upsilon }^2{\sigma }_s{{\mathcal{T}}_{\infty }^{\prime }}^3}{k{u}_0^2}\end{array}\}$$

(9)

where “$X$—boundless spatial coordinate along the plate”, “$Y$—boundless spatial coordinate normal to the plate”, $U, V$—boundless velocity components in $X, Y$—direction respectively, $t$—boundless time, $\omega$—frequency of oscillation, $\mathcal{T}$—boundless temperature, $\mathcal{C}$—boundless concentration, “$Gr$—thermal Grashof number”, “$Gc$—mass Grashof number”, “$Pr$—Prandtl number”, “$Sc$—Schmidt number”, “$Ec$—Eckert number”, “$R$—radiation parameter”, $\alpha$—thermal diffusivity, and $D$—mass diffusion coefficient.

After applying Equation (9) in Equations (1)–(4), the following boundless equations are obtained:

$$U_X+V_Y=0$$

(10)

$$U_t+U{U}_X+V{U}_Y=Gr\mathcal{T}+Gc\mathcal{C}+U_{YY}$$

(11)

$${\mathcal{T}}_t+U{\mathcal{T}}_X+V{\mathcal{T}}_Y=\frac{1}{\mathrm{Pr}}\left({\mathcal{T}}_{YY}\right)-\frac{R\mathcal{T}}{\mathrm{Pr}}+Ec{\left(U_Y\right)}^2$$

(12)

$${\mathcal{C}}_t+U{\mathcal{C}}_X+V{\mathcal{C}}_Y=\frac{1}{Sc}{\mathcal{C}}_{YY}$$

(13)

The associated boundless boundary premises are as follows:

$$\begin{array}{l}t\le 0: U=0, V=0, \mathcal{T}=0, \mathcal{C}=0\\ t^{\prime }>0: U=\cos \omega t, V=0, \mathcal{T}=1, \mathcal{C}=1 at Y=0\\ U=0, \mathcal{T}=0, \mathcal{C}=0 at X=0\\ U\to 0, \mathcal{T}\to 0, \mathcal{C}\to 0, as Y\to \infty \end{array}\}$$

(14)

where $\omega t$—boundless phase angle.

The boundless form of the local and average skin friction is shown here:

$${\tau }_X=-{\left(\frac{\partial U}{\partial Y}\right)}_{Y=0} \mathrm{and} \overline{\tau }=-{\displaystyle \underset{0}{\overset{1}{\int }}{\left(\frac{\partial U}{\partial Y}\right)}_{Y=0}dX}$$

(15)

The boundless form of the local and average Nusselt number is as follows:

$$N{u}_X=-X\left[{\left(\frac{\partial \mathcal{T}}{\partial Y}\right)}_{Y=0}/{\mathcal{T}}_{Y=0}\right] \mathrm{and} \overline{Nu}=-{\displaystyle \underset{0}{\overset{1}{\int }}\left[{\left(\frac{\partial \mathcal{T}}{\partial Y}\right)}_{Y=0}/{\mathcal{T}}_{Y=0}\right]dX}$$

(16)

The boundless form of the local and average Sherwood number is as follows:

$$S{h}_X=-X\left[{\left(\frac{\partial \mathcal{C}}{\partial Y}\right)}_{Y=0}/{\mathcal{C}}_{Y=0}\right] \mathrm{and} \overline{Sh}=-{\displaystyle \underset{0}{\overset{1}{\int }}\left[{\left(\frac{\partial \mathcal{C}}{\partial Y}\right)}_{Y=0}/{\mathcal{C}}_{Y=0}\right]dX}$$

(17)

3. Numerical Solution of the Problem

The coupled non-linear partial differential Equations (10)–(13) under Equation (14) are converted into finite-difference equations by the Crank−Nicolson method.

$$\frac{\left[U_{i,j}^{n+1}-U_{i-1,j}^{n+1}+U_{i,j}^n-U_{i-1,j}^n+U_{i,j-1}^{n+1}-U_{i-1,j-1}^{n+1}+U_{i,j-1}^n-U_{i-1,j-1}^n\right]}{4\Delta X}+\frac{\left[V_{i,j}^{n+1}-V_{i,j-1}^{n+1}+V_{i,j}^n-V_{i,j-1}^n\right]}{2\Delta Y}=0$$

(18)

$$\begin{array}{l}\frac{\left[U_{i,j}^{n+1}-U_{i,j}^n\right]}{\Delta t}+U_{i,j}^n\frac{\left[U_{i,j}^{n+1}-U_{i-1,j}^{n+1}+U_{i,j}^n-U_{i-1,j}^n\right]}{2\Delta X}+V_{i,j}^n\frac{\left[U_{i,j+1}^{n+1}-U_{i,j-1}^{n+1}+U_{i,j+1}^n-U_{i,j-1}^n\right]}{4\Delta Y}\\ =\frac{Gr}{2}\left[{\mathcal{T}}_{i,j}^{n+1}+{\mathcal{T}}_{i,j}^n\right]+\frac{Gc}{2}\left[{\mathcal{C}}_{i,j}^{n+1}+{\mathcal{C}}_{i,j}^n\right]+\frac{\left[U_{i,j-1}^{n+1}-2U_{i,j}^{n+1}+U_{i,j+1}^{n+1}+U_{i,j-1}^n-2U_{i,j}^n+U_{i,j+1}^n\right]}{2{\left(\Delta Y\right)}^2}\end{array}$$

(19)

$$\begin{array}{l}\frac{\left[{\mathcal{T}}_{i,j}^{n+1}-{\mathcal{T}}_{i,j}^n\right]}{\Delta t}+U_{i,j}^n\frac{\left[{\mathcal{T}}_{i,j}^{n+1}-{\mathcal{T}}_{i-1,j}^{n+1}+{\mathcal{T}}_{i,j}^n-{\mathcal{T}}_{i-1,j}^n\right]}{2\Delta X}+V_{i,j}^n\frac{\left[{\mathcal{T}}_{i,j+1}^{n+1}-{\mathcal{T}}_{i,j-1}^{n+1}+{\mathcal{T}}_{i,j+1}^n-{\mathcal{T}}_{i,j-1}^n\right]}{4\Delta Y}\\ =\frac{1}{\mathrm{Pr}}\frac{\left[{\mathcal{T}}_{i,j-1}^{n+1}-2{\mathcal{T}}_{i,j}^{n+1}+{\mathcal{T}}_{i,j+1}^{n+1}+{\mathcal{T}}_{i,j-1}^n-2{\mathcal{T}}_{i,j}^n+{\mathcal{T}}_{i,j+1}^n\right]}{2{\left(\Delta Y\right)}^2}-\frac{R}{2\mathrm{Pr}}\left[{\mathcal{T}}_{i,j}^{n+1}+{\mathcal{T}}_{i,j}^n\right]+Ec{\left[\frac{U_{i,j+1}^n-U_{i,j}^n}{\Delta Y}\right]}^2\end{array}$$

(20)

$$\begin{array}{l}\frac{\left[{\mathcal{C}}_{i,j}^{n+1}-{\mathcal{C}}_{i,j}^n\right]}{\Delta t}+U_{i,j}^n\frac{\left[{\mathcal{C}}_{i,j}^{n+1}-{\mathcal{C}}_{i-1,j}^{n+1}+{\mathcal{C}}_{i,j}^n-{\mathcal{C}}_{i-1,j}^n\right]}{2\Delta X}+V_{i,j}^n\frac{\left[{\mathcal{C}}_{i,j+1}^{n+1}-{\mathcal{C}}_{i,j-1}^{n+1}+{\mathcal{C}}_{i,j+1}^n-{\mathcal{C}}_{i,j-1}^n\right]}{4\Delta Y}\\ =\frac{1}{Sc}\frac{\left[{\mathcal{C}}_{i,j-1}^{n+1}-2{\mathcal{C}}_{i,j}^{n+1}+{\mathcal{C}}_{i,j+1}^{n+1}+{\mathcal{C}}_{i,j-1}^n-2{\mathcal{C}}_{i,j}^n+{\mathcal{C}}_{i,j+1}^n\right]}{2{\left(\Delta Y\right)}^2}\end{array}$$

(21)

The detailed description of the functions and interrelationship connecting Equations (18)–(21) is referred to by John D. Anderson, Jr. [18]. At each interior nodal point on a specific i-level, the outputs of $U, V,\mathcal{T} \mathrm{and} \mathcal{C}$ are obtained from the finite-difference Equations (18)–(21), forming a tri-diagonal system of equations that is solved by the Thomas algorithm as discussed by Carnahan et al. [19]. A rectangular mesh is assumed here with sides $X_{max}=1 \mathrm{and} Y_{max}=10$ where $Y_{max}$ corresponds to $Y=\infty$ or those that lie beyond the thermal and momentum boundary layers. The limit of $Y$ was picked as 10 after some fundamental mathematical calculations to such an extent that the last two of the limit conditions of Equation (14) are fulfilled inside as far as possible at ${10}^{-5}$. The mesh sizes were set to $“\Delta X=0.05”, “\Delta Y=0.125”$ with a time period of “$\Delta t=0.01”.$

4. Graphical Results and Discussion

We used the Windows version of FORTRAN (Force 2.0) to compute the numerical values of $U, V,\mathcal{T} \mathrm{and} \mathcal{C}$ using the iterative procedure, and the outcomes of the numerical results were plotted graphically using MATLAB software. The steady-state computational values of velocity, concentration, temperature, local and average skin friction, Nusselt number, and Sherwood number were computed using physical parameters such as the phase angle “t = π/6”, thermal radiation “R = 2”, Grashof numbers “Gr = 5, Gc = 5”, Prandtl number “Pr = 0.71 (air)”, Schmidt number “Sc = 0.6 (water vapour)”, and Eckert number “Ec = 0.5”. All figures are based on these values unless specified otherwise.

Figure 2 illustrates the discrimination of the present velocity profile against the exact solution unveiled by Muthucumaraswamy [4]. The parameters “R = 0.2, 5, 20”, “ωt = π/6”, “Gr = 5”, “Gc = 2”, “Pr = 0.71”, “Sc = 0.3”, and “t = 0.4” are used for comparison, and the results are compatible with the exact solution. Figure 3a depicts velocity profiles for various phase angle values. The velocity is found to decrease as the phase angle increases. It can also be seen that velocity diminishes in the presence of high wavering. Furthermore, it has been noted that the time required to attain the consistent state on a vertical plate “ωt = 0” is much greater than on a flat plate “ωt = π/2”.

A consistent velocity profile for thermal and mass Grashof numbers “Gr” and “Gc” can be seen in Figure 3b. Rising the thermal Grashof number (or mass Grashof number) will result in more incredible air velocity. In the context of mass buoyancy, inflation in thermal and mass Grashof numbers increases the effect of mass buoyancy. The velocity is revamped by essential plate cooling. The steady-state velocity pattern corresponding to a range of the radiation parameter “R” is exhibited in Figure 3c. It was noticed that increasing the radiation parameter resulted in a decrease in velocity.

Figure 3d depicts the impact of velocity patterns for various Schmidt number “Sc” values. It has been found that velocity diminishes while expanding the Schmidt number. Furthermore, the time it takes to attain a consistent state depends on the Schmidt number. In addition, the limit layer is formed along the major end of a semi-endless upward plate moving in a liquid and the plate’s direction. According to the results, maximum overall velocity is produced by mass dispersion and buoyancy force. The consistent state velocity pattern for the various range of the Eckert number “Ec” is represented in Figure 3e. The graph clearly illustrates that velocity increases as the Eckert number increases.

Figure 4 depicts the impact of various Schmidt numbers on the concentration profile. It has been noticed that the concentration of plates diminished as the Schmidt number value was increased. Generally, it is true that an ascent in ‘Sc’ firmly squashes the molecular diffusivity. The temperature profile of various radiation parameters is depicted in Figure 5a. It can be identified that extending the radiation boundary “R” brings about a lessening of the velocity just as it does the temperature inside the limit layer. The temperature drops dramatically from the plate peak value at “y = 0” to the free stream “y → ∞” as the radiation parameter “R” increases. The Eckert number “Ec” has a significant impact on temperature, as can be seen in Figure 5b. The temperature rises as the dissemination boundary is extended. When viscous dissipation takes place in the stream field, there is an increase in energy, causing a rise in the temperature of the liquid and, therefore, in buoyancy.

Figure 6 demonstrates the local skin friction for various values of the phase angle “ωt” as an element of the pivotal coordinate “X”. The shear stress local wall increments with diminishing upsides of stage point “ωt”. As a result, an upward plate “ωt = 0” will have more significant wall shear stress than a flat plate “ωt = π/2”. Figure 7 illustrates the impacts of various phase angles on the average skin friction values over the time “t” at “X = 1.0”. When the phase angle “ωt” is expanded, the value of average skin friction diminishes. The impact of the Eckert number “Ec” over the local Nusselt number is represented in Figure 8 as a component of the pivotal coordinate “X”. The heat transfer rate generally increases as the dissipative parameter “Ec” decreases. The average Nusselt number as a function of time “t” at “X = 1.0” is shown in Figure 9. As the dissipative parameter “Ec” diminishes, the average Nusselt number increases. A local Sherwood number is shown in Figure 10 for various upsides of the Schmidt number “Sc”. When the Schmidt number “Sc” increases, the rate of mass transfer increases. On the other hand, the concentration profile on Figure 4 reverses this pattern. Figure 11 demonstrates the relation between the average Sherwood number and the Schmidt number. As the Schmidt number rises, the average Sherwood number increases as well.

5. Conclusions

In the existence of warm radiation and viscous dissipation, an upward swaying semi-endless plate with a constant mass and temperature is investigated. Analyzing the results for several parameters is depicted in the graph. The current study reveals the following intriguing features:

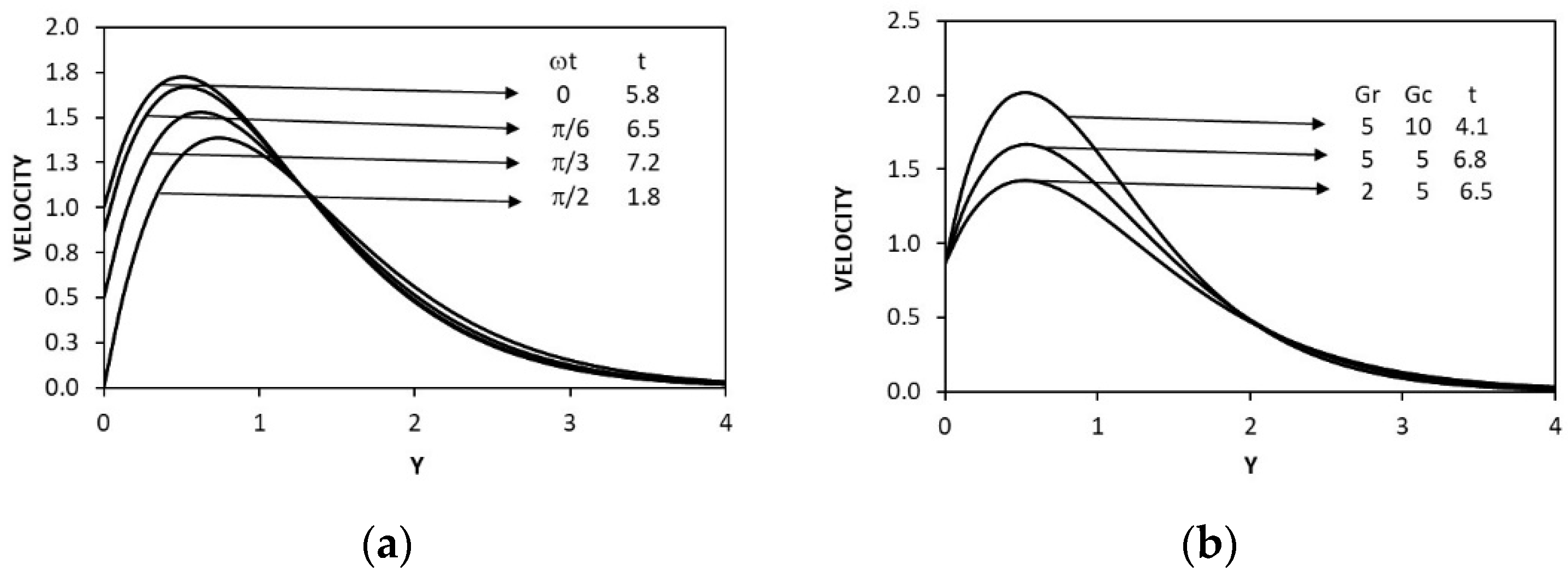
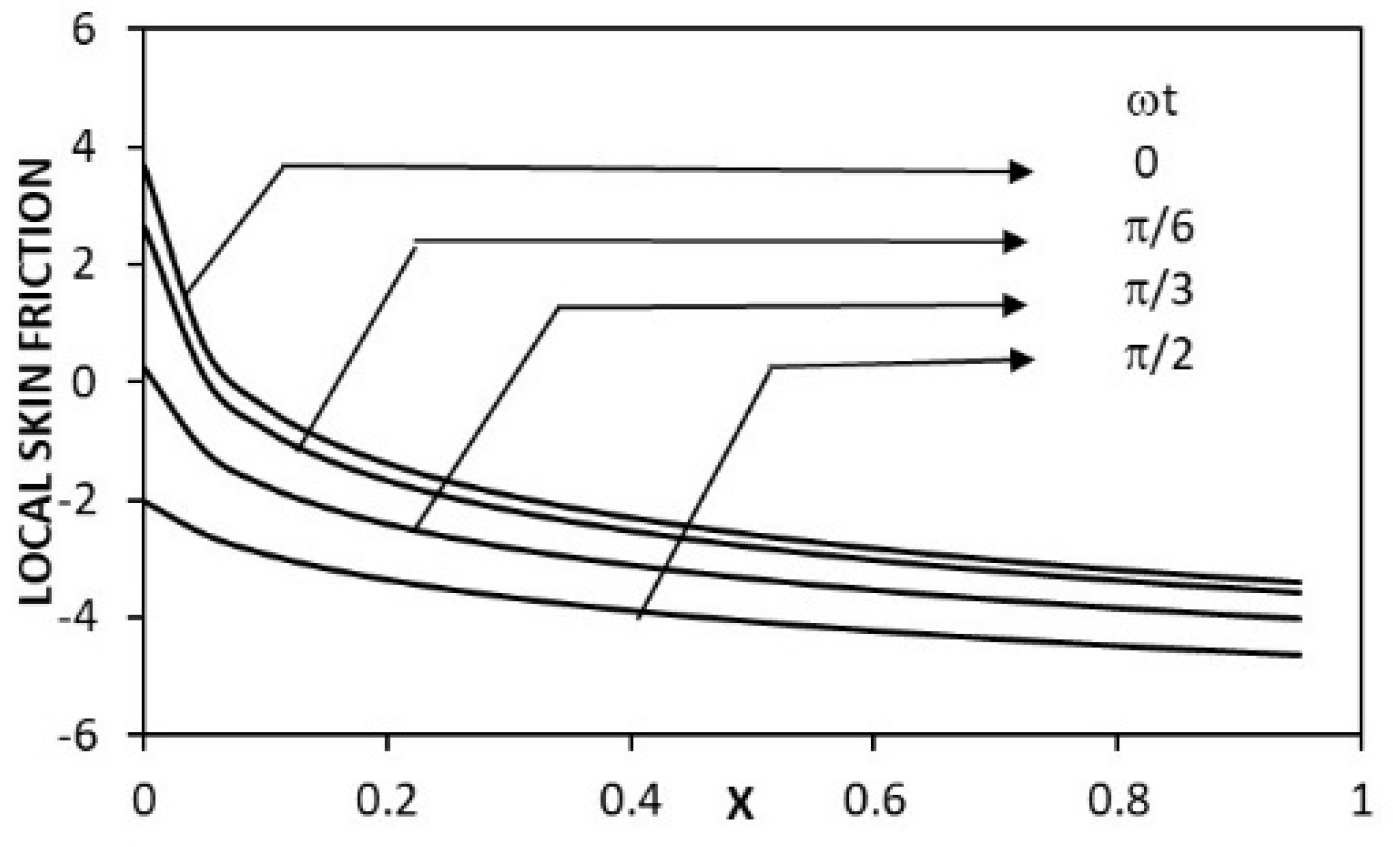
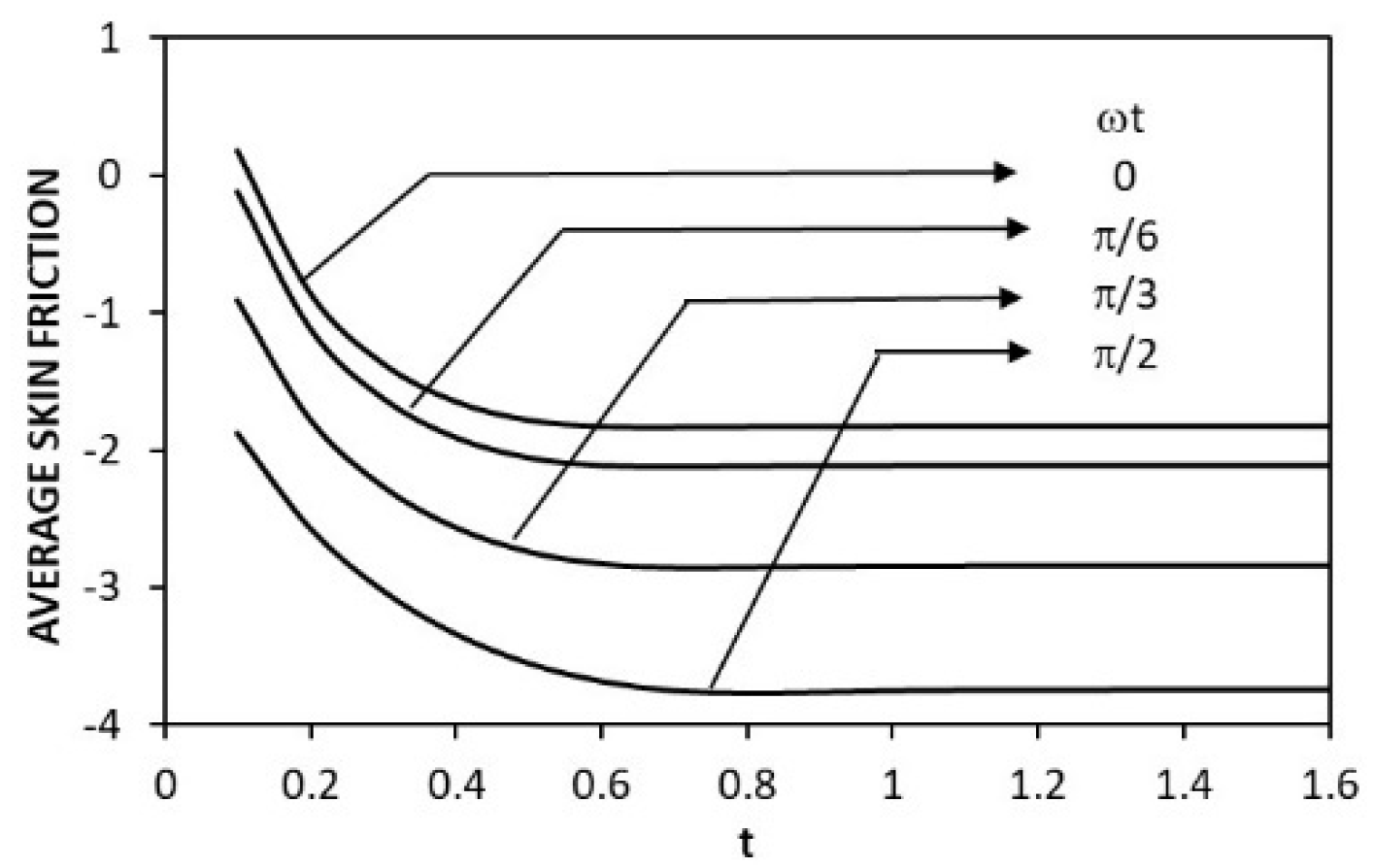
• The velocity decreases when the phase angle for air in the cooling of the plate is raised.
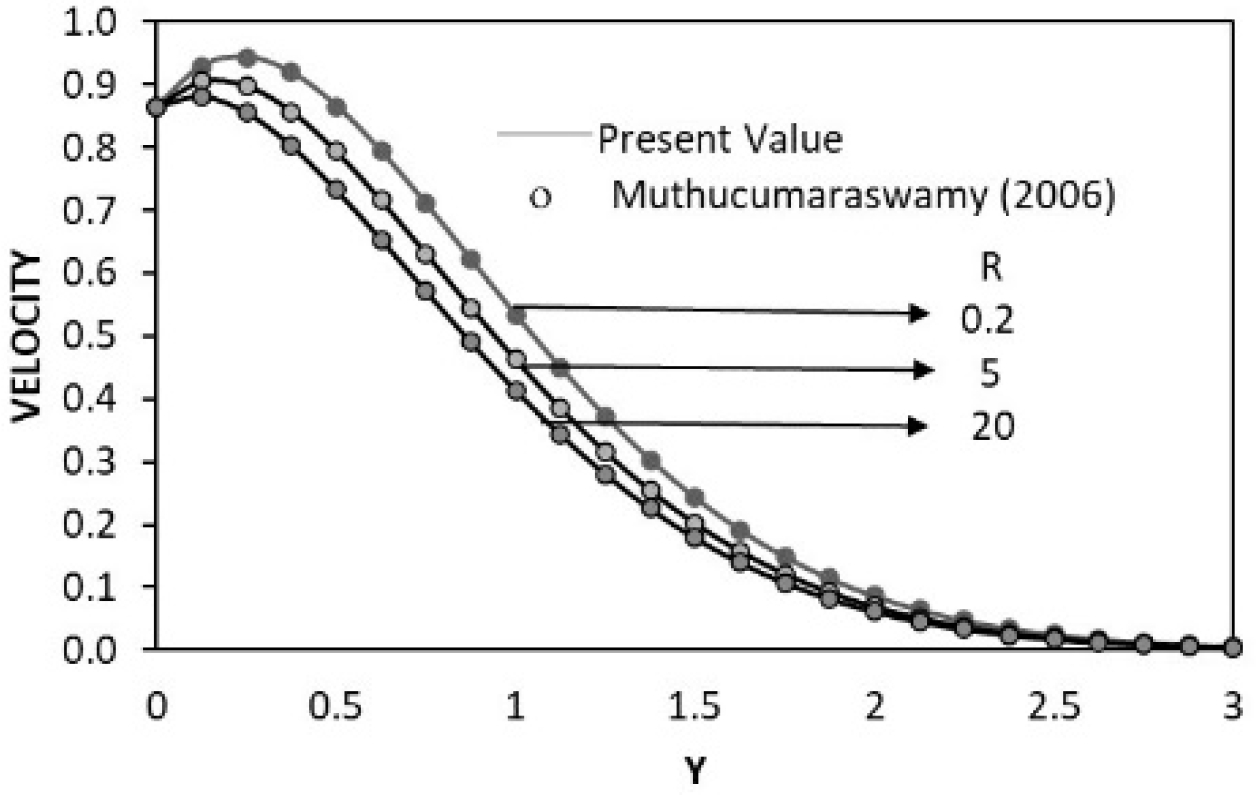
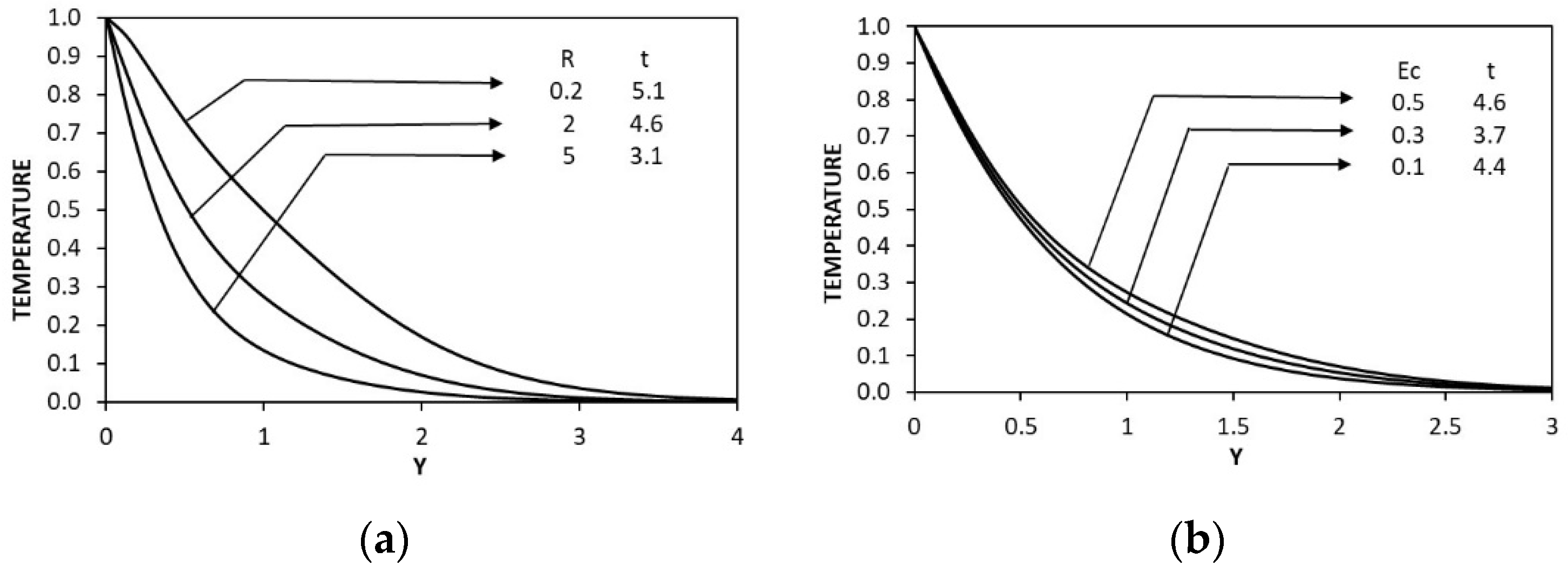
• Both the velocity and temperature fall while the radiation parameter increases.
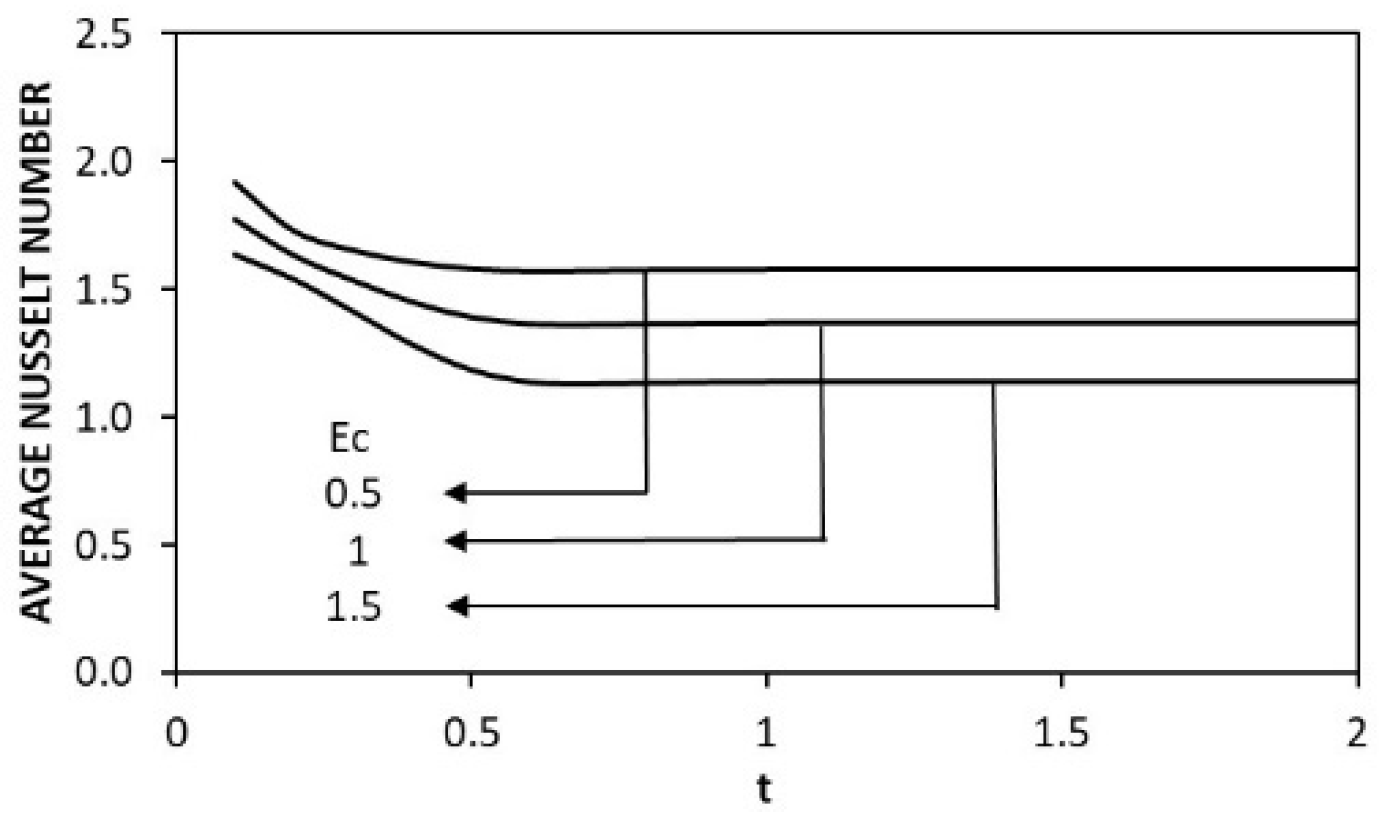
• Temperature falls while expanding the radiation parameter but rises when increasing the Eckert number.
• Local and average skin friction decrease as the phase angle increases.
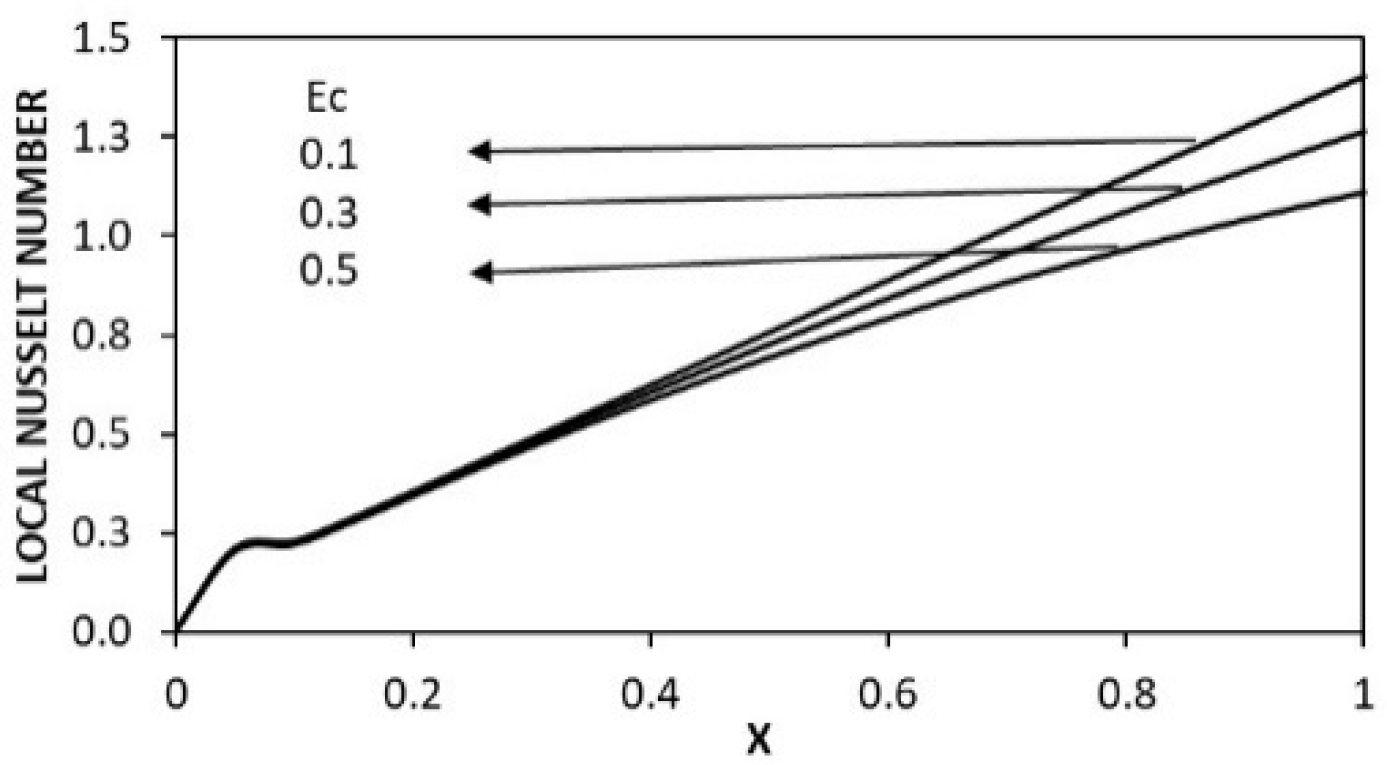
• Eckert numbers enhance velocity and temperature profiles but diminish local and average Nusselt numbers.
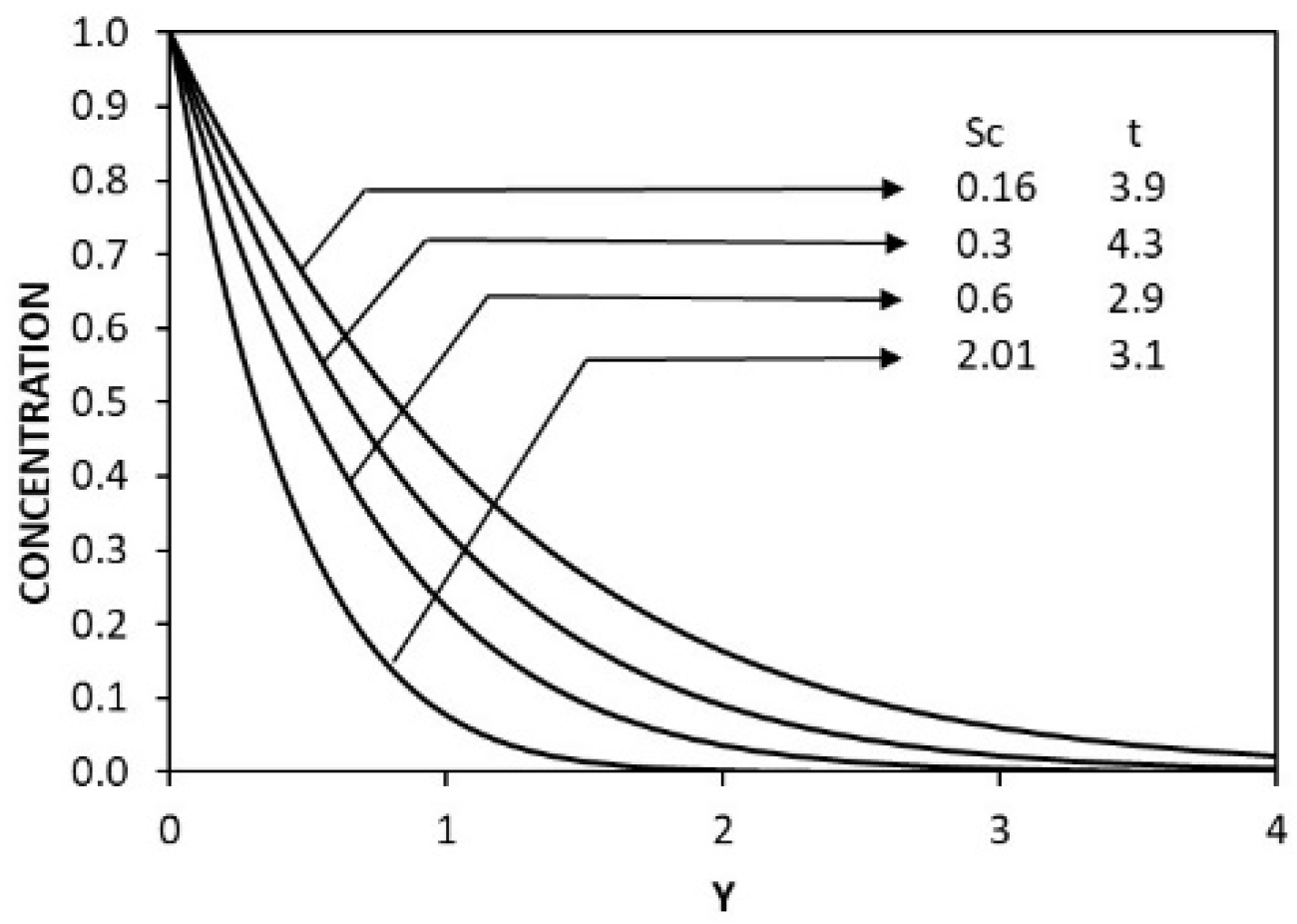
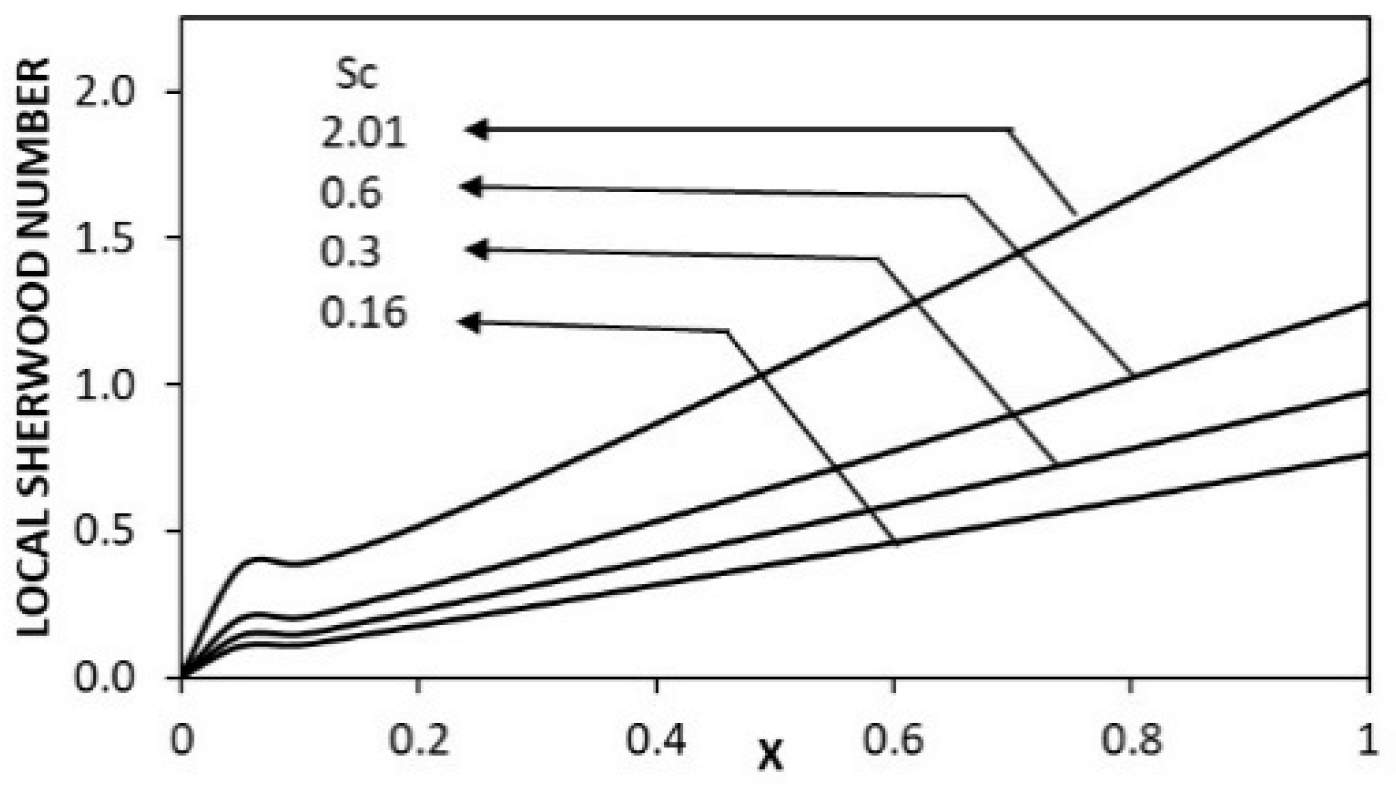
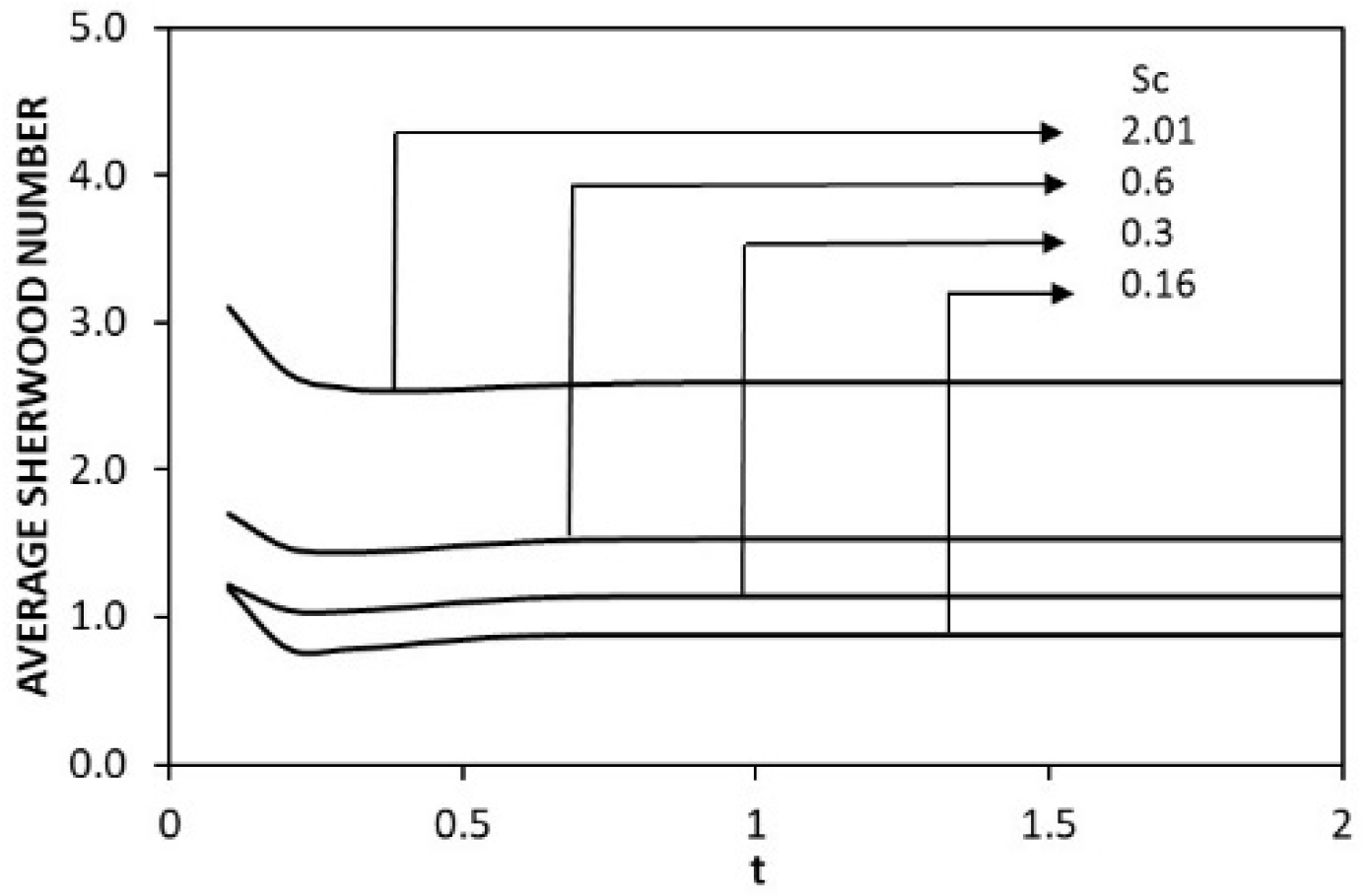
• Local and average Sherwood numbers are enhanced by an expanding Schmidt number, but in concentration, the pattern reverses.
• According to the results of this study, the number of steps required to achieve a consistent state is highly influenced by the radiation parameter and phase angle.