Josephson-like Oscillations in Toroidal Spinor Bose–Einstein Condensates: A Prospective Symmetry Probe
Abstract
:1. Introduction
2. General Modeling of Spinor BECs
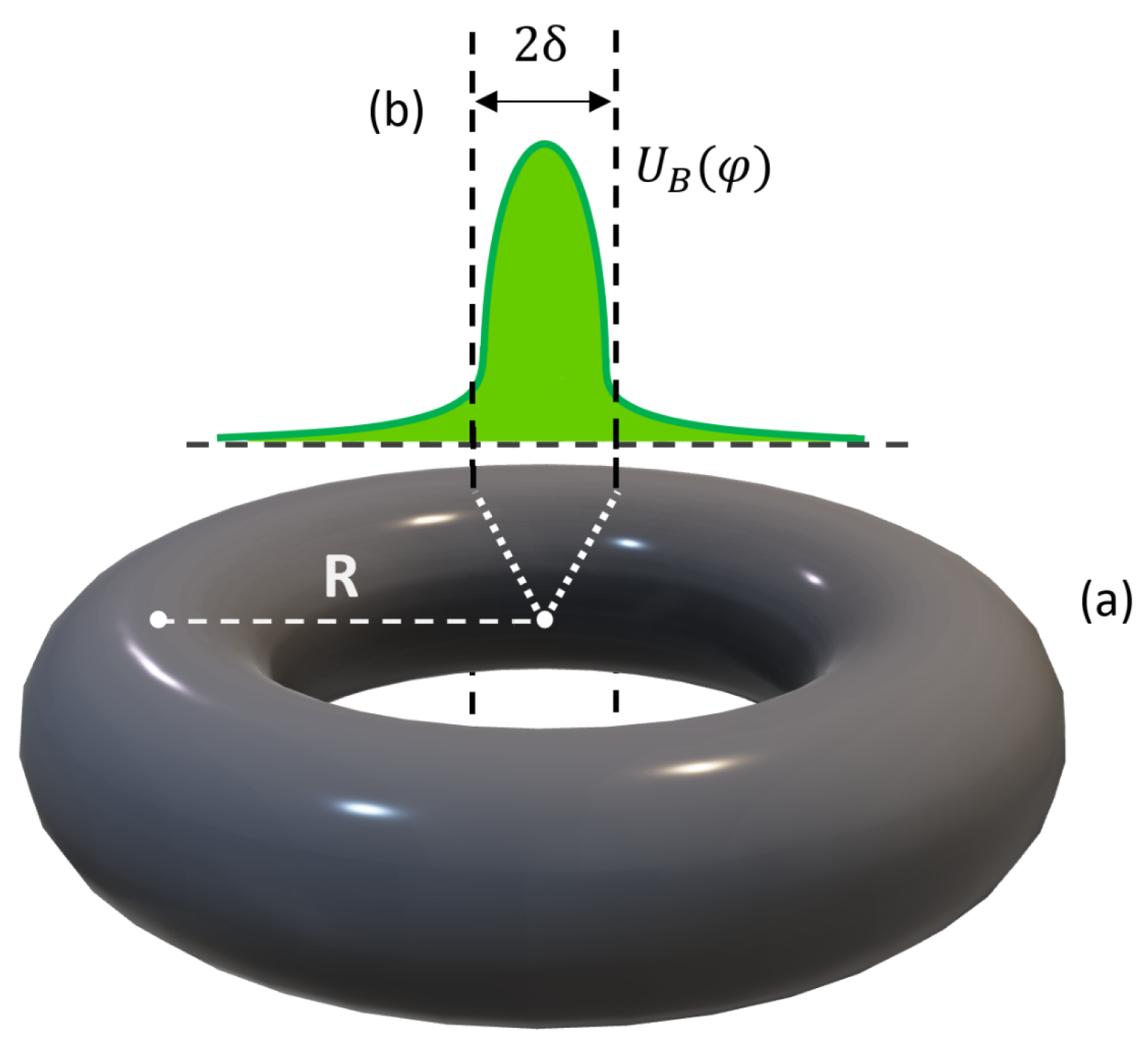
3. Modeling the Potential Barrier and Defining Some Approximations
4. Current Density and the Parity of the Order Parameter
5. The Broken-Symmetry Spinor BEC Case
5.1. Ferromagnetic States
5.2. Polar State
6. Landau Critical Velocity, Lower Bound for R, and Experimental Protocol
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Josephson, B.D. Possible new effects in superconductive tunnelling. Phys. Lett. 1962, 1, 251–253. [Google Scholar] [CrossRef]
- Josephson, B.D. The discovery of tunnelling supercurrents. Rev. Mod. Phys. 1974, 46, 251–254. [Google Scholar] [CrossRef] [Green Version]
- Barone, A.; Paterno, G. Physics and Applications of the Josephson Effect; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 1982. [Google Scholar] [CrossRef]
- Tinkham, M. Introduction to Superconductivity; Dover Books on Physics Series; Dover Publications: Mineola, NY, USA, 2004. [Google Scholar]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175–1204. [Google Scholar] [CrossRef] [Green Version]
- Cummings, F.W.; Johnston, J.R. Theory of Superfluidity. Phys. Rev. 1966, 151, 105–112. [Google Scholar] [CrossRef]
- Pethick, C.; Smith, H. Bose–Einstein condensation in Dilute Gases; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Pitaevskii, L.; Stringari, S. Bose-Einstein Condensation and Superfluidity; Oxford University Press: Oxford, UK, 2016; p. 576. [Google Scholar] [CrossRef]
- Kawaguchi, Y.; Ueda, M. Spinor Bose–Einstein condensates. Phys. Rep. 2012, 520, 253–381. [Google Scholar] [CrossRef] [Green Version]
- Stamper-Kurn, D.M.; Ueda, M. Spinor Bose gases: Symmetries, magnetism, and quantum dynamics. Rev. Mod. Phys. 2013, 85, 1191–1244. [Google Scholar] [CrossRef] [Green Version]
- Yukalov, V.I. Dipolar and spinor bosonic systems. Laser Physics 2018, 28, 053001. [Google Scholar] [CrossRef] [Green Version]
- Penrose, O.; Onsager, L. Bose-Einstein Condensation and Liquid Helium. Phys. Rev. 1956, 104, 576–584. [Google Scholar] [CrossRef]
- Bogoliubov, N. On the Theory of Superfluidity. J. Phys. 1947, 11, 3–20. [Google Scholar] [CrossRef] [Green Version]
- Zapata, I.; Sols, F.; Leggett, A.J. Josephson effect between trapped Bose-Einstein condensates. Phys. Rev. A 1998, 57, R28–R31. [Google Scholar] [CrossRef] [Green Version]
- Williams, J.; Walser, R.; Cooper, J.; Cornell, E.; Holland, M. Nonlinear Josephson-type oscillations of a driven, two-component Bose-Einstein condensate. Phys. Rev. A 1999, 59, R31–R34. [Google Scholar] [CrossRef] [Green Version]
- Hall, D.S.; Matthews, M.R.; Wieman, C.E.; Cornell, E.A. Measurements of Relative Phase in Two-Component Bose-Einstein Condensates. Phys. Rev. Lett. 1998, 81, 1543–1546. [Google Scholar] [CrossRef] [Green Version]
- Albiez, M.; Gati, R.; Fölling, J.; Hunsmann, S.; Cristiani, M.; Oberthaler, M.K. Direct Observation of Tunneling and Nonlinear Self-Trapping in a Single Bosonic Josephson Junction. Phys. Rev. Lett. 2005, 95, 010402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Levy, S.; Lahoud, E.; Shomroni, I.; Steinhauer, J. The a.c. and d.c. Josephson effects in a Bose–Einstein condensate. Nature 2007, 449, 579–583. [Google Scholar] [CrossRef] [PubMed]
- Ryu, C.; Blackburn, P.W.; Blinova, A.A.; Boshier, M.G. Experimental Realization of Josephson Junctions for an Atom SQUID. Phys. Rev. Lett. 2013, 111, 205301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sadler, L.E.; Higbie, J.M.; Leslie, R.; Vengalattore, M.; Stamper-Kurn, D.M. Spontaneous symmetry breaking in a quenched ferromagnetic spinor Bose–Einstein condensate. Nature 2006, 443, 312–315. [Google Scholar] [CrossRef] [Green Version]
- Faddeev, L.; Niemi, A.J. Stable knot-like structures in classical field theory. Nature 1997, 387, 58–61. [Google Scholar] [CrossRef] [Green Version]
- Kawaguchi, Y.; Nitta, M.; Ueda, M. Knots in a Spinor Bose-Einstein Condensate. Phys. Rev. Lett. 2008, 100, 180403. [Google Scholar] [CrossRef] [PubMed]
- Savage, C.M.; Ruostekoski, J. Dirac monopoles and dipoles in ferromagnetic spinor Bose-Einstein condensates. Phys. Rev. A 2003, 68, 043604. [Google Scholar] [CrossRef] [Green Version]
- Khawaja, U.A.; Stoof, H. Skyrmions in a Ferromagnetic Bose-Einstein Condensate. Nature 2001, 411, 918–920. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grimm, R.; Weidemüller, M.; Ovchinnikov, Y.B. Optical Dipole Traps for Neutral Atoms. In Advances In Atomic, Molecular, and Optical Physics; Academic Press: Cambridge, MA, USA, 2000; pp. 95–170. [Google Scholar] [CrossRef] [Green Version]
- Pasienski, M.; DeMarco, B. A high-accuracy algorithm for designing arbitrary holographic atom traps. Opt. Express 2008, 16, 2176–2190. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gaunt, A.L.; Hadzibabic, Z. Robust Digital Holography for Ultracold Atom Trapping. Sci. Rep. 2012, 2, 721. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gauthier, G.; Lenton, I.; Parry, N.M.; Baker, M.; Davis, M.J.; Rubinsztein-Dunlop, H.; Neely, T.W. Direct imaging of a digital-micromirror device for configurable microscopic optical potentials. Optica 2016, 3, 1136–1143. [Google Scholar] [CrossRef] [Green Version]
- Silva, P.F.; Muniz, S.R. Generating arbitrary laser beam shapes through phase-mapped designed beam splitting. In Proceedings of the 2021 SBFoton International Optics and Photonics Conference (SBFoton IOPC), Sao Carlos, Brazil, 31 May–2 June 2021; pp. 1–4. [Google Scholar] [CrossRef]
- Ramanathan, A.; Wright, K.C.; Muniz, S.R.; Zelan, M.; Hill III, W.T.; Lobb, C.J.; Helmerson, K.; Phillips, W.D.; Campbell, G.K. Superflow in a Toroidal Bose-Einstein Condensate: An Atom Circuit with a Tunable Weak Link. Phys. Rev. Lett. 2011, 106, 130401. [Google Scholar] [CrossRef] [Green Version]
- Wright, K.C.; Blakestad, R.B.; Lobb, C.J.; Phillips, W.D.; Campbell, G.K. Driving Phase Slips in a Superfluid Atom Circuit with a Rotating Weak Link. Phys. Rev. Lett. 2013, 110, 025302. [Google Scholar] [CrossRef]
- Eckel, S.; Lee, J.G.; Jendrzejewski, F.; Murray, N.; Clark, C.W.; Lobb, C.J.; Phillips, W.D.; Edwards, M.; Campbell, G.K. Hysteresis in a quantized superfluid ‘atomtronic’ circuit. Nature 2014, 506, 200–203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Beattie, S.; Moulder, S.; Fletcher, R.J.; Hadzibabic, Z. Persistent Currents in Spinor Condensates. Phys. Rev. Lett. 2013, 110, 025301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldman, N.; Juzeliūnas, G.; Öhberg, P.; Spielman, I.B. Light-induced gauge fields for ultracold atoms. Rep. Prog. Phys. 2014, 77, 126401. [Google Scholar] [CrossRef] [PubMed]
- Baym, G.; Pethick, C.J. Landau critical velocity in weakly interacting Bose gases. Phys. Rev. A 2012, 86, 023602. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.; Yi, S.; You, L. Mean field ground state of a spin-1 condensate in a magnetic field. New J. Phys. 2003, 5, 77. [Google Scholar] [CrossRef]
- Andrews, M.R.; Mewes, M.O.; van Druten, N.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Direct, Nondestructive Observation of a Bose Condensate. Science 1996, 273, 84–87. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ramanathan, A.; Muniz, S.R.; Wright, K.C.; Anderson, R.P.; Phillips, W.D.; Helmerson, K.; Campbell, G.K. Partial-transfer absorption imaging: A versatile technique for optimal imaging of ultracold gases. Rev. Sci. Instr. 2012, 83, 083119. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Moulder, S.; Beattie, S.; Smith, R.P.; Tammuz, N.; Hadzibabic, Z. Quantized supercurrent decay in an annular Bose-Einstein condensate. Phys. Rev. A 2012, 86, 013629. [Google Scholar] [CrossRef] [Green Version]
- Leanhardt, A.E.; Görlitz, A.; Chikkatur, A.P.; Kielpinski, D.; Shin, Y.; Pritchard, D.E.; Ketterle, W. Imprinting Vortices in a Bose-Einstein Condensate using Topological Phases. Phys. Rev. Lett. 2002, 89, 190403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leanhardt, A.E.; Shin, Y.; Kielpinski, D.; Pritchard, D.E.; Ketterle, W. Coreless Vortex Formation in a Spinor Bose-Einstein Condensate. Phys. Rev. Lett. 2003, 90, 140403. [Google Scholar] [CrossRef] [Green Version]
- Kumakura, M.; Hirotani, T.; Okano, M.; Takahashi, Y.; Yabuzaki, T. Topological formation of a multiply charged vortex in the Rb Bose-Einstein condensate: Effectiveness of the gravity compensation. Phys. Rev. A 2006, 73, 063605. [Google Scholar] [CrossRef] [Green Version]
- Wright, K.C.; Leslie, L.S.; Bigelow, N.P. Optical control of the internal and external angular momentum of a Bose-Einstein condensate. Phys. Rev. A 2008, 77, 041601. [Google Scholar] [CrossRef]
- Wright, K.C.; Leslie, L.S.; Hansen, A.; Bigelow, N.P. Sculpting the Vortex State of a Spinor BEC. Phys. Rev. Lett. 2009, 102, 030405. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leslie, L.S.; Hansen, A.; Wright, K.C.; Deutsch, B.M.; Bigelow, N.P. Creation and Detection of Skyrmions in a Bose-Einstein Condensate. Phys. Rev. Lett. 2009, 103, 250401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Juliá-Díaz, B.; Guilleumas, M.; Lewenstein, M.; Polls, A.; Sanpera, A. Josephson oscillations in binary mixtures of F = 1 spinor Bose-Einstein condensates. Phys. Rev. A 2009, 80, 023616. [Google Scholar] [CrossRef] [Green Version]
- Juliá-Díaz, B.; Melé-Messeguer, M.; Guilleumas, M.; Polls, A. Spinor Bose-Einstein condensates in a double well: Population transfer and Josephson oscillations. Phys. Rev. A 2009, 80, 043622. [Google Scholar] [CrossRef] [Green Version]
- Muñoz Mateo, A.; Gallemí, A.; Guilleumas, M.; Mayol, R. Persistent currents supported by solitary waves in toroidal Bose-Einstein condensates. Phys. Rev. A 2015, 91, 063625. [Google Scholar] [CrossRef] [Green Version]
- Petrov, D.S.; Shlyapnikov, G.V.; Walraven, J.T.M. Phase-Fluctuating 3D Bose-Einstein Condensates in Elongated Traps. Phys. Rev. Lett. 2001, 87, 050404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Al Khawaja, U.; Proukakis, N.P.; Andersen, J.O.; Romans, M.W.J.; Stoof, H.T.C. Dimensional and temperature crossover in trapped Bose gases. Phys. Rev. A 2003, 68, 043603. [Google Scholar] [CrossRef] [Green Version]
- Gerbier, F. Quasi-1D Bose-Einstein condensates in the dimensional crossover regime. Europhys. Lett. (EPL) 2004, 66, 771–777. [Google Scholar] [CrossRef]
- Mathey, L.; Ramanathan, A.; Wright, K.C.; Muniz, S.R.; Phillips, W.D.; Clark, C.W. Phase fluctuations in anisotropic Bose-Einstein condensates: From cigars to rings. Phys. Rev. A 2010, 82, 033607. [Google Scholar] [CrossRef] [Green Version]
- Piazza, F.; Collins, L.A.; Smerzi, A. Critical velocity for a toroidal Bose–Einstein condensate flowing through a barrier. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 095302. [Google Scholar] [CrossRef] [Green Version]
- Mathey, A.C.; Clark, C.W.; Mathey, L. Decay of a superfluid current of ultracold atoms in a toroidal trap. Phys. Rev. A 2014, 90, 023604. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Donato, M.H.F.; Muniz, S.R. Josephson-like Oscillations in Toroidal Spinor Bose–Einstein Condensates: A Prospective Symmetry Probe. Symmetry 2022, 14, 867. https://doi.org/10.3390/sym14050867
Donato MHF, Muniz SR. Josephson-like Oscillations in Toroidal Spinor Bose–Einstein Condensates: A Prospective Symmetry Probe. Symmetry. 2022; 14(5):867. https://doi.org/10.3390/sym14050867
Chicago/Turabian StyleDonato, Mário H. Figlioli, and Sérgio R. Muniz. 2022. "Josephson-like Oscillations in Toroidal Spinor Bose–Einstein Condensates: A Prospective Symmetry Probe" Symmetry 14, no. 5: 867. https://doi.org/10.3390/sym14050867
APA StyleDonato, M. H. F., & Muniz, S. R. (2022). Josephson-like Oscillations in Toroidal Spinor Bose–Einstein Condensates: A Prospective Symmetry Probe. Symmetry, 14(5), 867. https://doi.org/10.3390/sym14050867






