Next Article in Journal
Algebra of the Symmetry Operators of the Klein–Gordon–Fock Equation for the Case When Groups of Motions G3 Act Transitively on Null Subsurfaces of Spacetime
Previous Article in Journal
A Software Reliability Model with Dependent Failure and Optimal Release Time
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Symmetry
Volume 14
Issue 2
10.3390/sym14020344
symmetry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

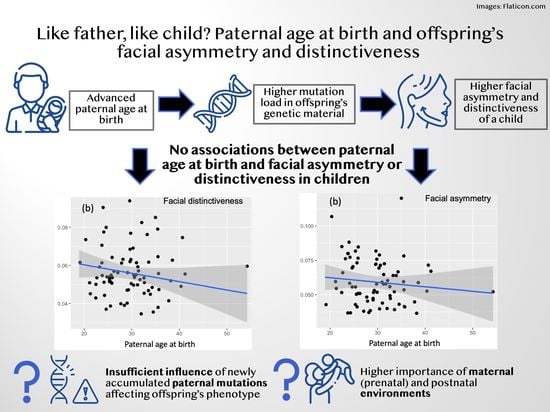
Like Father, Like Child? Paternal Age at Birth and Offspring’s Facial Asymmetry and Distinctiveness

by
Magdalena Klimek
1,*,
Urszula M. Marcinkowska
1,
Pawel Fedurek
2,
Karel Kleisner
3 and
Dariusz P. Danel
4
1
Department of Environmental Health, Faculty of Health Sciences, Jagiellonian University Medical College, 31-066 Krakow, Poland
2
Division of Psychology, Faculty of Natural Sciences, University of Stirling, Stirling FK9 4LA, UK
3
Department of Philosophy and History of Science, Faculty of Science, Charles University, 128 00 Prague, Czech Republic
4
Department of Anthropology, Hirszfeld Institute of Immunology and Experimental Therapy Polish Academy of Sciences, 53-114 Wrocław, Poland
*
Author to whom correspondence should be addressed.
Symmetry 2022, 14(2), 344; https://doi.org/10.3390/sym14020344
Submission received: 24 November 2021 / Revised: 21 January 2022 / Accepted: 1 February 2022 / Published: 8 February 2022
(This article belongs to the Section Life Sciences)
Browse Figures
Versions Notes

Abstract

:
Paternal age at birth, a potential proxy of mutation load transmitted to the offspring, has previously been related to the offspring’s health, biological condition and reproductive potential. As higher facial asymmetry and distinctiveness serve as putative markers of the lower genetic quality of an individual, we hypothesize that more advanced paternal age at birth will be related to children’s higher levels of facial asymmetry and distinctiveness. To the best of our knowledge, this is the first study evaluating the link between paternal age at birth and facial asymmetry in offspring. Based on archived photographs of 159 children born within 47 Polish families, we have conducted facial geometric morphometric measurements and calculated the levels of facial asymmetry and distinctiveness. The relationship between paternal age at birth and the offspring’s facial features was explored with the use of Bayesian Linear Mixed-effects Models, controlling for sex, age and birth order of the offspring, and maternal age at child’s birth. No associations between paternal age at birth and facial asymmetry or distinctiveness in children were found. The lack of such a relationship might be a result of the potentially insufficient influence of newly accumulated paternal mutations affecting the offspring’s phenotype or higher importance of maternal (prenatal) and postnatal environments in shaping facial features.

Graphical Abstract

1. Introduction

Within the last decades of Developmental Origins of Health and Disease (DOHaD) research, maternal factors were designated as having primary importance in shaping the in utero environment and later-life characteristics of the offspring, leading to a large imbalance in DOHaD research towards maternal exposures [1]. Recently, various paternal factors, e.g., father’s age, stress exposure, cigarette smoking or metabolic condition [2,3,4], have received greater attention and were suggested as equally important to maternal factors in forming a child’s biological status and health, e.g., [5]. A growing body of evidence indicating the significance of paternal factors gave rise to the newly emerged paradigm of POHaD (Paternal Origins of Health and Disease) as an extension of the DOHaD concept [6].
Paternal age at birth (but not maternal) is suggested as a proxy of mutation load in the genetic material accumulated over an extended period of time and passed to the offspring [7]. The discrepancy between paternal and maternal age effects on de novo mutations is explained by the fact that women are born with their full egg supply and all germ cell divisions already completed. Paternal genetic material, however, especially in advanced-aged fathers, undergoes many cell divisions and chromosome replications which are continuously occurring in the sperm cells throughout the reproductive life span [8]. Therefore, paternal genetic material carries a much higher mutation load passed to the embryo than maternal genetic material [7]. Other possible mechanisms suggested as mediators of the effects of advanced paternal age on a child’s health or reproductive outcomes (acting in isolation or interplaying with the maternal factors) might be epigenetic changes in the paternal genetic material (e.g., DNA methylation) or an accumulation of other stressful environmental exposures during the lifetime [9,10].
Paternal age at birth (PAB) is used as a proxy reflecting the quality of genetic material inherited from father and was recently designated as a crucial determinant of offspring’s health status, e.g., risk of neurodevelopmental disorders such as schizophrenia or autism spectrum disorders, childhood cancers, or advanced biological age reflected in shortened telomere length (for a review see: [11]). Advanced paternal age might also contribute to the reproductive capabilities of children, e.g., lower chances of getting married and higher chances of remaining childless [12], and lower reproductive success, which was manifested among 1.4 million individuals from four populations living across four centuries [13]. However, on the other hand, the advanced PAB was formerly also considered as potentially beneficial. The offspring of late-reproducing fathers might inherit longer telomeres due to the fact that their sperm was (among other explanations) exposed to telomerase activity for a prolonged period of time before conception [14].
Facial features are proposed to be one of the biomarkers of health and reproductive status of an individual. Level of asymmetry, attractiveness or distinctiveness (the opposite of averageness) are thought to result as a positive outcome of the interaction between genetic, developmental and environmental processes taking place in the ontogeny [15]. The main up to date interest in PAB effects on facial features in offspring concentrated, for the most part, on facial attractiveness. Two large recent studies presented a negative association between PAB and attractiveness level, suggesting that the higher attractiveness of children conceived by younger fathers might be a sign of lesser sensitivity for mutations and higher fitness indicators [16,17]. Nevertheless, a study by Lee and colleagues [18] yielded a contrary result of a positive association between advanced PAB and the subject’s attractiveness level, yet in a relatively smaller and less representative sample than in Huber and Fieder [16] and Woodley of Menie and Kanazawa [17] studies. What is also worth mentioning, Lee and colleagues [18] extended the tested facial features with facial averageness level but found no association with PAB. None of the previous studies examined the association between the level of facial asymmetry in the offspring and paternal age at birth.
Average and symmetrical faces are perceived as more healthy and more attractive than their less average and less symmetrical counterparts [19,20]. The preference for symmetrical and average faces was found cross-culturally [21]. It is not clear, though, why in some studies, averageness appears to be more important for judgements of attractiveness than symmetry per se [22].
Here we explore the association between paternal age at birth and the level of facial asymmetry and distinctiveness in offspring. We hypothesize that more advanced paternal age at birth will be related to children’s higher levels of facial asymmetry and distinctiveness (indicating putatively lower genetic quality) controlling for sex, age and birth order of the offspring, and maternal age at birth. This is the first study evaluating the link between paternal age at birth and facial asymmetry in offspring.

2. Materials and Methods

2.1. Study Group

We have used archival data and facial photographs that were collected between 1967 and 1979 in Miękinia, southwest Poland of children within 55 families. Four families with stepchildren were excluded from the current analyses, as well as another four families who did not have facial photographs of the desired quality. Finally, data from 47 families were explored, that is 47 mothers (mean age 38.33, SD = 5.59), 47 fathers (mean age 41.42, SD = 7.04), and 159 children (mean age 11.66, SD = 4.23). Demographic and family structure data (i.e., birth order, sex of each child) were gathered via in-person questionnaires. Black and white facial photographs were collected from all available children in each family. None of the participants presented visual facial deformities.
The negatives with facial portraits were digitalized and, together with the data, are currently archived at the Department of Anthropology, Hirszfeld Institute of Immunology and Experimental Therapy, Polish Academy of Sciences in Wrocław, Poland. The photos were used in our previous study [23].
Participants’ birth dates and examination dates were collected accurately to one day. Maternal and paternal age, as well as the age of the offspring at the moment of entering the study, were computed based on a decimal calendar. Subsequently, the paternal and maternal ages at birth for each child were calculated. The descriptive statistics of the investigated variables are presented in Table 1.
The study was approved by the Ethical Committee of the Institute of Anthropology, Polish Academy of Sciences, Wrocław. All subjects gave their informed consent for inclusion before they participated in the study, and it was conducted in accordance with the Declaration of Helsinki. A further description of the “family survey” data can be found in Danel et al. [23].

2.2. Geometric Morphometrics

We placed 72 points (landmarks) on each facial portrait to capture information about the facial shape. Landmarks were digitized using TpsDig2 software, version 2.31 [24]. From the overall number of 72 landmarks, we specified 36 as semilandmarks that describe the shape variation along the curves and outlines. In this study, we used the same landmark configuration as in our previous studies; for exact positions and definitions of landmarks and semilandmarks on human faces see: [23,25]. The position of the selected landmarks, along with the description of their exact location on the facial photographs, are presented in the Electronic Supplementary Materials (Figure S1, Table S1).
Subsequently, all landmark configurations represented by a 2D array of cartesian coordinates were subjected to Generalized Procrustes Analysis (GPA) using the gpagen() function from the ‘geomorph’ package in R [26]. Procrustes analysis centered, scaled and rotated all facial configurations giving aligned shape coordinates, i.e., Procrustes residuals. The semilandmark positions along the curves were optimized by a method that minimizes bending energy between each specimen and the mean configuration [27]. We ran GPA separately for each group (daughters and sons) and used the resulting Procrustes residuals for the calculation of facial distinctiveness and asymmetry. Facial distinctiveness was computed as the Procrustes distance between each configuration in the set and the group average. The longer the distance of a face from average, the more distinct the face. Greater values indicate higher levels of distinctiveness. To compute the facial asymmetry, the group-specific Procrustes residuals were first laterally reflected along the midline axis. In the next step, the corresponding paired landmarks on the left and right sides of faces were relabeled; the numeric indexes of landmarks on the left side were swapped for the indexes of landmarks from the right side and vice versa. Subsequently, we calculated Procrustes distances between the original and the mirrored configurations. The higher values of asymmetry scores indicate greater facial asymmetry.

2.3. Statistical Analysis

Two Bayesian Linear Mixed-effects Models (LMMs) were used in the analyses (see: [28] for mathematical details of Bayesian LMMs). To examine the relationship between facial distinctiveness and the relevant variables, we created an LMM with facial distinctiveness as the dependent variable, and maternal age at birth, paternal age at birth, birth order, sex (0-female, 1-male) and age of a child, as the independent variables. To examine the relationship between facial asymmetry and the relevant variables, we built an LMM with facial asymmetry as the dependent variable and the same independent variables as in the previous model. Family ID was set as a random intercept. To reduce Type I error and to account for potential nonuniform variability of the independent variables within the random effects, we included random slopes for all the independent variables within the family [29].
There was no collinearity between the independent variables (variance inflation factors of the independent variables were <2.5). The values of all quantitative variables were z transformed into a mean of 0 and a standard deviation of 1. Statistical analyses were conducted using R, version 3.3.0, and the ‘brme’ package [28], version 1.0-5. We used weakly regularizing prior for the fixed effects (Normal (0,1)) since they deal effectively with outliers and restrict model predictions to biologically meaningful estimates and CIs [30,31]. For the random effects, we used the default priors using the function ‘get_prior’ from the package ‘brms’ (i.e., Student’s t (3, 0, 2.5) for the random intercepts and slopes, and lkj (1) for their correlation [31].

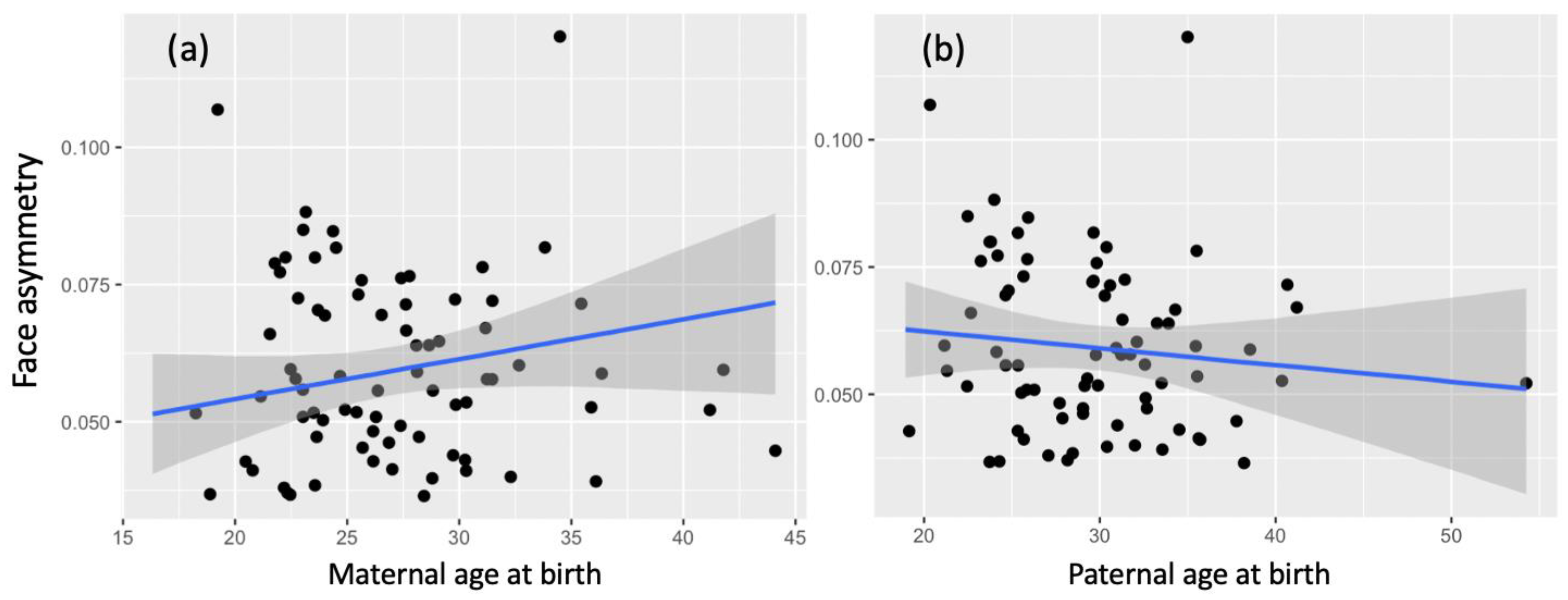
3. Results

The mean offspring’s facial distinctiveness was 0.06 (SD = 0.01) and mean facial asymmetry reached 0.06 (SD = 0.02) (Table 1). Males had higher facial distinctiveness than females (Table 2), but there was no relationship between facial asymmetry and the sex of the offspring (Table 3).

3.1. Facial Distinctiveness

There was a negative relationship between facial distinctiveness and both own age and birth order (Table 2) of a child. There was a positive relationship between facial distinctiveness and maternal age at birth (Table 2, Figure 1a), but no relationship between facial distinctiveness and paternal age at birth (the 95% CI for the estimate of this variable overlapped with 0; Table 2, Figure 1b).

3.2. Facial Asymmetry

There was a negative relationship between facial asymmetry and both age and birth order (Table 3). Both maternal and paternal age at birth (Table 3, Figure 2a,b) were not related to the facial asymmetry of the offspring (the 95% CI for the estimate of these variables overlapped with 0).

4. Discussion

In this study, we did not find support for the hypothesis that an offspring’s facial asymmetry and distinctiveness are related to paternal age at birth (PAB), taking into account the offspring’s sex, age, birth order and maternal age at birth (MAB). The lack of association between PAB and facial distinctiveness is in accordance with the results of the previous study by Lee et al. [18]. However, we did find, contrary to Lee et al. [18], partial support for a relationship between facial features and maternal age at birth—the higher the MAB, the greater facial distinctiveness of her offspring. There was no relationship between facial asymmetry and MAB.
A plausible explanation for the lack of relationship between the PAB and the offspring’s facial asymmetry and distinctiveness is a potentially insufficient influence of newly accumulated mutations which might not affect the phenotype, as their effects are buffered by conserved core processes that facilitate the generation of phenotypic variation in the offspring [32]. Nonetheless, we cannot rule out the paternal effects on offspring facial morphology, as the recent metanalysis revealed the increased risk of facial deformities (mostly cleft lip) in children of older fathers [33]. In the current study, however, we did not enroll participants who had facial deformities hence we could not evaluate this hypothesis.
Another possibility is that MAB is of more importance due to the crucial role of the prenatal environment for bilateral development. For instance, sons of the mothers that experienced a traumatic event during pregnancy had less symmetrical faces [34]. In addition, mothers who experienced high levels of stress caused by a natural disaster during gestation gave birth to children with high dermatoglyphic asymmetry (a putative marker of intrauterine instabilities) [35]. However, in our study, we did not find support for an association between MAB and the facial symmetry of the offspring, but only their facial distinctiveness. It was previously suggested that it is facial distinctiveness (not facial asymmetry) that reflects the actual health status of adolescents [20] therefore this parameter might be more strongly correlated with maternal age at birth (and therefore, the quality of intrauterine environment). Additionally, the postnatal environment might have a stronger influence on facial asymmetry/distinctiveness than the prenatal one, e.g., childhood social status [36], living conditions [37], etc. (see: [38] for the lack of relationship between longitudinal health measures and facial characteristics).
Based on the current dataset, we cannot rule out a possibility that fathers who were capable of siring children at older ages (thus potentially having resources for a prolonged reproductive span) were also more attractive due to, among others, lower facial asymmetry and distinctiveness. Putatively, only more attractive or healthy fathers could sire children at older ages or for a longer period of time. If facial asymmetry and distinctiveness were heritable (although see: Lee et al. [18] for mixed results on the heritability of these facial characteristics), children of older fathers would inherit lowered asymmetry and distinctiveness, and that in turn would rescind the effect of mutation load accumulation. It is also possible that late-reproducing men had higher socioeconomic status, and they were able to secure more resources for their partners (increasing quality of the prenatal environment) and their children (increasing quality of the postnatal environment) [39,40]. Future studies exploring PAB and the offspring’s facial features with the simultaneous control for paternal asymmetry or socio-economic status, could shed more light on this idea.
Our results are unlikely to be explained by a slow de novo mutations accumulation throughout the age span of fathers in the current sample (as suggested by Lee et al. [18], to explain their lack of significant relation). Although the de novo mutation accumulation is only approximately two mutations per year [7], PAB in the current sample spanned from 18 to 54 years of age, covering almost the entire reproductive lifespan of men. Therefore, some of the surveyed men could have accumulated relatively high levels of de novo mutations, yet no effect was found.
The lack of relationship between PAB and facial asymmetry or distinctiveness adds interesting insight into the previously reported relation between PAB and the offspring’s facial attractiveness [16,17], but see: [18]. It is plausible that the previously found decrease in offspring’s attractiveness along with increasing PAB is not related to facial shape. Facial attractiveness is a multifaceted concept that conveys numerous characteristics, such as skin texture, pigmentation, smoothness, symmetry, averageness and sexual dimorphism [41]. Recent studies also suggested that facial attractiveness can stem from (i) a so called face space that is “a multi-dimensional space representing global shape and color properties of faces derived from Principal Component Analysis” [42,43], or (ii) representational sparseness that is based on an “algorithm that estimates the sparseness of neurons in the visual cortex required to represent a given face image”, and so it is suggested to predict judgements of facial attractiveness based on the facial cognition efficiency [44]. Either of these two possible mechanisms behind facial attractiveness would not need a significant relationship between facial asymmetry/distinctiveness and attractiveness and hence are a culprit for mediating the effect between PAB and the offspring’s attractiveness. In future studies, correlating either of the two suggested facial features of offspring with PAB and MAB could aid in testing this hypothesis.
The main limitation of the current study might be a relatively insufficient number of participants compared to the previous studies on the effect of PAB on the facial features of the offspring [17]. Additionally, as the presence of secular trends in paternal age was formerly raised [45] and the photographs used in this study were taken several decades ago, our results might not reflect the currently existing associations. Future studies replicating our analyses among contemporary families could verify if the potential effect of the shift in reproductive timing in men is influential for the investigated hypothesis.

5. Conclusions

To the best of our knowledge, this is the first study evaluating the link between paternal age at birth and facial asymmetry in offspring. In summary, based on a sample of 47 mothers and fathers and 159 children, we did not find a relationship between paternal age at birth and the offspring’s facial asymmetry or distinctiveness. In contrast, we found that maternal age at birth is positively related to the facial distinctiveness (but not asymmetry) of the offspring. Future studies may profit from using up-to-date data and information on heritable effects and/or additional facial measurements to better understand the reflections of PAB in a child’s facial morphology and their potential link to biological quality. We also suggest that future studies investigate the link between MAB and facial distinctiveness in more detail.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/sym14020344/s1, Figure S1: Positions of landmarks and semilandmarks on a face. Landmarks are marked as white filled circles and semilandmarks as black circles; Table S1: The location of each selected landmark on the facial photographs.

Author Contributions

Conceptualization, M.K. and U.M.M.; methodology, D.P.D.; formal analysis, P.F. and D.P.D.; investigation, M.K. and U.M.M.; data curation, D.P.D. and M.K.; writing—original draft preparation, M.K., U.M.M., P.F. and K.K.; writing—review and editing, M.K., U.M.M., P.F., K.K. and D.P.D.; visualization, P.F.; supervision, D.P.D.; project administration, D.P.D.; funding acquisition, D.P.D. and K.K. All authors have read and agreed to the published version of the manuscript.

Funding

D.P.D. was supported by the National Science Centre, Poland (research grant number: 2020/04/X/NZ8/00865). K.K. was supported by the Czech Science Foundation project reg. no 21-10527S.

Institutional Review Board Statement

The study was conducted in accordance with the Declaration of Helsinki, and approved by the Ethical Committee of the Institute of Anthropology, Polish Academy of Sciences, Wrocław, Poland.

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Data Availability Statement

The data presented in this study are available in the ESM2.

Acknowledgments

The authors are grateful to all the families who devoted their time and participated in this study. We also thank all colleagues who, currently or in the past, made our study possible.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Sharp, G.C.; Schellhas, L.; Richardson, S.S.; Lawlor, D.A. Time to cut the cord: Recognizing and addressing the imbalance of DOHaD research towards the study of maternal pregnancy exposures. J. Dev. Orig. Health Dis. 2019, 10, 509–512. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  2. Sartorius, G.A.; Nieschlag, E. Paternal age and reproduction. Hum. Reprod. Update 2010, 16, 65–79. [Google Scholar] [CrossRef] [Green Version]
  3. Pang, T.Y.; Short, A.K.; Bredy, T.W.; Hannan, A.J. Transgenerational paternal transmission of acquired traits: Stress-induced modification of the sperm regulatory transcriptome and offspring phenotypes. Curr. Opin. Behav. Sci. 2017, 14, 140–147. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  4. Eberle, C.; Kirchner, M.F.; Herden, R.; Stichling, S. Paternal metabolic and cardiovascular programming of their offspring: A systematic scoping review. PLoS ONE 2020, 15, e0244826. [Google Scholar] [CrossRef]
  5. Braun, J.M.; Messerlian, C.; Hauser, R. Fathers matter: Why it’s time to consider the impact of paternal environmental exposures on children’s health. Curr. Epidemiol. Rep. 2017, 4, 46–55. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  6. Soubry, A. POHaD: Why we should study future fathers. Environ. Epigenetics 2018, 4, dvy007. [Google Scholar] [CrossRef] [PubMed]
  7. Kong, A.; Frigge, M.L.; Masson, G.; Besenbacher, S.; Sulem, P.; Magnusson, G.; Gudjonsson, S.A.; Sigurdsson, A.; Jonasdottir, A.; Jonasdottir, A. Rate of de novo mutations and the importance of father’s age to disease risk. Nature 2012, 488, 471–475. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  8. Crow, J.F. The origins, patterns and implications of human spontaneous mutation. Nat. Rev. Genet. 2000, 1, 40–47. [Google Scholar] [CrossRef] [PubMed]
  9. Janecka, M.; Mill, J.; Basson, M.; Goriely, A.; Spiers, H.; Reichenberg, A.; Schalkwyk, L.; Fernandes, C. Advanced paternal age effects in neurodevelopmental disorders—Review of potential underlying mechanisms. Transl. Psychiatry 2017, 7, e1019. [Google Scholar] [CrossRef] [Green Version]
  10. Curley, J.P.; Mashoodh, R.; Champagne, F.A. Epigenetics and the origins of paternal effects. Horm. Behav. 2011, 59, 306–314. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  11. Simard, M.; Laprise, C.; Girard, S.L. Impact of paternal age at conception on human health. Clin. Chem. 2019, 65, 146–152. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  12. Fieder, M.; Huber, S. Paternal age predicts offspring chances of marriage and reproduction. Am. J. Hum. Biol. 2015, 27, 339–343. [Google Scholar] [CrossRef] [PubMed]
  13. Arslan, R.C.; Willführ, K.P.; Frans, E.M.; Verweij, K.J.; Bürkner, P.-C.; Myrskylä, M.; Voland, E.; Almqvist, C.; Zietsch, B.P.; Penke, L. Older fathers’ children have lower evolutionary fitness across four centuries and in four populations. Proc. R. Soc. B Biol. Sci. 2017, 284, 20171562. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  14. Eisenberg, D.T.; Kuzawa, C.W. The paternal age at conception effect on offspring telomere length: Mechanistic, comparative and adaptive perspectives. Philos. Trans. R. Soc. B 2018, 373, 20160442. [Google Scholar] [CrossRef] [Green Version]
  15. Gangestad, S.W.; Thornhill, R. Facial masculinity and fluctuating asymmetry. Evol. Hum. Behav. 2003, 24, 231–241. [Google Scholar] [CrossRef]
  16. Huber, S.; Fieder, M. Advanced paternal age is associated with lower facial attractiveness. Evol. Hum. Behav. 2014, 35, 298–301. [Google Scholar] [CrossRef]
  17. Woodley of Menie, M.A.; Kanazawa, S. Paternal age negatively predicts offspring physical attractiveness in two, large, nationally representative datasets. Personal. Individ. Differ. 2017, 106, 217–221. [Google Scholar] [CrossRef]
  18. Lee, A.J.; Mitchem, D.G.; Wright, M.J.; Martin, N.G.; Keller, M.C.; Zietsch, B.P. Facial averageness and genetic quality: Testing heritability, genetic correlation with attractiveness, and the paternal age effect. Evol. Hum. Behav. 2016, 37, 61–66. [Google Scholar] [CrossRef] [Green Version]
  19. Grammer, K.; Thornhill, R. Human (Homo sapiens) facial attractiveness and sexual selection: The role of symmetry and averageness. J. Comp. Psychol. 1994, 108, 233. [Google Scholar] [CrossRef]
  20. Rhodes, G.; Yoshikawa, S.; Clark, A.; Lee, K.; McKay, R.; Akamatsu, S. Attractiveness of facial averageness and symmetry in non-Western cultures: In search of biologically based standards of beauty. Perception 2001, 30, 611–625. [Google Scholar] [CrossRef]
  21. Apicella, C.L.; Little, A.C.; Marlowe, F.W. Facial averageness and attractiveness in an isolated population of hunter-gatherers. Perception 2007, 36, 1813–1820. [Google Scholar] [CrossRef]
  22. Valentine, T.; Darling, S.; Donnelly, M. Why are average faces attractive? The effect of view and averageness on the attractiveness of female faces. Psychon. Bull. Rev. 2004, 11, 482–487. [Google Scholar] [CrossRef] [Green Version]
  23. Danel, D.; Dziedzic-Danel, A.; Kleisner, K. Does age difference really matter? Facial markers of biological quality and age difference between husband and wife. Homo 2016, 67, 337–347. [Google Scholar] [CrossRef]
  24. Rohlf, F.J. The tps series of software. Hystrix 2015, 26, 9–12. [Google Scholar] [CrossRef]
  25. Kleisner, K.; Pokorný, Š.; Saribay, S.A. Toward a new approach to cross-cultural distinctiveness and typicality of human faces: The cross-group typicality/distinctiveness metric. Front. Psychol. 2019, 10, 124. [Google Scholar] [CrossRef] [Green Version]
  26. Adams, D.C.; Otárola-Castillo, E. geomorph: An R package for the collection and analysis of geometric morphometric shape data. Methods Ecol. Evol. 2013, 4, 393–399. [Google Scholar] [CrossRef]
  27. Bookstein, F.L. Landmark methods for forms without landmarks: Morphometrics of group differences in outline shape. Med. Image Anal. 1997, 1, 225–243. [Google Scholar] [CrossRef]
  28. Bürkner, P.-C. Advanced Bayesian multilevel modeling with the R package brms. arXiv 2018, arXiv:1705.11123. [Google Scholar] [CrossRef]
  29. Barr, D.J. Random effects structure for testing interactions in linear mixed-effects models. Front. Psychol. 2013, 4, 328. [Google Scholar] [CrossRef] [Green Version]
  30. Lemoine, N.P. Moving beyond noninformative priors: Why and how to choose weakly informative priors in Bayesian analyses. Oikos 2019, 128, 912–928. [Google Scholar] [CrossRef] [Green Version]
  31. Girard-Buttoz, C.; Tkaczynski, P.J.; Samuni, L.; Fedurek, P.; Gomes, C.; Löhrich, T.; Manin, V.; Preis, A.; Valé, P.F.; Deschner, T. Early maternal loss leads to short-but not long-term effects on diurnal cortisol slopes in wild chimpanzees. eLife 2021, 10, e64134. [Google Scholar] [CrossRef]
  32. Gerhart, J.; Kirschner, M. The theory of facilitated variation. Proc. Natl. Acad. Sci. USA 2007, 104, 8582–8589. [Google Scholar] [CrossRef] [Green Version]
  33. Fang, Y.; Wang, Y.; Peng, M.; Xu, J.; Fan, Z.; Liu, C.; Zhao, K.; Zhang, H. Effect of paternal age on offspring birth defects: A systematic review and meta-analysis. Aging 2020, 12, 25373. [Google Scholar] [CrossRef]
  34. Zadzińska, E.; Kozieł, S.; Kurek, M.; Spinek, A. Mother’s trauma during pregnancy affects fluctuating asymmetry in offspring’s face. Anthropol. Anz. 2013, 70, 427–437. [Google Scholar] [CrossRef]
  35. King, S.; Mancini-Marïe, A.; Brunet, A.; Walker, E.; Meaney, M.J.; Laplante, D.P. Prenatal maternal stress from a natural disaster predicts dermatoglyphic asymmetry in humans. Dev. Psychopathol. 2009, 21, 343–353. [Google Scholar] [CrossRef] [Green Version]
  36. Hope, D.; Bates, T.; Penke, L.; Gow, A.J.; Starr, J.M.; Deary, I.J. Symmetry of the face in old age reflects childhood social status. Econ. Hum. Biol. 2013, 11, 236–244. [Google Scholar] [CrossRef] [PubMed]
  37. Özener, B.; Fink, B. Facial symmetry in young girls and boys from a slum and a control area of Ankara, Turkey. Evol. Hum. Behav. 2010, 31, 436–441. [Google Scholar] [CrossRef]
  38. Pound, N.; Lawson, D.W.; Toma, A.M.; Richmond, S.; Zhurov, A.I.; Penton-Voak, I.S. Facial fluctuating asymmetry is not associated with childhood ill-health in a large British cohort study. Proc. R. Soc. B Biol. Sci. 2014, 281, 20141639. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  39. Kuna, B.; Galbarczyk, A.; Klimek, M.; Nenko, I.; Jasienska, G. Age difference between parents influences parity and number of sons. Am. J. Hum. Biol. 2018, 30, e23095. [Google Scholar] [CrossRef] [PubMed]
  40. Jasienska, G. Reproduction and lifespan: Trade-offs, overall energy budgets, intergenerational costs, and costs neglected by research. Am. J. Hum. Biol. 2009, 21, 524–532. [Google Scholar] [CrossRef]
  41. Little, A.C.; Jones, B.C.; DeBruine, L.M. The many faces of research on face perception. Philos. Trans. R. Soc. B Biol. Sci. 2011, 366, 1634–1637. [Google Scholar] [CrossRef] [PubMed]
  42. Holzleitner, I.J.; Lee, A.J.; Hahn, A.C.; Kandrik, M.; Bovet, J.; Renoult, J.P.; Simmons, D.; Garrod, O.; DeBruine, L.M.; Jones, B.C. Comparing theory-driven and data-driven attractiveness models using images of real women’s faces. J. Exp. Psychol. Hum. Percept. Perform. 2019, 45, 1589. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  43. O’Toole, A.J.; Castillo, C.D.; Parde, C.J.; Hill, M.Q.; Chellappa, R. Face space representations in deep convolutional neural networks. Trends Cogn. Sci. 2018, 22, 794–809. [Google Scholar] [CrossRef] [PubMed]
  44. Renoult, J.P.; Bovet, J.; Raymond, M. Beauty is in the efficient coding of the beholder. R. Soc. Open Sci. 2016, 3, 160027. [Google Scholar] [CrossRef] [Green Version]
  45. Bray, I.; Gunnell, D.; Smith, G.D. Advanced paternal age: How old is too old? J. Epidemiol. Community Health 2006, 60, 851–853. [Google Scholar] [CrossRef] [Green Version]
Symmetry 14 00344 g001 550
Figure 1. The relationship between facial distinctiveness and the maternal age at birth (a) and paternal age at birth (b). The line represents the model estimate, and the shaded area represents 95% confidence intervals. Dots represent data points.
Figure 1. The relationship between facial distinctiveness and the maternal age at birth (a) and paternal age at birth (b). The line represents the model estimate, and the shaded area represents 95% confidence intervals. Dots represent data points.
Symmetry 14 00344 g001
Symmetry 14 00344 g002 550
Figure 2. The relationship between facial asymmetry and maternal age at birth (a) and paternal age at birth (b). The line represents the model estimate, and the shaded area represents 95% confidence intervals. Dots represent data points.
Figure 2. The relationship between facial asymmetry and maternal age at birth (a) and paternal age at birth (b). The line represents the model estimate, and the shaded area represents 95% confidence intervals. Dots represent data points.
Symmetry 14 00344 g002
Table
Table 1. Mean, standard deviation, maximum and minimum of the variables included in the study (facial distinctiveness, facial asymmetry, age and birth order are reported for the offspring).
Table 1. Mean, standard deviation, maximum and minimum of the variables included in the study (facial distinctiveness, facial asymmetry, age and birth order are reported for the offspring).
MeanSDMaxMin
Facial distinctiveness0.060.010.130.03
Facial asymmetry0.060.020.160.03
Age11.664.2321.563.01
Birth order2.411.1861
Maternal age at birth (years)26.845.1944.1016.32
Paternal age at birth (years)29.745.9054.2518.94
Table
Table 2. The relationship between facial distinctiveness and the investigated independent variables (n = 159).
Table 2. The relationship between facial distinctiveness and the investigated independent variables (n = 159).
EstimateSE95% CI Low95% CI High
Intercept−0.260.12−0.49−0.04
Sex (reference: male)0.450.160.130.76
Age−0.270.11−0.48−0.06
Birth order−0.260.13−0.51−0.01
Maternal age at birth (years)0.360.140.100.64
Paternal age at birth (years)−0.180.14−0.440.09
Table
Table 3. The relationship between facial asymmetry and the investigated independent variables (n = 159).
Table 3. The relationship between facial asymmetry and the investigated independent variables (n = 159).
EstimateSE95% CI Low95% CI High
Intercept−0.080.12−0.320.15
Sex (reference: male)0.110.17−0.220.44
Age−0.260.11−0.47−0.06
Birth order−0.290.13−0.56−0.02
Maternal age at birth (years)0.200.14−0.070.48
Paternal age at birth (years)−0.100.14−0.360.17
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Klimek, M.; Marcinkowska, U.M.; Fedurek, P.; Kleisner, K.; Danel, D.P. Like Father, Like Child? Paternal Age at Birth and Offspring’s Facial Asymmetry and Distinctiveness. Symmetry 2022, 14, 344. https://doi.org/10.3390/sym14020344

AMA Style

Klimek M, Marcinkowska UM, Fedurek P, Kleisner K, Danel DP. Like Father, Like Child? Paternal Age at Birth and Offspring’s Facial Asymmetry and Distinctiveness. Symmetry. 2022; 14(2):344. https://doi.org/10.3390/sym14020344

Chicago/Turabian Style

Klimek, Magdalena, Urszula M. Marcinkowska, Pawel Fedurek, Karel Kleisner, and Dariusz P. Danel. 2022. "Like Father, Like Child? Paternal Age at Birth and Offspring’s Facial Asymmetry and Distinctiveness" Symmetry 14, no. 2: 344. https://doi.org/10.3390/sym14020344

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop