An Efficient Analytical Method for Analyzing the Nonlinear Fractional Klein–Fock–Gordon Equations
Abstract
1. Introduction
2. Preliminaries
3. Main Work
4. Algorithm of the Suggested Technique
4.1. Implementation to Caputo–Fabrizio Fractional Differential Equations
4.2. Convergence and Error Analysis
5. Applications
Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liouville, J. Memoire surquelques questions de geometrieet de mecanique, etsurun nouveau genre de calcul pour resoudreces questions. J. Ec. Polytech. 1832, 13, 1–69. [Google Scholar]
- Riemann, G.F.B. Versucheinerallgemeinen Auffassung der Integration und Differentiation. In Gesammelte Mathematische Werke; Springer: Leipzig, Germany, 1896. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Liu, K.; Yang, Z.; Wei, W.; Gao, B.; Xin, D.; Sun, C.; Wu, G. Novel detection approach for thermal defects: Study on its feasibility and application to vehicle cables. High Voltage 2022, 1–10. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, K.; Li, J.; Guo, Y.; Zhang, A.; Chen, Q. Millimeter-Wave E-Plane Waveguide Bandpass Filters Based on Spoof Surface Plasmon Polaritons. IEEE Trans. Microw. Theory Tech. 2022, 70, 4399–4409. [Google Scholar] [CrossRef]
- Xi, Y.; Jiang, W.; Wei, K.; Hong, T.; Cheng, T.; Gong, S. Wideband RCS Reduction of Microstrip Antenna Array Using Coding Metasurface with Low Q Resonators and Fast Optimization Method. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 656–660. [Google Scholar] [CrossRef]
- Baleanu, D.; Guvenc, Z.B.; Machado, J.A.T. New Trends in Nanotechnology and Fractional Calculus Applications; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Baleanu, D.; Wu, G.-C.; Zeng, S.-D. Chaos analysis and asymptotic stability of generalized Caputo fractional differential equations. Chaos Solitons Fractals 2017, 102, 99–105. [Google Scholar] [CrossRef]
- Sweilam, N.H.; Hasan, M.M.A.; Baleanu, D. New studies for general fractional financial models of awareness and trial advertising decisions. Chaos Solitons Fractals 2017, 104, 772–784. [Google Scholar] [CrossRef]
- Esen, A.; Sulaiman, T.A.; Bulut, H.; Baskonus, H.M. Optical solitons and other solutions to the conformable space-time fractional Fokas-Lenells equation. Optik 2018, 167, 150–156. [Google Scholar] [CrossRef]
- Veeresha, P.; Prakasha, D.G. Solution for fractional Zakharov-Kuznetsov equations by using two reliable techniques. Chin. J. Phys. 2019, 60, 313–330. [Google Scholar] [CrossRef]
- Prakasha, D.G.; Veeresha, P.; Baskonus, H.M. Analysis of the dynamics of hepatitis E virus using the Atangana-Baleanu fractional derivative. Eur. Phys. J. Plus 2019, 134, 241. [Google Scholar] [CrossRef]
- Prakash, A.; Veeresha, P.; Prakasha, D.G.; Goyal, M. A new efficient technique for solving fractional coupled Navier-Stokes equations using q-homotopy analysis transform method. Pramana-J. Phys. 2019, 93, 6. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Ullah, R.; Khan, A.; Shah, R.; Kafle, J.; Mahariq, I.; Jarad, F. Numerical analysis of the fractional-order nonlinear system of Volterra integro-differential equations. J. Funct. Spaces 2021, 2021, 1537958. [Google Scholar] [CrossRef]
- Al-Habahbeh, A. Exact solution for commensurate and incommensurate linear systems of fractional differential equations. J. Math. Comput. Sci. 2023, 28, 123–136. [Google Scholar] [CrossRef]
- Wang, Y.; Han, X.; Jin, S. MAP based modeling method and performance study of a task offloading scheme with time-correlated traffic and VM repair in MEC systems. Wirel. Netw. 2022, 1–22. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, L.; Pan, G.; Zhang, S. Robust yaw control of autonomous underwater vehicle based on fractional-order PID controller. Ocean Eng. 2022, 257, 111493. [Google Scholar] [CrossRef]
- Alharthi, M.R.; Alharbey, R.A.; El-Tantawy, S.A. Novel analytical approximations to the nonplanar Kawahara equation and its plasma applications. Eur. Phys. J. Plus 2022, 137, 1172. [Google Scholar] [CrossRef]
- El-Tantawy, S.A.; Salas, A.H.; Alyousef, H.A.; Alharthi, M.R. Novel approximations to a nonplanar nonlinear Schrodinger equation and modeling nonplanar rogue waves/breathers in a complex plasma. Chaos Solitons Fractals 2022, 163, 112612. [Google Scholar] [CrossRef]
- Aljahdaly, N.H.; El-Tantawy, S.A. Novel anlytical solution to the damped Kawahara equation and its application for modeling the dissipative nonlinear structures in a fluid medium. J. Ocean Eng. Sci. 2022, 7, 492. [Google Scholar] [CrossRef]
- Shohaib, M.; Masood, W.; Alyousef, H.A.; Siddiq, M.; El-Tantawy, S.A. Formation and interaction of multi-dimensional electrostatic ion-acoustic solitons in two-electron temperature plasmas. Phys. Fluids 2022, 34, 093107. [Google Scholar] [CrossRef]
- Alyousef, H.A.; Salas, A.H.; Matoog, R.T.; El-Tantawy, S.A. On the analytical and numerical approximations to the forced damped Gardner Kawahara equation and modeling the nonlinear structures in a collisional plasma. Phys. Fluids 2022, 34, 103105. [Google Scholar] [CrossRef]
- Douanla, D.V.; Tiofack, C.G.L.; Alim; Aboubakar, M.; Mohamadou, A.; Albalawi, W.; El-Tantawy, S.A.; El-Sherif, L.S. Three-dimensional rogue waves and dust-acoustic dark soliton collisions in degenerate ultradense magnetoplasma in the presence of dust pressure anisotropy. Phys. Fluids 2022, 34, 087105. [Google Scholar] [CrossRef]
- Chaurasiya, V.; Wakif, A.; Shah, N.A.; Singh, J. A study on cylindrical moving boundary problem with variable thermal conductivity and convection under the most realistic boundary conditions. Int. Commun. Heat Mass Transf. 2022, 138, 106312. [Google Scholar] [CrossRef]
- Chaurasiya, V.; Upadhyay, S.; Rai, K.N.; Singh, J. A new look in heat balance integral method to a two-dimensional Stefan problem with convection. Numer. Heat Transf. Part A Appl. 2022, 82, 529–542. [Google Scholar] [CrossRef]
- Zheng, H.; Jin, S. A Multi-Source Fluid Queue Based Stochastic Model of the Probabilistic Offloading Strategy in a MEC System with Multiple Mobile Devices and a Single MEC Server. Int. J. Appl. Math. Comput. Sci. 2022, 32, 125–138. [Google Scholar] [CrossRef]
- Lu, S.; Ban, Y.; Zhang, X.; Yang, B.; Liu, S.; Yin, L.; Zheng, W. Adaptive control of time delay teleoperation system with uncertain dynamics. Front. Neurorobot. 2022, 16, 928863. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Tian, J.; Zhang, X.; Yang, B.; Liu, S.; Yin, L.; Zheng, W. Control of Time Delay Force Feedback Teleoperation System with Finite Time Convergence. Front. Neurorobot. 2022, 16, 877069. [Google Scholar] [CrossRef]
- Alaoui, M.K.; Fayyaz, R.; Khan, A.; Shah, R.; Abdo, M.S. Analytical investigation of Noyes-Field model for time-fractional Belousov-Zhabotinsky reaction. Complexity 2021, 2021, 3248376. [Google Scholar] [CrossRef]
- Zidan, A.M.; Khan, A.; Shah, R.; Alaoui, M.K.; Weera, W. Evaluation of time-fractional Fisher’s equations with the help of analytical methods. AIMS Math. 2022, 7, 18746–18766. [Google Scholar] [CrossRef]
- Yel, G.; Baskonus, H.M.; Bulut, H. Novel archetypes of new coupled Konno-Oono equation by using sine-Gordon expansion method. Opt. Quantum Electron. 2017, 49, 285. [Google Scholar] [CrossRef]
- Wu, G.C. A fractional variational iteration method for solving fractional nonlinear differential equations. Comput. Math. Appl. 2011, 61, 2186–2190. [Google Scholar] [CrossRef]
- Singh, J.; Kumar, D.; Al Qurashi, M.; Baleanu, D. A novel numerical approach for a nonlinear fractional dynamical model of interpersonal and romantic relationships. Entropy 2017, 19, 375. [Google Scholar] [CrossRef]
- Alaoui, M.K.; Nonlaopon, K.; Zidan, A.M.; Khan, A.; Shah, R. Analytical investigation of fractional-order cahn-hilliard and gardner equations using two novel techniques. Mathematics 2022, 10, 1643. [Google Scholar] [CrossRef]
- Shah, N.A.; Hamed, Y.S.; Abualnaja, K.M.; Chung, J.D.; Shah, R.; Khan, A. A comparative analysis of fractional-order kaup-kupershmidt equation within different operators. Symmetry 2022, 14, 986. [Google Scholar] [CrossRef]
- Gepreel, K.A.; Mahdy, A.M.S.; Mohamed, M.S.; Al-Amiri, A. Reduced differential transform method for solving nonlinear biomathematics models. Comput. Mater. Contin. 2019, 61, 979–994. [Google Scholar]
- Singh, J.; Kumar, D.; Sushila, D. Homotopy perturbation Sumudu transform method for nonlinear equations. Adv. Theor. Appl. Mech. 2011, 4, 165–175. [Google Scholar]
- Shah, N.A.; El-Zahar, E.R.; Akgül, A.; Khan, A.; Kafle, J. Analysis of Fractional-Order Regularized Long-Wave Models via a Novel Transform. J. Funct. Spaces 2022, 2022, 2754507. [Google Scholar] [CrossRef]
- Areshi, M.; Khan, A.; Shah, R.; Nonlaopon, K. Analytical investigation of fractional-order Newell-Whitehead-Segel equations via a novel transform. AIMS Math. 2022, 7, 6936–6958. [Google Scholar] [CrossRef]
- Alderremy, A.A.; Aly, S.; Fayyaz, R.; Khan, A.; Shah, R.; Wyal, N. The analysis of fractional-order nonlinear systems of third order KdV and Burgers equations via a novel transform. Complexity 2022, 2022, 4935809. [Google Scholar] [CrossRef]
- Kaur, B.; Gupta, R.K. Dispersion analysis and improved F-expansion method for space-time fractional differential equations. Nonlinear Dyn. 2019, 96, 837–852. [Google Scholar] [CrossRef]
- Al-Sawalha, M.M.; Khan, A.; Ababneh, O.Y.; Botmart, T. Fractional view analysis of Kersten-Krasil’shchik coupled KdV-mKdV systems with non-singular kernel derivatives. AIMS Math. 2022, 7, 18334–18359. [Google Scholar] [CrossRef]
- Nonlaopon, K.; Alsharif, A.M.; Zidan, A.M.; Khan, A.; Hamed, Y.S.; Shah, R. Numerical investigation of fractional-order Swift-Hohenberg equations via a Novel transform. Symmetry 2021, 13, 1263. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Alyousef, H.A.; El-Tantawy, S.A.; Khan, A.; Wyal, N. Solving Fractional-Order Diffusion Equations in a Plasma and Fluids via a Novel Transform. J. Funct. Spaces 2022, 2022, 1899130. [Google Scholar] [CrossRef]
- Alshehry, A.S.; Imran, M.; Khan, A.; Shah, R.; Weera, W. Fractional View Analysis of Kuramoto-Sivashinsky Equations with Non-Singular Kernel Operators. Symmetry 2022, 14, 1463. [Google Scholar] [CrossRef]
- Nikan, O.; Avazzadeh, Z.; Machado, J.T. An efficient local meshless approach for solving nonlinear time-fractional fourth-order diffusion model. J. King Saud Univ.-Sci. 2021, 33, 101243. [Google Scholar] [CrossRef]
- Jassim, H.K.; Shareef, M.A. On approximate solutions for fractional system of differential equations with Caputo–Fabrizio fractional operator. J. Math. Comput. Sci. 2021, 23, 58–66. [Google Scholar] [CrossRef]
- Khan, N.A.; Rasheed, S. Analytical solutions of linear and nonlinear Klein–Fock–Gordon equation. Nonlinear Eng.-Model. Appl. 2015, 4, 43–48. [Google Scholar] [CrossRef]
- Yusufoglu, E. The variational iteration method for studying the Klein-Gordon equation. Appl. Math. Lett. 2008, 21, 669–674. [Google Scholar] [CrossRef]
- Aruna, K.; Ravi Kanth, A.S.V. Two-dimensional differential transform method and modifed differential transform method for solving nonlinear fractional Klein-Gordon equation. Nat. Acad. Sci. Lett. 2014, 37, 163–171. [Google Scholar] [CrossRef]
- Ravi Kanth, A.S.V.; Aruna, K. Differential transform method for solving the linear and nonlinear Klein-Gordon equation. Comput. Phys. Commun. 2009, 180, 708–711. [Google Scholar] [CrossRef]
- Veeresha, P.; Prakasha, D.G.; Kumar, D. An effcient technique for nonlinear time-fractional Klein–Fock–Gordon equation. Appl. Math. Comput. 2020, 364, 124637. [Google Scholar] [CrossRef]
- Kumar, D.; Singh, J.; Kumar, S. Numerical computation of Klein-Gordon equations arising in quantum feld theory by using homotopy analysis transform method. Alex. Eng. J. 2014, 53, 469–474. [Google Scholar] [CrossRef]
- Rehman, H.U.; Iqbal, I.; Subhi Aiadi, S.; Mlaiki, N.; Saleem, M.S. Soliton Solutions of Klein–Fock–Gordon Equation Using Sardar Subequation Method. Mathematics 2022, 10, 3377. [Google Scholar] [CrossRef]
- Alquran, M.; Yousef, F.; Alquran, F.; Sulaiman, T.A.; Yusuf, A. Dual-wave solutions for the quadratic-cubic conformable-Caputo time-fractional Klein–Fock–Gordon equation. Math. Comput. Simul. 2021, 185, 62–76. [Google Scholar] [CrossRef]
- He, J.H. Homotopy perturbation technique. Comput. Methods Appl. Mech. Eng. 1999, 178, 257–262. [Google Scholar] [CrossRef]
- He, J.H. Application of homotopy perturbation method to nonlinear wave equations. Chaos Solitons Fractals 2005, 26, 695–700. [Google Scholar] [CrossRef]
- Das, S.; Gupta, P.K. An approximate analytical solution ofthe fractional diffusion equation with absorbent term and external force by homotopy perturbation method. Z. Naturforsch. A 2010, 65, 182–190. [Google Scholar] [CrossRef]
- Atangana, A.; Alkahtani, B.S.T. Extension of the resistance, inductance, capacitance electrical circuit to fractional derivative without singular kernel. Adv. Mech. Eng. 2015, 7, 1687814015591937. [Google Scholar] [CrossRef]
- Gómez-Aguilar, J.F.; López-López, M.G.; Alvarado-Martínez, V.M.; Reyes-Reyes, J.; Adam-Medina, M. Modeling diffusive transport with a fractional derivative without singular kernel. Phys. A Stat. Mech. Appl. 2016, 447, 467–481. [Google Scholar] [CrossRef]
- Ahmad, S.; Ullah, A.; Akgül, A.; De la Sen, M. A novel homotopy perturbation method with applications to nonlinear fractional order KdV and Burger equation with exponential-decay kernel. J. Funct. Spaces 2021, 2021, 8770488. [Google Scholar] [CrossRef]
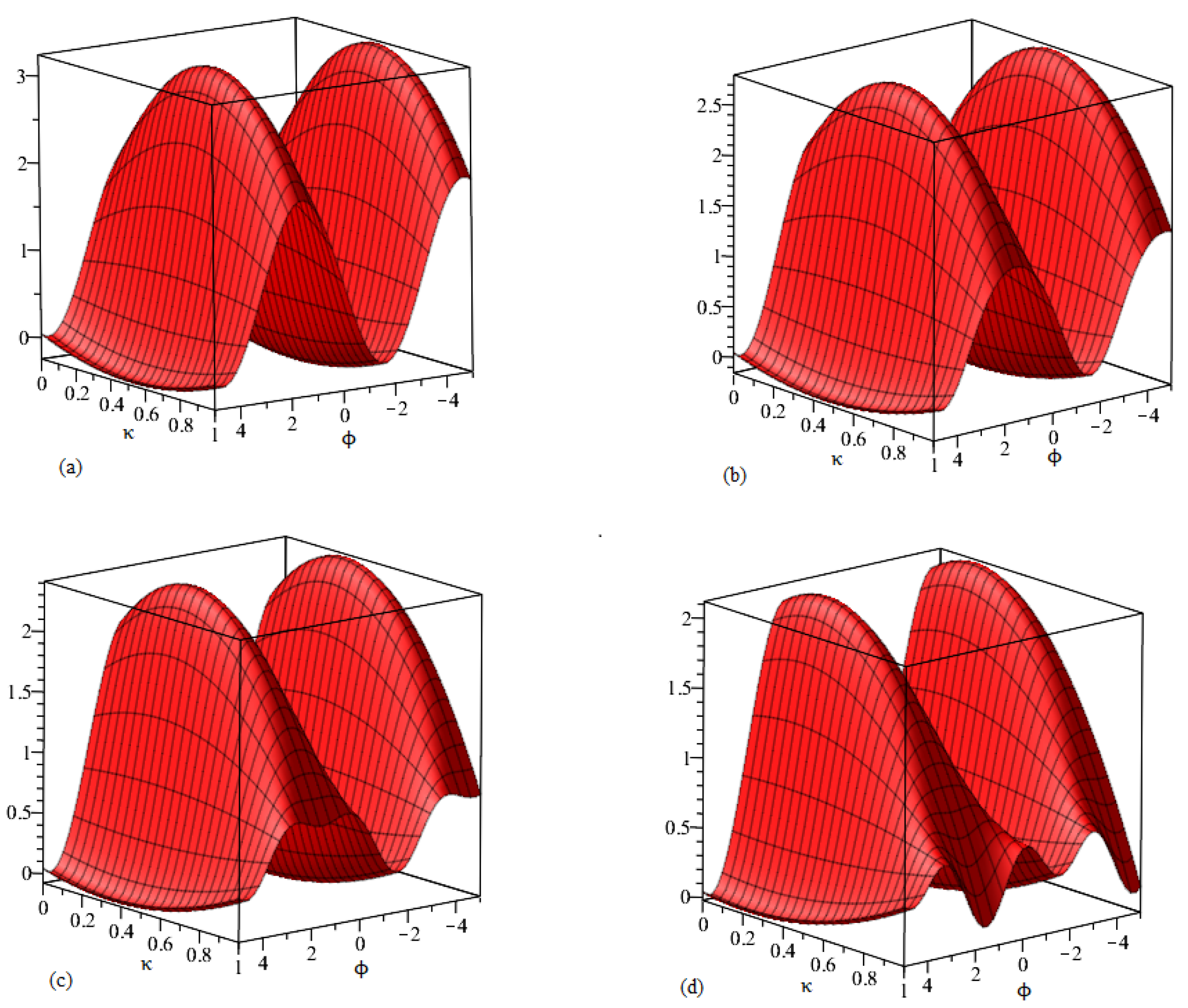
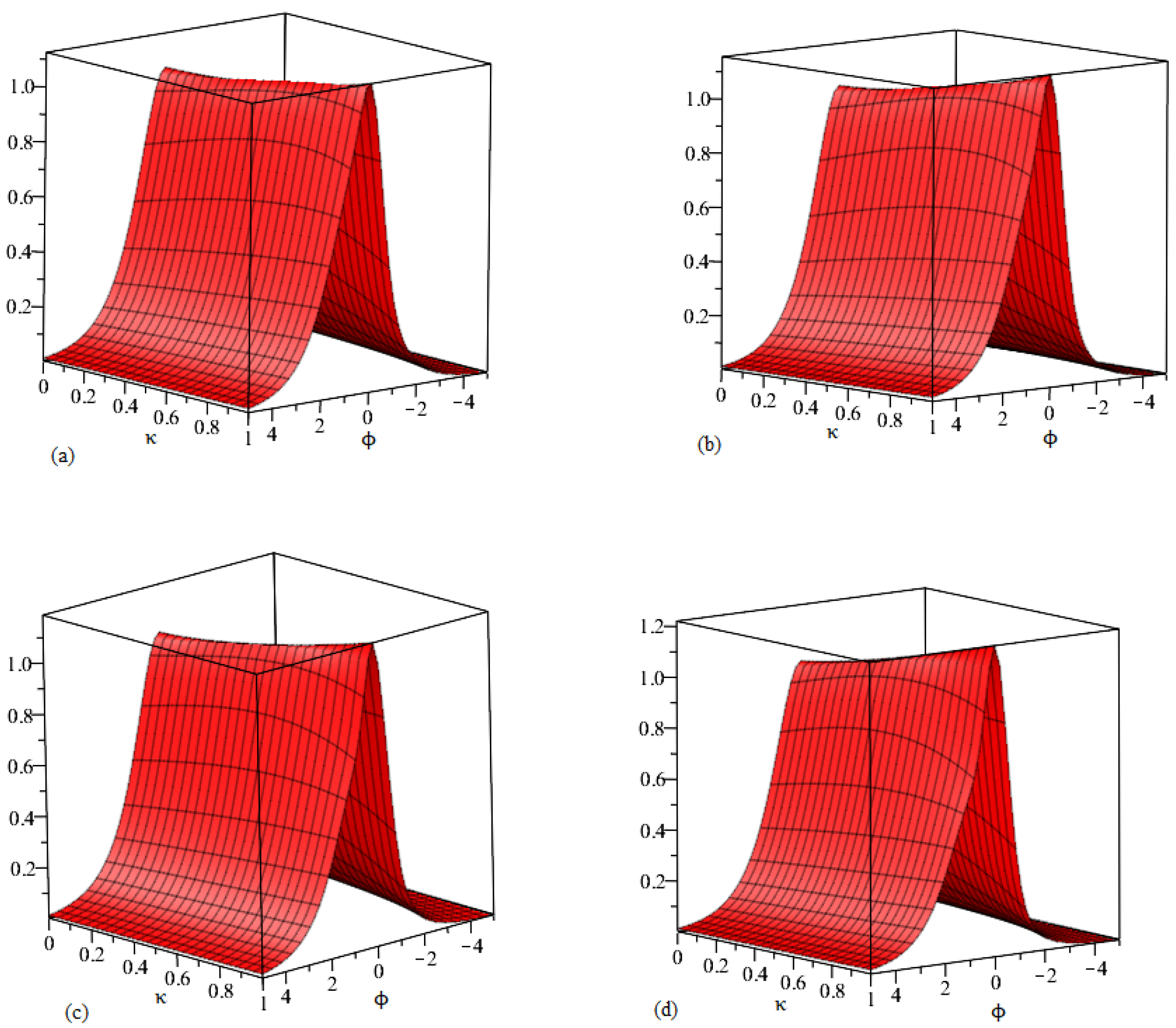
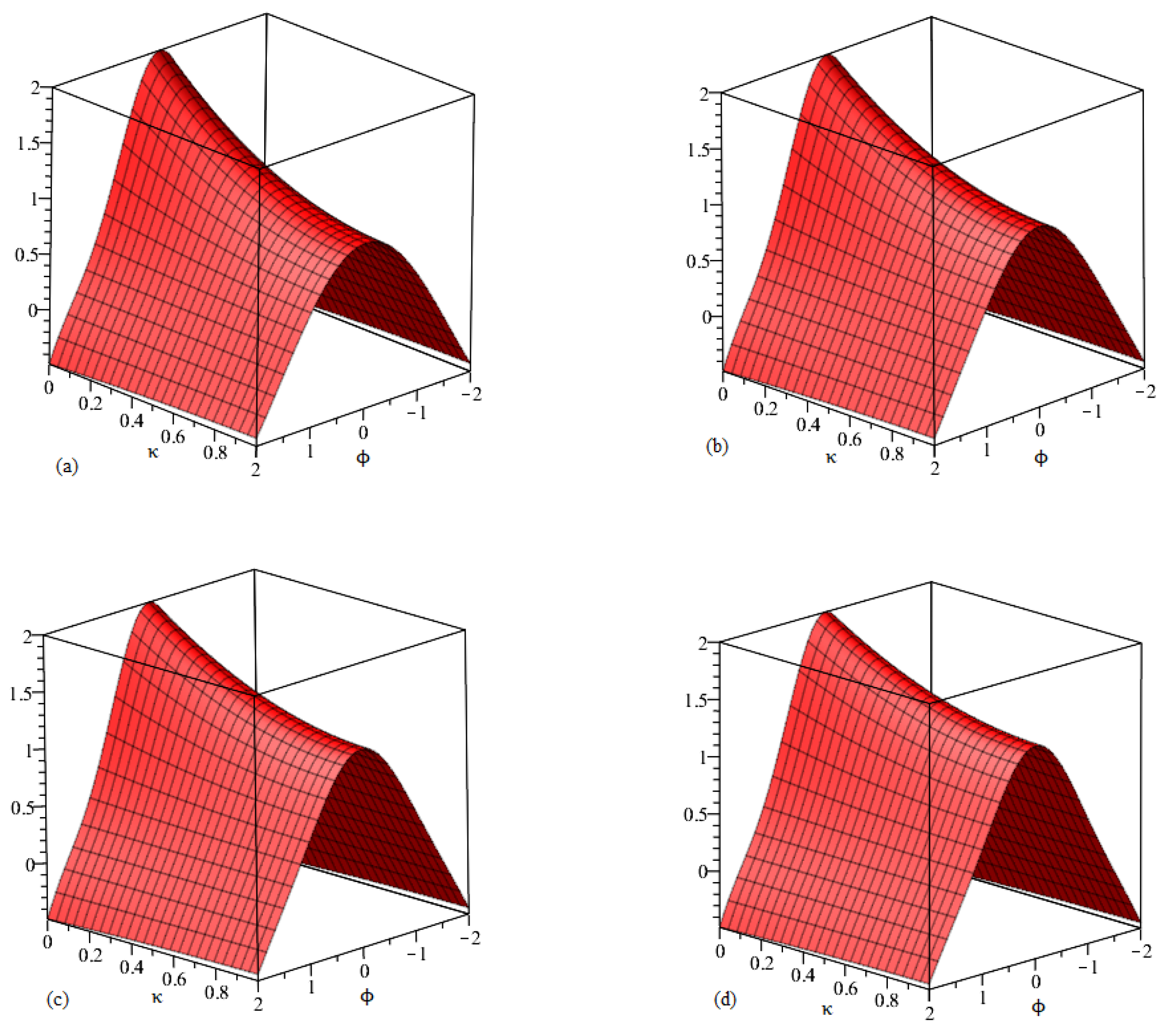
| 1 | 0.25 | 2.17213 | 2.40359 | 2.63506 |
| 2 | 2.26513 | 2.51422 | 2.76330 | |
| 3 | 1.25387 | 1.33280 | 1.41173 | |
| 4 | 0.18869 | 0.15053 | 0.11238 | |
| 5 | −0.03370 | −0.08605 | −0.13840 | |
| 1 | 0.50 | 2.10600 | 2.50279 | 2.89959 |
| 2 | 2.19396 | 2.62097 | 3.04797 | |
| 3 | 1.23132 | 1.36663 | 1.50193 | |
| 4 | 0.19959 | 0.13418 | 0.06878 | |
| 5 | −0.01875 | −0.10849 | −0.19823 | |
| 1 | 0.75 | 1.64307 | 2.13906 | 2.63506 |
| 2 | 1.69579 | 2.22955 | 2.76330 | |
| 3 | 1.07346 | 1.24260 | 1.41173 | |
| 4 | 0.27590 | 0.19414 | 0.11238 | |
| 5 | 0.08594 | −0.02623 | −0.13840 | |
| 1 | 1 | 0.78334 | 1.31241 | 1.84147 |
| 2 | 0.77061 | 1.33995 | 1.90929 | |
| 3 | 0.78030 | 0.96071 | 1.14112 | |
| 4 | 0.41761 | 0.33040 | 0.24319 | |
| 5 | 0.28038 | 0.16073 | 0.04107 |
| 1 | 0.25 | 0.70409 | 0.70644 | 0.70786 |
| 2 | 0.29278 | 0.29488 | 0.29615 | |
| 3 | 0.10966 | 0.11050 | 0.11101 | |
| 4 | 0.04044 | 0.04075 | 0.04094 | |
| 5 | 0.01488 | 0.01499 | 0.01506 | |
| 1 | 0.50 | 0.74101 | 0.75029 | 0.75714 |
| 2 | 0.31593 | 0.32018 | 0.32331 | |
| 3 | 0.11904 | 0.12056 | 0.12168 | |
| 4 | 0.04393 | 0.04449 | 0.04490 | |
| 5 | 0.01617 | 0.01637 | 0.01652 | |
| 1 | 0.75 | 0.74194 | 0.76299 | 0.78064 |
| 2 | 0.33602 | 0.34199 | 0.34700 | |
| 3 | 0.12849 | 0.13031 | 0.13184 | |
| 4 | 0.04753 | 0.04817 | 0.04872 | |
| 5 | 0.01749 | 0.01773 | 0.01793 | |
| 1 | 1 | 0.68815 | 0.72400 | 0.75745 |
| 2 | 0.35248 | 0.35967 | 0.36637 | |
| 3 | 0.13849 | 0.14027 | 0.14193 | |
| 4 | 0.05142 | 0.05203 | 0.05259 | |
| 5 | 0.01894 | 0.01916 | 0.01936 |
| 1 | 0.25 | 0.64712 | 0.63908 | 0.63105 |
| 2 | −0.46521 | −0.46173 | −0.45824 | |
| 3 | −1.64344 | −1.59146 | −1.53948 | |
| 4 | −0.84229 | −0.82774 | −0.81318 | |
| 5 | 0.29944 | 0.29850 | 0.29756 | |
| 1 | 0.50 | 0.60998 | 0.59620 | 0.58243 |
| 2 | −0.44819 | −0.44222 | −0.43624 | |
| 3 | −1.41572 | −1.32661 | −1.23751 | |
| 4 | −0.77664 | −0.75168 | −0.72672 | |
| 5 | 0.29400 | 0.29239 | 0.29078 | |
| 1 | 0.75 | 0.58662 | 0.56940 | 0.55218 |
| 2 | −0.43715 | −0.42968 | −0.42221 | |
| 3 | −1.27711 | −1.16573 | −1.05434 | |
| 4 | −0.73593 | −0.70474 | −0.67354 | |
| 5 | 0.29017 | 0.28816 | 0.28615 | |
| 1 | 1 | 0.57703 | 0.55866 | 0.54030 |
| 2 | −0.43208 | −0.42411 | −0.41614 | |
| 3 | −1.22761 | −1.10880 | −0.98999 | |
| 4 | −0.72019 | −0.68691 | −0.65364 | |
| 5 | 0.28795 | 0.28580 | 0.28366 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alyousef, H.A.; Shah, R.; Nonlaopon, K.; El-Sherif, L.S.; El-Tantawy, S.A. An Efficient Analytical Method for Analyzing the Nonlinear Fractional Klein–Fock–Gordon Equations. Symmetry 2022, 14, 2640. https://doi.org/10.3390/sym14122640
Alyousef HA, Shah R, Nonlaopon K, El-Sherif LS, El-Tantawy SA. An Efficient Analytical Method for Analyzing the Nonlinear Fractional Klein–Fock–Gordon Equations. Symmetry. 2022; 14(12):2640. https://doi.org/10.3390/sym14122640
Chicago/Turabian StyleAlyousef, Haifa A., Rasool Shah, Kamsing Nonlaopon, Lamiaa S. El-Sherif, and Samir A. El-Tantawy. 2022. "An Efficient Analytical Method for Analyzing the Nonlinear Fractional Klein–Fock–Gordon Equations" Symmetry 14, no. 12: 2640. https://doi.org/10.3390/sym14122640
APA StyleAlyousef, H. A., Shah, R., Nonlaopon, K., El-Sherif, L. S., & El-Tantawy, S. A. (2022). An Efficient Analytical Method for Analyzing the Nonlinear Fractional Klein–Fock–Gordon Equations. Symmetry, 14(12), 2640. https://doi.org/10.3390/sym14122640








