Some New Optical Solitons of the Generalized Radhakrishnan–Kundu–Lakshmanan Equations with Powers of Nonlinearity
Abstract
:1. Introduction
2. Mathematical Analysis of Equation (2)
3. Analytical Soliton Solutions for Equation (2) by the Auxiliary Equation
- Case 1:
- Case 2:
- If , we have the following solutions of Equation (11):
- Case 3:
- If , we have the following solutions of Equation (11):
- Case 4:
- If , we have the following solutions of Equation (11):
- Case 5:
- If , we have the following solutions of Equation (11):
- Case 6:
- Case 7:
- Case 8:
- If , we have the following solutions of Equation (11):
- Case 1:
- Case 2:
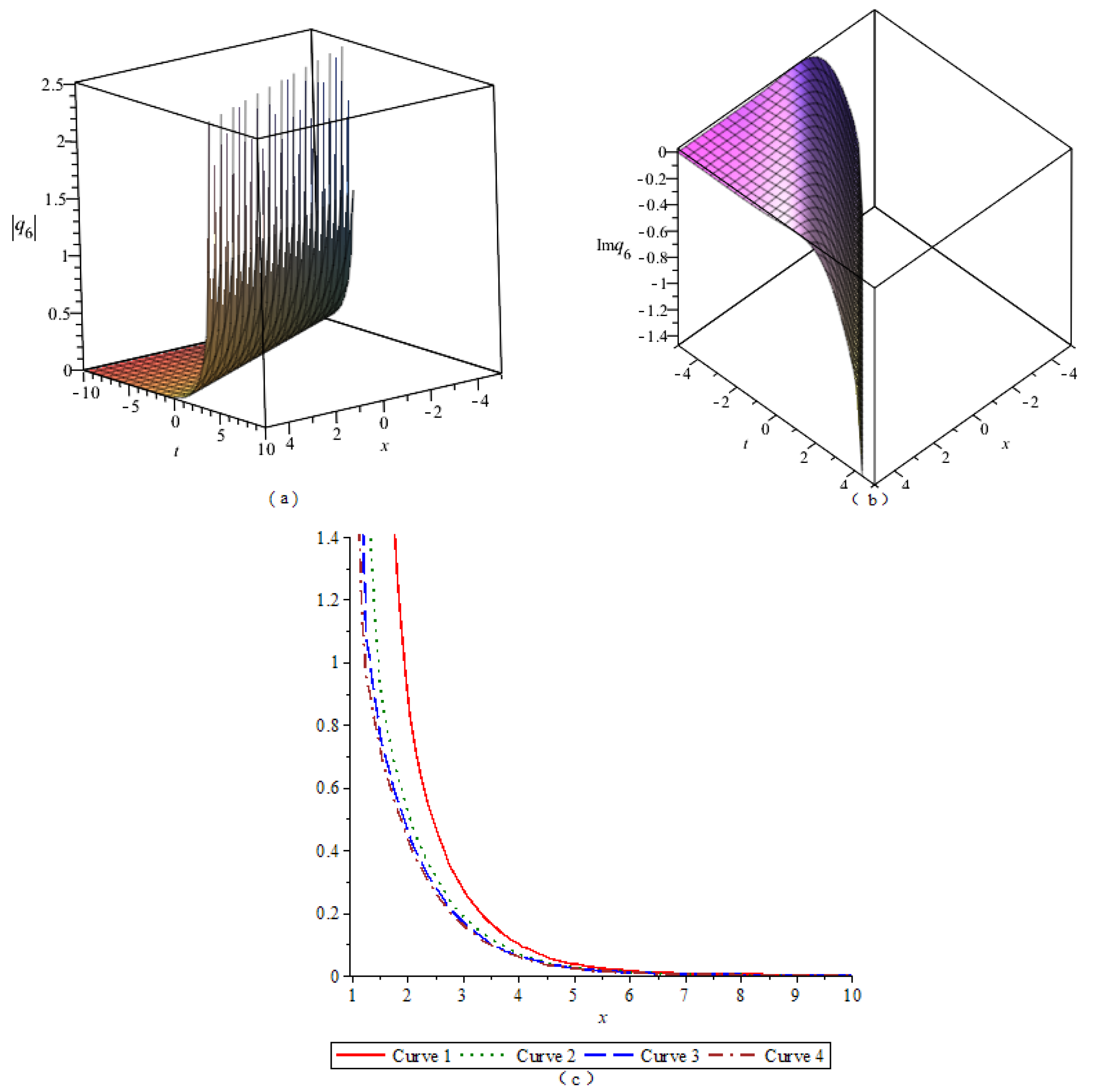
- If , the solutions of Equation (2) can be obtained as below:where This solution is a solitary-type solution.
- Case 3:
- If , the solutions of Equation (2) can be obtained as below:where This solution is a singular-soliton-type solution.
- Case 4:
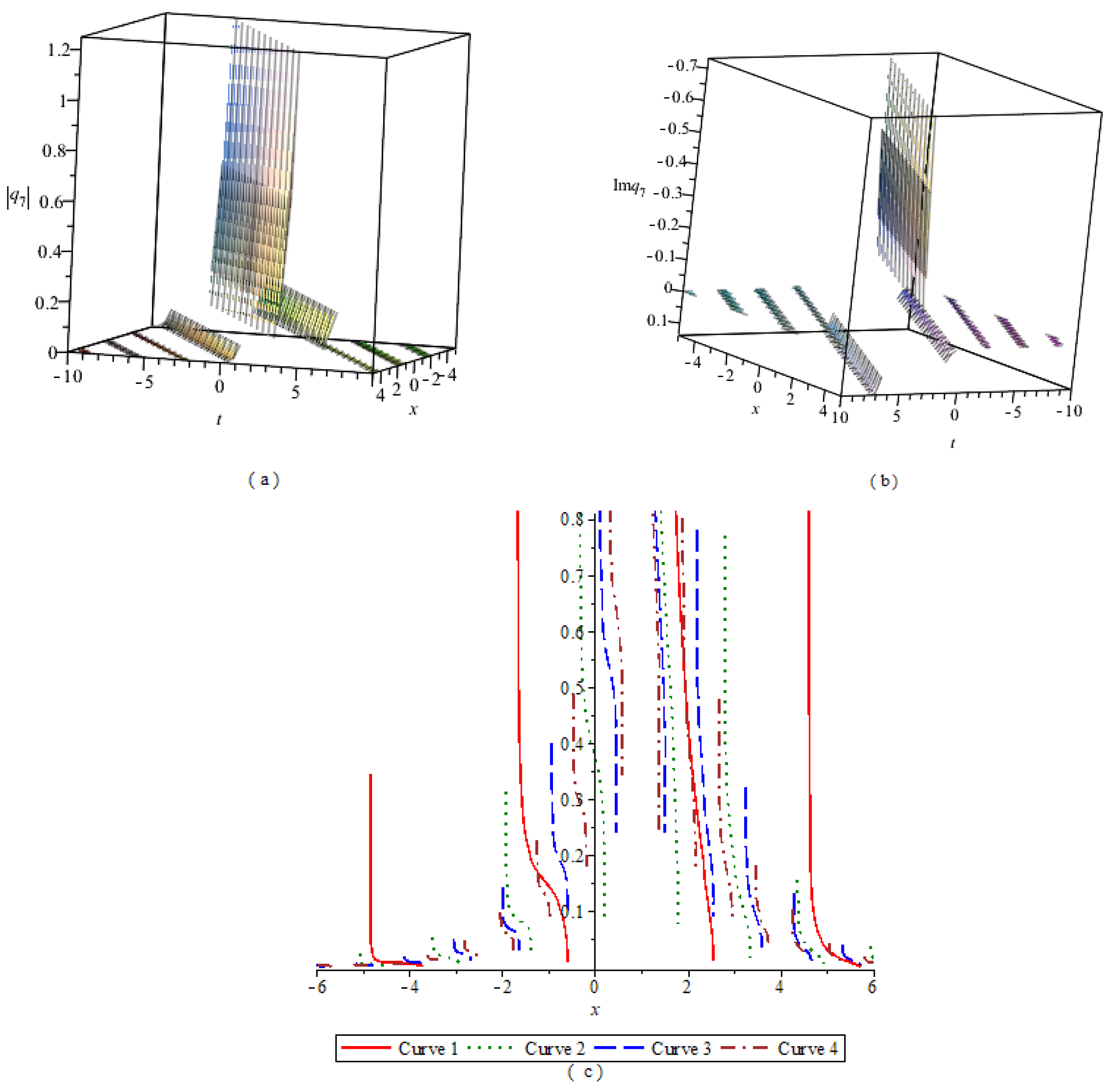
- If , the solutions of Equation (2) can be obtained as below:where This solution is a periodic-soliton-type solution.
- Case 5:
- If , the solutions of Equation (2) can be obtained as below:where This solution is a singular-soliton-type solution.
- Case 6:
- Case 7:
- Case 8:
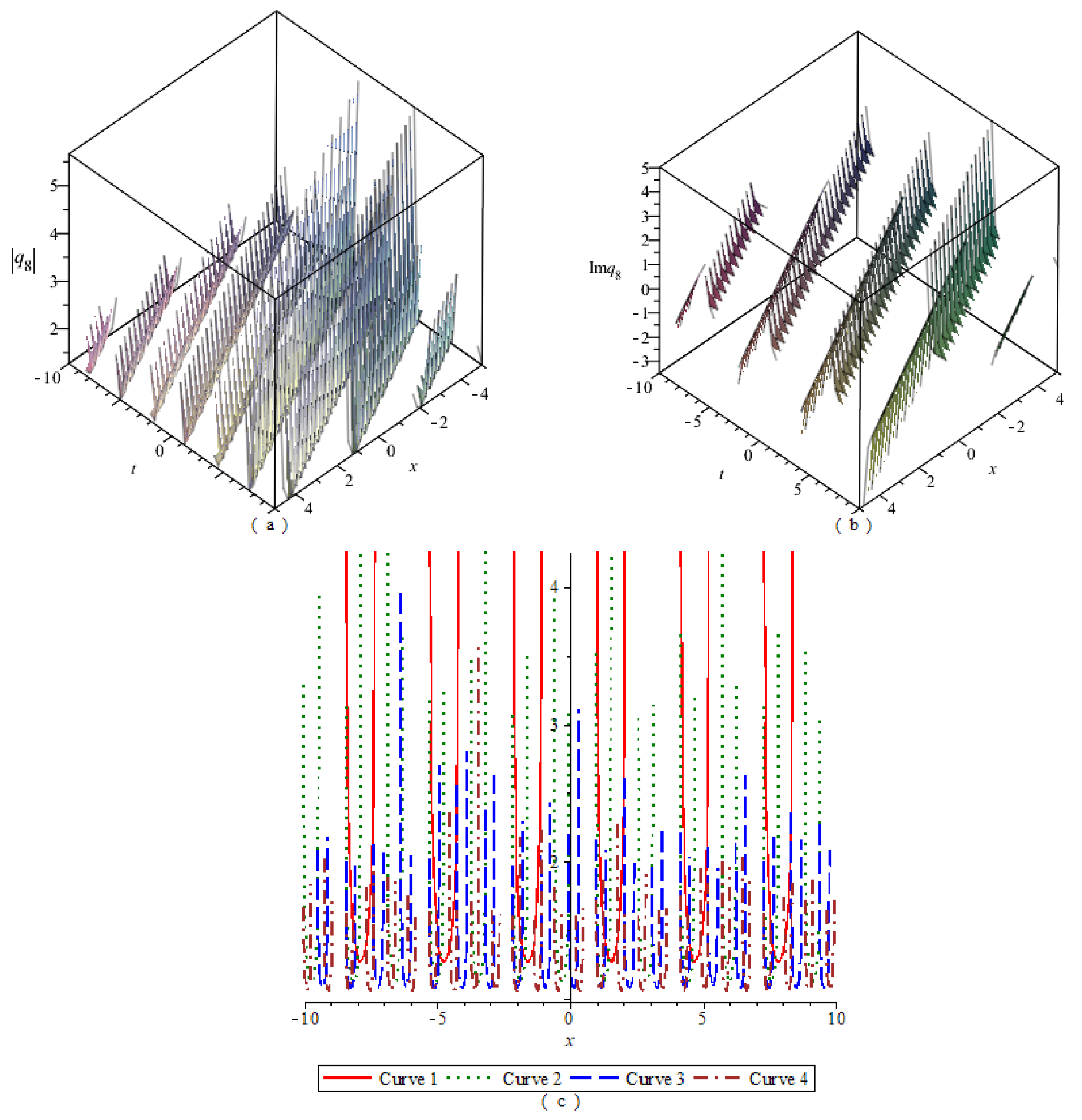
- If , the solutions of Equation (2) can be obtained as below:where This solution is an exponential-type soliton solution.
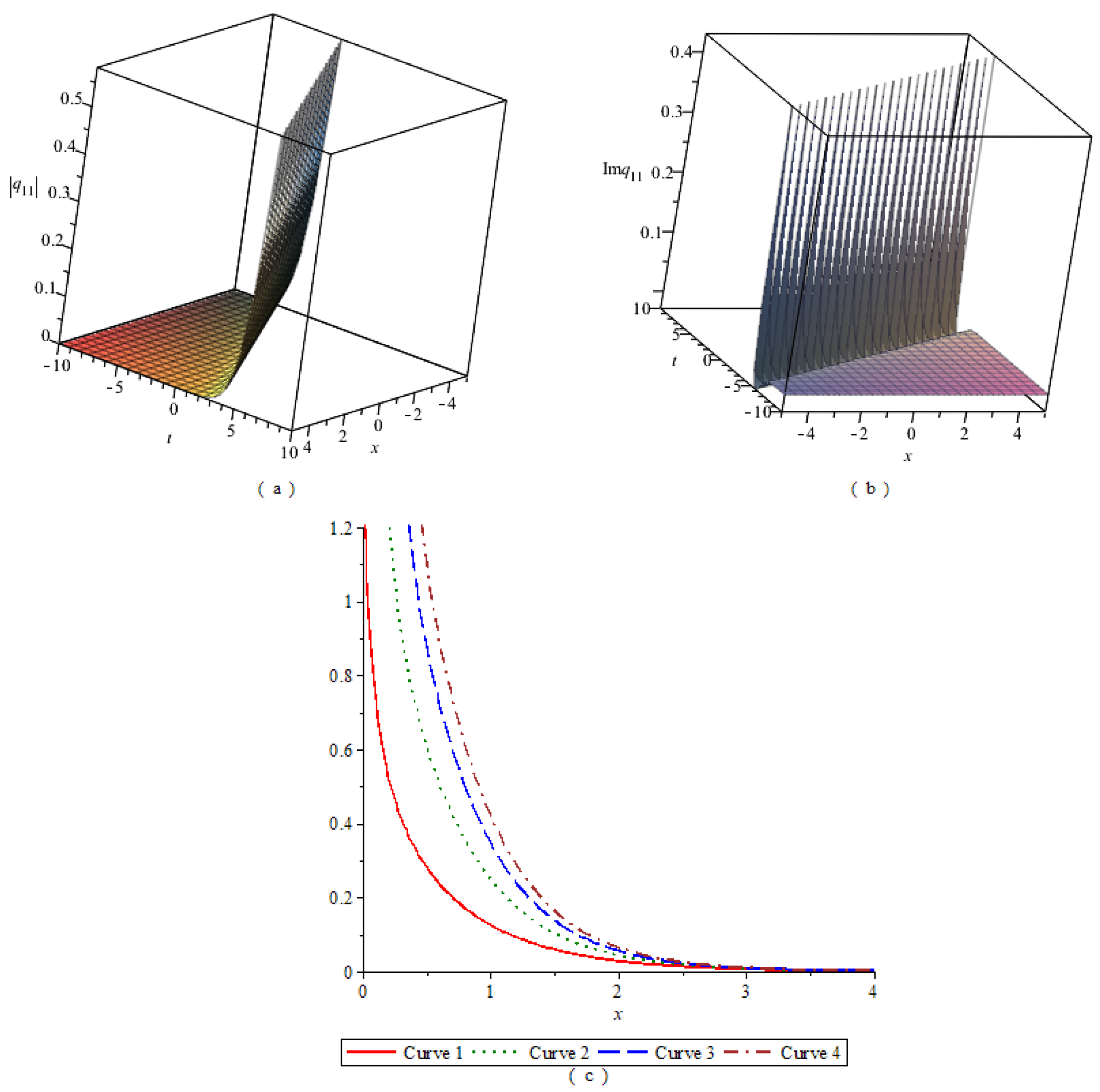
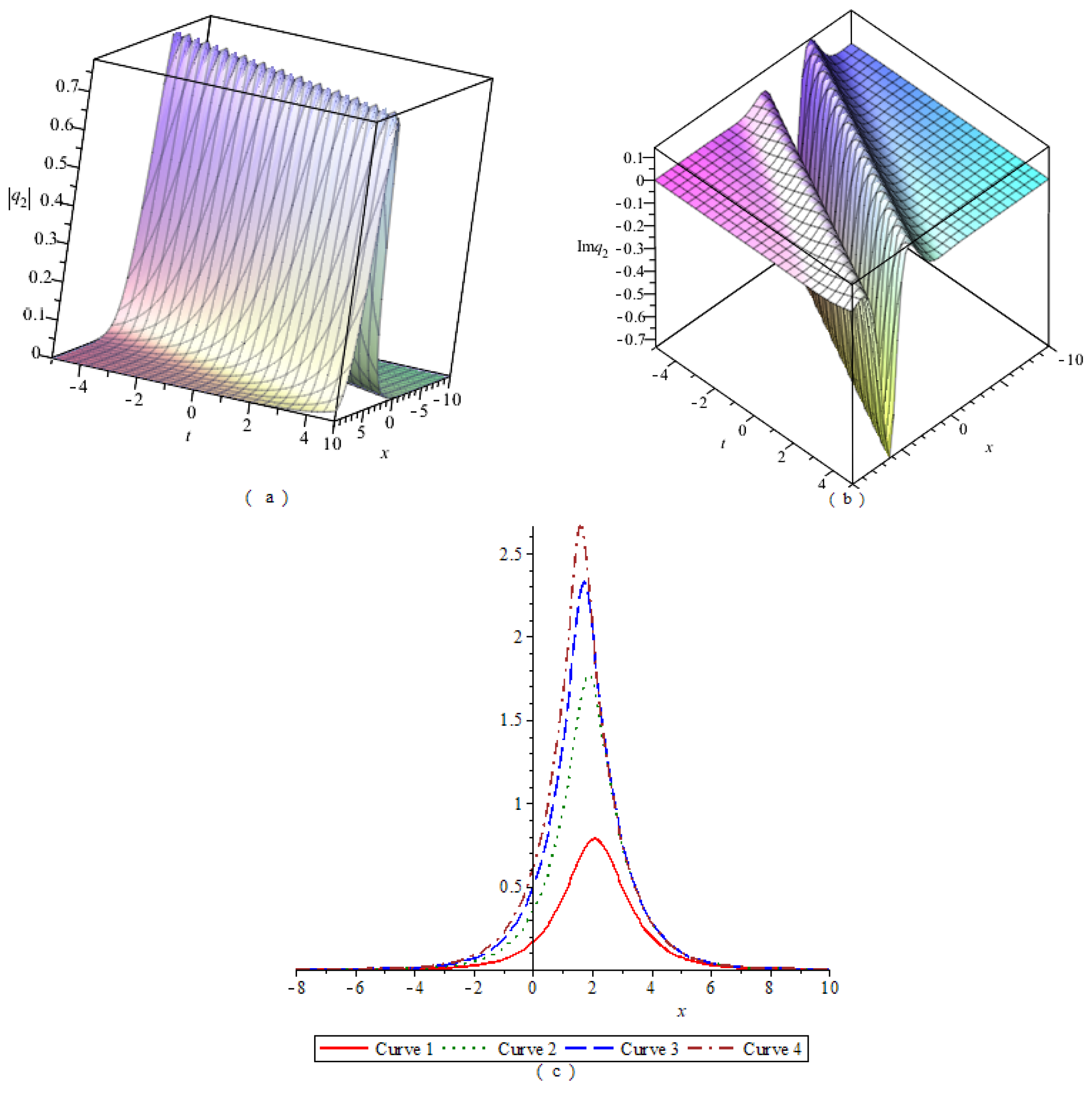
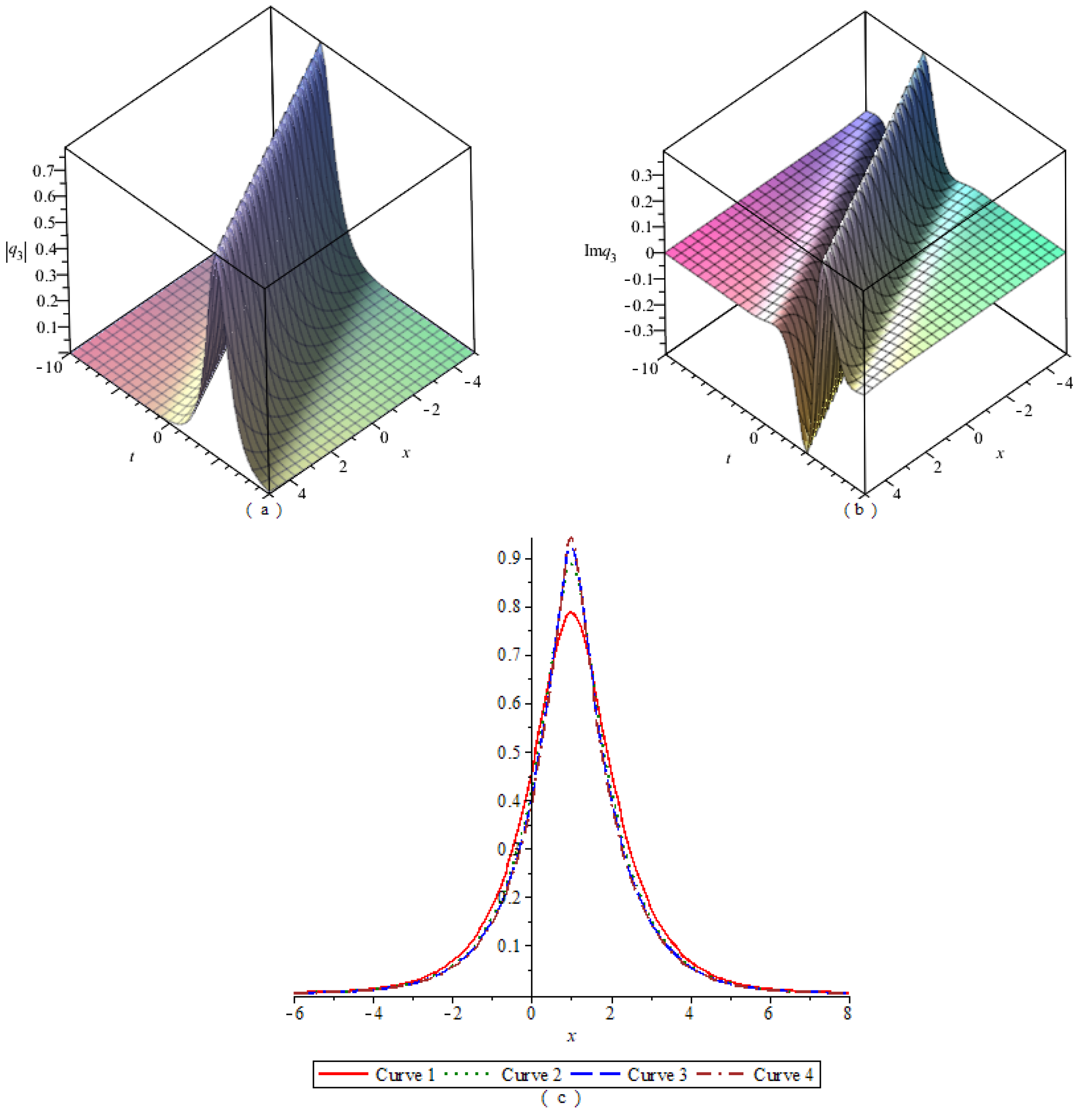
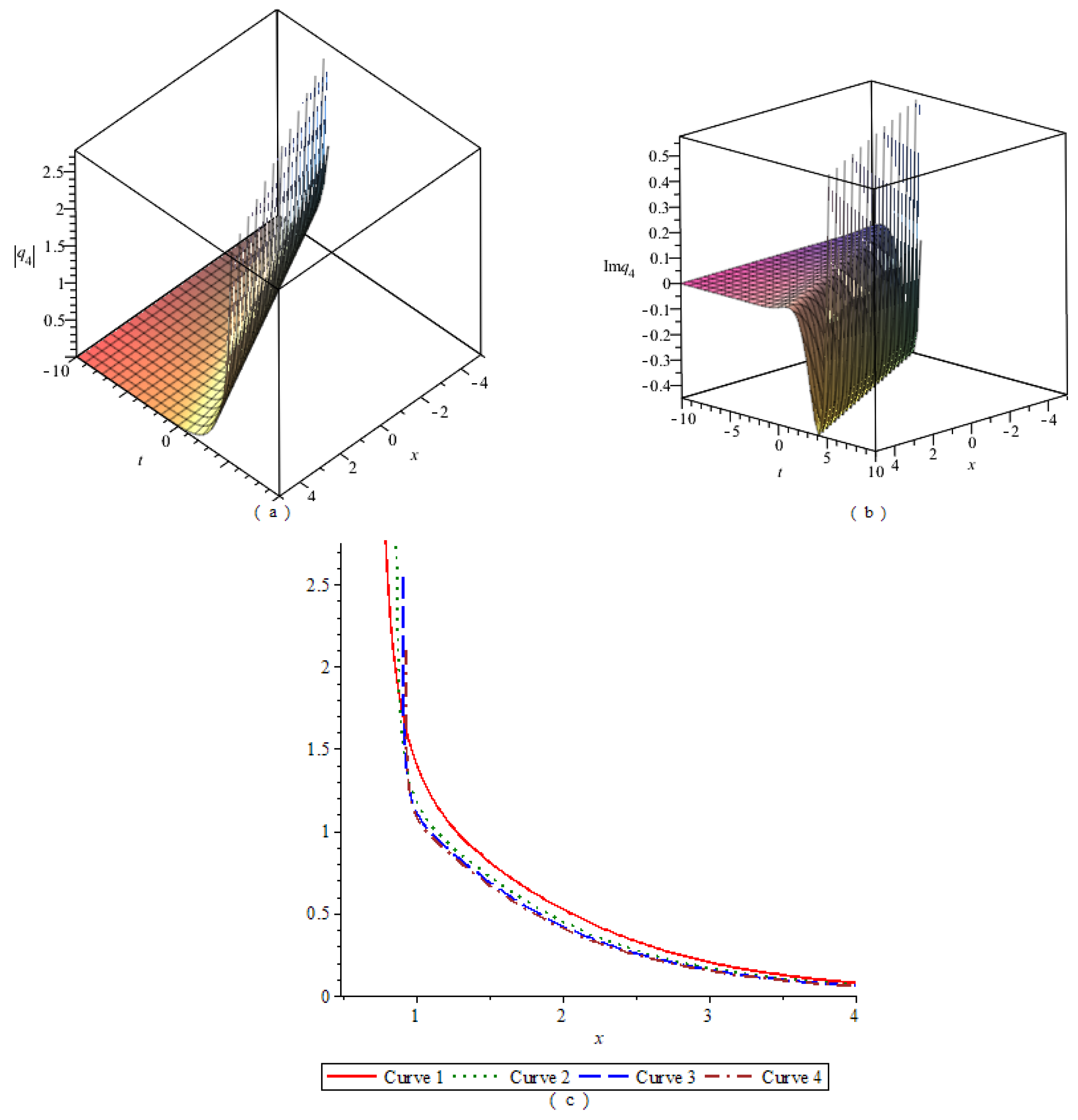
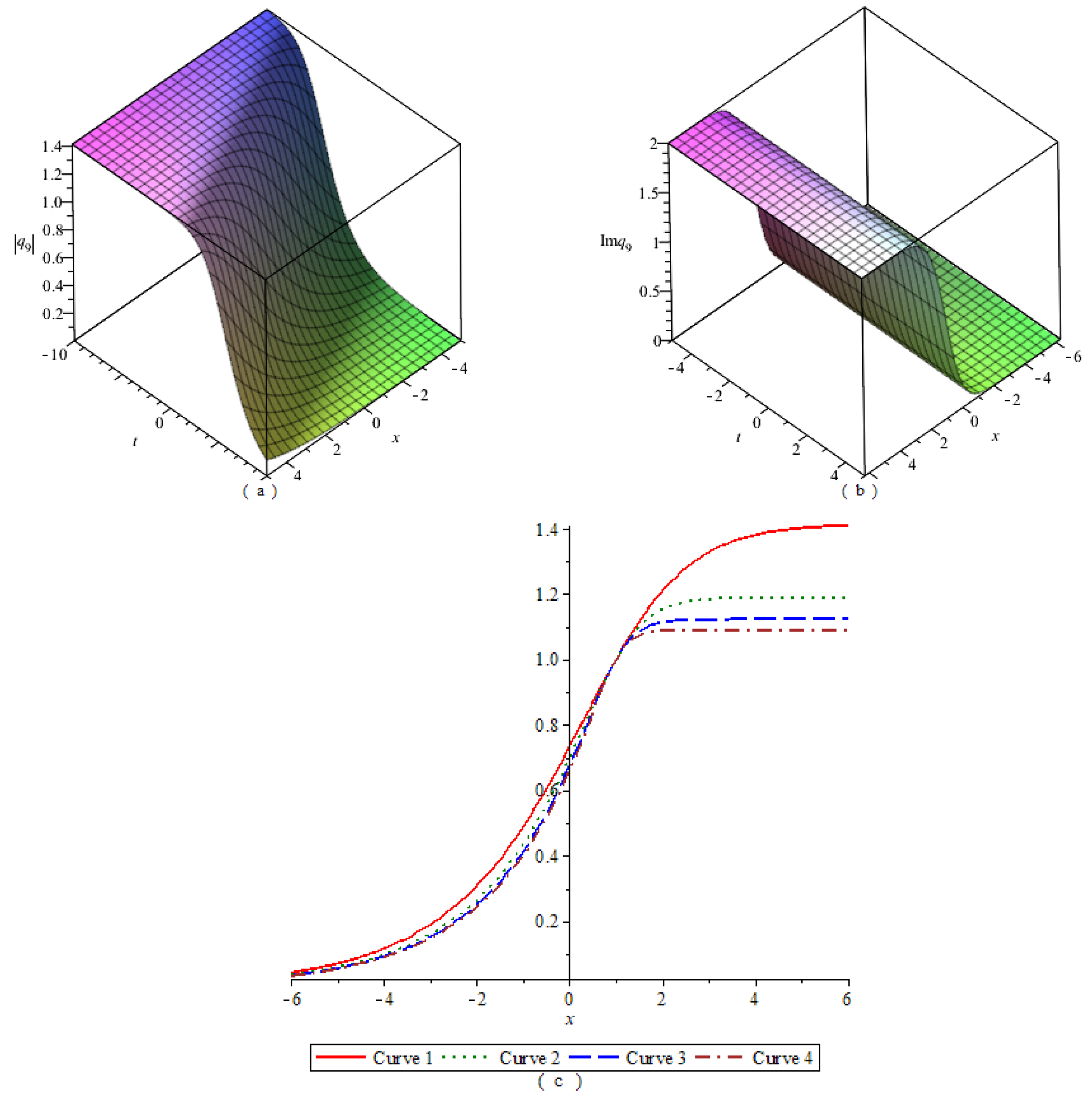
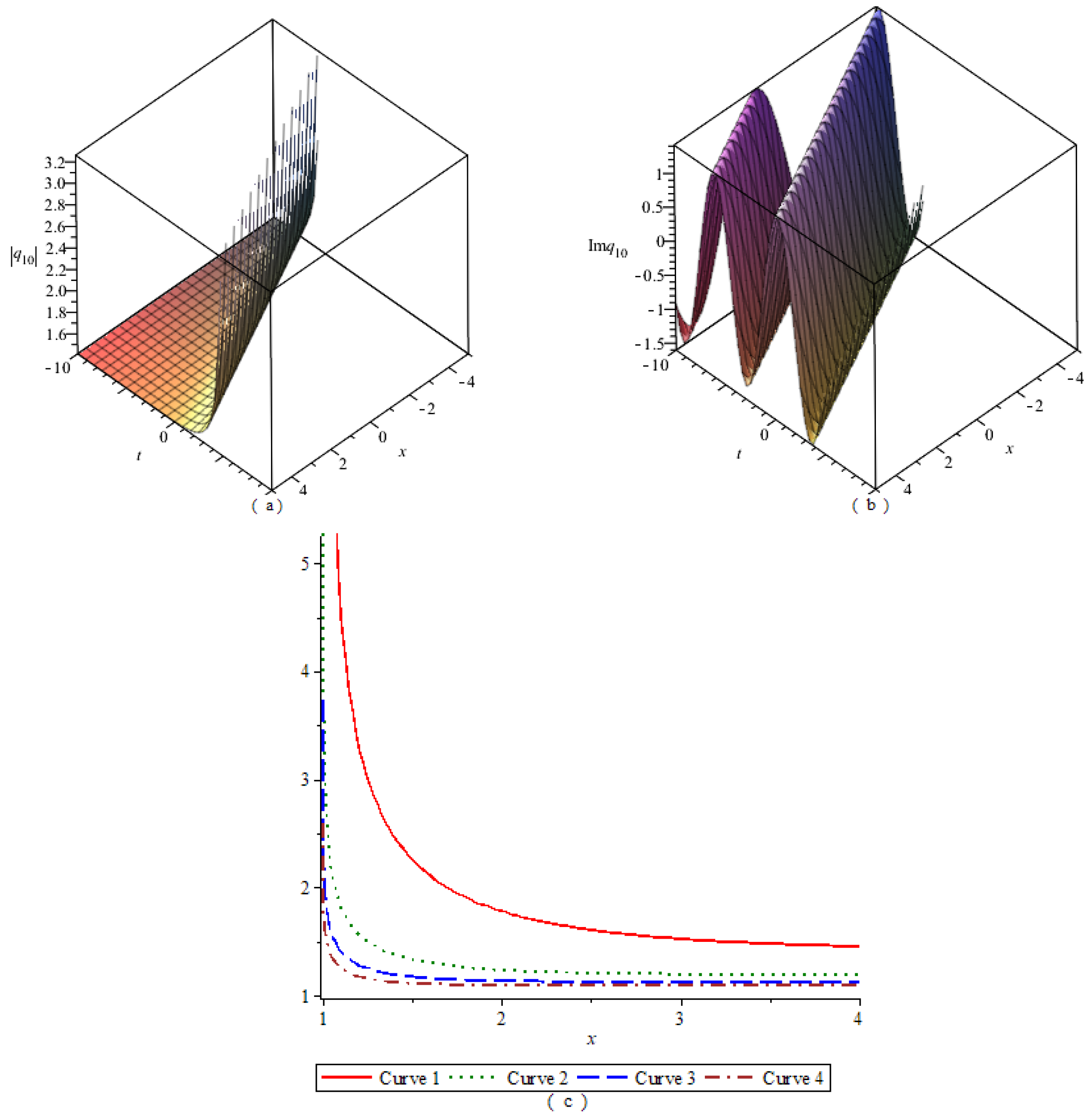
4. Graphical Representations of the Solutions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| No. | No. | ||
|---|---|---|---|
| 1 | 7 | ||
| 2 | 8 | ||
| 3 | 9 | ||
| 4 | 10 | ||
| 5 | 11 | ||
| 6 |
References
- González-Gaxiola, O.; Biswas, A. Optical solitons with Radhakrishnan–Kundu–Lakshmanan equation by Laplace-Adomian decomposition method. Optik 2019, 179, 434–442. [Google Scholar] [CrossRef]
- Ghanbari, B.; Gómez-Aguilar, J.F. Optical soliton solutions for the nonlinear Radhakrishnan–Kundu–Lakshmanan equation. Mod. Phys. Lett. B 2019, 33, 1950402. [Google Scholar] [CrossRef]
- Ozisik, M.; Secer, A.; Bayram, M.; Yusuf, A.; Sulaiman, T.A. On the analytical optical soliton solutions of perturbed Radhakrishnan–Kundu–Lakshmanan model with Kerr law nonlinearity. Opt. Quant. Electron. 2022, 54, 371. [Google Scholar] [CrossRef]
- Ganji, D.D.; Asgari, A.; Ganji, Z.Z. Exp-Function Based Solution of Nonlinear Radhakrishnan, Kundu and Laskshmanan (RKL) Equation. Acta Appl. Math. 2018, 104, 201–209. [Google Scholar] [CrossRef]
- Biswas, A.; Yildirim, Y.; Yasar, E.; Mahmood, M.F.; Alshomrani, A.S.; Zhou, Q.; Moshokoa, S.P.; Belic, M. Optical soliton perturbation for Radhakrishnan–Kundu–Lakshmanan equation with a couple of integration schemes. Optik 2018, 163, 126–136. [Google Scholar] [CrossRef]
- Biswas, A. 1-Soliton solution of the generalized Radhakrishnan, Kundu, Lakshmanan equation. Phys. Lett. A 2009, 373, 2546–2548. [Google Scholar] [CrossRef]
- Arshed, S.; Biswas, A.; Guggilla, P.; Alshomrani, A.S. Optical solitons for Radhakrishnan–Kundu–Lakshmanan equation with full nonlinearity. Phys. Lett. A 2020, 384, 126191. [Google Scholar] [CrossRef]
- Tarla, S.; Ali, K.K.; Yilmazer, R.; Osman, M.S. The dynamic behaviors of the Radhakrish-nan-Kundu-Lakshmanan equation by Jacobi elliptic function expansion technique. Opt. Quant. Electron. 2022, 54, 292. [Google Scholar] [CrossRef]
- Lu, D.; Seadawy, A.R.; Khater, M.M.A. Dispersive optical soliton solutions of the generalized Radha-krishnan-Kundu-Lakshmanan dynamical equation with power law nonlinearity and its applications. Optik 2018, 164, 54–64. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Solitary waves of the generalized Radhakrishnan–Kundu–Lakshmanan equation with four powers of nonlinearity. Phys. Lett. A 2022, 448, 128327. [Google Scholar] [CrossRef]
- Kudryashov, N.A. The Radhakrishnan–Kundu–Lakshmanan equation with arbitrary refractive index and its exact solutions. Optik 2021, 238, 166738. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Safonova, D.V.; Biswas, A. Painleve analysis and a solution to the traveling wave reduction of the Radhakrishnan–Kundu–Lakshmanan equation. Regul. Chaotic Dyn. 2019, 24, 607–614. [Google Scholar] [CrossRef]
- Alshehri, A.M.; Alshehri, H.M.; Alshreef, A.N.; Kara, A.H.; Biswas, A.; Yıldırım, Y. Conservation laws for dispersive optical solitons with Radhakrishnan–Kundu–Lakshmanan model having quadrupled power-law of self-phase modulation. Optik 2022, 267, 169715. [Google Scholar] [CrossRef]
- Annamalai, M.; Veerakumar, N.; Narasimhan, S.L.; Selvaraj, A.; Zhou, Q.; Biswas, A.; Ekici, M.; Alshehri, H.M.; Belić, M.R. Algorithm for dark solitons with Radhakrishnan–Kundu–Lakshmanan model in an optical fiber. Results Phys. 2021, 30, 104806. [Google Scholar] [CrossRef]
- Yildirim, Y.; Biswas, A.; Zhou, Q.; Alzahrani, A.K.; Belic, M.R. Optical solitons in birefringent fibers with Radhakrishnan–Kundu–Lakshmanan equation by a couple of strate gically sound integration architectures. Chin. J. Phys. 2020, 65, 341–354. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Bilal, M.; Younis, M.; Rizvi, S.T.R.; Makhlouf, M.M.; Althobaiti, S. Optical solitons to birefringent fibers for coupled Radhakrishnan–Kundu–Lakshmanan model without four-wave mixing. Opt. Quant. Electron. 2021, 53, 324. [Google Scholar] [CrossRef]
- Wang, K.J.; Si, J. Optical solitons to the Radhakrishnan–Kundu–Lakshmanan equation by two effective approaches. Eur. Phys. J. Plus 2022, 137, 1016. [Google Scholar] [CrossRef]
- Yildirim, Y.; Biswas, A.; Ekici, M.; Triki, H.; Gonzalez-Gaxiola, O.; Alzahrani, A.K.; Belic, M.R. Optical solitons in birefringent fibers for Radhakrishnan–Kundu–Lakshmanan with five prolific integration norms. Optik 2020, 208, 164550. [Google Scholar] [CrossRef]
- Zhang, K.; He, X.; Li, Z. Bifurcation analysis and classification of all single traveling wave solution in fiber Bragg gratings with Radhakrishnan–Kundu–Lakshmanan equation. AIMS Math. 2022, 7, 16733–16740. [Google Scholar] [CrossRef]
- Miura, M.R. Bäcklund Transformation; Springer: Berlin/Heidelberg, Germany, 1978. [Google Scholar]
- Al Qurashi, M.M.; Baleanu, D.; Inc, M. Optical solitons of transmission equation of ultra-short optical pulse in parabolic law media with the aid of Backlund transformation. Optik 2017, 140, 114–122. [Google Scholar] [CrossRef]
- Wang, P.; Tian, B.; Sun, K.; Qi, F.-H. Bright and dark soliton solutions and Bäcklund transformation for the Eckhaus-Kundu equation with the cubic-quintic nonlinearity. Appl. Math. Comput. 2015, 251, 233–242. [Google Scholar] [CrossRef]
- Inc, M.; Aliyu, A.I.; Yusuf, A. Optical solitons to the nonlinear Shrödinger’s equation with spatio-temporal dispersion using complex amplitude ansatz. J. Mod. Opt. 2017, 64, 2273–2280. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhu, Q.P. Optical solitons in medium with parabolic law nonlinearity and higher order dispersion. Waves Random Complex Media 2015, 25, 52–59. [Google Scholar] [CrossRef]
- Bekir, A.; Aksoy, E.; Güner, Ö. Optical soliton solutions of the long-short-wave interaction system. J. Nonlinear Opt. Phys. 2013, 22, 1350015. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alngar, M.E.M.; El-Horbaty, M.; Biswas, A.; Alshomrani, A.S.; Khan, S.; Ekici, M.; Triki, H. Optical solitons in fiber Bragg gratings having Kerr law of refractive index with extended Kudryashov’s method and new extended auxiliary equation approach. Chin. J. Phys. 2020, 66, 187–205. [Google Scholar] [CrossRef]
- Kilic, B.; Inc, M. Soliton solutions for the Kundu-Eckhaus equation with the aid of unified algebraic and auxiliary equation expansion methods. J. Electromagnet. Wave 2016, 30, 871–879. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alurrfi, K.A.E. Extended auxiliary equation method and its applications for finding the exact solutions for a class of nonlinear Schrödinger-type equations. Appl. Math. Comput. 2016, 289, 111–131. [Google Scholar] [CrossRef]
- Kader, A.H.A.; Latif, A.; Zhou, Q. Exact optical solitons in metamaterials with anti-cubic law of nonline-arity by Lie group method. Opt. Quant. Electron. 2019, 51, 30. [Google Scholar] [CrossRef]
- Iskenderoglu, G.; Kaya, D. Chirped self-similar pulses and envelope solutions for a nonlinear Schrödinger’s in optical fibers using Lie group method. Chaos Soliton Fract. 2022, 162, 112453. [Google Scholar] [CrossRef]
- Jafari, H.; Lia, A.; Tejadodi, H.; Baleanu, D. Analysis of Riccati differential equations within a new fractional derivative without singular kernel. Fundam. Inform. 2017, 151, 161–171. [Google Scholar] [CrossRef]
- Tajadodi, H.; Khan, Z.A.; ur Rehman Irshad, A.; Gömez-Aguilar, J.F.; Khan, A.; Khan, H. Exact solutions of conformable fractional differential equations. Results Phys. 2021, 22, 103916. [Google Scholar] [CrossRef]
- Yomba, E. The sub-ODE method for finding exact travelling wave solutions of generalized nonlinear Camassa-Holm, and generalized nonlinear Schrödinger equations. Phys. Lett. A 2008, 372, 215–222. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, C.; Li, L.; Liu, W. Some New Optical Solitons of the Generalized Radhakrishnan–Kundu–Lakshmanan Equations with Powers of Nonlinearity. Symmetry 2022, 14, 2626. https://doi.org/10.3390/sym14122626
Chen C, Li L, Liu W. Some New Optical Solitons of the Generalized Radhakrishnan–Kundu–Lakshmanan Equations with Powers of Nonlinearity. Symmetry. 2022; 14(12):2626. https://doi.org/10.3390/sym14122626
Chicago/Turabian StyleChen, Cheng, Lu Li, and Wei Liu. 2022. "Some New Optical Solitons of the Generalized Radhakrishnan–Kundu–Lakshmanan Equations with Powers of Nonlinearity" Symmetry 14, no. 12: 2626. https://doi.org/10.3390/sym14122626
APA StyleChen, C., Li, L., & Liu, W. (2022). Some New Optical Solitons of the Generalized Radhakrishnan–Kundu–Lakshmanan Equations with Powers of Nonlinearity. Symmetry, 14(12), 2626. https://doi.org/10.3390/sym14122626