The 2+1-Dimensional Special Relativity

Abstract

In the new mathematical description of special relativity in terms of the relativistic velocity space, many physical aspects acquire new geometric meanings. Performing conformal deformations upon the 2-dimensional relativistic velocity space for the (2+1)-dimensional special relativity, we find that these conformal deformations correspond to the generalized Lorentz transformations, which are akin to the ordinary Lorentz transformation, but are morphed by a global rescaling of the polar angle and correspondingly characterized by a topological integral index. The generalized Lorentz transformations keep the two fundamental principles of special relativity intact, suggesting that the indexed generalization may be related to the Bondi–Metzner–Sachs (BMS) group of the asymptotic symmetries of the spacetime metric. Furthermore, we investigate the Doppler effect of light, the Planck photon rocket, and the Thomas precession, affirming that they all remain in the same forms of the standard special relativity under the generalized Lorentz transformation. Additionally, we obtain the general formula of the Thomas precession, which gives a clear geometric meaning from the perspective of the gauge field theory in the relativistic velocity space.

1. Introduction

The special theory of relativity (also known as special relativity for short) is the foundational cornerstone of modern physics. It is replaced by the general theory of relativity (also known as general relativity) in the regime of strong gravitation and is expected to be modified by the effects of quantum gravity in the Planckian regime of the minuscule scale or extremely high energy. In the macroscopic scale with weak gravitational fields, special relativity has been experimentally verified to extremely high accuracy [1,2]. Nevertheless, considerable effort has continuously been devoted to modifying special relativity for various reasons. One prominent example is doubly special relativity (DSR) (also known as deformed special relativity) [3,4], in which not only the speed of light is constant for all observers, but additionally, a certain energy scale (at the scale of Plank energy) is also presupposed to be constant. The main motivation of DSR is to take into account the effects of quantum gravity, which are believed to be inappreciable until the energy approaches the Planck scale, while keeping the principle of relativity intact. The mathematical beauty of DSR has provided a new insightful perspective of the spacetime structure of special relativity.

Another direction that may lead to a modified theory of special relativity is the new mathematical description of special relativity in terms of the relativistic velocity space [5,6]. In $d+1$ dimensions, the relativistic velocity space is an abstract d-dimensional space that is composed of all possible velocities of particles in special relativity and endowed with a particular metric. Many physical aspects of special relativity acquire new geometric meanings in view of the relativistic velocity space. The velocity space possesses constant negative curvature $K=-1/c^2$, and thus, the maximum approachable velocity can be viewed as determined by the radius of curvature [1,2]. From the perspective of the velocity space, the Thomas precession can be understood in an elegant manner, which gives a direct geometric picture of this relativistic effect [7,8,9,10]. Furthermore, the velocity space also offers a beautiful approach to obtaining the laws of relativistic mechanics via the laws of hyperbolic geometry [11,12,13]. Recently, the form-invariant solutions have received much attention for many intriguing applications (see, e.g., [14,15]). The geometric origin of these solutions is the form-invariant deformation of the line element of the physical spacetime.

The aim of this paper is to further explore form-invariant deformations upon the velocity space and to see what modified theories of special relativity these deformations may lead to. Inspired by the deformation upon the hyperbolic surface of the momentum space investigated by the recent work for the magnetic Hook–Newton transmutation [16,17], we study the conformal deformations upon the 2-dimensional ($2d$) relativistic velocity space of the (2+1)d special relativity. The 2d velocity space naturally admits conformal deformations. These conformal deformations correspond to the generalized Lorentz transformations of the (2+1)d spacetime that are akin to the ordinary Lorentz transformation, but are morphed by a global rescaling of the polar angle and correspondingly characterized by a topological integral index. The two fundamental principles of special relativity—the principle of relativity and the principle of the constancy of the speed of light—remain intact under the generalized Lorentz transformations. In a sense, we found many different theories of special relativity that are consistent with the two fundamental principles. Furthermore, we investigate various relativistic effects and show that they all remain in the same forms of the standard special relativity under the generalized Lorentz transformation.

Although quite transparent in view of the velocity space, the geometric and physical meanings of the generalized Lorentz transformation with the integral index other than unity remain obscure from the perspective of the (2+1)d spacetime. The extra structure characterized by the integral index is reminiscent of the Bondi–Metzner–Sachs (BMS) group of the asymptotic symmetries of the spacetime metric [18,19,20]. In the asymptotically flat region, in addition to the Poincaré group, the spacetime symmetry also exhibits the extra symmetry of the BMS group. The BMS symmetry may be understood in terms of the generalized Lorentz transformations revealed in this paper. If this is indeed the case, the conformal symmetry of the relativistic velocity space is related to the BMS symmetry of the asymptotically flat spacetime. The study of this paper gives preliminary results that suggest this correspondence.

This paper is arranged as follows: In Section 2, by starting with the line element of the velocity space, it is found that there exists a family of conformal transformation that produces the form-invariant deformation of the line element while maintaining the geometric attributes of negative constant curvature and the curvature radius of the velocity space. The geometric invariances imply that the relativity principle and the absolute attribute of the light speed for all observers are preserved under the conformal deformation. Then, we show that the relative relation of the velocity observed by two inertial frames of reference is established by a generalized velocity transformation with conformal freedom. The spacetime transformation corresponding to the velocity transformation is given. In Section 3, the equation of motion for the (2+1)d relativity established by the generalized velocity transformation (referred to as conformal relativity in some places later) is presented. The Doppler effect of light and the photon rocket are used to illustrate the change of the relativistic effect with the deformation of the velocity space. In Section 4, the Thomas precession in the relativity is discussed. The general expression and an infinitesimal representation are presented. Finally, several observations about the presented conformal relativity are discussed. We also give a perspective of the gauge field for the Thomas precession in the Conclusion.

2. The Lorentz Transformation Emerging from the Conformal Velocity Space

Given the position vector $\mathbf{r}$ of a 2d particle in Newtonian mechanics, its velocity is determined by the time derivative $d\mathbf{r}/dt=\mathbf{v}$. Let $0\le \theta <2\pi$ be the orientation angle of the velocity measured from the basis axis $(v_x,0)$ with $0\le v_x<\infty$ in the velocity space. The velocity vector can be labeled by $\mathbf{v}=(v,\theta )$ with $v=\left|\mathbf{v}\right|$ being the magnitude of the velocity. Using the variables $(v,\theta )$, it was pointed out that the line element $d{s}_v$ of the relativistic velocity space can be expressed as (the Klein model) [5,6,10]

$${\left(d{s}_v\right)}^2=\frac{1}{{\left[1-{(v/c)}^2\right]}^2}{\left(dv\right)}^2+\frac{v^2}{\left[1-{(v/c)}^2\right]}{\left(d\theta \right)}^2,$$

(1)

where c is the light speed and $d{s}_v$ has the meaning of the relative velocity between the velocity intervals $(v,\theta )$ and $(v+dv,\theta +d\theta )$. When $v<<c$, the line element reduces to

$${\left(d{s}_v\right)}^2={\left(dv\right)}^2+v^2{\left(d\theta \right)}^2,$$

(2)

which is the line element of the Euclidean velocity space in Newtonian mechanics. It is easy to show that the relativistic space has negative Gaussian curvature $K=-1/c^2$, and the curvature radius of the space is the light speed $\rho =1/\sqrt{-K}=c$. There are two significant features in (1), which differ from (2). (i) The particle velocity cannot be faster than the light speed, and (ii) the line element is invariant under the Lorentz velocity transformation. Since the relativistic physical laws can be established from (1), the second point also means that the principle of relativity for the physical laws is true under the Lorentz velocity transformation. To generalize the relativity principle, we investigate the form-invariant line element:

$${\left(d{s}_{\overline{v}}\right)}^2=\frac{1}{{\left[1-{(\overline{v}/c)}^2\right]}^2}{\left(d\overline{v}\right)}^2+\frac{{\overline{v}}^2}{\left[1-{(\overline{v}/c)}^2\right]}{\left(d\overline{\theta }\right)}^2,$$

(3)

where the relation between $(\overline{v},\overline{\theta })$ and $(v,\theta )$ is established by the transformation (see Appendix A for details):

$$\frac{\overline{v}}{c}=\frac{2x^a{(1+\sqrt{1-x^2})}^a}{{(1+\sqrt{1-x^2})}^{2a}+x^{2a}}\mathrm{with}x=v/c,\mathrm{and}\overline{\theta }=a\theta .$$

(4)

The constant a is a real number, and $\overline{v}$ is a symmetric function with respect to the indexes a and $-a$. The velocity space with the coordinates $(\overline{v},\overline{\theta })$ still has the constant light speed c as the limit of the speed and the curvature radius of the space. The graphs in Figure 1 exhibit the relations between $\overline{v}$ and v, where two graphs with a fractional index are also presented. Since the fractional index makes the coordinates $(\overline{v},\overline{\theta })$ become multivalued, the corresponding theory would not be acceptable physically. The space formed by all points of $(\overline{v},\overline{\theta })$ is the conformal deformation of the relativistic velocity space of $(v,\theta )$. This is manifested by the representation:

$${\left(d{s}_{\overline{v}}\right)}^2={\left(\frac{d\overline{v}}{dv}\right)}^2{\left[\frac{1-{(v/c)}^2}{1-{(\overline{v}/c)}^2}\right]}^2{\left(d{s}_v\right)}^2$$

(5)

with the derivative:

$$\frac{d\overline{v}}{dv}=\frac{2a{x}^{a-1}{(1+\sqrt{1-x^2})}^a\left[{(1+\sqrt{1-x^2})}^{2a}-x^{2a}\right]}{\sqrt{1-x^2}{\left[{(1+\sqrt{1-x^2})}^{2a}+x^{2a}\right]}^2}.$$

(6)

When $v<<c,$ thus $\overline{v}<<c$, the line element becomes

$${\left(d{s}_{\overline{v}}\right)}^2={\left(d\overline{v}\right)}^2+{\overline{v}}^2{\left(d\overline{\theta }\right)}^2=\frac{a{v}^{a-1}}{{\left(2c\right)}^{a-1}}\left[{\left(dv\right)}^2+v^2{\left(d\theta \right)}^2\right],$$

(7)

where $\overline{v}=v^a/{\left(2c\right)}^{a-1}$ and $\overline{\theta }=a\theta$ are the conformal transformation of the $2d$ Euclidean velocity space [17]. All the points $(\overline{v},\overline{\theta })$ form the conformal Klein model of velocity. Figure 2 is an illustration of the model. Since the conformal space has negative constant curvature, it is a conformal hyperbolic surface. This is manifested by executing the isometric transformation:

$$\overline{v}/c=tanh(\overline{w}/c),\mathrm{and}\overline{\theta }=\overline{\Theta }$$

(8)

such that

$${\left(d{s}_{\overline{v}}\right)}^2=d{\overline{w}}^2+c^2{sinh}^2(\overline{w}/c){\left(d\overline{\Theta }\right)}^2$$

(9)

$$={\left(\frac{d\overline{w}}{dw}\right)}^2\left[d{w}^2+c^2{sinh}^2(w/c){(d\Theta )}^2\right],$$

(10)

where the derivative in the conformal factor is given by [16]

$$\frac{d\overline{w}}{dw}=\frac{2a{\left[sinh(w/c)\right]}^{a-1}}{{\left[cosh(w/c)+1\right]}^a-{\left[cosh(w/c)-1\right]}^a}.$$

(11)

Equation (9) is the common expression of the $2d$ hyperbolic surface, but with the deformed coordinates $(\overline{w},\overline{\Theta })$.

In the following, we shall discuss the special relativity based on (3). For this, we need to know the Lorentz transformation in the theory, which represents the relative distance and orientation of two points in the Klein model. They can be obtained by evaluating the length $s_{\overline{v}}$ of the geodesic in the model and the angle ${\overline{\theta }}^{\prime }$ of the geodesic with a basis axis. The geodesics satisfy the equations:

$$\frac{d^2{\overline{v}}^k}{d{s}_{\overline{v}}^2}+{\Gamma }_{ij}^k\frac{d{\overline{v}}^i}{d{s}_{\overline{v}}}\frac{d{\overline{v}}^j}{d{s}_{\overline{v}}}=0,i,j,\mathrm{and}k=1,2.$$

(12)

Using the representation (3), we have the component ${\Gamma }_{\overline{v}\overline{\theta }}^{\overline{\theta }}=1/2g^{\overline{\theta }\overline{\theta }}{\partial }_{\overline{v}}{g}_{\overline{\theta }\overline{\theta }}$, and the corresponding equation for $\overline{\theta }$ is

$$\frac{d}{d{s}_{\overline{v}}}\left(g_{\overline{\theta }\overline{\theta }}\frac{d\overline{\theta }}{d{s}_{\overline{v}}}\right)=0,$$

(13)

which implies $d\overline{\theta }/d{s}_{\overline{v}}=h/g_{\overline{\theta }\overline{\theta }}$ with h being a constant. It follows from Equation (3) that the trajectory of a geodesic satisfies

$$\frac{d\overline{v}}{d\overline{\theta }}=\frac{\overline{v}\sqrt{{\overline{v}}^2-D^2}}{D},\mathrm{with}\mathrm{the}\mathrm{constant}D={\left(\frac{1}{h^2}+\frac{1}{c^2}\right)}^{-1}.$$

(14)

The equation can be integrated, and we obtain the geodesic equation on the conformal model:

$$A\overline{v}cos\overline{\theta }+B\overline{v}sin\overline{\theta }+Dc=0,$$

(15)

where the constant coefficients A and B satisfy $A^2+B^2=c^2$, and D is the perpendicular distance from the geodesic to the origin in the model. Therefore, using the conformal Klein model to represent the velocity space, the equation of a geodesic is the same as the equation for a straight line in the Euclidean plane with variables $(\overline{v},\overline{\theta })$ and can be labeled by the constants $(A,B,D)$.

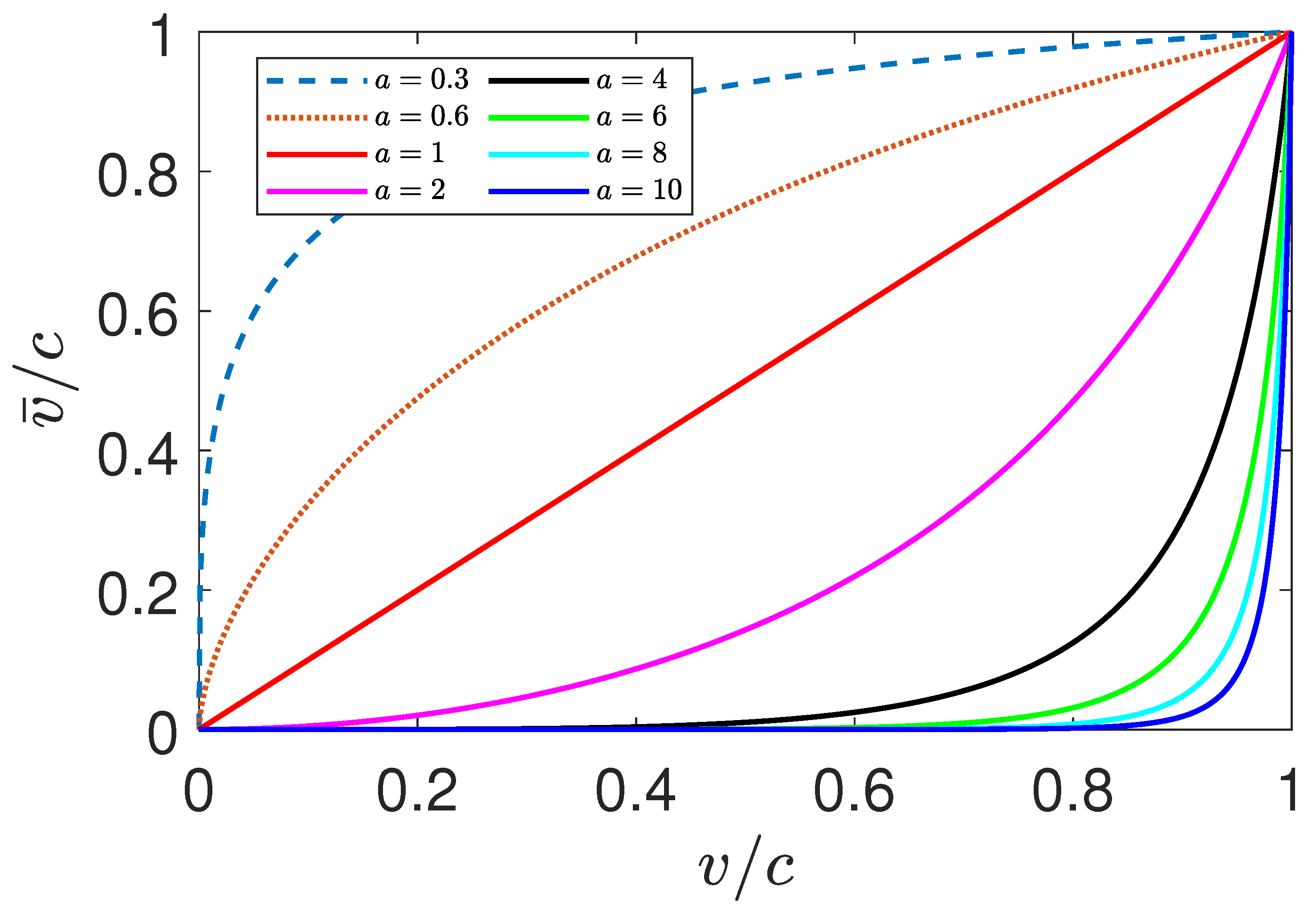
2.1. The Expression of Relative Velocity

The length of a geodesic connected by two points on the Klein model represents the relative velocity of two inertial frames relative to each other. It can be obtained by evaluating the finite length $s_{\overline{v}}$ of a geodesic. From (3), the length $s_{\overline{v}}$ that connects the points $P_1$ and $P_2$ has the expression:

$$s_{\overline{v}}={\int }_{P_1}^{P_2}\sqrt{\frac{1}{{\left[1-{(\overline{v}/c)}^2\right]}^2}{(d\overline{v}/d\overline{\theta })}^2+\frac{{\overline{v}}^2}{1-{(\overline{v}/c)}^2}}d\overline{\theta }.$$

(16)

By substituting (14) in it, we find

$$s_{\overline{v}}=\frac{c}{2}ln{\left[\frac{\sqrt{c^2-D^2}-\sqrt{{\overline{v}}^2-D^2}}{\sqrt{c^2-D^2}+\sqrt{{\overline{v}}^2-D^2}}\right]}_{P_1}^{P_2}.$$

(17)

If we explicitly assign the coordinates $P_1=({\overline{v}}_{a}cos{\overline{\theta }}_a,{\overline{v}}_{a}sin{\overline{\theta }}_a)$ and $P_2=({\overline{v}}_{b}cos{\overline{\theta }}_b,{\overline{v}}_{b}sin{\overline{\theta }}_b)$, it can be expressed as

$$cosh\left(\frac{{\overline{v}}_{ab}}{c}\right)=\frac{1-{\overline{v}}_a{\overline{v}}_{b}cos({\overline{\theta }}_b-{\overline{\theta }}_a)/c^2}{\sqrt{1-{({\overline{v}}_a/c)}^2}\sqrt{1-{({\overline{v}}_b/c)}^2}},$$

(18)

where the length $s_{\overline{v}}$ with the terminal points is named as ${\overline{v}}_{ab}$. It can also be expressed as

$${\overline{v}}_{ab}=c{\left\{1-\frac{\left[1-{({\overline{v}}_a/c)}^2\right]\left[1-{({\overline{v}}_b/c)}^2\right]}{{\left[1-{\overline{v}}_a{\overline{v}}_{b}cos({\overline{\theta }}_b-{\overline{\theta }}_a)/c^2\right]}^2}\right\}}^{1/2}.$$

(19)

The expression is maintained form invariance [6,13], but contains the deformation information.

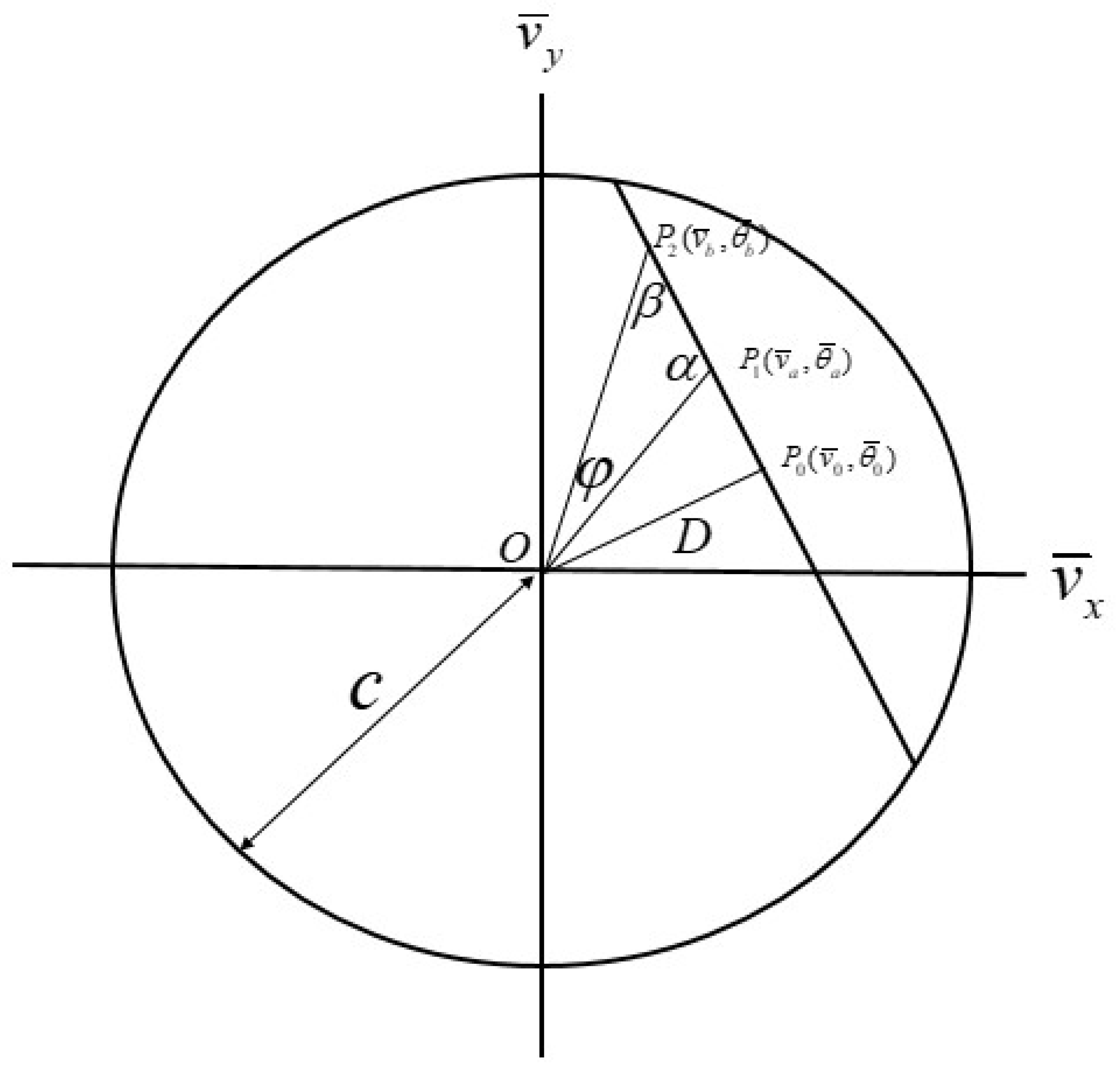
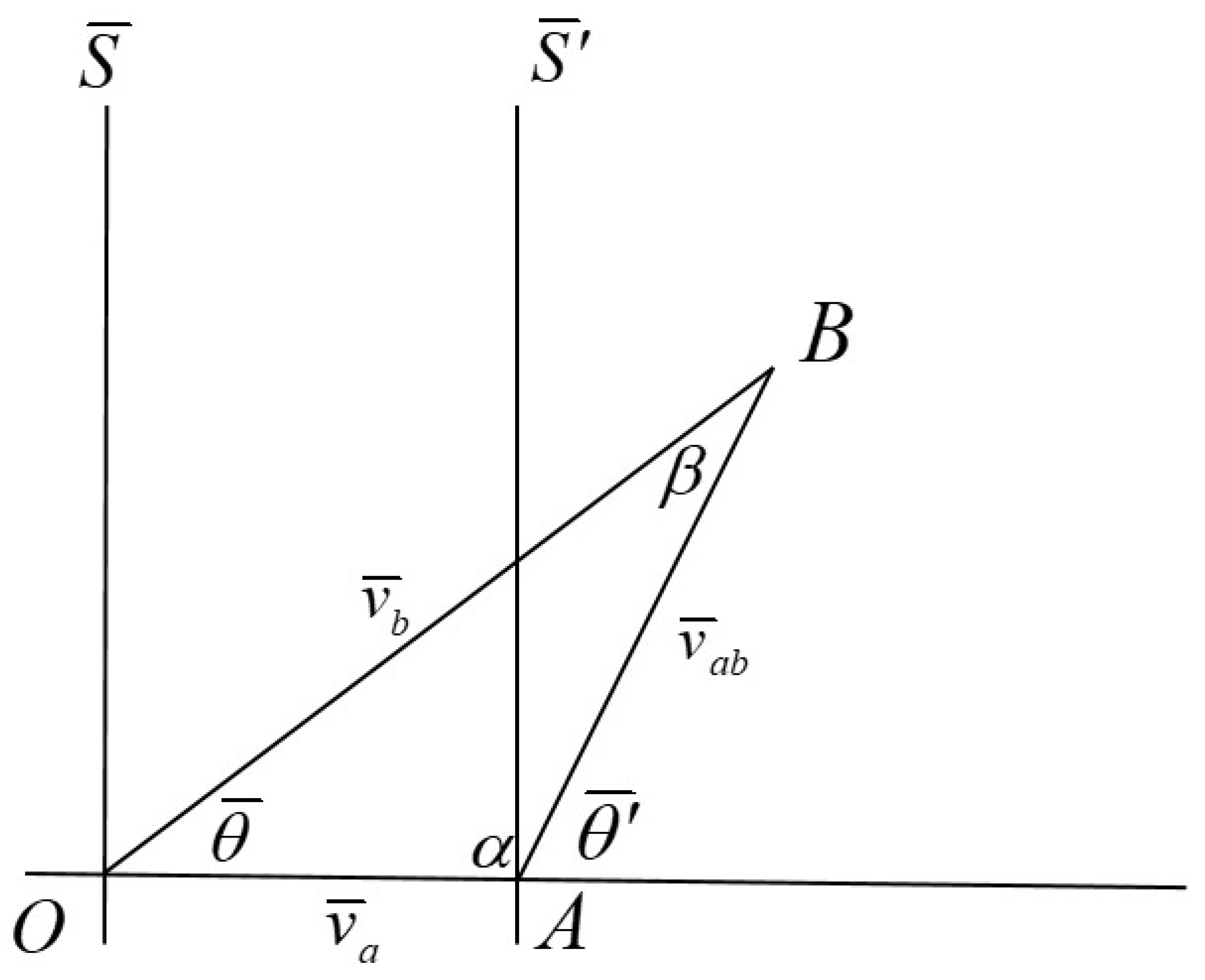
2.2. The Measurement of the Intersecting Angle between Two Geodesics on the Conformal Klein Model

Assume that two straight lines $(A_1,B_1,D_1)$ and $(A_2,B_2,D_2)$ intersect at a point. The displacements along these two lines at the point are $\left(d{\overline{v}}^i\right)$ and $\left(\delta {\overline{v}}^j\right)$. The intersection angle ${\overline{\theta }}^{\prime }$ satisfies (e.g., Equation (56) in [21])

$$cos{\overline{\theta }}^{\prime }=\frac{g_{ij}d{\overline{v}}^i\delta {\overline{v}}^j}{\sqrt{g_{ij}d{\overline{v}}^{i}d{\overline{v}}^j}\sqrt{g_{ij}\delta {\overline{v}}^i\delta {\overline{v}}^j}}.$$

(20)

Using the relation:

$${\left(\frac{d\overline{v}}{d\overline{\theta }}\right)}_n=\overline{v}\left(\frac{A_{n}sin\overline{\theta }-B_{n}cos\overline{\theta }}{A_{n}cos\overline{\theta }+B_{n}sin\overline{\theta }}\right)$$

(21)

derived from (15) and (3), it can be shown that

$$cos{\overline{\theta }}^{\prime }=\frac{A_1{A}_2+B_1{B}_2-D_1{D}_2}{\sqrt{A_1^2+B_1^2-D_1^2}\sqrt{A_2^2+B_2^2-D_2^2}}$$

(22)

The condition of the perpendicularity of two geodesics is given by

$$A_1{A}_2+B_1{B}_2-D_1{D}_2=0.$$

(23)

Two usual cases are as follows: (i) If two geodesics pass through the origin, i.e., $D_1=D_2=0$,

$$cos{\overline{\theta }}^{\prime }=\frac{A_1{A}_2+B_1{B}_2}{\sqrt{A_1^2+B_1^2}\sqrt{A_2^2+B_2^2}}.$$

(24)

The measurement of the angle ${\overline{\theta }}^{\prime }$ is the same as on the Euclidean plane. (ii) If only a line passes through the origin, i.e., $D_1=0$ or $D_2=0$, the condition of two lines with the intersection angle ${\overline{\theta }}^{\prime }=\pi /2$ is

$$A_1{A}_2+B_1{B}_2=0.$$

(25)

Therefore, the condition of the right angle for one of the lines passing through the origin is the same as in the Euclidean space. The conformal condition makes these two results the same as the Klein model (e.g., [10]). Assume that one straight line $(A_1,B_1,D_1)$ is determined by two points with coordinates $({\overline{v}}_a,{\overline{\theta }}_a)$ and $({\overline{v}}_b,{\overline{\theta }}_b)$. The other line is the basis axis $(0,B_2,0)$ with $\overline{\theta }=0$. Equation (22) reduces to

$$cos{\overline{\theta }}^{\prime }=\frac{B_1}{\sqrt{A_1^2+B_1^2-D_1^2}}.$$

(26)

Expressing $(A_1,B_1,D_1)$ in terms of the coordinates gives the orientation angle of the relative velocity:

$$cos{\overline{\theta }}^{\prime }=\frac{{\overline{v}}_{b}cos{\overline{\theta }}_b-{\overline{v}}_{a}cos{\overline{\theta }}_a}{{\left[{\overline{v}}_b^2+{\overline{v}}_a^2-2{\overline{v}}_b{\overline{v}}_{a}cos({\overline{\theta }}_b-{\overline{\theta }}_a)-{\left({\overline{v}}_b{\overline{v}}_a/c\right)}^2{sin}^2({\overline{\theta }}_b-{\overline{\theta }}_a)\right]}^{1/2}}.$$

(27)

Without loss of generality, choose $({\overline{v}}_a,{\overline{\theta }}_a)=$$({\overline{v}}_a,0)$ and $({\overline{v}}_b,{\overline{\theta }}_b)=({\overline{v}}_b,\overline{\theta })$. Equations (19) and (27) reduce to

$${\overline{v}}_{ab}=c{\left\{1-\frac{\left[1-{({\overline{v}}_a/c)}^2\right]\left[1-{({\overline{v}}_b/c)}^2\right]}{{\left[1-{\overline{v}}_a{\overline{v}}_{b}cos\overline{\theta }/c^2\right]}^2}\right\}}^{1/2},$$

(28)

and

$$cos{\overline{\theta }}^{\prime }=\frac{{\overline{v}}_{b}cos\overline{\theta }-{\overline{v}}_a}{{\left[{\overline{v}}_b^2+{\overline{v}}_a^2-2{\overline{v}}_b{\overline{v}}_{a}cos\overline{\theta }-{\left({\overline{v}}_b{\overline{v}}_a/c\right)}^2{sin}^2\overline{\theta }\right]}^{1/2}}.$$

(29)

The angle ${\overline{\theta }}^{\prime }$ can also be expressed as

$$cot{\overline{\theta }}^{\prime }=\frac{1}{\sqrt{1-{\left({\overline{v}}_a/c\right)}^2}}\left[cot\overline{\theta }-\frac{{\overline{v}}_a}{{\overline{v}}_b}csc\overline{\theta }\right].$$

(30)

Equations (28) and (29) or (30) are a generalized Lorentz velocity transformation with conformal deformation. They maintain form invariance. The illustration in Figure 3 shows the relative relation of a particle with velocity ${\overline{v}}_{ab}$ observed by the frame ${\overline{S}}^{\prime }$ at Point A, which has the velocity $({\overline{v}}_a,0)$ relative to the frame $\overline{S}$ at Point O on the conformal Klein model. The relative relation between the velocities ${\overline{v}}_b$ and ${\overline{v}}_{ab}$ observed by $\overline{S}$ and ${\overline{S}}^{\prime }$ are established by (28) and (29). If we choose $\overline{\theta }=\pi /2$ in the velocity triangle $OAB$, we have

$$cot\alpha =-cot{\overline{\theta }}^{\prime }=\frac{{\overline{v}}_a/{\overline{v}}_b}{\sqrt{1-{\left({\overline{v}}_a/c\right)}^2}}$$

(31)

from (30). By interchanging ${\overline{v}}_b$ and ${\overline{v}}_a$, we find

$$cot\beta =\frac{{\overline{v}}_b/{\overline{v}}_a}{\sqrt{1-{\left({\overline{v}}_b/c\right)}^2}}.$$

(32)

Thus,

$$cot(\alpha +\beta )=\frac{cot\alpha cot\beta -1}{cot\alpha +cot\beta }>0,$$

(33)

which shows $\alpha +\beta <\pi /2$, resulting in $\alpha +\beta +\overline{\theta }<\pi$. This manifests the attribute of the hyperbolic triangle. When ${\overline{v}}_a$ and ${\overline{v}}_b\ll c$, the sum of the inner angles becomes $\alpha +\beta +\overline{\theta }=\pi$, reducing to the velocity transformation satisfying the plane geometry. To have the common transformation with the Cartesian components, let us call ${\overline{v}}_a=\overline{V}$, ${\overline{v}}_b=\overline{v}$, and ${\overline{v}}_{ab}={\overline{v}}^{\prime }$. By setting $\overline{V}$ as the basis axis with $\overline{\theta }=0$ and establishing the frames ${\overline{S}}^{\prime }$ and $\overline{S}$, we have ${\overline{v}}_x^{\prime }={\overline{v}}^{\prime }cos{\overline{\theta }}^{\prime }$ and ${\overline{v}}_y^{\prime }={\overline{v}}^{\prime }sin{\overline{\theta }}^{\prime }$. It follows that the transformation:

$${\overline{v}}_x^{\prime }=\frac{{\overline{v}}_x-\overline{V}}{1-{\overline{v}}_x\overline{V}/c^2},\mathrm{and}{\overline{v}}_y^{\prime }=\frac{{\overline{v}}_y}{{\gamma }_{\overline{V}}(1-{\overline{v}}_x\overline{V}/c^2)},$$

(34)

from (28) and (29), where ${\gamma }_{\overline{V}}=1/\sqrt{1-{\left(\overline{V}/c\right)}^2}$. These are the Cartesian components of the velocity transformation in the conformal velocity space. They also retain form invariance.

The representation of the Cartesian components of the line element in (3) can be obtained by the isometric transformation:

$$\overline{v}=\sqrt{{\overline{v}}_x^2+{\overline{v}}_y^2},\mathrm{and}tan\overline{\theta }=\frac{{\overline{v}}_y}{{\overline{v}}_x},$$

(35)

which makes (3) have the expression:

$${\left(d{s}_{\overline{v}}\right)}^2={\overline{g}}_{ij}d{\overline{v}}^{i}d{\overline{v}}^j,(i,j=1,2)$$

(36)

with

$${\overline{g}}_{ij}=\frac{1}{{\left[1-{\left(\overline{v}/c\right)}^2\right]}^2}\left[\begin{array}{cc}1-{({\overline{v}}_y/c)}^2& {\overline{v}}_x{\overline{v}}_y/c^2\\ {\overline{v}}_x{\overline{v}}_y/c^2& 1-{({\overline{v}}_x/c)}^2\end{array}\right],$$

(37)

where we introduced the notations ${\overline{v}}^1={\overline{v}}_x$ and ${\overline{v}}^2={\overline{v}}_y$ to denote the x- and y-components of velocity. It can be shown that

$${\left(d{s}_{\overline{v}}\right)}^2={\overline{g}}_{ij}d{\overline{v}}^{i}d{\overline{v}}^j={\overline{g}}_{ij}^{\prime }d{\overline{v}}^{\prime i}d{\overline{v}}^{\prime j},$$

(38)

with

$${\overline{g}}_{ij}^{\prime }=\frac{1}{{\left[1-{\left({\overline{v}}^{\prime }/c\right)}^2\right]}^2}\left[\begin{array}{cc}1-{({\overline{v}}_y^{\prime }/c)}^2& {\overline{v}}_x^{\prime }{\overline{v}}_y^{\prime }/c^2\\ {\overline{v}}_x^{\prime }{\overline{v}}_y^{\prime }/c^2& 1-{({\overline{v}}_x^{\prime }/c)}^2\end{array}\right],$$

(39)

in which ${\overline{v}}^{\prime 1}={\overline{v}}_x^{\prime },{\overline{v}}^{\prime 2}={\overline{v}}_y^{\prime }$ and ${\overline{v}}^{\prime }=\sqrt{{\overline{v}}_x^{\prime 2}+{\overline{v}}_y^{\prime 2}}$. Therefore, the Lorentz velocity transformation with the conformal coordinates leaves the line element of the conformal velocity space form-invariant.

Corresponding to the conformal velocity transformation (34), there must exist a (2+1)d spacetime transformation. They possess the form:

$$\left\{\begin{array}{c}{\overline{x}}^{\prime }={\gamma }_{\overline{V}}(\overline{x}-\overline{V}\overline{t})\hfill \\ {\overline{y}}^{\prime }=\overline{y}\hfill \\ {\overline{t}}^{\prime }={\gamma }_{\overline{V}}(\overline{t}-\frac{\overline{V}}{c^2}\overline{x})\hfill \end{array}\right..$$

(40)

Using the notations:

$$\frac{d{\overline{x}}^{\prime }}{d{\overline{t}}^{\prime }}={\overline{v}}_x^{\prime },\frac{d{\overline{y}}^{\prime }}{d{\overline{t}}^{\prime }}={\overline{v}}_y^{\prime },\frac{d\overline{x}}{d\overline{t}}={\overline{v}}_x,\frac{d\overline{y}}{d\overline{t}}={\overline{v}}_y,$$

it is easy to obtain the velocity transformation in (34) from (40). Equation (40) exhibits the invariant spacetime interval:

$${\left(c{\overline{t}}^{\prime }\right)}^2-({\overline{x}}^{\prime 2}+{\overline{y}}^{\prime 2})={\left(c\overline{t}\right)}^2-({\overline{x}}^2+{\overline{y}}^2).$$

(41)

For an infinitesimal interval, this can be expressed as

$$c^2{\left(d{\overline{t}}^{\prime }\right)}^2-{\left(d{\overline{x}}^{{}^{\prime }}\right)}^2-{\left(d{\overline{y}}^{\prime }\right)}^2=c^2{\left(d\overline{t}\right)}^2-{\left(d\overline{x}\right)}^2-{\left(d\overline{y}\right)}^2,$$

(42)

or

$${\left(cd{\overline{t}}^{\prime }\right)}^2\left[1-\frac{1}{c^2}\left({\left(\frac{d{\overline{x}}^{\prime }}{d{\overline{t}}^{\prime }}\right)}^2+{\left(\frac{d{\overline{y}}^{\prime }}{d{\overline{t}}^{\prime }}\right)}^2\right)\right]={\left(cd\overline{t}\right)}^2\left[1-\frac{1}{c^2}\left({\left(\frac{d\overline{x}}{d\overline{t}}\right)}^2+{\left(\frac{d\overline{y}}{d\overline{t}}\right)}^2\right)\right].$$

(43)

Since there always exists the local initial frame ${\overline{S}}^{\prime }$ in which $d{\overline{x}}^{\prime }/d{\overline{t}}^{\prime }=d{\overline{y}}^{\prime }/d{\overline{t}}^{\prime }=0$, we thus have the interval relation between the proper time $d\tau =d{\overline{t}}^{\prime }$ and the coordinate time $d\overline{t}$:

$$d\tau =d\overline{t}\sqrt{1-{\left(\frac{\overline{V}}{c}\right)}^2}.$$

(44)

The relation between the conformal velocity $\overline{V}$ and the velocity v is established by (4). Only when the conformal parameter $a=1$ does the relation reduce to

$$d\tau =dt\sqrt{1-{\left(\frac{v}{c}\right)}^2},$$

(45)

given in Einstein’s special relativity.

3. Equation of Motion, Momentum, and Energy

In this section, we present the equation of motion based on the conformal velocity space and discuss its relativistic effect. For this, we introduce the isometric transformation:

$${\overline{u}}^i=\frac{{\overline{v}}^i}{\sqrt{1-{(\overline{v}/c)}^2}},\mathrm{and}{\overline{u}}^0=\frac{c}{\sqrt{1-{(\overline{v}/c)}^2}}$$

(46)

for (36), which makes the line element have the representation with the dimension of time labeled by the zeroth component:

$${\left(d{s}_{\overline{v}}\right)}^2={\eta }_{\mu \nu }d{\overline{u}}^{\mu }d{\overline{u}}^{\nu },(\mu ,\nu =0,1,2),$$

(47)

where ${\eta }_{\mu \nu }$ is the Minkowski metric ${\eta }_{\mu \nu }=$ diag$(-1,1,1)$. Accordingly, one can define the (2+1)d velocity as

$${\overline{u}}^{\mu }=({\overline{u}}^0,{\overline{u}}^i)={\gamma }_{\overline{v}}(c,{\overline{v}}^i).$$

(48)

It follows that the three-force and -momentum of a particle are given by

$$f^{\mu }=\frac{d{\overline{p}}^{\mu }}{d\tau },\mathrm{and}{\overline{p}}^{\mu }=M_0{\overline{u}}^{\mu },$$

(49)

where $M_0$ is the proper mass and $\tau$ is the proper time measured from the local inertial frame of reference. According to the traditional convention, the equation of motion of a relativistic particle can be defined by

$$\overline{\mathbf{F}}=\frac{d}{d\overline{t}}\overline{\mathbf{p}}=\frac{d}{d\overline{t}}\left({\gamma }_{\overline{v}}{M}_0\overline{\mathbf{v}}\right).$$

(50)

Expanding the derivative on the right-hand side gives

$$\frac{d}{d\overline{t}}\overline{\mathbf{p}}=M_0\left[\frac{{\gamma }_{\overline{v}}^3}{c^2}\left(\overline{\mathbf{a}}·\overline{\mathbf{v}}\right)\overline{\mathbf{v}}+{\gamma }_{\overline{v}}\overline{\mathbf{a}}\right],$$

(51)

where the acceleration $\overline{\mathbf{a}}=d\overline{\mathbf{v}}/d\overline{t}$. If $\overline{\mathbf{a}}$ is perpendicular to $\overline{\mathbf{v}}$, then $\overline{\mathbf{a}}·\overline{\mathbf{v}}=0$ and $d\overline{\mathbf{p}}/d\overline{t}={\gamma }_{\overline{v}}{M}_0\overline{\mathbf{a}}$. We can thus define the transverse mass:

$$M_{\mathrm{transverse}}=\frac{d\overline{\mathbf{p}}/d\overline{t}}{\overline{\mathbf{a}}}={\gamma }_{\overline{v}}{M}_0,$$

(52)

resembling the definition of Einstein’s original paper in 1905. If $\overline{\mathbf{a}}$ is parallel to $\overline{\mathbf{v}}$, then $d\overline{\mathbf{p}}/d\overline{t}=M_0{\gamma }_{\overline{v}}\left[{\gamma }_{\overline{v}}^2({\overline{v}}^2/c^2)+1\right]\overline{\mathbf{a}}$. We have the longitudinal mass:

$$M_{\mathrm{longitudinal}}=\frac{d\overline{\mathbf{p}}/d\overline{t}}{\overline{\mathbf{a}}}={\gamma }_{\overline{v}}^3{M}_0.$$

(53)

The relativistic energy of the particle is given by

$$\overline{E}=\overline{M}{c}^2=\frac{M_0{c}^2}{\sqrt{1-{(\overline{v}/c)}^2}}.$$

(54)

The proper energy in the local inertial frame is $E_0=M_0{c}^2$. The relativistic kinetic energy in the theory is given by

$$E_k=(\overline{M}-M_0)c^2=({\gamma }_{\overline{v}}-1)M_0{c}^2.$$

(55)

The graphs in Figure 4 show the variation of the relativistic effect of $\overline{E}$ with a.

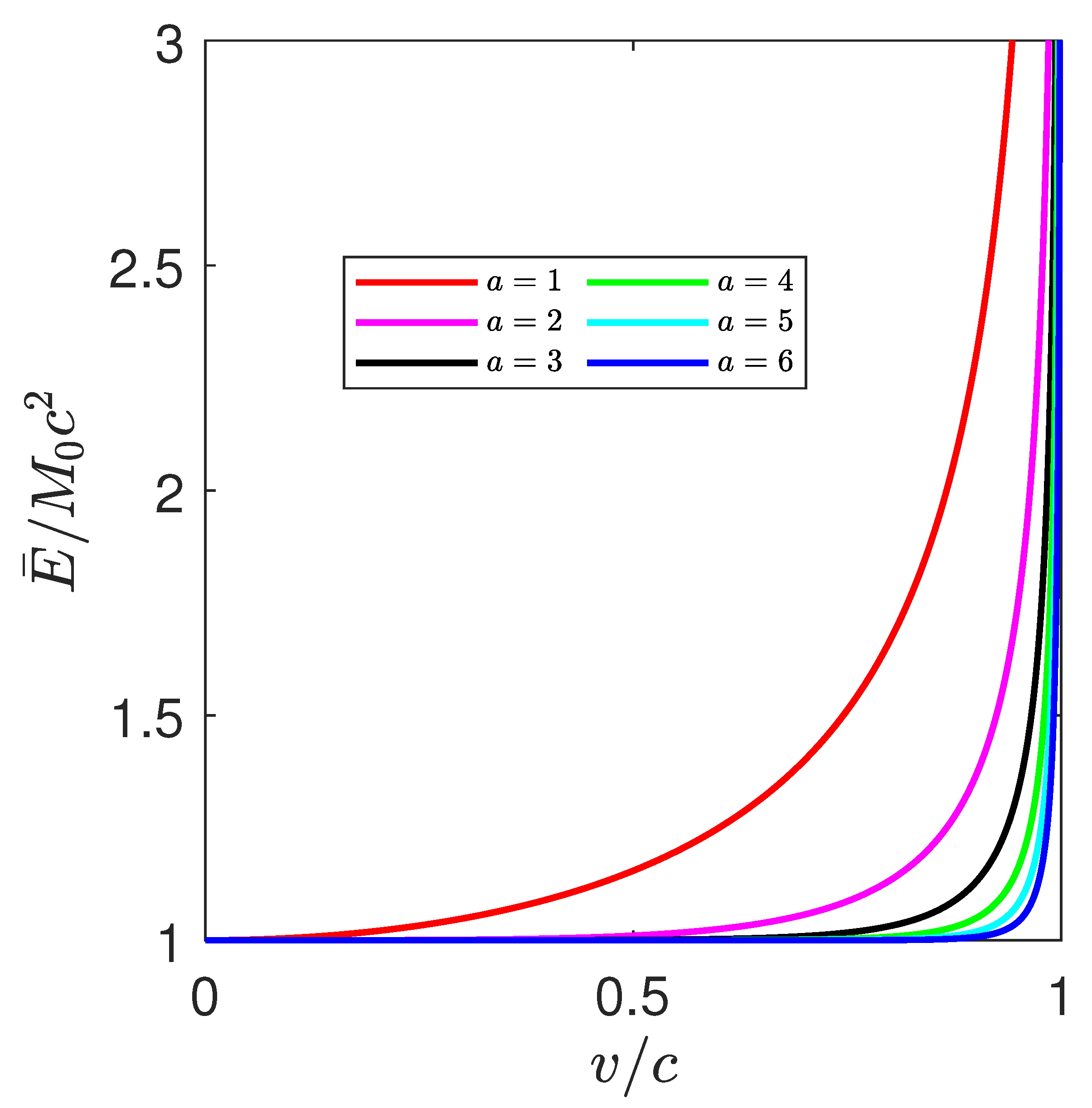
3.1. Energy–Momentum Vector and Its Transformation

Define the energy–momentum vector of the particle:

$${\overline{P}}^{\mu }=M_0{\overline{u}}^{\mu }=(\overline{E}/c,\overline{\mathbf{p}}).$$

(56)

Let ${\overline{P}}^{\prime \mu }$ be the three-vector in the local inertial frame. Since ${\overline{P}}^{\mu }{\overline{P}}_{\mu }={\overline{\mathbf{p}}}^2-{\left(\overline{M}c\right)}^2$ is an invariant quantity, we have ${\overline{\mathbf{p}}}^2-{\left(\overline{M}c\right)}^2=-M_0{c}^2$ from ${\overline{P}}^{\mu }{\overline{P}}_{\mu }={\overline{P}}^{\prime \mu }{\overline{P}}_{\mu }^{\prime }$. This results in the energy–momentum relation:

$${\overline{E}}^2=(c{\overline{\mathbf{p}})}^2+{\left(M_0{c}^2\right)}^2.$$

(57)

Assume that the inertial frame ${\overline{S}}^{\prime }$ has velocity $\overline{\mathbf{v}}=\overline{v}{\widehat{e}}_{\overline{x}}$ relative to the frame $\overline{S}$. A particle has velocity ${\overline{\mathbf{u}}}^{\prime }$ relative to the frame ${\overline{S}}^{\prime }$ and $\overline{\mathbf{u}}$ relative to $\overline{S}$. Using the relation ${\gamma }_{{\overline{u}}^{\prime }}={\gamma }_{\overline{v}}{\gamma }_{\overline{u}}(1-\overline{\mathbf{u}}·\overline{\mathbf{v}}/c^2)$, one can show that

$${\overline{p}}_x^{\prime }=\frac{M_0{\overline{u}}_x^{\prime }}{\sqrt{1-{({\overline{u}}^{\prime }/c)}^2}}=M_0{\gamma }_{{\overline{u}}^{\prime }}{\overline{u}}_x^{\prime }$$

$$={\gamma }_{\overline{v}}\left({\overline{p}}_x-\frac{\overline{v}}{c^2}\overline{E}\right),$$

(58)

and ${\overline{p}}_y^{\prime }={\overline{p}}_y$. The particle’s energies in the frames ${\overline{S}}^{\prime }$ and $\overline{S}$ are

$${\overline{E}}^{\prime }=\frac{M_0{c}^2}{\sqrt{1-{({\overline{u}}^{\prime }/c)}^2}},\mathrm{and}\overline{E}=\frac{M_0{c}^2}{\sqrt{1-{(\overline{u}/c)}^2}}.$$

(59)

Their relation is established by the transformation:

$${\overline{E}}^{\prime }={\gamma }_{\overline{v}}\left(\overline{E}-\overline{v}{\overline{p}}_x\right).$$

(60)

We then have the energy–momentum transformation:

$$\left\{\begin{array}{c}{\overline{p}}_x^{\prime }={\gamma }_{\overline{v}}\left[{\overline{p}}_x-(\overline{v}/c^2)\overline{E}\right],\hfill \\ {\overline{p}}_y^{\prime }={\overline{p}}_y,\hfill \\ {\overline{E}}^{\prime }={\gamma }_{\overline{v}}\left(\overline{E}-\overline{v}{\overline{p}}_x\right).\hfill \end{array}\right.$$

(61)

It is easy to check that the transformation satisfies the invariant form ${\overline{P}}^{\mu }{\overline{P}}_{\mu }={\overline{P}}^{\prime \mu }{\overline{P}}_{\mu }^{\prime }$.

3.2. The Relativistic Doppler Effect

Assume that a photon has the energy and momentum $\overline{E}=h\overline{\nu }=\hslash \overline{\omega }$ and $\overline{\mathbf{p}}=h/\overline{\lambda }\widehat{\mathbf{n}}=\hslash \overline{\mathbf{k}}$ with $\widehat{\mathbf{n}}$ being the unit vector in the propagation direction. The photon’s three-momentum is given by ${\overline{P}}^{\mu }=(\overline{E}/c,\overline{\mathbf{p}})=\hslash (\overline{\omega }/c,\overline{\mathbf{k}})$. It follows that the components of the wave vector can be expressed as ${\overline{k}}^{\mu }={\overline{P}}^{\mu }/\hslash =(\overline{\omega }/c,\overline{\mathbf{k}})$, which results in ${\overline{k}}^{\mu }{\overline{k}}_{\mu }=-{(\overline{\omega }/c)}^2+{\overline{\mathbf{k}}}^2=0$ since photons are massless, and we have $\overline{\lambda }\overline{\nu }=c$. The transformations of the wave vector and frequency of the photon are given by

$$\left\{\begin{array}{c}{\overline{k}}_x^{\prime }={\gamma }_{\overline{v}}\left[{\overline{k}}_x-(\overline{v}/c^2)\overline{\omega }\right],\hfill \\ {\overline{k}}_y^{\prime }={\overline{k}}_y,\hfill \\ {\overline{\omega }}^{\prime }={\gamma }_{\overline{v}}\left[\overline{\omega }-\overline{v}{\overline{k}}_x\right],\hfill \end{array}\right.$$

(62)

according to (61). Assuming that the frame ${\overline{S}}^{\prime }$ has velocity $\overline{\mathbf{v}}$ relative to the frame $\overline{S}$, the angular frequency and wave vector of a monochromatic light in ${\overline{S}}^{\prime }$ and $\overline{S}$ are $({\overline{\omega }}^{\prime },{\overline{\mathbf{k}}}^{\prime })$ and $(\overline{\omega },\overline{\mathbf{k}})$. The angles among $\overline{\mathbf{k}}$, ${\overline{\mathbf{k}}}^{\prime }$, and $\overline{\mathbf{v}}$ are

$$cos\overline{\theta }=\frac{\overline{\mathbf{v}}·\overline{\mathbf{k}}}{\overline{v}\overline{k}},\mathrm{and}cos{\overline{\theta }}^{\prime }=\frac{{\overline{\mathbf{v}}·\overline{\mathbf{k}}}^{\prime }}{\overline{v}{\overline{k}}^{\prime }}.$$

(63)

It follows from the third relation in (62) that

$${\overline{\omega }}^{\prime }={\gamma }_{\overline{v}}\overline{\omega }\left[1-(\overline{v}/c)cos\overline{\theta }\right].$$

(64)

If the source is at rest relative to ${\overline{S}}^{\prime }$, the proper frequency of the light is ${\overline{\nu }}^{\prime }={\overline{\nu }}_0$. The frequency $\overline{\nu }$ measured from the observer in the frame $\overline{S}$ is given by

$$\overline{\nu }=\frac{{\overline{\nu }}_0\sqrt{1-{(\overline{v}/c)}^2}}{1-(\overline{v}/c)cos\overline{\theta }}.$$

(65)

Several interesting cases are as follows: (i) When $\overline{\theta }=0$, the source is moving toward the observer, and the observer receives the frequency:

$$\overline{\nu }={\overline{\nu }}_0\sqrt{\frac{1+\overline{v}/c}{1-\overline{v}/c}}>{\overline{\nu }}_0,$$

(66)

which exhibits the blue shift phenomenon. Figure 5 shows how the frequency of the receiver changes with the deformation of the velocity space. (ii) When $\overline{\theta }=(2n+1)\pi$, the source moves away from the observer. The observer will receive the red shift signal:

$$\overline{\nu }={\overline{\nu }}_0\sqrt{\frac{1-\overline{v}/c}{1+\overline{v}/c}}<{\overline{\nu }}_0.$$

(67)

Figure 6 shows the effect of the conformal deformation on the phenomenon. (iii) When $\overline{\theta }=(1/2+2n)\pi$, the source executes the transverse motion relative to the observer. The observer will receive the frequency:

$$\overline{\nu }={\overline{\nu }}_0\sqrt{1-{(\overline{v}/c)}^2}<{\overline{\nu }}_0.$$

(68)

Figure 7 shows the effect of the conformal deformation on the transverse Doppler effect.

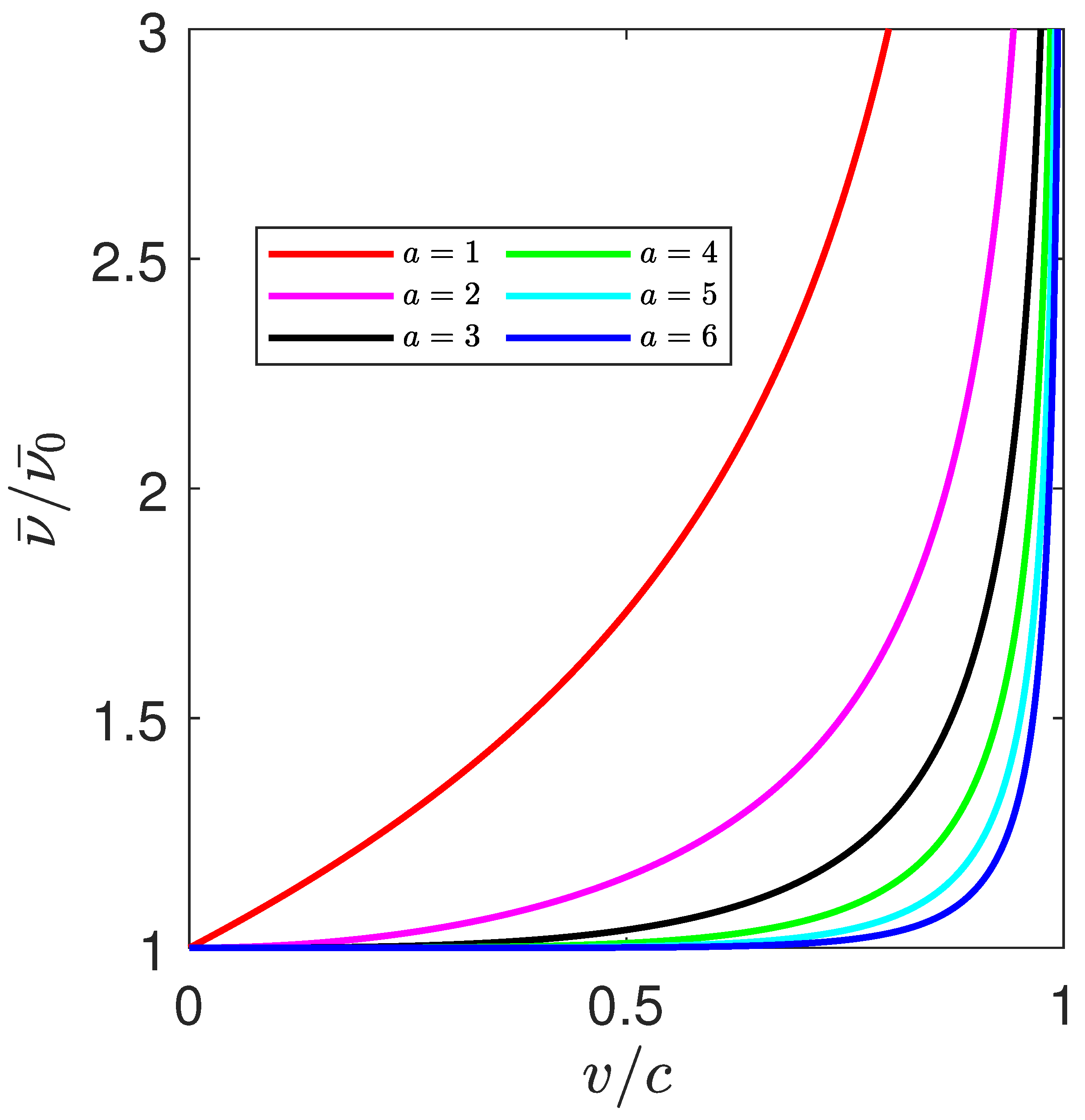
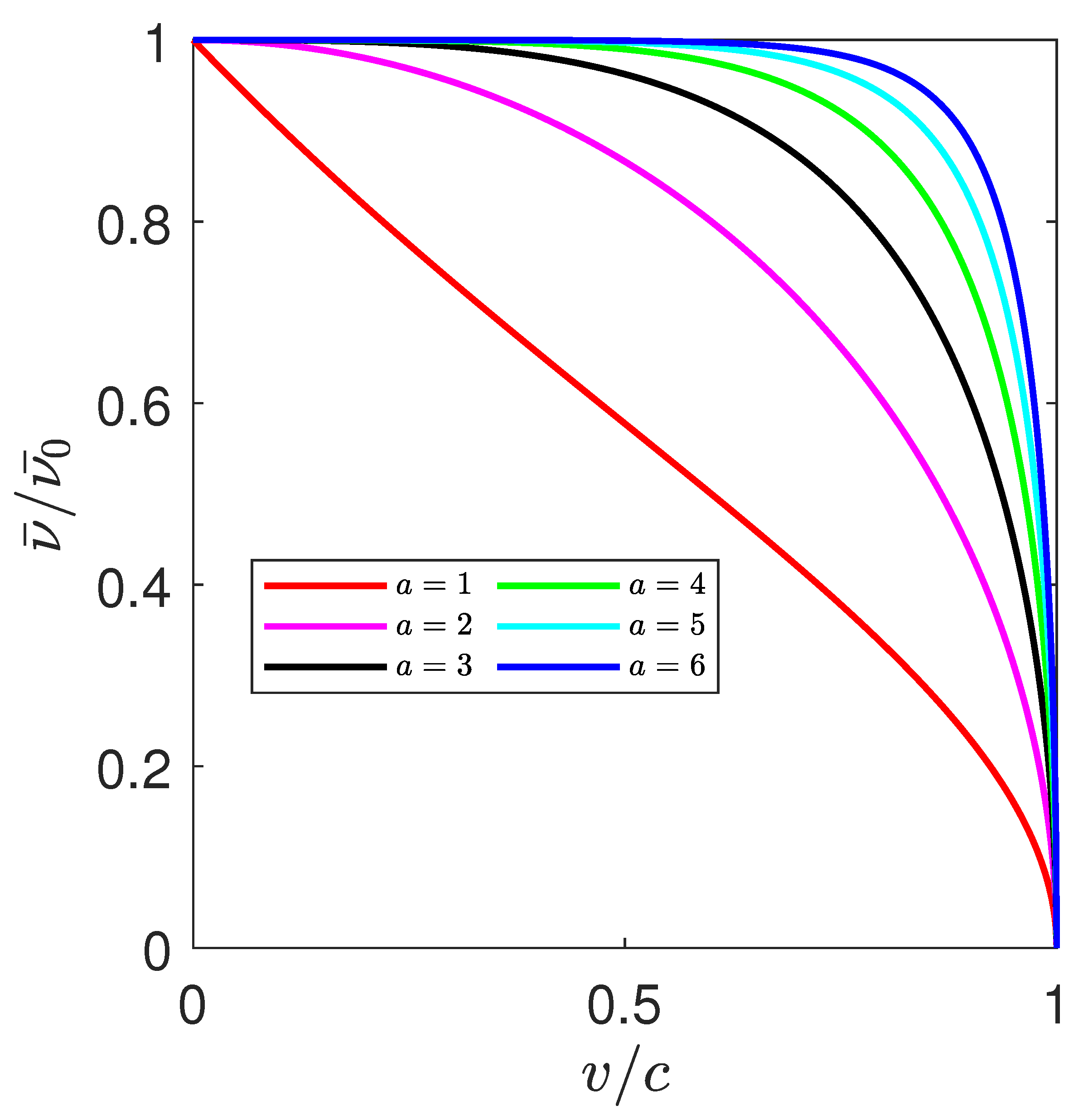
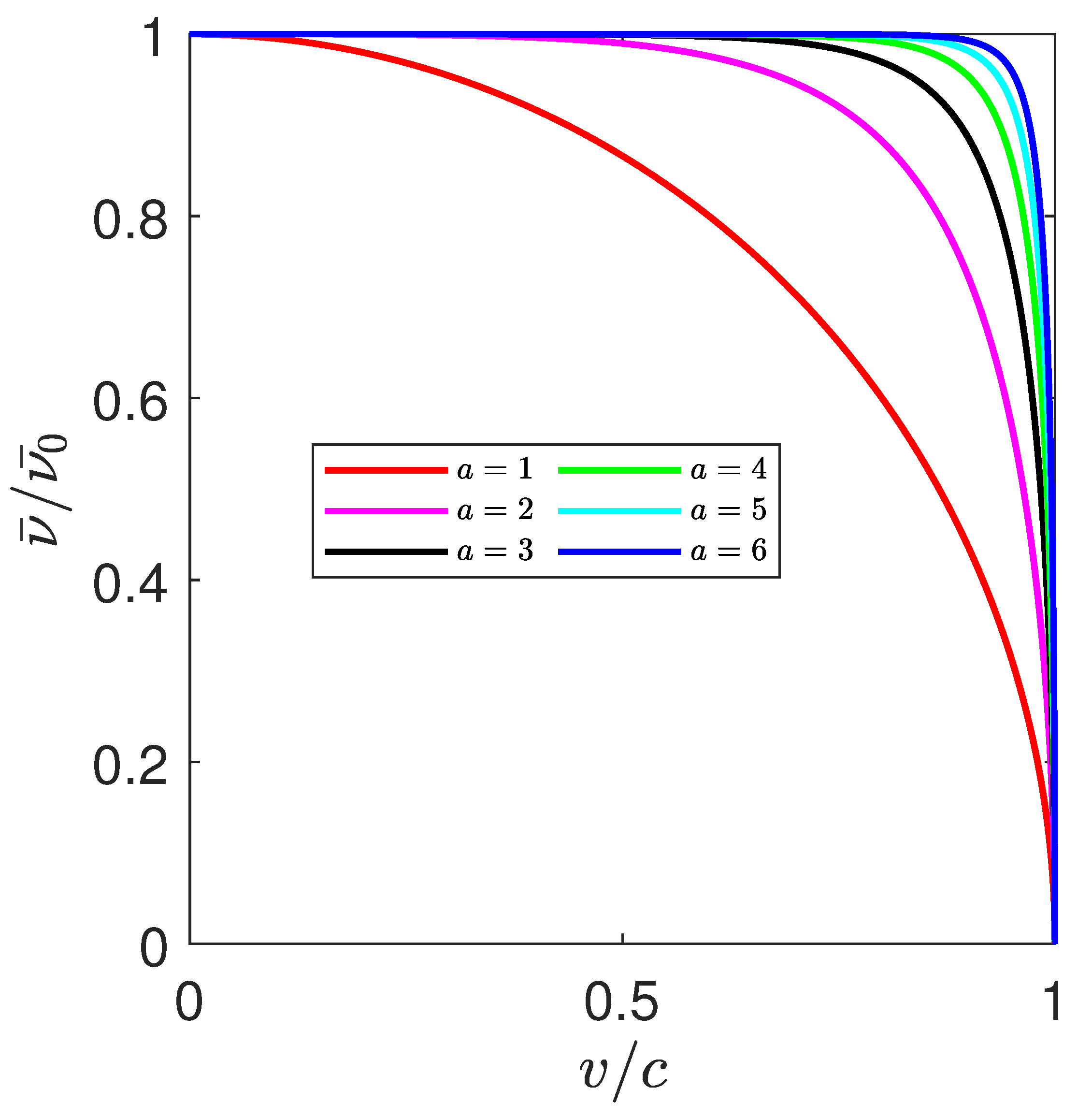
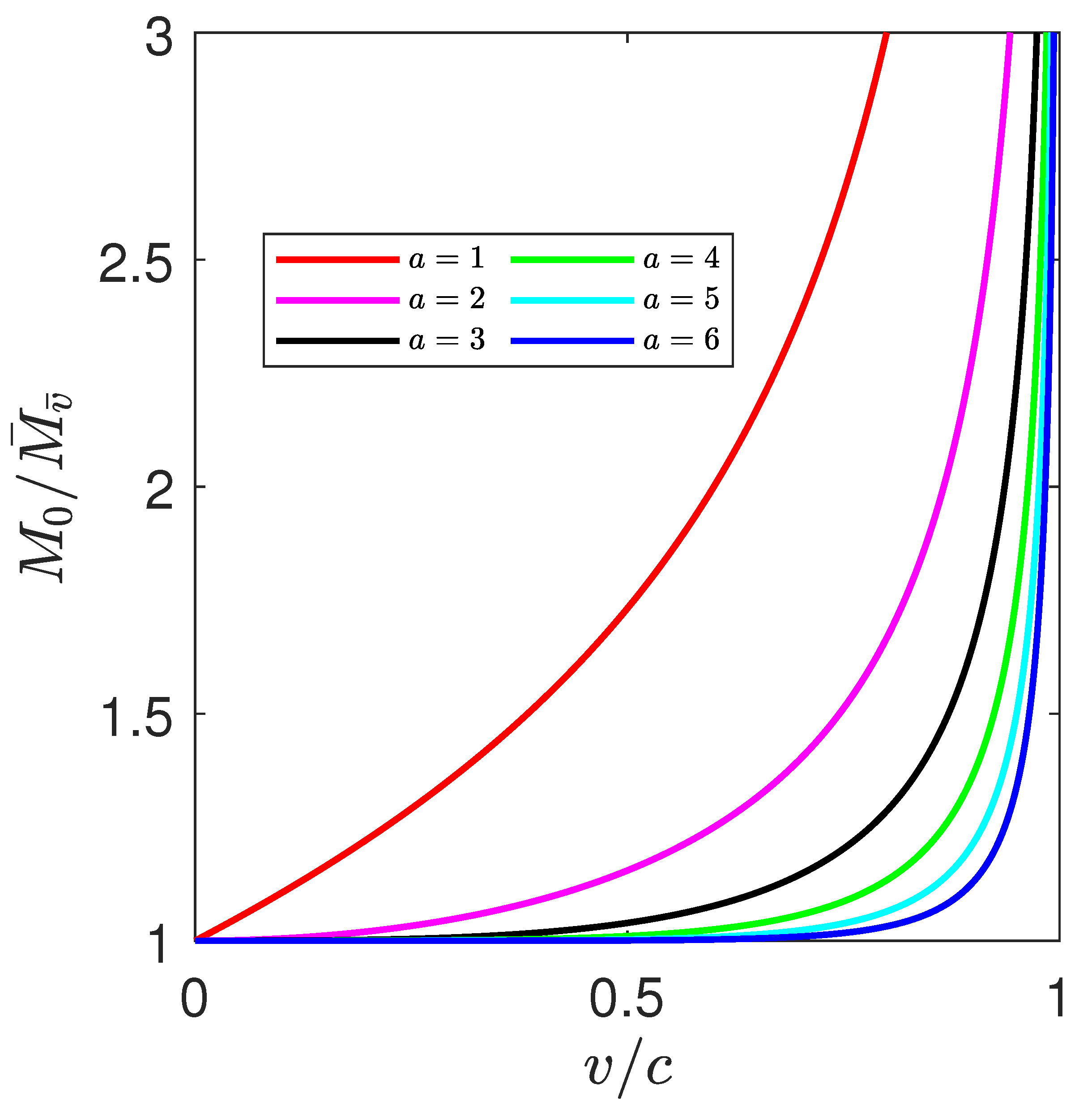
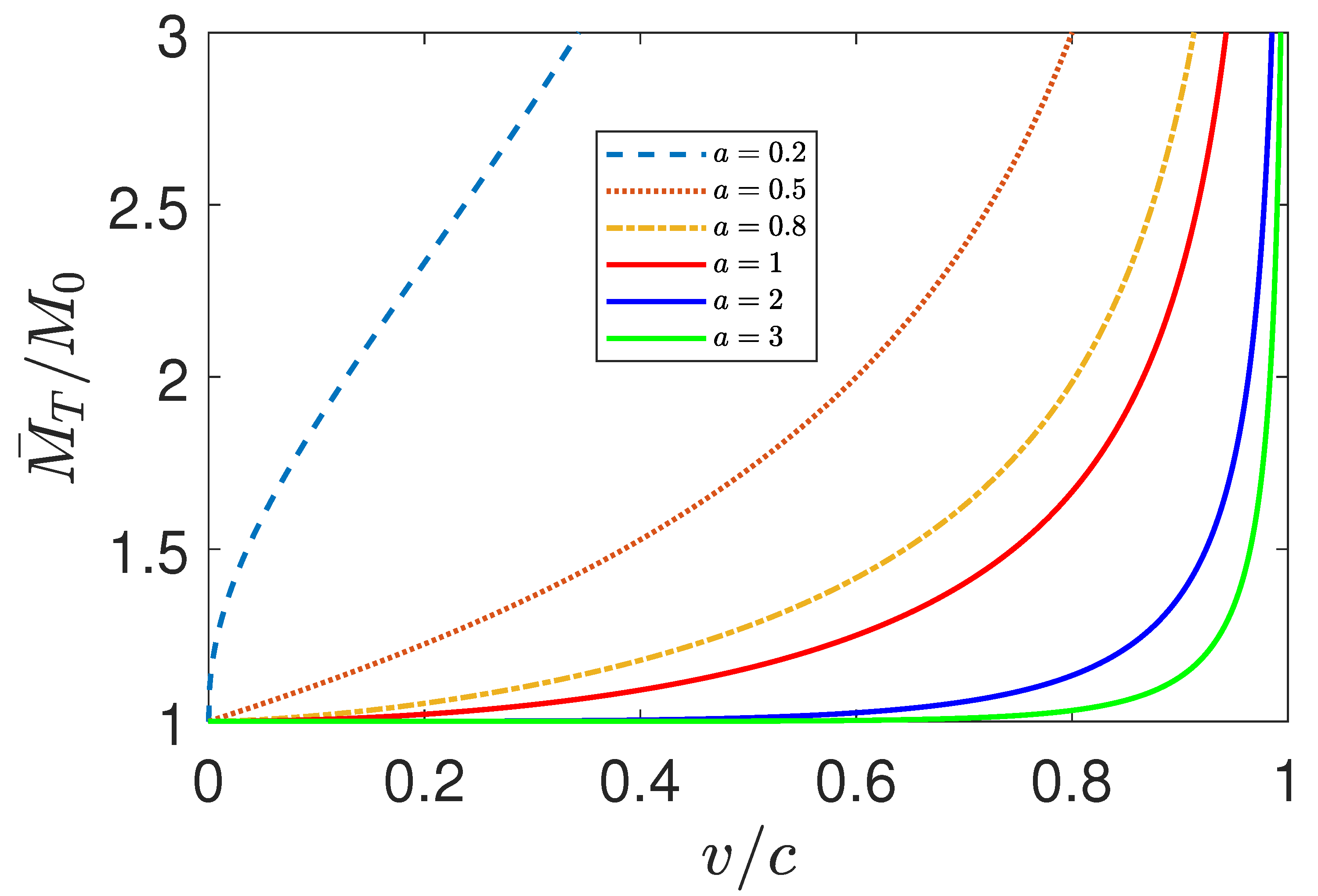
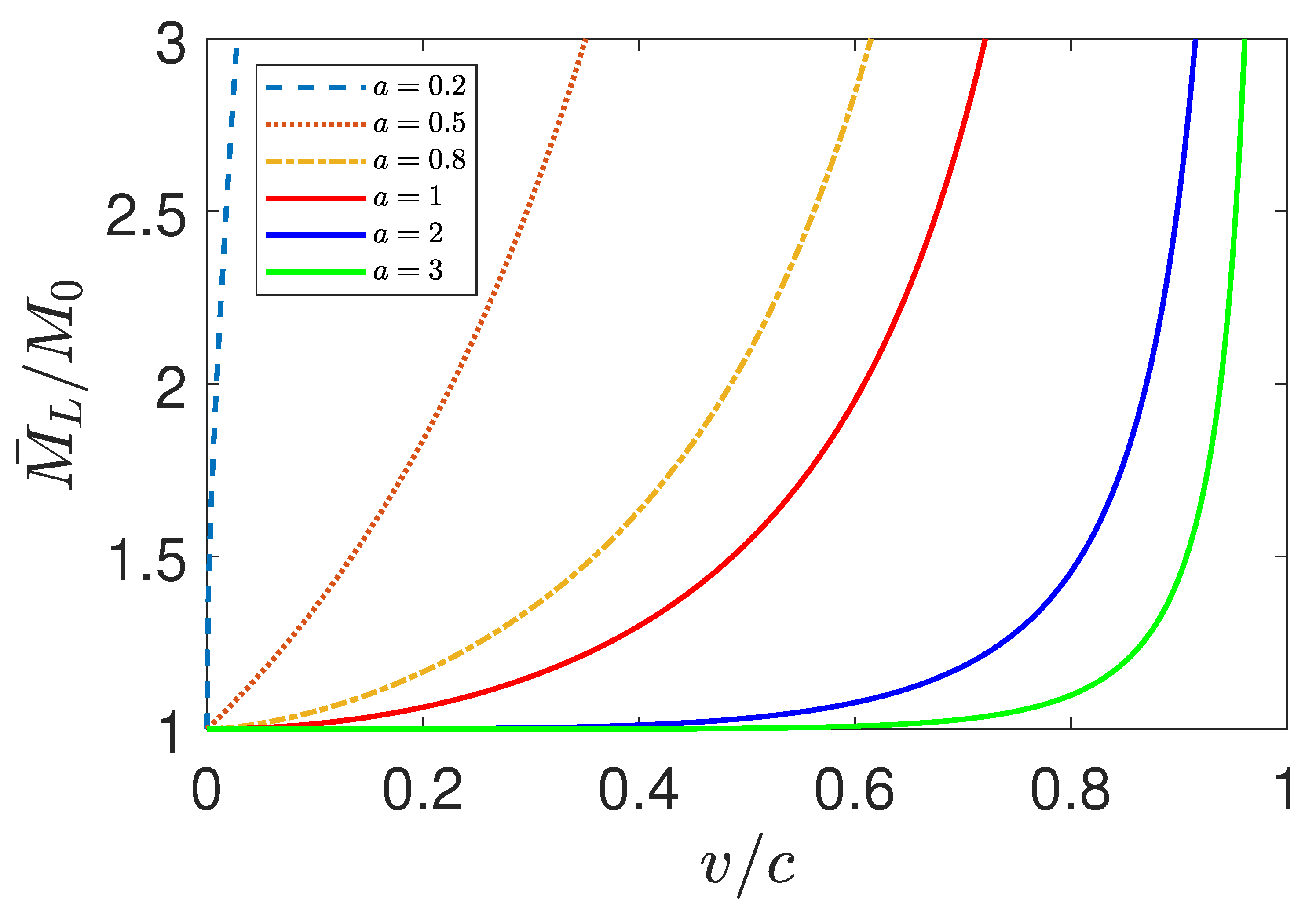
3.3. The Relativistic Rocket Equation

Assume that $M_0$ is the initial proper mass including the propellant of a rocket; ${\overline{M}}_{\overline{w}}$ is the total proper mass when the velocity of the rocket comes to $\overline{w}$; $-{\overline{u}}_r$ is the effective exhaust velocity, where the negative sign stands for the direction of the exhaust opposite the rocket. As the velocity of the rocket changes from $\overline{v}$ to $\overline{v}+d\overline{v}$, the proper mass changes from $\overline{M}$ to $\overline{M}+d\overline{M}$ ($d\overline{M}<0$). At the same time, the rocket releases the proper mass $m_0$, which has the velocity $\overline{u}$ relative to the Earth. The conservation laws of the energy and momentum for the rocket give the relations:

$$\frac{\left(\overline{M}+d\overline{M}\right)c^2}{\sqrt{1-{(\overline{v}+d\overline{v})}^2/c^2}}+\frac{m_0{c}^2}{\sqrt{1-{(\overline{u}/c)}^2}}=\frac{\overline{M}{c}^2}{\sqrt{1-{(\overline{v}/c)}^2}},$$

(69)

and

$$\frac{\left(\overline{M}+d\overline{M}\right)(\overline{v}+d\overline{v})}{\sqrt{1-{(\overline{v}+d\overline{v})}^2/c^2}}+\frac{m_0\overline{u}}{\sqrt{1-{(\overline{u}/c)}^2}}=\frac{\overline{M}\overline{v}}{\sqrt{1-{(\overline{v}/c)}^2}}.$$

(70)

They can be expressed in terms of the form:

$$d\left({\gamma }_{\overline{v}}\overline{M}\right)+{\gamma }_{\overline{u}}{m}_0=0,$$

(71)

and

$$d\left({\gamma }_{\overline{v}}\overline{M}\overline{v}\right)+{\gamma }_{\overline{u}}{m}_0\overline{u}=0.$$

(72)

The operation of the quotient (72)/(71) gives the ratio of the mass change:

$$\frac{d\overline{M}}{\overline{M}}=\left[\frac{1}{\overline{u}-\overline{v}}-{\gamma }_{\overline{v}}^2\frac{\overline{v}}{c^2}\right]d\overline{v}.$$

(73)

Using the Lorentz velocity transformation:

$$\overline{u}=\frac{-{\overline{u}}_r+\overline{v}}{1+(-{\overline{u}}_r)\overline{v}/c^2},$$

(74)

the representation is reduced to become

$$\frac{d\overline{M}}{\overline{M}}=-\frac{{\gamma }_{\overline{v}}^2}{{\overline{u}}_r}d\overline{v}.$$

(75)

By performing the integration ${\int }_{M_0}^{{\overline{M}}_{\overline{v}}}d\overline{M}/\overline{M}$, it is found that

$$\frac{M_0}{{\overline{M}}_{\overline{v}}}={\left(\frac{c+\overline{v}}{c-\overline{v}}\right)}^{c/2{\overline{u}}_r}.$$

(76)

This is the Ackeret formula [22] for the relativistic rocket equation in the conformal relativity. The final velocity can be expressed as

$$\frac{\overline{v}}{c}=\frac{{(M_0/{\overline{M}}_{\overline{v}})}^{2{\overline{u}}_r/c}-1}{{(M_0/{\overline{M}}_{\overline{v}})}^{2{\overline{u}}_r/c}+1}.$$

(77)

It exhibits the relation among the final velocity of the rocket, the exhaust velocity, and the ratio of the initial and final masses. Figure 8 shows how the mass ratio of a photon rocket is subjected to the conformal deformation. It is seen that the rocket in the relativity with the larger index n would have less deviation from the classical outcome. When $\overline{v}<<c$, the formula reduces to

$$\overline{v}={\overline{u}}_{r}ln\frac{M_0}{{\overline{M}}_{\overline{v}}}$$

or

$$\frac{M_0}{{\overline{M}}_{\overline{v}}}=exp\left(\frac{\overline{v}}{{\overline{u}}_r}\right).$$

(78)

This is the Tsiolkovsky formula for the low-speed rocket with conformal freedom.

4. Thomas Precession in the Conformal Velocity Space

Thomas precession is a purely kinematic effect (e.g., [23,24]). Thus, it reflects the geometric attribute of the physical space. An elegant way to comprehend and obtain the precession angle is to evaluate the angular defect of a closed path in the velocity space by the Gauss–Bonnet theorem [7,8,9,10]. Following the consideration, we explore the precession by the theorem in the conformal velocity space. The angular defect of a geodesic triangle is defined by $\Delta \phi =\pi -(\phi +\alpha +\beta )$, where $\phi ,$$\alpha ,$ and $\beta$ are the inner angles of the considered triangle illustrated in Figure 2. According to the Gauss–Bonnet theorem, it is related to the curvature K and the area S of the corresponding region by

$${\displaystyle \Delta \phi =-\int \int KdS=\frac{S}{c^2}.}$$

(79)

For our consideration of the line element (3), the area is given by

$${\displaystyle S=\int \int \sqrt{g}d\overline{v}d\overline{\theta }={\int }_{{\overline{\theta }}_1}^{{\overline{\theta }}_2}{\int }_0^{\overline{v}\left(\overline{\theta }\right)}{\gamma }_{\overline{v}}^3\overline{v}d\overline{v}d\overline{\theta }=c^2{\int }_{{\overline{\theta }}_1}^{{\overline{\theta }}_2}({\gamma }_{\overline{v}}-1)d\overline{\theta }.}$$

(80)

The expression of the angular defect follows:

$$\Delta \phi ={\int }_{{\overline{\theta }}_1}^{{\overline{\theta }}_2}({\gamma }_{\overline{v}}-1)d\overline{\theta }.$$

(81)

From (15), if the segment $\overline{O{P}_0}$ connecting the origin and the point $P_0({\overline{v}}_0,{\overline{\theta }}_0)$ on a geodesic is the shortest distance from the geodesic to the origin, i.e., $D={\overline{v}}_0$, the coordinates $(\overline{v},\overline{\theta })$ of the geodesic can be expressed as

$$\overline{v}\left(\overline{\theta }\right)=\frac{{\overline{v}}_0}{cos(\overline{\theta }-{\overline{\theta }}_0)}.$$

(82)

Consider the geodesic triangle with a vertex at O in the velocity space shown in Figure 2. The points $P_1$ and $P_2$ have the coordinates $({\overline{v}}_a,{\overline{\theta }}_a)$ and $({\overline{v}}_b,{\overline{\theta }}_b)$. The angular defect of the triangle has the expression:

$$\Delta \phi ={\int }_{{\overline{\theta }}_a}^{{\overline{\theta }}_b}\left[\frac{cos(\overline{\theta }-{\overline{\theta }}_0)}{\sqrt{{cos}^2(\overline{\theta }-{\overline{\theta }}_0)-{({\overline{v}}_0/c)}^2}}-1\right]d\overline{\theta }.$$

(83)

By employing the formula ([25], p. 198):

$$\int \frac{cosx}{\sqrt{1-k^2{sin}^{2}x}}dx=\frac{1}{k}{sin}^{-1}(ksinx),$$

(84)

the precession angle is found to be

$$\Delta \phi ={sin}^{-1}\left({\gamma }_{0}sin{\overline{\phi }}_b\right)-{sin}^{-1}\left({\gamma }_{0}sin{\overline{\phi }}_a\right)-\overline{\phi },$$

(85)

where ${\gamma }_0=1/\sqrt{1-{({\overline{v}}_0/c)}^2}$, ${\overline{\phi }}_b={\overline{\theta }}_b-{\overline{\theta }}_0$, ${\overline{\phi }}_a={\overline{\theta }}_a-{\overline{\theta }}_0$, and $\overline{\phi }={\overline{\theta }}_b-{\overline{\theta }}_a$. Using the relation between the inverse sine functions ([25], p. 57):

$${sin}^{-1}x-{sin}^{-1}y={sin}^{-1}\left[x\sqrt{1-y^2}-y\sqrt{1-x^2}\right],$$

(86)

we obtain the expression for the precession angle:

$$sin\left(\Delta \phi +\overline{\phi }\right)={\gamma }_0^2\left[sin{\overline{\phi }}_{b}cos{\overline{\phi }}_a\sqrt{1-{({\overline{v}}_a/c)}^2}-sin{\overline{\phi }}_{a}cos{\overline{\phi }}_b\sqrt{1-{({\overline{v}}_b/c)}^2}\right],$$

(87)

where $cos{\overline{\phi }}_a={\overline{v}}_0/{\overline{v}}_a$ and $cos{\overline{\phi }}_b={\overline{v}}_0/{\overline{v}}_b$. This can also be expressed as

$$sin\left(\Delta \phi +\overline{\phi }\right)=\frac{{\gamma }_0{\overline{v}}_0}{c{\overline{v}}_a{\overline{v}}_b}\left[\sqrt{(c^2-{\overline{v}}_a^2)({\overline{v}}_b^2-{\overline{v}}_0^2)}-\sqrt{(c^2-{\overline{v}}_b^2)({\overline{v}}_a^2-{\overline{v}}_0^2)}\right].$$

(88)

The shape was found in [10]. Here, it contains the information of the deformed velocity when two postulations of special relativity are maintained. When ${\overline{\mathbf{v}}}_a$ and ${\overline{\mathbf{v}}}_b$ only have an infinitesimal difference, say ${\overline{\mathbf{v}}}_a=(\overline{v},\overline{\theta })$, and ${\overline{\mathbf{v}}}_b=(\overline{v}+d\overline{v},\overline{\theta }+d\overline{\theta })$, the attribute of the hyperbolic geometry of the considered area reduces to the attribute of the Euclidean geometry. We can always choose the reference frame such that $\overline{v}={\overline{v}}_0$. It follows that

$$(\Delta \phi +\overline{\phi })\to (d\phi +d\overline{\theta })={\gamma }_{\overline{v}}^2\sqrt{1-{(\overline{v}/c)}^2}d\overline{\theta }={\gamma }_{\overline{v}}d\overline{\theta },$$

(89)

i.e.,

$$d\phi =({\gamma }_{\overline{v}}-1)d\overline{\theta }.$$

(90)

Since

$$d\overline{\theta }\approx sind\overline{\theta }=\frac{|(\overline{\mathbf{v}}+d\overline{\mathbf{v}})\times \overline{\mathbf{v}}|}{{\overline{v}}^2}=\frac{|d\overline{\mathbf{v}}\times \overline{\mathbf{v}}|}{{\overline{v}}^2},$$

(91)

the precession angle with the direction is found to be

$$d\mathit{\phi }=({\gamma }_{\overline{v}}-1)\frac{d\overline{\mathbf{v}}\times \overline{\mathbf{v}}}{{\overline{v}}^2}=\frac{{\gamma }_{\overline{v}}^2(d\overline{\mathbf{v}}\times \overline{\mathbf{v}})}{c^2({\gamma }_{\overline{v}}+1)},$$

(92)

and the precession velocity is

$$\omega =\frac{d\mathit{\phi }}{d\overline{t}}=\frac{{\gamma }_{\overline{v}}^2(\overline{\mathbf{a}}\times \overline{\mathbf{v}})}{c^2({\gamma }_{\overline{v}}+1)},$$

(93)

with $\overline{\mathbf{a}}=d\overline{\mathbf{v}}/d\overline{t}$. The representation retains form invariance (e.g., [10]). However, it would change with the index a since it contains additional information about the conformal deformation. An interesting spacial case is that an object executes circular motion with a uniform velocity. The motion corresponds to a circular trajectory with $\overline{v}=$ constant in the velocity space. The accumulated angle due to the Thomas precession is given by

$${\displaystyle \Delta \phi =\oint ({\gamma }_{\overline{v}}-1)d\overline{\theta }=2\pi a({\gamma }_{\overline{v}}-1)}$$

(94)

when $\theta$ changes from 0 to $2\pi$. The graphs in Figure 9 show the influence of the conformal deformation on the angle. The theories with the higher index n exhibit their precession effects only in the higher-speed region. If we adopt the hyperbolic coordinate $\overline{w}$ in (9) to represent ${\gamma }_{\overline{v}}$ such that $cosh(\overline{w}/c)={\gamma }_{\overline{v}}$, the angle becomes

$$\Delta \phi =2\pi a\left[cosh(\overline{w}/c)-1\right].$$

(95)

It represents the precession angle accumulated around a closed path on the conformal hyperbolic velocity surface. This is similar to the Berry phase of an electron’s spin accumulated around a closed circle on the conformal Bloch sphere [26]. Both the angles of the Thomas precession and Berry phase have the constant curvature as their geometric origin.

5. Conclusions

Exploring conformal deformations upon the $2d$ relativistic velocity space for the (2+1)d special relativity, we find that the deformations that leave the velocity space metric form-invariant correspond to the generalized Lorentz transformations that are morphed by a global rescaling of the polar angle and characterized by a topological integral index. The generalized Lorentz transformations are akin to the ordinary Lorentz transformation in the sense that they keep the two fundamental principles of special relativity intact.

To illustrate the relativistic effects and affirm that they remain in the same forms of the standard special relativity, we investigated the Doppler effect, the Planck photon rocket, and the Thomas precession. We made a few notable observations, listed as follows:

(1) Our analysis revealed that there exist many different theories of special relativity in (2+1) dimensions, each of which is characterized by an integral index $a=n$. They all retain the following features of special relativity: (i) The speed of light c is the maximum limit of all possible velocities. (ii) The speed of light is constant under any generalized Lorentz transformations. (iii) All the physical laws remain form-invariant. (iv) The principle of relativity still holds true in regard to the generalized Lorentz transformations. The integral index n controls the deviation of the relativistic effects from those of Einstein’s special relativity and the Newtonian mechanics. Figure 10 and Figure 11 show the relativistic effects of the transverse and longitudinal masses in response to different values of n. A few unphysical curves given with fractional values of n are also presented for comparison. The theory with a higher value of n yields results less deviated from those of the Newtonian mechanics. Most intervals meet the Newtonian mechanics when $n>3$. Einstein’s special relativity, corresponding to the index $n=1$, exhibits the maximum deviation among all physically acceptable theories.

(2) It remains unclear whether our analysis of the (2+1)d special relativity with the conformal deformation can be generalized to (3+1)d, since a 3d relativistic velocity space endowed with the metric of a form-invariant line element has not been obtained so far.

(3) The Thomas precession in view of the relativistic velocity bears a close geometric resemblance to the Berry phase. Here, we illustrate this aspect from the perspective of gauge field theory. The precession angle accumulated around a closed geodesic loop in the relativistic space is given by

$$\Delta \phi =-\int \int KdS$$

(96)

according to the Gauss–Bonnet theorem. Here, the curvature K for a $2d$ surface with the orthogonal metric $g_{ij}=$ diag$(g_{11},g_{22})$ can be calculated by

$$K=-\frac{1}{2\sqrt{g}}\left[{\partial }_1\left(\frac{1}{\sqrt{g}}{\partial }_1{g}_{22}\right)+{\partial }_2\left(\frac{1}{\sqrt{g}}{\partial }_2{g}_{11}\right)\right].$$

(97)

Define the components of a gauge field:

$$A_1=\left(\frac{1}{2\sqrt{g}}{\partial }_2{g}_{11}\right),\mathrm{and}{A}_2=-\left(\frac{1}{2\sqrt{g}}{\partial }_1{g}_{22}\right).$$

(98)

Thomas precession can be expressed as

$$\Delta \phi =\int \int F_{12}d{\overline{v}}^{1}d{\overline{v}}^2,$$

(99)

where the field strength $F_{12}={\partial }_1{A}_2-{\partial }_2{A}_1$. For our consideration of the $2d$ relativistic velocity space (3), the field strength is given by

$$F_{12}=F_{\overline{v}\overline{\theta }}={\partial }_{\overline{v}}{A}_{\overline{\theta }}-{\partial }_{\overline{\theta }}{A}_{\overline{v}}=\frac{\overline{v}/c^2}{{\left[1-{\left(\overline{v}/c\right)}^2\right]}^{3/2}}.$$

(100)

We thus have the precession angle

$$\Delta \phi =\int \int F_{\overline{v}\overline{\theta }}d\overline{v}d\overline{\theta }={\int }_{{\overline{\theta }}_1}^{{\overline{\theta }}_2}({\gamma }_{\overline{v}}-1)d\overline{\theta }.$$

(101)

The gauge field corresponding to $\Delta \phi$ can be chosen as

$$\mathbf{A}=({\gamma }_{\overline{v}}-1){\widehat{e}}_{\overline{\theta }},$$

(102)

where ${\widehat{e}}_{\overline{\theta }}$ is the unit vector along the coordinate $\overline{\theta }$. The gauge field $\mathbf{A}$ possesses singular points at the boundary, and the zero point is at the origin of the Klein model of the velocity space. Compared with the magnetic monopole field as the source of the Berry phase of a spinor in the parameter space [26,27], the angle of the Thomas precession may be viewed as a nonintegrable phase caused by the vector potential in the $(\overline{v},\overline{\theta })$ plane.

(4) Although the modification of special relativity resulting from conformal deformations is purely a theoretical consideration, it may still bear particular relevance to some unsolved problems in general relativity. In the asymptotically flat region far away from all sources of the gravitational field, the symmetry of spacetime exhibits not only the Poincaré group, but also the extra part known as the Bondi–Metzner–Sachs (BMS) group. That is, the spacetime symmetry in general relativity does not simply reduce to the Poincaré group in the asymptotically flat limit, but also exhibits the BMS group, which corresponds to the symmetry of transformation beyond Einstein’s special relativity. As the two fundamental principles of special relativity—the principle of relativity and the principle of the constancy of the speed of light—remain intact in the modified theories revealed in this paper, it strongly suggests that the extra structure characterized by the integral index n may be related to the BMS group of the asymptotic symmetries. The study of this paper provides preliminary results showing that the BMS group may be understood in terms of conformal deformation upon the relativistic velocity space.