Quantum Explosions of Black Holes and Thermal Coordinates
Abstract
1. Introduction
2. Exponential Coordinates
2.1. Kruskal Coordinates
2.2. E-Coordinates for the Schwarzschild Metric
2.3. Temperature of Schwarzschild Black Holes in E-Coordinates
3. E-Coordinates in Minkowski Space
3.1. Minkowski Space in Terms of New Coordinates and
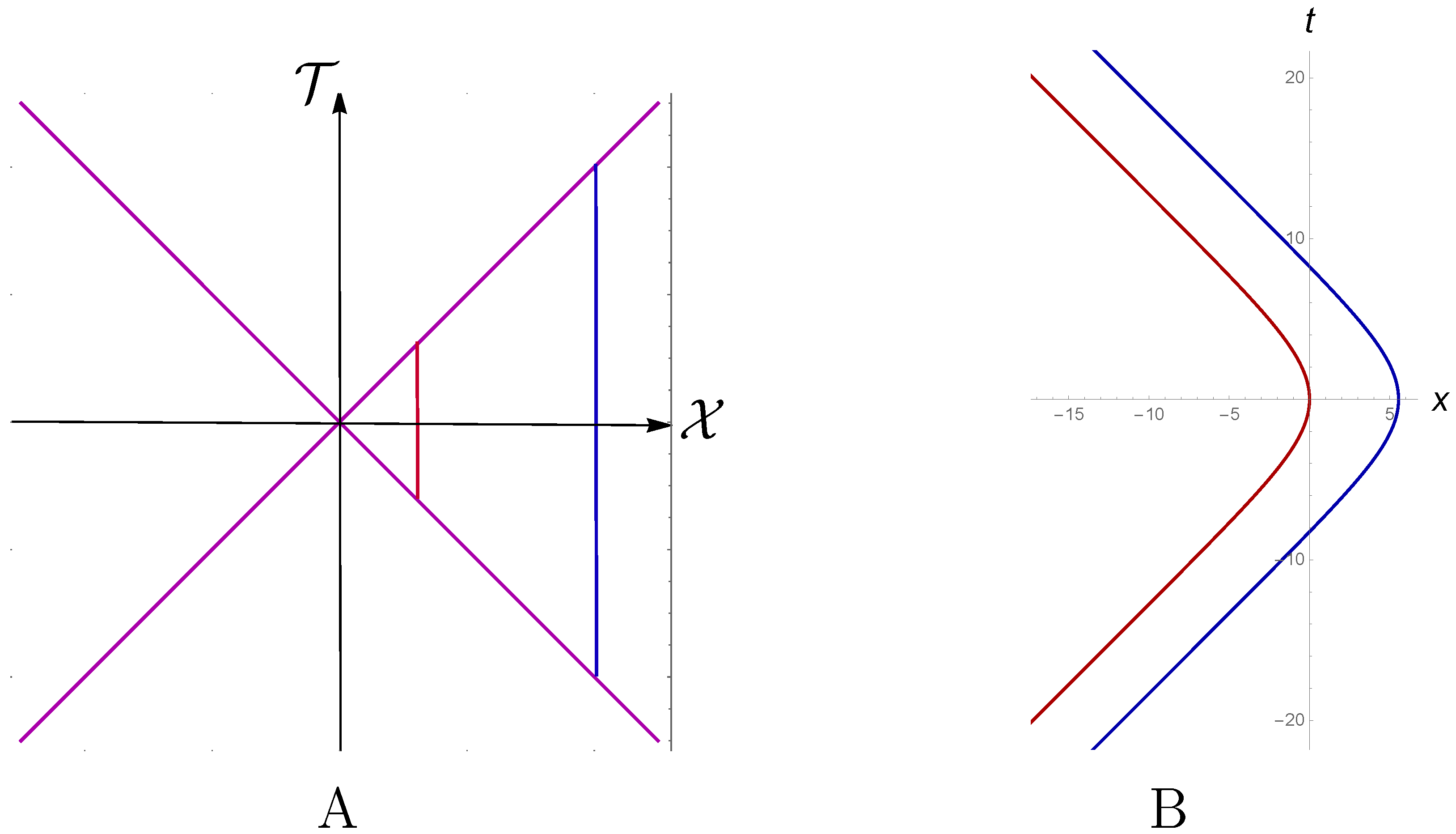
3.2. Geodesics in E-Coordinates
- . In this case, both “ends” of the geodesics are in infinities; see, for example, the plot in Figure 2A.
- . In this case, one “end” of the geodesics is at infinity and the second one is at zero; see, for example, the plot in Figure 2B.
- or . In this case, the geodesics are bounded by the characteristics or ; see Figure 3.
3.3. Acceleration in E-Coordinates
3.4. Comparison of Exponential and Rindler Coordinates
4. General E-Coordinates
4.1. E-Coordinates
4.2. Acceleration of the E-Observer in Black Holes
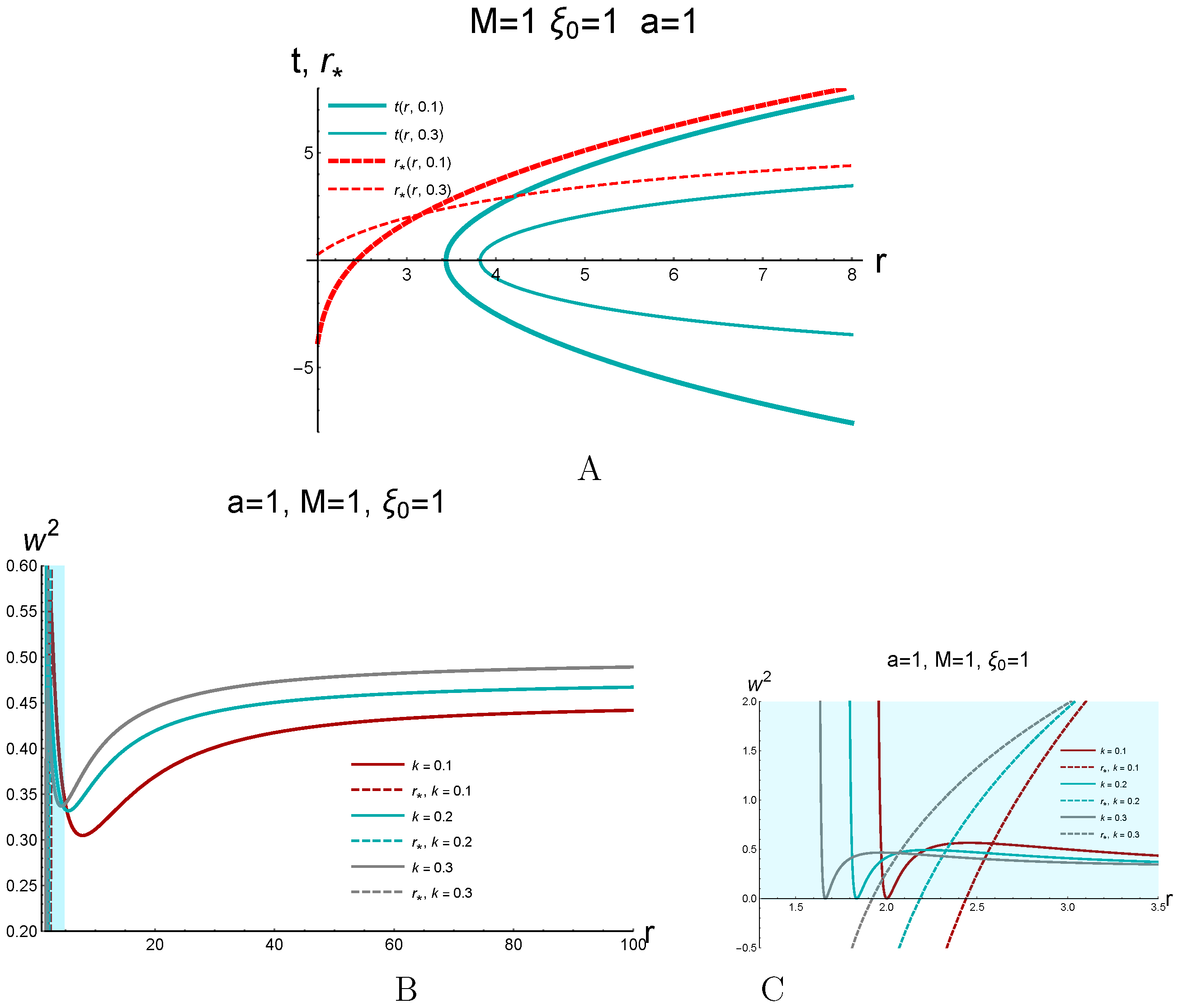
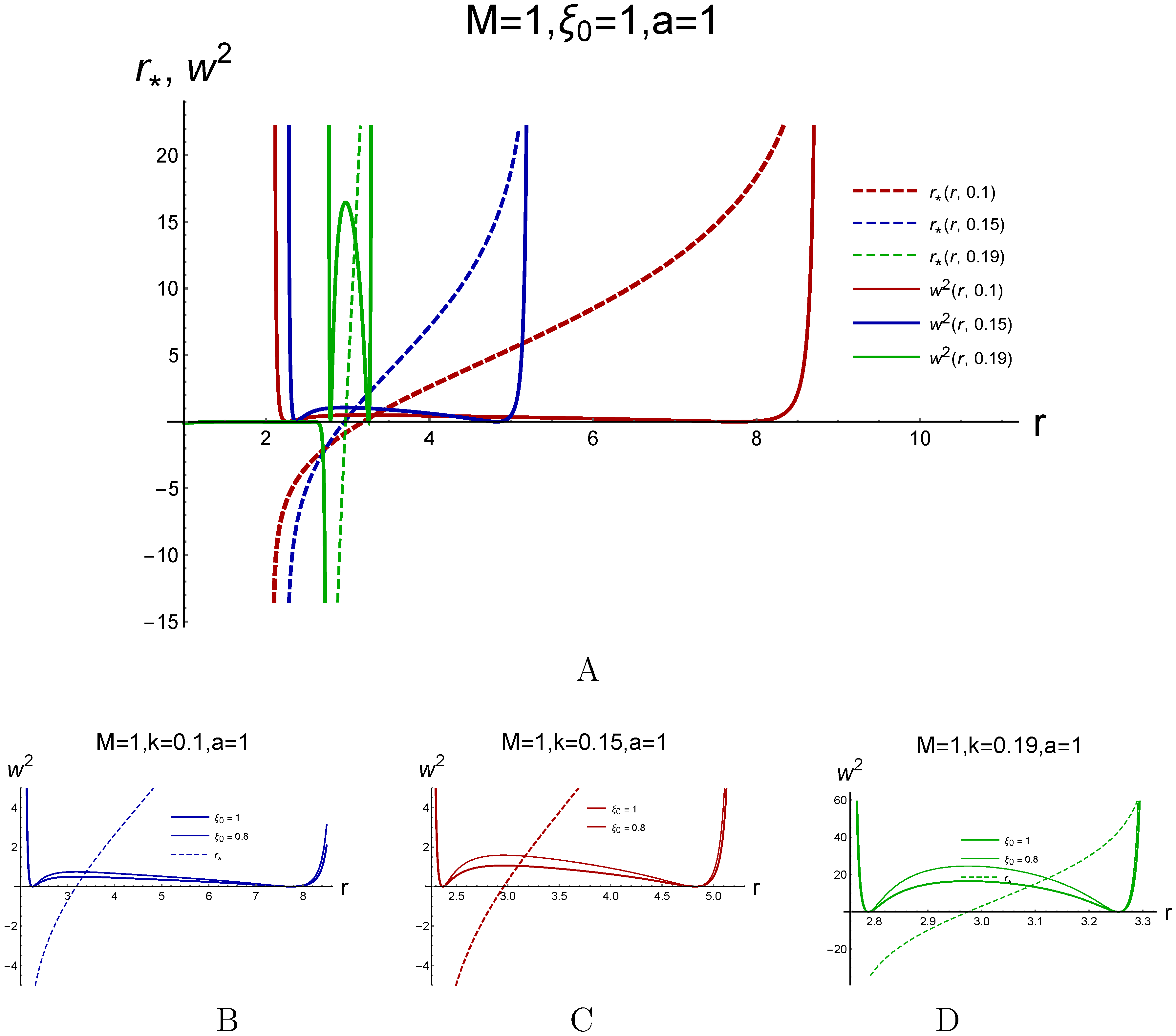
4.3. Examples: Black Holes in E-Coordinates
4.3.1. E-Observer in the Schwarzschild Black Hole
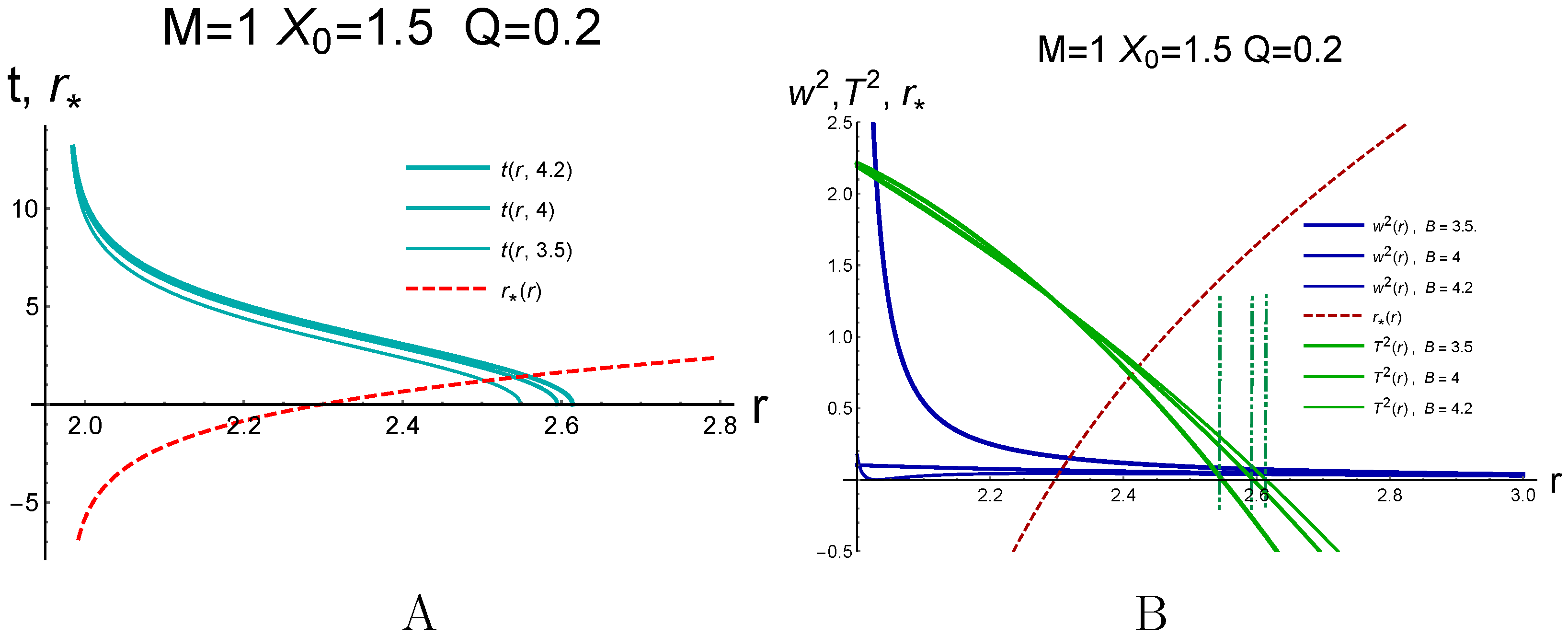
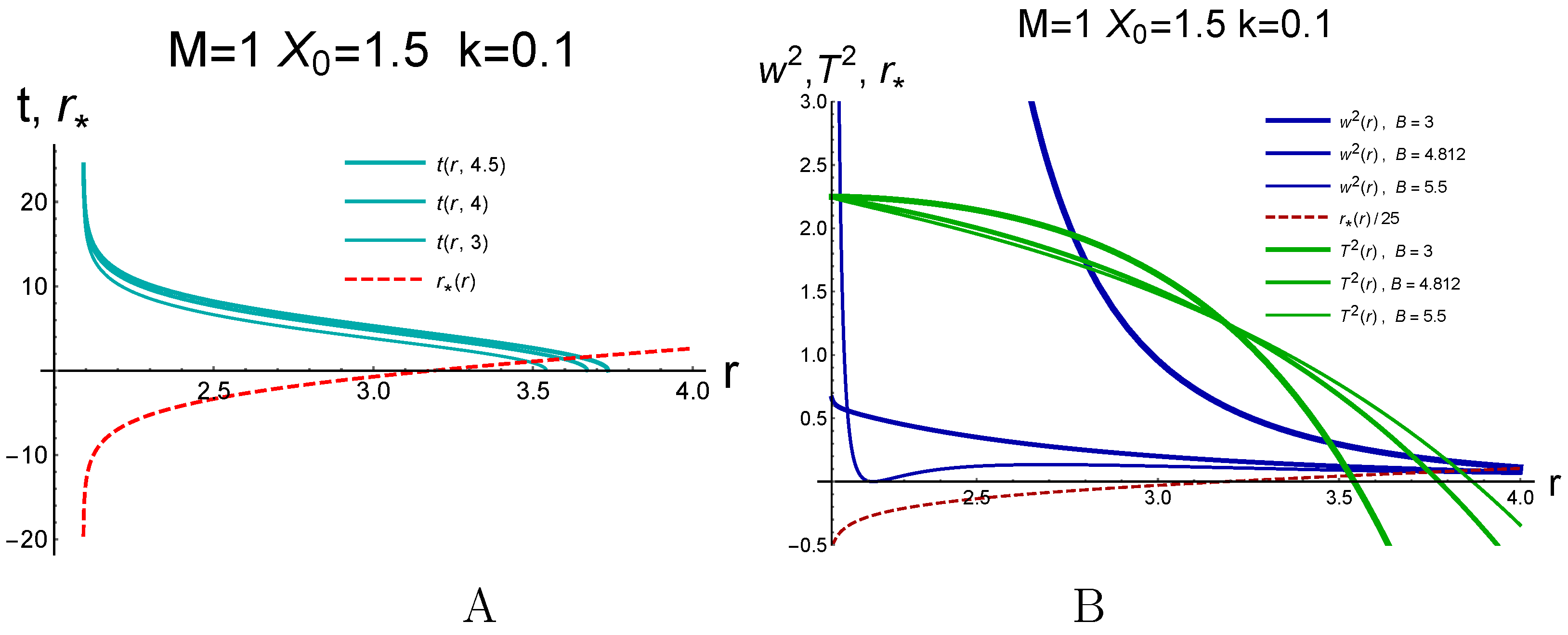
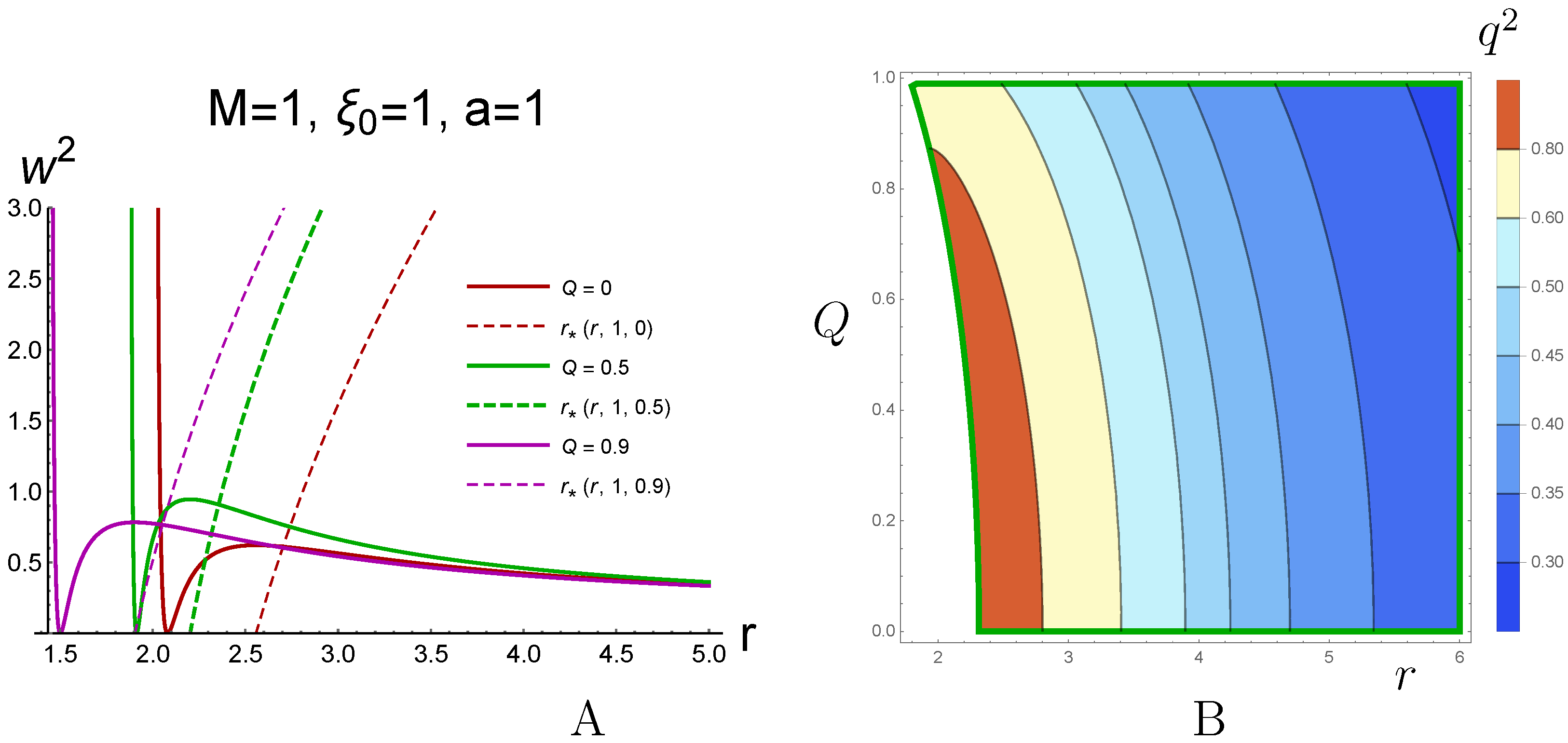
4.3.2. E-Observer in the Reissner–Nordstrom Black Hole
4.3.3. E-Observer in Schwarzschild-AdS
4.3.4. E-Observer in Schwarzschild-dS
5. General L-Coordinates
5.1. L-Coordinates
5.2. Acceleration along Trajectories in Black Hole Backgrounds
5.3. Examples
5.3.1. Schwarzschild Metricin L-Coordinates
5.3.2. Reissner–Nordstrom Metricin L-Coordinates
5.3.3. Schwarzschild-AdS Metricin L-Coordinates
5.3.4. Schwarzschild-dS in L-Coordinates
5.4. Temperature in L-Coordinates
6. Characteristic Times
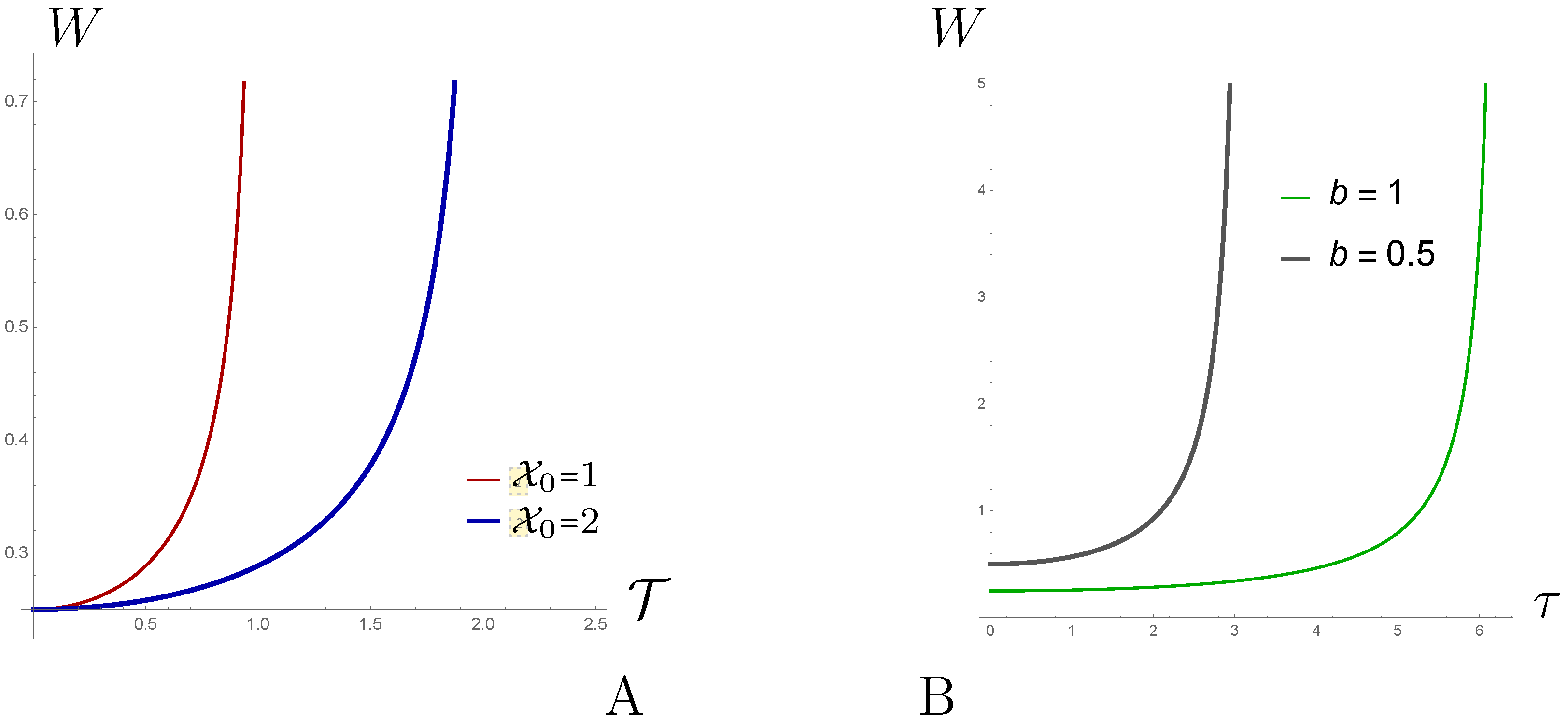
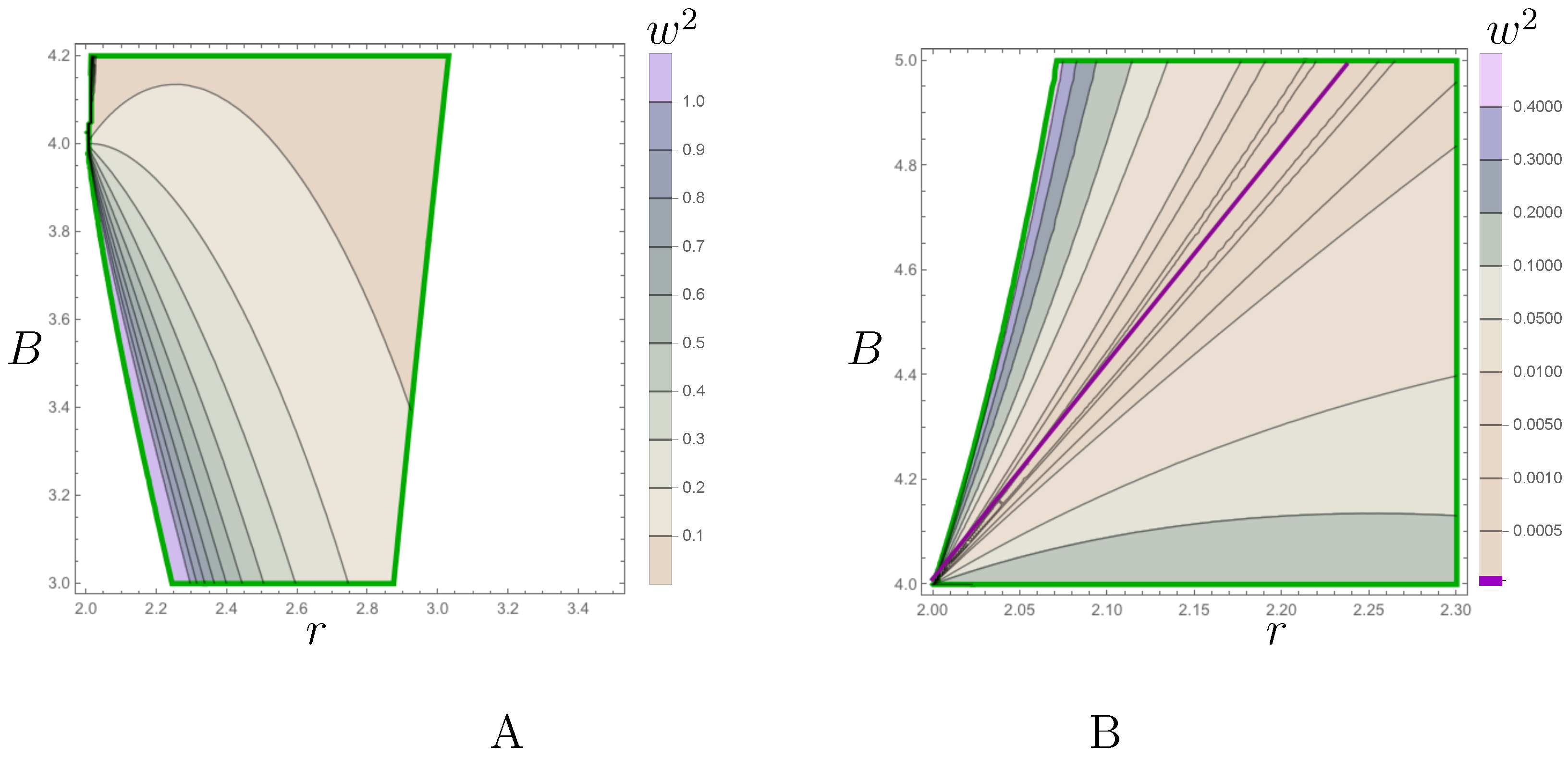
6.1. Time of Black Hole Evaporation
6.2. Small Black Holes and Free-Falling Observer
7. Discussions and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hawking, S.W. Black hole explosions? Nature 1974, 248, 30–31. [Google Scholar] [CrossRef]
- Hawking, S.W. Particle creation by black holes. Comm. Math. Phys. 1975, 43, 199. [Google Scholar] [CrossRef]
- Hawking, S.W. Breakdown of Predictability in Gravitational Collapse. Phys. Rev. D 1976, 14, 2460–2473. [Google Scholar] [CrossRef]
- Susskind, L.; Lindesay, J. An Introduction to Black Holes, Information and the String Theory Revolution: The Holographic Universe; World Scientific: Singapore, 2004. [Google Scholar]
- Hooft, G.’t. Introduction to General Relativity; Spinoza Institute: Utrecht, The Netherlands, 2002; Available online: http://www.staff.science.uu.nl/~hooft101/ (accessed on 7 September 2022).
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University Press: Cambridge, UK, 1973. [Google Scholar]
- Frolov, V.; Novikov, I. Black Hole Physics; Basic concepts and new developments; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 96. [Google Scholar]
- Wald, R.M. General Relativity; University of Chicago Press: Chicago, IL, USA, 2010. [Google Scholar]
- Ydri, B. Quantum Black Holes. arXiv 2017, arXiv:1708.00748. [Google Scholar]
- Unruh, W.G. Notes on black hole evaporation. Phys. Rev. D 1976, 14, 870. [Google Scholar] [CrossRef]
- Birell, N.D.; Davies, P.C.W. Quantum Fields in Curved Space; Cambridge University Press: Cambridge, UK, 1982. [Google Scholar]
- Rindler, W. Kruskal Space and the Uniformly Accelerated Frame. Am. J. Phys. 1966, 34, 1174. [Google Scholar] [CrossRef]
- Singleton, D.; Wilburn, S. Hawking radiation, Unruh radiation and the equivalence principle. Phys. Rev. Lett. 2011, 107, 081102. [Google Scholar] [CrossRef] [PubMed]
- Emparan, R. Heat kernels and thermodynamics in Rindler space. Phys. Rev. D 1995, 51, 5716. [Google Scholar] [CrossRef] [PubMed]
- de Alwis, S.P.; Ohta, N. Thermodynamics of quantum fields in black hole backgrounds. Phys. Rev. D 1995, 52, 3529. [Google Scholar] [CrossRef] [PubMed]
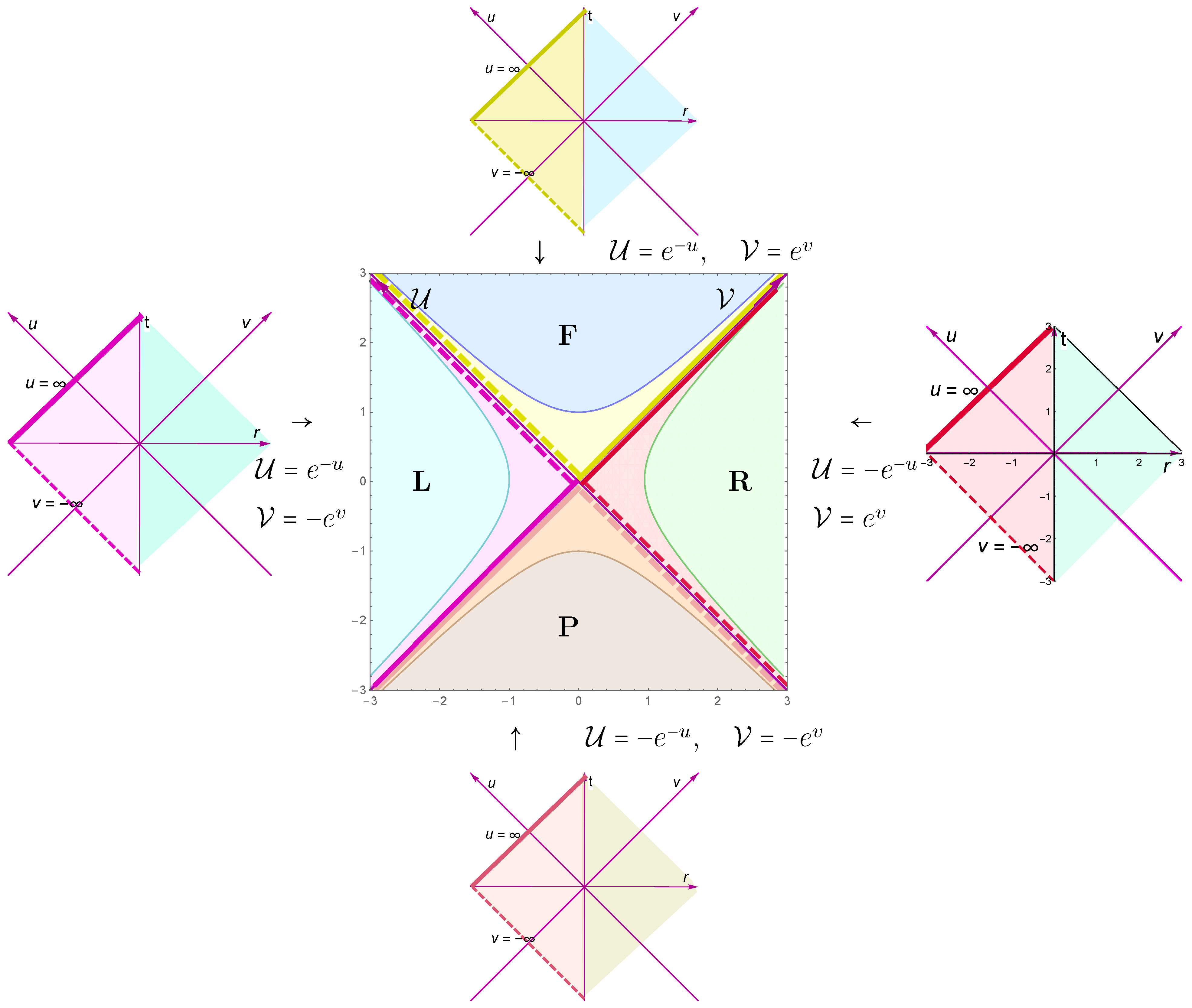
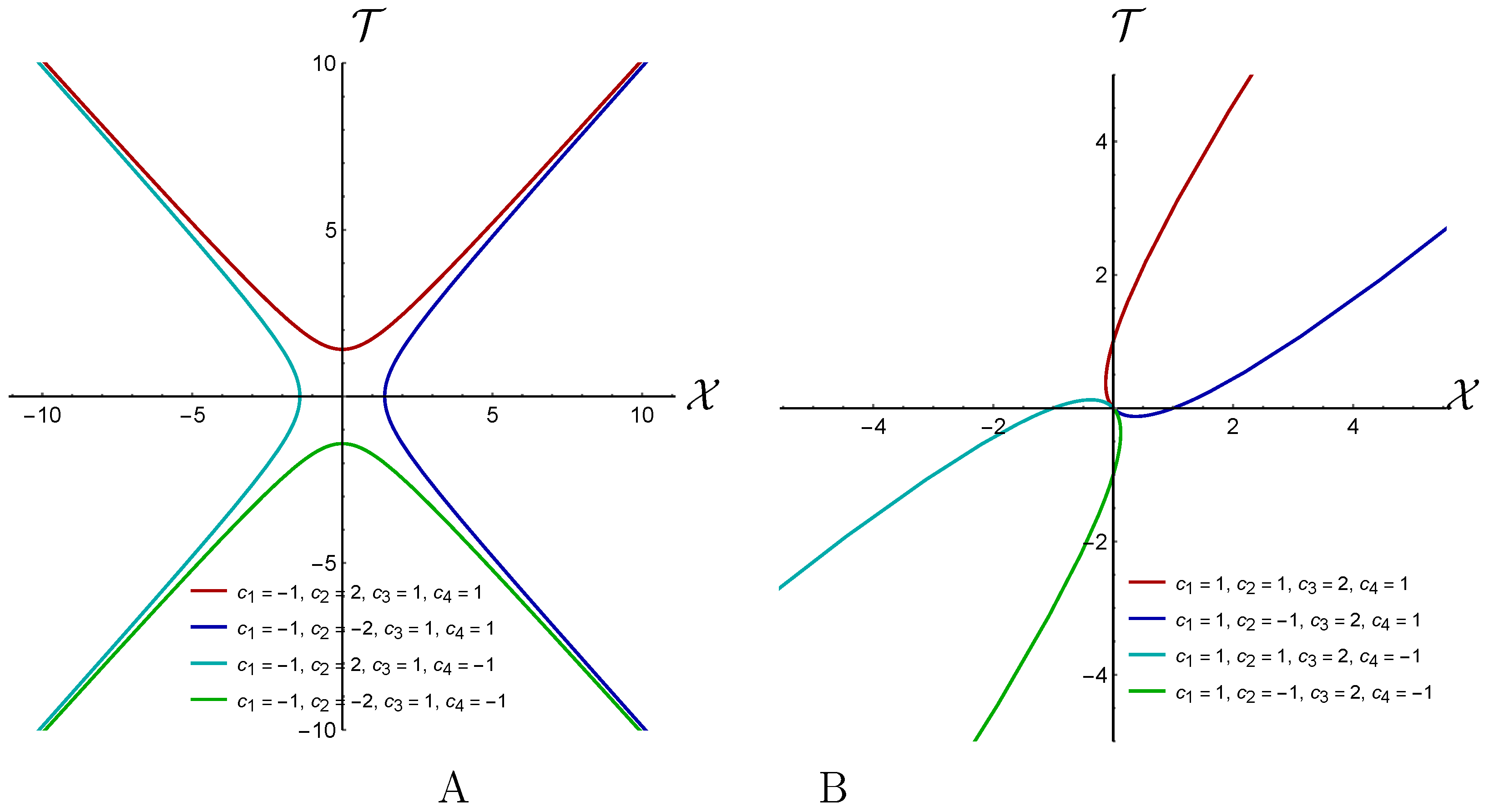
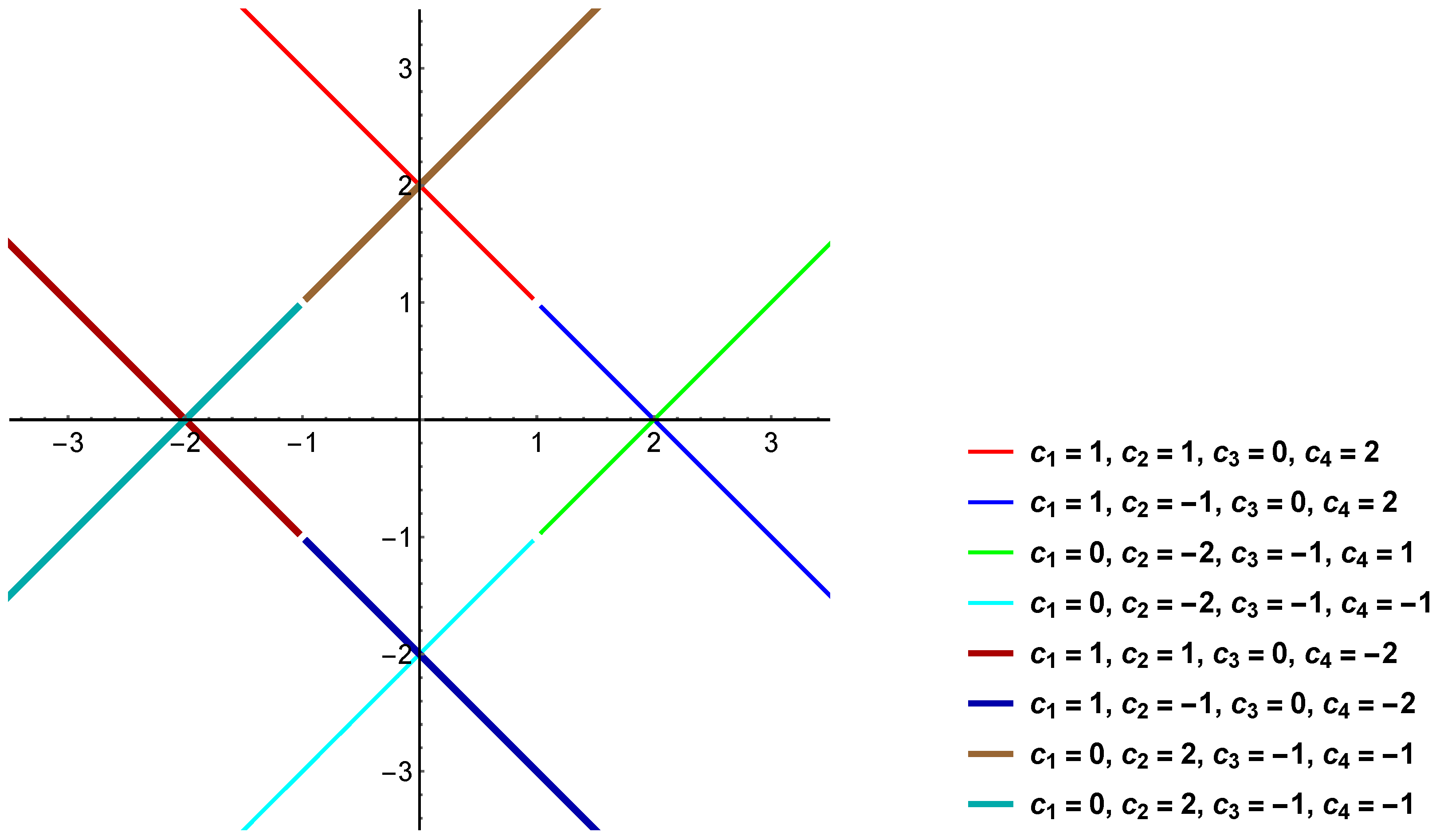
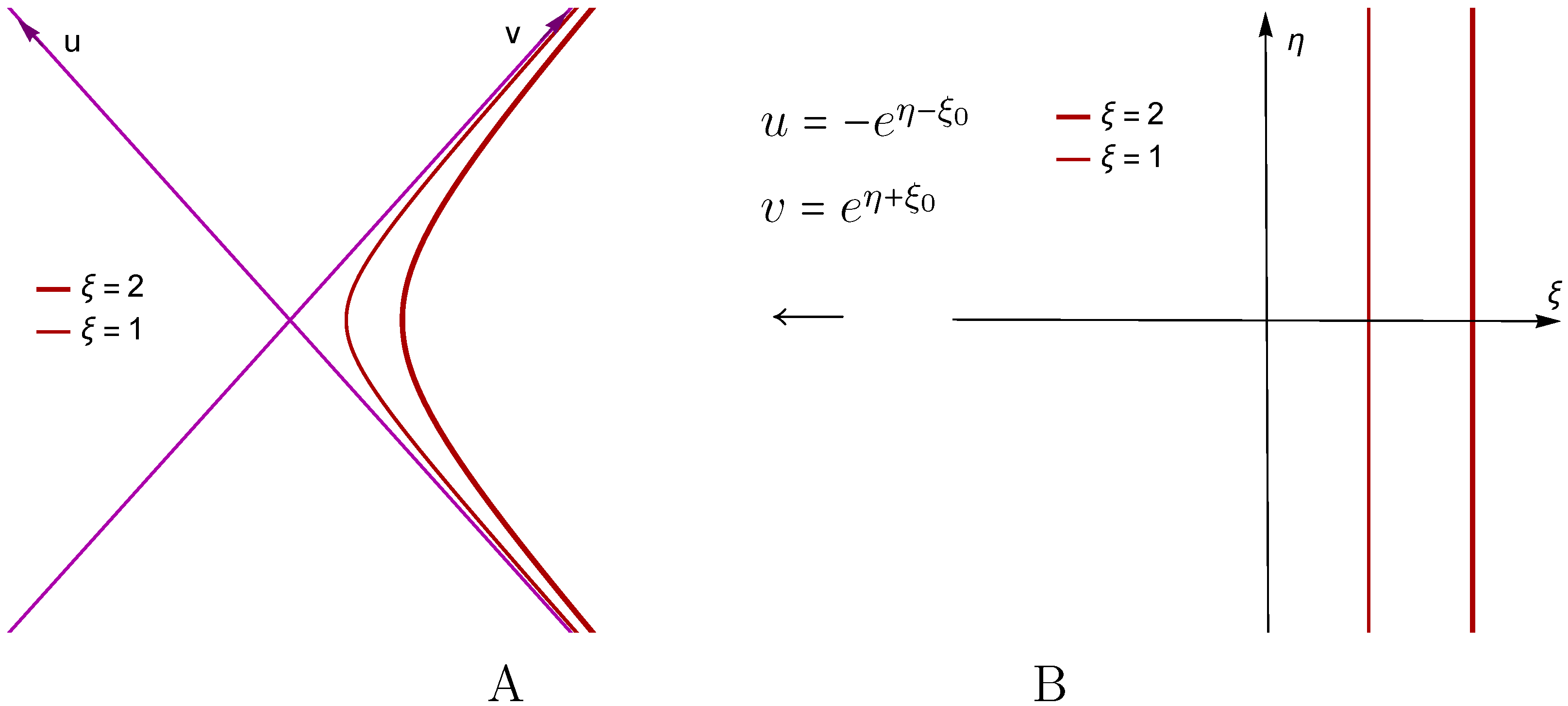
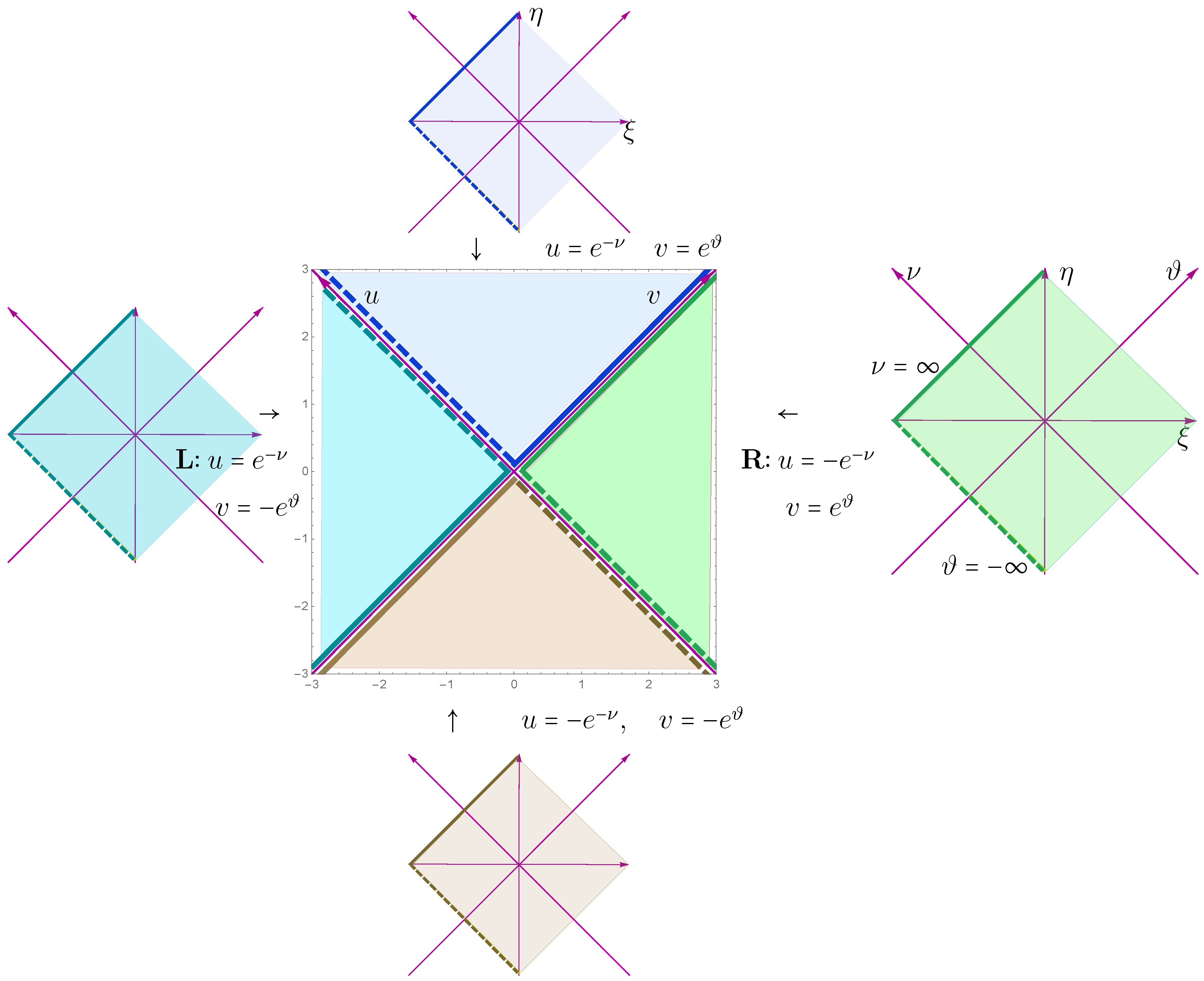


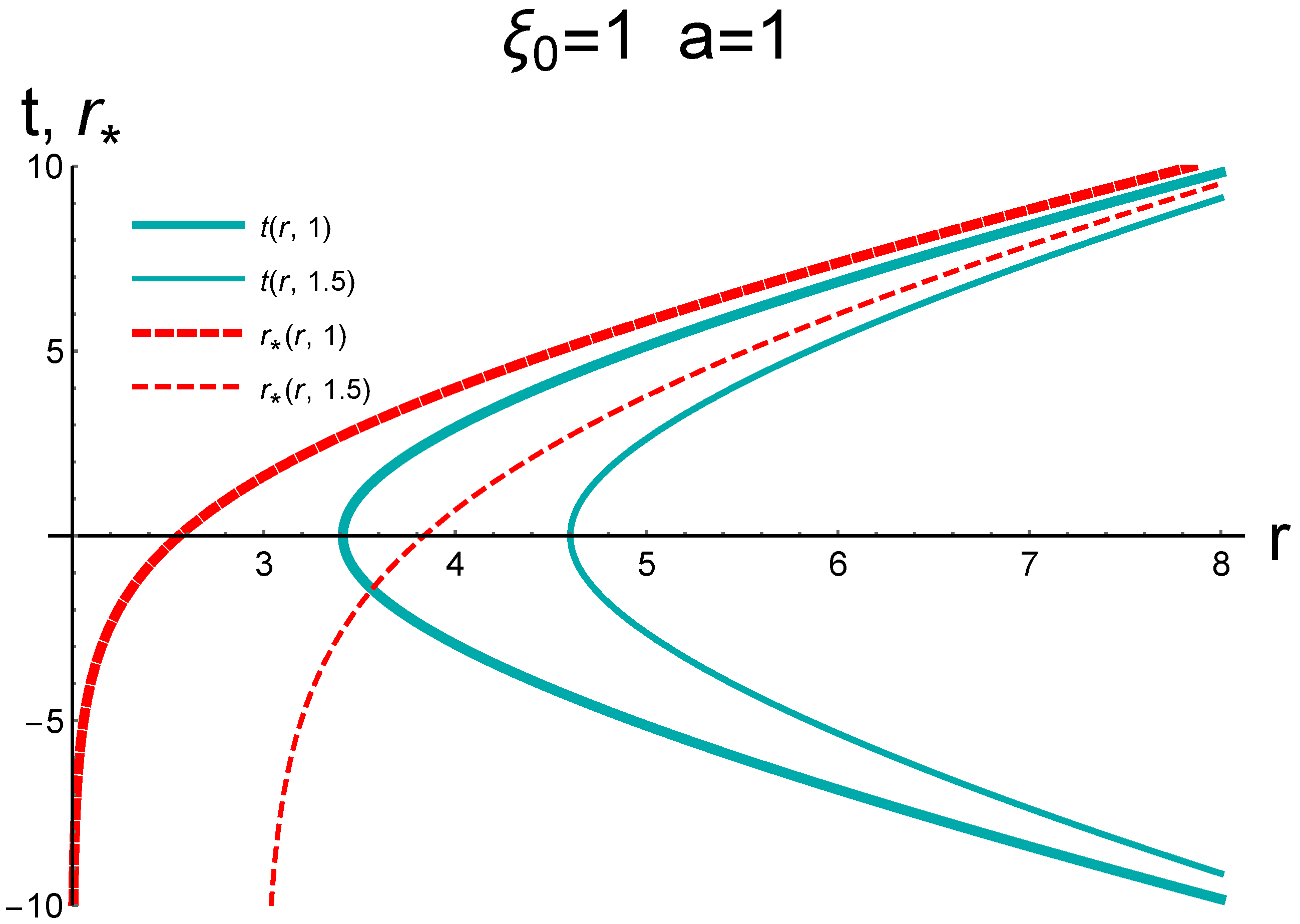

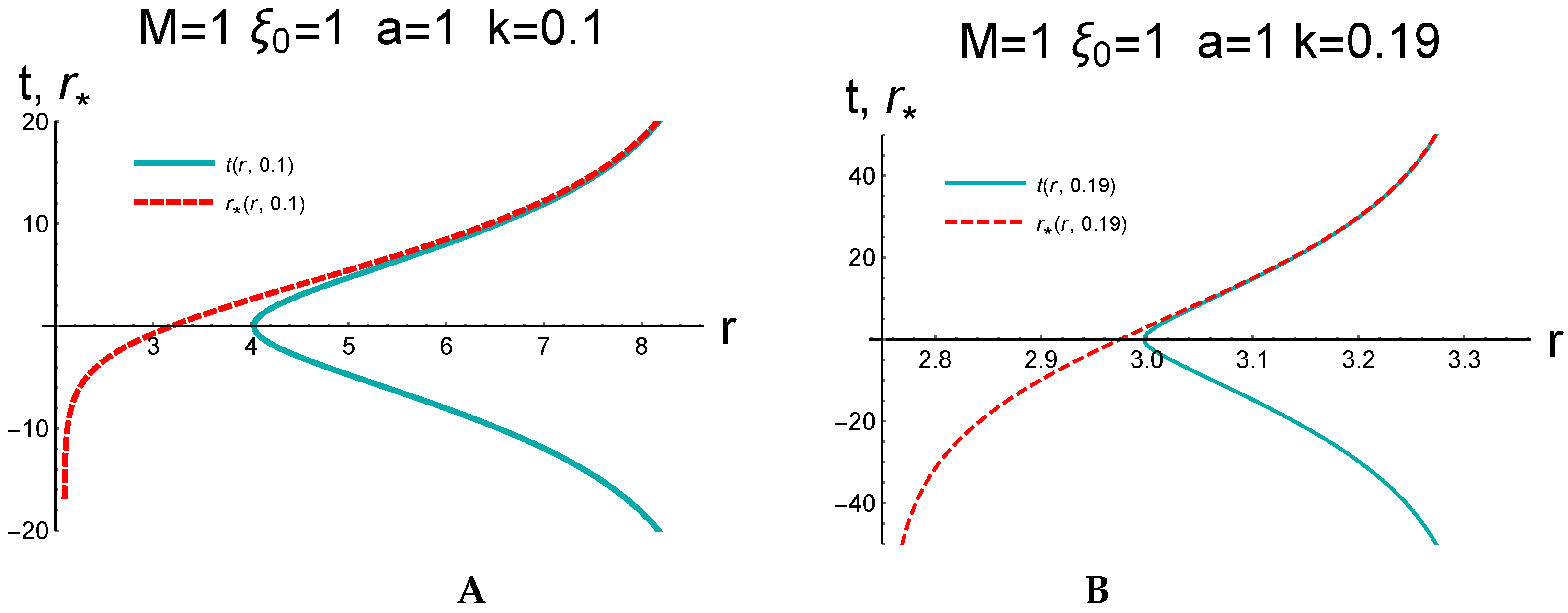
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aref’eva, I.; Volovich, I. Quantum Explosions of Black Holes and Thermal Coordinates. Symmetry 2022, 14, 2298. https://doi.org/10.3390/sym14112298
Aref’eva I, Volovich I. Quantum Explosions of Black Holes and Thermal Coordinates. Symmetry. 2022; 14(11):2298. https://doi.org/10.3390/sym14112298
Chicago/Turabian StyleAref’eva, Irina, and Igor Volovich. 2022. "Quantum Explosions of Black Holes and Thermal Coordinates" Symmetry 14, no. 11: 2298. https://doi.org/10.3390/sym14112298
APA StyleAref’eva, I., & Volovich, I. (2022). Quantum Explosions of Black Holes and Thermal Coordinates. Symmetry, 14(11), 2298. https://doi.org/10.3390/sym14112298




