Diverse Variety of Exact Solutions for Nonlinear Gilson–Pickering Equation
Abstract
1. Introduction
2. Sardar’s Subequation Method (SSM)
3. Implementation of the Method
4. Implementation of Jacobi Elliptic Function Method Depending on
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abdelrahman, M.A.; Kunik, M. The ultra-relativistic Euler equations. Math. Methods Appl. Sci. 2015, 38, 1247–1264. [Google Scholar] [CrossRef]
- Abdelrahman, M.A. Global solutions for the ultra-relativistic Euler equations. Nonlinear Anal. 2017, 155, 140–162. [Google Scholar] [CrossRef]
- Abdelrahman, M.A. On the shallow water equations. Zeitschrift fur Naturforschung A 2017, 72, 873–879. [Google Scholar] [CrossRef]
- Younis, M.; Ali, S.; Mahmood, S.A. Solitons for compound KdV Burgers equation with variable coefficients and power law nonlinearity. Nonlinear Dyn. 2015, 81, 1191–1196. [Google Scholar] [CrossRef]
- Bhrawy, A.H. An efficient Jacobi pseudo spectral approximation for nonlinear complex generalized Zakharov system. Appl. Math. Comput. 2014, 247, 30–46. [Google Scholar]
- Vinogradov, A.M. What are symmetries of nonlinear PDEs and what are they themselves? arXiv 2013, arXiv:1308.5861. [Google Scholar]
- Aliyu, A.I.; Inc, M.; Yusuf, A.; Baleanu, D. Symmetry analysis, explicit solutions, and conservation laws of a sixth-order nonlinear ramani equation. Symmetry 2018, 10, 341. [Google Scholar] [CrossRef]
- Olver, P.J. Applications of Lie Groups to Differential Equations; Springer Science & Business Media: Berlin, Germany, 2018; Volume 107. [Google Scholar]
- Lou, S.Y.; Hu, X.; Chen, Y. Nonlocal symmetries related to Bcklund transformation and their applications. J. Phys. A Math. Theor. 2012, 45, 155209. [Google Scholar] [CrossRef]
- Buhe, E.; Bluman, G.W. Symmetry reductions, exact solutions, and conservation laws of the generalized Zakharov equations. J. Math. Phys. 2012, 56, 101501. [Google Scholar] [CrossRef]
- Gao, B. Symmetry analysis and explicit power series solutions of the Boussinesq Whitham Broer Kaup equation. Waves Random Complex Media 2017, 27, 700–710. [Google Scholar] [CrossRef]
- Feng, L.L.; Tian, S.F.; Zhang, T.T.; Zhou, J. Lie symmetries, conservation laws and analytical solutions for two-component integrable equations. Chin. J. Phys. 2017, 55, 996–1010. [Google Scholar] [CrossRef]
- Kayum, M.; Akbar, M.A.; Osman, M.S. Competent closed form soliton solutions to the nonlinear transmission and the low-pass electrical transmission lines. Eur. Phys. J. Plus 2020, 135, 1–20. [Google Scholar] [CrossRef]
- Kayum, M.A.; Ara, S.; Barman, H.K.; Akbar, M.A. Soliton solutions to voltage analysis in nonlinear electrical transmission lines and electric signals in telegraph lines. Results Phys. 2020, 18, 103269. [Google Scholar] [CrossRef]
- Kayum, M.A.; Seadawy, A.R.; Akbar, A.M.; Sugati, T.G. Stable solutions to the nonlinear RLC transmission line equation and the Sinh Poisson equation arising in mathematical physics. Open Phys. 2020, 18, 710–725. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Kaur, L. Optical solitons and Peregrine solitons for nonlinear Schrodinger equation by variational iteration method. Optik 2019, 179, 804–809. [Google Scholar] [CrossRef]
- Gao, W.; Veeresha, P.; Baskonus, H.M.; Prakasha, D.G.; Kumar, P. A new study of unreported cases of 2019-nCOV epidemic outbreaks. Chaos Solitons Fractals 2020, 138, 109929. [Google Scholar] [CrossRef]
- Seadawy, A.R. Approximation solutions of derivative nonlinear Schrodinger equation with computational applications by variational method. Eur. Phys. J. Plus 2015, 130, 1–10. [Google Scholar] [CrossRef]
- Kudryashov, N.A. First integrals and solutions of the traveling wave reduction for the Triki Biswas equation. Optik 2019, 185, 275–281. [Google Scholar] [CrossRef]
- He, J.H. The homotopy perturbation method for nonlinear oscillators with discontinuities. Appl. Math. Comput. 2004, 151, 287–292. [Google Scholar] [CrossRef]
- Liu, J.G.; Yang, X.J.; Feng, Y.Y. On integrability of the time fractional nonlinear heat conduction equation. J. Geom. Phys. 2019, 144, 190–198. [Google Scholar] [CrossRef]
- Momani, S. Non-perturbative analytical solutions of the space-and time-fractional Burgers equations. Chaos Solitons Fractals 2006, 28, 930–937. [Google Scholar] [CrossRef]
- Xue-Qin, Z.; Hong-Yan, Z. An improved F-expansion method and its application to coupled Drinfel’dr Sokolov Wilson equation. Commun. Theor. Phys. 2008, 50, 309. [Google Scholar] [CrossRef]
- Abdou, M.A. The extended F-expansion method and its application for a class of nonlinear evolution equations. Chaos Solitons Fractals 2007, 31, 95–104. [Google Scholar] [CrossRef]
- Khater, M.M.A.; Akinyemi, L.; Elagan, S.K.; El-Shorbagy, M.A.; Alfalqi, S.H.; Alzaidi, J.F.; Alshehri, N.A. Bright dark soliton waves dynamics in pseudo spherical surfaces through the nonlinear kaup-Kupershmidt equation. Symmetry 2021, 13, 13060963. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A. Traveling wave solutions of the (2+ 1)-dimensional Zoomeron equation and the Burgers equations via the MSE method and the Exp-function method. Ain Shams Eng. J. 2014, 5, 247–256. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A. The exp(-F(ϕ)) expansion method for finding travelling wave solutions of Vakhnenko Parkes equation. Int. J. Dyn. Syst. Differ. 2014, 5, 72–83. [Google Scholar]
- Wazwaz, A.M. A sine-cosine method for handling nonlinear wave equations. Math. Comput. Model. 2004, 40, 499–508. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The sine cosine method for obtaining solutions with compact and noncompact structures. Appl. Math. Comput. 2004, 159, 559–576. [Google Scholar] [CrossRef]
- Raza, N.; Aslam, M.R.; Rezazadeh, H. Analytical study of resonant optical solitons with variable coefficients in Kerr and non-Kerr law media. Opt. Quantum Electron. 2019, 51, 1–12. [Google Scholar] [CrossRef]
- Albosaily, S.; Mohammed, W.W.; Aiyashi, M.A.; Abdelrahman, M.A. Exact solutions of the (2+ 1)-dimensional stochastic chiral nonlinear Schrodinger equation. Symmetry 2020, 12, 1874. [Google Scholar] [CrossRef]
- Abdelijabbar, A.; Roshid, H.-O.; Abdurayhim, A. Bright, Dark, and Rogue Wave soliton solutions of the quadratic nonlinear Klein-Gordon equation. Symmetry 2022, 14, 1223. [Google Scholar] [CrossRef]
- Raza, N.A. Bright, dark and dark-singular soliton solutions of nonlinear Schrodinger’s equation with spatio-temporal dispersion. J. Mod. Opt. 2018, 65, 1975–1982. [Google Scholar] [CrossRef]
- Qian, L.; Attia, R.A.; Qiu, Y.; Lu, D.; Khater, M.M. The shock peakon wave solutions of the general Degasperis-Procesi equation. Int. J. Mod. Physics B 2019, 33, 1950351. [Google Scholar] [CrossRef]
- Sirisubtawee, S.; Koonprasert, S.; Sungnul, S. New exact solutions of the conformable space-time Sharma Tasso Olver equation using two reliable methods. Symmetry 2020, 12, 644. [Google Scholar] [CrossRef]
- Çenesiz, Y.; Tasbozan, O.; Kurt, A. Functional variable method for conformable fractional modified KdV-ZK equation and Maccari system. Tbil. Math. J. 2017, 10, 117–125. [Google Scholar] [CrossRef]
- Eslami, M.; Rezazadeh, H.; Rezazadeh, M.; Mosavi, S.S. Exact solutions to the space time fractional Schrodinger Hirota equation and the space time modified KDV Zakharov Kuznetsov equation. Opt. Quantum Electron. 2017, 49, 1–15. [Google Scholar] [CrossRef]
- Khater, M.; Park, C.; Lu, D.; Attia, R.A. Analytical, semi-analytical, and numerical solutions for the Cahn Allen equation. Adv. Differ. Equ. 2020, 2020, 1–12. [Google Scholar] [CrossRef]
- Khater, M.M.; Attia, R.A.; Lu, D. Computational and numerical simulations for the nonlinear fractional Kolmogorov Petrovskii Piskunov (FKPP) equation. Phys. Scripta 2020, 95, 055213. [Google Scholar] [CrossRef]
- Tozar, A.; Kurt, A.; Tasbozan, O. New wave solutions of time fractional integrable dispersive wave equation arising in ocean engineering models. Kuwait J. Sci. 2020, 47, 22–33. [Google Scholar]
- Rezazadeh, H.; Mirhosseini-Alizamini, S.M.; Eslami, M.; Rezazadeh, M.; Mirzazadeh, M.; Abbagari, S. New optical solitons of nonlinear conformable fractional Schrodinger-Hirota equation. Optik 2018, 172, 545–553. [Google Scholar] [CrossRef]
- Rehman, H.U.; Ullah, N.; Imran, M.A.; Akgul, A. Optical solitons of two non-linear models in birefringent fibres using extended direct algebraic method. Int. J. Appl. Comput. Math. 2021, 7, 1–26. [Google Scholar] [CrossRef]
- Kumar, S.; Dhiman, S.K.; Baleanu, D.; Osman, M.S.; Wazwaz, A.-M. Lie symmetries, closed-form solutions, and various dynamical profiles of solitons for the variable coefficient (2+1)-Dimensional KP equations. Symmetry 2022, 14, 597. [Google Scholar] [CrossRef]
- Bruzon, M.S.; Garrido, T.M.; de la Rose, R. Symmetry analysis, Exact Solutions and conservation laws of a Benjamin-Bona-Mahony-Burgers equation in 2+1-Dimensions. Symmetry 2021, 13, 2083. [Google Scholar] [CrossRef]
- Alam, M.N.; Akbar, M.A.; Mohyud-Din, S.T. A novel (G’/G)-expansion method and its application to the Boussinesq equation. Chin. Phys. B 2013, 23, 020203. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh coth method for solitons and kink solutions for nonlinear parabolic equations. Appl. Math. Comput. 2007, 188, 1467–1475. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh coth method for new compactons and solitons solutions for the K (n, n) and the K (n+ 1, n+ 1) equations. Appl. Math. Comput. 2007, 188, 1930–1940. [Google Scholar] [CrossRef]
- Liu, C.; Liu, X. A note on the auxiliary equation method for solving nonlinear partial differential equations. Phys. Lett. A 2006, 348, 222–227. [Google Scholar] [CrossRef]
- Khater, M.M.; Seadawy, A.R.; Lu, D. Dispersive optical soliton solutions for higher order nonlinear Sasa-Satsuma equation in mono mode fibers via new auxiliary equation method. Superlattices Microstruct. 2018, 113, 346–358. [Google Scholar] [CrossRef]
- Miah, M.M.; Ali, H.S.; Akbar, M.A.; Seadawy, A.R. New applications of the two variable (G’/G, 1/G)-expansion method for closed form traveling wave solutions of integro-differential equations. J. Ocean. Eng. Sci. 2019, 4, 132–143. [Google Scholar] [CrossRef]
- Durur, H.; Ilhan, E.; Bulut, H. Novel complex wave solutions of the (2+ 1)-dimensional hyperbolic nonlinear Schrodinger equation. Fractal Fract. 2020, 4, 41. [Google Scholar] [CrossRef]
- Alotaibi, H. Travelling wave solutions to the nonlinear evoluation equation using expension and addendum to kudryashove’s method. Symmetry 2021, 13, 2126. [Google Scholar] [CrossRef]
- Ramzan, M.; Chu, Y.M.; ur Rehman, H.; Saleem, M.S.; Park, C. Soliton solutions for anti-cubic nonlinearity using three analytical approches. J. Appl. Anal. Comput. 2021, 11, 2177–2192. [Google Scholar]
- Awan, A.U.; Tahir, M.; Rehman, H.U. Singular and bright-singular combo optical solitons in birefringent fibers to the Biswas-Arshed equation. Optik 2020, 210, 164489. [Google Scholar] [CrossRef]
- Tahir, M.; Awan, A.U.; Rehman, H.U. Dark and singular optical solitons to the Biswas-Arshed model with Kerr and power law nonlinearity. Optik 2019, 185, 777–783. [Google Scholar] [CrossRef]
- Awan, A.U.; Tahir, M.; Rehman, H.U. On traveling wave solutions: The WuZhang system describing dispersive long waves. Mod. Phys. Lett. B 2019, 33, 1950059. [Google Scholar] [CrossRef]
- Rehman, H.U.; Awan, A.U.; Abro, K.A.; El Din, E.M.T.; Jafar, S.; Galal, A.M. A non-linear study of optical solitons for Kaup-Newell equation without four-wave mixing. J. King-Saud Univ.-Sci. 2022, 34, 102056. [Google Scholar] [CrossRef]
- Rehman, H.U.; Awan, A.U.; Habib, A.; Gamaoun, F.; El Din, E.M.T.; Galal, A.M. Solitary wave solutions for a strain wave equation in a microstructured solid. Results Phys. 2022, 39, 105755. [Google Scholar] [CrossRef]
- Gilson, C.; Pickering, A. Factorization and painlev analysics of a class of nonlinear third-order partial differential equations. J. Phys. A Math. Gen. 1995, 28, 2871. [Google Scholar] [CrossRef]
- Hadi, R.; Adil, J.; Eric, T.; Mir, S.H.; Sumaira, S.; Hijab, A.; Shao-wen, Y. New wave surfaces and bifurcation of nonlinear periodic waves for Gilson-Pickering equation. Results Phys. 2021, 24, 104192. [Google Scholar]
- Rezazadeh, H.; Inc, M.; Baleanu, D. New solitary wave solutions for variants of (3+ 1)-dimensional Wazwaz-Benjamin-Bona-Mahony equations. Front. Phys. 2020, 8, 332. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Abazari, R.; Khater, M.M.; Baleanu, D. New optical solitons of conformable resonant nonlinear Schrodingers equation. Open Phys. 2020, 18, 761–769. [Google Scholar] [CrossRef]
- Rehman, H.U.; Seadawy, A.R.; Younis, M.; Yasin, S.; Raza, S.T.; Althobaiti, S. Monochromatic optical beam propagation of paraxial dynamical model in Kerr media. Results Phys. 2021, 31, 105015. [Google Scholar] [CrossRef]
- Rehman, H.U.; Awan, A.U.; Allahyani, S.A.; Tag-ElDin, E.M.; Binyamin, M.A.; Yasin, S. Exact solution of paraxial wave dynamical model with Kerr Media by using ϕ6 model expansion technique. Results Phys. 2022, 42, 105975. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Mohammed, W.W. The analytical solutions of the stochastic fractional RKL equation via Jacobi elliptic function method. Adv. Math. Phys. 2022, 2022, 1534067. [Google Scholar] [CrossRef]
- Ali, M.N.; Husnine, S.M.; Saha, A.; Bhowmik, S.K.; Dhawan, S.; Ak, T. Exact solutions, conservation laws, bifurcation of nonlinear and supernonlinear traveling waves for Sharma Tasso Olver equation. Nonlinear Dyn. 2018, 94, 1791–1801. [Google Scholar] [CrossRef]
- Hyder, A.-A.; Barakat, M.A.; Soliman, A.H.; Almoneef, A.A.; Cesarano, C. New analytical solutions for coupled Stochastic korteweg-de Vries equation via Generalized Derivatives. Symmetry 2022, 14, 1770. [Google Scholar] [CrossRef]
- Ebadi, G.; Kara, A.H.; Petkovic, M.D.; Biswas, A. Soliton solutions and conservation laws of the Gilson Pickering equation. Waves Random Complex Media 2011, 21, 378–385. [Google Scholar] [CrossRef]
- Aslan, I. Exact and explicit solutions to nonlinear evolution equations using the division theorem. Appl. Math. Comput. 2011, 217, 8134–8139. [Google Scholar] [CrossRef]
- Zhang, Z.; Xia, F.L.; Li, X.P. Bifurcation analysis and the travelling wave solutions of the Klein Gordon Zakharov equations. Pramana 2013, 80, 41–59. [Google Scholar] [CrossRef]
- Aksoy, E.; Kaplan, M.; Bekir, A. Exponential rational function method for space time fractional differential equations. Waves Random Complex Media 2016, 26, 142–151. [Google Scholar] [CrossRef]
- Liu, S.; Fu, Z.; Liu, S.; Zhao, Q. Jacobi elliptic function expansion method and periodic wave solutions of nonlinear wave equations. Phys. Lett. A 2001, 289, 69–74. [Google Scholar] [CrossRef]
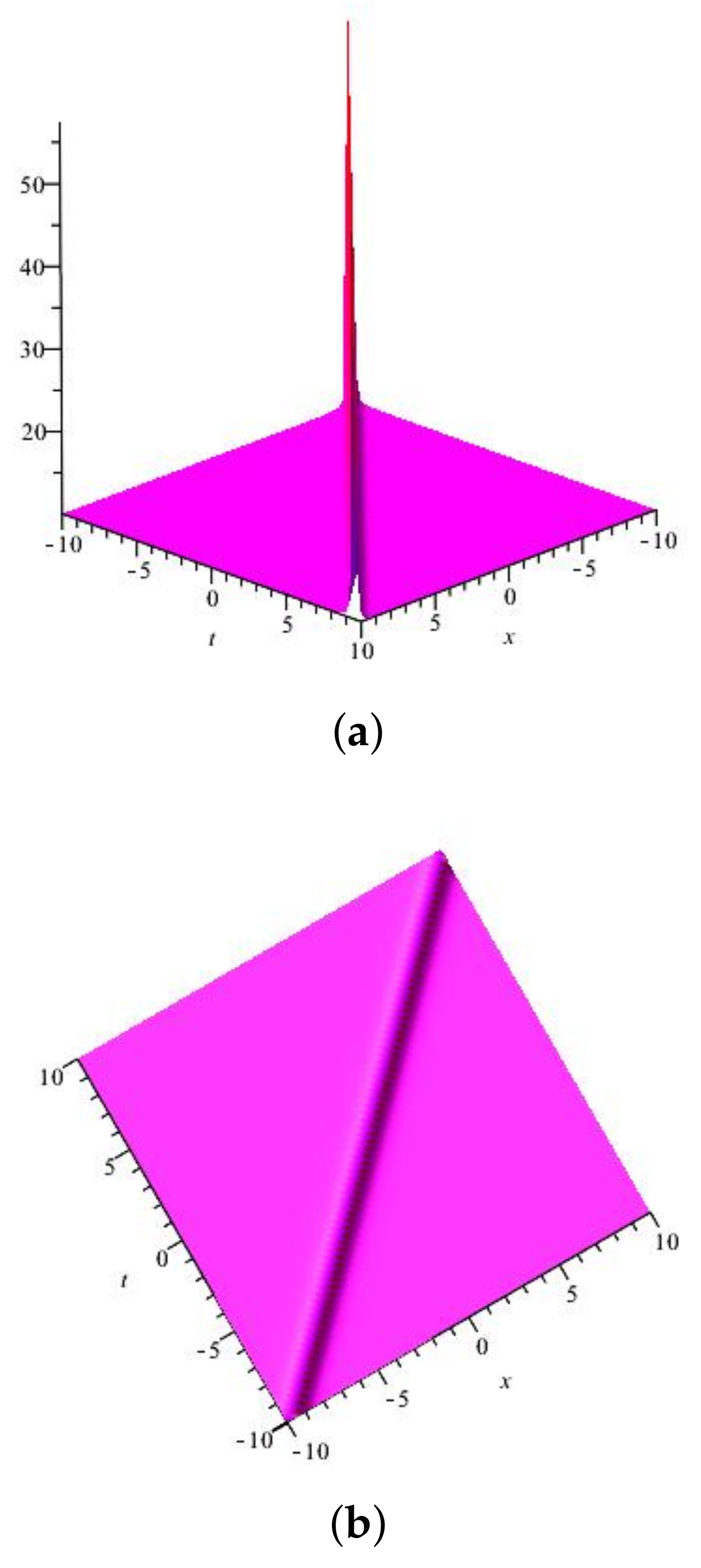
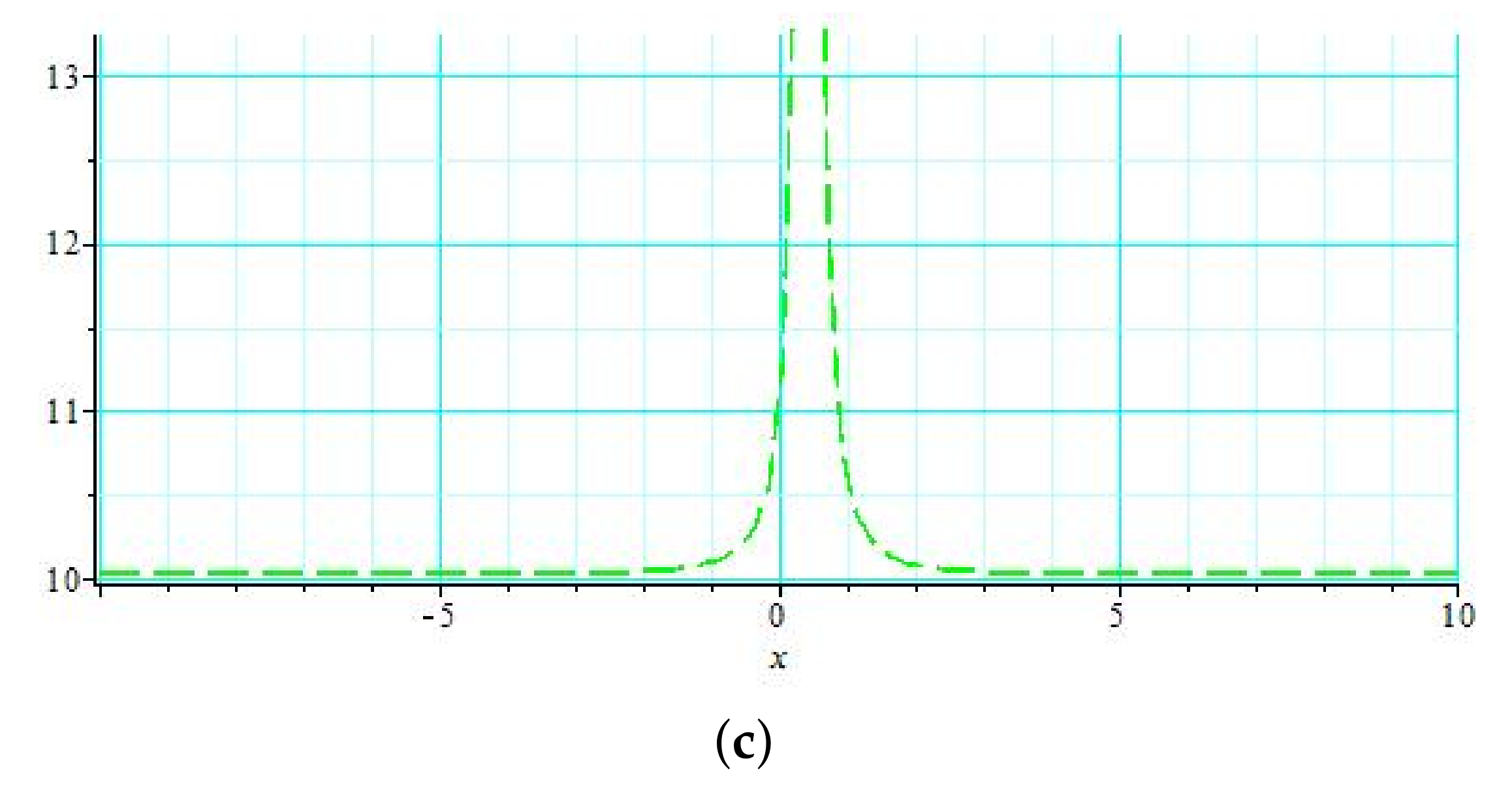
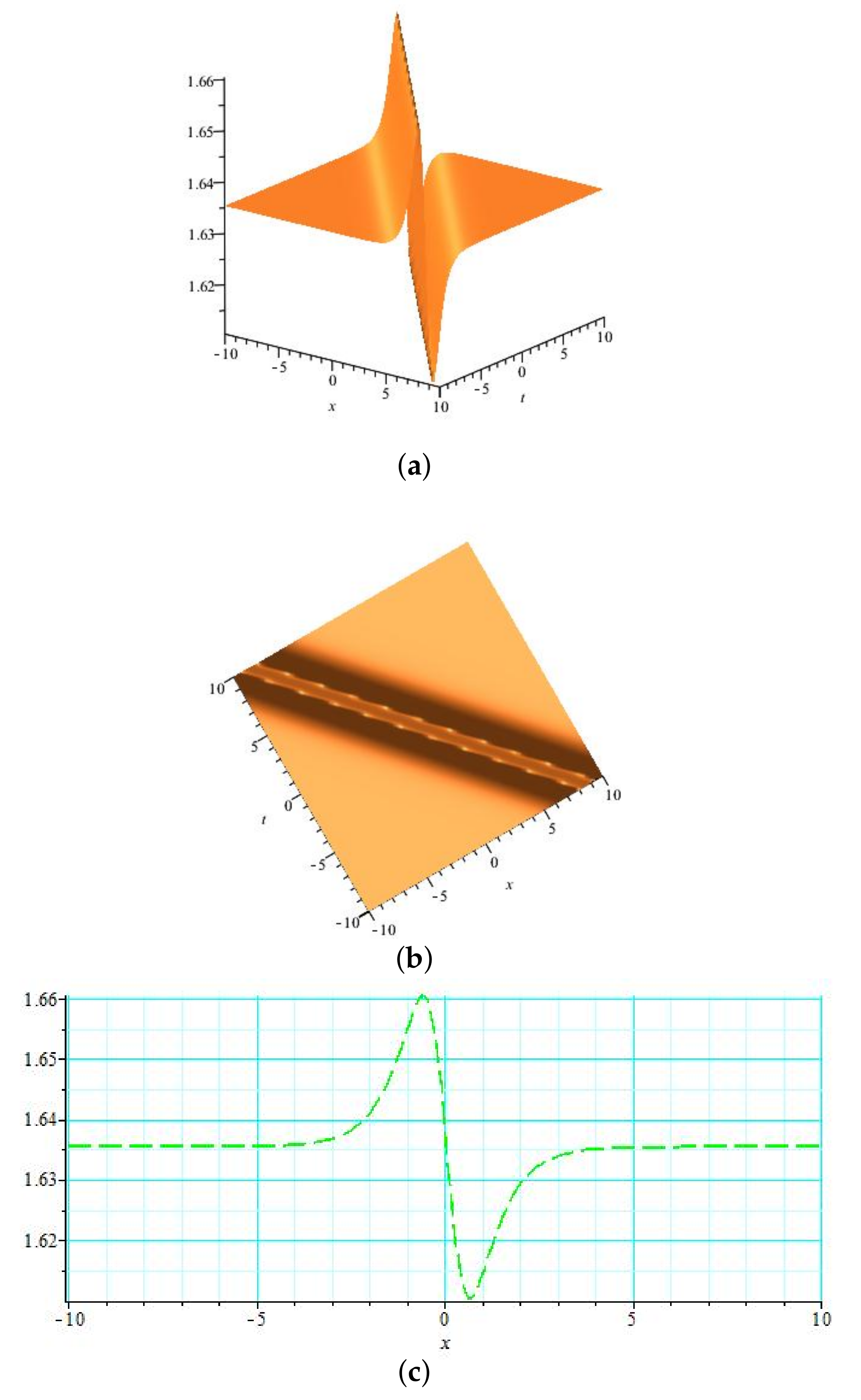

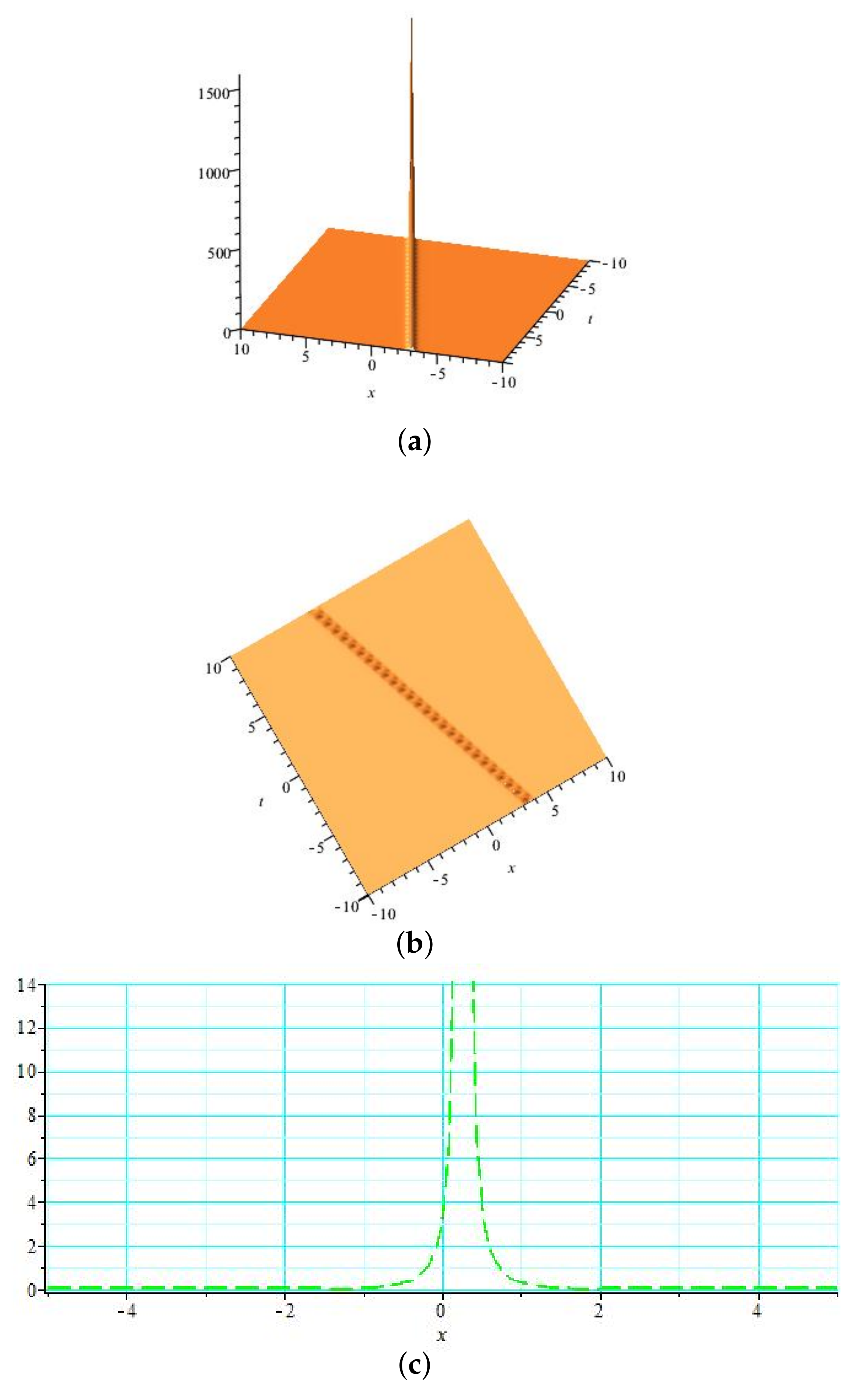

| Case | p | q | r | F |
|---|---|---|---|---|
| 1 | 1 | |||
| 2 | 2 | |||
| 3 | 2 | |||
| 4 | ||||
| 5 | -2 | |||
| 6 | ||||
| 7 | ||||
| 8 | ||||
| 9 | ||||
| 10 | ||||
| 11 | ||||
| 12 | 2 | 0 | 0 | |
| 13 | 0 | 1 | 0 |
| Case | p | q | r | F |
|---|---|---|---|---|
| 1 | 2 | 1 | ||
| 2 | 2 | 1 | 0 | |
| 3 | 2 | 1 | 0 | |
| 4 | 1 | 0 | ||
| 5 | -2 | 1 | 0 | |
| 6 | ||||
| 7 | ||||
| 8 | 1 | 0 | ||
| 9 | 0 | 1 | 0 | |
| 10 | 0 | 0 | 0 | |
| 11 | 0 | 0 | ||
| 12 | 2 | 0 | 0 | |
| 13 | 0 | 1 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Allahyani, S.A.; Rehman, H.U.; Awan, A.U.; Tag-ElDin, E.M.; Hassan, M.U. Diverse Variety of Exact Solutions for Nonlinear Gilson–Pickering Equation. Symmetry 2022, 14, 2151. https://doi.org/10.3390/sym14102151
Allahyani SA, Rehman HU, Awan AU, Tag-ElDin EM, Hassan MU. Diverse Variety of Exact Solutions for Nonlinear Gilson–Pickering Equation. Symmetry. 2022; 14(10):2151. https://doi.org/10.3390/sym14102151
Chicago/Turabian StyleAllahyani, Seham Ayesh, Hamood Ur Rehman, Aziz Ullah Awan, ElSayed M. Tag-ElDin, and Mahmood Ul Hassan. 2022. "Diverse Variety of Exact Solutions for Nonlinear Gilson–Pickering Equation" Symmetry 14, no. 10: 2151. https://doi.org/10.3390/sym14102151
APA StyleAllahyani, S. A., Rehman, H. U., Awan, A. U., Tag-ElDin, E. M., & Hassan, M. U. (2022). Diverse Variety of Exact Solutions for Nonlinear Gilson–Pickering Equation. Symmetry, 14(10), 2151. https://doi.org/10.3390/sym14102151







