Iterative Learning Control for Actuator Fault Uncertain Systems
Abstract
:1. Introduction
2. Problem Description
2.1. System Description
2.2. Equivalent Two-Dimensional Model
3. 2D Roesser System Control Theory
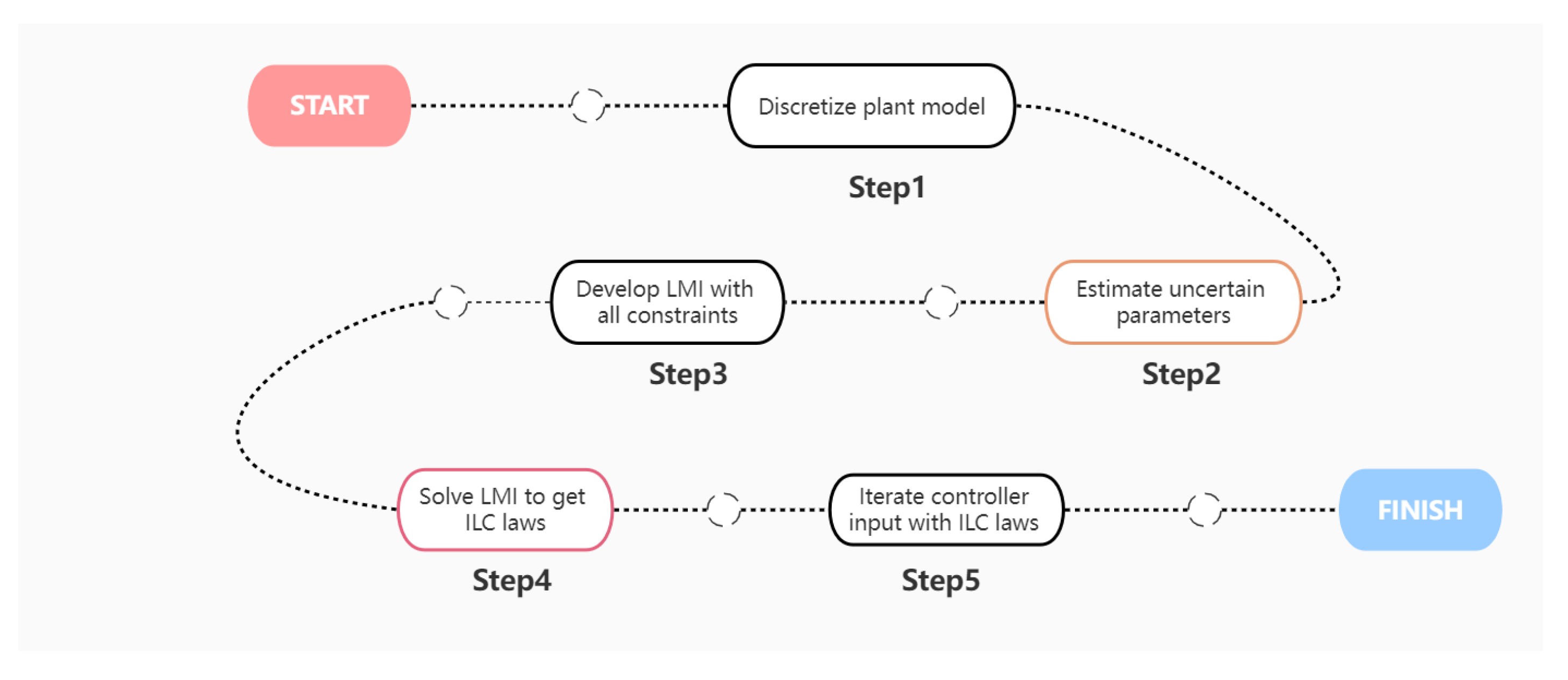
4. Iterative Learning Controller Design
| Algorithm 1: Actuator Fault Uncertainty System ILC Algorithm |
|
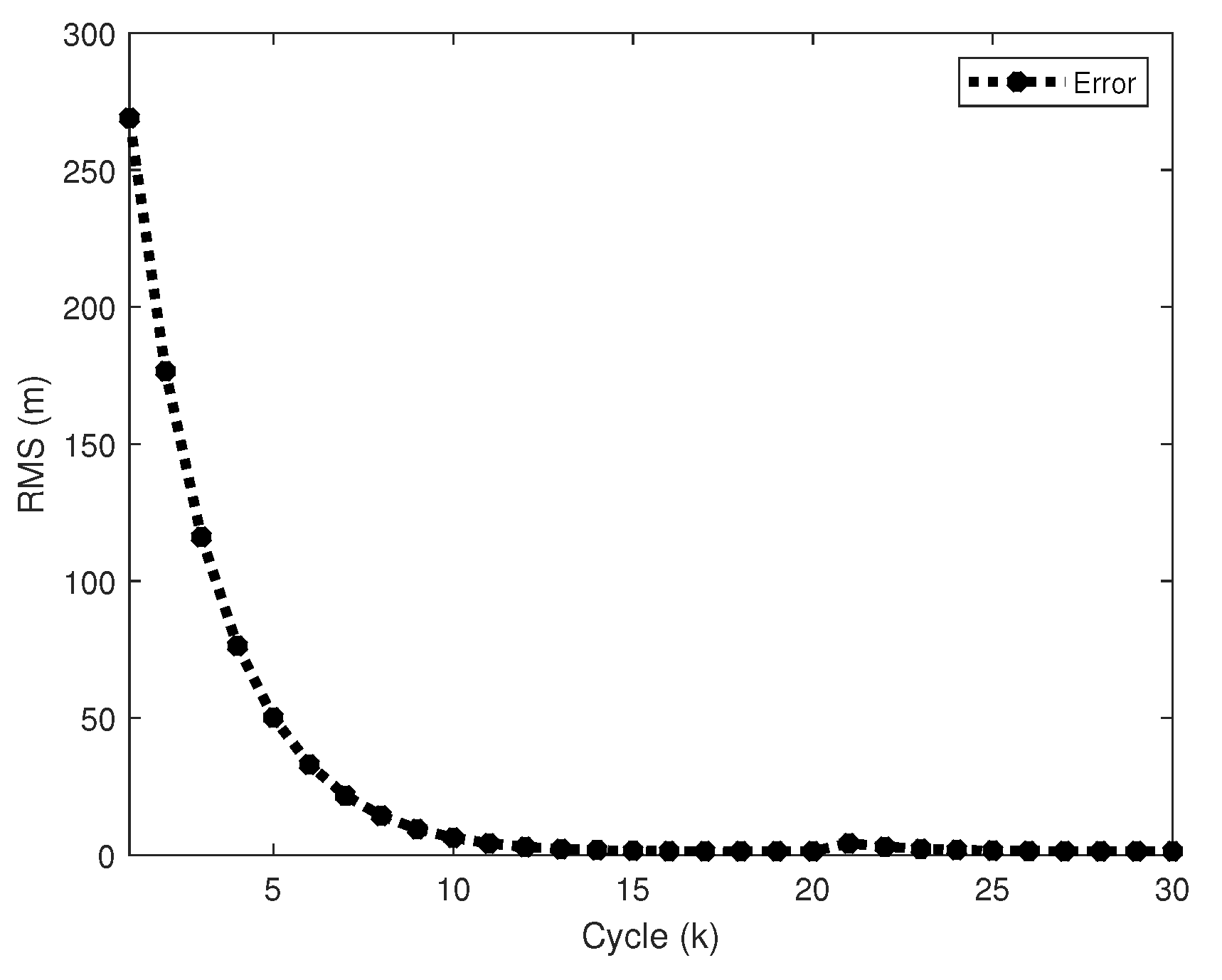
5. Simulation Results
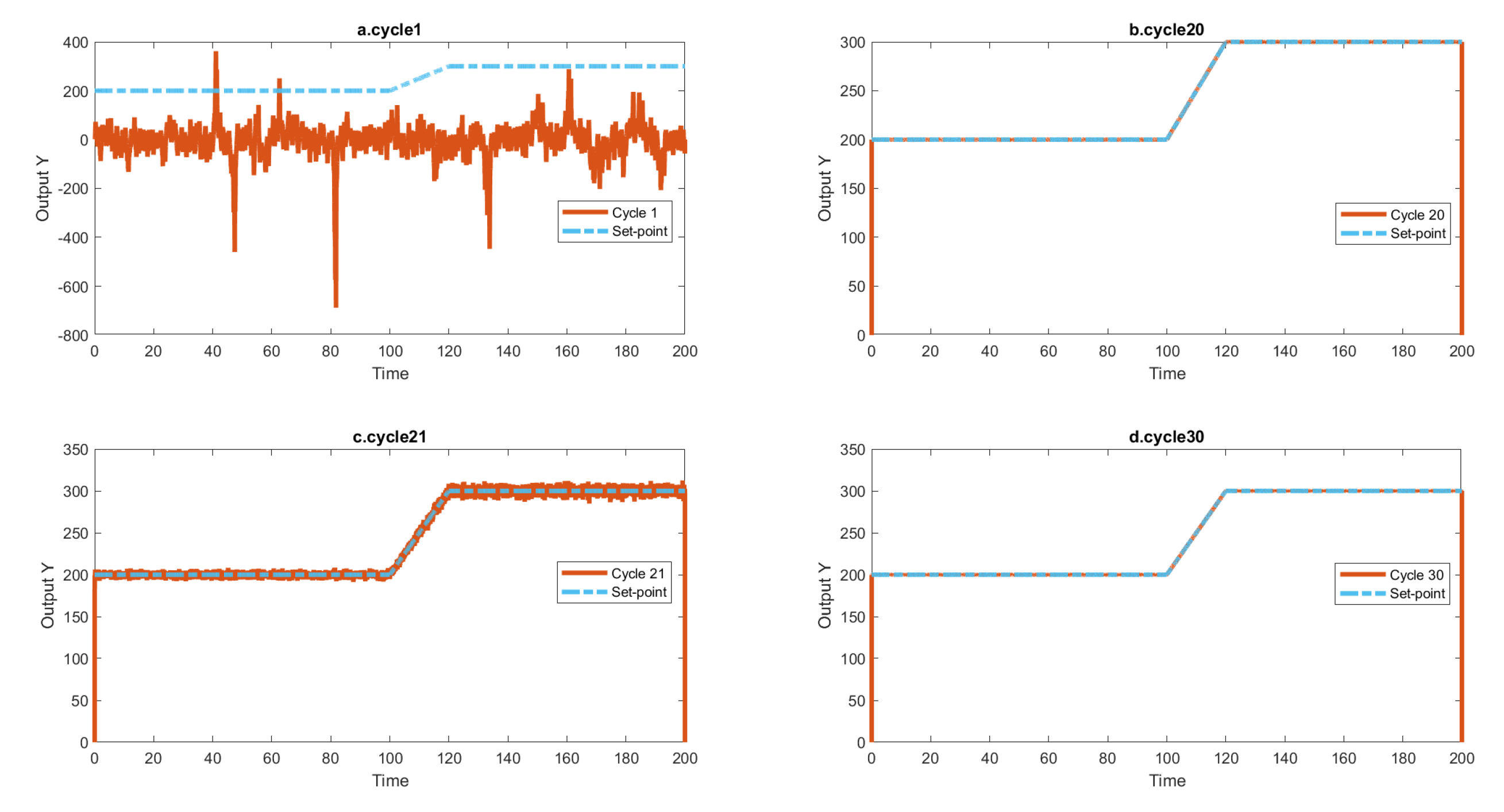
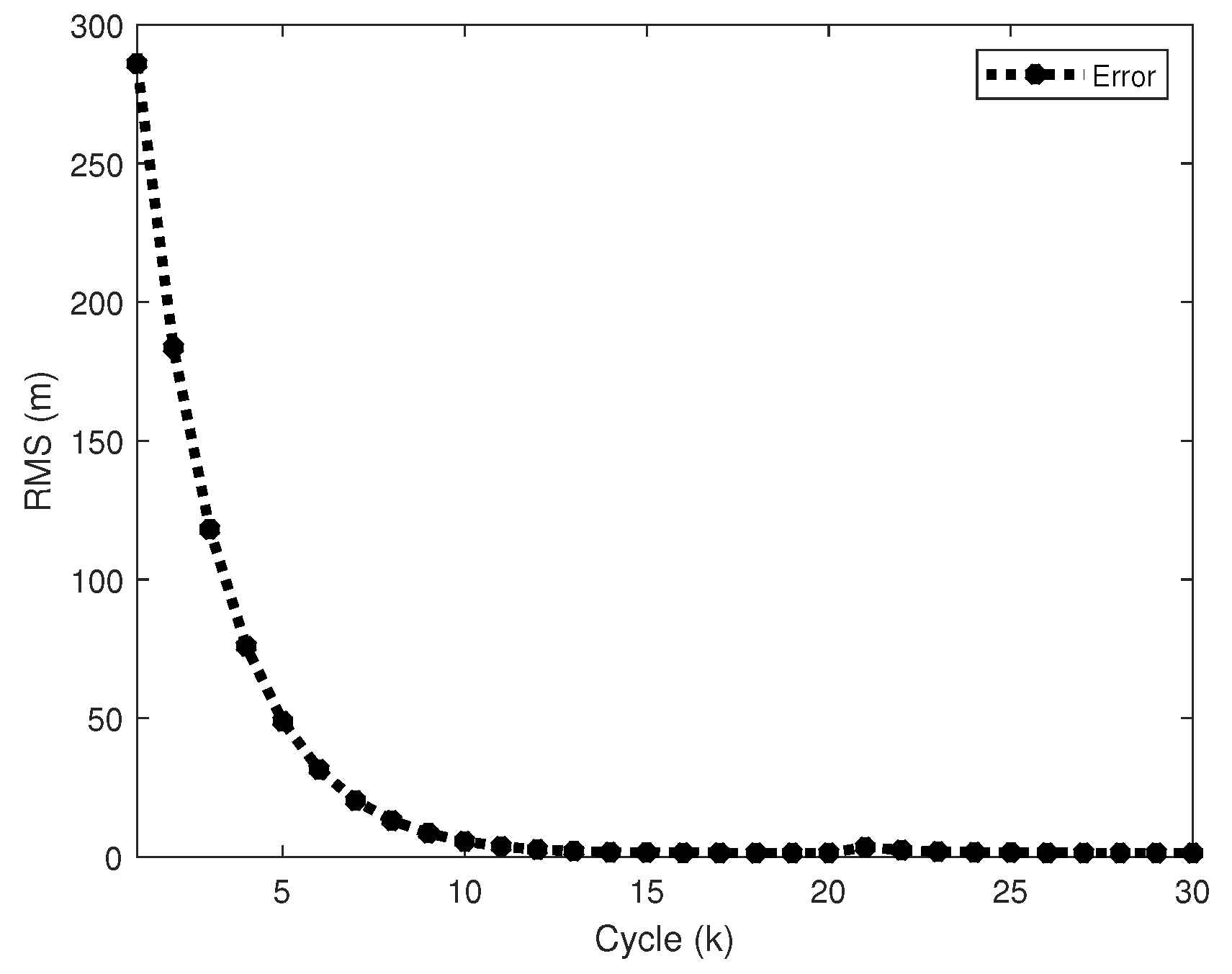
5.1. Scenario 1: Steady Faults and Repetitive Disturbances
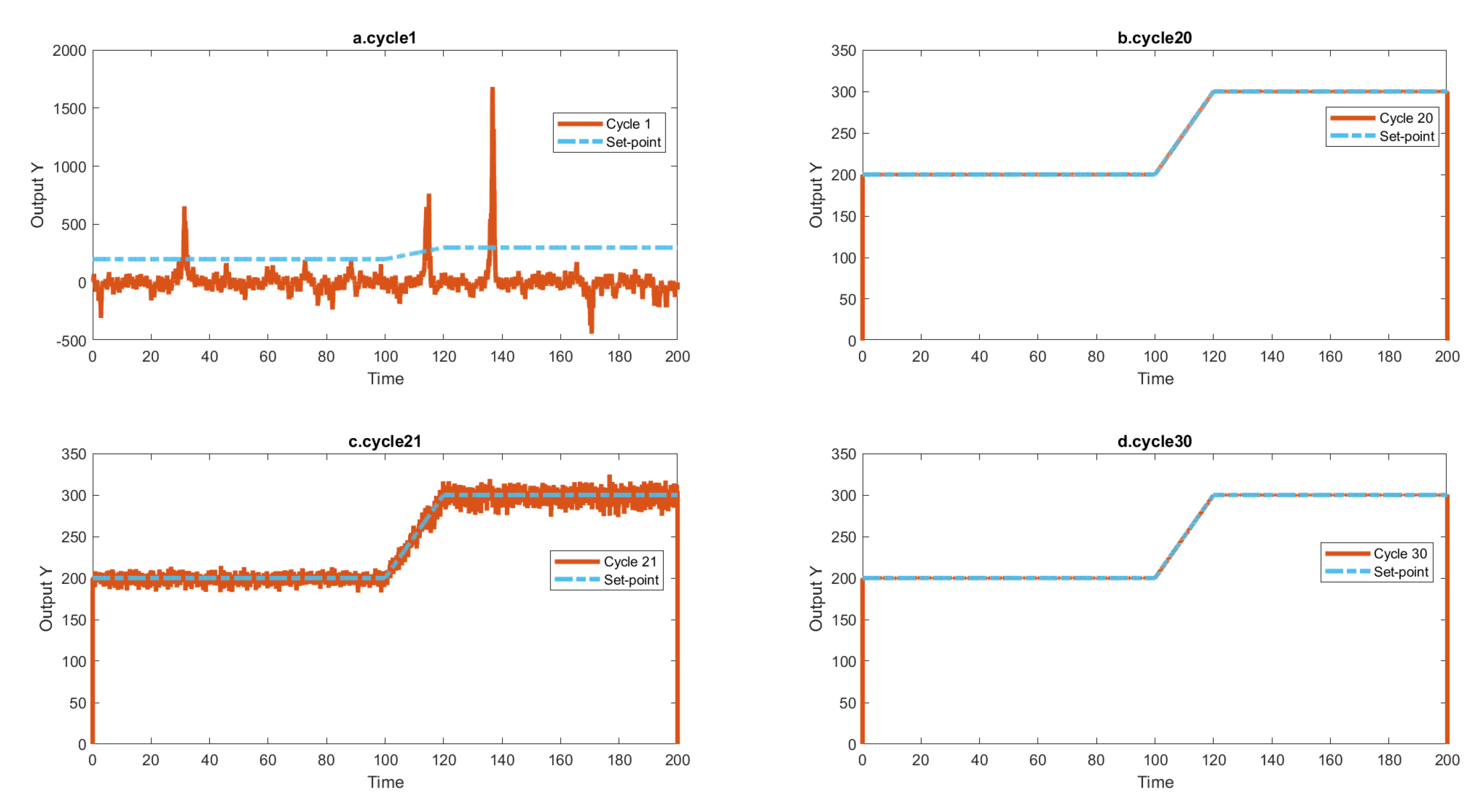
5.2. Scenario 2:Time-Varying Faults and Non-Repeating Disturbances
6. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Hao, S.L. Two-dimensional delay compensation based iterative learning control scheme for batch processes with both input and state delays. J. Frankl. Inst. 2019, 356, 8118–8137. [Google Scholar] [CrossRef]
- Zhang, K.J.; Peng, G.H. PD α-type Iterative Learning Control for a Class of Fractional-order Linear Switched Systems with Time-delay. Control Eng. China 2021, 28, 1179–1186. [Google Scholar]
- Shen, D.; Liu, C.; Wang, D.D. Iterative Learning Tracking for Multisensor Systems: A Weighted Optimization Approach. J. Process Control 2017, 51, 1286–1299. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Chu, B.; Freeman, C.T. Generalized Iterative Learning Control Using Successive Projection: Algorithm, Convergence, and Experimental Verification. IEEE Trans. Control Syst. Technol. 2020, 28, 2079–2091. [Google Scholar] [CrossRef]
- Chaibet, A.; Boukhnifer, M. Experimental Passive Fault Tolerant Control for Gyroscope System; IEEE: Crete, Greece, 2013; pp. 167–172. [Google Scholar]
- Hu, Q.L.; Niu, G.L.; Wang, C.L. Spacecraft attitude fault-tolerant control based on iterative learning observer and control allocation. Aerosp. Sci. Technol. 2018, 75, 245–253. [Google Scholar] [CrossRef]
- Mazare, M.; Taghizadeh, M. Uncertainty estimator-based dual layer adaptive fault-tolerant control for wind turbines. Renew. Energy 2022, 188, 545–560. [Google Scholar] [CrossRef]
- Mazare, M.; Taghizadeh, M.; Ghaf-Ghanbari, P. Pitch actuator fault-tolerant control of wind turbines based on time delay control and disturbance observer. Ocean Eng. 2021, 238, 109724. [Google Scholar] [CrossRef]
- Chen, Y.; Jiang, W.; Charalambous, T. Machine learning based iterative learning control for non-repetitive time-varying systems. Int. J. Robust Nonlinear Control 2021. Early Access. [Google Scholar] [CrossRef]
- Chen, Y.; Chu, B.; Freeman, C.T. Iterative Learning Control for Robotic Path Following With Trial-Varying Motion Profiles. IEEE/ASME Trans. Mechatron. 2022, 1–10, Early Access. [Google Scholar]
- Chen, Y.; Chu, B.; Freeman, C.T. Spatial path tracking using iterative learning control. In Proceedings of the IEEE 55th Conference on Decision and Control (CDC), Las Vegas, NV, USA, 12–14 December 2016; pp. 7189–7194. [Google Scholar]
- Yang, G.H.; Wang, J.L.; Soh, Y.C. Generalized iterative learning control with mixed system constraints: A gantry robot based verification. Control Eng. Pract. 2020, 95, 104260. [Google Scholar]
- Wang, L.; Yang, H.; Paszke, W. Iterative Learning Control for Linear Differential Systems With Additional Performance Requirements. IEEE Access 2020, 107013–107024. [Google Scholar] [CrossRef]
- Ding, J.; Cichy, B.; Galkowski, K. Parameter-dependent Lyapunov function-based robust iterative learning control for discrete systems with actuator faults. Int. J. Adapt. Control Signal Process. 2016, 30, 1714–1732. [Google Scholar] [CrossRef]
- Chen, Y.; Chu, B.; Freeman, C.T. Iterative Learning Control for Path-Following Tasks With Performance Optimization. IEEE Trans. Control Syst. Technol. 2022, 30, 234–246. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zhou, D.H.; Gao, F.R. Iterative learning reliable control of batch processes with sensor faults. Chem. Eng. Sci. 2008, 63, 1039–1051. [Google Scholar] [CrossRef]
- Shi, J.; Gao, F.R. Integrated design and structure analysis of robust iterative learning control system based on a two-dimensional model. Ind. Eng. Chem. Res. 2005, 44, 8095–8105. [Google Scholar] [CrossRef]
- Wang, L.; Mo, S.Y.; Qu, H.Y. H∞ design of 2D controller for batch processes with uncertainties and interval time-varying delays. Control Eng. Pract. 2013, 21, 1321–1333. [Google Scholar] [CrossRef]
- Tao, H.F.; Liu, Y.; Yang, H.Z. Output Information Based Fault-Tolerant Iterative Learning Control for Dual-Rate Sampling Process with Disturbances and Output Delay. Math. Probl. Eng. 2018, 1–15. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, Y.M.; Ruan, X.E. Iterative learning control for a class of uncertain nonlinear systems with current state feedback. Int. J. Syst. Sci. 2019, 50, 1889–1901. [Google Scholar] [CrossRef]
- Yu, L. An LMI approach to reliable guaranteed cost control of discrete-time systems with actuator failure. Appl. Math. Comput. 2005, 162, 1325–1331. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Shi, J.; Zhou, D.H. Iterative learning fault-tolerant control for batch processes. Ind. Eng. Chem. Res. 2006, 45, 9050–9060. [Google Scholar] [CrossRef]
- Du, C.; Xie, L.; Zhang, C. H∞ control and robust stabilization of two-dimensional systems in Roesser models. Automatica 2001, 37, 205–211. [Google Scholar] [CrossRef]
- Boyd, S.; Ghaoui, L.E. Linear Matrix Inequalities in System and Control Theory, 3rd ed.; Society for Industrial Mathematics: Philadelphia, PA, USA, 2008. [Google Scholar]
- Sun, M.X.; Huang, B.J. Iterative Learning Control, 3rd ed.; National Defence Industry Press: Beijing, China, 1999. [Google Scholar]
- Wang, L.; Yang, H.Z.; Tao, H.F. Dynamic iterative learning control for linear repetitive processes over finite frequency ranges. Control Decis. 2021, 36, 599–608. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Dong, L.; Chen, Y.; Wang, K.; Gao, F. Iterative Learning Control for Actuator Fault Uncertain Systems. Symmetry 2022, 14, 1969. https://doi.org/10.3390/sym14101969
Wang L, Dong L, Chen Y, Wang K, Gao F. Iterative Learning Control for Actuator Fault Uncertain Systems. Symmetry. 2022; 14(10):1969. https://doi.org/10.3390/sym14101969
Chicago/Turabian StyleWang, Lei, Liangxin Dong, Yiyang Chen, Keqing Wang, and Feng Gao. 2022. "Iterative Learning Control for Actuator Fault Uncertain Systems" Symmetry 14, no. 10: 1969. https://doi.org/10.3390/sym14101969
APA StyleWang, L., Dong, L., Chen, Y., Wang, K., & Gao, F. (2022). Iterative Learning Control for Actuator Fault Uncertain Systems. Symmetry, 14(10), 1969. https://doi.org/10.3390/sym14101969






