Model Test Study on the Influence of Ground Surcharges on the Deformation of Shield Tunnels
Abstract
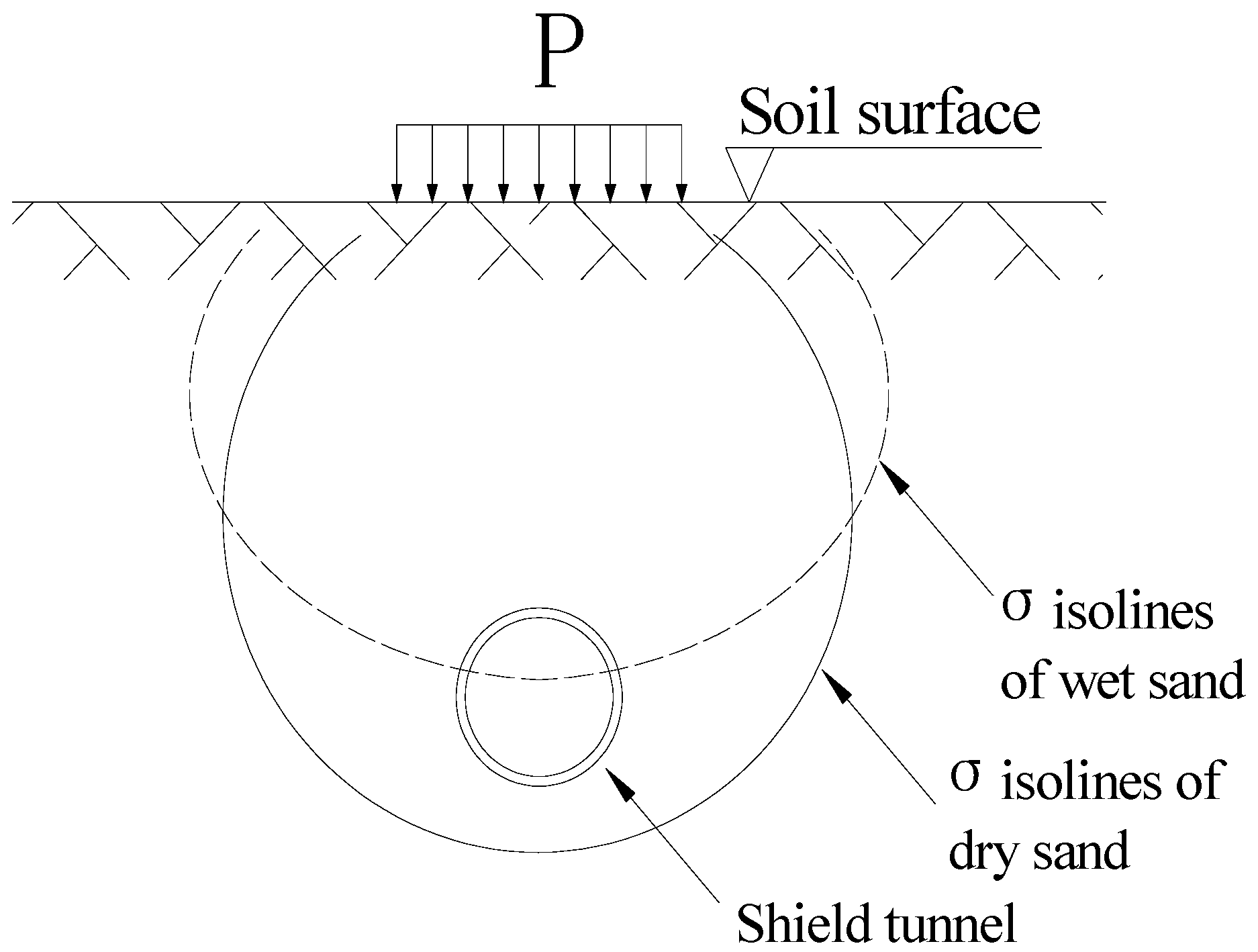
:1. Introduction
2. Indoor Scaled Model Test
2.1. Introduction of Test Model
- (1)
- Shield tunnel model
- (2)
- Indoor model box
2.2. Test Soil Material
2.3. Test Conditions
2.4. Test Procedure
3. Analysis of Indoor Model Test Results
3.1. Analysis of Test Data under Standard Operating Condition
3.2. Analysis of Influencing Factors
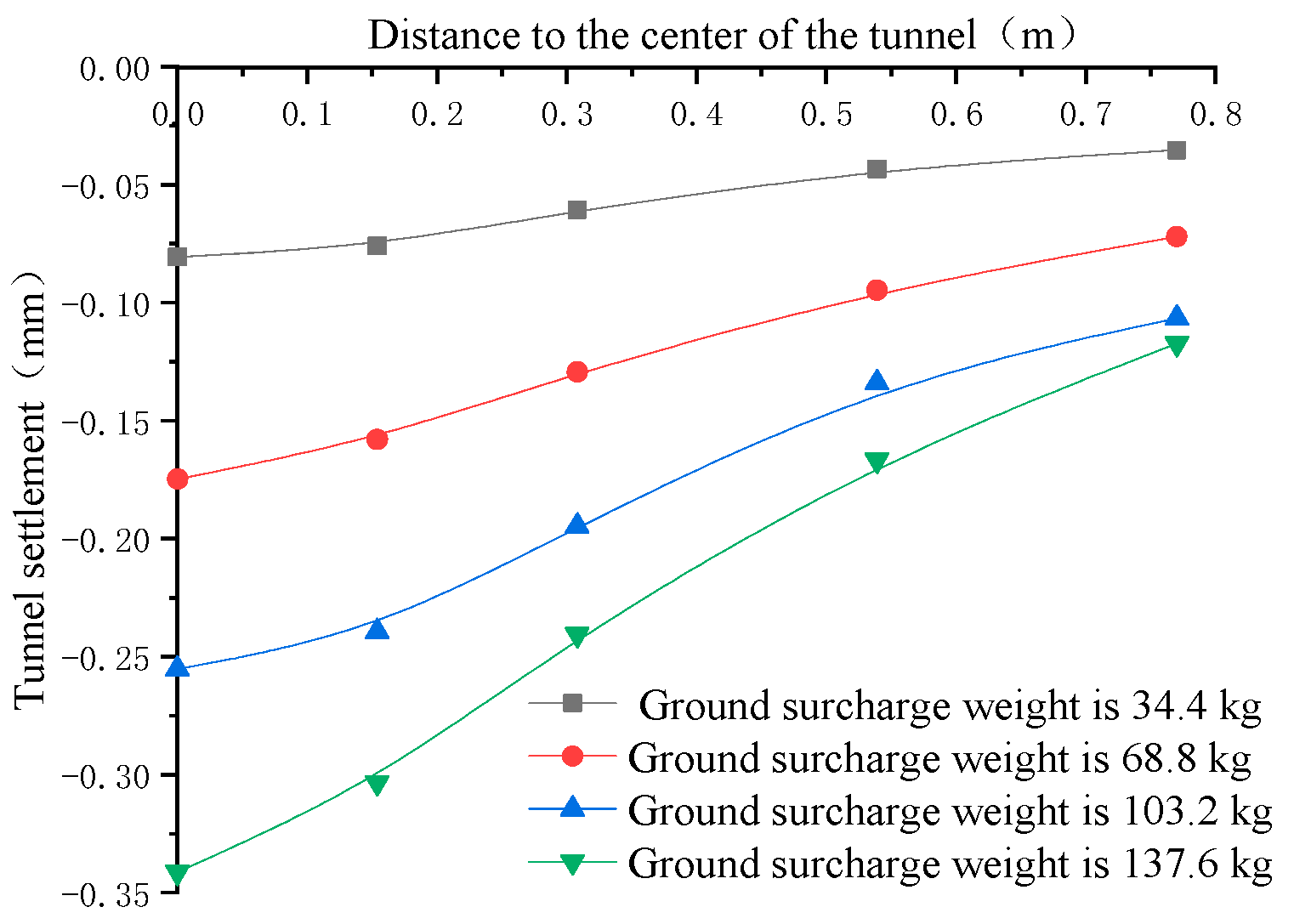
3.2.1. Influence of Ground Surcharge on Vertical Convergence Deformation and Settlement of Tunnel
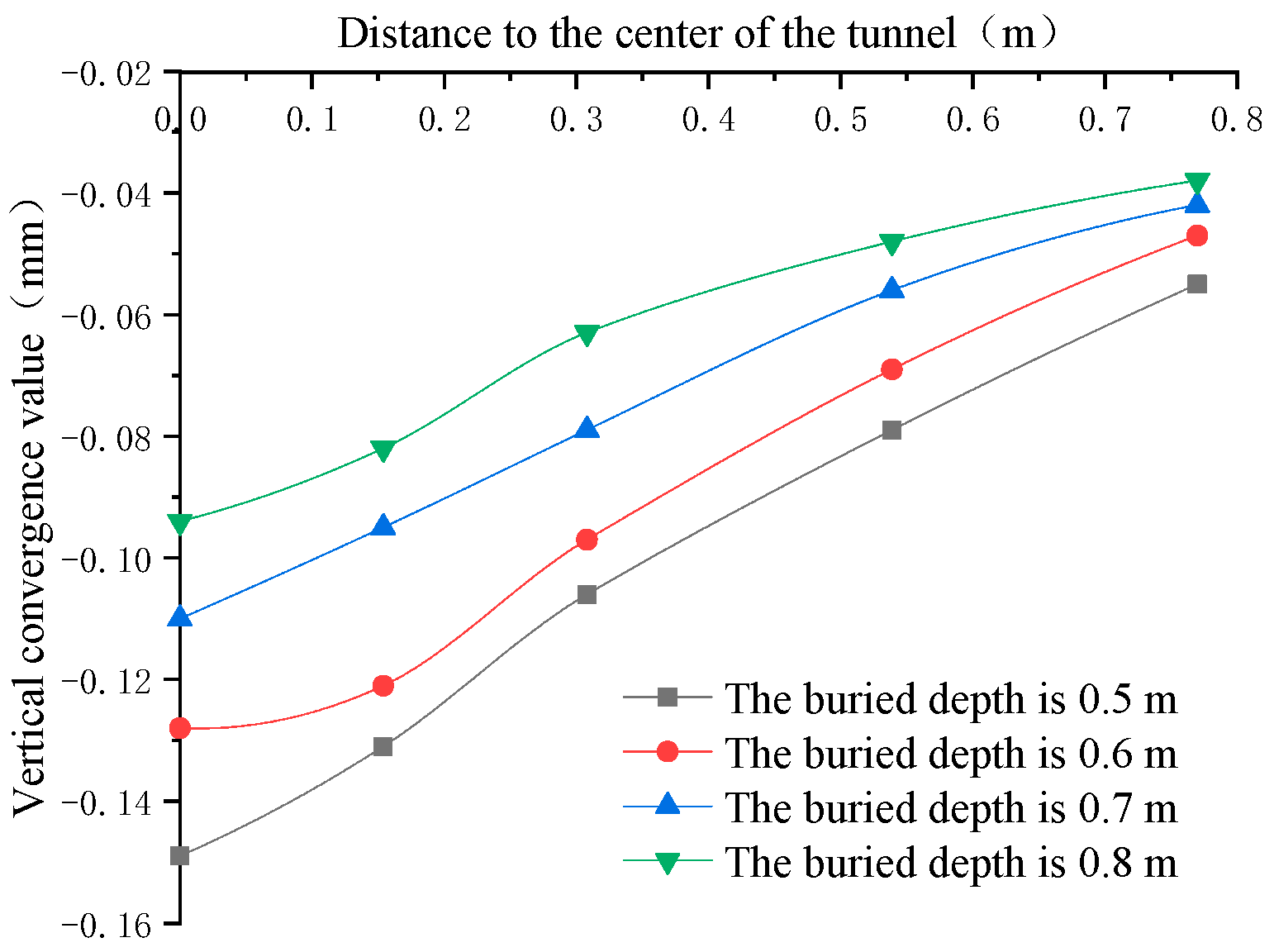
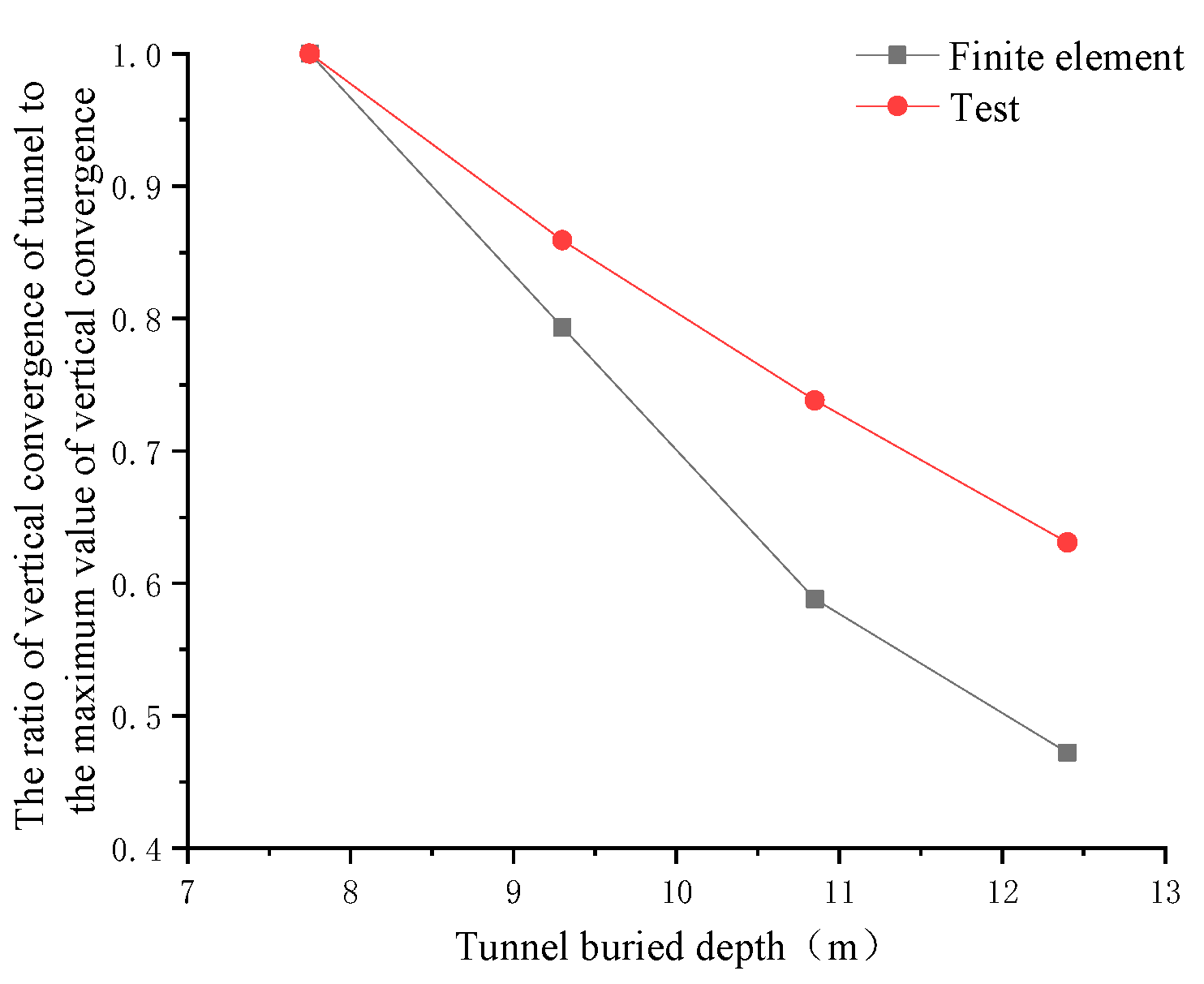
3.2.2. Influence of Tunnel Depth on Vertical Deformation and Settlement of Tunnel
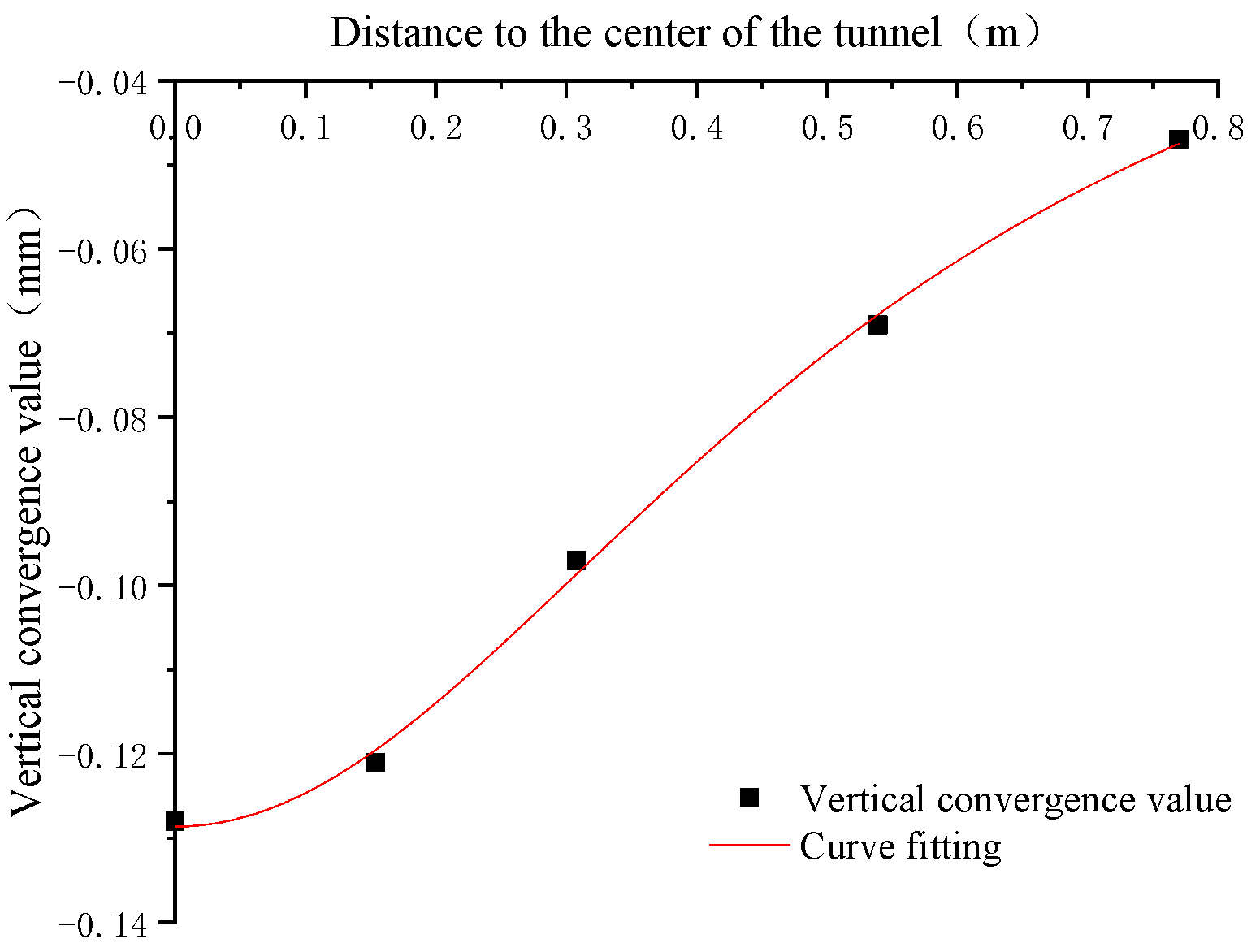
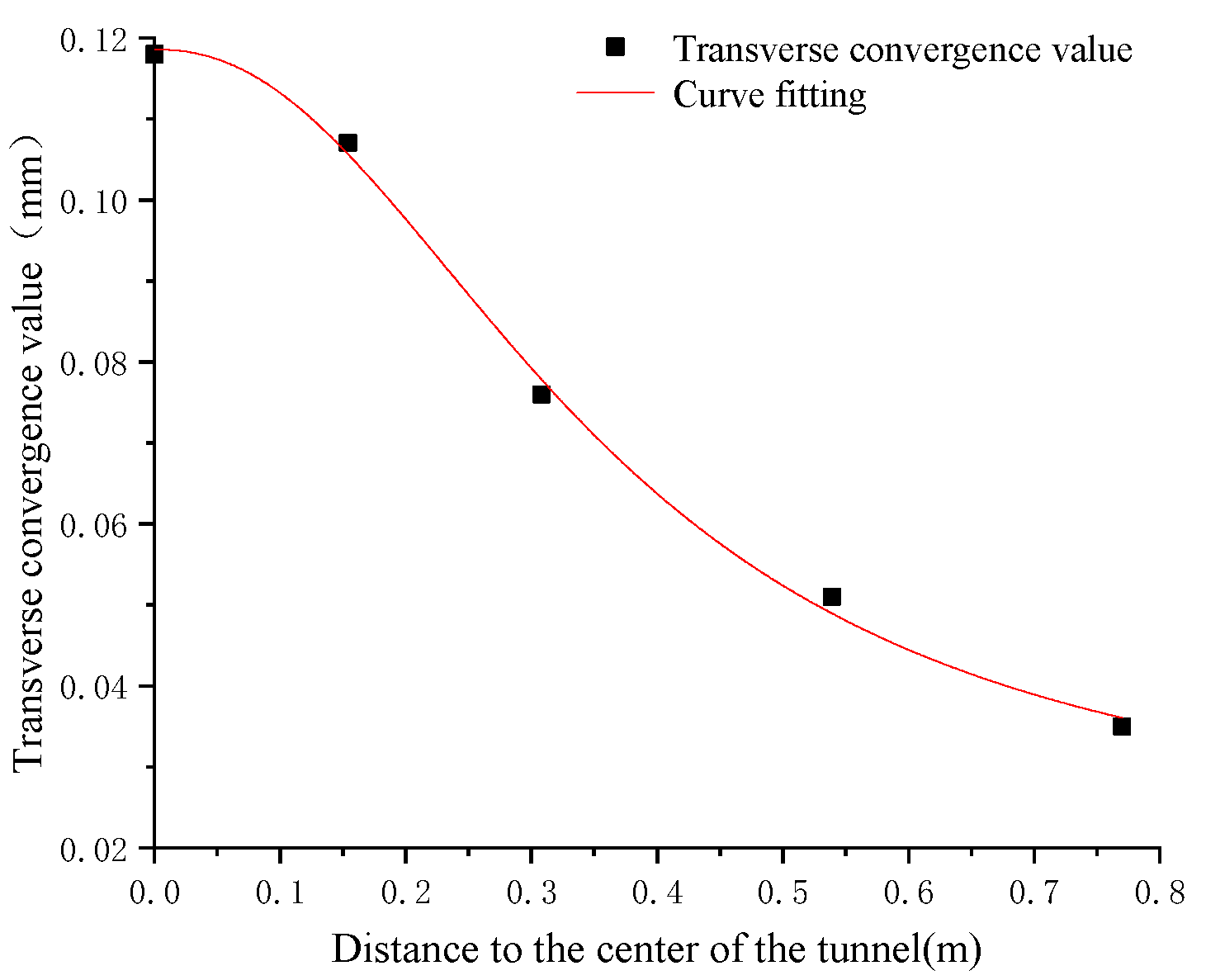
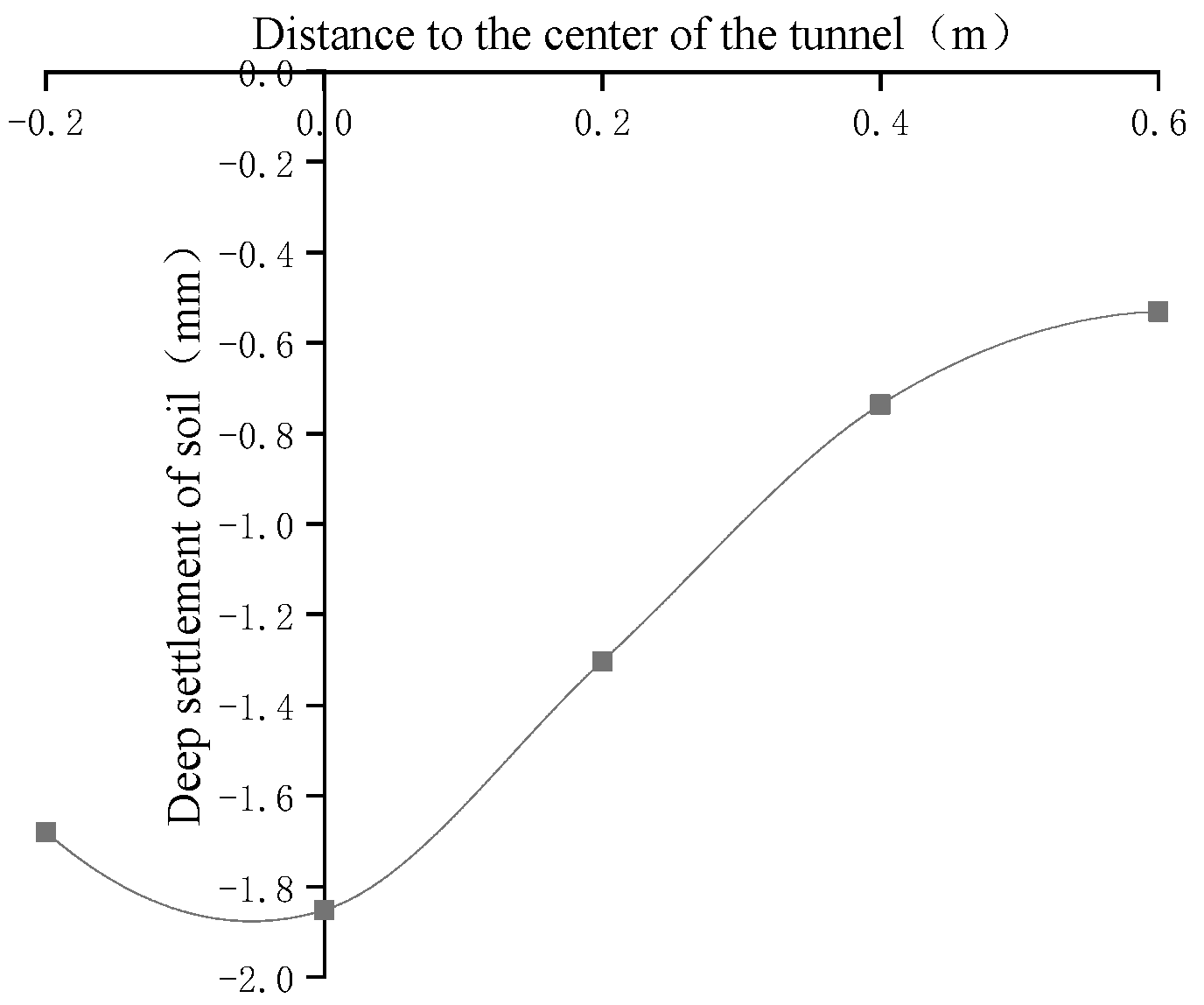
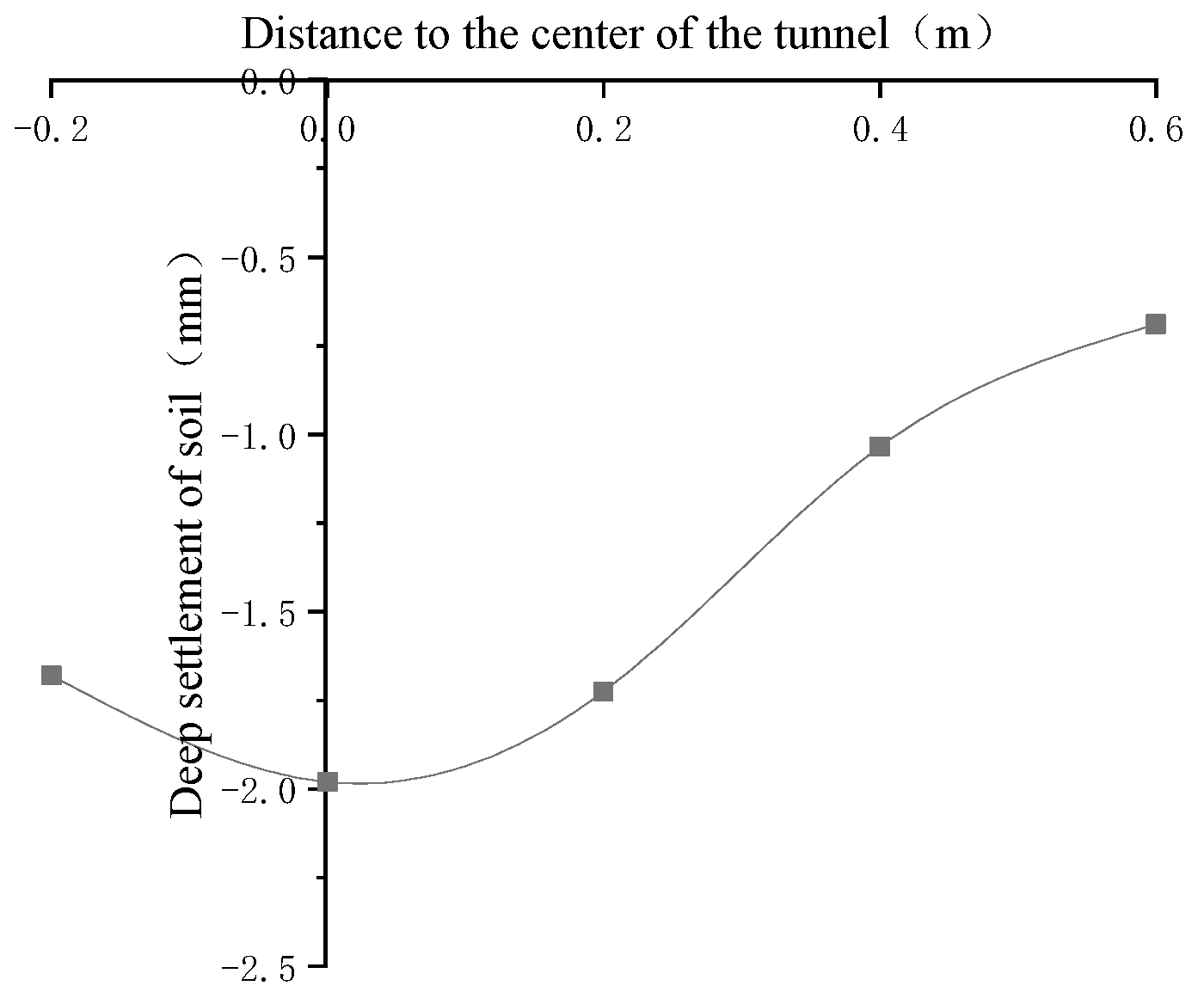
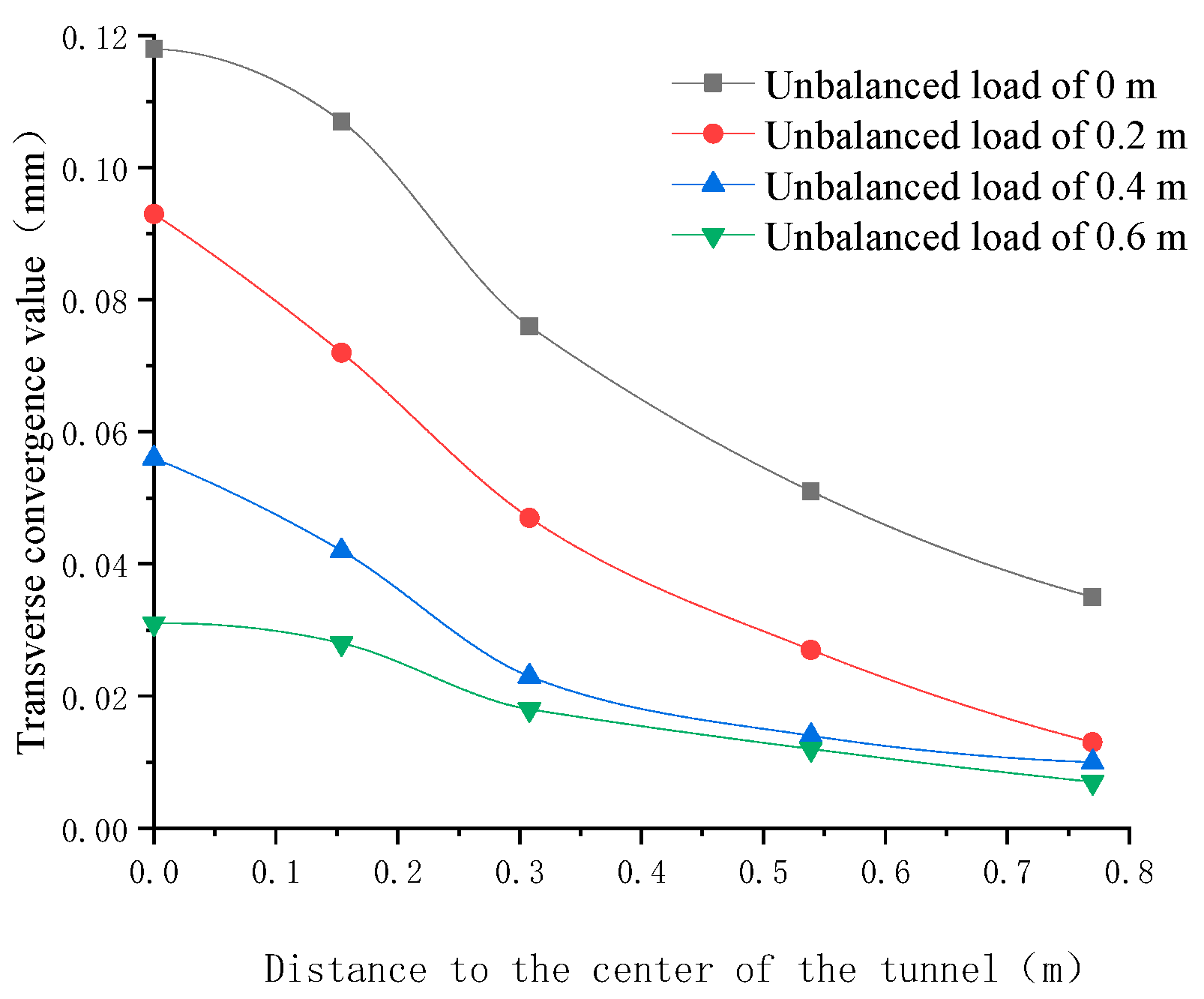
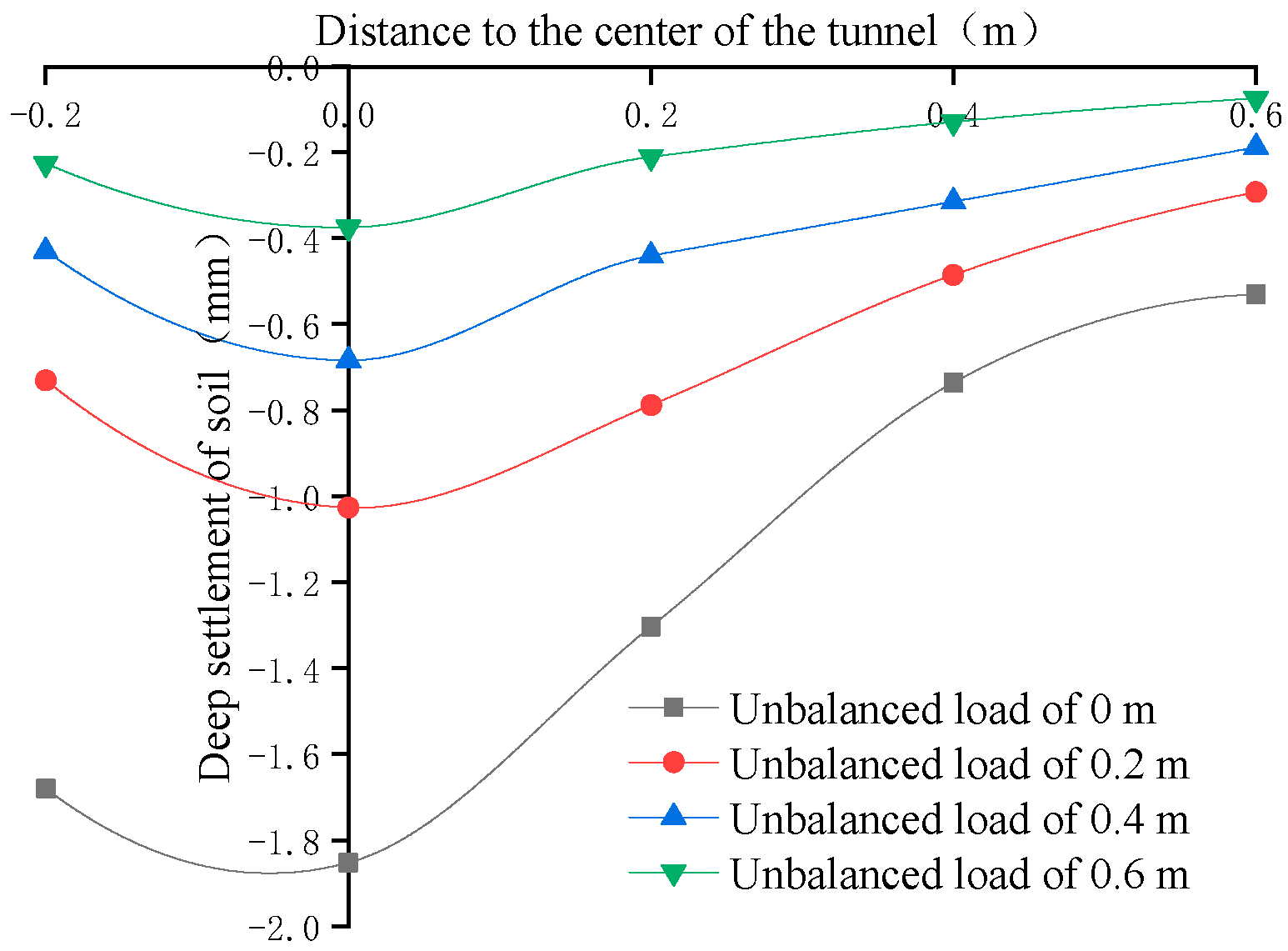
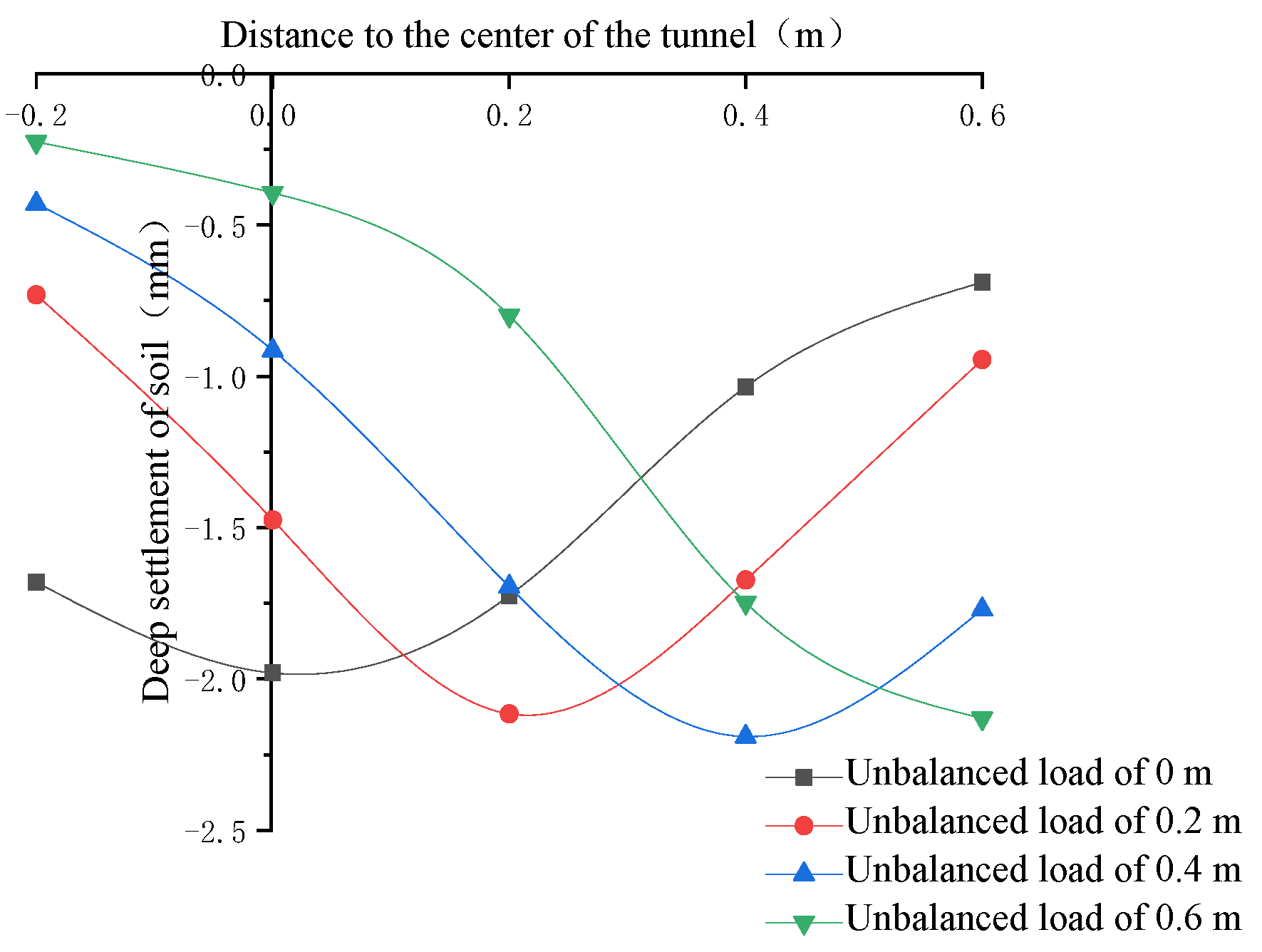
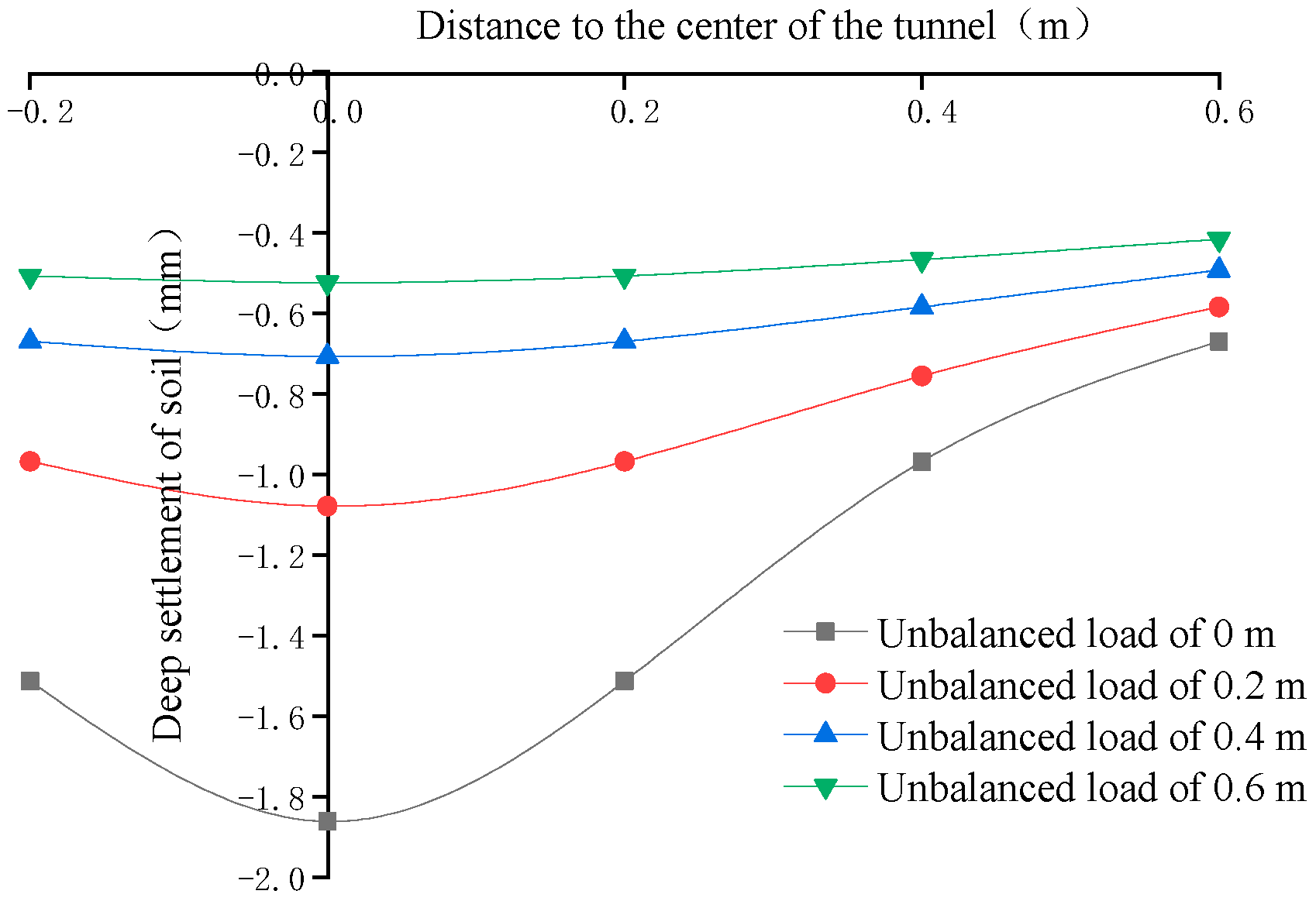
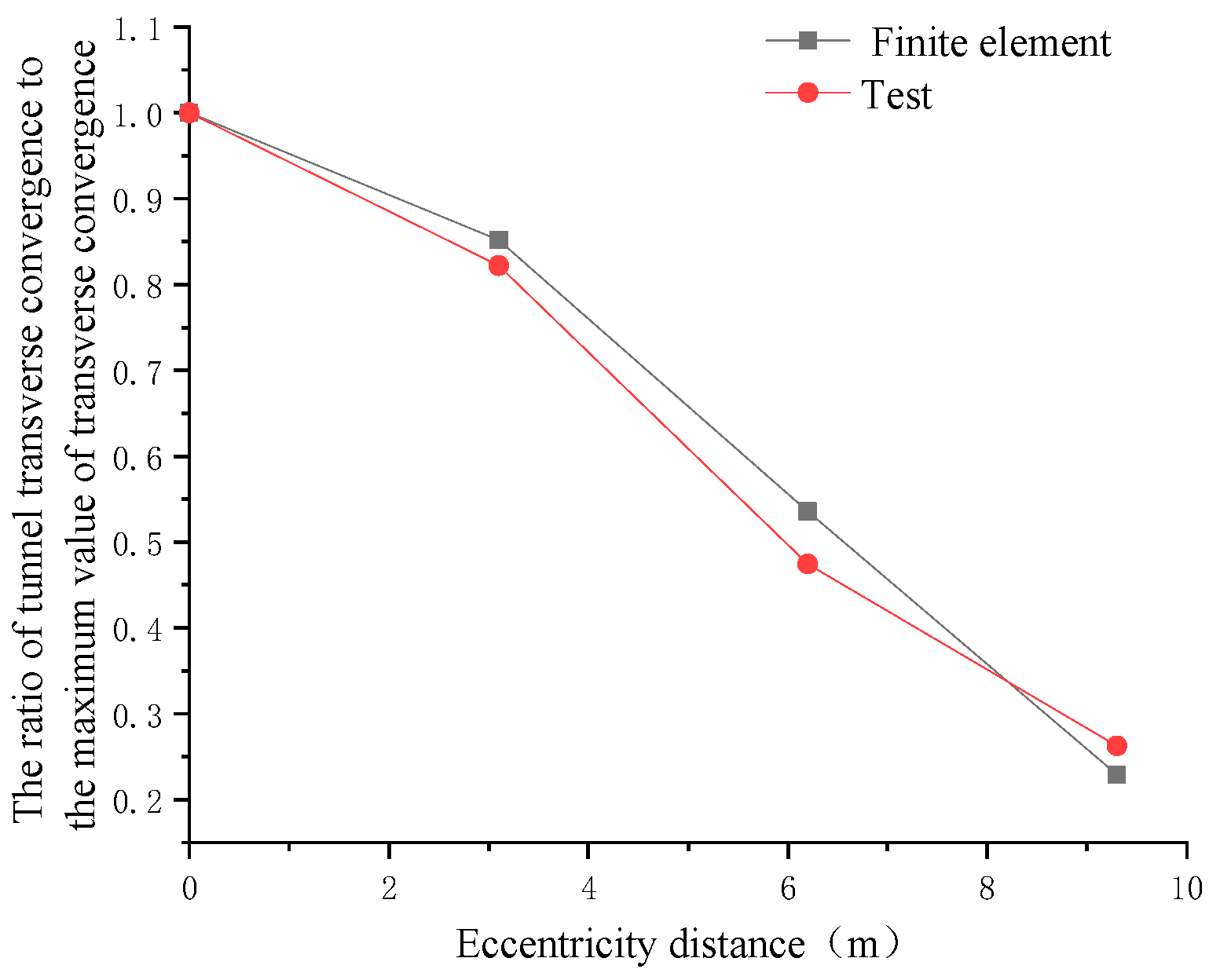
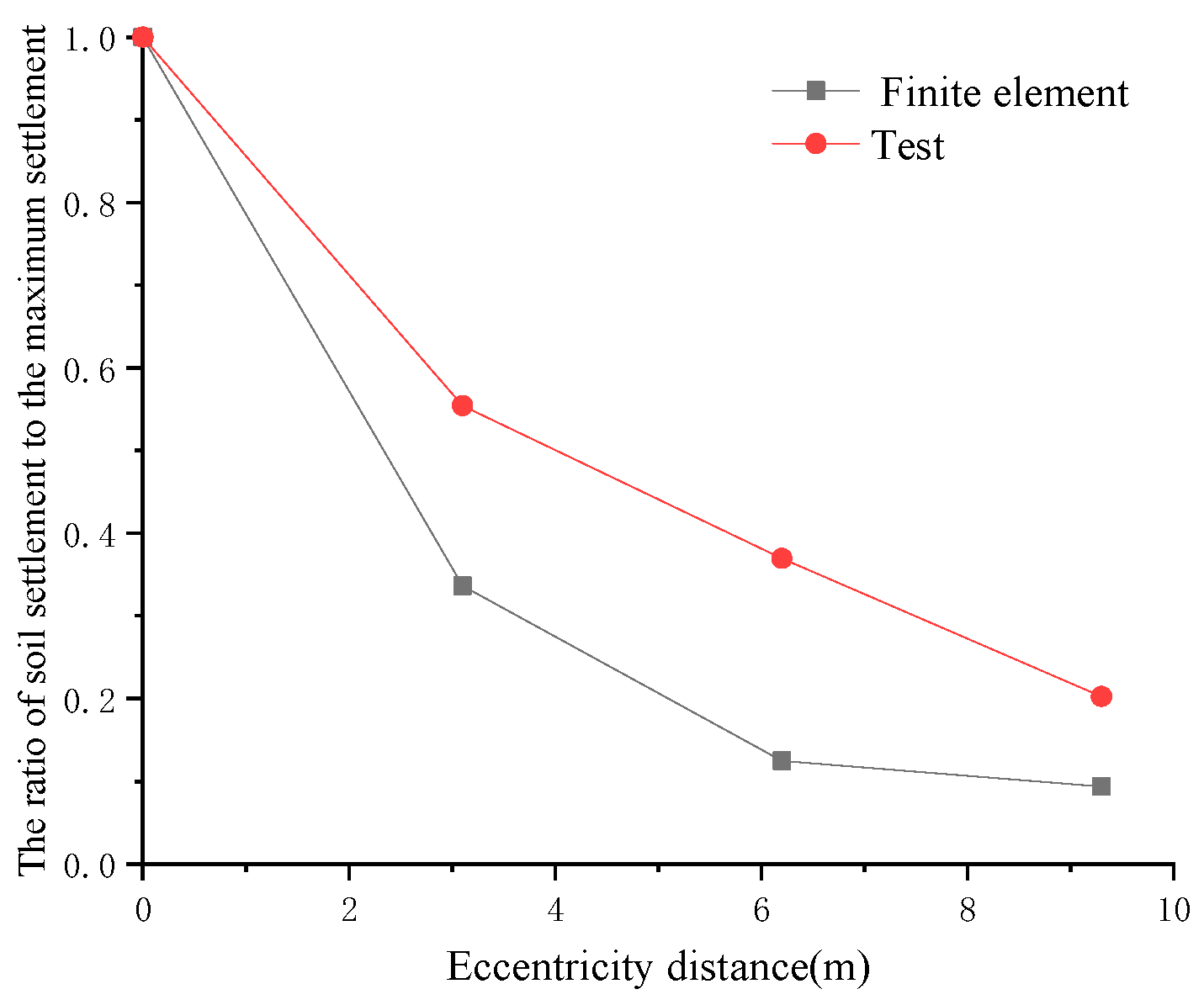
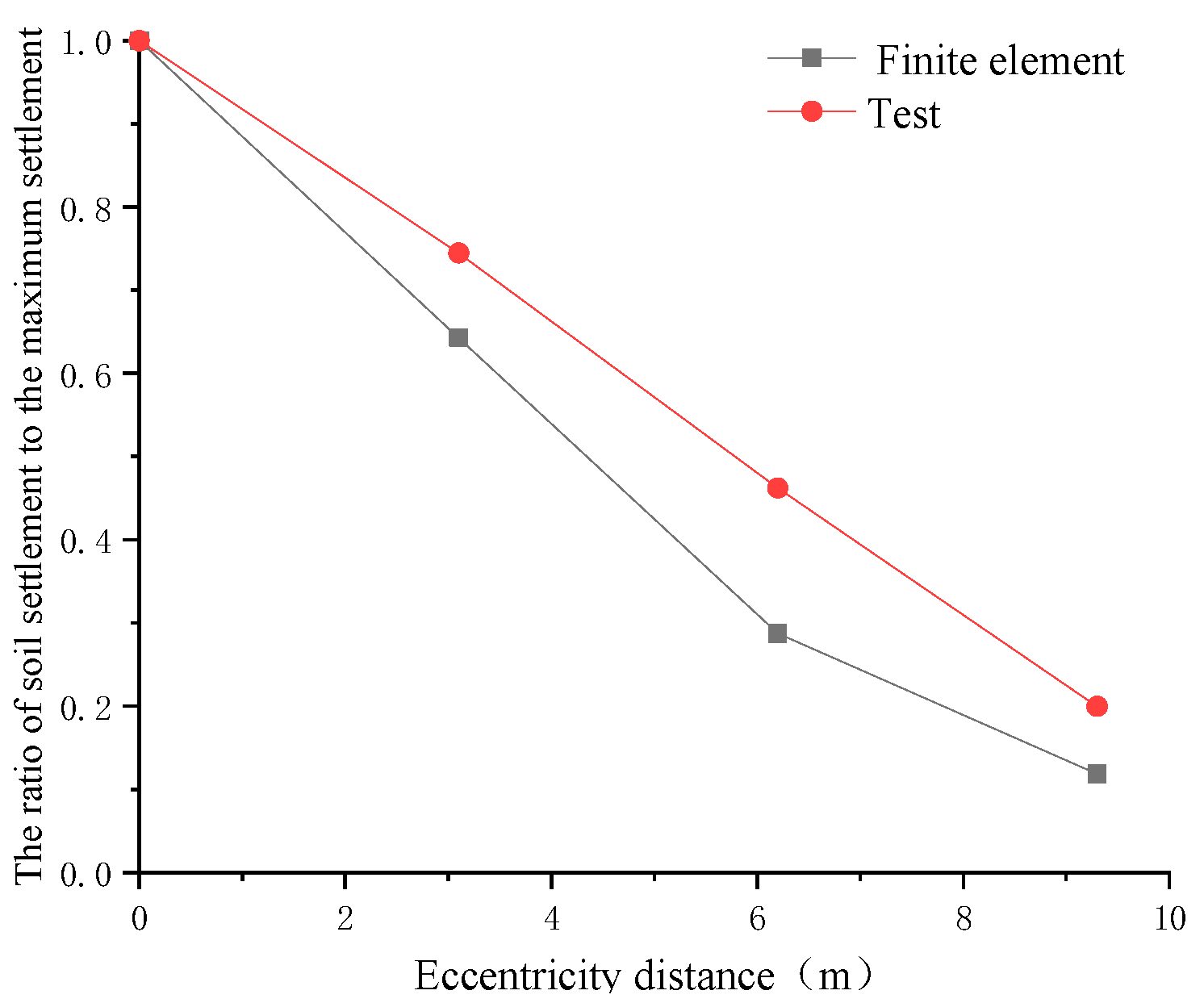
3.2.3. The Influence of Ground Surcharge Position on Tunnel Transverse Convergence Deformation and Deep Settlement of Soil
- (1)
- Analysis of model test results
- (2)
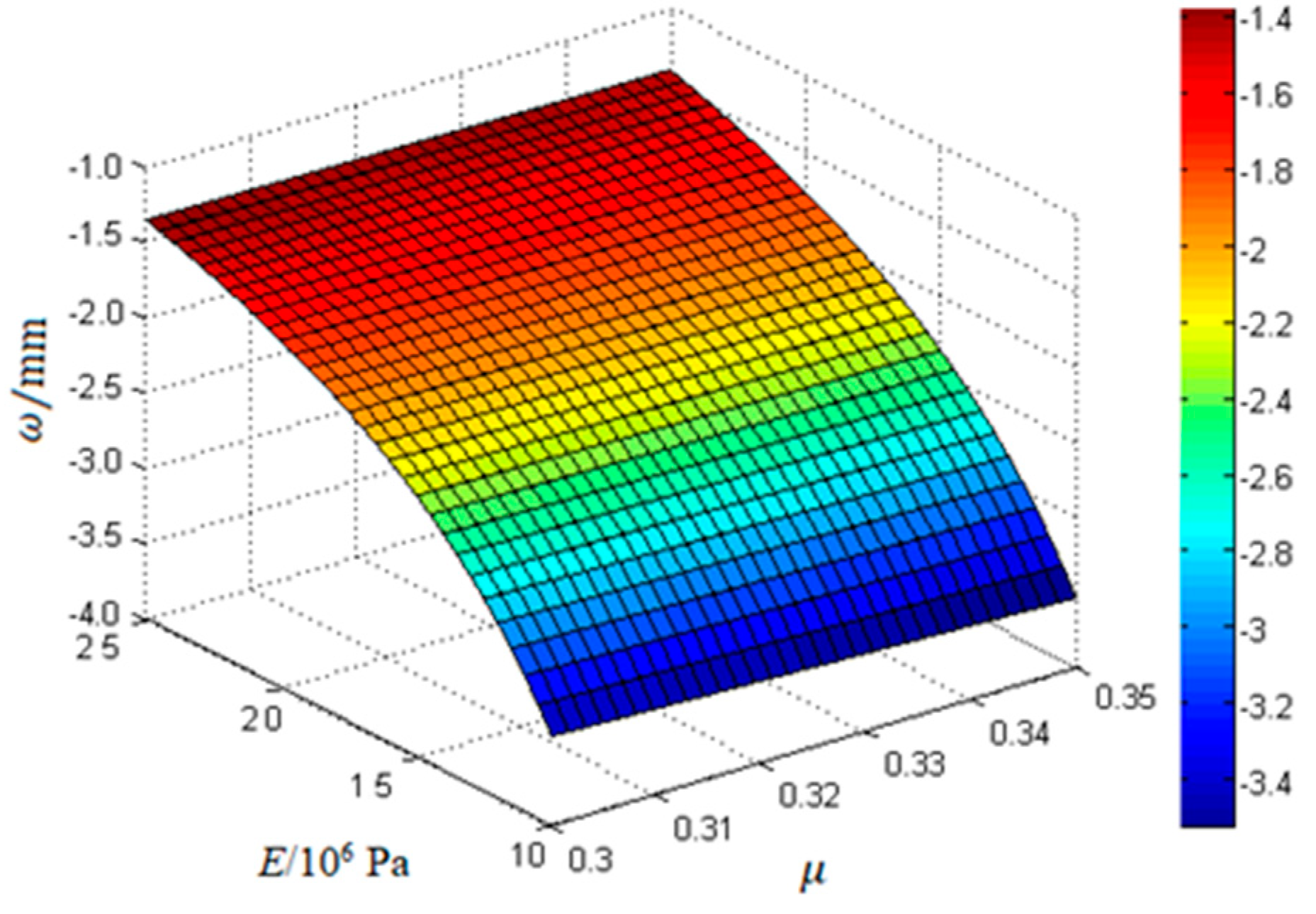
- Verification of the Boussinesq solution
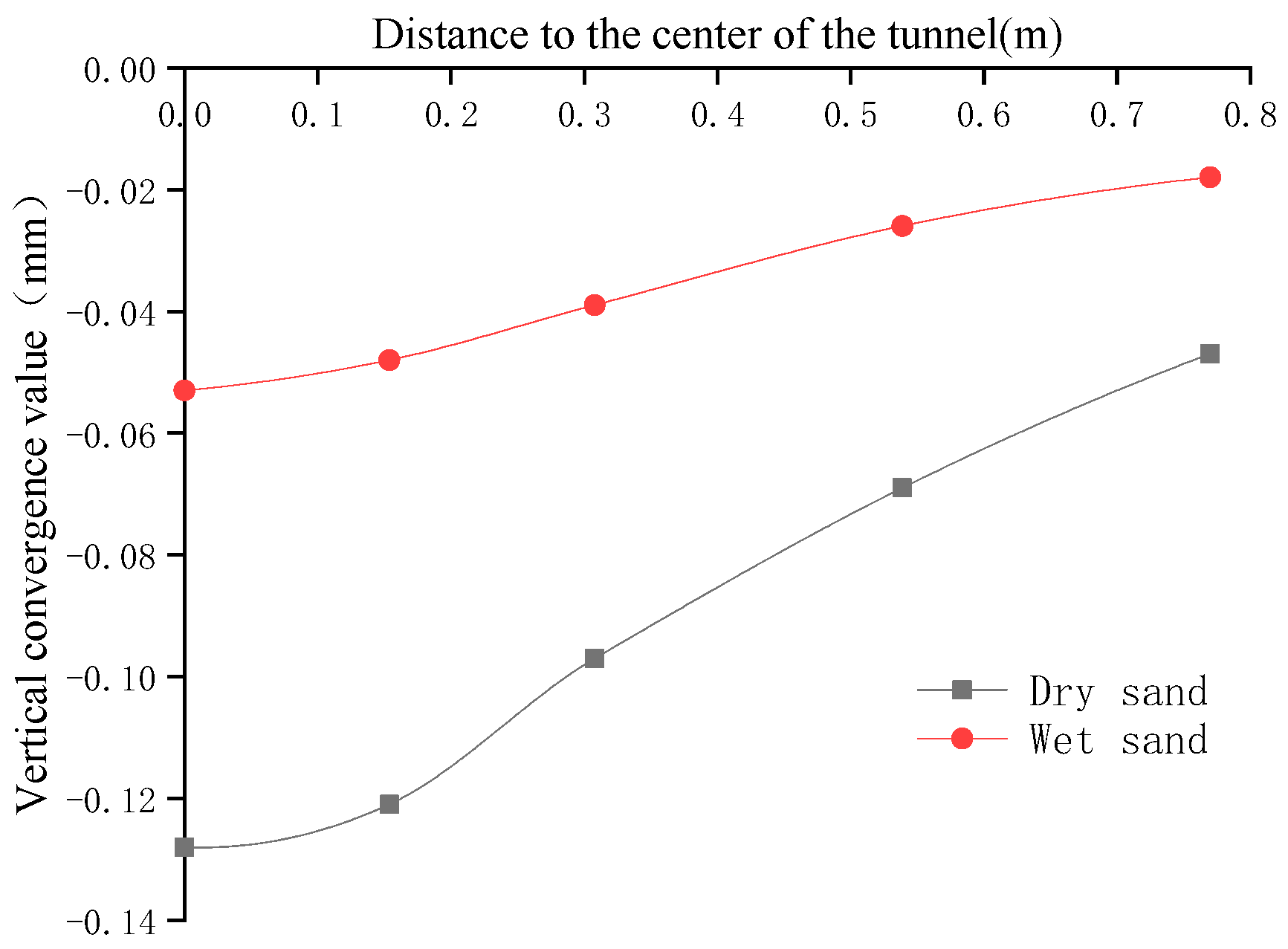
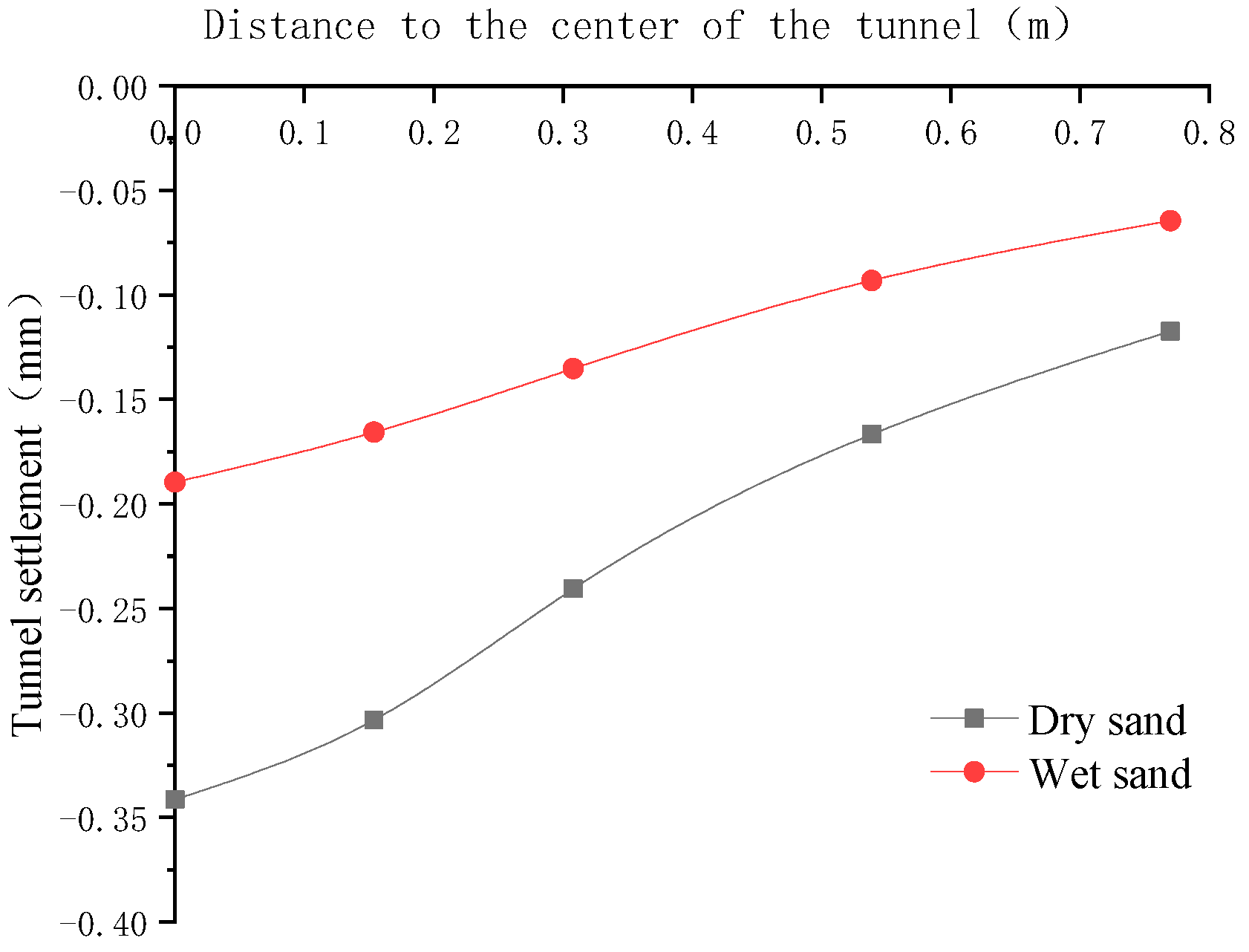
3.2.4. Influence of Different Soil Properties on Vertical Convergence Deformation and Settlement of Tunnel
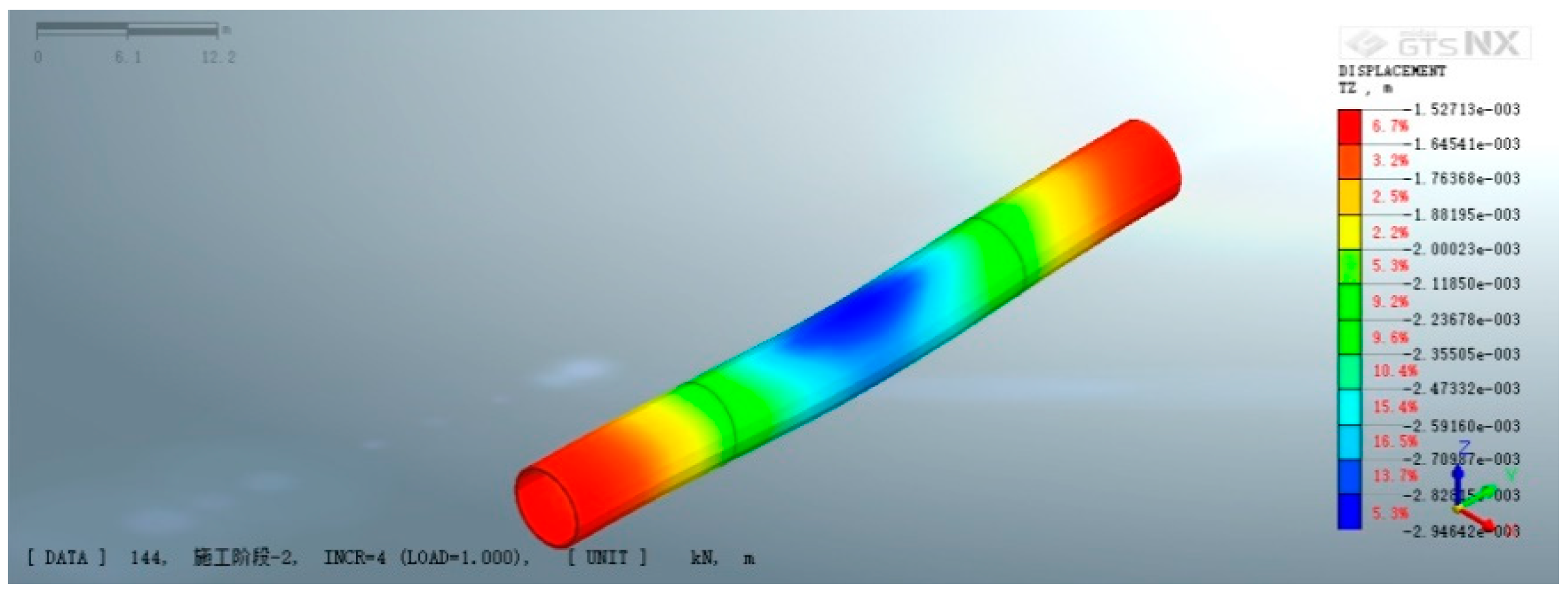
4. Comparative Analysis of Indoor Model Test and Numerical Simulation
4.1. Establishment of 3D Finite Element Model
4.2. Model Calculation Parameters
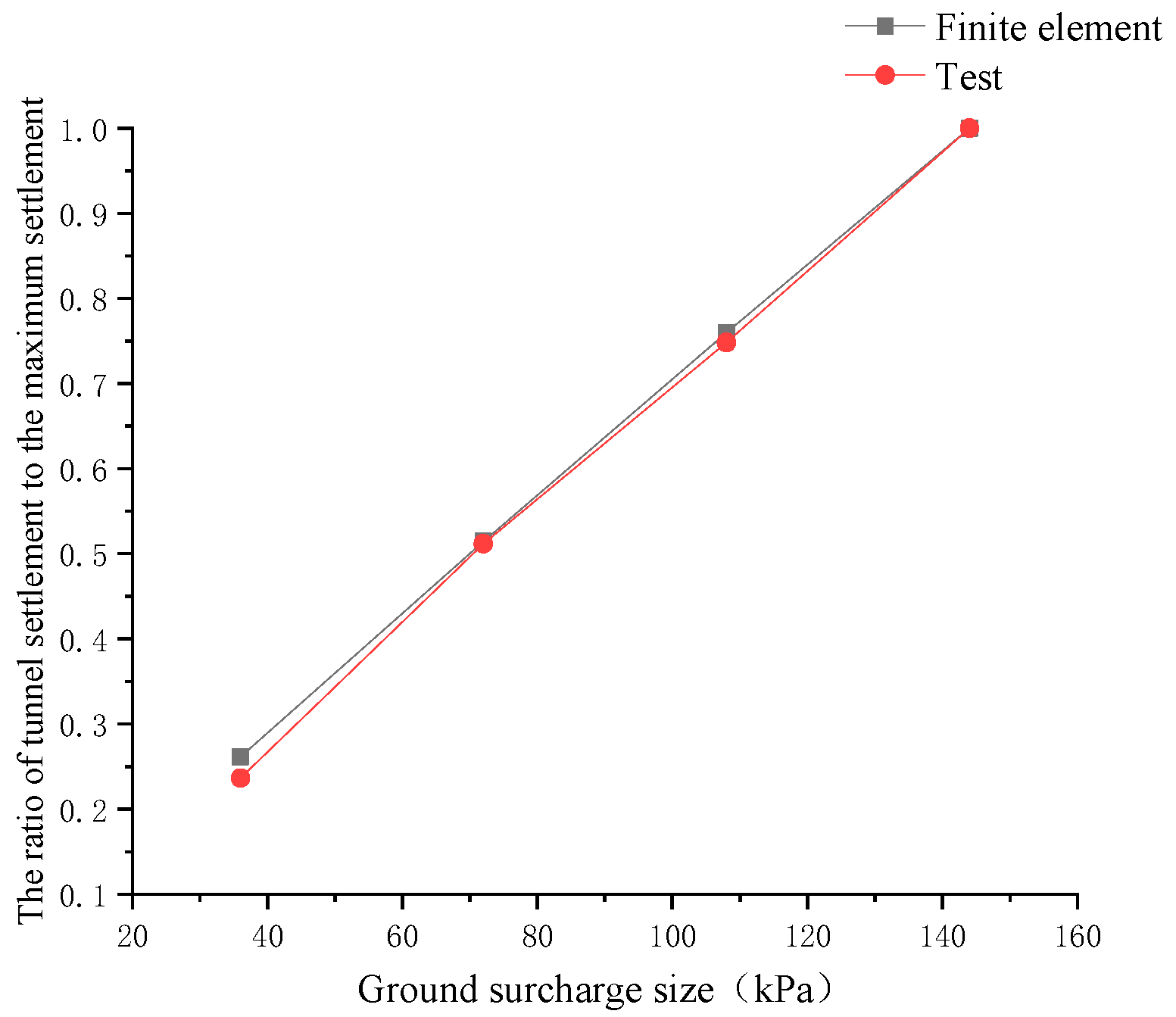
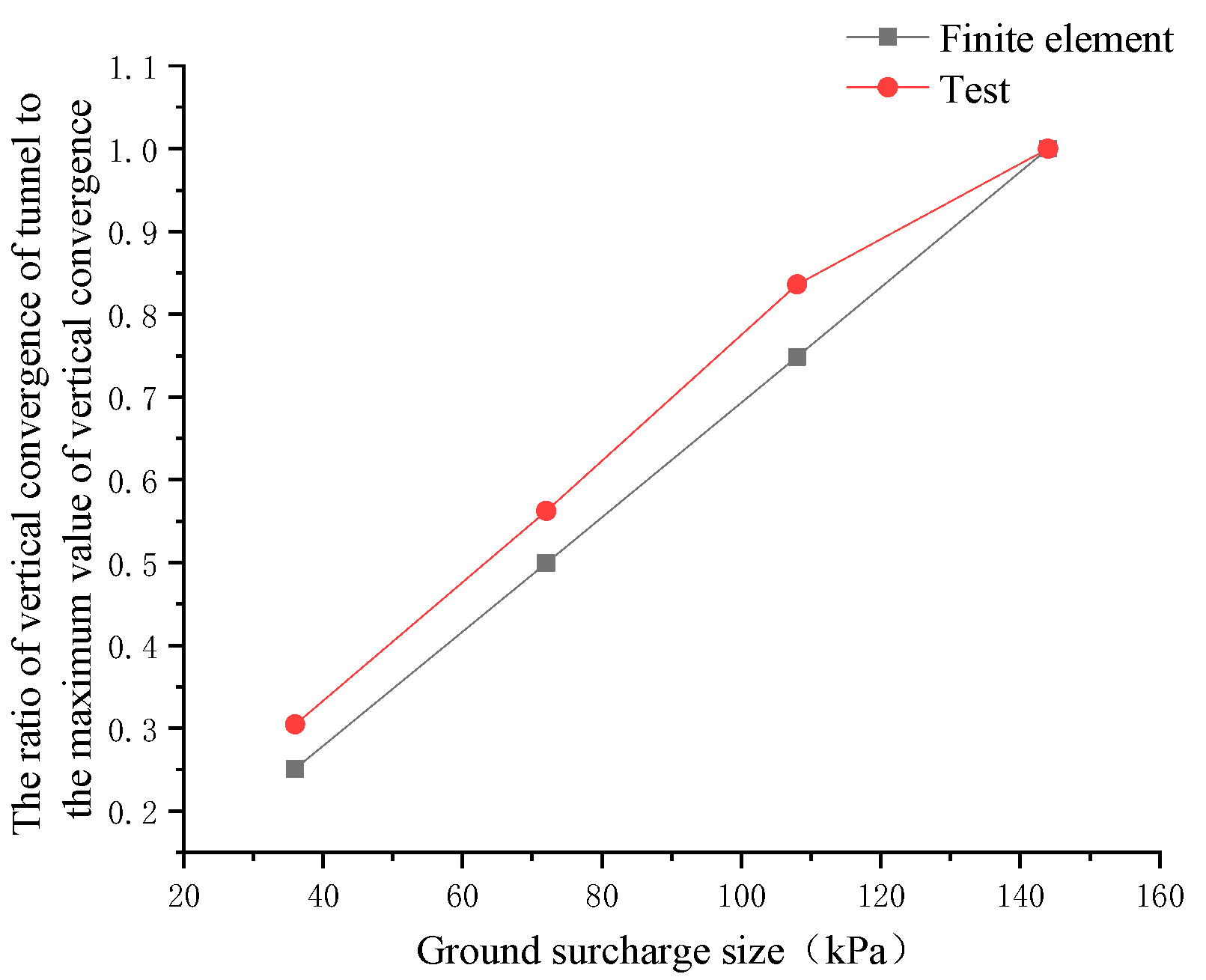
4.3. Comparative Analysis of Simulation Results and Test Results
5. Conclusions
- (1)
- The maximum values of tunnel settlement, vertical convergence deformation, transverse convergence deformation and deep settlement of soil all occur at the nearest place to the center of ground surcharge, which is the weakest point of the tunnel under ground surcharge conditions.
- (2)
- When the ground surcharge is the same, the vertical convergence deformation and settlement of the shield tunnel are obviously reduced with the increase of the burial depth of the shield tunnel, which indicates that increasing the burial depth of the shield tunnel can reduce the harm caused by ground surcharges.
- (3)
- Compared with the dry sand, when the test soil is wet sand, the vertical convergence deformation and settlement of the tunnel are significantly reduced, indicating that the nature of the soil has a great impact on the vertical convergence deformation and settlement of the tunnel, and the wet sand is more resistant to the vertical convergence deformation and settlement of the tunnel caused by the ground surcharge than the dry sand.
- (4)
- In calculating the deep settlement of soil caused by surface loading using the Boussinesq solution, the Poisson’s ratio μ and the modulus of elasticity E of the soil have a greater influence on the calculation results of the deep settlement of the soil, and the influence of E on the deep settlement of the soil is greater than that of when comparing the two.
- (5)
- The finite element method can simulate well the variation laws of transverse and vertical convergence deformation, tunnel settlement and deep settlement of soil caused by sudden surcharges on the ground. Using less model tests to determine the model and parameters of finite element, and then using finite element to carry out a large number of simulations can improve the research efficiency while ensuring the research accuracy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, J.T.; Chen, S.W.; Ye, Z.W. Analysis of disease and structural safety of shield tunnel under accidental surface surcharge. J. Railw. Eng. Soc. 2019, 36, 67–73. [Google Scholar]
- Shao, H.; Huang, H.W.; Zhang, D.M.; Wang, R.L. Case study on repair work for excessively deformed shield tunnel under accidental surface surcharge in soft clay. Chin. J. Geotech. Eng. 2016, 38, 1036–1043. [Google Scholar]
- Lin, Y.G.; Liao, S.M.; Liu, G.B. A discussion of the factors effecting on longitudinal deformation of subway tunnel. Undergr. Space 2000, 20, 264–267, 289. [Google Scholar]
- Lo, K.Y.; Ramsay, J.A. The effect of construction on existing subway tunnels—A case study from Toronto. Tunn. Undergr. Space Technol. 1991, 6, 287–297. [Google Scholar] [CrossRef]
- Xie, J.C.; Wang, J.C.; Huang, W.M. Nonlinear structural analysis on cracking behavior of shield tunnel segment under surface loading. J. Railw. Sci. Eng. 2021, 18, 162–171. [Google Scholar]
- Wei, G.; Hong, W.Q.; Wei, X.J.; Jiang, J.Q. Deformation law and control limit of shield tunnel cross-section under eccentric surcharge load. J. Cent. South Univ. (Sci. Technol.) 2020, 51, 750–757. [Google Scholar]
- Sang, Y.L.; Liu, X.Z.; Zhang, Q. Stiffness analysis and application of segment annular joint based on bolt-concave and convex tenon connection in metro shield tunnel. Tunn. Constr. 2020, 40, 19–27. [Google Scholar]
- Lu, D.Y.; Xu, G.W.; Wang, S.M. Effects of material damage and structural characteristics on tunnel shield during loading and unloading. J. Southwest Jiaotong Univ. 2017, 52, 1104–1112. [Google Scholar]
- Sun, L.W.; Qin, J.S.; Hong, Y.; Wang, L.Z.; Zhao, C.J.; Qin, X. Shield tunnel segment and circumferential joint performance under surface surcharge. J. Zhejiang Univ. (Eng. Sci.) 2017, 51, 1509–1518. [Google Scholar]
- Liu, X.; Zhang, H.L.; Lu, L.; Wang, X.Z. Experimental study on load bearing capacity of shield tunnel structure under overload condition. Undergr. Eng. Tunn. 2013, 4, 10–15, 59. [Google Scholar]
- Wu, Q.; Du, S.J. Model test on influence of ground heaped load on existing shield tunnel structure. Chin. J. Undergr. Space Eng. 2014, 10, 57–66. [Google Scholar]
- Huang, D.W.; Zhou, S.H.; Lai, G.Q.; Feng, Q.S.; Liu, L.Y. Mechanisms and characteristics for deterioration of shield tunnels under surface surcharge. Chin. J. Geotech. Eng. 2017, 39, 1173–1181. [Google Scholar]
- Atkinson, J.H.; Potts, D.M. Stability of a shallow circular tunnel in cohesionless soil. Geotechnique 1977, 27, 203–215. [Google Scholar] [CrossRef]
- Ma, Z.Z.; Luo, Z.H.; Zhang, J.; Xin, H.H. Study on Influence of Ground Surcharge on Deformation of Subway Shield Tunnel. Mod. Tunn. Technol. 2018, 55, 573–578. [Google Scholar]
- Lu, P.Y.; Wang, M.L.; Yang, J.M. Influences of surface surcharge on the deformation of shield tunnel in soft ground area. Build. Struct. 2019, 49, 999–1003. [Google Scholar]
- Wei, G.; Hong, W.Q.; Wei, X.J.; Lin, X.P.; Wang, L.Z. Theoretical calculations of transverse force on shield tunnel caused by eccentric load. J. Cent. South Univ. (Sci. Technol.) 2019, 50, 1645–1654. [Google Scholar]
- Kentaro, Y.; Andrei, V.L.; Daniel, W.W.; Scott, W.S.; Andrew, J.A. Stability of a circular tunnel in cohesive-frictional soil subjected to surcharge loading. Comput. Geotech. 2011, 38, 504–514. [Google Scholar]
- Kentaro, Y.; Andrei, V.L.; Daniel, W.W.; Scott, W.S.; Andrew, J.A. Stability of dual circular tunnels in cohesive-frictional soil subjected to surcharge loading. Comput. Geotech. 2013, 50, 41–54. [Google Scholar]
- Huang, H.W.; Zhang, D.M. Resilience analysis of shield tunnel lining under extreme surcharge: Characterization and field application. Tunn. Undergr. Space Technol. 2016, 51, 301–312. [Google Scholar] [CrossRef]
- Liang, F.Y.; Yuan, Q.; Li, J.P.; Zhang, S.X. Influences of soil characteristics on longitudinal deformation of shield tunnels induced by surface surcharge. Chin. J. Geotech. Eng. 2020, 42, 63–71. [Google Scholar]
- Sun, H.S.; Zhou, T.; Dong, Y.; Chen, Y.D.; Zhang, J.H. Effect of ground surface on the deformation of an existing tunnel. Chin. J. Undergr. Space Eng. 2019, 15, 900–907, 913. [Google Scholar]
- Kang, C.; Mei, G.X.; Liang, R.Z.; Wu, W.B.; Fang, Y.X.; Ke, Z.B. Analysis of the longitudinal deformation of existing shield tunnel induced by temporary surface surcharge. Rock Soil Mech. 2018, 39, 4605–4616. [Google Scholar]
- Mindlin, R.D. Force at a point in the interior of a semiinfinite solid. Physics 1936, 7, 195–202. [Google Scholar] [CrossRef]
- Malt, R.J.; Taylor, R.N. Bored tunneling in the urban environment. In Proceedings of the Fourteenth International Conference on Soil Mechanics and Foundation Engineering, Hamburg, Germany, 6–12 September 1997; pp. 2353–2380. [Google Scholar]
- Koyama, Y. Present Status and Technology of Shield Tunneling Method in Japan. Tunn. Undergr. Space Technol. 2003, 18, 145–159. [Google Scholar] [CrossRef]
- Ye, X.Y. Study on the Deformation Test of Subway Tunnel Based on Optical Fiber Sensing Technology. Master’s Thesis, Anhui University of Science & Technology, Huainan, China, 2018. [Google Scholar]



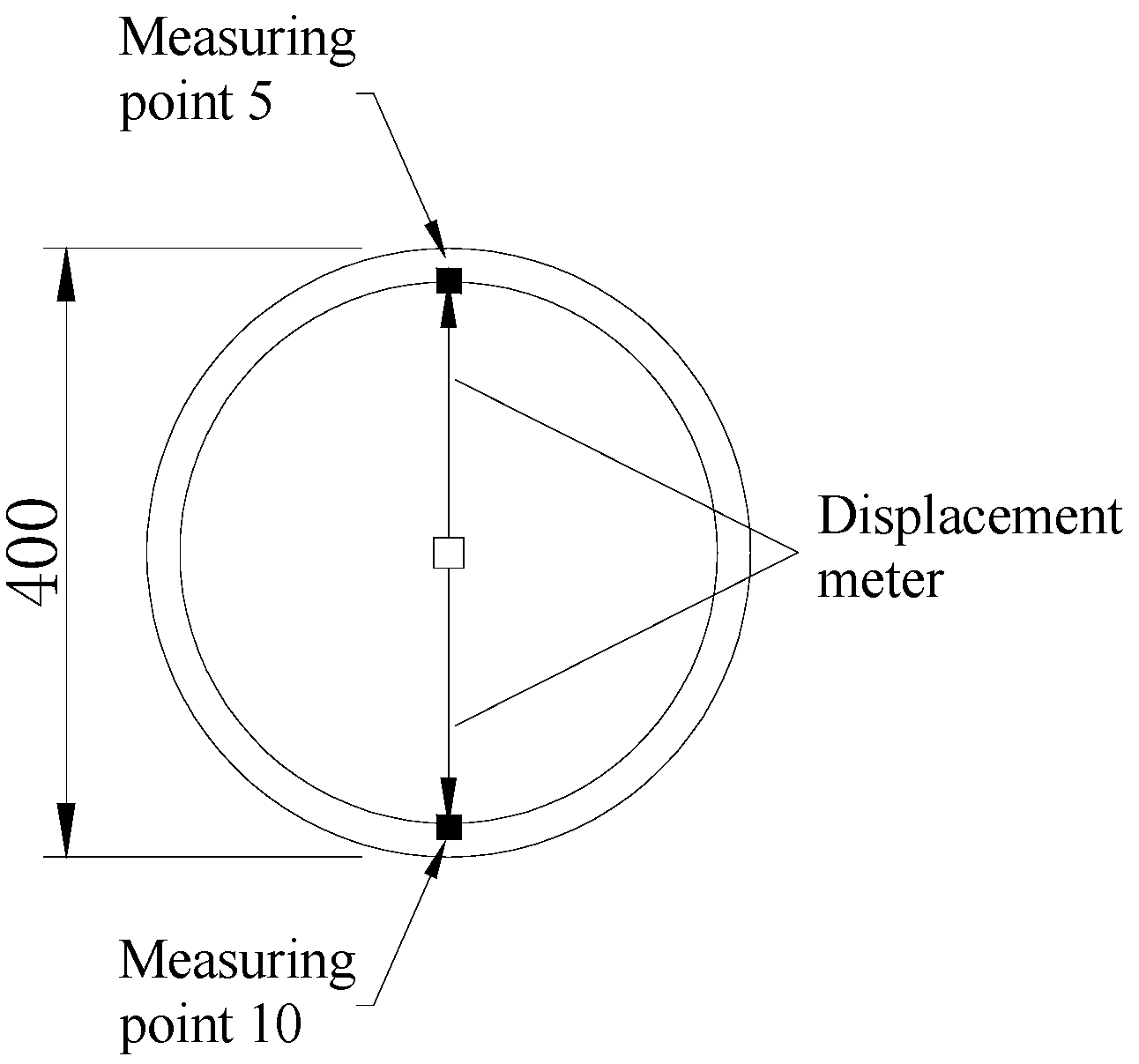
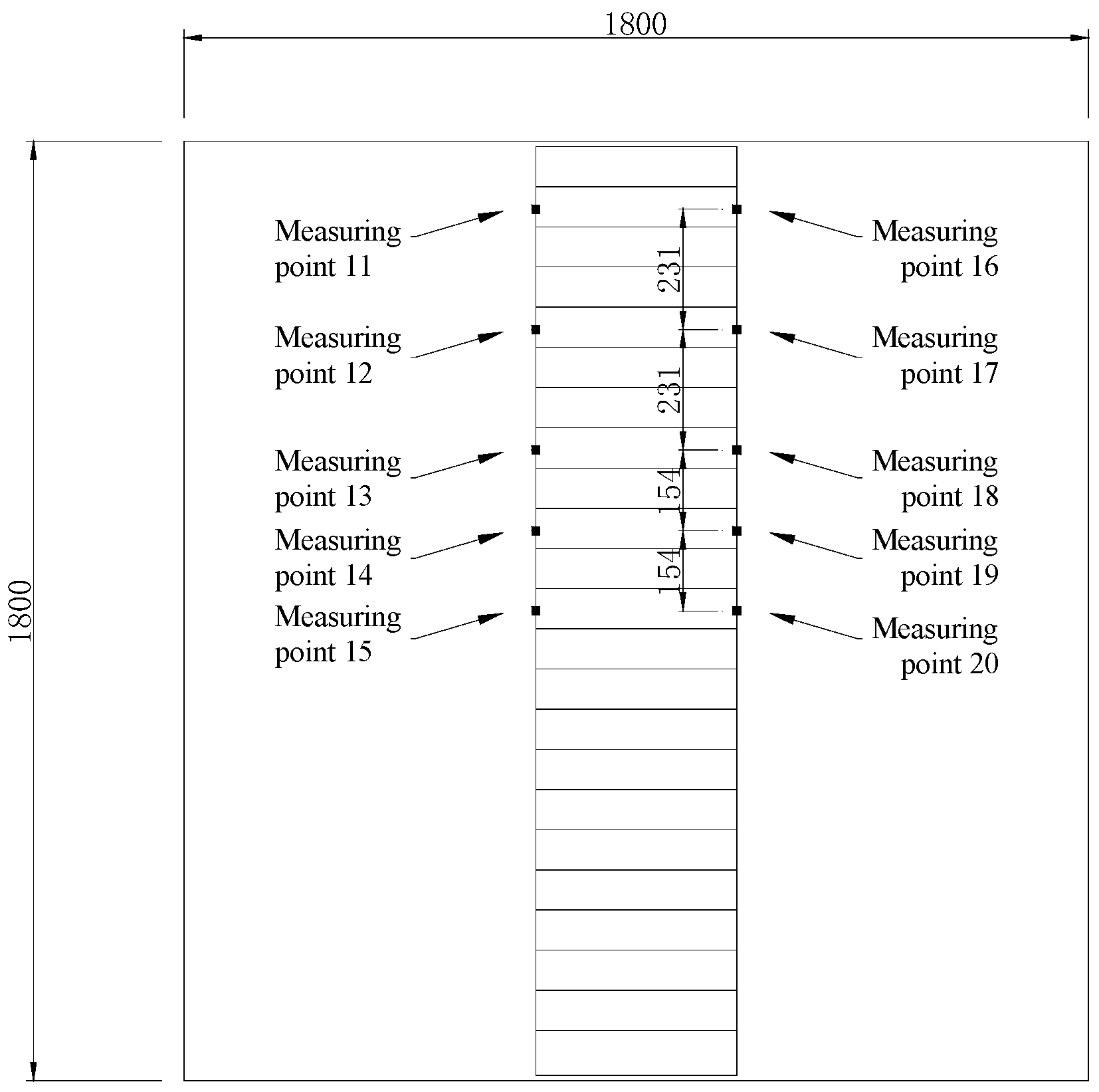

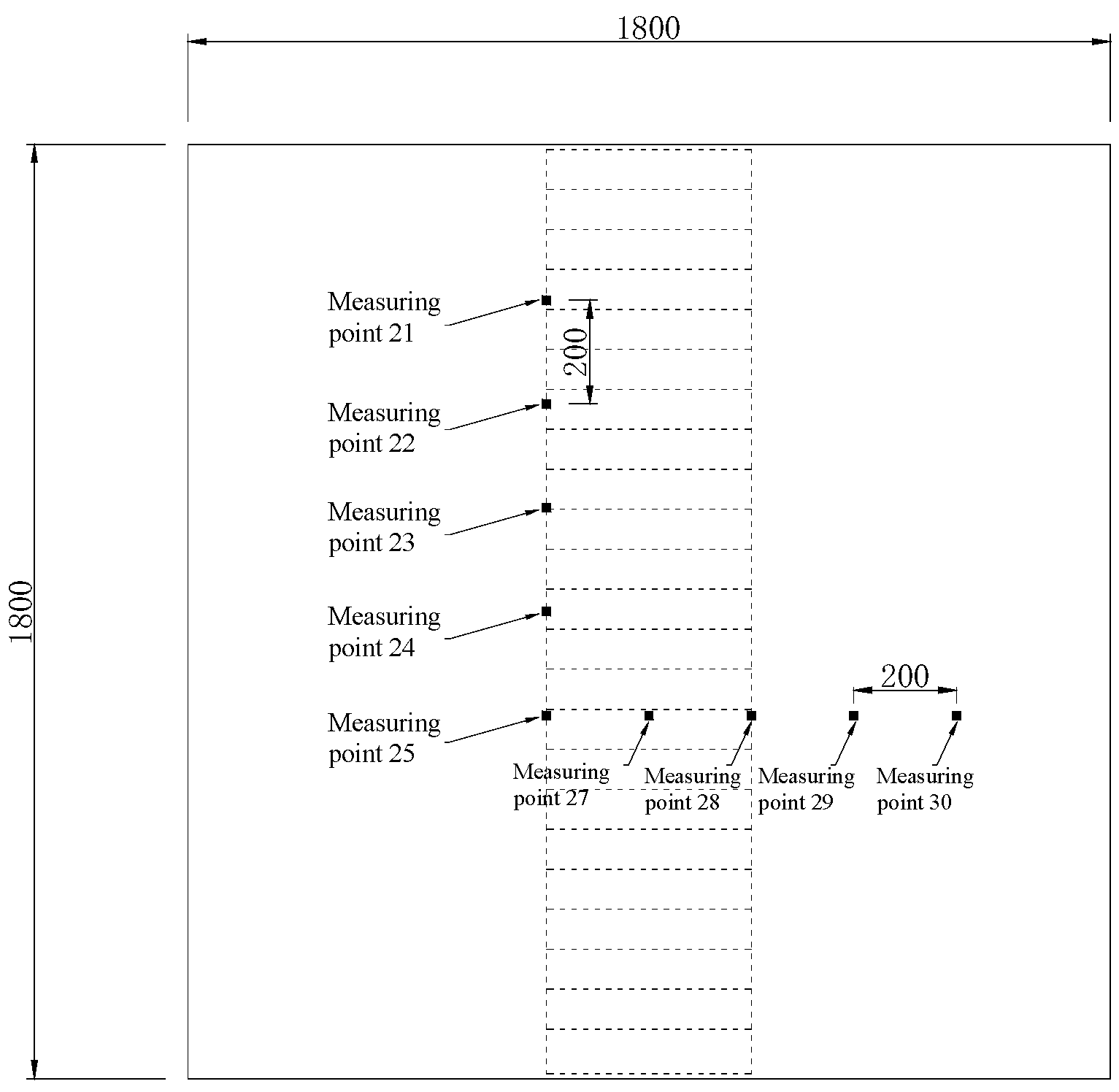








| Physical Quantity | Similarity Relation | Similarity Constant | Physical Quantity | Similarity Relation | Similarity Constant |
|---|---|---|---|---|---|
| Geometric dimensions | Basic quantity | 15.5 | Pressure | 16.75 | |
| Elastic modulus | Basic quantity | 16.75 | Axial force | 4024 | |
| Strain | 1 | Bending stiffness | 57720 | ||
| Stress | 16.75 | Axial stiffness | 3724 | ||
| Displacement | 15.5 | Shear stiffness | 3724 |
| Outer Diameter of Segment (m) | Segment Inner Diameter (m) | Segment Thickness (m) | Ring Width (m) | Elastic Modulus of Segment (MPa) | SEGMENT Poisson’s Ratio | |
|---|---|---|---|---|---|---|
| Prototype | 6.200 | 5.504 | 0.348 | 1.200 | 34,500 | 0.2 |
| Model | 0.400 | 0.356 | 0.022 | 0.077 | 2060 | 0.3 |
| Bolt Length (m) | Diameter of Bolt (m) | Number of Bolts | Elastic Modulus of Bolt (MPa) | Poisson’s Ratio of Bolts | |
|---|---|---|---|---|---|
| Prototype | 0.400 | 0.030 | 17 | 200,000 | 0.30 |
| Model | 0.027 | 0.002 | 6 | 33,800 | 0.32 |
| Density (g) | Moisture Content (%) | Internal Friction Angle (°) | Cohesion (kPa) | Compressive Modulus (MPa) |
|---|---|---|---|---|
| 1.495 | 0.23 | 29 | 0 | 2.89 |
| Test Number | Ground Surcharge Position | Total Thickness of Soil Layer | Tunnel Buried Depth | Sand for Test |
|---|---|---|---|---|
| 1 | Eccentricity 0 m | 1.2 m | 0.6 m | Dry sand |
| 2 | Eccentricity 0.2 m | 1.2 m | 0.6 m | Dry sand |
| 3 | Eccentricity 0.4 m | 1.2 m | 0.6 m | Dry sand |
| 4 | Eccentricity 0.6 m | 1.2 m | 0.6 m | Dry sand |
| 5 | Eccentricity 0 m | 1.1 m | 0.5 m | Dry sand |
| 6 | Eccentricity 0 m | 1.2 m | 0.6 m | Dry sand |
| 7 | Eccentricity 0 m | 1.3 m | 0.7 m | Dry sand |
| 8 | Eccentricity 0 m | 1.4 m | 0.8 m | Dry sand |
| 9 | Eccentricity 0 m | 1.2 m | 0.6 m | Wet sand |
| Test Number | Ground Surcharge Position | Tunnel Buried Depth | Type of Soil |
|---|---|---|---|
| 1 | Eccentricity 0 m | 9.3 m | Sand |
| 2 | Eccentricity 3.1 m | 9.3 m | Sand |
| 3 | Eccentricity 6.2 m | 9.3 m | Sand |
| 4 | Eccentricity 9.3 m | 9.3 m | Sand |
| 5 | Eccentricity 0 m | 7.75 m | Sand |
| 6 | Eccentricity 0 m | 9.3 m | Sand |
| 7 | Eccentricity 0 m | 10.85 m | Sand |
| 8 | Eccentricity 0 m | 12.4 m | Sand |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, G.; Zhang, S.; Xiang, P. Model Test Study on the Influence of Ground Surcharges on the Deformation of Shield Tunnels. Symmetry 2021, 13, 1565. https://doi.org/10.3390/sym13091565
Wei G, Zhang S, Xiang P. Model Test Study on the Influence of Ground Surcharges on the Deformation of Shield Tunnels. Symmetry. 2021; 13(9):1565. https://doi.org/10.3390/sym13091565
Chicago/Turabian StyleWei, Gang, Shuming Zhang, and Pengfei Xiang. 2021. "Model Test Study on the Influence of Ground Surcharges on the Deformation of Shield Tunnels" Symmetry 13, no. 9: 1565. https://doi.org/10.3390/sym13091565
APA StyleWei, G., Zhang, S., & Xiang, P. (2021). Model Test Study on the Influence of Ground Surcharges on the Deformation of Shield Tunnels. Symmetry, 13(9), 1565. https://doi.org/10.3390/sym13091565







