A Hopf Algebra on Permutations Arising from Super-Shuffle Product
Abstract
1. Introduction
2. Preliminaries
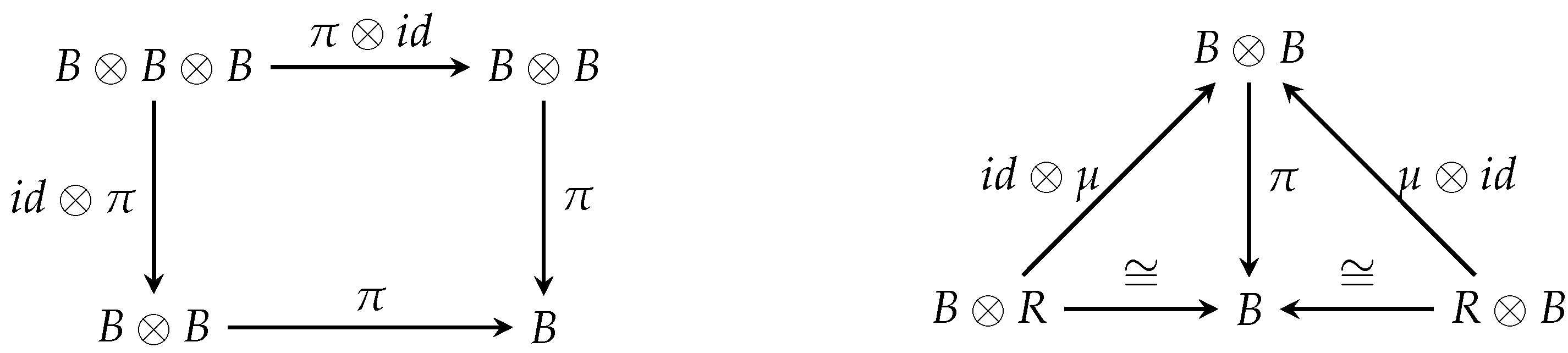
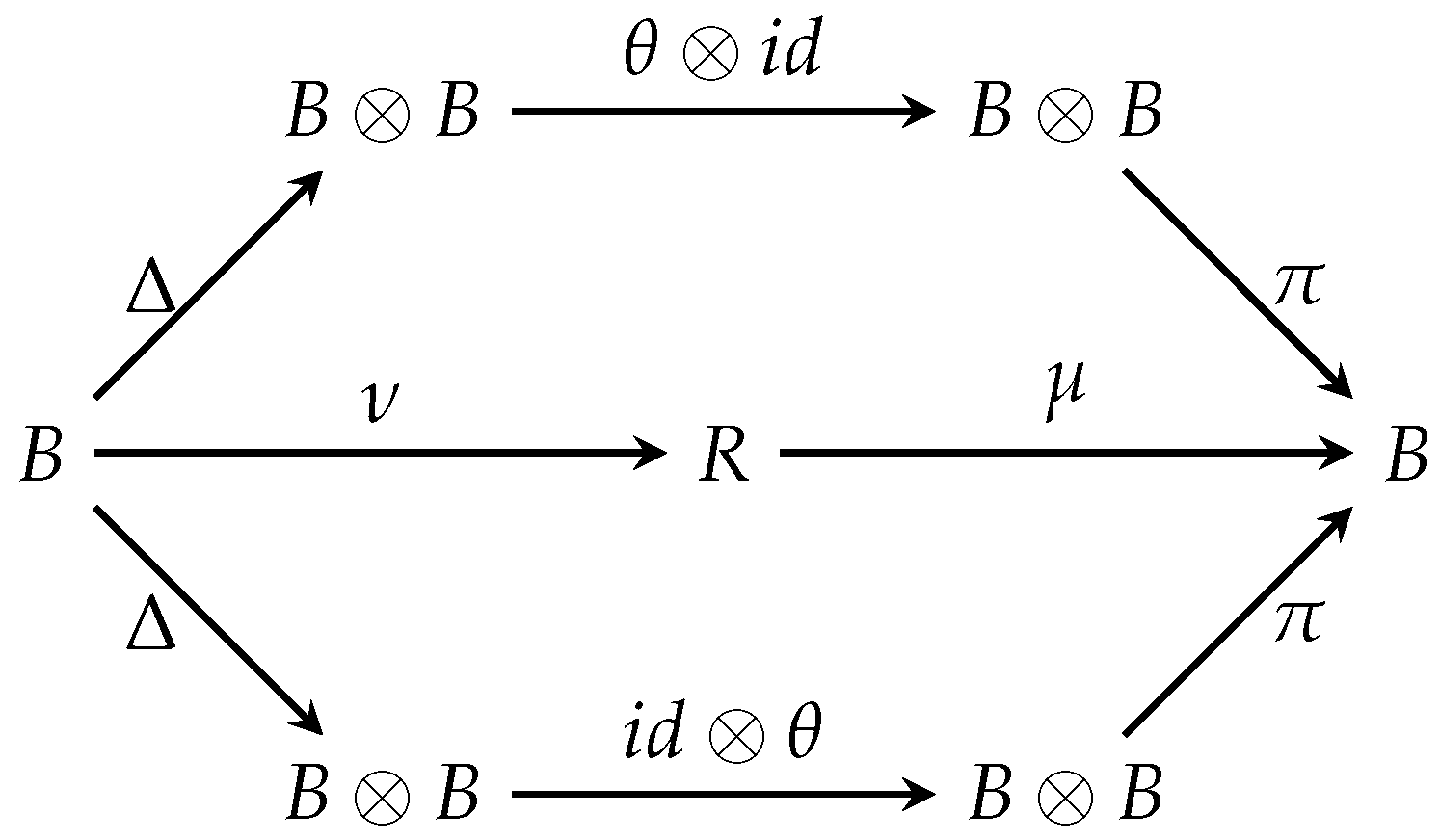
2.1. Basic Definitions
2.2. Basic Notations
3. Super-Shuffle Product and Cut-Box Coproduct
4. The Hopf Algebra Arising from the Super-Shuffle
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hopf, H. Verallgemeinerungen. In Selecta Heinz Hopf; Springer: Berlin/Heidelberg, Germany, 1941; pp. 119–151. [Google Scholar]
- Milnor, J. W.; Moore, J.C. On the structure of Hopf algebras. Ann. Math. 1965, 81, 211–264. [Google Scholar] [CrossRef]
- Sweedler, M. E. Hopf algebras. In Mathematics Lecture Note Series; W. A. Benjamin, Inc.: New York, NY, USA, 1969; pp. 49–91. [Google Scholar]
- Foissy, L. Free and cofree Hopf algebras. J. Pure Appl. Algebra 2012, 216, 480–494. [Google Scholar] [CrossRef]
- Bergeron, N.; Zabrockiv, M. The Hopf algebras of symmetric functions and quasi-symmetric functions in noncommutative variables are free and cofree. J. Algebra Appl. 2009, 8, 581–600. [Google Scholar] [CrossRef]
- Agular, M.; Sottile, F. Cocommutative Hopf Algebras of permutations and trees. J. Algebraic Comb. 2005, 22, 451–470. [Google Scholar]
- Bergeron, N.; Hohlweg, C. Grothendieck bialgebras, partition lattices, and symmetric functions in noncommutative variables. Electron. J. Comb. 2006, 13. Available online: http://eudml.org/doc/126516 (accessed on 14 November 2020). [CrossRef]
- Agular, M.; Sottile, F. Structure of the Malvenuto-Reutenauer Hopf Algebra of permutations. Adv. Math. 2005, 191, 225–275. [Google Scholar]
- Lando, S. K. On a Hopf algebra in graph theory. J. Comb. Theory Ser. B 2000, 80, 104–121. [Google Scholar] [CrossRef]
- Loday, J. L.; Ronco, M. O. Hopf Algebra of the planar binary trees. Adv. Math. 1998, 139, 293–309. [Google Scholar] [CrossRef]
- Malvenuto, C. Produits et coproduits des fonctions quasi-symétriques et de l’algebre des descents. Laboratoire de Combinatoire et D’informatique Mathématique (LACIM), Univ. du Québeca Montréal, Montréal. 1994, Volume 16. Available online: https://core.ac.uk/display/80315097 (accessed on 14 November 2020).
- Li, H.; Morse, J.; Shields, P. Structure constants for K-theory of Grassmannians. J. Comb. Theory Ser. A 2016, 144, 306–325. [Google Scholar] [CrossRef]
- Van Den Hijligenberg, N.; Martini, R. Differential Hopf algebra structures on the universal enveloping algebra of a Lie algebra. J. Math. Phys. 1996, 37, 524–532. [Google Scholar] [CrossRef]
- Reutenauer, C. Free Lie algebras. Handb. Algebra 2003, 3, 887–903. [Google Scholar]
- Cheng, J.; Zeng, Z. T. Irreducible Wakimoto-like Modules for the Lie Superalgebra D(2,1;α). Acta. Math. Sin. Engl. Ser. 2018, 34, 1578–1588. [Google Scholar] [CrossRef]
- Cheng, J.; Gao, Y. Generalized P(N)-Graded Lie Superalgebras. Front. Math. China 2021. Available online: https://doi.org/10.1007/s11464-021-0888-7 (accessed on 14 November 2020).
- Cheng, T.; Huang, H. L.; Yang, Y. Generalized clifford algebras as algebras in suitable symmetric linear Gr-categories. SIGMA Symmetry Integr. Geom. Methods Appl. 2016, 12, 004. [Google Scholar] [CrossRef]
- Cheng, T.; Li, H.; Yang, Y. A New View of Generalized Clifford Algebras. Adv. Appl. Clifford Algebras 2019, 29, 42. [Google Scholar] [CrossRef]
- Cheng, T.; Huang, H. L.; Yang, Y.; Zhang, Y. The octonions form an Azumaya algebra in certain braided linear Gr-categories. Adv. Appl. Clifford Algebras 2017, 27, 1055–1064. [Google Scholar] [CrossRef]
- Cheng, J.; Wang, Y.; Zhang, R. Degenerate quantum general linear groups. Adv. Thero. Math. Phys. 2020, 24, 1371–1418. [Google Scholar]
- Umirbaev, U. U. On an extension of automorphisms of polynomial rings. Sibirsk. Mat. 1995, 36, 787–791. [Google Scholar] [CrossRef]
- Belov, A.; Bokut, L.; Rowen, L.; Yu, J. T. The Jacobian Conjecture, Together with Specht and Burnside-Type Problems. In Automorphisms in Birational and Affine Geometry; Cheltsov, I., Ciliberto, C., Flenner, H., McKernan, J., Prokhorov, Y., Zaidenberg, M., Eds.; Springer Proceedings in Mathematics Statistics; Springer: Cham, Switzerland, 2014; Volume 79, pp. 249–285. [Google Scholar]
- Duffaut Espinosa, L. A.; Ebrahimi-Fard, K.; Gray, W. S. A combinatorial Hopf Algebra for nonlinear output feedback control systems. J. Algebra 2016, 453, 609–643. [Google Scholar] [CrossRef]
- Malvenuto, C.; Reutenauer, C. Duality between quasi-symmetrical functions and the solomon descent algebra. J. Algebra 1995, 177, 967–982. [Google Scholar] [CrossRef]
- Hoffman, M. S. Quasi-shuffle products. J. Algebr. Comb. 1999, 11, 49–68. [Google Scholar] [CrossRef]
- Zhao, M.; Li, H. A Hopf algebra structure on permutations. J. Shandong Norm. Univ. Nat. Sci. 2020, 4, 395–401. [Google Scholar]
- Manchon, D. Hopf Algebras in Renormalisation. Handb. Algebra 2008, 5, 365–427. [Google Scholar]
- Zhao, M.; Li, H. A pair of dual Hopf algebras on permutations. AIMS Math. 2021, 6, 5106–5123. [Google Scholar] [CrossRef]
- Li, H.; MacHenry, T.; Conci, A. Rational convolution roots of isobaric polynomials. Rocky Mt. J. Math. 2017, 4, 1259–1275. [Google Scholar] [CrossRef]
- Cao, L.; Chen, Z.; Duan, X.; Koyuncu, S.; Li, H. Diagonal Sums of Doubly Substochastic Matrices. Electronic J. Linear Algebra 2017, 35, 42–52. [Google Scholar] [CrossRef]
- Cao, L.; Chen, Z.; Duan, X.; Li, H. Permanents of doubly substochastic matrices. Linear Multilinear Algebra 2018, 68, 594–605. [Google Scholar] [CrossRef]
- Cao, L.; Chen, Z. Partitions of the polytope of doubly substochastic matrices. Linear Algebra App. 2019, 563, 98–122. [Google Scholar] [CrossRef]
- Cao, L. A minimal completion of (0,1)-matrices without total support. Linear Multilinear Algebra 2018, 67, 1522–1530. [Google Scholar] [CrossRef]
- Vargas, Y. Hopf algebra of permutation pattern functions. In Proceedings of the 26th International Conference on Formal Power Series and Algebraic Combinatorics (FPSAC 2014), Chicago, IL, USA, 29 June–3 July 2014; pp. 839–850. [Google Scholar]
- Bergeron, N.; Ceballos, C.; Pilaud, V. Hopf Dreams and Diagonal Harmonics. 2018. Available online: https://arxiv.org/abs/1807.03044 (accessed on 14 November 2020).
- Aval, J. C.; Bergeron, N.; Machacek, J. New Invariants for Permutations, Orders and Graphs. Adv. Appl. Math. 2020, 12, 102080. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, M.; Li, H. A Hopf Algebra on Permutations Arising from Super-Shuffle Product. Symmetry 2021, 13, 1010. https://doi.org/10.3390/sym13061010
Liu M, Li H. A Hopf Algebra on Permutations Arising from Super-Shuffle Product. Symmetry. 2021; 13(6):1010. https://doi.org/10.3390/sym13061010
Chicago/Turabian StyleLiu, Mengyu, and Huilan Li. 2021. "A Hopf Algebra on Permutations Arising from Super-Shuffle Product" Symmetry 13, no. 6: 1010. https://doi.org/10.3390/sym13061010
APA StyleLiu, M., & Li, H. (2021). A Hopf Algebra on Permutations Arising from Super-Shuffle Product. Symmetry, 13(6), 1010. https://doi.org/10.3390/sym13061010





