Invariant Solutions of Black–Scholes Equation with Ornstein–Uhlenbeck Process

Abstract

This paper analyses the model of Black–Scholes option pricing from the point of view of the group theoretic approach. The study identified new independent variables that lead to the transformation of the Black–Scholes equation. Furthermore, corresponding determining equations were constructed and new symmetries were found. As a result, the findings of the study demonstrate of the integrability of the model to present an invariant solution for the Ornstein–Uhlenbeck stochastic process.

1. Introduction

In 1973, Fischer Black and Myron Scholes formulated a mathematical model that describes option pricing. Their model forms the cornerstone for modern financial theory; it is not often that one can talk about modern finance without ever mentioning the revolutionary Black–Scholes (BS) model. The central goal of the BS model is to find fair prices of options by combining the price variation of the stock, the time value of money, the option’s stock price, and the time to the option’s expiry [1]. In 1976, Robert Merton provided several extensions to the BS model, the most prominent of these extensions being the ability to account for dividend yields [2]. The importance of the BS model was recognised in 1997 by Royal Swedish Academy of Sciences, where Scholes and Merton were awarded the Nobel Prize in Economics. Unfortunately, having died two years earlier, Fischer Black was not present for the award.

In this paper, we demonstrate how the theory of Lie symmetry analysis can be used to find invariant solutions of the Black–Scholes option pricing model under stochastic volatility. The stochastic volatility case was handled using the method introduced by Palianthanasis et al. [3], where the volatility was assumed to follow the Ornstein–Uhlenbeck process. The model is governed by the given partial differential equation [3]:

$$\begin{array}{c}\hfill V_t+\frac{1}{2}{f}^2\left(y\right)S^2{V}_{SS}+\rho \beta Sf\left(y\right)V_{Sy}+\frac{1}{2}{\beta }^2{V}_{yy}\\ \hfill +rS{V}_S+[\alpha (m-y)-\beta \rho \frac{\mu -r}{f\left(y\right)}]V_y-rV=0,\end{array}$$

(1)

where S is the price of an underlying asset (the stock); $\rho$ is the correlation coefficient; $\beta$ is the drift; r is the riskless rate; $\alpha$ is the rate of mean reversion; m is the long-run mean of Y; $\sigma$ is the volatility of the stock. To derive the model for stochastic volatility with the Ornstein–Uhlenbeck process, it is convenient to regard the volatility of the stock price as being a function of $Y_t$, i.e., $\sigma =f\left(Y_t\right)$, where $Y_t$ is the stochastic process that contains the Ornstein–Uhlenbeck term [3].

This paper is organised as follows. In Section 3, a heuristic background of the concepts underlying Lie symmetry analysis are introduced. In Section 4, Lie symmetry analysis is presented for the case where the volatility follows a stochastic process. The obtained symmetries were used to reduce Equation (1) into a one dimensional linear ordinary differential equation of order two. In Section 5, the modified local one-parameter transformations are presented and used to calculate invariant solutions of Equation (1). Furthermore, Lie symmetry analysis was used to examine a stochastic Heston model. The numerical solution that represents the evolution of the solutions is presented graphically in Section 6. The conclusion is provided in Section 7.

2. Fundamental Definitions and Theorems

In this section, a comprehensive review of a group theoretic approach to the solution of differential equations is given. The theory entails the tools necessary for subsequent employment throughout the paper. To start with, the mathematical idea of a symmetry is explained, and then the general properties of groups are explained; the properties are then extended to the Lie groups. Numerous textbooks are accessible [4,5,6,7,8], as well as research papers extensively published on the symmetry analysis of ordinary differential equations (ODEs) and partial differential equations (PDEs). In [5], Bluman and Kumei described the significance of the theory of Lie symmetry for a PDE. The authors pointed out that invariant functions can be constructed to reduce the order of a differential equation or the number of dependent variables [9].

According to Lie theory, the kth-order partial differential equation [10,11]:

$$\begin{array}{c}\hfill u_t-F(t,x,u,u_{\left(1\right)},u_{\left(2\right)},\dots ,u_{\left(k\right)})=0,\end{array}$$

(2)

admits the given Lie group of transformations of one-parameter:

$$\begin{array}{cc}\hfill \widehat{t}& \approx t+a{\xi }^0(t,x,u,u_{\left(1\right)},u_{\left(2\right)},\dots ,u_{\left(k\right)}),\\ \hfill {\widehat{x}}^i& \approx x^i+a{\xi }^i(t,x,u,u_{\left(1\right)},u_{\left(2\right)},\dots ,u_{\left(k\right)}),\\ \hfill {\widehat{u}}_i^{\alpha }& \approx u_i^{\alpha }+a{\eta }_i^{\alpha }(t,x,u,u_{\left(1\right)},u_{\left(2\right)},\dots ,u_{\left(k\right)}),\end{array}$$

with infinitesimal Lie generators [12,13]:

$$\begin{array}{c}\hfill X={\xi }^0\frac{\partial }{\partial t}+{\xi }^i\frac{\partial }{\partial x^i}+{\eta }^{\alpha }\frac{\partial }{\partial u^{\alpha }},\end{array}$$

(3)

if:

$$\begin{array}{c}\hfill {\widehat{u}}_t-F(\widehat{t},\widehat{x},\widehat{u},{\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},\dots ,{\widehat{u}}_{\left(k\right)})=0.\end{array}$$

(4)

the group transformations $\widehat{t}$, $\widehat{x}$, and $\widehat{u}$ are obtained by solving the following Lie equations [14]:

$$\begin{array}{ccc}\hfill \frac{d\widehat{t}}{da}& =& {\xi }^0(\widehat{t},\widehat{x},\widehat{u},{\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},\dots ,{\widehat{u}}_{\left(k\right)}),\hfill \\ \hfill \frac{d{\widehat{x}}^i}{da}& =& {\xi }^i(\widehat{t},\widehat{x},\widehat{u},{\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},\dots ,{\widehat{u}}_{\left(k\right)}),\hfill \\ \hfill \frac{d{\widehat{u}}_i^{\alpha }}{da}& =& {\eta }_i^{\alpha }(\widehat{t},\widehat{x},\widehat{u},{\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},\dots ,{\widehat{u}}_{\left(k\right)}),\hfill \end{array}$$

(5)

with the initial conditions:

$$\widehat{t}{\mid }_{a=0}=t,{\widehat{x}}^i{\mid }_{a=0}=x^i,{\widehat{u}}_i^{\alpha }{\mid }_{a=0}=u_i^{\alpha }.$$

the infinitesimal form of ${\widehat{u}}_{\overline{t}},{\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},...,{\widehat{u}}_{\left(k\right)}$ are found by the given formulas [15,16]:

$$\begin{array}{ccc}\hfill {\widehat{u}}_i^{\alpha }& \approx & u_i^{\alpha }+a{\eta }_i^{\alpha }(x,u,u_1),\hfill \\ \hfill {\widehat{u}}_{ij}^{\alpha }& \approx & u_{ij}^{\alpha }+a{\eta }_{ij}^{\alpha }(x,u,u_1,u_2),\hfill \\ & \dots \\ \hfill {\widehat{u}}_{i_1\dots i_k}^{\alpha }& \approx & u_{i_1\dots i_k}^{\alpha }+a{\eta }_{i_1\dots i_k}^{\alpha }(x,u,u_1,\dots ,u_k).\hfill \end{array}$$

(6)

the functions ${\eta }_i^{\alpha }(x,u,u_1)$, ${\eta }_{ij}^{\alpha }(x,u,u_1,u_2)$, and ${\eta }_{i_1\dots i_k}^{\alpha }(x,u,u_1,...,u_k)$ are obtained from the following prolongation formulas [6]:

$$\begin{array}{ccc}\hfill {\eta }_i^{\alpha }& =& D_i\left({\eta }^{\alpha }\right)-u_j^{\alpha }{D}_i\left({\xi }^j\right),\hfill \\ \hfill {\eta }_{ij}^{\alpha }& =& D_j\left({\eta }_i^{\alpha }\right)-u_{il}^{\alpha }{D}_j\left({\xi }^l\right),\hfill \\ & \dots \\ \hfill {\eta }_{i_1\dots i_k}^{\alpha }& =& D_{i_k}\left({\eta }_{i_1...i_{k-1}}^{\alpha }\right)-u_{i_1\dots i_{k-1}l}^{\alpha }{D}_{i_k}\left({\xi }^l\right),\hfill \end{array}$$

(7)

where $D_i$ denotes the operator of total differentiation with respect to $(x_1,x_2...x_n)$, then:

$$\begin{array}{c}\hfill D_i=\frac{\partial }{\partial x_i}+u_i^{\alpha }\frac{\partial }{\partial u^{\alpha }}+u_{ij}^{\alpha }\frac{\partial }{\partial u_j^{\alpha }}.\end{array}$$

(8)

the transformed derivatives ${\widehat{u}}_{\left(1\right)},{\widehat{u}}_{\left(2\right)},...,{\widehat{u}}_{\left(k\right)}$ can be computed from the formulas:

$$\begin{array}{c}\hfill D_i=D_i\left(f^i\right){\widehat{D}}_j.\end{array}$$

(9)

the generators are therefore given by:

$$\begin{array}{ccc}\hfill X^{\left[1\right]}& =& X+{\eta }_i^{\alpha }(x,u,u_1)\frac{\partial }{\partial u_i^{\alpha }},\hfill \\ & \dots \\ \hfill X^{\left[k\right]}& =& X^{\left[1\right]}+\dots +{\eta }_{i_1\dots i_k}^{\alpha }(x,u,\dots ,u_k)\frac{\partial }{\partial u_{i_1...i_k}^{\alpha }}.\hfill \end{array}$$

(10)

Theorem 1.

A function $F(x,u,\cdots ,u_k)$ is invariant under the prolonged group G, if and only if [17]

$$\begin{array}{c}\hfill X^{\left[k\right]}F=0,\end{array}$$

(11)

where $X^{\left[k\right]}$ is the generator of G.

Theorem 2.

Every one-parameter group of transformations $(\widehat{x}=f(x,y,\epsilon ),\widehat{y}=g(x,y,\epsilon ))$ is reduced to a group of translations $\widehat{t}=t+\epsilon$, $\widehat{u}=u$ with the generator [17]:

$$\begin{array}{c}\hfill X=\frac{\partial }{\partial t},\end{array}$$

by suitable change of variables

$$\begin{array}{c}\hfill t=t(x,y),u=u(x,y).\end{array}$$

Considering the Lie groups of point transformations related to a given differential equation $\mathcal{E}$ involve n independent variables $x=(x_1,x_2,...,x_n)\in {\mathbb{R}}^n$ and m dependent variables $u=(u^1,u^2,...,u^m)\in {\mathbb{R}}^m$ [4,18], let:

$$x^{*}=X(x,u;a),u^{*}=U(x,u;a)$$

(12)

be a group of transformations in the space $\in {\mathbb{R}}^{n+m}$ of the variables $(x,u)$ [4,18]. Furthermore, for:

$$u=\mathsf{\Theta }\left(x\right)\equiv ({\mathsf{\Theta }}^1\left(x\right),{\mathsf{\Theta }}^2\left(x\right),...,{\mathsf{\Theta }}^m\left(x\right)),$$

(13)

be a solution of the equation $\mathcal{E}$. A Lie group of transformations of the form (12) admitted by $\mathcal{E}$ has the following two corresponding properties [4,18]:

1

A transformation of the group maps any solution of $\mathcal{E}$ into another solution of $\mathcal{E}$;

2

A transformation of the group leaves $\mathcal{E}$ invariant, say, $\mathcal{E}$ reads the same in terms of the variables $(x,u)$ and in terms of the transformed variables $(x^{*},u^{*})$.

Definition 1.

[18]-The function $u=\mathsf{\Theta }\left(x\right)$ with components $u^A={\mathsf{\Theta }}^A\left(x\right)$$(A=1,2,\dots ,m)$ is said to be an invariant solution of (2) if $u^A={\mathsf{\Theta }}^A\left(x\right)$ is an invariant surface of (12) and is a solution of (2).

3. Symmetry Analysis for Black–Scholes with the Ornstein–Uhlenbeck Process

We assume that Equation (1) admits a Lie symmetry satisfying the generator:

$$\begin{array}{c}\hfill X^{\left[1\right]}={\xi }^1{\partial }_t+{\xi }^2{\partial }_S+{\xi }^3{\partial }_y+\eta {\partial }_V.\end{array}$$

(14)

the prolonged symbol is then:

$$\begin{array}{cc}\hfill X^{\left[2\right]}& =X^{\left[1\right]}+{\eta }^t{\partial }_{V_t}+{\eta }^S{\partial }_{V_S}+{\eta }^y{\partial }_{V_y}\\ & +{\eta }^{Sy}{\partial }_{V_{Sy}}+{\eta }^{SS}{\partial }_{V_{SS}}+{\eta }^{yy}{\partial }_{V_{yy}}.\end{array}$$

(15)

using Equation (7), we can obtain the following exact expressions for the prolonged coefficients:

$$\begin{array}{ccc}\hfill {\eta }^t& =& {\eta }_t+V_t{\eta }_V-{\xi }_t^1{V}_t-V_t{V}_t{\xi }_V^1-{\xi }_t^2{V}_S-V_t{V}_S{\xi }_V^2\hfill \\ & -& {\xi }_t^3{V}_y-V_t{V}_y{\xi }_V^3\hfill \\ \hfill {\eta }^S& =& {\eta }_S+V_S{\eta }_V-{\xi }_S^1{V}_t-V_t{V}_S{\xi }_V^1-{\xi }_S^2{V}_S\hfill \\ & -& V_S{V}_S{\xi }_V^2-{\xi }_S^3{V}_y-V_S{V}_y{\xi }_V^3\hfill \\ \hfill {\eta }^y& =& {\eta }_y+V_y{\eta }_V-{\xi }_y^1{V}_t-{\xi }_V^2{V}_y{V}_S-V_t{V}_y{\xi }_V^1-{\xi }_y^2{V}_S\hfill \\ & -& {\xi }_y^3{V}_{yy}-V_y{V}_y{\xi }_V^3\hfill \\ \hfill {\eta }_{SS}& =& {\eta }_{SS}+2{\eta }_{SV}{V}_S+{\eta }_V{V}_{SS}+{\eta }_{VV}{V}_S{V}_S-{\xi }_{SS}^2{V}_S-{\xi }_{SS}^3{V}_y\hfill \\ & -& {\xi }_{SS}^1{V}_t-2{\xi }_S^2{V}_{SS}-2{\xi }_S^3{V}_{Sy}-{\xi }_S^1{V}_{St}-2{\xi }_{SV}^2{V}_S{V}_S\hfill \\ & -& 2{\xi }_{SV}^3{V}_S{V}_y-2{\xi }_{SV}^1{V}_S{V}_t-{\xi }_V^2{V}_S{V}_{SS}-2{\xi }_V^2{V}_S{V}_{SS}\hfill \\ & -& {\xi }_V^3{V}_y{V}_{SS}-2{\xi }_V^3{V}_{Sy}-{\xi }_V^1{V}_t{V}_{SS}-2{\xi }_V^1{V}_S{V}_{St}\hfill \\ & -& {\xi }_{VV}^2{V}_S{V}_S{V}_S-{\xi }_{VV}^3{V}_S{V}_S{V}_y-{\xi }_{VV}^1{V}_S{V}_S{V}_t\hfill \\ \hfill {\eta }^{yy}& =& {\eta }_{yy}+2{\eta }_{yV}{V}_y+{\eta }_V{V}_{yy}+{\eta }_{VV}{V}_y{V}_y-{\xi }_{yy}^2{V}_S-{\xi }_{yy}^3{V}_y\hfill \\ & -& {\xi }_{yy}^1{V}_t-2{\xi }_y^2{V}_{yS}-2{\xi }_y^3{V}_{yy}-{\xi }_y^1{V}_{yt}-2{\xi }_{yV}^2{V}_y{V}_S\hfill \\ & -& 2{\xi }_{yV}^3{V}_y{V}_y-2{\xi }^{yV}{V}_y{V}_t-{\xi }_V^2{V}_S{V}_{yy}-2{\xi }_V^2{V}_y{V}_{yS}-{\xi }_V^3{V}_y{V}_{yy}\hfill \\ & -& {\xi }_{VV}^2{V}_y{V}_y{V}_S-{\xi }_{VV}^3{V}_y{V}_y{V}_y-{\xi }_{VV}^1{v}_y{V}_y{V}_t\hfill \\ \hfill {\eta }^{Sy}& =& {\eta }_{Sy}+{\eta }_{yV}{V}_S-{\xi }_{Sy}^2{V}_S+{\eta }_{SV}{V}_y-{\xi }_{Sy}^3{V}_y-{\xi }_{Sy}^1{V}_t\hfill \\ & +& {\eta }_{VV}{V}_S{V}_y-{\xi }_{yV}^3{V}_S{V}_y-{\xi }_{SV}^2{V}_S{V}_y-{\xi }_{yV}^1{V}_S{V}_t\hfill \\ & -& {\xi }_{yV}^1{V}_y{V}_t-{\xi }_{yV}^1{V}_S{V}_S-{\xi }_{SV}^3{V}_y{V}_y-{\xi }_y^2{V}_{SS}-{\xi }_S^3{V}_{yy}\hfill \\ & +& {\eta }_V{V}_{Sy}-{\xi }_y^3{V}_{Sy}-{\xi }_S^2{V}_{Sy}-{\xi }_y^1{V}_{St}-{\xi }_S^1{V}_{yt}-{\xi }_V^2{V}_S{V}_{Sy}\hfill \\ & -& {\xi }_V^1{V}_{SS}{V}_y-{\xi }_V^3{V}_S{V}_{yy}-{\xi }_{VV}^3{V}_S{V}_y{V}_y.\hfill \end{array}$$

the symbols ${\eta }^t$, ${\eta }^S$, ${\eta }^y$, ${\eta }^{Sy}$, ${\eta }^{SS}$, and ${\eta }^{yy}$ are substituted into the determining equation. Using the SYM Mathematica package conceived by Dimas and Tsoubelis in [19], we obtained the following two Lie operators, which are inline in [20]:

$$\begin{array}{ccc}\hfill X_1& =& {\partial }_t,\hfill \end{array}$$

(16)

$$\begin{array}{ccc}\hfill X_2& =& S{\partial }_S.\hfill \end{array}$$

(17)

in as much as Equation (1) is a linear equation, it therefore constantly admits the respective linear and infinite symmetries given by:

$$\begin{array}{ccc}\hfill X_V& =& V{\partial }_V,\hfill \end{array}$$

(18)

$$\begin{array}{ccc}\hfill X_b& =& b(t,S,y){\partial }_V,\hfill \end{array}$$

(19)

in constructing invariant solutions, the following three facts must be taken into consideration: First, the infinite symmetry cannot be used for the reduction of the differential equation; therefore, they are not considered here. Second, all those solutions in which V does not depend on one of the independent variables are discarded. Third, linear combinations of symmetries are also symmetries. These three facts mean that a reduction of Equation (1) can be performed using the following symmetry generators, which are linear combinations of the two symmetries obtained:

$$\begin{array}{ccc}\hfill Y_1& =& X_1+k_1{X}_u,\hfill \end{array}$$

(20)

$$\begin{array}{ccc}\hfill Y_2& =& X_2+k_2{X}_u,\hfill \end{array}$$

(21)

$$\begin{array}{ccc}\hfill Y_{12}& =& X_1+c{X}_2+k_3{X}_u.\hfill \end{array}$$

(22)

4. Invariant Solution through Lie Operators

4.1. Invariant Solution through Symmetry $Y_1$

The symmetry $Y_1$ is given by:

$$\begin{array}{c}\hfill Y_1={\partial }_t+k_{1}V{\partial }_V.\end{array}$$

(23)

the characteristic system is therefore given by:

$$\begin{array}{c}\hfill \frac{k_{1}dt}{1}=\frac{dV}{V}\end{array}$$

(24)

integrating both sides, we can obtain:

$$\begin{array}{c}\hfill k_{1}t=lnV+ln\nu (S,y),\end{array}$$

(25)

which results in:

$$\begin{array}{c}\hfill V(t,S,y)=\nu (S,y)e^{k_{1}t}.\end{array}$$

(26)

the computation of the partial derivatives of V gives:

$$\begin{array}{ccc}\hfill V_t& =& k_1\nu (S,y)e^{k_{1}t},\hfill \\ \hfill V_y& =& {\nu }_y{e}^{k_{1}t},\hfill \\ \hfill V_S& =& {\nu }_S{e}^{k_{1}t},\hfill \\ \hfill V_{SS}& =& {\nu }_{SS}{e}^{k_{1}t},\hfill \\ \hfill V_{Sy}& =& {\nu }_{Sy}{e}^{k_{1}t},\hfill \\ \hfill V_{yy}& =& {\nu }_{yy}{e}^{k_{1}t}.\hfill \end{array}$$

(27)

the substitution of Equation (27) into Equation (1) gives the following one-dimensional second-order partial differential equation of function $\nu (S,y)$:

$$\begin{array}{cc}\hfill 0=& \frac{1}{2}{f}^2\left(y\right)S^2{\nu }_{SS}+\rho \beta Sf\left(y\right){\nu }_{Sy}+\frac{1}{2}{\beta }^2{\nu }_{yy}+\end{array}$$

(28)

$$\begin{array}{cc}& rS{\nu }_S+\left[\alpha (m-y)-\beta \rho \frac{\mu -r}{f\left(y\right)}\right]{\nu }_y-(r-k_1)\nu .\end{array}$$

(29)

using the SYM [19], it can be found that Equation (28) admits one symmetry $X_2=S{\partial }_S$. Applying the symmetry $S{\partial }_S+k_{2}VdV$, which is equivalent to the symmetry vector $Y_2$, to Equation (28), a second-order differential equation can be obtained. This is shown below in detail.

4.2. Invariant Solution through Symmetry $X_2$

The symmetry $Y_2$ is given by:

$$\begin{array}{c}\hfill Y_2=S{\partial }_S+k_2\nu {\partial }_{\nu },\end{array}$$

(30)

which gives the following characteristic system:

$$\begin{array}{c}\hfill k_2\frac{dS}{S}=\frac{d\nu }{\nu }.\end{array}$$

(31)

the integration of Equation (31) gives:

$$\begin{array}{cc}\hfill k_{2}lnS+lnw\left(y\right)& =ln\nu ,\end{array}$$

(32)

i.e.:

$$\begin{array}{cc}\hfill ln{S}^{k_2}+lnw\left(y\right)& =ln\nu .\end{array}$$

(33)

hence

$$\begin{array}{c}\hfill \nu (S,y)=S^{k_2}w\left(y\right).\end{array}$$

(34)

substituting Equation (34) into Equation (26), we can obtain:

$$\begin{array}{c}\hfill V(t,S,y)=e^{k_{1}t}{S}^{k_2}w\left(y\right).\end{array}$$

(35)

one can use Equation (34) to reduce Equation (28) by computing partial derivatives of $\nu$ with respect to S and y as follows:

$$\begin{array}{ccc}\hfill {\nu }_y& =& S^{k_2}{w}_y,\hfill \\ \hfill {\nu }_S& =& k_2{S}^{k_2-1}w,\hfill \\ \hfill {\nu }_{Sy}& =& k_2{S}^{k_2-1}{w}_y,\hfill \\ \hfill {\nu }_{SS}& =& k_2(k_2-1)S^{k_2-2}w,\hfill \\ \hfill {\nu }_{yy}& =& S^{k_2}{w}_{yy}.\hfill \end{array}$$

(36)

substituting these partial derivatives into Equation (28) gives:

$$\begin{array}{ccc}\hfill 0& =& {\beta }^2{w}_{yy}+\left[2\alpha (m-y)+\frac{2\rho \beta }{f\left(y\right)}(k_2{f}^2\left(y\right)-\mu +r)\right]w_y\hfill \\ & +& [(k_2^2-k_2)f^2\left(y\right)+2(r{k}_2-r+k_1)]w.\hfill \end{array}$$

(37)

4.3. Invariant Solution through Symmetry $Y_{12}$

The symmetry $Y_{12}$ is given by:

$$\begin{array}{c}\hfill Y_{12}={\partial }_t+cS{\partial }_S+k_{3}V{\partial }_V.\end{array}$$

(38)

the characteristic is given by:

$$\begin{array}{c}\hfill \frac{dt}{1}=\frac{dS}{cS}=\frac{dV}{k_{3}V}.\end{array}$$

(39)

the first characteristic is solved by integrating:

$$\begin{array}{c}\hfill \frac{dt}{1}=\frac{dS}{cS},\end{array}$$

(40)

to get:

$$\begin{array}{c}\hfill tc=lnS+lnz.\end{array}$$

(41)

hence:

$$\begin{array}{c}\hfill S=z{e}^{ct},\end{array}$$

(42)

and so:

$$\begin{array}{c}\hfill z=S{e}^{-ct}.\end{array}$$

(43)

the second characteristic is solved by integrating:

$$\begin{array}{c}\hfill \frac{dt}{1}=\frac{dV}{k_{3}V},\end{array}$$

(44)

to get:

$$\begin{array}{c}\hfill k_{3}t=lnV+lnv(z,y),\end{array}$$

(45)

which then gives:

$$\begin{array}{c}\hfill V(t,S,y)=e^{k_{3}t}v(z,y).\end{array}$$

(46)

now, derivatives are obtained as follows:

$$\begin{array}{ccc}\hfill V_t& =& k_3{e}^{k_{3}t}v-cS{e}^{k_{3}t}{e}^{-ct},\hfill \\ \hfill V_S& =& e^{k_{3}t}{e}^{-ct}{v}_z,\hfill \\ \hfill V_y& =& e^{k_{3}t}{v}_y,\hfill \\ \hfill V_{yy}& =& e^{k_{3}t}{v}_{yy},\hfill \\ \hfill V_{SS}& =& e^{k_{3}t}{e}^{(-2ct)}{v}_{zz},\hfill \\ \hfill V_{Sy}& =& e^{k_{3}t}{e}^{-ct}{v}_{zy}.\hfill \end{array}$$

(47)

substituting these derivatives into Equation (1), we can find:

$$\begin{array}{ccc}\hfill 0& =& \frac{1}{2}{f}^2\left(y\right)S^2{e}^{k_{3}t}{e}^{-2ct}{v}_{zz}+\rho \beta Sf\left(y\right)e^{k_{3}t}{e}^{-ct}{v}_{zy}+\hfill \\ & +& \frac{1}{2}{\beta }^2{e}^{k_{3}t}{v}_{yy}+rS{e}^{k_{3}t}{e}^{-ct}{v}_z+\left[\alpha (m-y)-\rho \beta \frac{\mu -r}{f\left(y\right)}\right]e^{k_{3}t}{v}_y\hfill \\ & & r{e}^{k_{3}t}v+e^{k_{3}t}v-cS{e}^{-ct}{v}_z.\hfill \end{array}$$

(48)

multiplying throughout by 2, cancelling out the term $e^{k_{3}t}$, and imposing the equation $z=S{e}^{-ct}$ leads to:

$$\begin{array}{ccc}\hfill 0& =& f^2{z}^2\left(y\right)v_{zz}+2\rho \beta zf\left(y\right)v_{zy}+{\beta }^2{v}_{yy}+2(r-c)z{v}_z+\hfill \\ & & 2\left[\alpha (m-y)-\rho \beta \frac{\mu -r}{f\left(y\right)}\right]v_y-2(r-k_3)v.\hfill \end{array}$$

(49)

using the SYM, it can be found that Equation (49) admits the symmetry $z\partial z$, which is a reduced symmetry [3]. Hence, one can apply the zeroth-order invariant of the symmetry vector $z{\partial }_z+w{\partial }_w$ to Equation (49) to get a reduced form, as follows: Taking the characteristic of $z{\partial }_z+k_{4}v{\partial }_v$, we get:

$$\begin{array}{c}\hfill \frac{dz}{z}=\frac{dv}{k_{4}v}.\end{array}$$

(50)

integrating gives:

$$\begin{array}{c}\hfill k_{4}lnz+lnu\left(y\right)=lnv,\end{array}$$

(51)

and hence:

$$\begin{array}{c}\hfill v(z,y)=z^{k_4}u\left(y\right).\end{array}$$

(52)

the substitution of Equation (52) into (46) gives

$$\begin{array}{c}\hfill V(t,S,y)=e^{k_{3}t}{z}^{k_4}u\left(y\right).\end{array}$$

(53)

now, from Equation (52), we can compute the derivatives of v with respect to z and y as follows:

$$\begin{array}{ccc}\hfill v_z& =& k_4{z}^{k_4-1}u,\hfill \\ \hfill v_{zz}& =& k_4(k_4-1)z^{(k_4-2)}u,\hfill \\ \hfill v_{zy}& =& k_4{z}^{k_4-1}{u}_y,\hfill \\ \hfill v_y& =& z^{k_4}{u}_y,\hfill \\ \hfill v_{yy}& =& z^{k_4}{u}_{yy}.\hfill \end{array}$$

(54)

substituting these into Equation (49) gives the following second-order differential equation:

$$\begin{array}{ccc}\hfill 0& =& {\beta }^2{u}_{yy}+\left[2\alpha (m-y)+\frac{2\rho \beta }{f\left(y\right)}(k_2{f}^2\left(y\right)-\mu +r)\right]u_y\hfill \\ & +& [(k_2^2-k_2)f^2\left(y\right)+2(r{k}_2-r+k_1)]u.\hfill \end{array}$$

(55)

the solution of differential Equation (55) can be obtained using Maple software. This results in a very complicated solution that contains hypergeometric functions. However, this solution can then be substituted into Equation (53) to get the complete solution $V(t,S,y)$ for the original partial differential equation.

5. Symmetry Analysis of the Heston Model

This section is dedicated to performing Lie symmetry analysis for the Heston model. The Black–Scholes equation for the Heston model is given by [3]:

$$\begin{array}{ccc}\hfill 0& =& \frac{1}{2}Y{S}^2{V}_{SS}+\rho \delta YS{V}_{SY}+\frac{1}{2}{\delta }^{2}Y{V}_{YY}+rS{V}_S\hfill \\ & +& (\alpha (m-Y)-\lambda Y)V_Y-rV+V_t.\hfill \end{array}$$

(56)

in order to ease the process of calculating symmetries for Equation (56), the following change of variables was employed:

$$\begin{array}{ccc}\hfill Y& =& y^2\hfill \\ \hfill \beta & =& \frac{\delta }{2}\hfill \\ \hfill c_1& =& (\alpha -\lambda )\hfill \\ \hfill c_2& =& \alpha m-{\beta }^2\hfill \end{array}$$

(57)

which provided the new differential equation below:

$$\begin{array}{ccc}\hfill 0& =& \frac{1}{2}{y}^2{S}^2{V}_{SS}+\beta \rho yS{V}_{Sy}+\frac{1}{2}{\beta }^2{V}_{yy}+rS{V}_S\hfill \\ & +& \frac{1}{2}\left[c_{1}y+\left(\frac{c_2}{y}\right)\right]V_y-rV+V_t.\hfill \end{array}$$

(58)

using the SYM [19], we obtained the given Lie operators:

$$\begin{array}{ccc}\hfill X_1& =& {\partial }_t,\hfill \\ \hfill X_2& =& S{\partial }_S,\hfill \\ \hfill X_V& =& V{\partial }_V,\hfill \\ \hfill X_b& =& b(t,S,y){\partial }_V,\hfill \end{array}$$

(59)

where $X_V$ and $X_b$ are linear symmetry and infinite symmetry, respectively. Next, the linear combinations of these symmetries were considered to obtain:

$$\begin{array}{ccc}\hfill Y_1& =& X_1+k_1{X}_v,\hfill \end{array}$$

(60)

$$\begin{array}{ccc}\hfill Y_2& =& X_2+k_2{X}_V,\hfill \end{array}$$

(61)

$$\begin{array}{ccc}\hfill Y_{12}& =& X_1+c{X}_2+k_3{X}_V.\hfill \end{array}$$

(62)

5.1. Invariant Solution through Symmetry $Y_1$

The symmetry $Y_1$ is given by:

$$\begin{array}{c}\hfill Y_1={\partial }_t+k_{1}V{\partial }_V.\end{array}$$

(63)

the characteristic system is therefore given by:

$$\begin{array}{c}\hfill \frac{k_{1}dt}{1}=\frac{dV}{V}.\end{array}$$

(64)

integrating both sides, we can obtain:

$$\begin{array}{c}\hfill k_{1}t=lnV+ln\mathsf{\Phi }(S,y),\end{array}$$

(65)

which results in:

$$\begin{array}{c}\hfill V(t,S,y)=\mathsf{\Phi }(S,y)e^{k_{1}t}.\end{array}$$

(66)

the computation of partial derivatives of V with respect to its parameters gives:

$$\begin{array}{ccc}\hfill V_t& =& k_1\mathsf{\Phi }(S,y)e^{k_{1}t},\hfill \\ \hfill V_y& =& {\mathsf{\Phi }}_y{e}^{k_{1}t},\hfill \\ \hfill V_S& =& {\mathsf{\Phi }}_S{e}^{k_{1}t},\hfill \\ \hfill V_{SS}& =& {\mathsf{\Phi }}_{SS}{e}^{k_{1}t},\hfill \\ \hfill V_{Sy}& =& {\mathsf{\Phi }}_{Sy}{e}^{k_{1}t},\hfill \\ \hfill V_{yy}& =& {\mathsf{\Phi }}_{yy}{e}^{k_{1}t}.\hfill \end{array}$$

substituting these in Equation (58), the function $\mathsf{\Phi }(S,y)$ satisfies the following equation:

$$\begin{array}{ccc}\hfill 0& =& \frac{1}{2}{y}^2{S}^2{\mathsf{\Phi }}_{SS}+\beta \rho yS{\mathsf{\Phi }}_{Sy}+\frac{1}{2}{\beta }^2{\mathsf{\Phi }}_{yy}\hfill \\ & +& \frac{1}{2}\left[c_{1}y+\left(\frac{c_2}{y}\right)\right]{\mathsf{\Phi }}_y-r\mathsf{\Phi }+k_1\mathsf{\Phi }.\hfill \end{array}$$

(67)

using the SYM, it can be found that Equation (67) admits one symmetry $X_2=S{\partial }_S$. This means that if the symmetry $S{\partial }_S+k_{2}VdV$, which is equivalent to the symmetry vector $Y_2$ applied to Equation (46) being able to reduce the equation into a second-order ordinary differential equation. This is shown below in detail.

5.2. Invariant Solution through Symmetry $X_2$

The symmetry $Y_2$ is given by:

$$\begin{array}{c}\hfill Y_2=S{\partial }_S+k_2\mathsf{\Phi }{\partial }_{\mathsf{\Phi }},\end{array}$$

which gives the following characteristic system:

$$\begin{array}{c}\hfill k_2\frac{dS}{S}=\frac{d\mathsf{\Phi }}{\mathsf{\Phi }}.\end{array}$$

integrating both sides gives:

$$\begin{array}{cc}\hfill k_{2}lnS+lnW\left(y\right)& =ln\mathsf{\Phi }\end{array}$$

i.e.:

$$\begin{array}{cc}\hfill ln{S}^{k_2}+lnW\left(y\right)& =ln\mathsf{\Phi }\end{array}$$

and therefore:

$$\begin{array}{c}\hfill \mathsf{\Phi }(S,y)=S^{k_2}W\left(y\right).\end{array}$$

(68)

substituting Equation (68) where there is $\mathsf{\Phi }$ into Equation (66), we can find:

$$\begin{array}{c}\hfill V(t,S,y)=e^{k_{1}t}{S}^{k_2}W\left(y\right).\end{array}$$

(69)

one can use Equation (68) to reduce Equation (46) by computing partial derivatives of $\mathsf{\Phi }$ with respect to S and y as follows:

$$\begin{array}{ccc}\hfill {\mathsf{\Phi }}_y& =& S^{k_2}{W}_y,\hfill \\ \hfill {\mathsf{\Phi }}_S& =& k_2{S}^{k_2-1}W,\hfill \\ \hfill {\mathsf{\Phi }}_{Sy}& =& k_2{S}^{k_2-1}{W}_y,\hfill \\ \hfill {\mathsf{\Phi }}_{SS}& =& k_2(k_2-1)S^{k_2-2}W,\hfill \\ \hfill {\mathsf{\Phi }}_{yy}& =& S^{k_2}{W}_{yy}.\hfill \end{array}$$

substituting these partial derivatives into Equation (46) gives:

$$\begin{array}{ccc}\hfill 0& =& {\beta }^2{W}_{yy}+\left(2\beta \rho y{k}_2+c_{1}y+\frac{c_2}{y}\right)W_y\hfill \\ & +& \left(2k_1-2r(1-k_2)+y^2(k_2^2-k_2)\right).\hfill \end{array}$$

(70)

as explained in Section 4, Maple software can be used to obtain asolution of differential Equation (70). The complete solution $V(t,S,y)$ of the original partial differential equation can then be found by substituting the obtained solution into Equation (69).

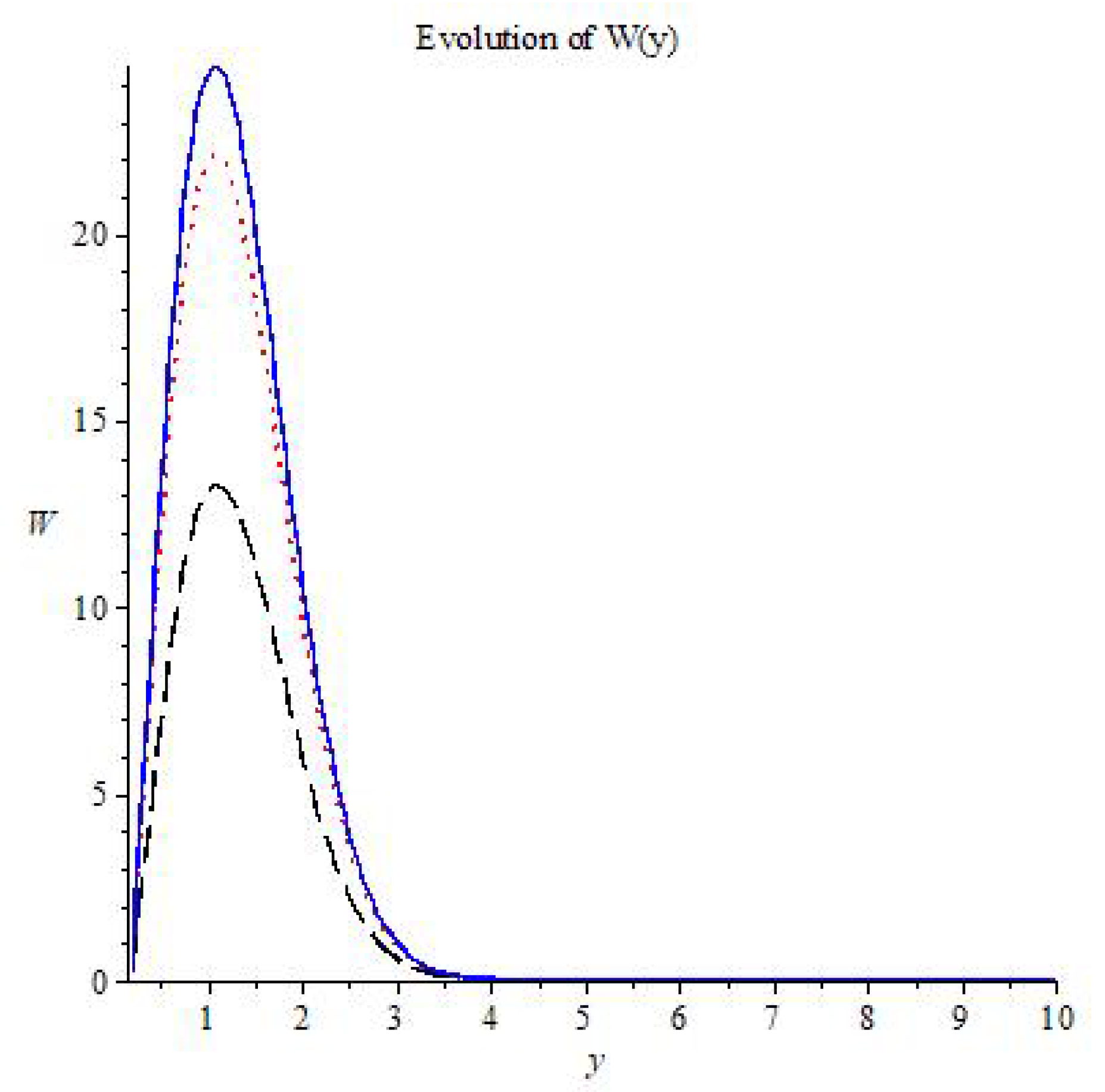
6. Numerical Solutions

The numerical solutions were computed using Maple software, and they are depicted in Figure 1 and Figure 2 below. The parameters in Figure 1 are chosen as $\rho =0.5$, $\beta =0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$, $c_2=-0.05$, for the dashed line; $\rho =0.5$, $\beta =0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$, $c_2=-0.003$, for solid blue line and $\rho =0.5$, $\beta =0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$$c_2=-0.01$, for red dotted line. However, parameters in Figure 2 were chosen as: $\rho =0.5$, $\beta =-0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$, $c_2=-0.05$, for the dashed line; $\rho =0.5$, $\beta =-0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$, $c_2=0.003$, for solid blue line; $\rho =0.5$, $\beta =0.7$, $k_1=1$, $k_2=0.5$, $r=0.5$, $c_1=-0.01$, and $c_2=-0.01$, for the red dotted line.

7. Conclusions

This study looked at the evolution of the solution of the Black–Scholes model for stochastic volatility using the technique known as the modified local one-parameter transformation, and symmetries were obtained and then used to obtain an invariant solution. The model was assumed to follow the Ornstein–Uhlenbeck process, and the Lie symmetry analysis reduced the model to a second-order ordinary differential equation. This process was then applied to the Heston model. The future work in this regard will be to incorporate the dividend yield and observe how the solutions evolve. Another possible extension of the model is to consider an interest rate that is not constant, as an instance interest rate can be considered to be a function of time or a stochastic process.