A Statistical Analysis of Observational Hubble Parameter Data to Discuss the Cosmology of Holographic Chaplygin Gas
Abstract
1. Introduction
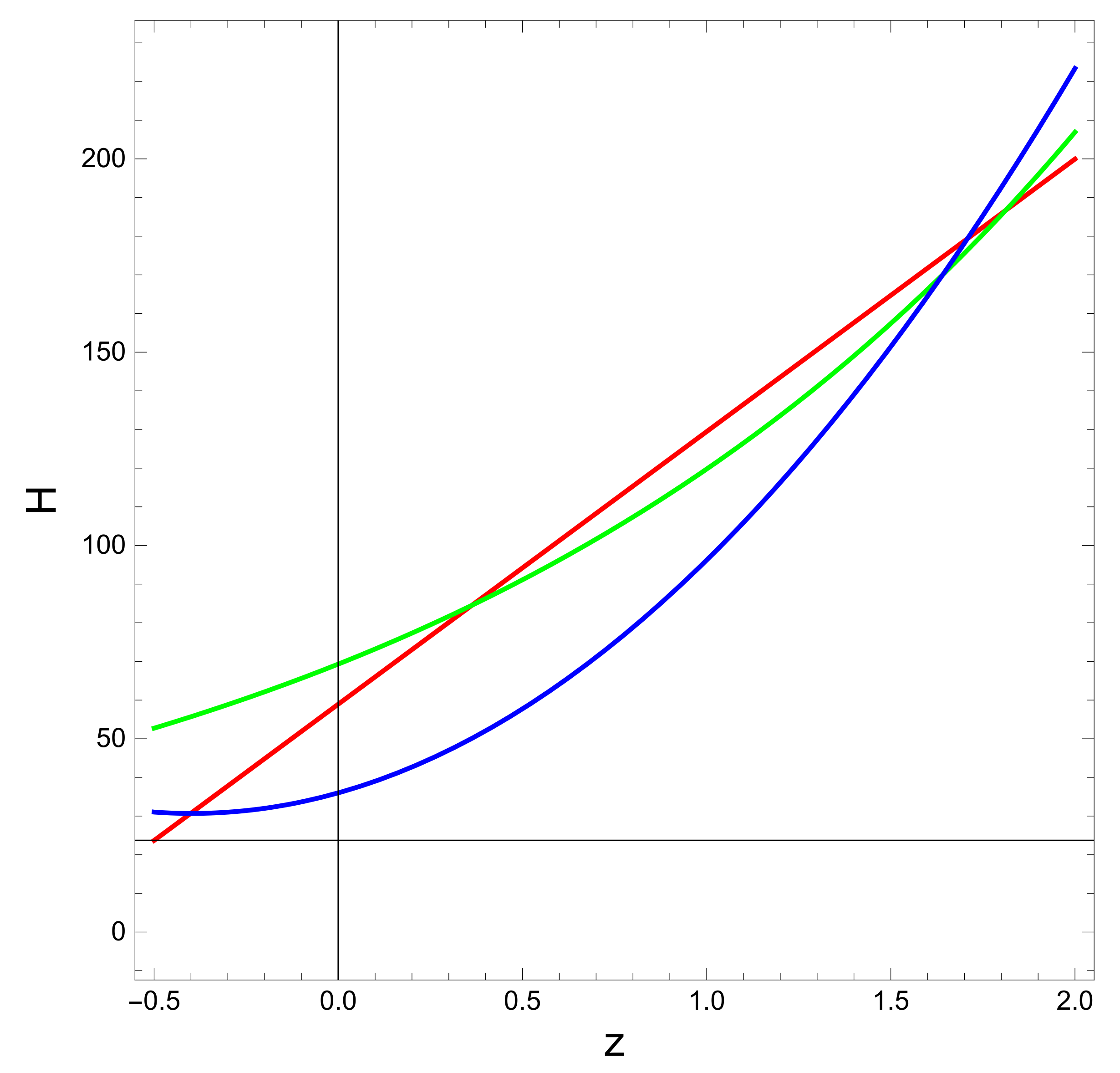
2. Fitting Regression Equation to the Observational Data
3. Generalized Chaplygin Gas (GCG) in Holographic Ricci Dark Energy (HRDE) Framework
4. Concluding Remarks and Future Developments
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DE | Dark Energy |
| EoS | Equation of State |
| GCG | Generalized Chaplygin Gas |
| HRDE | Holographic Ricci Dark Energy |
| CG | Chaplygin Gas |
References
- Amendola, L. Dark Energy: Theory and Observations; Reprint edition; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. J. 1998, 116, 1009. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Ω and Λ from 42 High-Redshift Supernovae. Astrophys. J. 1999, 517, 565. [Google Scholar] [CrossRef]
- Astier, P.; Guy, J.; Regnault, N.; Pain, R.; Aubourg, E.; Balam, D.; Basa, S.; Carlberg, R.G.; Fabbro, S.; Fouchez, D.; et al. The Supernova Legacy Survey: Measurement of ΩM, ΩΛ and w from the first year data set. Astron. Astrophys. 2006, 447, 31. [Google Scholar] [CrossRef]
- Tegmark, M.; Strauss, M.; Blanton, M.; Abazajian, K.; Dodelson, S.; Sandvik, H.; Wang, X.; Weinberg, D.; Zehavi, I.; Bahcall, N.; et al. Cosmological parameters from SDSS and WMAP. Phys. Rev. D 2004, 69, 103501. [Google Scholar] [CrossRef]
- Abazajian, K.; Adelman-McCarthy, J.K.; Agueros, M.A.; Allam, S.S.; Anderson, K.S.J.; Anderson, S.F.; Annis, J.; Bahcall, N.A.; Baldry, I.K.; Bastian, S.; et al. The Second Data Release of the Sloan Digital Sky Survey. Astron. J. 2004, 128, 502. [Google Scholar] [CrossRef]
- Abazajian, K.; Adelman-McCarthy, J.K.; Agueros, M.A.; Allam, S.S.; Anderson, K.S.J.; Anderson, S.F.; Annis, J.; Bahcall, N.A.; Baldry, I.K.; Bastian, S.; et al. The third data release of the sloan digital sky survey. Astron. J. 2005, 129, 1755. [Google Scholar] [CrossRef]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Determination of Cosmological Parameters. Astrophys. J. Suppl. 2003, 148, 175. [Google Scholar] [CrossRef]
- Spergel, D.N.; Bean, R.; Dore, O.; Nolta, M.R.; Bennett, C.L.; Dunkley, J.; Hinshaw, G.; Jarosik, N.; Komatsu, E.; Page, L.; et al. Three-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Implications for Cosmology. Astrophys. J. Suppl. 2007, 170, 377. [Google Scholar] [CrossRef]
- Padmanabhan, T. Cosmological Constant—The Weight of the Vacuum. Phys. Rep. 2003, 380, 235. [Google Scholar] [CrossRef]
- Peebles, P.J.E.; Ratra, B. The cosmological constant and dark energy. Rev. Mod. Phys. 2003, 75, 559. [Google Scholar] [CrossRef]
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of dark energy. Int. J. Mod. Phys. D 2006, 15, 1753. [Google Scholar] [CrossRef]
- Salahedin, F.; Pazhouhesh, R.; Malekjani, M. Cosmological constrains on new generalized Chaplygin gas model. Eur. Phys. J. Plus 2020, 135, 1. [Google Scholar] [CrossRef]
- vom Marttens, R.F.; Casarini, L.; Zimdahl, W.; Hipólito-Ricaldi, W.S.; Mota, D.F. Does a generalized Chaplygin gas correctly describe the cosmological dark sector? Phys. Dark Univ. 2017, 15, 114. [Google Scholar] [CrossRef]
- Baffou, E.H.; Houndjo, M.J.S.; Salako, I.G. Viscous generalized Chaplygin gas interacting with f (R, T) gravity. Int. J. Geom. Methods Mod. Phys. 2017, 14, 1750051. [Google Scholar] [CrossRef]
- Carneiro, S.; Pigozzo, C. Observational tests of non-adiabatic Chaplygin gas. J. Cosmol. Astropart. Phys. 2014, 2014, 060. [Google Scholar] [CrossRef]
- Di Valentino, E.; Mukherjee, A.; Sen, A.A. Dark Energy with Phantom Crossing and the H0 tension. Entropy 2021, 23, 404. [Google Scholar] [CrossRef]
- Chimento, L.P.; Richarte, M.G. Dark radiation and dark matter coupled to holographic Ricci dark energy. Eur. Phys. J. C 2013, 73, 2352. [Google Scholar] [CrossRef]
- Gao, C.; Chen, X.; Faraoni, V.; Shen, Y.G. Does the mass of a black hole decrease due to the accretion of phantom energy? Phys. Rev. Lett. 2008, 78, 024008. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Generalized Chaplygin gas, accelerated expansion, and dark-energy-matter unification. Phys. Rev. D 2002, 66, 043507. [Google Scholar] [CrossRef]
- Carroll, S.M. Quintessence and the rest of the world: Suppressing long-range interactions. Phys. Rev. Lett. 1998, 81, 3067. [Google Scholar] [CrossRef]
- Jamil, M. Evolution of a Schwarzschild black hole in phantom-like Chaplygin gas cosmologies. Eur. Phys. J. C 2009, 62, 609–614. [Google Scholar] [CrossRef]
- Babichev, E.; Dokuchaev, V.; Eroshenko, Y. Black hole mass decreasing due to phantom energy accretion. Phys. Rev. Lett. 2004, 93, 021102. [Google Scholar] [CrossRef] [PubMed]
- Nojiri, S.I.; Odintsov, S.D.; Saridakis, E.N. Holographic inflation. Phys. Lett. B 2019, 797, 134829. [Google Scholar] [CrossRef]
- Pourhassan, B. Unified universe history through phantom extended Chaplygin gas. Can. J. Phys. 2016, 94, 659–670. [Google Scholar] [CrossRef]
- Wilks, D.S. Statistical Method in the Atomspheric Sciences; Elsevier Inc.: Burlington, MA, USA, 2006; ISBN 13: 978-0-12-751966-1. [Google Scholar]
- Yu, H.; Ratra, B.; Wang, F.Y. Hubble Parameter and Baryon Acoustic Oscillation Measurement Constraints on the Hubble Constant, the Deviation from the Spatially Flat ΛCDM Model, the Deceleration—Acceleration Transition Redshift, and Spatial Curvature. Astrophys. J. 2018, 856, 3. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Unifying phantom inflation with late-time acceleration: Scalar phantom-non-phantom transition model and generalized holographic dark energy. Gen. Rel. Grav. 2006, 38, 1285. [Google Scholar] [CrossRef]
- Zhang, X. Holographic Ricci dark energy: Current observational constraints, quintom feature, and the reconstruction of scalar-field dark energy. Phys. Rev. D 2009, 79, 103509. [Google Scholar] [CrossRef]
- De Rham, C.; Gabadadze, G.; Tolley, A.J. Resummation of massive gravity. Phys. Rev. Lett. 2011, 106, 231101. [Google Scholar] [CrossRef]
- Arraut, I.; Chelabi, K. Non-linear massive gravity as a gravitational σ-model. EPL (Europhys. Lett.) 2016, 115, 31001. [Google Scholar] [CrossRef]
- De Rham, C.; Gabadadze, G. Generalization of the Fierz-Pauli action. Phys. Rev. D 2010, 82, 044020. [Google Scholar] [CrossRef]
- Wu, Y.; MacFadyen, A. Constraining the outflow structure of the binary neutron star merger event GW170817GRB170817A with a Markov chain Monte Carlo analysis. Astrophys. J. 2018, 11, 869. [Google Scholar]
- Ni, T.-U. Gavitational waves, dark energy and inflation. Mod. Phys. Lett. A 2010, 25, 922. [Google Scholar] [CrossRef]
- Shibata, M.; Nakao, K.-I.; Nakamura, T.; Maeda, K.-I. Dynamical evolution of gravitational waves in asymptotically de Sitter spacetime. Phys. Rev. D 1994, 50, 708. [Google Scholar] [CrossRef]
- Arraut, I. About the propagation of the Gravitational Waves in an asymptotically de-Sitter space: Comparing two points of view. Mod. Phys. Lett. A 2013, 28, 150019. [Google Scholar] [CrossRef]

| z | H (z) (km/s/Mpc) |
|---|---|
| 0.07 | 69 |
| 0.09 | 69 |
| 0.12 | 68.6 |
| 0.17 | 83 |
| 0.179 | 75 |
| 0.199 | 75 |
| 0.2 | 72.9 |
| 0.27 | 77 |
| 0.28 | 88.8 |
| 0.352 | 83 |
| 0.38 | 81.9 |
| 0.3802 | 83 |
| 0.4 | 95 |
| 0.4004 | 77 |
| 0.4247 | 87.1 |
| 0.4497 | 92.8 |
| 0.47 | 89 |
| 0.4783 | 80.9 |
| 0.48 | 97 |
| 0.52 | 90.8 |
| 0.593 | 104 |
| 0.61 | 97.8 |
| 0.68 | 92 |
| 0.781 | 105 |
| 0.875 | 125 |
| 0.88 | 90 |
| 0.9 | 117 |
| 1.037 | 154 |
| 1.3 | 168 |
| 1.363 | 160 |
| 1.43 | 177 |
| 1.53 | 140 |
| 1.75 | 202 |
| 1.965 | 186.5 |
| 2.34 | 223 |
| 2.36 | 227 |
| Regression Model | |
|---|---|
| 0.9394 | |
| 0.9246 | |
| 0.9404 |
| Cases | PCC | Conclusion on | ||
|---|---|---|---|---|
| c = 0.45, , B = 106 | 0.531 | 0.282 | 22.933 | Accepted at level |
| c = 0.45, , B = 108 | 0.528 | 0.278 | 68.399 | Not accepted |
| c = 0.45, , B = 109 | 0.524 | 0.274 | 118.481 | Not accepted |
| c = 0.50, , B = 106 | 0.531 | 0.282 | 17.146 | Accepted at all levels |
| c = 0.50, , B = 108 | 0.528 | 0.278 | 46.843 | Not accepted |
| c = 0.50, , B = 109 | 0.524 | 0.275 | 77.761 | Not accepted |
| c = 0.75, , B = 107 | 0.531 | 0.282 | 54.615 | Not accepted |
| c = 0.75, , B = 109 | 0.528 | 0.278 | 108.695 | Not accepted |
| c = 0.75, , B = 1010 | 0.524 | 0.275 | 153.534 | Not accepted |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sarkar, A.; Chattopadhyay, S.; Güdekli, E. A Statistical Analysis of Observational Hubble Parameter Data to Discuss the Cosmology of Holographic Chaplygin Gas. Symmetry 2021, 13, 701. https://doi.org/10.3390/sym13040701
Sarkar A, Chattopadhyay S, Güdekli E. A Statistical Analysis of Observational Hubble Parameter Data to Discuss the Cosmology of Holographic Chaplygin Gas. Symmetry. 2021; 13(4):701. https://doi.org/10.3390/sym13040701
Chicago/Turabian StyleSarkar, Amrita, Surajit Chattopadhyay, and Ertan Güdekli. 2021. "A Statistical Analysis of Observational Hubble Parameter Data to Discuss the Cosmology of Holographic Chaplygin Gas" Symmetry 13, no. 4: 701. https://doi.org/10.3390/sym13040701
APA StyleSarkar, A., Chattopadhyay, S., & Güdekli, E. (2021). A Statistical Analysis of Observational Hubble Parameter Data to Discuss the Cosmology of Holographic Chaplygin Gas. Symmetry, 13(4), 701. https://doi.org/10.3390/sym13040701







