Recoil Reduction Method of Gun with Side to Rear Jet Controlled by Piston Motion
Abstract
1. Introduction
2. Principle of the Piston-Controlled Gun
3. Theoretical Models
3.1. Assumptions of the Model
- (1)
- Each phase is a continuum in line with the assumption of two-fluid model.
- (2)
- One-dimensional unsteady flow is considered in the barrel, the piston cavity, and the exhaust pipe.
- (3)
- The viscous dissipation of propellant gas and its heat loss to the wall are ignored.
- (4)
- The propellant gas flow is treated as an isentropic flow when the propellant gas flows through a bifurcated tube or elbow.
3.2. The Governing Equations of the Two-Phase Flow in the Barrel
3.3. Dynamic Model of Gas–Solid Coupling in the Piston Cavity
3.4. The Governing Equations of Two-Phase Flow in the Exhaust Pipe
3.5. Gas Flow Model at the Barrel Vent and Rear Spray Channel
3.6. Calculation of the Recoil Reduction Efficiency
4. Numerical Method
4.1. Difference Scheme
4.2. Boundary Conditions
5. Results and Discussion
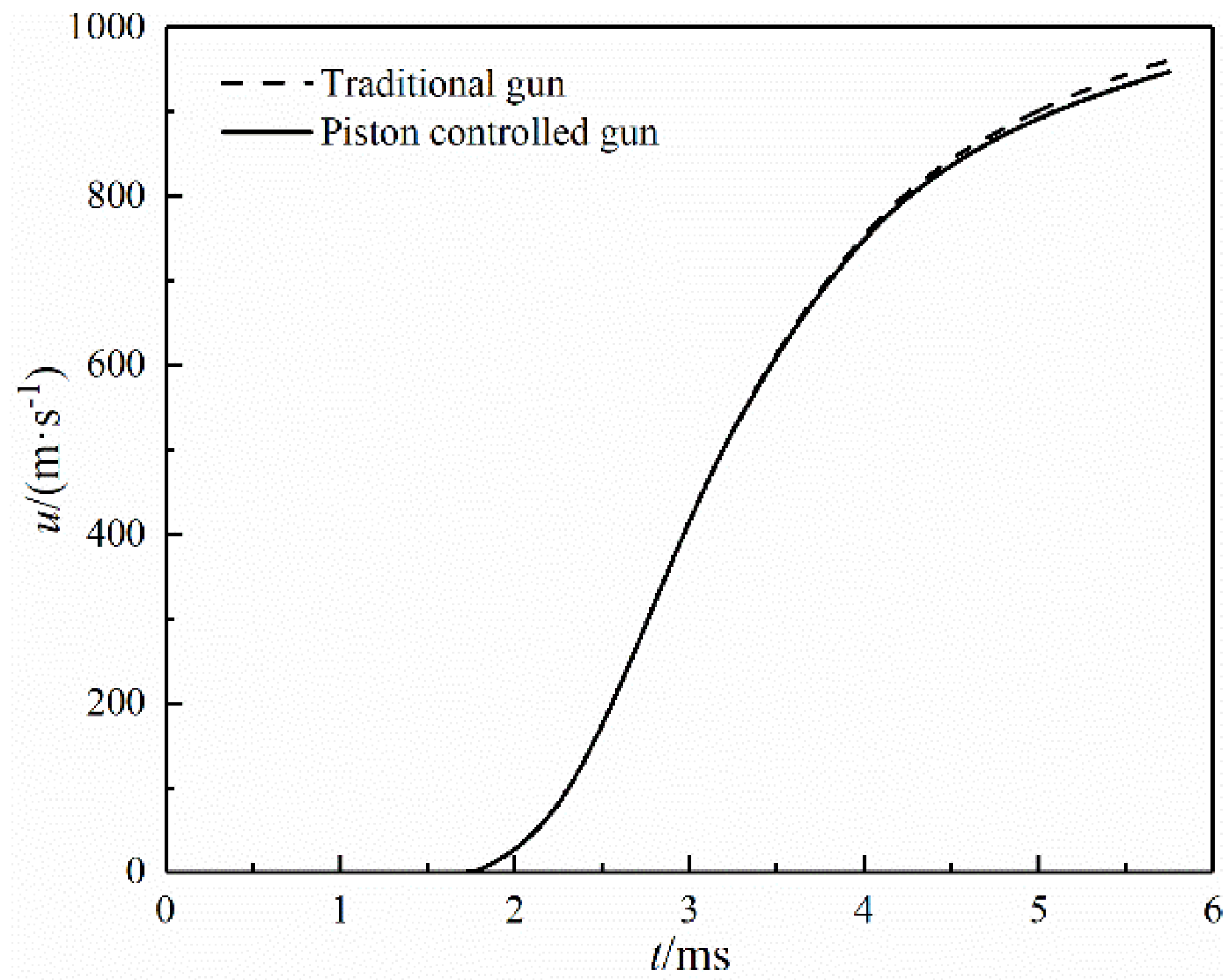
5.1. Validation
5.2. Decision of the Opening Time of the Rear Spray Channel
5.3. Flow Field in the Piston Cavity
5.4. Rarefaction Wave Front Propagation
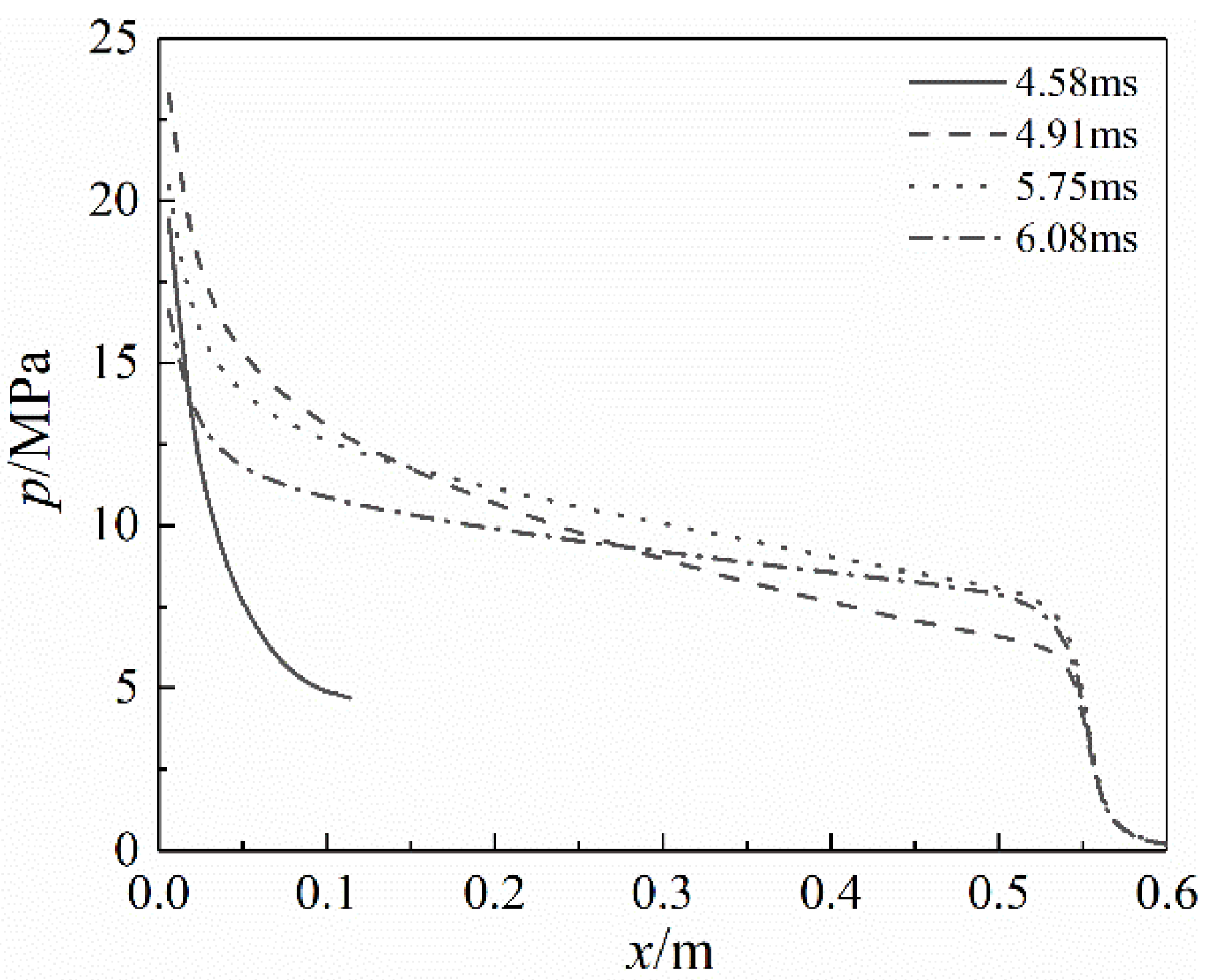
5.5. Flow Field in the Barrel
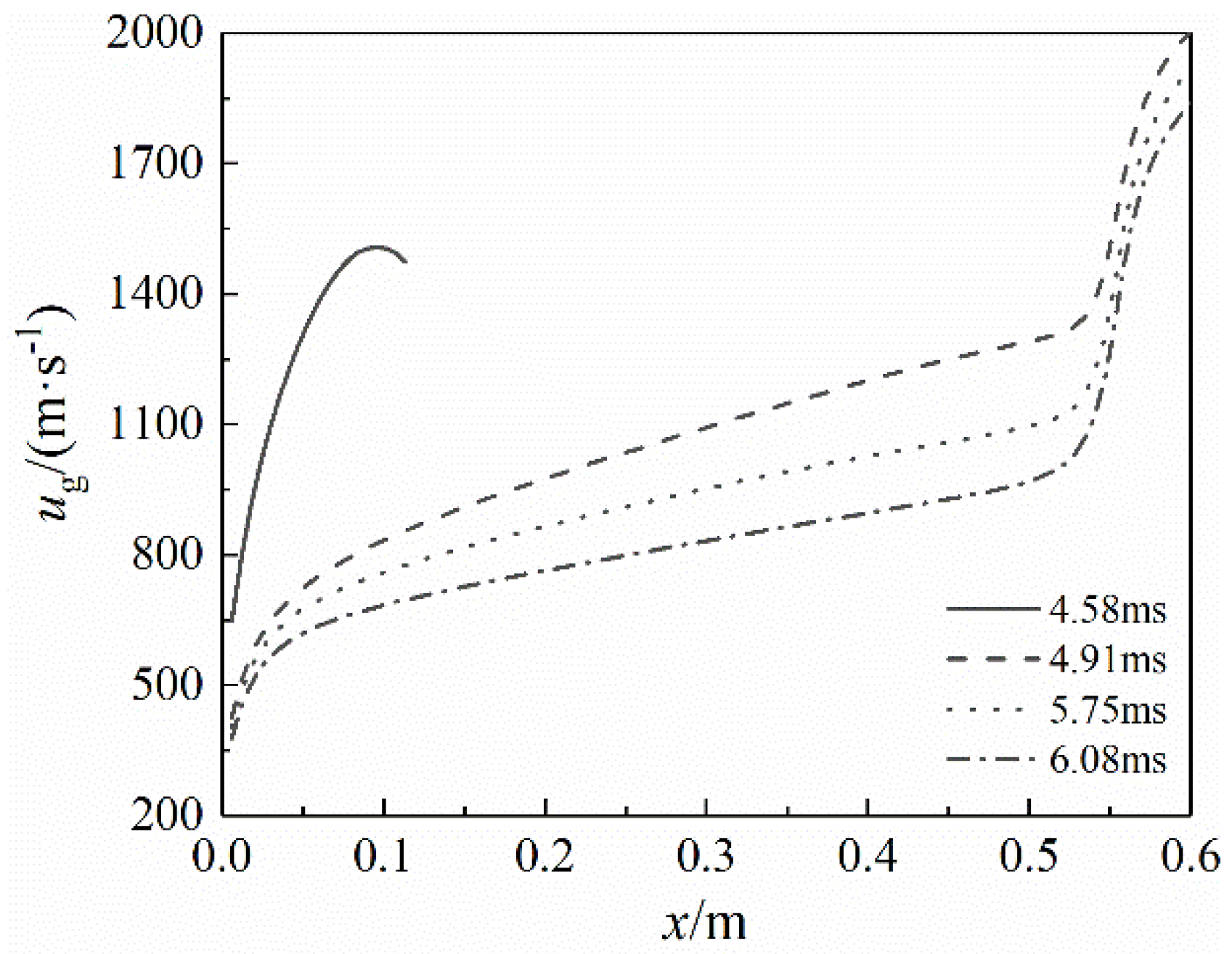
5.6. Flow Field in the Exhaust Pipe
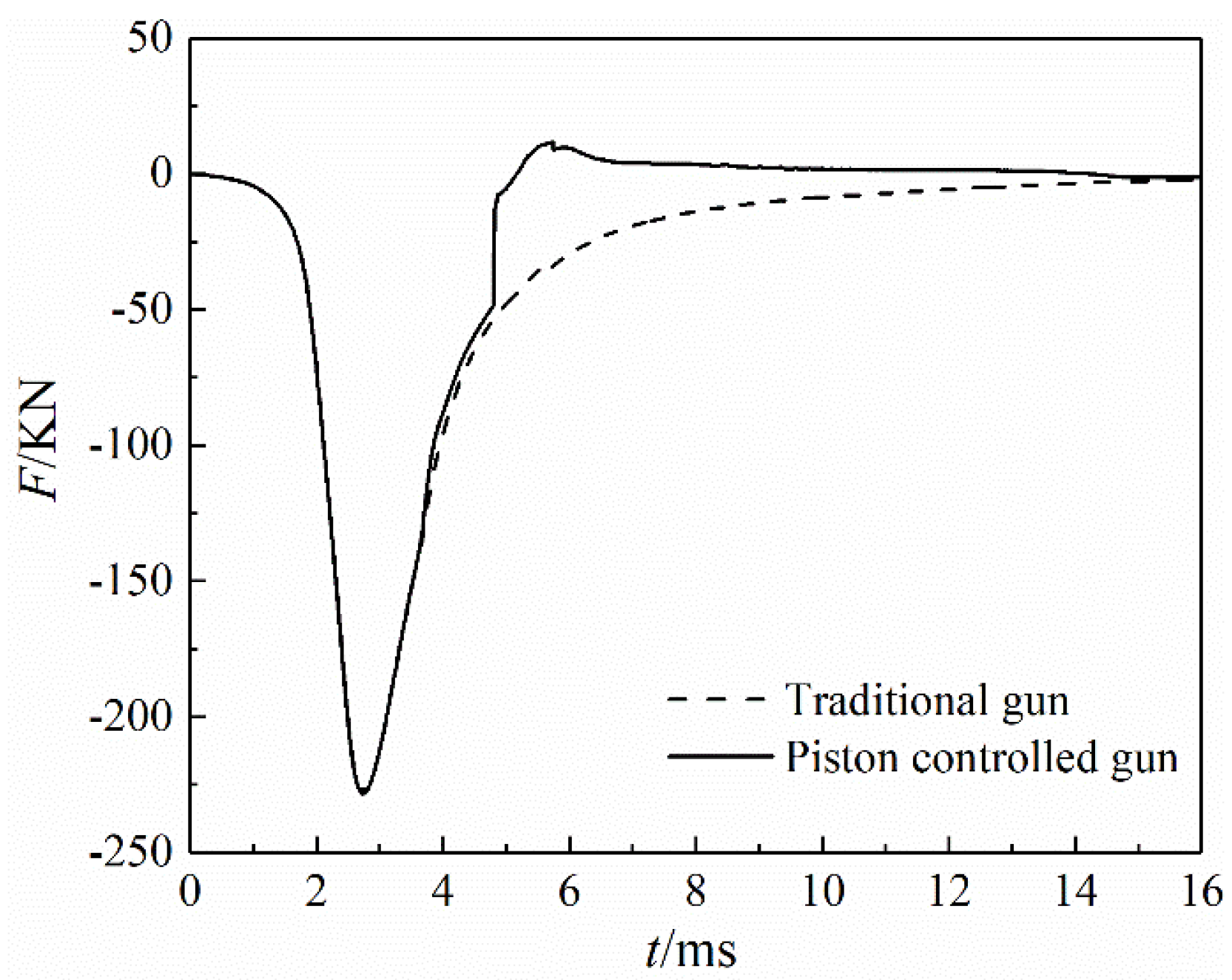
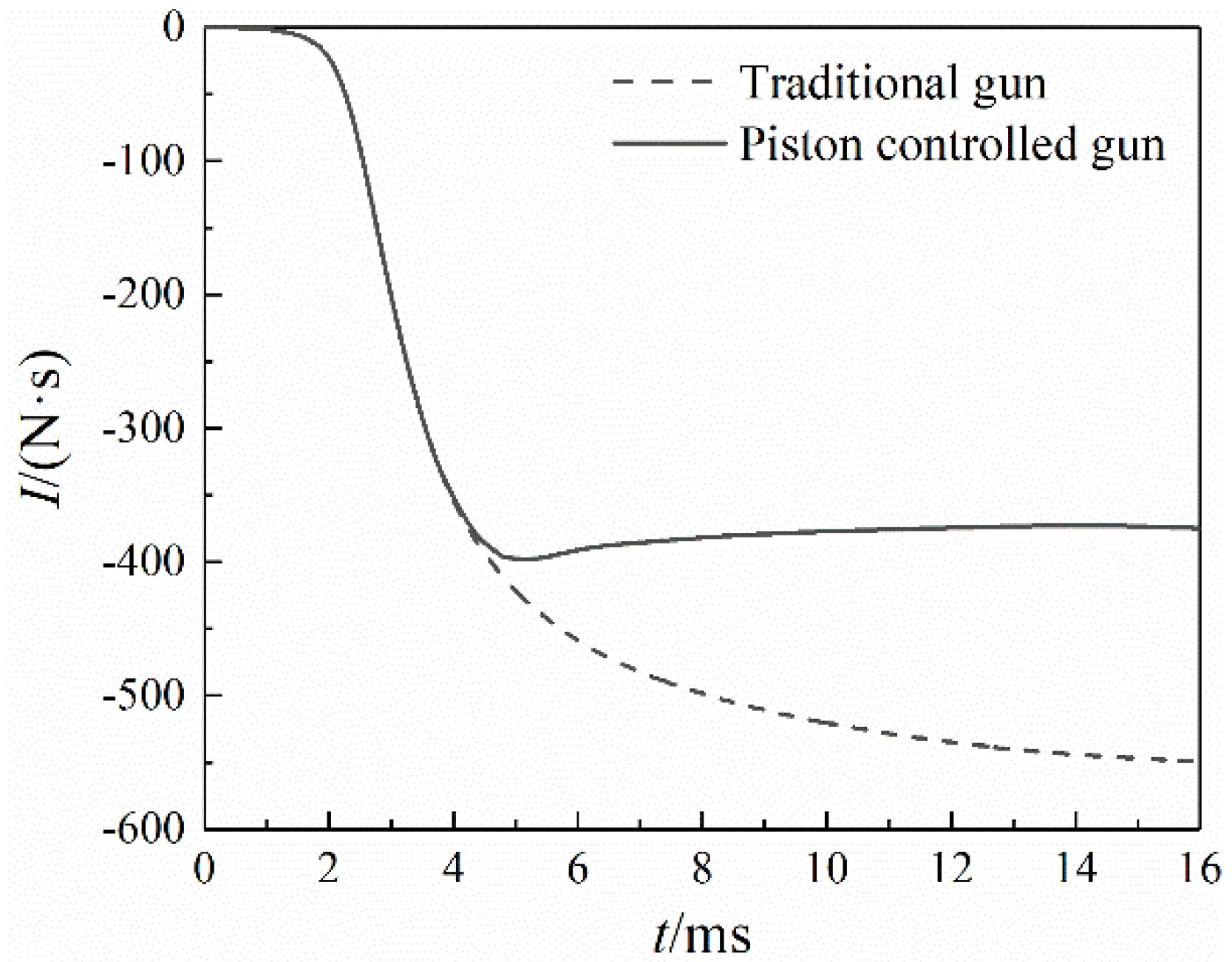
5.7. Analysis of the Recoil Reduction Efficiency
6. Conclusions
- (1)
- The recoil reduction mechanism of the piston-controlled gun was revealed. By controlling the lateral ejecting of propellant gas with the piston, the rarefaction wave produced at the barrel vent just caught the projectile at the muzzle exit. Thus, the projectile base pressure did not reduce too much during the propulsion process, minimally reducing the muzzle velocity. Meanwhile, the recoil momentum was significantly reduced by venting high-pressure propellant gas from the barrel as much as possible.
- (2)
- The piston-controlled gun could be reset within the firing cycle, so the continuous firing mode is not affected. However, the rear spray channel will be closed during the piston reset motion, which is negative to the recoil reduction efficiency.
- (3)
- After the projectile reached the barrel vent, some high-pressure propellant gas flowed into the piston cavity, so the projectile base pressure dropped slightly. However, the piston cavity was closed before the rear spray channel was opened, so the velocity loss of the piston-controlled gun is similar to that of a traditional air-guided gun.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kang, K.J.; Gimm, H.I. Numerical and experimental studies on the dynamic behaviors of a gun that uses the soft recoil system. J. Mech. Sci. Technol. 2012, 26, 2167–2170. [Google Scholar] [CrossRef]
- Qing, O.Y.; Zheng, J.J.; Li, Z.C. Controllability analysis and testing of a novel magnetorheological absorber for field gun recoil mitigation. Smart Mater. Struct. 2016, 25, 115041. [Google Scholar]
- Ahmadian, M.; Poynor, J.C. An evaluation of magneto rheological dampers for controlling gun recoil dynamics. Shock Vib. 2001, 8, 147–155. [Google Scholar] [CrossRef]
- Li, J.S.; Qiu, M.; Liao, Z.Q. Dynamic modeling and simulation of gatling gun with muzzle assistant-rotating and recoil absorber. Acta Armam. 2014, 35, 1344–1349. [Google Scholar]
- Cayzac, R.; Carettet, E.; Alziary de Roquefort, T. 3D unsteady intermediate ballistics modelling: Muzzle brake and sabot separation. In Proceedings of the 24th International Symposium on Ballistics, New Orleans, LA, USA, 22–26 September 2008. [Google Scholar]
- Kathe, E. Sonic Rarefaction Wave Recoilless Gun System. Patent 6460446B1-EN, 8 August 2002. [Google Scholar]
- Kathe, E.; Dillon, R. Sonic Rarefaction Wave Low Recoil Gun; US Army Armament Research, No. ARCCB-TR-02001; Watervilet: New York, NY, USA, 2002. [Google Scholar]
- Liao, Z.Q.; Qiu, M. Gas Dynamics of Automatic Weapons, 1st ed.; Defense Industry Press: Beijing, China, 2015; p. 247. [Google Scholar]
- Chen, Y.; Liao, Z.Q.; Liu, G.X.; Wang, T. Performance comparison of the two recoilless structure with Laval nozzles. J. Ball 2008, 20, 88–91. [Google Scholar]
- Cheng, C.; Wang, C.; Zhang, X.B. A prediction method for the performance of a low-recoil gun with front nozzle. Def. Technol. 2019, 15, 703–712. [Google Scholar] [CrossRef]
- Zhang, X.B.; Wang, Y.Z. Analysis of dynamic characteristics for rarefaction wave gun during the launching. J. Appl. Mech. 2010, 77, 051601. [Google Scholar] [CrossRef]
- Wang, Y.Z.; Zhang, X.B. Numerical analysis on launch property of rarefaction wave gun. Chin. J. High Pressure Phys. 2009, 23, 433–440. [Google Scholar]
- Zhang, F.; Liao, Z.Q.; Liu, G.X.; Chen, Y. Interior ballistics study on rarefaction wave gun based on theory of two-phase flow. J. Ball 2007, 19, 9–12. [Google Scholar]
- Zhang, F.; Liao, Z.Q.; Liu, G.X.; Chen, Y. Study of influence factors on launching performance of rarefaction wave gun. Acta Armam. 2008, 29, 23–27. [Google Scholar]
- Zhang, F.; Liao, Z.Q.; Liu, G.X.; Chen, Y. Numerical simulation and analysis on front orifice rarefaction wave gun propulsion. J. Syst. Simul. 2008, 20, 5032–5034. [Google Scholar]
- Coffee, T.P. Modeling of the 35-mm Rarefaction Wave Gun; Army Research Laboratory, No. ARL-TR-3792; Aberdeen Province Ground: Harford County, MD, USA, 2006. [Google Scholar]
- Xiao, J.; Yang, G.; Li, H.; Qiu, M.; Liao, Z. Dynamic modeling and simulation of recoil reduction of automatic weapon by time-delay nozzle. Acta Armam. 2017, 38, 1909–1917. [Google Scholar]
- Weng, C.S.; Wang, H. Computational Interior Ballistics, 1st ed.; Defense Industry Press: Beijing, China, 2006; p. 71. [Google Scholar]
- Nussbaum, J.; Helluy, P.; Hérard, J.-M.; Carriére, A. Numerical simulations of gas-particle flows with combustion. Flow Turbul. Combust. 2006, 76, 403–417. [Google Scholar] [CrossRef]
- Rashad, M.M.; Zhang, X.B.; Sadek, H.E.; Cheng, C. Two-phase flow interior ballistics model of laval large caliber guided projectile gun system. Appl. Mech. Mater. 2014, 465, 592–596. [Google Scholar]
- Jang, J.; Oh, S.; Roh, T. Development of three-dimensional numerical model for combustion-flow in interior ballistics. J. Mech. Sci. Technol. 2016, 30, 1631–1637. [Google Scholar] [CrossRef]
- Chirame, N.A.; Pradhan, D.; Naik, S.D. Operator splitting technique with FORCE scheme employed to simulate pressure wave motion inside gun chamber. Appl. Math. Model. 2015, 39, 2139–2154. [Google Scholar] [CrossRef]
- Otón-Martínez, R.A.; Monreal-González, G.; García-Cascales, J.R.; Vera-García, F.F.; Velasco, J.S.F.; Ramírez-Fernández, F.J. An approach formulated in terms of conserved variables for the characterisation of propellant combustion in internal ballistics. Int. J. Numer. Meth. FLUIDS 2015, 79, 394–415. [Google Scholar] [CrossRef]
- Monreal-González, G.; Otón-Martínez, R.A.; Velasco, F.J.S.; García-Cascáles, J.R.; Ramírez-Fernández, F.J. One-dimensional modelling of internal ballistics. J. Energ. Mater. 2017, 35, 397–420. [Google Scholar]
- Menshov, I.S.; Nemtsev, M.Y.; Semenov, I.V. Numerical modeling of wave processes accompanying combustion of in-homogeneously distributed composite propellant. Comp. Math. Math. Phys. 2019, 59, 1528–1541. [Google Scholar] [CrossRef]
- Hu, C.B.; Zhang, X.B. A Riemann problem based coupling method for predicting the combustion of propellant in a gun launching process. Propellants Explos. Pyrotech. 2019, 44, 751–758. [Google Scholar] [CrossRef]
- Cheng, C.; Zhang, X.B. Two-dimensional numerical investigation of gas-solid reactive flow with moving boundary. Combust. Sci. Technol. 2015, 187, 977–998. [Google Scholar] [CrossRef]
- Cheng, C.; Zhang, X.B. Numerical investigation of two-phase reactive flow with two moving boundaries in a two-stage combustion system. Appl. Therm. Eng. 2019, 156, 422–431. [Google Scholar] [CrossRef]
- Cao, R.D.; Zhang, X.B. Design optimization for a launching system with novel structure. Def. Technol. 2019, 15, 680–689. [Google Scholar] [CrossRef]
- Cao, R.D.; Zhang, X.B. A novel launching system applying a relay chamber technology and its optimization. Propellants Explos. Pyrotech. 2019, 44, 1199–1205. [Google Scholar] [CrossRef]



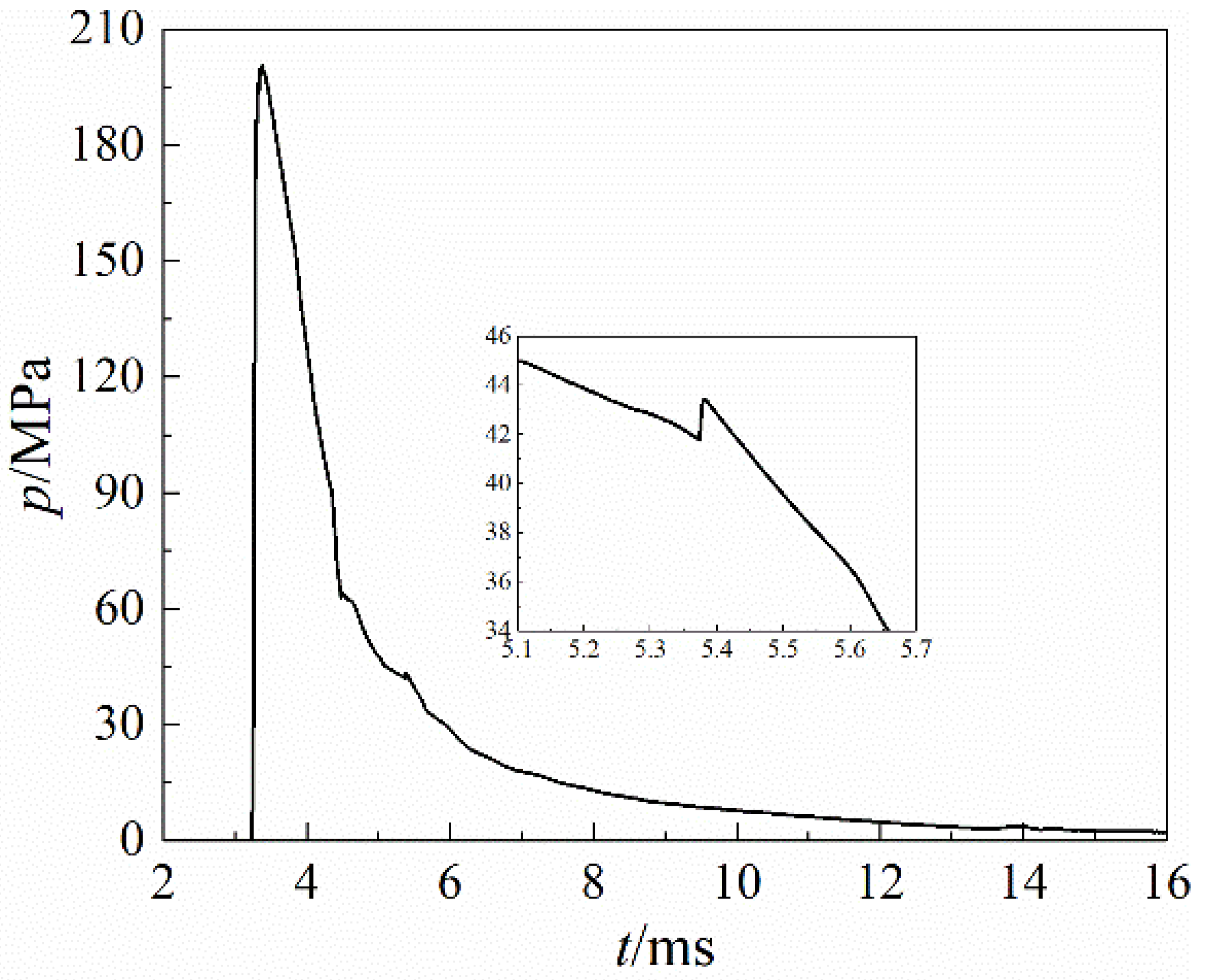
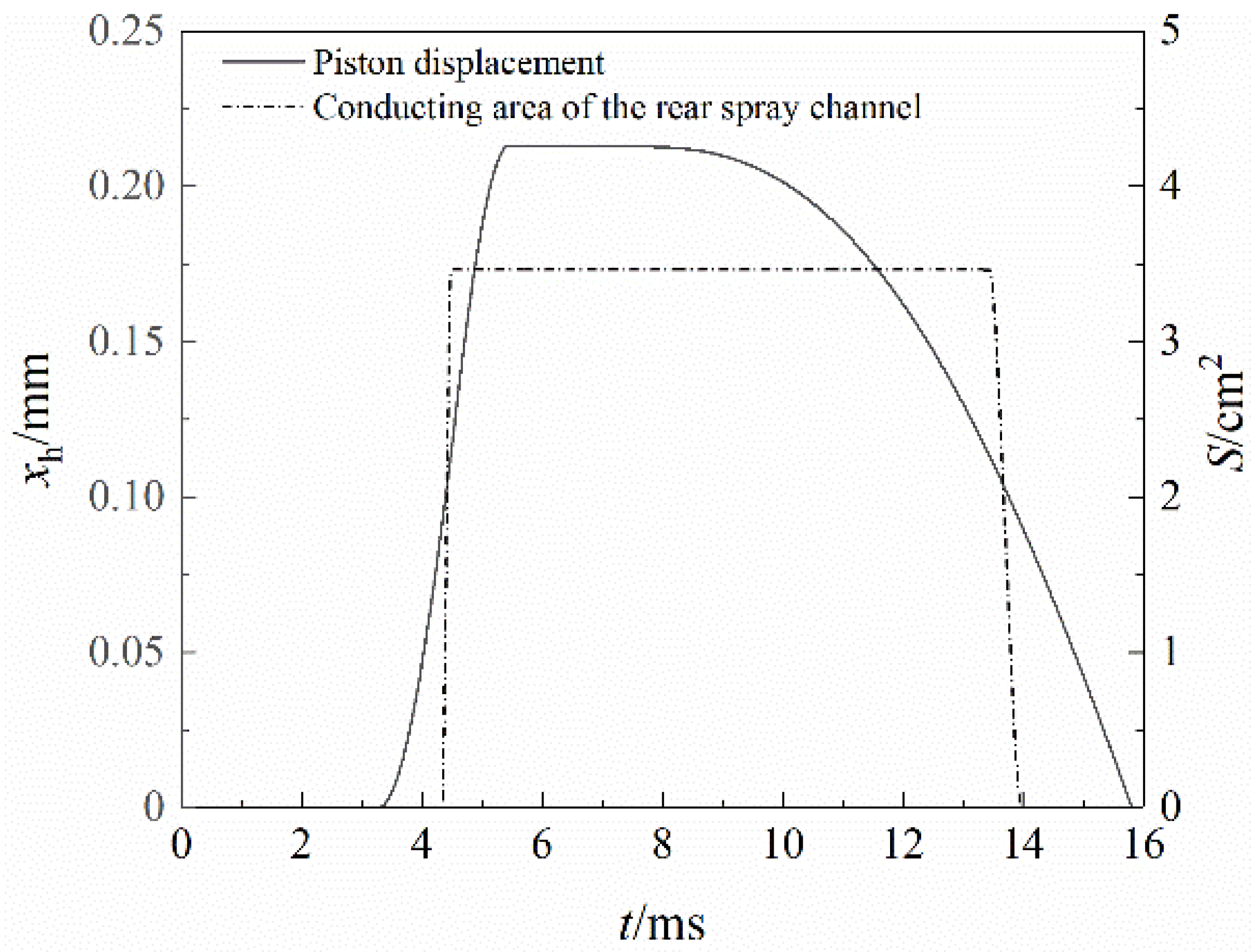


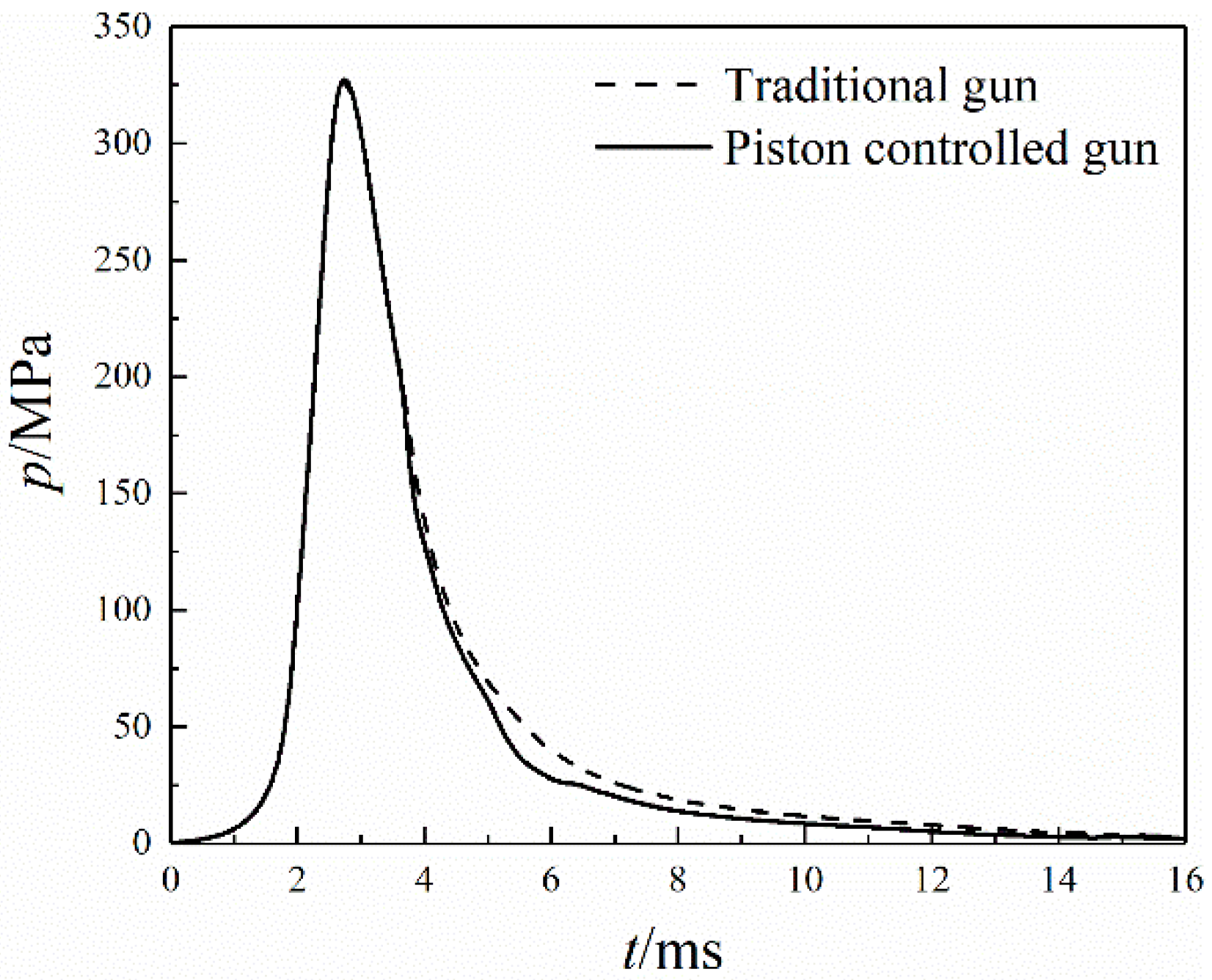
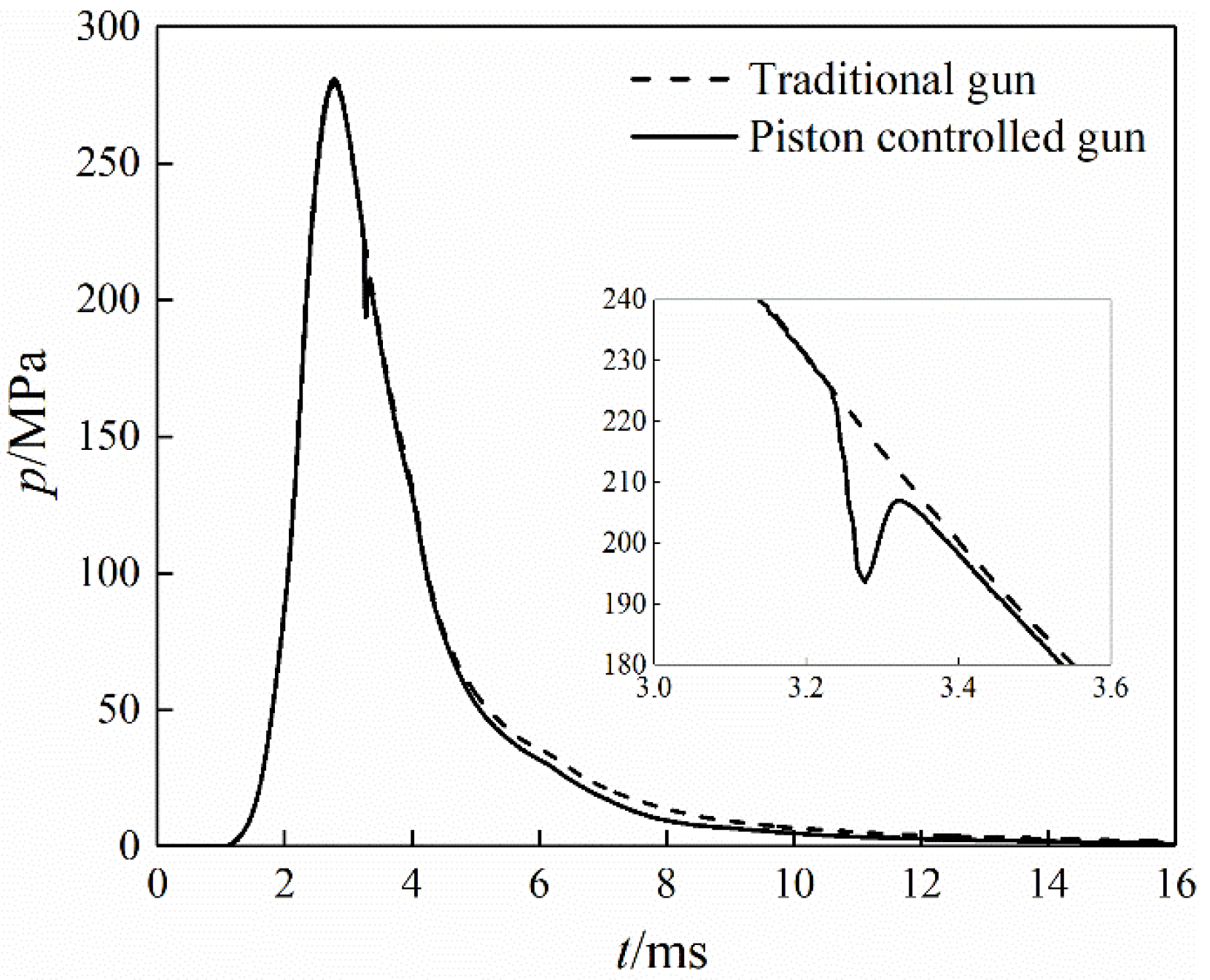
| Computed Value | Acceptable Range | Present |
|---|---|---|
| Maximum breech pressure (MPa) | 355–400 | 367 |
| Maximum projectile base pressure (MPa) | 325–360 | 336 |
| Muzzle velocity (m·s−1) | 660–705 | 673 |
| Exit time (ms) | 14.66–16.58 | 15.57 |
| Parameter | Value |
|---|---|
| Barrel diameter (m) | 0.030 |
| Length of barrel (m) | 2.457 |
| Propellant mass (kg) | 0.119 |
| Propellant density (kg·m−3) | 1600 |
| Projectile mass (kg) | 0.389 |
| Parameter | Value |
|---|---|
| Position of barrel vent (m) | 0.45 |
| Diameter of the piston cavity (m) | 0.021 |
| Piston mass (kg) | 0.3588 |
| Spring stiffness (N·m−1) | 10000 |
| Length of side nozzle (m) | 0.6 |
| Sectional area of the barrel vent (mm2) | 314 |
| Sectional area of the rear spray channel (mm2) | 346 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, M.; Si, P.; Song, J.; Liao, Z. Recoil Reduction Method of Gun with Side to Rear Jet Controlled by Piston Motion. Symmetry 2021, 13, 396. https://doi.org/10.3390/sym13030396
Qiu M, Si P, Song J, Liao Z. Recoil Reduction Method of Gun with Side to Rear Jet Controlled by Piston Motion. Symmetry. 2021; 13(3):396. https://doi.org/10.3390/sym13030396
Chicago/Turabian StyleQiu, Ming, Peng Si, Jie Song, and Zhenqiang Liao. 2021. "Recoil Reduction Method of Gun with Side to Rear Jet Controlled by Piston Motion" Symmetry 13, no. 3: 396. https://doi.org/10.3390/sym13030396
APA StyleQiu, M., Si, P., Song, J., & Liao, Z. (2021). Recoil Reduction Method of Gun with Side to Rear Jet Controlled by Piston Motion. Symmetry, 13(3), 396. https://doi.org/10.3390/sym13030396






