A Reduction of Peak-to-Average Power Ratio Based Faster-Than-Nyquist Quadrature Signals for Satellite Communication
Abstract
1. Introduction
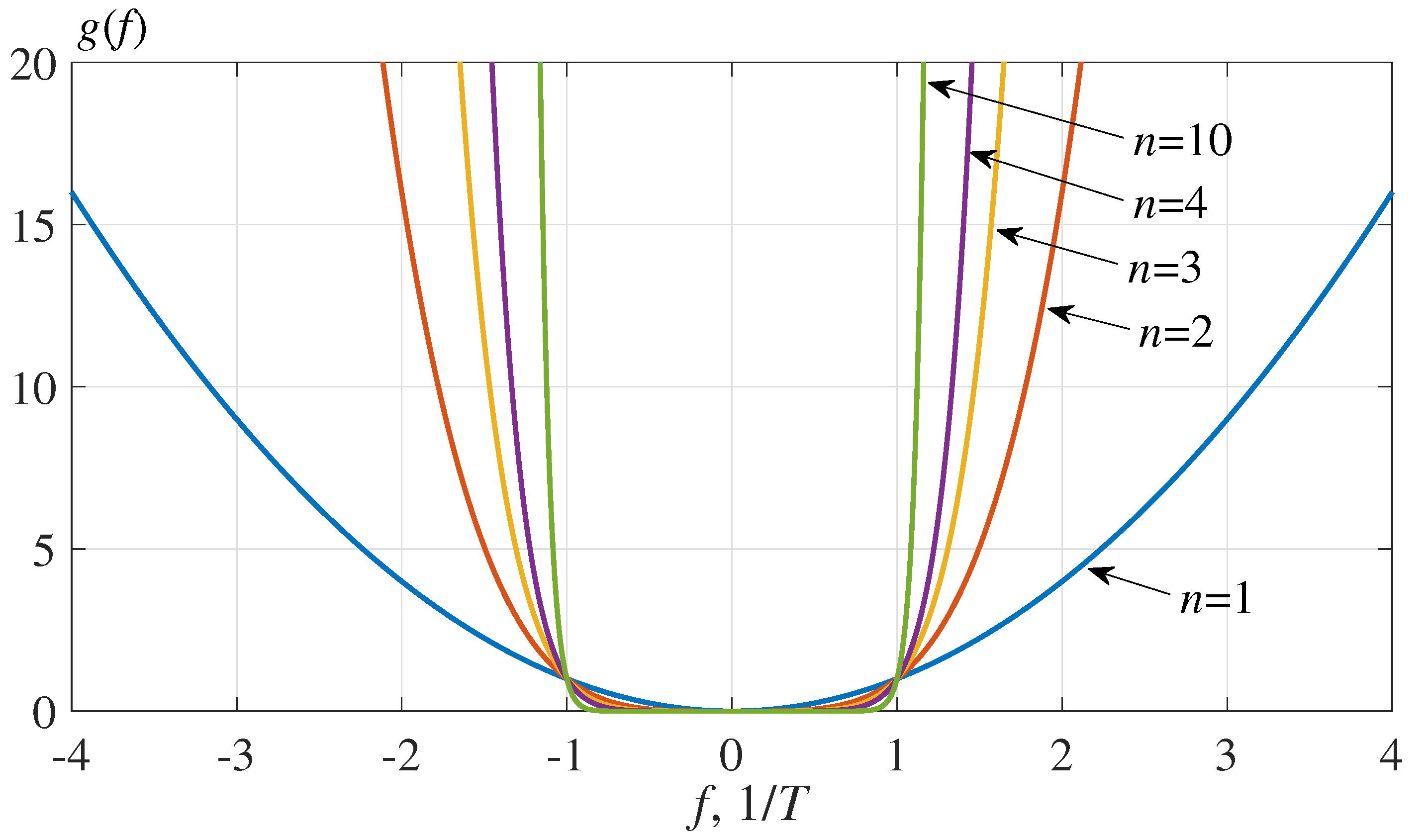
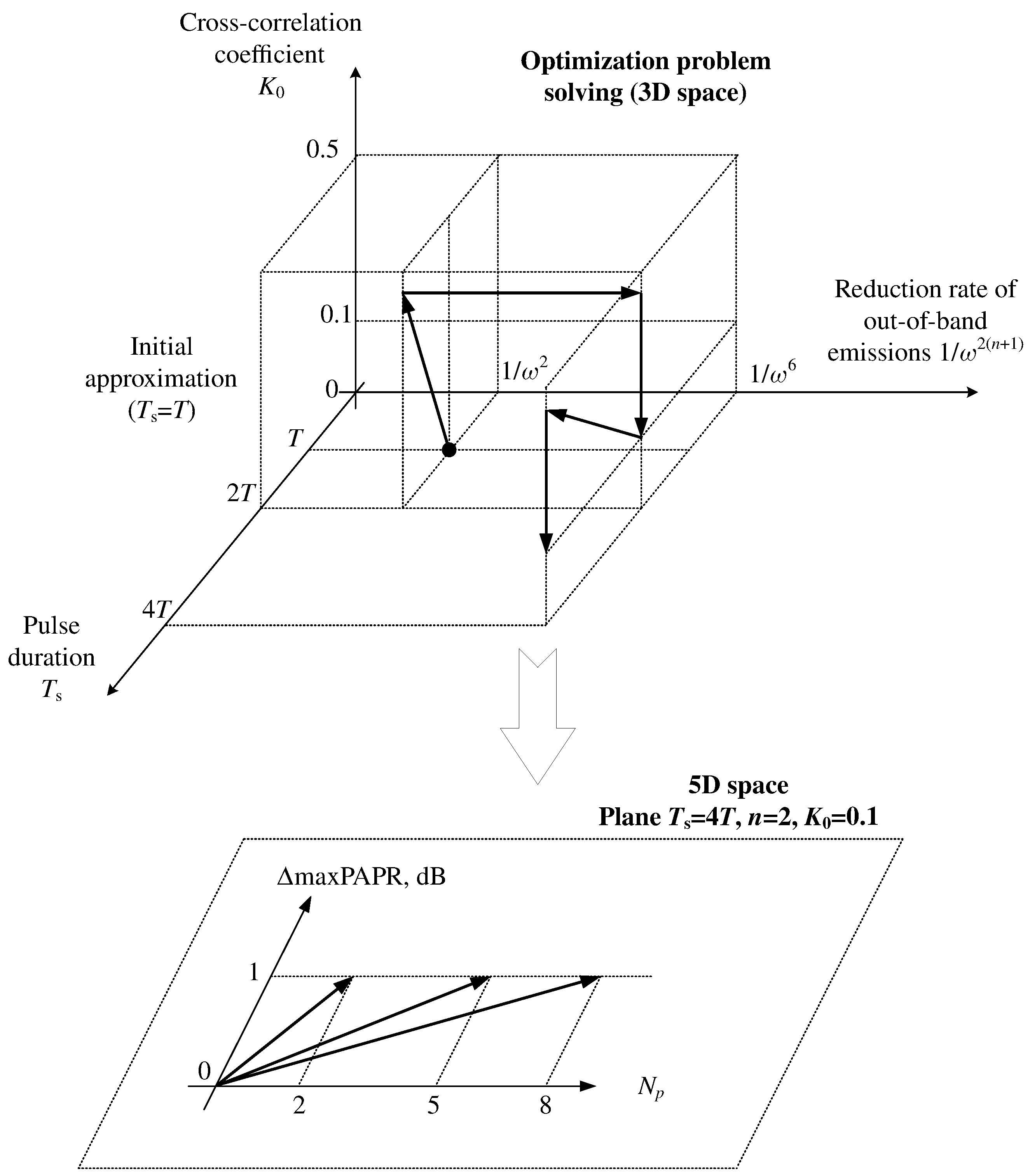
2. Optimization Problem with Constraint on PAPR
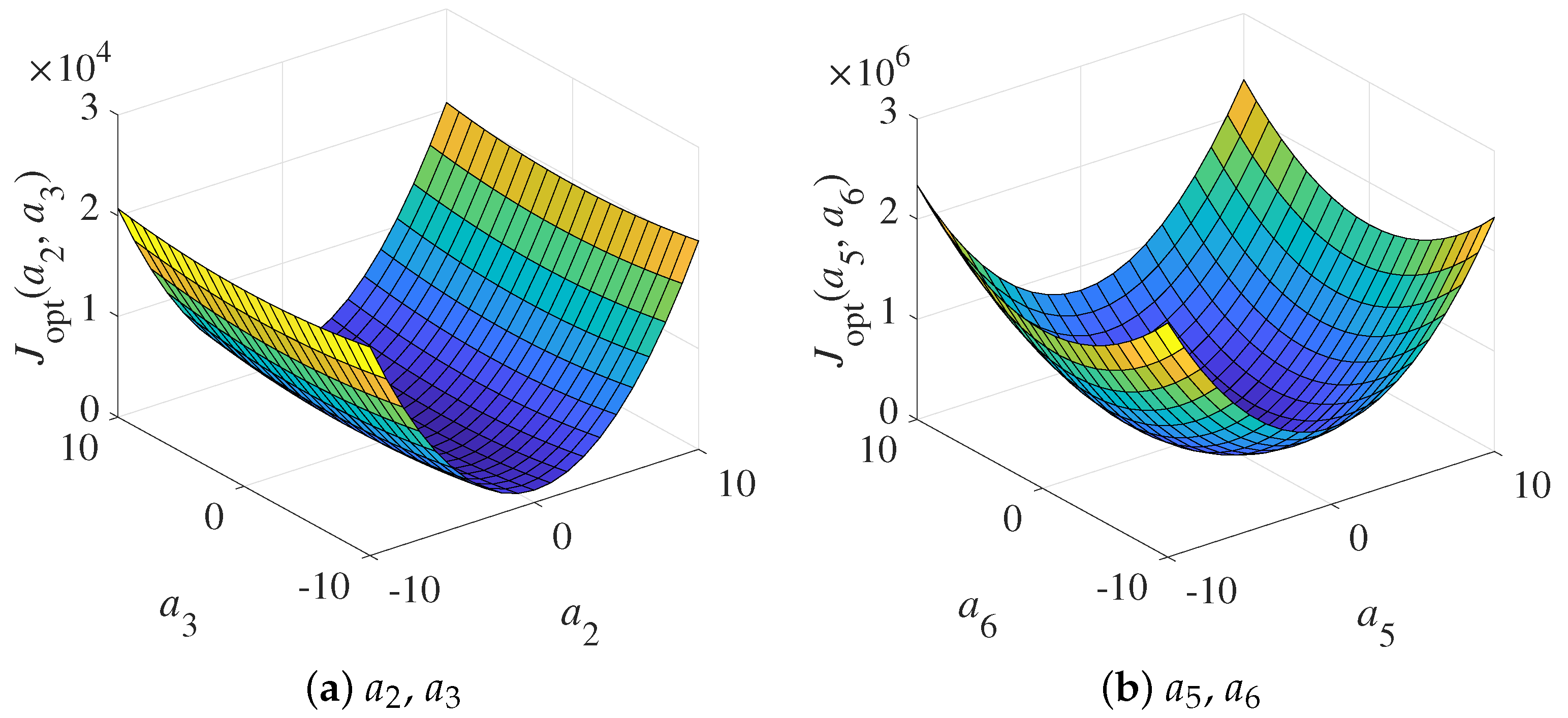
2.1. Optimization Problem-Solving Method
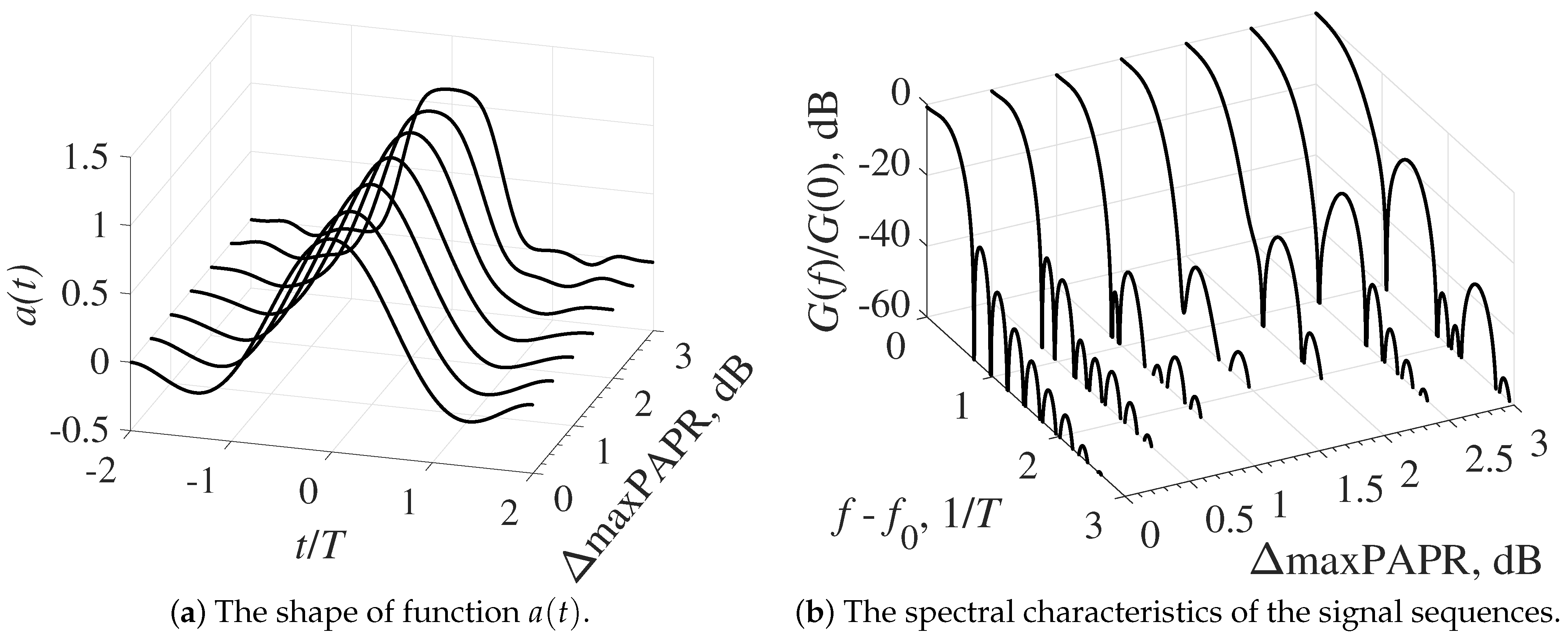
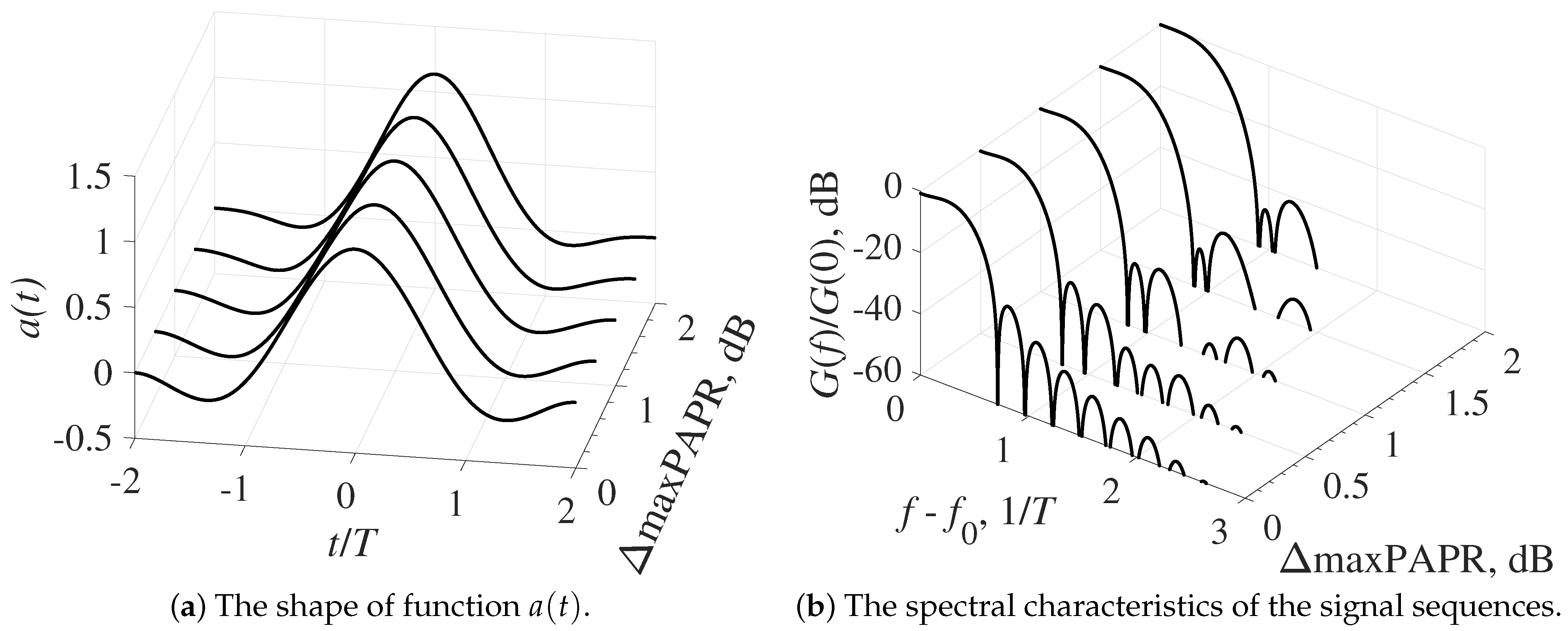
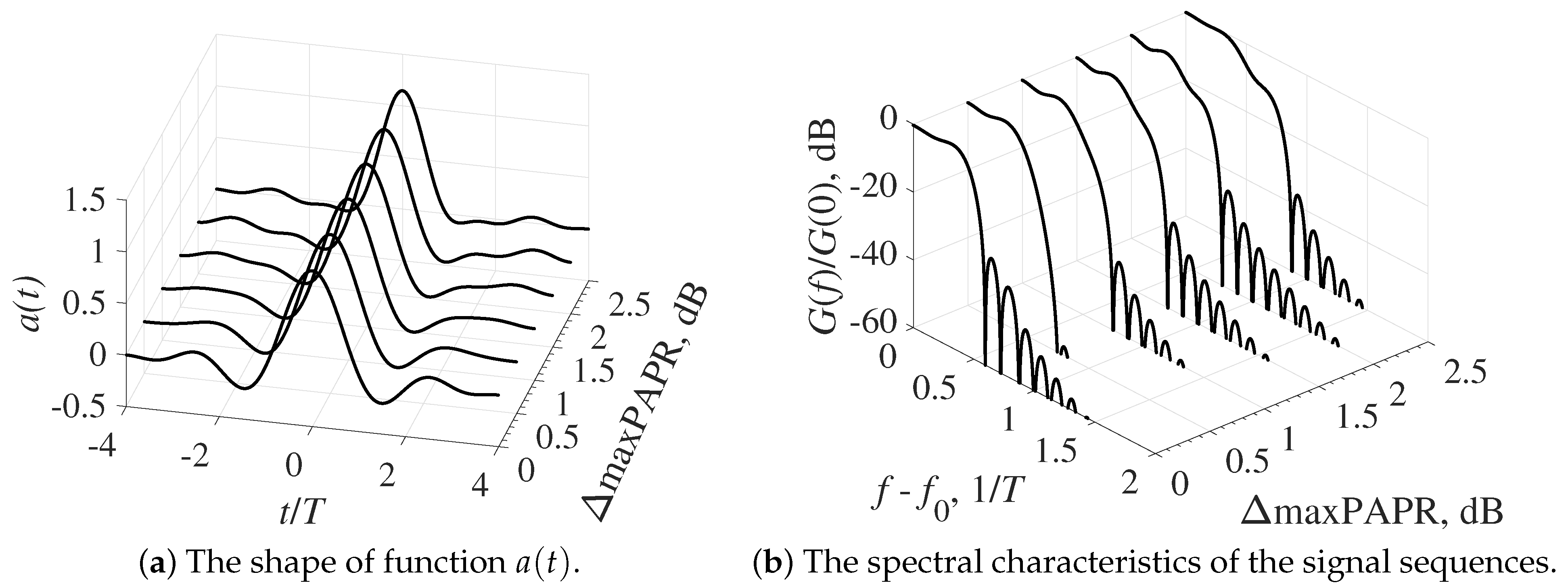
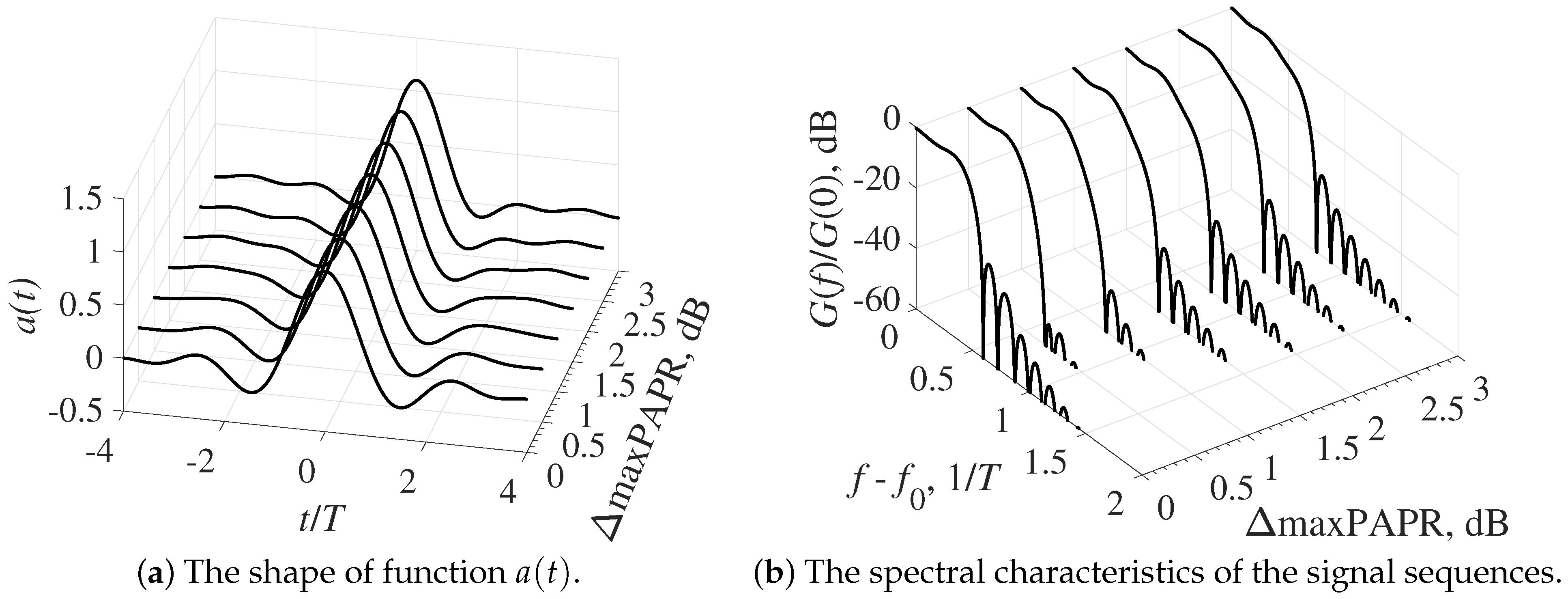
2.2. Results of Optimization Problem Solving
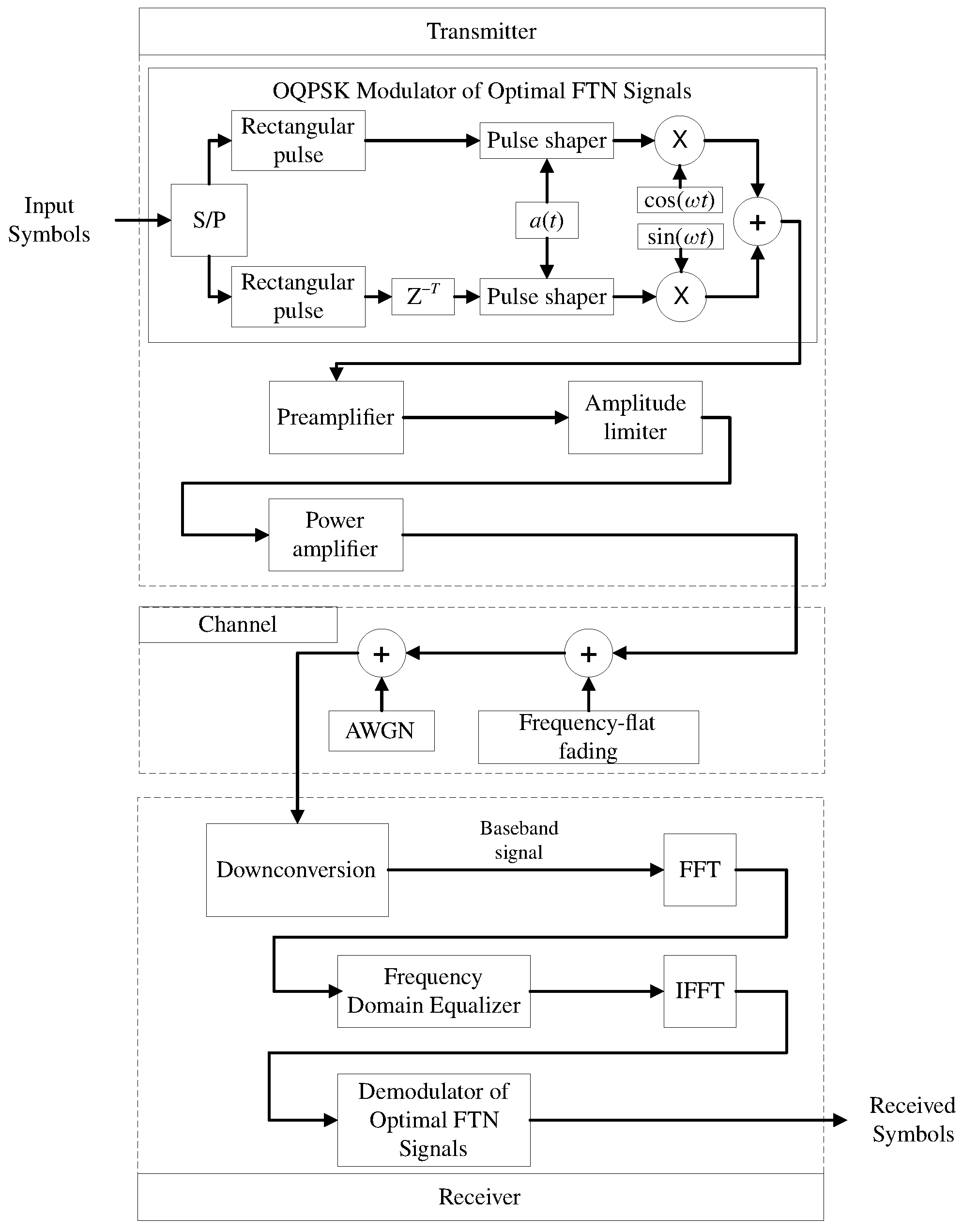
3. Simulation Model
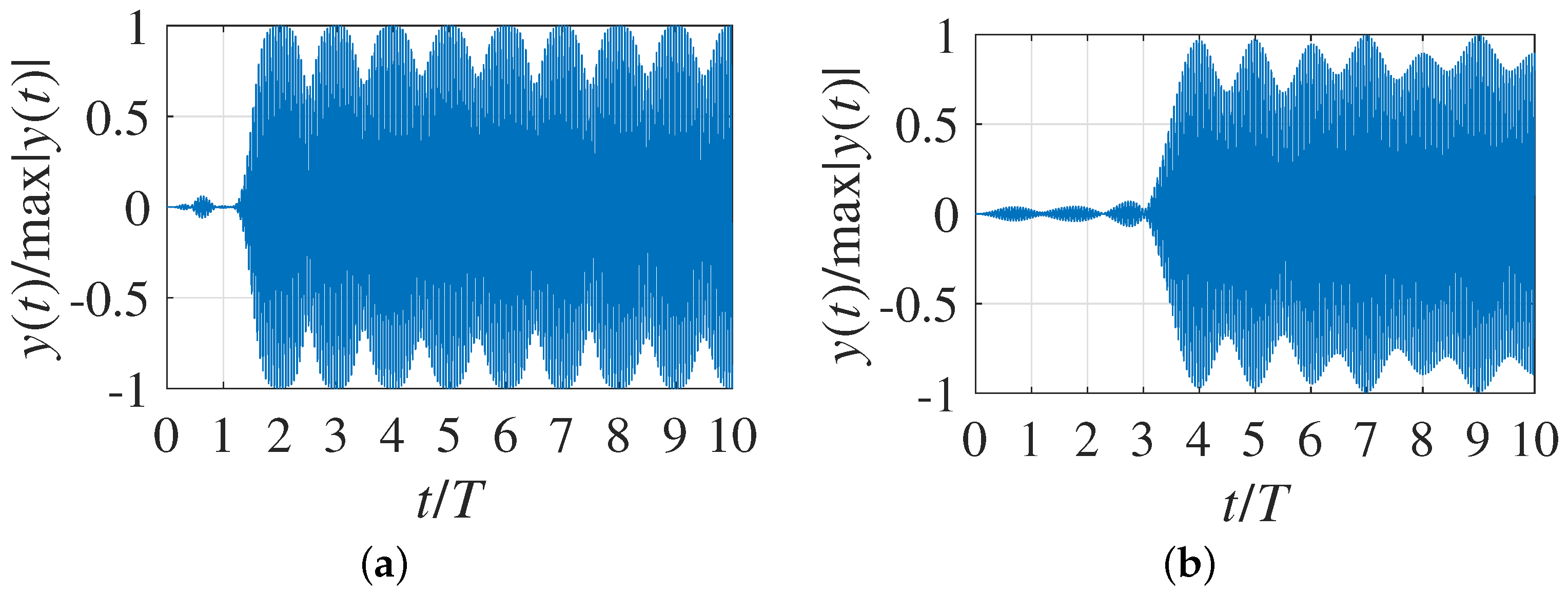
4. Simulation Modeling Results
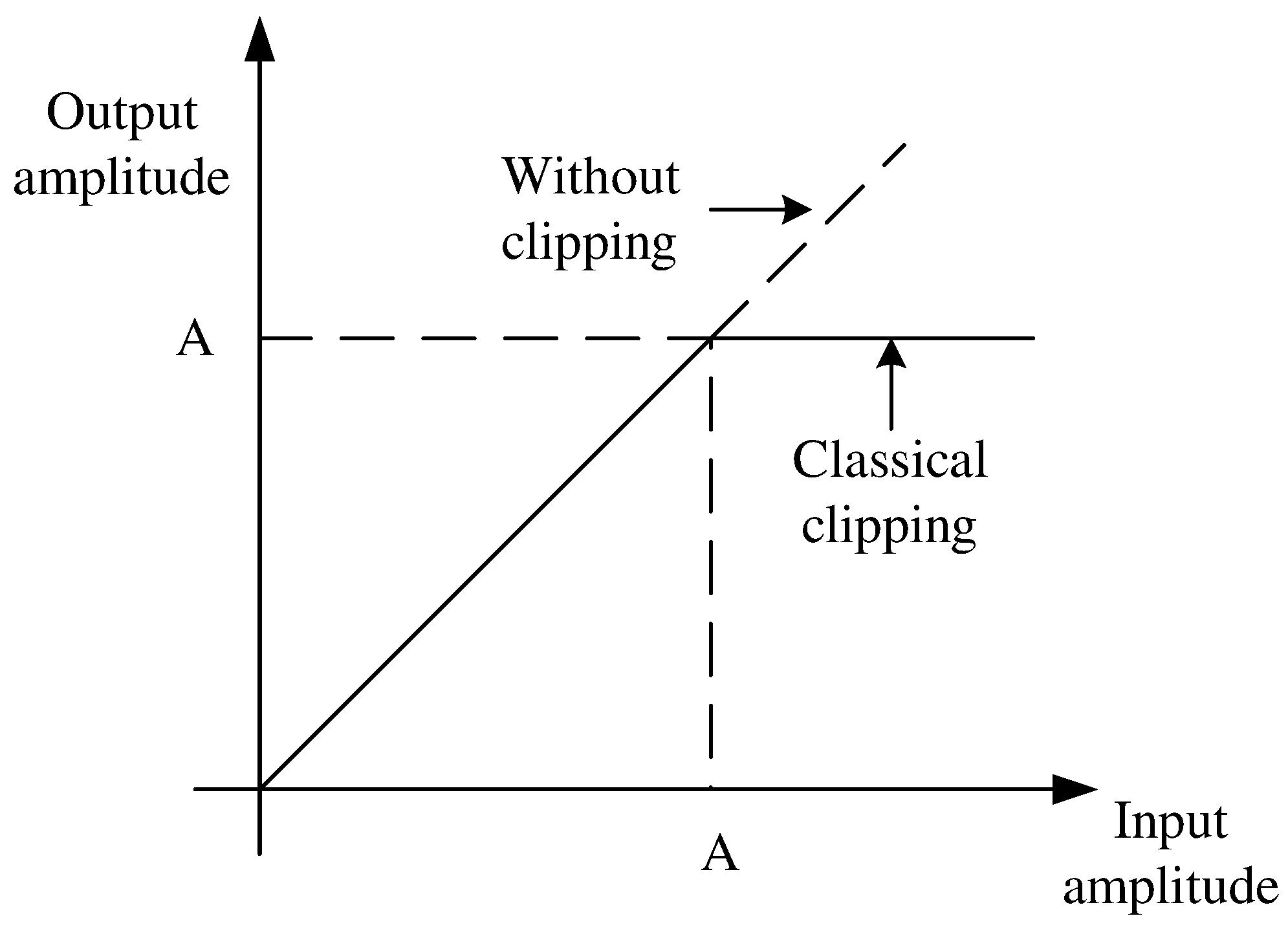
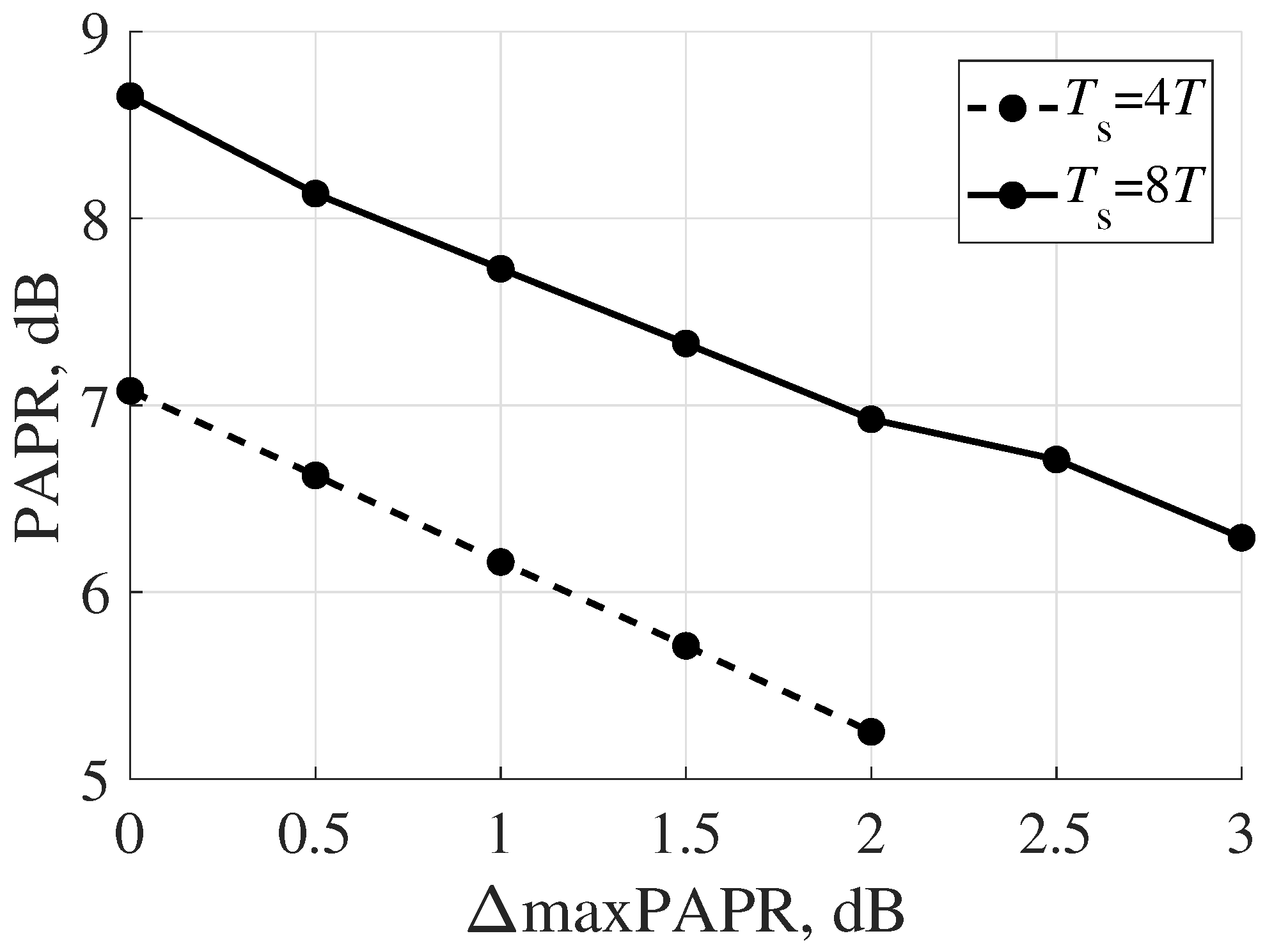
4.1. Occupied Frequency Band and PAPR of Oscillations
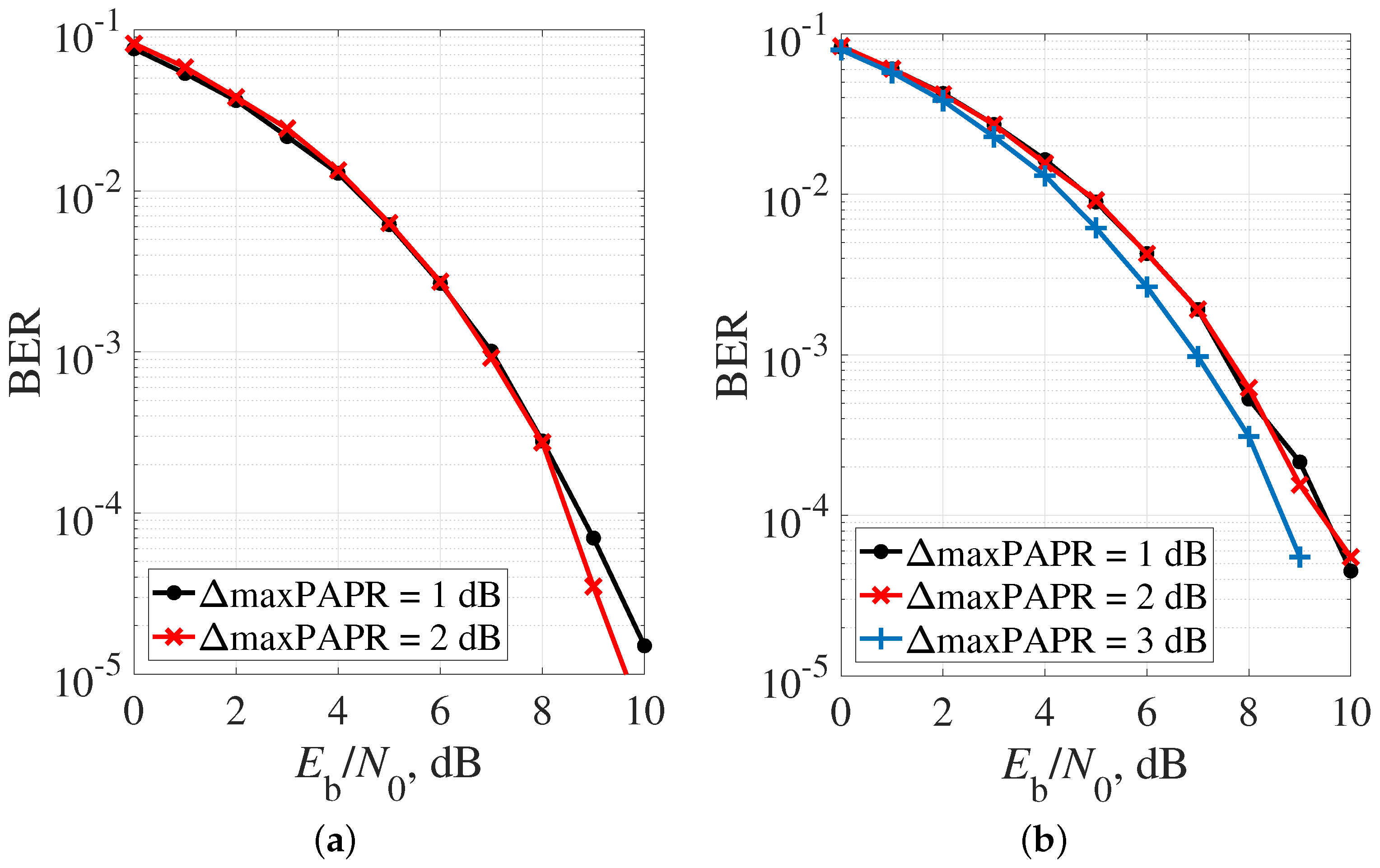
4.2. BER Performance in AWGN Channel
4.3. BER Performance in Rayleigh-Fading Channel
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Xing, Z.; Liu, K.; Tang, B.; Liu, Y. Novel PAPR Reduction Scheme Based on Piecewise Nonlinear Companding Transform in OFDM Systems. IEEE Commun. Lett. 2020, 24, 1757–1761. [Google Scholar] [CrossRef]
- Gao, S.; Zheng, J. Peak-to-Average Power Ratio Reduction in Pilot-Embedded OTFS Modulation Through Iterative Clipping and Filtering. IEEE Commun. Lett. 2020, 24, 2055–2059. [Google Scholar] [CrossRef]
- Ghosh, S.K. Travelling-Wave Tubes for Space Application: Present and Future. In Proceedings of the 2020 URSI Regional Conference on Radio Science ( URSI-RCRS), Varanasi, India, 12–14 February 2020; pp. 1–3. [Google Scholar] [CrossRef]
- Nguyen, K.T.; Pasour, J.; Vlasov, A.; Cooke, S.; Ludeking, L.; Levush, B.; Abe, D.K. US Naval Research Laboratory, Washington, DC, USA Oscillation characteristics in waveguide-based TWT amplifiers. In Proceedings of the 2015 IEEE International Vacuum Electronics Conference (IVEC), Beijing, China, 27–29 April 2015; pp. 1–2. [Google Scholar] [CrossRef]
- Smirnova, E.N.; Makarov, S.B.; Zavjalov, S.V.; Polozhintsev, B. Influence of the Amplitude Limitation of Signals with the sin(x)/x Envelope on the Spectral and Energy Characteristics. In Proceedings of the 2020 IEEE International Conference on Electrical Engineering and Photonics (EExPolytech), St. Petersburg, Russia, 15–16 October 2020; pp. 164–167. [Google Scholar] [CrossRef]
- Shahzad, A.; Xiong, N.; Irfan, M.; Lee, M.; Hussain, S.; Khaltar, B. A SCADA Intermediate Simulation Platform to Enhance the System Security. In Proceedings of the 2015 17th International Conference on Advanced Communication Technology (ICACT), Global IT Res Inst (GiRI), PyeonhChang, South Africa, 1–3 July 2015; pp. 368–373. [Google Scholar]
- Li, Q.; Gong, F.-K.; Song, P.-Y.; Li, G.; Zhai, S.-H. Beyond DVB-S2X: Faster-Than-Nyquist Signaling with Linear Precoding. IEEE Trans. Broadcast. 2020, 66, 620–629. [Google Scholar] [CrossRef]
- Makarov, S.B.; Liu, M.; Ovsyannikova, A.S.; Zavjalov, S.V.; Lavrenyuk, I.I.; Xue, W. Optimizing the Shape of Faster-Than-Nyquist (FTN) Signals With the Constraint on Energy Concentration in the Occupied Frequency Bandwidth. IEEE Access 2020, 8, 130082–130093. [Google Scholar] [CrossRef]
- Kwon, H.; Baek, M.; Yun, J.; Lim, H.; Hur, N. Design and performance evaluation of DVB-S2 system with FTN signaling. In Proceedings of the 2016 International Conference on Information and Communication Technology Convergence (ICTC), Jeju Island, Korea, 19–21October 2016; pp. 1210–1212. [Google Scholar] [CrossRef]
- Kim, P.; Oh, D. Design of a receiver faster than Nyquist signalling in DVB-S2 standard. In Proceedings of the 2014 International Conference on Information and Communication Technology Convergence (ICTC), Busan, Korea, 22–24 October 2014; pp. 989–990. [Google Scholar] [CrossRef]
- Hussain, S.; Kim, Y.C. Multilayer Communication Network Architecture for Wind Power Farm. In Proceedings of the 19th IEEE International Workshop on Computer Aided Modeling and Design of Communication Links and Networks (CAMAD), Athens, Greece, 1–3 December 2014; pp. 105–109. [Google Scholar]
- Gökceli, S.; Campo, P.P.; Levanen, T.; Yli-Kaakinen, J.; Turunen, M.; Allé, M. SDR Prototype for Clipped and Fast-Convolution Filtered OFDM for 5G New Radio Uplink. IEEE Access 2020, 8, 89946–89963. [Google Scholar] [CrossRef]
- Peng, S.; Liu, A.; Song, L.; Memon, I.; Wang, H. Spectral Efficiency Maximization for Deliberate Clipping-Based Multicarrier Faster-Than-Nyquist Signaling. IEEE Access 2018, 6, 13617–13623. [Google Scholar] [CrossRef]
- Zhao, J.; Ni, S.; Gong, Y. Peak-to-Average Power Ratio Reduction of FBMC/OQAM Signal Using a Joint Optimization Scheme. IEEE Access 2017, 5, 15810–15819. [Google Scholar] [CrossRef]
- Darwazeh, I.; Ghannam, H.; Xu, T. The First 15 Years of SEFDM: A Brief Survey. In Proceedings of the 2018 11th International Symposium on Communication Systems, Networks & Digital Signal Processing (CSNDSP), Budapest, Hungary, 18–20 July 2018; pp. 1–7. [Google Scholar] [CrossRef]
- Said, A.; Anderson, J.B. Bandwidth-efficient coded modulation with optimized linear partial-response signals. IEEE Trans. Inf. Theory 1998, 44, 701–713. [Google Scholar] [CrossRef]
- Snyman, J.A.; Wilke, D.N. Practical Mathematical Optimization: Basic Optimization; Springer: Berlin, Germany, 2018. [Google Scholar]
- Anderson, J.B.; Rusek, F.; Owall, V. Faster-Than-Nyquist Signaling. Proc. IEEE 2013, 101, 1817–1830. [Google Scholar] [CrossRef]
- Liu, M.; Xue, W.; Makarov, S.B.; Qi, J.; Li, B. Comparative Study of Analytic Solution and Numerical Solution of Baseband Symbol Signal Based on Optimal Generic Function. Math. Probl. Eng. 2019, 8045217. [Google Scholar] [CrossRef]
- Zavjalov, S.V.; Ovsyannikova, A.S.; Volvenko, S.V. On the Necessary Accuracy of Representation of Optimal Signals. Lect. Notes Comput. Sci. 2018, 11118 LNCS, 153–161. [Google Scholar] [CrossRef]
- Waldman, D.G.; Makarov, S.B. Synthesis of spectral-effective modulation techniques for digital communication systems. In Proceedings of the ICCSC’02. 1st IEEE International Conference on Circuits and Systems for Communications. Proceedings (IEEE Cat. No.02EX605), St. Petersburg, Russia, 26–28 June 2002; pp. 432–435. [Google Scholar] [CrossRef]
- Shang, W.; Xu, Y.; Qi, J.; Xue, W.; Makarov, S.B. Optimal waveform of the partial-respond signal based on minimum out-of-band radiation criterion. Appl. Sci. 2017, 7, 1086. [Google Scholar] [CrossRef]
- Liu, M.; Xue, W.; Jia, P.; Makarov, S.B.; Li, B. Research on spectrum optimization technology for a wireless communication system. Symmetry 2020, 12, 34. [Google Scholar] [CrossRef]
- Mason, S.J.; Zimmermann, H.J. Electronic Circuits, Signals and Systems; Wiley: New York, NY, USA, 1960. [Google Scholar]
- Feher, K. Wireless Digital Communications: Modulation And Spread Spectrum Applications; Prentice-Hall Of India Pvt. Limited: New Delhi, India, 1995. [Google Scholar]
- Anderson, J.B. Bandwidth Efficient Coding; John Wiley Sons: Hoboken, NJ, USA, 2017. [Google Scholar]






| maxPAPR, dB | ||
| 0 | 1.12 | 0.99 |
| 1 | 1.18 | 1.06 |
| 2 | 1.27 | 1.17 |
| 3 | - | 1.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Makarov, S.B.; Liu, M.; Ovsyannikova, A.S.; Zavjalov, S.V.; Lavrenyuk, I.; Xue, W.; Xu, Y. A Reduction of Peak-to-Average Power Ratio Based Faster-Than-Nyquist Quadrature Signals for Satellite Communication. Symmetry 2021, 13, 346. https://doi.org/10.3390/sym13020346
Makarov SB, Liu M, Ovsyannikova AS, Zavjalov SV, Lavrenyuk I, Xue W, Xu Y. A Reduction of Peak-to-Average Power Ratio Based Faster-Than-Nyquist Quadrature Signals for Satellite Communication. Symmetry. 2021; 13(2):346. https://doi.org/10.3390/sym13020346
Chicago/Turabian StyleMakarov, Sergey B., Mingxin Liu, Anna S. Ovsyannikova, Sergey V. Zavjalov, ILya Lavrenyuk, Wei Xue, and Yidong Xu. 2021. "A Reduction of Peak-to-Average Power Ratio Based Faster-Than-Nyquist Quadrature Signals for Satellite Communication" Symmetry 13, no. 2: 346. https://doi.org/10.3390/sym13020346
APA StyleMakarov, S. B., Liu, M., Ovsyannikova, A. S., Zavjalov, S. V., Lavrenyuk, I., Xue, W., & Xu, Y. (2021). A Reduction of Peak-to-Average Power Ratio Based Faster-Than-Nyquist Quadrature Signals for Satellite Communication. Symmetry, 13(2), 346. https://doi.org/10.3390/sym13020346









