Evaluation of Online Teaching Quality: An Extended Linguistic MAGDM Framework Based on Risk Preferences and Unknown Weight Information
Abstract
1. Introduction
- Inspired by the decision method in Lin and Wang [25], this framework extends the linguistic MAGDM method involving risk preferences from the single-level EIS to the multi-level one.
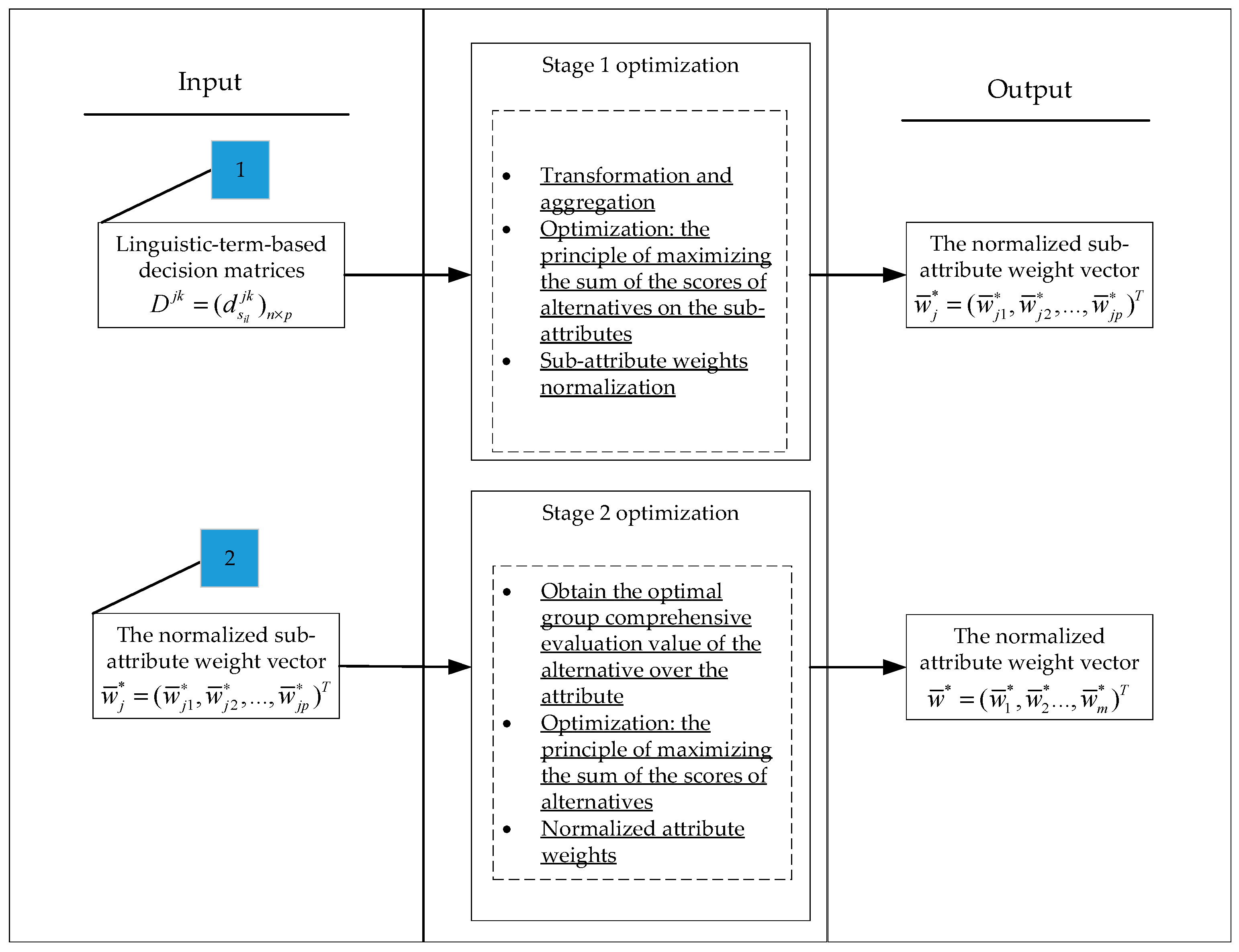
- In teaching quality evaluation problems based on multi-level EISs, many works of literature adopted the AHP approach to derive the weights of attributes and sub-attributes [8,9,10,11,12,13]. Sometimes AEs cannot make pairwise comparisons on the importance of attributes or sub-attributes, that is, they cannot use the AHP method to calculate attribute weights. Thus, based on the principle of maximizing the group comprehensive evaluation value of each alternative, a two-stage attribute and sub-attribute weight solution optimization model is established in this framework.
- The evaluation ranking results obtained by this framework are divided into the overall ranking result and partial ranking results, which are helpful for teachers and universities to understand their own situation and make targeted improvements.
2. Preliminaries
2.1. Linguistic Term Set
- The LTSis ordered:if and only if;
- There is a negation operator:where.
2.2. Group Generalized Linguistic Term Set
2.2.1. Euclidean Distance
2.2.2. Score Function
2.2.3. Group Generalized Linguistic Term Set Based on Risk Preferences
2.2.4. Determination of Risk Preference Parameters
3. EIS of Online Teaching Quality
3.1. Selection of Indicators for Evaluating the Online Teaching Quality
3.2. Determination of the Weights of Evaluation Indicators
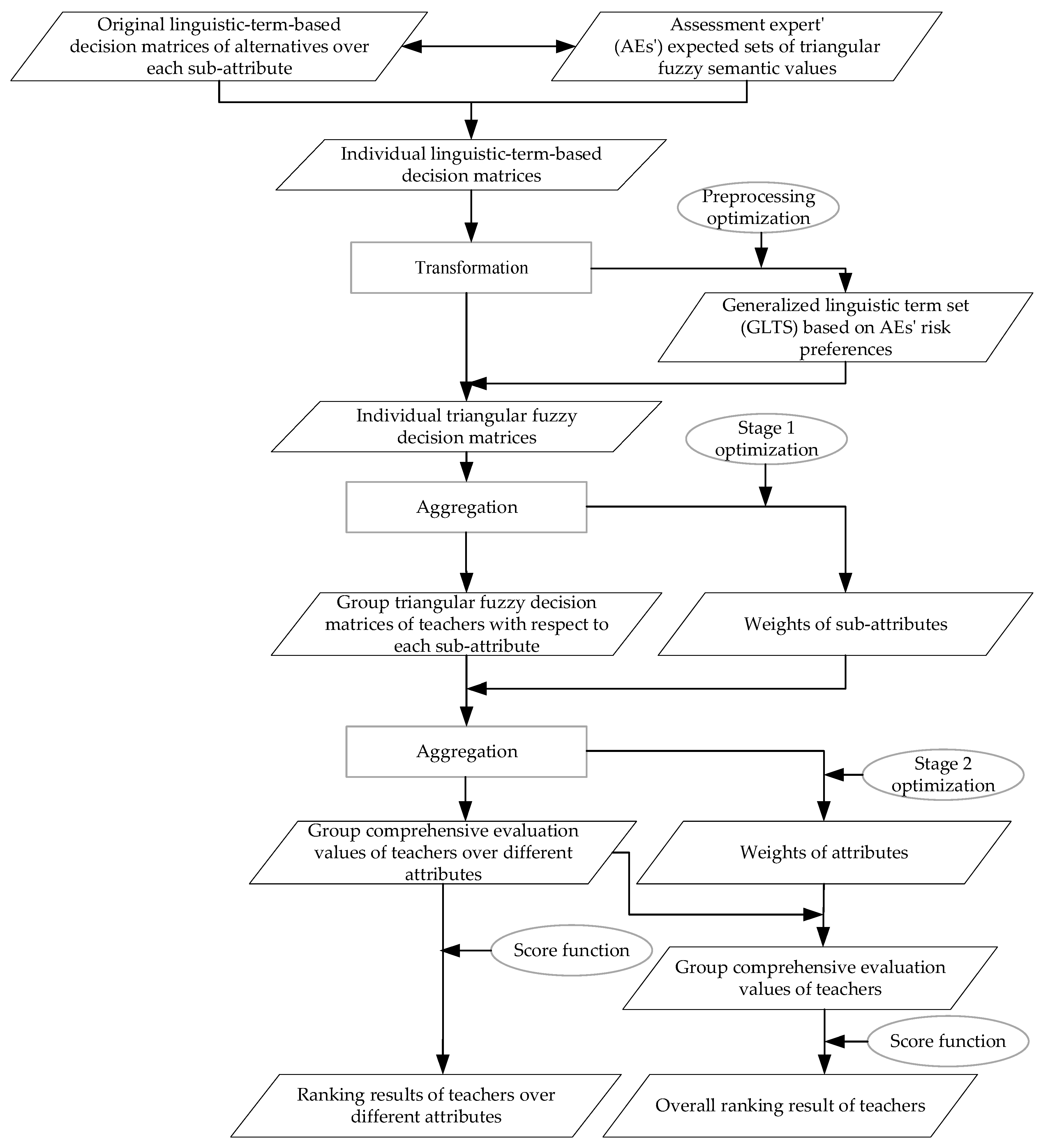
4. A Method for Linguistic MAGDM Involving Risk Preferences and Completely Unknown Weight Information
5. A Case Study about Online Teaching Quality Evaluation
5.1. Problem Description
5.2. Evaluation of Online Teaching Quality among Four Teachers
6. Practical Discussion
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Huang, R.; Liu, D.; Zhan, T. Guidance on Flexible Learning during Campus Closures: Ensuring Course Quality of Higher Education in COVID-19 Outbreak; Beijing Smart Learning Institute of Beijing Normal University: Beijing, China, 2020. [Google Scholar]
- Wang, X. Online Teaching Launched on a Massive Scale, this Year may Usher in the First Year of a New Wave of Change in Higher Education. Available online: http://www.whb.cn/zhuzhan/xue/20200521/349052.html (accessed on 21 May 2020).
- Zhang, X.; Wang, J.; Zhang, H.; Hu, J. A Heterogeneous Linguistic MAGDM Framework to Classroom Teaching Quality Evaluation. Eurasia J. Math. Sci. Technol. Educ. 2017, 13, 4929–4956. [Google Scholar] [CrossRef]
- Song, J.; Zheng, J.H. The Application of Grey-TOPSIS Method on Teaching Quality Evaluation of the Higher Education. Int. J. Emerg. Technol. Learn. 2015, 10, 42–45. [Google Scholar] [CrossRef]
- Ma, H.P. Research on Fuzzy Comprehensive Evaluation of Teaching Quality of College Public Physical Education based on Grey System Theory. Agro Food Ind. Hi-Tech 2017, 28, 2603–2607. [Google Scholar]
- Faizi, S.; Sałabun, W.; Rashid, T.; Watrobski, J.; Zafar, S. Group decision-making for hesitant fuzzy sets based on Characteristic Objects Method. Symmetry 2017, 9, 136. [Google Scholar] [CrossRef]
- Faizi, S.; Sałabun, W.; Rashid, T.; Zafar, S.; Watrobski, J. Intuitionistic fuzzy sets in multi-criteria group decision making problems using the Characteristic Objects Method. Symmetry 2020, 12, 1382. [Google Scholar] [CrossRef]
- Chen, J.F.; Hsieh, H.N.; Do, Q.H. Evaluating teaching performance based on fuzzy AHP and comprehensive evaluation approach. Appl. Soft. Comput. 2015, 28, 100–108. [Google Scholar] [CrossRef]
- Chang, T.C.; Wang, H. A Multi Criteria Group Decision-making Model for Teacher Evaluation in Higher Education Based on Cloud Model and Decision Tree. Eurasia J. Math. Sci. Technol. Educ. 2016, 12, 1243–1262. [Google Scholar] [CrossRef]
- Thanassoulis, E.; Dey, P.K.; Petridis, K.; Goniadis, I.; Georgiou, A.C. Evaluating higher education teaching performance using combined analytic hierarchy process and data envelopment analysis. J. Oper. Res. Soc. 2017, 68, 431–445. [Google Scholar] [CrossRef]
- Wang, Z.H. Fuzzy comprehensive evaluation of physical education based on high dimensional data mining. J. Intell. Fuzzy Syst. 2018, 35, 3065–3076. [Google Scholar] [CrossRef]
- Shen, L.Q.; Yang, J.; Jin, X.Y.; Hou, L.Y.; Shang, S.M.; Zhang, Y. Based on Delphi method and Analytic Hierarchy Process to construct the Evaluation Index system of nursing simulation teaching quality. Nurse Educ. Today 2019, 79, 67–73. [Google Scholar] [CrossRef]
- Yang, J.; Shen, L.Q.; Jin, X.Y.; Hou, L.Y.; Shang, S.M.; Zhang, Y. Evaluating the quality of simulation teaching in Fundamental Nursing Curriculum: AHP-Fuzzy comprehensive evaluation. Nurse Educ. Today 2019, 77, 77–82. [Google Scholar] [CrossRef] [PubMed]
- Kong, H.N.; Fan, H.J.; Zhao, Y.; Zhai, C.J.; Zhang, C.; Han, Y.J. Design of teaching quality evaluation model based on fuzzy mathematics and SVM algorithm. J. Intell. Fuzzy Syst. 2018, 35, 3091–3099. [Google Scholar]
- Peng, X.D.; Dai, J.G. Research on the assessment of classroom teaching quality with q-rung orthopair fuzzy information based on multiparametric similarity measure and combinative distance-based assessment. Int. J. Intell. Syst. 2019, 34, 1588–1630. [Google Scholar] [CrossRef]
- Herrera, F.; Martinez, L. A 2-tuple fuzzy linguistic representation model for computing with words. IEEE Trans. Fuzzy Syst. 2000, 8, 746–752. [Google Scholar]
- Wang, J.H.; Hao, J.Y. A new version of 2-tuple. fuzzy linguistic, representation model for computing with words. IEEE Trans. Fuzzy Syst. 2006, 14, 435–445. [Google Scholar] [CrossRef]
- Dong, Y.C.; Xu, Y.F.; Yu, S. Computing the Numerical Scale of the Linguistic Term Set for the 2-Tuple Fuzzy Linguistic Representation Model. IEEE Trans. Fuzzy Syst. 2009, 17, 1366–1378. [Google Scholar] [CrossRef]
- Rodriguez, R.M.; Martinez, L.; Herrera, F. Hesitant Fuzzy Linguistic Term Sets for Decision Making. IEEE Trans. Fuzzy Syst. 2012, 20, 109–119. [Google Scholar] [CrossRef]
- Dong, Y.C.; Li, C.C.; Herrera, F. Connecting the linguistic hierarchy and the numerical scale for the 2-tuple linguistic model and its use to deal with hesitant unbalanced linguistic information. Inf. Sci. 2016, 367, 259–278. [Google Scholar] [CrossRef]
- Li, C.C.; Dong, Y.C.; Herrera, F.; Herrera-Viedma, E.; Martinez, L. Personalized individual semantics in computing with words for supporting linguistic group decision making. An application on consensus reaching. Inf. Fusion 2017, 33, 29–40. [Google Scholar] [CrossRef]
- Liu, Y.Y.; Rodriguez, R.M.; Hagras, H.; Liu, H.B.; Qin, K.Y.; Martinez, L. Type-2 Fuzzy Envelope of Hesitant Fuzzy Linguistic Term Set: A New Representation Model of Comparative Linguistic Expression. IEEE Trans. Fuzzy Syst. 2019, 27, 2312–2326. [Google Scholar] [CrossRef]
- Lin, H.; Wang, Z.J. Linguistic multi-attribute decision making with considering decision makers’ risk preferences. J. Intell. Fuzzy Syst. 2017, 33, 1775–1784. [Google Scholar] [CrossRef]
- Zhou, W.; Xu, Z.S. Generalized asymmetric linguistic term set and its application to qualitative decision making involving risk appetites. Eur. J. Oper. Res. 2016, 254, 610–621. [Google Scholar] [CrossRef]
- Lin, H.; Wang, Z.J. Linguistic Multi-Attribute Group Decision Making with Risk Preferences and Its Use in Low-Carbon Tourism Destination Selection. Int. J. Environ. Res. Public Health 2017, 14, 1078. [Google Scholar] [CrossRef] [PubMed]
- Guo, Z.X.; Sun, F.F. Multi-attribute decision making method based on single-valued neutrosophic linguistic variables and prospect theory. J. Intell. Fuzzy Syst. 2019, 37, 5351–5362. [Google Scholar] [CrossRef]
- Ma, Z.Z.; Zhu, J.J.; Zhang, S.T. Probabilistic-based expressions in behavioral multi-attribute decision making considering pre-evaluation. Fuzzy Optim. Decis. Mak. 2020. [Google Scholar] [CrossRef]
- Liao, Z.Q.; Liao, H.C.; Tang, M.; Al-Barakati, A.; Llopis-Albert, C. A Choquet integral-based hesitant fuzzy gained and lost dominance score method for multi-criteria group decision making considering the risk preferences of experts: Case study of higher business education evaluation. Inf. Fusion 2020, 62, 121–133. [Google Scholar] [CrossRef]
- Bashir, Z.; Rashid, T.; Watrobski, J.; Sałabun, W.; Malik, A. Hesitant probabilistic multiplicative preference relations in group decision making. Appl. Sci. 2018, 8, 398. [Google Scholar] [CrossRef]
- Jin, F.; Garg, H.; Pei, L.; Liu, J.; Chen, H. Multiplicative consistency adjustment model and data envelopment analysis-driven decision-making process with probabilistic hesitant fuzzy preference relations. Int. J. Fuzzy Syst. 2020, 22, 2319–2332. [Google Scholar] [CrossRef]
- Gong, Y.B. Fuzzy Multi-Attribute Group Decision Making Method Based on Interval Type-2 Fuzzy Sets and Applications to Global Supplier Selection. Int. J. Fuzzy Syst. 2013, 15, 392–400. [Google Scholar]
- Gupta, P.; Mehlawat, M.K.; Grover, N. Intuitionistic fuzzy multi-attribute group decision-making with an application to plant location selection based on a new extended VIKOR method. Inf. Sci. 2016, 370, 184–203. [Google Scholar] [CrossRef]
- Wang, W.; Mendel, J.M. Multiple attribute group decision making with linguistic variables and complete unknown weight information. Iran J. Fuzzy Syst. 2019, 16, 145–157. [Google Scholar] [CrossRef]
- Touqeer, M.; Hafeez, A.; Arshad, M. Multi-attribute decision making using grey relational projection method based on interval type-2 trapezoidal fuzzy numbers. J. Intell. Fuzzy Syst. 2020, 38, 5979–5986. [Google Scholar] [CrossRef]
- Xu, Z.S. Deviation measures of linguistic preference relations in group decision making. Omega Int. J. Manag. Sci. 2005, 33, 249–254. [Google Scholar] [CrossRef]
- Chen, C.T. Extensions of the TOPSIS for group decision-making under fuzzy environment. Fuzzy Sets Syst. 2000, 114, 1–9. [Google Scholar] [CrossRef]
- Liou, T.S.; Wang, M.J.J. Ranking Fuzzy Numbers with Integral Value. Fuzzy Sets Syst. 1992, 50, 247–255. [Google Scholar] [CrossRef]
- Sałabun, W.; Watrobski, J.; Shekhovtsov, A. Are MCDA methods benchmarkable? A comparative study of TOPSIS, VIKOR, COPRAS, and PROMETHEE II methods. Symmetry 2020, 12, 1549. [Google Scholar] [CrossRef]
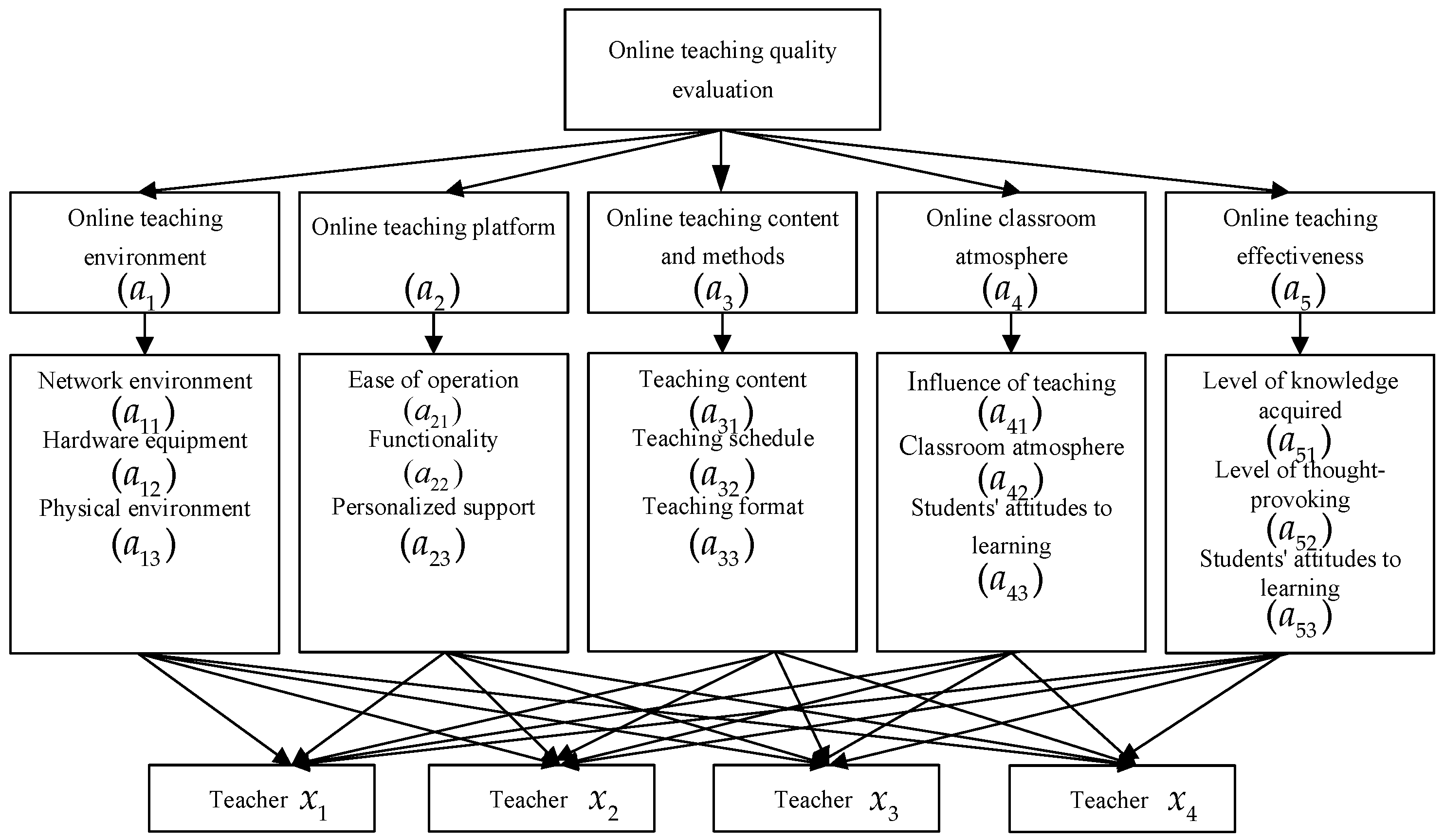
| Attribute | Sub-Attribute |
|---|---|
| Online teaching environment () | Network environment () |
| Hardware equipment () | |
| Physical environment () | |
| Online teaching platform () | Ease of operation () |
| Functionality () | |
| Personalized support () | |
| Online teaching content and methods () | Teaching content () |
| Teaching schedule () | |
| Teaching format () | |
| Online classroom atmosphere () | Influence of teaching () |
| Classroom atmosphere () | |
| Students’ attitudes to learning () | |
| Online teaching effectiveness () | Level of knowledge acquired () |
| Level of thought-provoking () | |
| Problem-solving skills () |
| Attribute | Ranking |
|---|---|
| Online teaching environment | |
| Online teaching platform | |
| Online teaching content and methods | |
| Online classroom atmosphere | |
| Online teaching effectiveness |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.; You, J.; Xu, T. Evaluation of Online Teaching Quality: An Extended Linguistic MAGDM Framework Based on Risk Preferences and Unknown Weight Information. Symmetry 2021, 13, 192. https://doi.org/10.3390/sym13020192
Lin H, You J, Xu T. Evaluation of Online Teaching Quality: An Extended Linguistic MAGDM Framework Based on Risk Preferences and Unknown Weight Information. Symmetry. 2021; 13(2):192. https://doi.org/10.3390/sym13020192
Chicago/Turabian StyleLin, Hui, Jianxin You, and Tao Xu. 2021. "Evaluation of Online Teaching Quality: An Extended Linguistic MAGDM Framework Based on Risk Preferences and Unknown Weight Information" Symmetry 13, no. 2: 192. https://doi.org/10.3390/sym13020192
APA StyleLin, H., You, J., & Xu, T. (2021). Evaluation of Online Teaching Quality: An Extended Linguistic MAGDM Framework Based on Risk Preferences and Unknown Weight Information. Symmetry, 13(2), 192. https://doi.org/10.3390/sym13020192






