Effects of Energy Dissipation and Deformation Function on the Entanglement, Photon Statistics and Quantum Fisher Information of Three-Level Atom in Photon-Added Coherent States for Morse Potential
Abstract
:1. Introduction
2. 3LA System and Its Dynamics
3. The Atomic Population
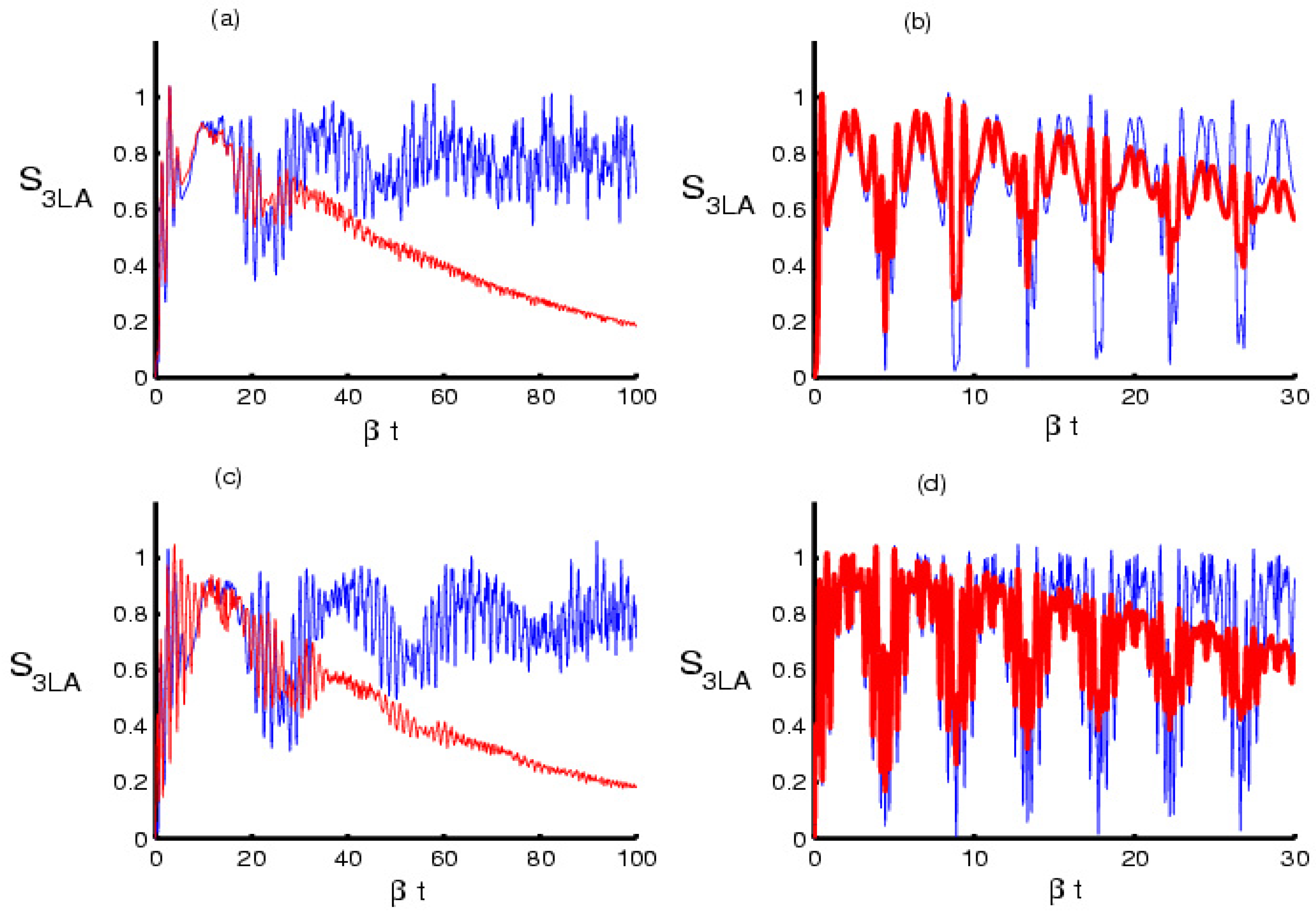
4. The Degree of Entanglement
5. Quantum Fisher Information Dynamics
6. Photon Statistics and Mandel Parameter
7. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Morse, P.M. Diatomic Molecules According to the Wave Mechanics. II. Vibrational Levels. Phys. Rev. 1929, 34, 57–64. [Google Scholar] [CrossRef]
- Sage, M.L. Energetics, wave functions, and spectroscopy of coupled anharmonic oscillators. Chem. Phys. 1978, 35, 375. [Google Scholar] [CrossRef]
- Popov, D.; Zaharie, I.; Dong, S.-H. Photon-added coherent states for the Morse oscillator. Czechoslov. J. Phys. 2006, 56, 157–176. [Google Scholar] [CrossRef]
- Vasan, V.S.; Cross, R.J. Matrix elements for Morse oscillators. J. Chem. Phys. 1983, 78, 3869–3871. [Google Scholar] [CrossRef]
- Tipping, R.H.; Ogilvie, J.F. Expectation values for Morse oscillators. J. Chem. Phys. 1983, 79, 2537–2540. [Google Scholar] [CrossRef]
- Iachello, F.; Oss, S. Flux dynamics and the growth of the superconducting phase. Phys. Rev. Lett. 1991, 66, 2946. [Google Scholar]
- Nieto, M.M.; Simmons, L.M. Eigenstates, coherent states, and uncertainty products for the Morse oscillator. Phys. Rev. A 1979, 19, 438–444. [Google Scholar] [CrossRef]
- Nieto, M.M. Coherent states for general potentials. V. Time evolution. Phys. Rev. D 1980, 22, 403. [Google Scholar] [CrossRef]
- Nieto, M.M.; Simmons, L.M. Coherent states for general potentials. I. Formalism. Phys. Rev. D 1979, 20, 1321. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and Incoherent States of the Radiation Field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Gerry, C.C. Coherent states and a path integral for the Morse oscillator. Phys. Rev. A 1986, 33, 2207–2211. [Google Scholar] [CrossRef]
- Drag, G.E.; Avram, N.M. Creation and annihilation operators for the Morse oscillator and the coherent states. Can. J. Phys. 1998, 76, 273. [Google Scholar]
- Dong, S.-H. The SU(2) realization for the Morse potential and its coherent states. Can. J. Phys. 2002, 80, 129–139. [Google Scholar] [CrossRef]
- Quesne, C. Deformed Shape Invariant Superpotentials in Quantum Mechanics and Expansions in Powers of ℏ. Symmetry 2020, 12, 1853. [Google Scholar] [CrossRef]
- Belfakir, A.; Hassouni, Y.; Curado, E.M.F. Construction of coherent states for Morse potential: A su(2)-like approach. Phys. Lett. A 2020, 384, 126553. [Google Scholar] [CrossRef]
- Angelova, M.N.; Hussin, V. Squeezed coherent states and the one-dimensional Morse quantum system. J. Phys. A Math. Theor. 2012, 45, 244007. [Google Scholar] [CrossRef] [Green Version]
- Alqannas, H.S.; Abdel-Khalek, S. Physical and nonclassical properties of the interaction between a four-level atom and field in coherent state of Morse potential. Opt. Quantum Electron. 2019, 51, 50. [Google Scholar] [CrossRef]
- Alsahhaf, M.A.; Aldaghfag, S.A.; Abdel-Khalek, S. Physical Properties, Field Purity, and Quantum Phase for a Two-Level Atom in Photon-Added Coherent States for the Morse Potential. J. Russ. Laser Res. 2017, 38, 437–445. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Yoshihisa, Y.; Kouichi, S. Principles and Methods of Quantum Information Technologies: Lecture Notes in Physics; Springer: Tokyo, Japan, 2016. [Google Scholar]
- Alber, G.; Beth, T.; Horodecki, M.; Horodecki, P.; Horodecki, R.; Rtteler, M.; Weinfurter, H.; Zeilinger, A. Quantum Information; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Gerry, C.; Knight, P. Introductory Quantum Optics; Cambridge University Press (CUP): Cambridge, UK, 2004. [Google Scholar]
- Bigelow, N.P.; Eberly, J.H.; Stroud, C.R., Jr.; AWalmsley, I. Introductory Quantum Optics. In Proceedings of the Eighth Rochester Conference on Coherence and Quantum Optics, Rochester, NY, USA, 13–16 June 2001. [Google Scholar]
- de Faria, J.G.P.; Nemes, M.C. Dissipative dynamics of the Jaynes-Cummings model in the dispersive approximation: Analytical results. Phys. Rev. A 1999, 59, 3918–3925. [Google Scholar] [CrossRef]
- Berrada, K.; Abdel-Khalek, S.; Ooi, R. Quantum metrology with entangled spin-coherent states of two modes. Phys. Rev. A 2012, 86, 033823. [Google Scholar] [CrossRef] [Green Version]
- Pezzè, L.; Smerzi, A.; Oberthaler, M.K.; Schmied, R.; Treutlein, P. Quantum metrology with nonclassical states of atomic ensembles. Rev. Mod. Phys. 2018, 90, 035005. [Google Scholar] [CrossRef] [Green Version]
- Einstein, A.; Podolsky, B.; Rosen, N. Can Quantum-Mechanical Description of Physical Reality Be Considered Complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef] [Green Version]
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865. [Google Scholar] [CrossRef] [Green Version]
- Joo, J.; Munro, W.J.; Spiller, T.P. Quantum Metrology with Entangled Coherent States. Phys. Rev. Lett. 2011, 107, 083601. [Google Scholar] [CrossRef]
- Marin, M. A domain of influence theorem for microstretch elastic materials. Nonlinear Anal. Real World Appl. 2010, 11, 3446–3452. [Google Scholar] [CrossRef]
- Marin, M.; Agarwal, R.P.; Mahmoud, S.R. Modeling a microstretch thermo-elastic body with two temperatures. Abstr. Appl. Anal. 2013, 2013, 583464. [Google Scholar] [CrossRef] [Green Version]
- Abbas, I.A.; Marin, M. Analytical Solutions of a Two-Dimensional Generalized Thermoelastic Diffusions Problem Due to Laser Pulse. Iran. J. Sci. Technol. Trans. Mech. Eng. 2018, 42, 57–71. [Google Scholar] [CrossRef]
- Marin, M.; Othman, M.I.A.; Seadawy, A.R.; Carstea, C. A domain of influence in the Moore–Gibson–Thompson theory of dipolar bodies. J. Taibah. Univ. Sci. 2020, 14, 653–660. [Google Scholar] [CrossRef]
- Phoenix, S.J.D.; Knight, P.L. Establishment of an entangled atom-field state in the Jaynes-Cummings model. Phys. Rev. A 1991, 44, 6023–6029. [Google Scholar] [CrossRef]
- Abdel-Aty, M. Quantum field entropy and entanglement of a three-level atom two-mode system with an arbitrary nonlinear medium. J. Mod. Opt. 2003, 50, 161–177. [Google Scholar] [CrossRef]
- Jamal Anwar, S.; Ramzan, M.M. Usman and M. Khalid Khan Entanglement Dynamics of Three and Four Level Atomic System under Stark Effect and Kerr-Like Medium. Quantum Rep. 2019, 1, 23–36. [Google Scholar] [CrossRef] [Green Version]
- Abdalla, M.S.; Khalil, E.; Obada, A.S.-F.; Peřina, J.; Křepelka, J. Quantum statistical characteristics of the interaction between two two-level atoms and radiation field. Eur. Phys. J. Plus 2015, 130, 1–19. [Google Scholar] [CrossRef]
- Obada, A.-S.F.; Khalil, E.M.; Ahmed, M. Generation of a nonlinear two-mode Stark shift through the adiabatic elimination method. J. Mod. Opt. 2006, 53, 1149–1163. [Google Scholar] [CrossRef]
- Popescu, S.; Rohrlich, D. Thermodynamics and the measure of entanglement. Phys. Rev. A 1997, 56, R3319–R3321. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H.; Brassard, G. WITHDRAWN: Quantum cryptography: Public key distribution and coin tossing. Theor. Comput. Sci. 2011, 175, 8. [Google Scholar] [CrossRef]
- Kak, S. A Three-Stage Quantum Cryptography Protocol. Found. Phys. Lett. 2006, 19, 293–296. [Google Scholar] [CrossRef] [Green Version]
- Abdalla, M.S.; Khalil, E.; Obada, A.-F. Quantum effect of the Kerr-like medium in terms of SU(1,1) Lie group in interaction with a two-level atom. Phys. A Stat. Mech. Its Appl. 2017, 466, 44–56. [Google Scholar] [CrossRef] [Green Version]
- Amari, S.-I.; Nagaoka, H. Methods of Information Geometry; American Mathematical Society: Providence, RI, USA, 2007; Volume 191. [Google Scholar]
- Abdel-Khalek, S. Quantum Fisher information flow and entanglement in pair coherent states. Opt. Quantum Electron. 2014, 46, 1055–1064. [Google Scholar] [CrossRef]
- Abu-Zinadah, H.H.; Abdel-Khalek, S. Fisher information and quantum state estimation of two-coupled atoms in presence of two external magnetic fields. Results Phys. 2017, 7, 4318–4323. [Google Scholar] [CrossRef]
- Barndorff-Nielsen, O.E.; Gill, R.D.; Jupp, P. On quantum statistical inference. J. R. Stat. Soc. Ser. B Stat. Methodol 2003, 65, 775–804. [Google Scholar] [CrossRef] [Green Version]
- Mandel, L. Sub-Poissonian photon statistics in resonance fluorescence. Opt. Lett. 1979, 4, 205–207. [Google Scholar] [CrossRef] [PubMed]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdel-Khalek, S.; Khalil, E.M.; Alotaibi, H.; Abo-Dahab, S.M.; Mahmoud, E.E.; Higazy, M.; Marin, M. Effects of Energy Dissipation and Deformation Function on the Entanglement, Photon Statistics and Quantum Fisher Information of Three-Level Atom in Photon-Added Coherent States for Morse Potential. Symmetry 2021, 13, 2188. https://doi.org/10.3390/sym13112188
Abdel-Khalek S, Khalil EM, Alotaibi H, Abo-Dahab SM, Mahmoud EE, Higazy M, Marin M. Effects of Energy Dissipation and Deformation Function on the Entanglement, Photon Statistics and Quantum Fisher Information of Three-Level Atom in Photon-Added Coherent States for Morse Potential. Symmetry. 2021; 13(11):2188. https://doi.org/10.3390/sym13112188
Chicago/Turabian StyleAbdel-Khalek, Sayed, Eied M. Khalil, Hammad Alotaibi, Sayed M. Abo-Dahab, Emad E. Mahmoud, Mahmoud Higazy, and Marin Marin. 2021. "Effects of Energy Dissipation and Deformation Function on the Entanglement, Photon Statistics and Quantum Fisher Information of Three-Level Atom in Photon-Added Coherent States for Morse Potential" Symmetry 13, no. 11: 2188. https://doi.org/10.3390/sym13112188
APA StyleAbdel-Khalek, S., Khalil, E. M., Alotaibi, H., Abo-Dahab, S. M., Mahmoud, E. E., Higazy, M., & Marin, M. (2021). Effects of Energy Dissipation and Deformation Function on the Entanglement, Photon Statistics and Quantum Fisher Information of Three-Level Atom in Photon-Added Coherent States for Morse Potential. Symmetry, 13(11), 2188. https://doi.org/10.3390/sym13112188








