Modern State of the Pauli Exclusion Principle and the Problems of Its Theoretical Foundation
Abstract
1. Introduction
“An antisymmetric eigenfunction vanishes identically when two of the electrons are in the same orbit. This means that in the solution of the problem with antisymmetric eigenfunctions there can be no stationary states with two or more electrons in the same orbit, which is just Pauli’s exclusion principle.”
“The only possible states of a system of identical particles possessing spin s are those for which the total wave function transforms upon interchange of any two particles asThat is, it is symmetric for integer values of s (the Bose-Einstein statistics) and antisymmetric for half-integer values of s (the Fermi-Dirac statistics)”.
2. Spin-Statistics Connection
“Already in my initial paper, I especially emphasized the fact that I could not find a logical substantiation for the exclusion principle nor derive it from more general assumptions. I always had a feeling, which remains until this day, that this is the fault of some flaw in the theory.”
“Why is it that particles with half-integral spin are Fermi particles whose amplitudes add with the minus sign, whereas particles with integral spin are Bose particles whose amplitudes add with the positive sign? We apologize for the fact that we cannot give you an elementary explanation… It appears to be one of the few places in physics where there is a rule which can be stated very simply, but for which no one found a simple and easy explanation. The explanation is deep down in relativistic quantum mechanics”.
3. Theoretical Foundations of PEP
3.1. Indistinguishability of Identical Particles and the Symmetrization Postulate
“whether the PEP limitation on the solution of the Schrödinger equation follows from the fundamental principles of quantum mechanics or it is an independent principle?”
“The exclusion principle could not be deduced from the new quantum mechanics but remains an independent principle which excludes a class of mathematically possible solutions of the wave equation. This excess of mathematical possibilities of the present-day theory, as compared with reality, is indications that in the region where it touches on relativity, quantum theory has not yet found its final form.”
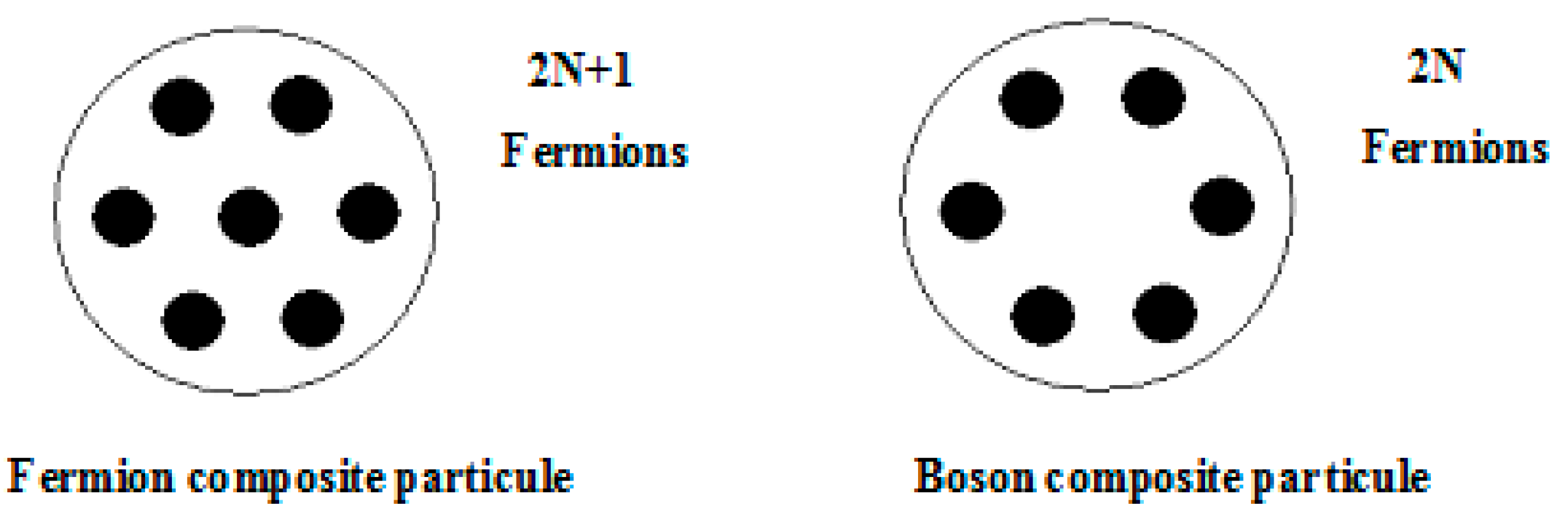
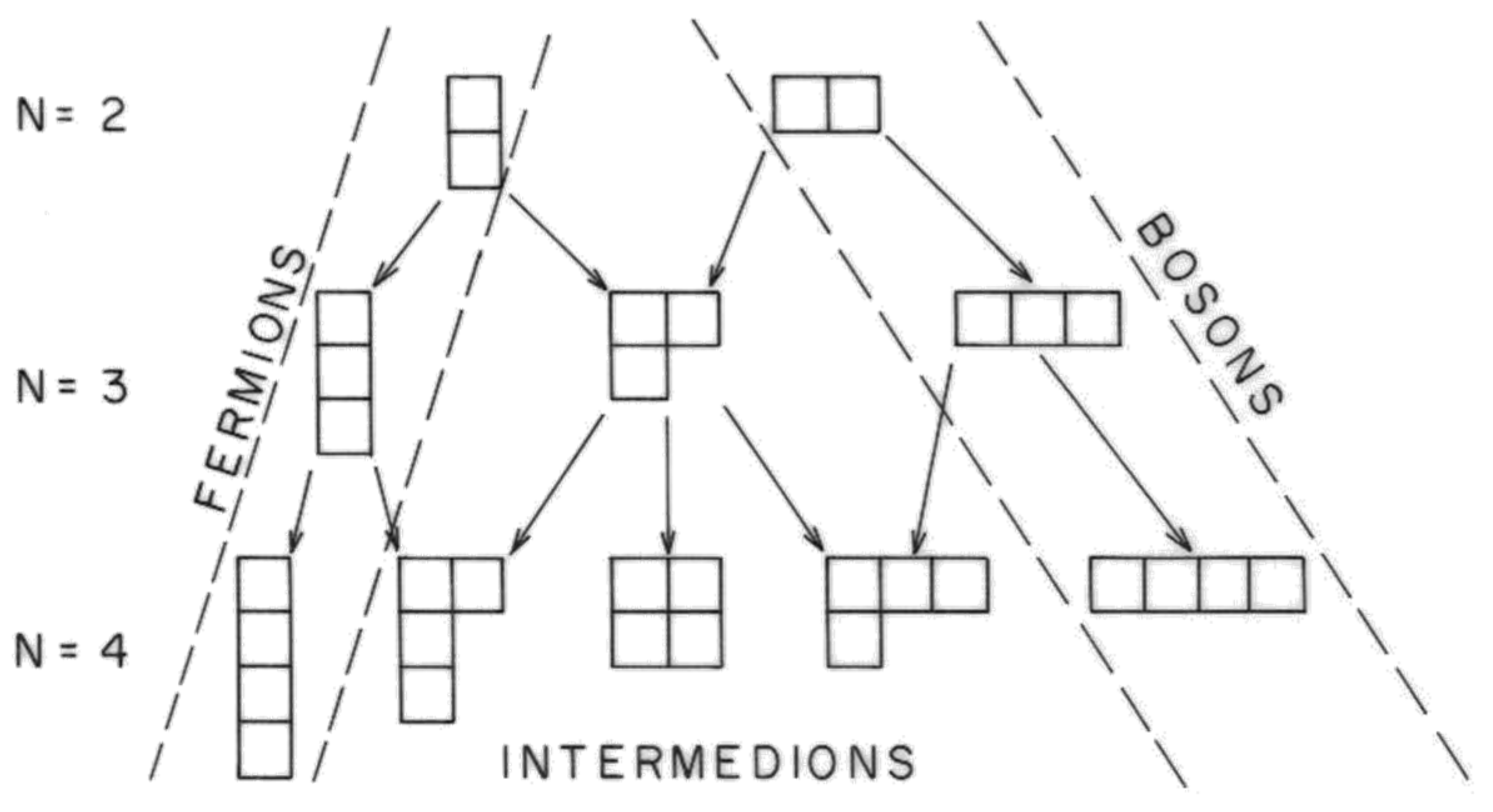
3.2. Analysis of the Properties of Identical Particle System Not Obeying PEP
- (1)
- transitions between states with different symmetry [λN] are strictly forbidden,
- (2)
- N-particle states with different [λN] have a different analytical formula for its energy.
4. Concluding Remarks
“We may not expect that in future some unknown elementary particles can be discovered that are not fermions or bosons”.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Necessary Minimum Knowledge on the Permutation Group
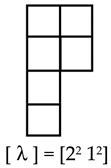

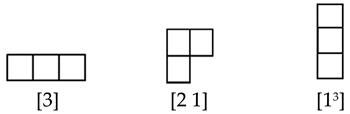

References
- Heisenberg, W. Über quantentheoretische Umdeutung kinematischer und mechanischer Beziehungen. Z. Phys. 1925, 33, 879–893. [Google Scholar] [CrossRef]
- Born, M.; Jordan, P. Zur Quantenmechanik. Zeitschrift für Physik 1925, 34, 858–888. [Google Scholar] [CrossRef]
- De Broglie, L. Recherches sur la théorie des Quanta. Ann. Phys. 1925, 10, 22–128. [Google Scholar] [CrossRef]
- Schrödinger, E. Quantisierung als Eigenwertproblem. Ann. Phys. 1926, 385, 437–490. [Google Scholar] [CrossRef]
- Schrödinger, E. An Undulatory Theory of the Mechanics of Atoms and Molecules. Phys. Rev. 1926, 28, 1049. [Google Scholar] [CrossRef]
- Heisenberg, W. Mehrkörperproblem und Resonanz in der Quantenmechanik. Z. Phys. 1926, 38, 411–426. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The physical interpretation of the quantum dynamics. Proc. R. Soc. Lond. A 1927, 113, 621–641. [Google Scholar]
- Slater, J.C. The Theory of Complex Spectra. Phys. Rev. 1929, 34, 1293. [Google Scholar] [CrossRef]
- Heisenberg, W.; Jordan, P. Anwendung der Quantenmechanik auf das Problem der anomalen Zeemaneffekte. Z. Phys. 1926, 37, 263–277. [Google Scholar] [CrossRef]
- Pauli, W., Jr. Zur Quantenmechanik des magnetischen Elektrons. Zeitschrift für Physik 1927, 43, 601–623. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The quantum theory of the electron. Proc. R. Soc. Lond. A 1928, 117, 610–624. [Google Scholar]
- Schrödinger, E. Sitzungsber. Preuss. Akad. Wiss. Phys. Math. Kl 1930, 24, 418–428. [Google Scholar]
- Barut, A.O.; Bracken, A.J. Zitterbewegung and the internal geometry of the electron. Phys. Rev. D 1981, 23, 2454. [Google Scholar] [CrossRef]
- Barut, A.O.; Zanghi, N. Classical Model of the Dirac Electron. Phys. Rev. Lett. 1984, 52, 2009. [Google Scholar] [CrossRef]
- Huang, K. On the Zitterbewegung of the Dirac Electron. Am. J. Phys. 1952, 20, 479. [Google Scholar] [CrossRef]
- Moor, S.M.; Ramirez, J.A. Electron spin in classical stochastic electrodynamics. Lett. Nuovo Cim. 1982, 33, 87–91. [Google Scholar] [CrossRef]
- Cavalleri, J. Schrödinger’s equation as a consequence of zitterbewegung. Lett. Nuovo Cim. 1985, 43, 285–291. [Google Scholar] [CrossRef]
- Hestenes, D. Zitterbewegung in Quantum Mechanics. Found. Phys. 2010, 40, 1. [Google Scholar] [CrossRef]
- Braffort, P.; Taroni, A. Mean Energy of a Free Electron in A Uniform Magnetic Field in Aleatory Electrodynamics. C. R. Acad. Sci. Paris 1967, 264, 1437–1440. [Google Scholar]
- Barranco, A.V.; Brunini, S.A.; Franca, H.M. Spin and paramagnetism in classical stochastic electrodynamics. Phys. Rev. A 1989, 39, 5492. [Google Scholar] [CrossRef]
- Muradlihar, K. Classical origin of quantum spin. Apeiron 2011, 18, 146–160. [Google Scholar]
- Muradlihar, K. The spin bivector and zeropoint energy in geometric algebra. Adv. Studies Theor. Phys. 2012, 6, 675–686. [Google Scholar]
- Marshall, T.W. Random electrodynamics. Proc. R. Soc. A 1963, 276, 475–491. [Google Scholar]
- Marshall, T.W. Statistical electrodynamics. Proc. Camb. Phil. Soc. 1965, 61, 537. [Google Scholar] [CrossRef]
- Boyer, T.H. Random electrodynamics: The theory of classical electrodynamics with classical electromagnetic zero-point radiation. Phys. Rev. D 1975, 11, 790. [Google Scholar] [CrossRef]
- Boyer, T.H. Connection between the adiabatic hypothesis of old quantum theory and classical electrodynamics with classical electromagnetic zero-point radiation. Phys. Rev. A 1978, 18, 1238. [Google Scholar] [CrossRef]
- Boyer, T.H. Any classical description of nature requires classical electromagnetic zero-point radiation. Am. J. Phys. 2011, 79, 1163. [Google Scholar] [CrossRef]
- Boyer, T.H. The contrasting roles of Planck’s constant in classical and quantum theories. Am. J. Phys. 2018, 86, 280. [Google Scholar] [CrossRef]
- Braffort, P.; Surdin, M.; Tarroni, A. Comptes Rendus Hebdomadaires des Seances de l’Academie des Sciences. Compt. Rend. 1965, 261, 4339. [Google Scholar]
- De la Peña, L.; Jáuregui, A. The spin of the electron according to stochastic electrodynamics. Found. Phys. 1982, 12, 441–465. [Google Scholar] [CrossRef]
- Chadwick, J. Possible Existence of a Neutron. Nature 1932, 129, 312. [Google Scholar] [CrossRef]
- Heisenberg, W. Über den Bau der Atomkerne. I. Z. Phys. 1932, 77, 1–11. [Google Scholar] [CrossRef]
- Wigner, E. On the Consequences of the Symmetry of the Nuclear Hamiltonian on the Spectroscopy of Nuclei. Phys. Rev. 1937, 51, 106. [Google Scholar] [CrossRef]
- Ehrenfest, P.; Oppenheimer, J.R. Note on the Statistics of Nuclei. Phys. Rev. 1931, 37, 333. [Google Scholar] [CrossRef]
- Kaplan, I.G. The Pauli Exclusion Principle. Can It Be Proved? Found. Phys. 2013, 43, 1233. [Google Scholar] [CrossRef]
- Pauli, W. Nobel Lecture. In Nobel Lectures, Physics, 1942–1962; Elsevier: Amsterdam, The Netherlands, 1964. [Google Scholar]
- Pauli, W. The Connection between Spin and Statistics. Phys. Rev. 1940, 58, 716. [Google Scholar] [CrossRef]
- Green, H.S. A Generalized Method of Field Quantization. Phys. Rev. 1953, 90, 270. [Google Scholar] [CrossRef]
- Volkov, D.V. On the quantization of half-integer spin fields. Sov. Phys. 1959, 9, 1107–1111. [Google Scholar]
- Greenberg, O.W.; Messiah, A.M. Selection Rules for Parafields and the Absence of Para Particles in Nature. Phys. Rev. 1965, 138, B1155. [Google Scholar] [CrossRef]
- Ohnuki, Y.; Kamefuchi, S. Quantum Field Theory and Parastatistics; Springer: Berlin, Germany, 1982. [Google Scholar]
- Kaplan, I.G. Statistics of molecular excitons and magnons at high concentrations. Theor. Math. Phys. 1976, 27, 254. [Google Scholar] [CrossRef]
- Avdyugin, A.N.; Zavorotnev, Y.D.; Ovander, L.N. Polaritons in highly excited crystals. Sov. Phys. Solid State 1983, 25, 2501–2502. [Google Scholar]
- Nguyen, B.A. A step-by-step Bogoliubov transformation method for diagonalising a kind of non-Hermitian effective Hamiltonian. J. Phys. C Solid State Phys. 1988, 21, L1209. [Google Scholar]
- Pushkarov, D.I. On the defecton statistics in quantum crystals. Phys. Status Solidi B 1986, 133, 525. [Google Scholar] [CrossRef]
- Kaplan, I.G.; Navarro, O. Charge transfer and the statistics of holons in a periodical lattice. J. Phys. Condens. Matter 1999, 11, 6187. [Google Scholar] [CrossRef]
- Nguyen, A.; Hoang, N.C. An approach to the many-exciton system. J. Phys. Condens. Matter 1990, 2, 4127. [Google Scholar] [CrossRef]
- Kaplan, I.G.; Navarro, O. Statistics and properties of coupled hole pairs in superconducting ceramics. Phys. C Supercond. 2000, 341, 217–220. [Google Scholar] [CrossRef]
- Pauli, W. Prog. On the Connection between Spin and Statistics. Theor. Phys. 1950, 5, 526. [Google Scholar] [CrossRef][Green Version]
- Feynman, R.P. Space-Time Approach to Quantum Electrodynamics. Phys. Rev. 1949, 76, 769. [Google Scholar] [CrossRef]
- Schwinger, J. Quantum Electrodynamics. I. A Covariant Formulation. Phys. Rev. 1948, 74, 1939. [Google Scholar] [CrossRef]
- Duck, I.; Sudarshan, E.C.G. Pauli and the Spin-Statistics Theorem; World Scientific: Singapore, 1997. [Google Scholar]
- Duck, I.; Sudarshan, E.C.G. Toward an understanding of the spin-statistics theorem. Am. J. Phys. 1998, 66, 284. [Google Scholar] [CrossRef]
- Wightman, A.S. Pauli and the Spin-Statistics Theorem. Am. J. Phys. 1999, 67, 742. [Google Scholar]
- Feynman, R.P. Feynman Lectures on Physics; R.B. Leighton and Sands, Addison-Wesley: Boston, MA, USA, 1965; Volume III, p. 3. [Google Scholar]
- Jabs, A. Connecting spin and statistics in quantum mechanics. Found. Phys. 2010, 40, 776. [Google Scholar] [CrossRef]
- Bennett, A.F. Spin-Statistics Connection for Relativistic Quantum Mechanics. Found. Phys. 2015, 45, 370. [Google Scholar] [CrossRef]
- De Martini, F.; Santamato, E. The intrinsic helicity of elementary particles and the spin-statistic connection. Int. J. Quantum Inf. 2014, 12, 1560004. [Google Scholar] [CrossRef]
- Santamato, E.; De Martini, F. Proof of the spin–statistics theorem. Found. Phys. 2015, 45, 858. [Google Scholar] [CrossRef]
- Santamato, E.; De Martini, F. Proof of the Spin Statistics Connection 2: Relativistic Theory. Found. Phys. 2017, 47, 1609–1625. [Google Scholar] [CrossRef]
- Dirac, P.A.M. The Principles of Quantum Mechanics; Clarendon Press: Oxford, UK, 1958. [Google Scholar]
- Schiff, L.I. Quantum Mechanics; Mc Graw-Hill: New York, NY, USA, 1955. [Google Scholar]
- Messiah, A.M. Quantum Mechanics; North-Holland: Amsterdam, The Netherlands, 1962. [Google Scholar]
- Paulli, W. Remarks on the History of the Exclusion Principle. Science 1946, 103, 213–215. [Google Scholar] [CrossRef] [PubMed]
- Messiah, A.M.; Greenberg, O.W. Symmetrization Postulate and Its Experimental Foundation. Phys. Rev. 1964, 136, B248. [Google Scholar] [CrossRef]
- Girardeau, M.D. Permutation Symmetry of Many-Particle Wave Functions. Phys. Rev. 1965, 139, B500. [Google Scholar] [CrossRef]
- Corson, E.M. Perturbation Methods in Quantum Mechanics of Electron Systems; University Press: Glasgow, UK, 1951. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics; Addison-Wesley: Boston, MA, USA, 1965. [Google Scholar]
- Blokhintzev, D.I. Principles of Quantum Mechanics; Allyn and Bacon: Boston, MA, USA, 1964. [Google Scholar]
- Kaplan, I.G. The Exclusion Principle and Indistinguishability of Identical Particles in Quantum Mechanics. Sov. Phys. Uspekhi 1976, 18, 988–994. [Google Scholar] [CrossRef]
- Kaplan, I.G. Is the Pauli exclusive principle an independent quantum mechanical postulate? Int. J. Quantum Chem. 2002, 89, 268–276. [Google Scholar] [CrossRef]
- Canright, G.S.; Girvin, S.M. Fractional Statistics: Quantum Possibilities in Two Dimensions. Science 1990, 247, 1197–1205. [Google Scholar] [CrossRef] [PubMed]
- Leinaas, J.M.; Myrheim, J. On the Theory of Identical Particles. Nuovo Cim. 1977, 37B, 1–23. [Google Scholar] [CrossRef]
- Mirman, R. Experimental meaning of the concept of identical particles. Nuovo Cim. 1973, 18B, 110–121. [Google Scholar]
- Khare, A. Fractional Statistics and Quantum Theory, 2nd ed.; World Scientific: Singapore, 2005. [Google Scholar]
- Kaplan, I.G. The Pauli Exclusion Principle: Origin, Verifications and Applications; Wiley: Chichester, UK, 2017. [Google Scholar]
- Piela, L. Ideas of Quantum Chemistry, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Girardeau, M.D. Proof of the Symmetrization Postulate. J. Math. Phys. 1969, 10, 1302. [Google Scholar] [CrossRef]
- Kaplan, I.G. Group Theoretical Methods in Physics, VI ed.; Man’ko, Nauka: Moscow, Russia, 1980; pp. 175–181. [Google Scholar]
- Kaplan, I.G. Problems in DFT with the total spin and degenerate states. Int. J. Quantum Chem. 2007, 107, 2595–2603. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys.Rev. 1965, 140, A1133. [Google Scholar] [CrossRef]
- Arita, M.; Araspan, S.; Bowler, D.R.; Miyazaki, T. Large-scale DFT simulations with a linear-scaling DFT code CONQUEST on K-computer. J. Adv. Simulat. Sci. Eng. 2014, 1, 87–97. [Google Scholar] [CrossRef][Green Version]
- Kaplan, I.G. Symmetry properties of the electron density and following from it limits on the KS-DFT applications. Mol. Phys. 2018, 116, 658–665. [Google Scholar] [CrossRef]
- Berry, M.V. Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. A 1984, 392, 45–57. [Google Scholar]
- Berry, M.V. The Geometric Phase. Sci. Am. 1988, 259, 46–55. [Google Scholar] [CrossRef]
- Kaplan, I.G. Exclusion principle and indistinguishability of identical particles in quantum mechanics. J. Mol. Struct. 1992, 272, 187–196. [Google Scholar] [CrossRef]
- Kaplan, I.G.; Rodimova, O.B. Matrix elements of general configuration of nonorthogonalized orbitals in state with definite spin. Int. J. Quantum Chem. 1973, 7, 1203–1220. [Google Scholar] [CrossRef]
- Kaplan, I.G. Symmetry of Many-Electron Systems; Academic Press: New York, NY, USA, 1975. [Google Scholar]
- Petrashen, M.I.; Trifonov, E.D. Applications of Group Theory in Quantum Mechanics; Cambridge M.I.T Press: Cambridge, MA, USA, 1969. [Google Scholar]
- Wilczek, F. Magnetic Flux, Angular Momentum, and Statistics. Phys. Rev. Lett. 1982, 48, 1144. [Google Scholar] [CrossRef]
- Kaplan, I.G. The Pauli Exclusion Principle and the Problems of Its Experimental Verification. Symmetry 2020, 12, 320. [Google Scholar] [CrossRef]
- Kolos, W.; Rychlewski, J. Improved theoretical dissociation energy and ionization potential for the ground state of the hydrogen molecule. J. Chem. Phys. 1993, 98, 3960. [Google Scholar] [CrossRef]
- Wolniewicz, L. Nonadiabatic energies of the ground state of the hydrogen molecule. J. Chem. Phys. 1995, 103, 1792. [Google Scholar] [CrossRef]
- Kaplan, I.G. Intermolecular Interactions: Physical Picture, Computational Methods and Model Potentials; John Wiley & Sons: Chichester, UK, 2006. [Google Scholar]
- Rutherford, D.E. Substitutional Analysis; Hafner Publishing Co.: New York, NY, USA; London, UK, 1968. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaplan, I.G. Modern State of the Pauli Exclusion Principle and the Problems of Its Theoretical Foundation. Symmetry 2021, 13, 21. https://doi.org/10.3390/sym13010021
Kaplan IG. Modern State of the Pauli Exclusion Principle and the Problems of Its Theoretical Foundation. Symmetry. 2021; 13(1):21. https://doi.org/10.3390/sym13010021
Chicago/Turabian StyleKaplan, Ilya G. 2021. "Modern State of the Pauli Exclusion Principle and the Problems of Its Theoretical Foundation" Symmetry 13, no. 1: 21. https://doi.org/10.3390/sym13010021
APA StyleKaplan, I. G. (2021). Modern State of the Pauli Exclusion Principle and the Problems of Its Theoretical Foundation. Symmetry, 13(1), 21. https://doi.org/10.3390/sym13010021



