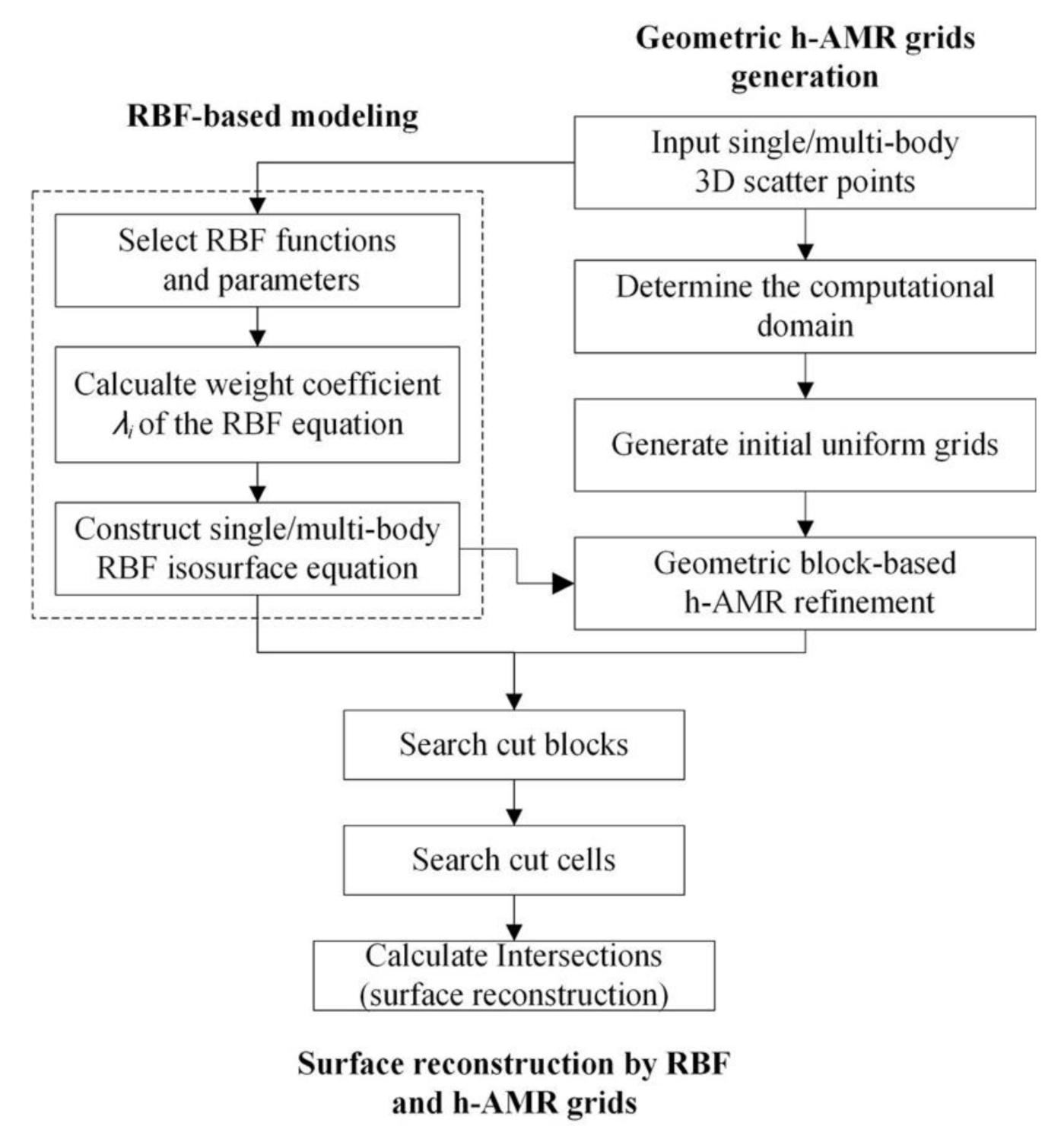
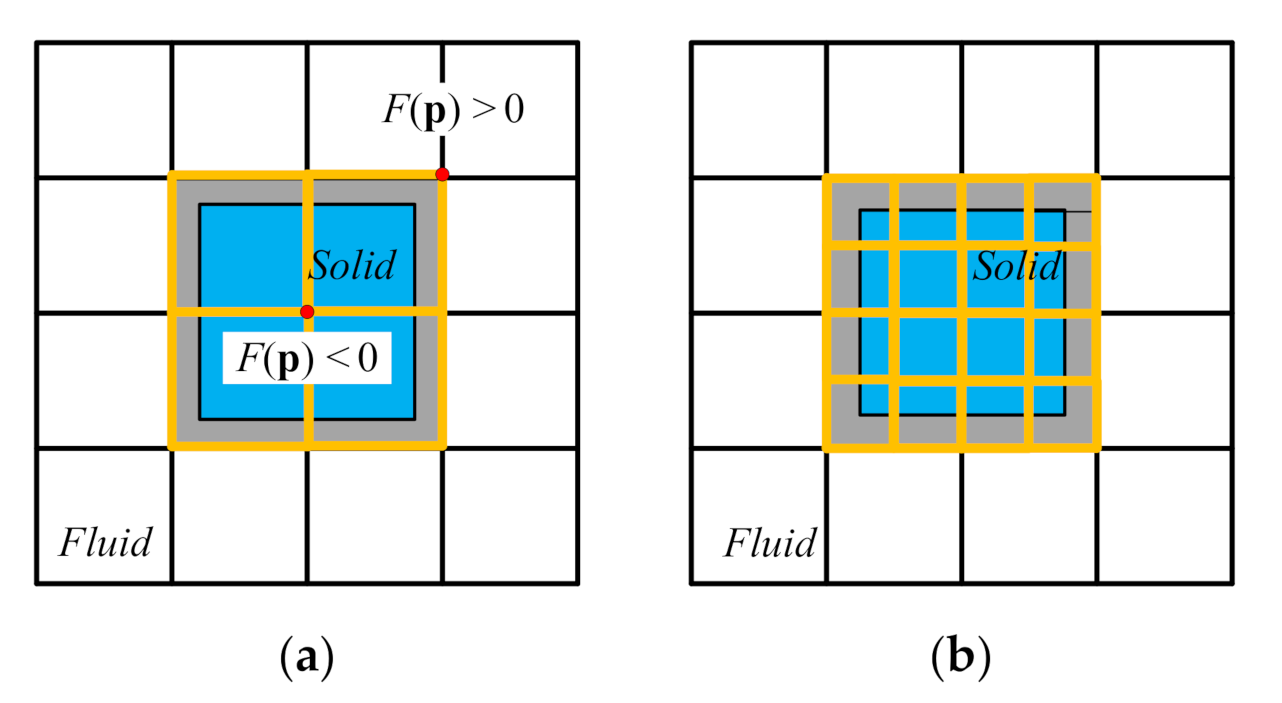
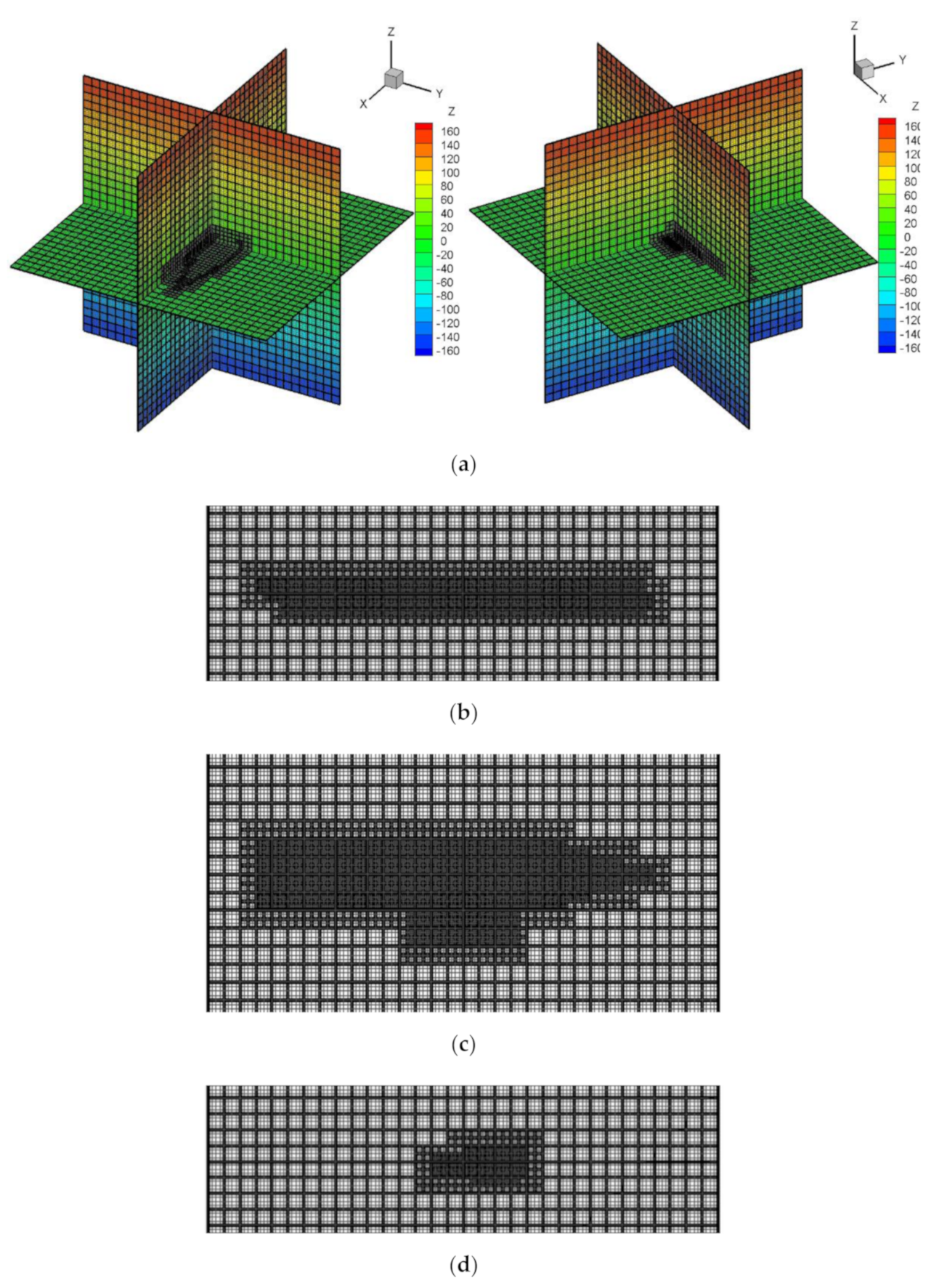
Figure 2.
(a) Uniform Cartesian grid; (b) geometric h-adaptive mesh refinement (AMR) grids (right).
Figure 2.
(a) Uniform Cartesian grid; (b) geometric h-adaptive mesh refinement (AMR) grids (right).
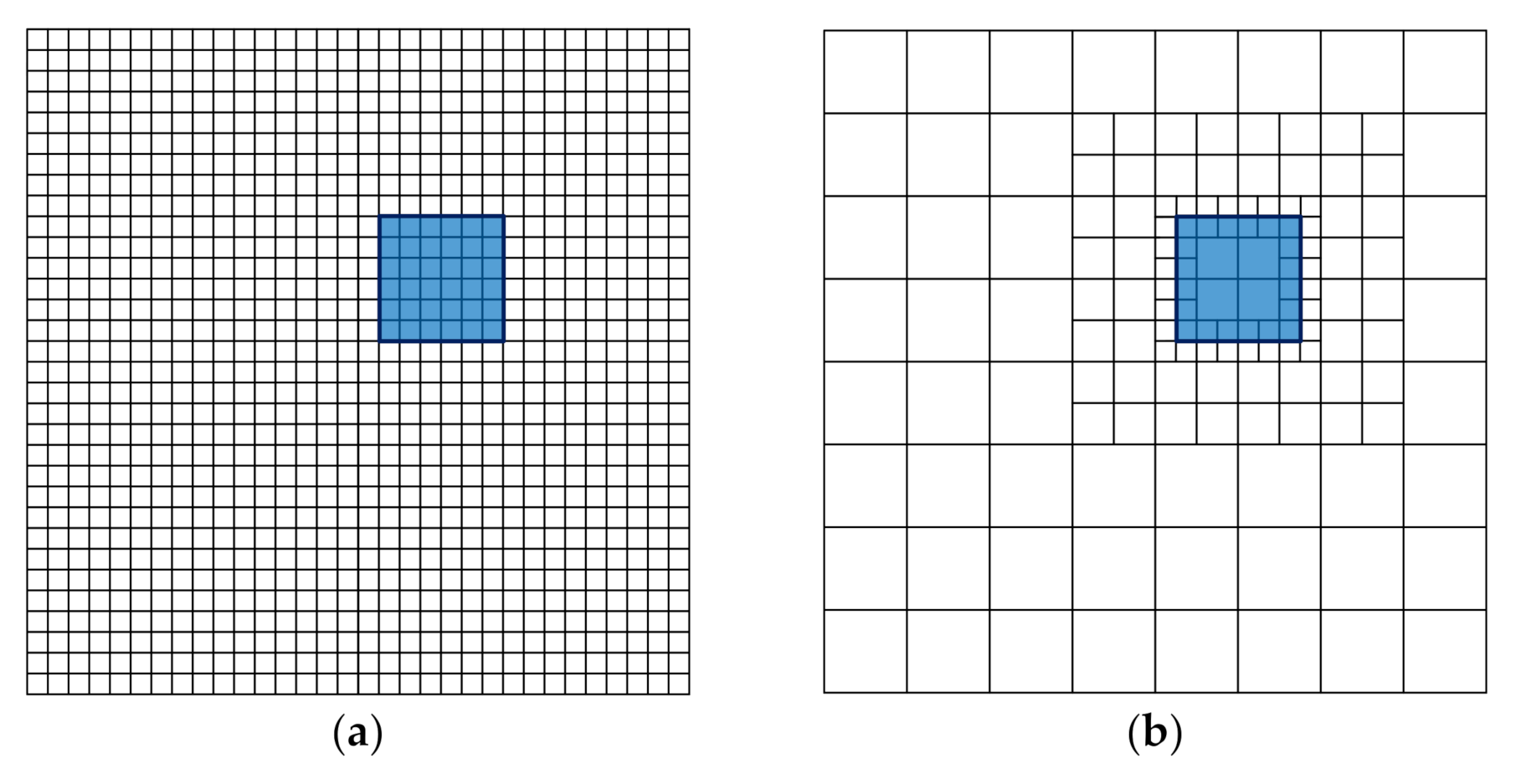
Figure 3.
Schematic diagram of three typical h-AMR geometric refinement process based on the 2D rectangle (a) patch-based; (b) cell-based; (c) block-based.
Figure 3.
Schematic diagram of three typical h-AMR geometric refinement process based on the 2D rectangle (a) patch-based; (b) cell-based; (c) block-based.
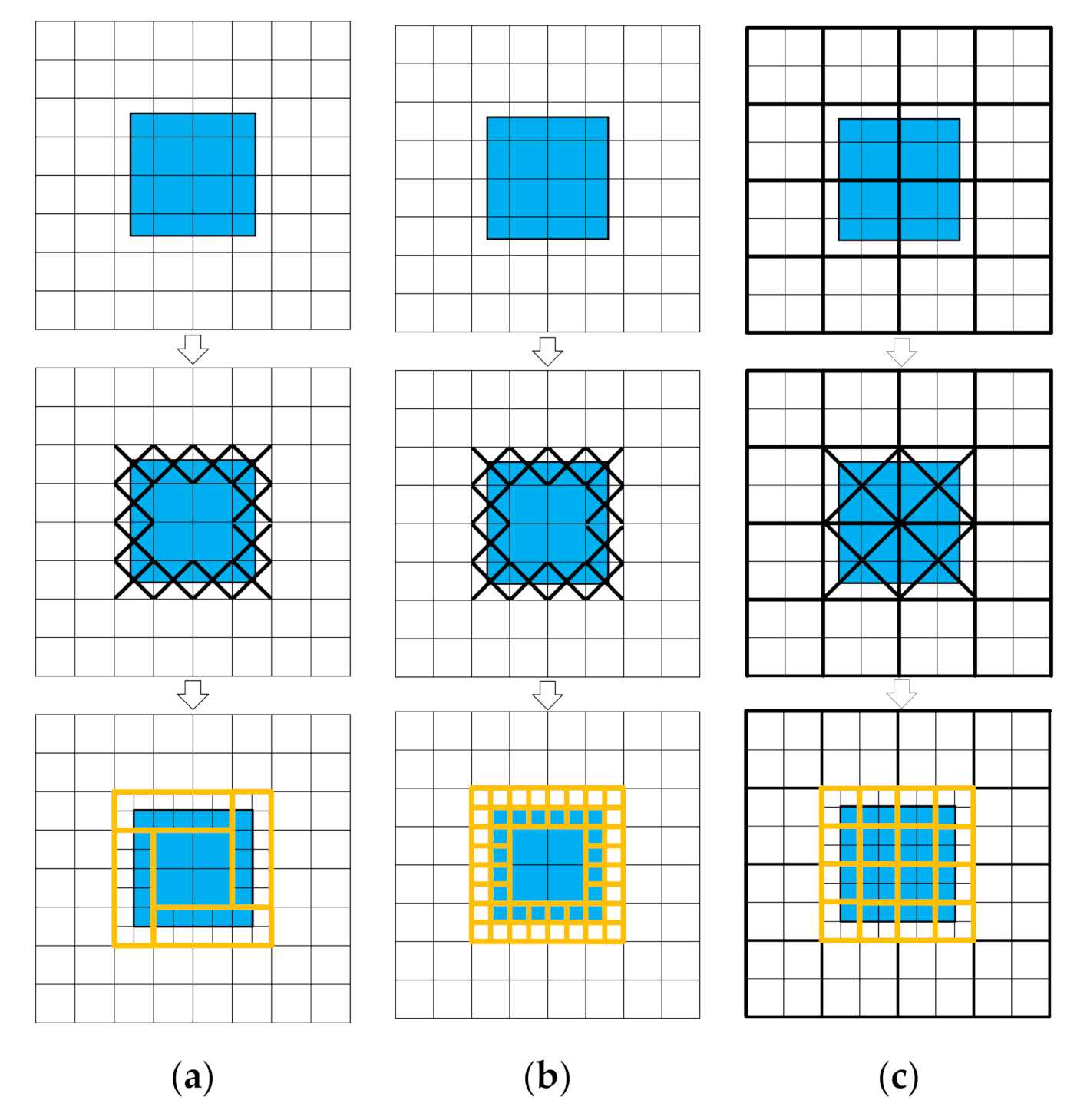
Figure 4.
Schematic diagram of a 2D block-based h-AMR grid of the 2D rectangle; (a) Geometric h-AMR grids (grid blocks: heavy lines, structured grid cells: light lines); (b) hierarchical data tree.
Figure 4.
Schematic diagram of a 2D block-based h-AMR grid of the 2D rectangle; (a) Geometric h-AMR grids (grid blocks: heavy lines, structured grid cells: light lines); (b) hierarchical data tree.
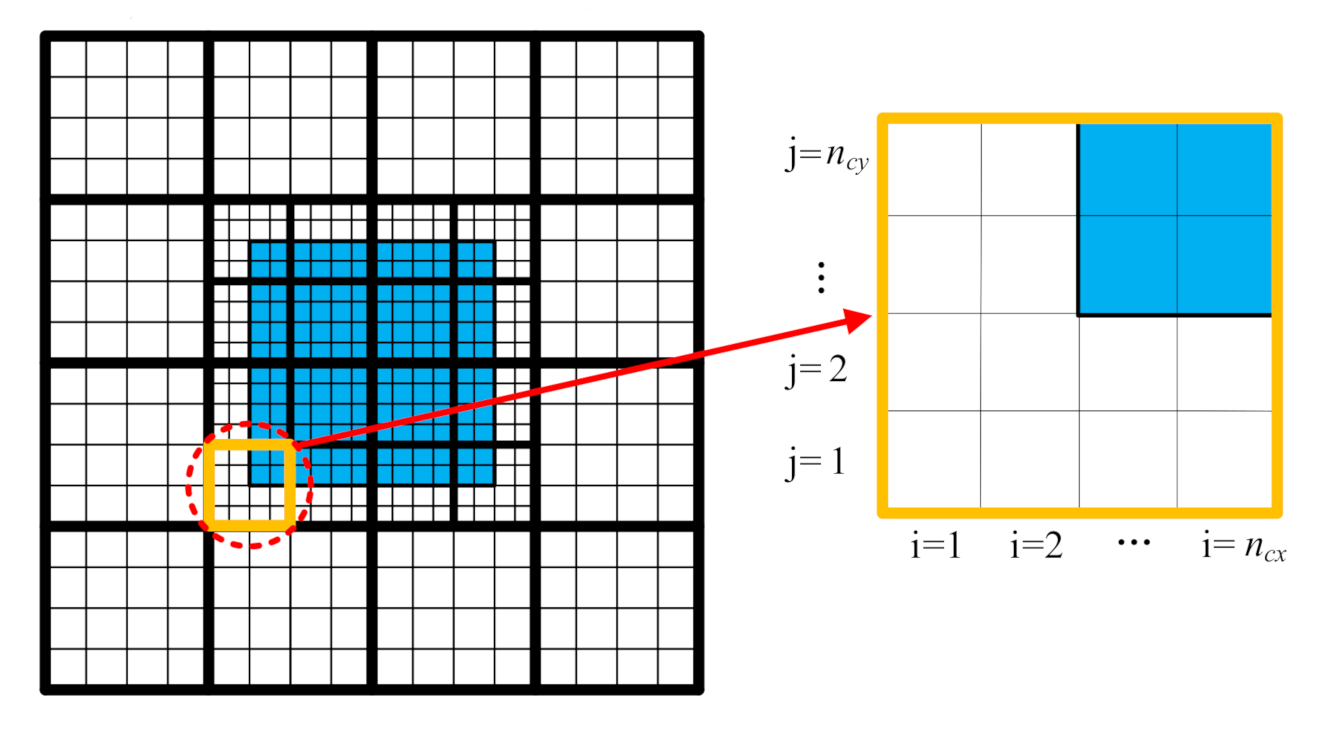
Figure 5.
Schematic diagram of index of structured grid cells (light lines) in each h-AMR block (heavy lines).
Figure 5.
Schematic diagram of index of structured grid cells (light lines) in each h-AMR block (heavy lines).
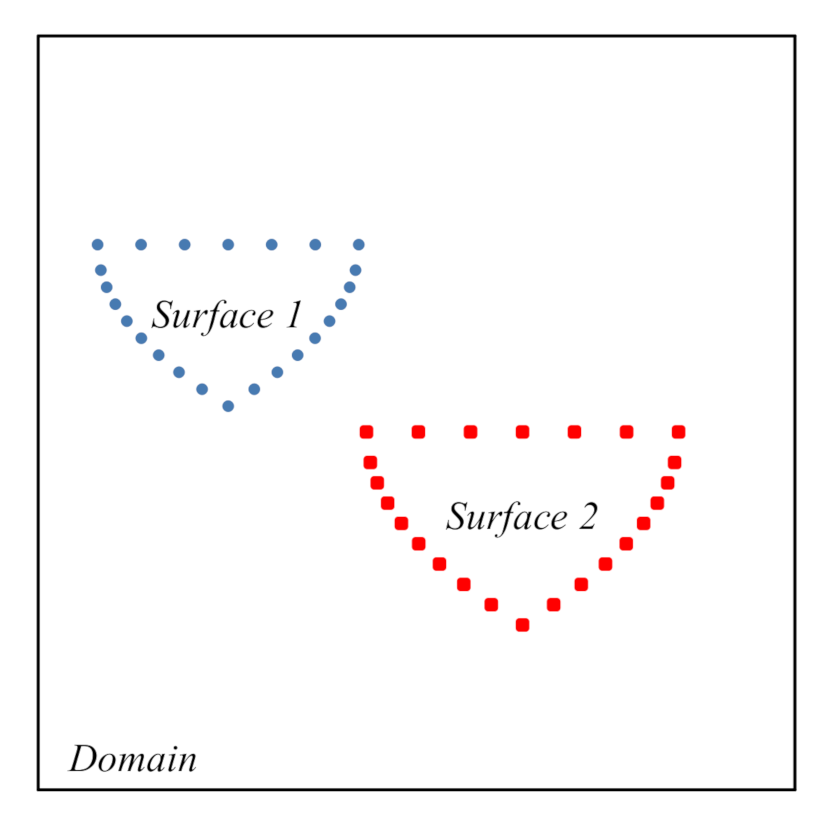
Figure 6.
Schematic diagram of scatter points of multi-body geometries in one domain.
Figure 6.
Schematic diagram of scatter points of multi-body geometries in one domain.
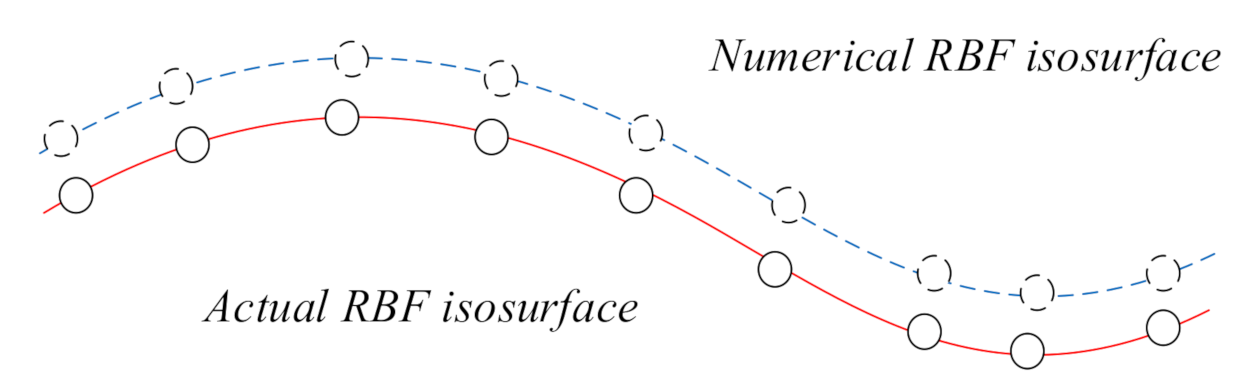
Figure 7.
Numerical radial basis function (RBF) isosurface by adding non-zero constant d.
Figure 7.
Numerical radial basis function (RBF) isosurface by adding non-zero constant d.
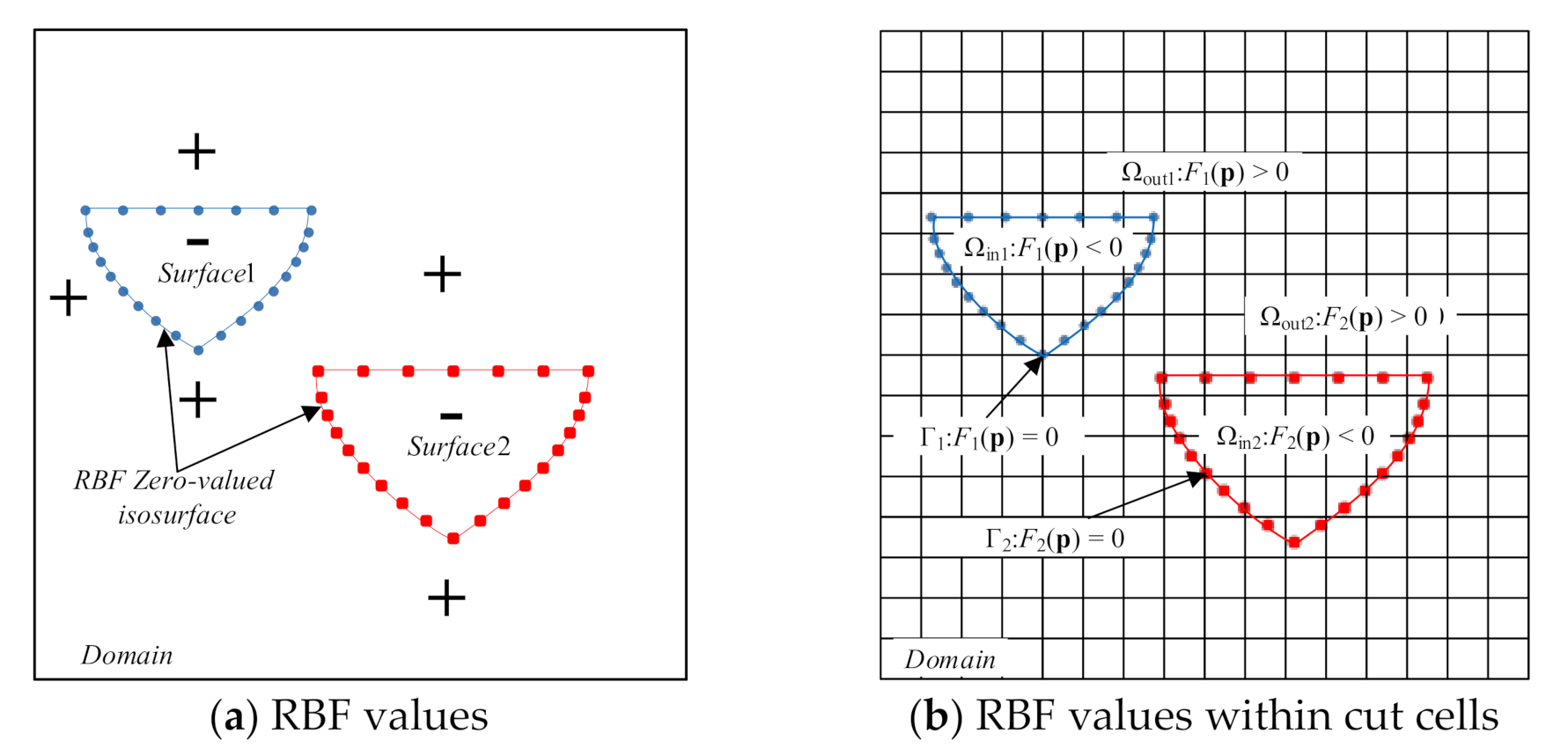
Figure 8.
Schematic diagram of RBF values inside/outside/on the multi-body isosurfaces; (a) RBF values in space; (b) RBF values in cut cells.
Figure 8.
Schematic diagram of RBF values inside/outside/on the multi-body isosurfaces; (a) RBF values in space; (b) RBF values in cut cells.
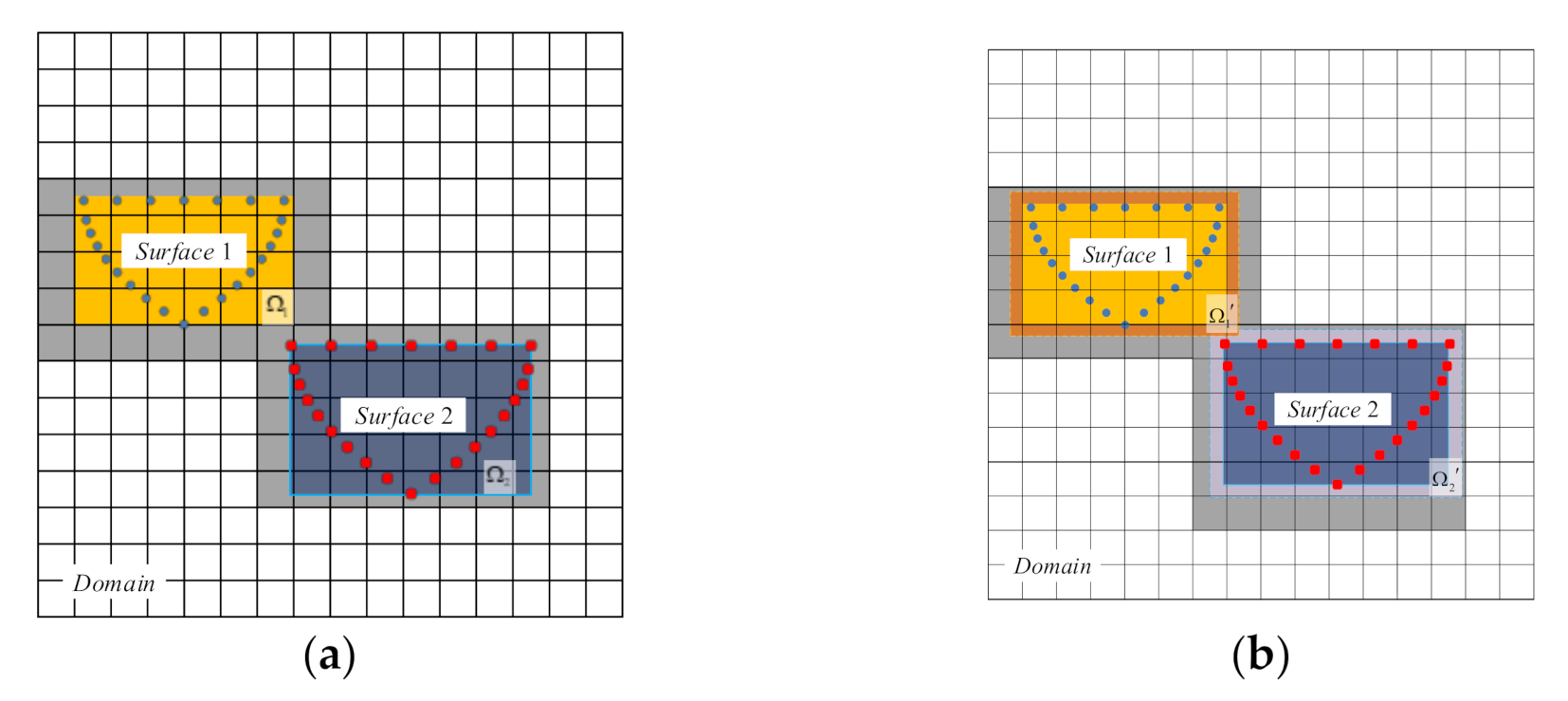
Figure 9.
Schematic diagram of bounding boxes for multi-body surfaces and the candidate cut blocks (grey); (a) Original bounding boxes; (b) Expanded bounding boxes.
Figure 9.
Schematic diagram of bounding boxes for multi-body surfaces and the candidate cut blocks (grey); (a) Original bounding boxes; (b) Expanded bounding boxes.
Figure 10.
(a) Uniform cut blocks (yellow lines) and RBF values of block nodes; (b) Geometric h-AMR cut blocks (yellow lines) of 2D rectangle (blue).
Figure 10.
(a) Uniform cut blocks (yellow lines) and RBF values of block nodes; (b) Geometric h-AMR cut blocks (yellow lines) of 2D rectangle (blue).
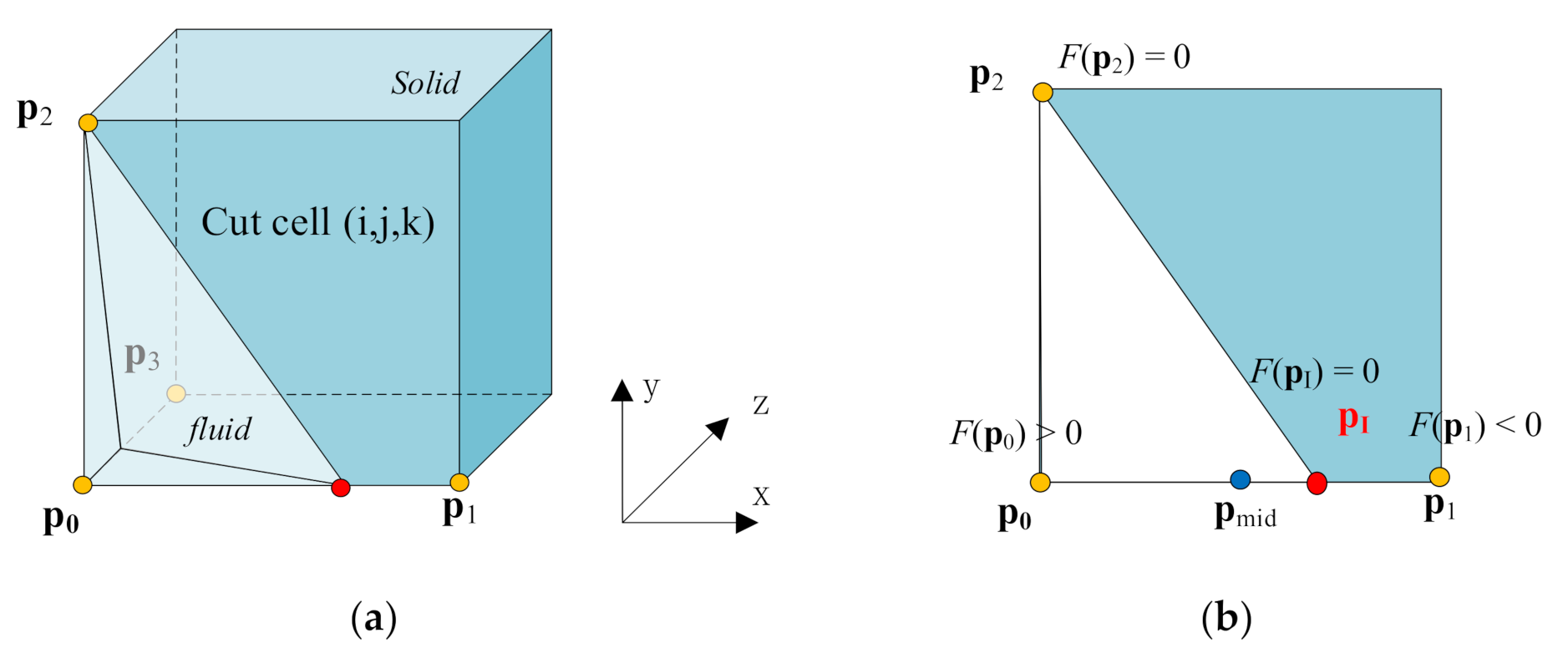
Figure 11.
(a) Schematic diagram of a 3D cut cell; (b) Intersection point on XY plan.
Figure 11.
(a) Schematic diagram of a 3D cut cell; (b) Intersection point on XY plan.
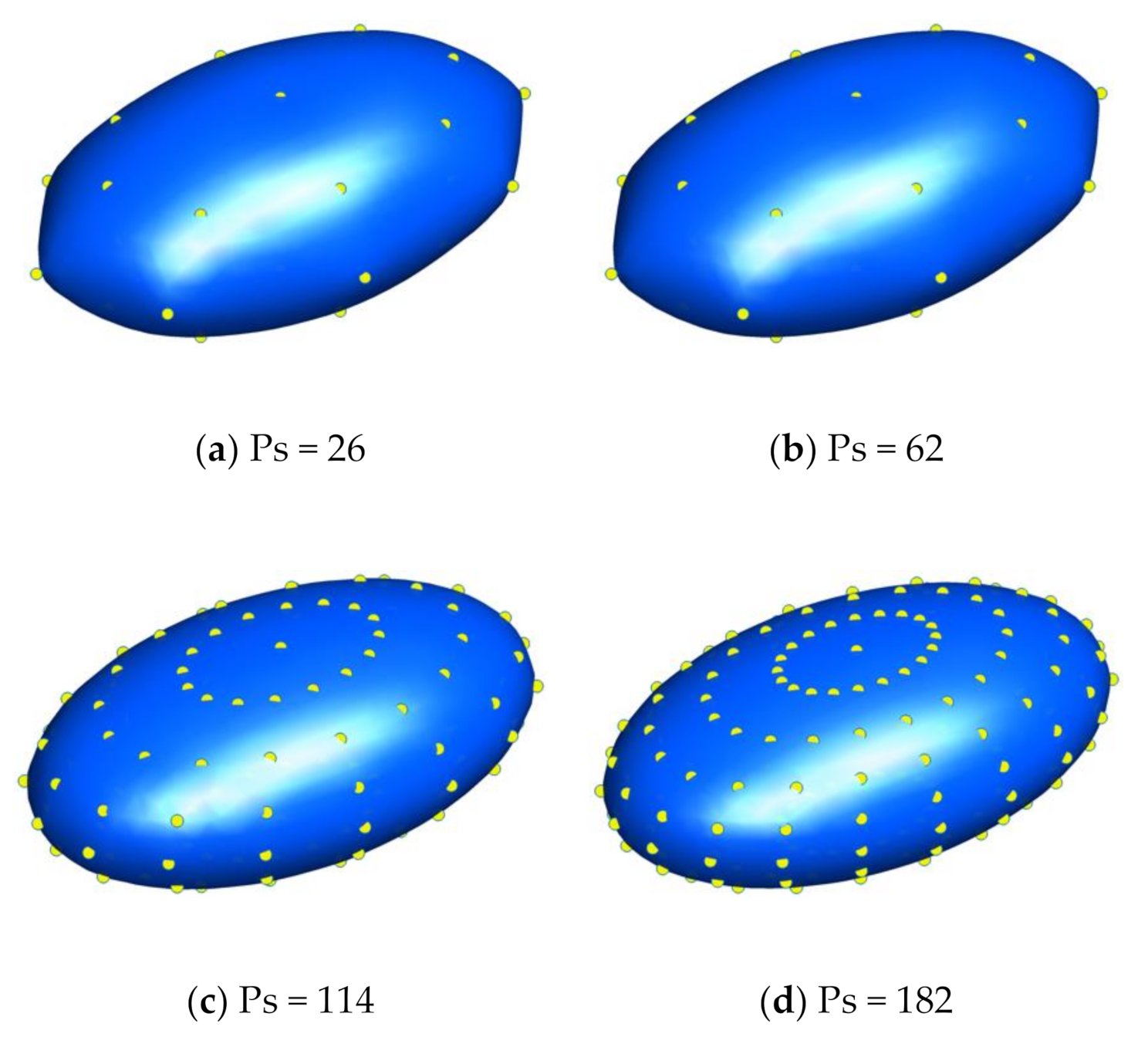
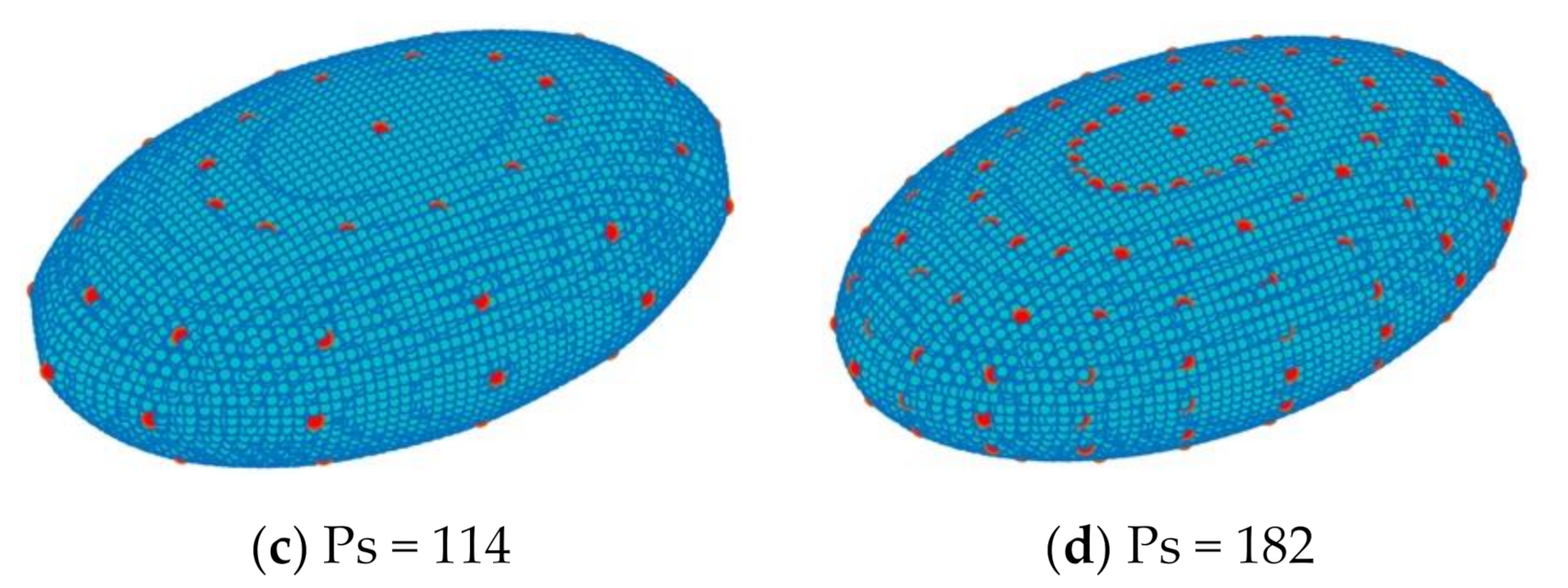
Figure 12.
Comparison of ellipsoidal RBF isosurface reconstructed by MQ-RBF of c = 0 and different numbers of sample points (yellow).
Figure 12.
Comparison of ellipsoidal RBF isosurface reconstructed by MQ-RBF of c = 0 and different numbers of sample points (yellow).
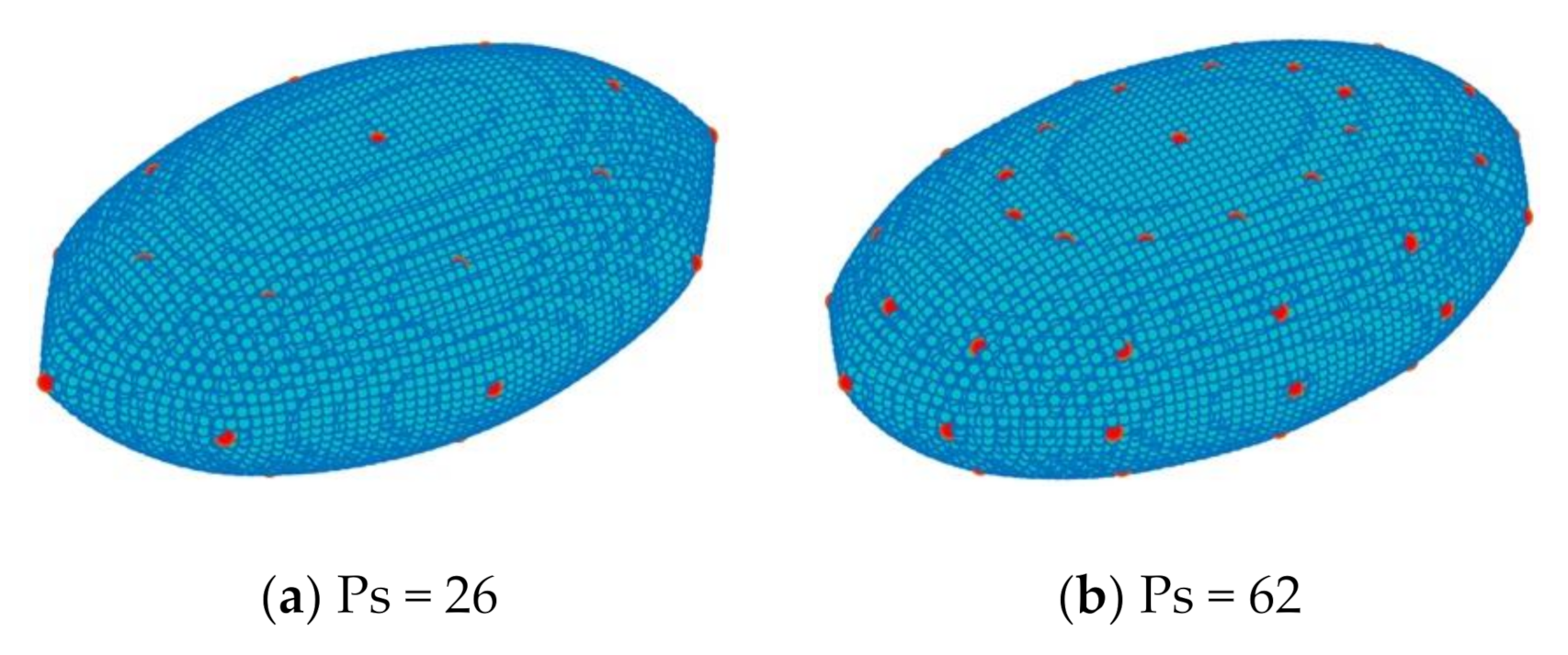
Figure 13.
Comparison of intersection points (cyan) of and different numbers of sample points (red).
Figure 13.
Comparison of intersection points (cyan) of and different numbers of sample points (red).
Figure 14.
Geometric h-AMR girds (grid blocks: heavy lines; grid cells: light lines) (left) and the intersection points (right) of cone reconstructed by C2- CSRBF of = 1.0 different cut cell sizes.
Figure 14.
Geometric h-AMR girds (grid blocks: heavy lines; grid cells: light lines) (left) and the intersection points (right) of cone reconstructed by C2- CSRBF of = 1.0 different cut cell sizes.
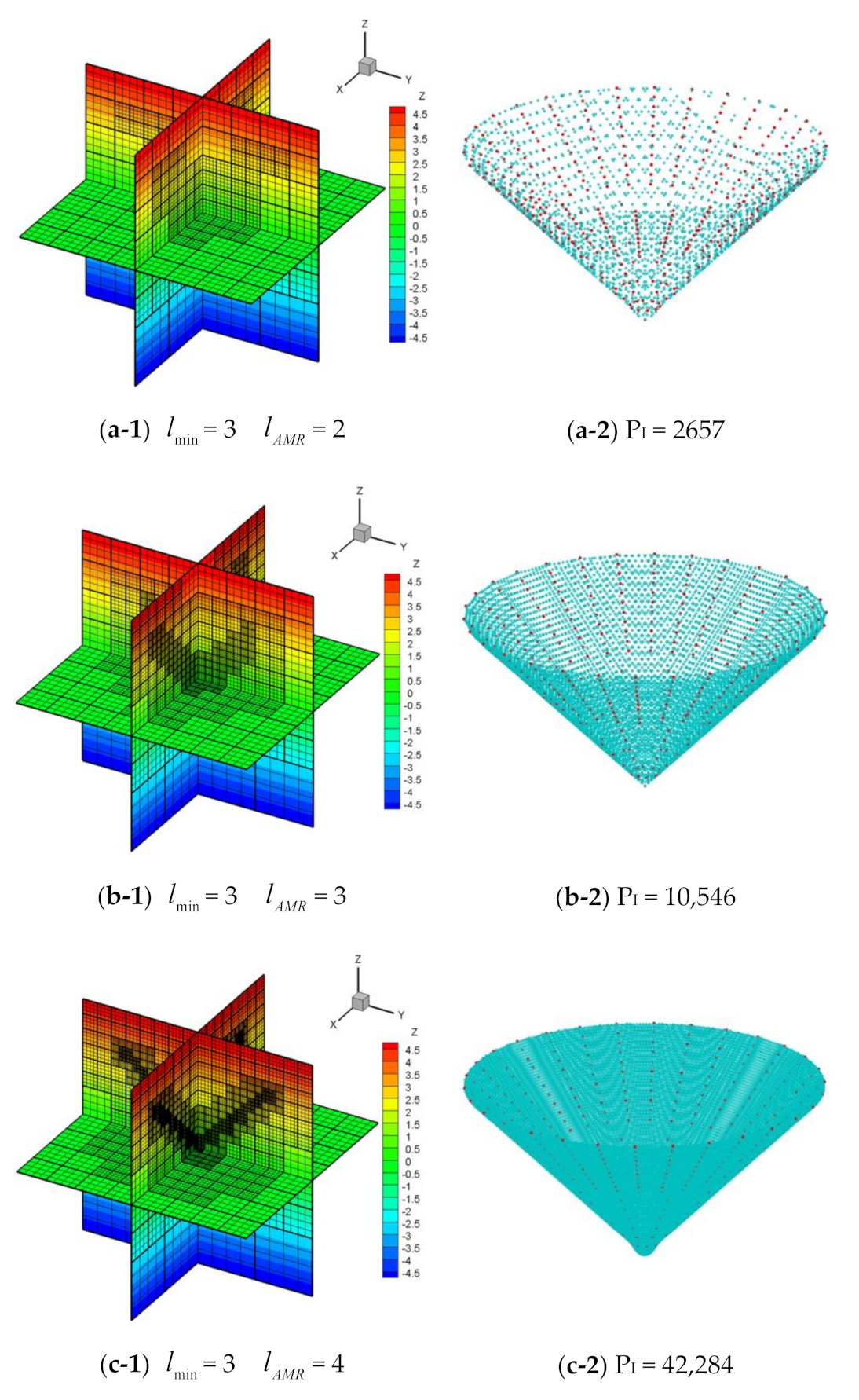
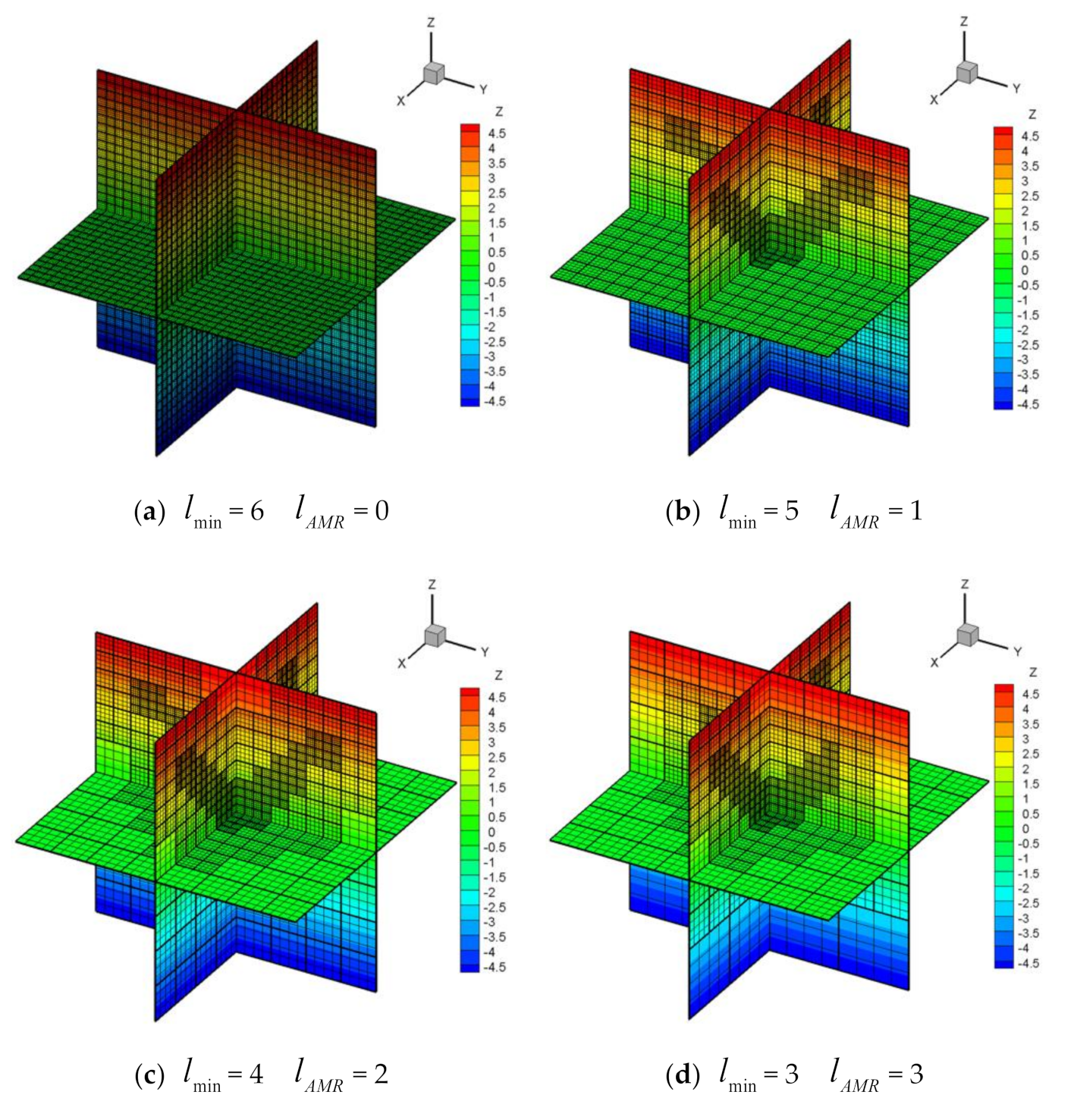
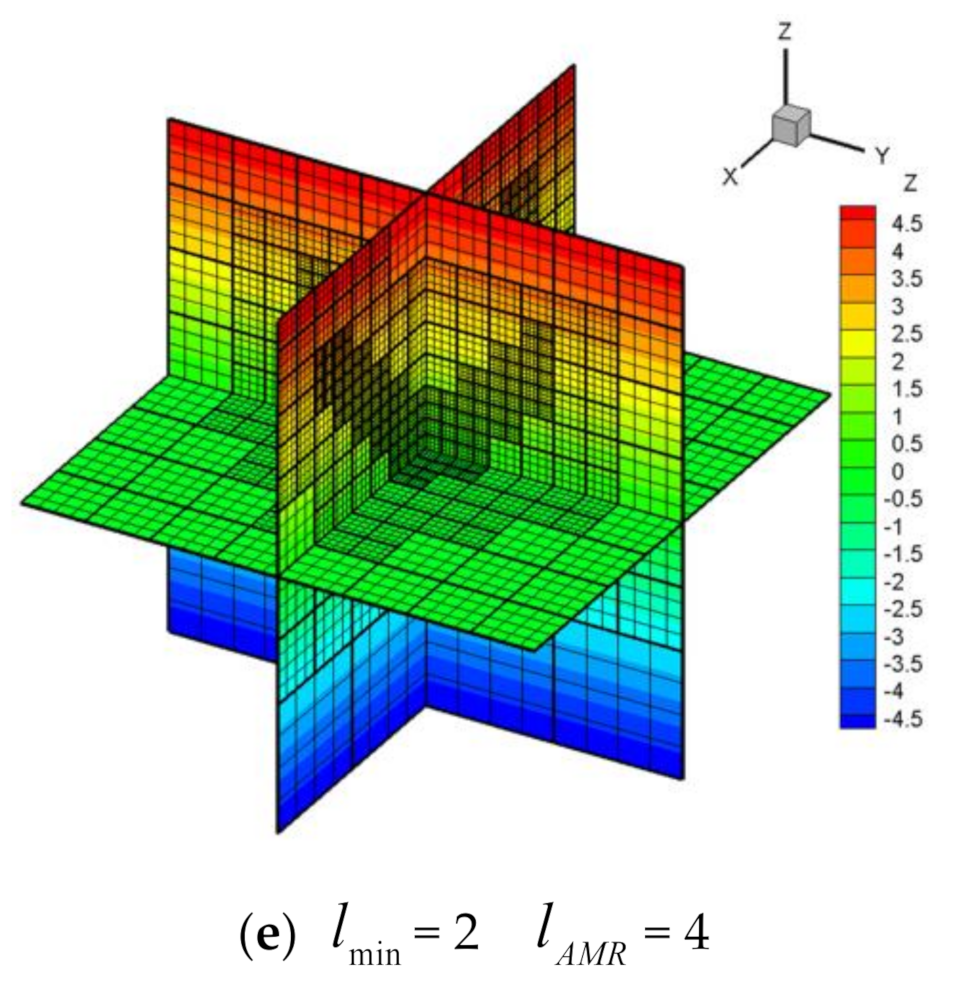
Figure 15.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of cone reconstructed by different AMR levels.
Figure 15.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of cone reconstructed by different AMR levels.
Figure 16.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of cone reconstructed by different numbers of grid cells.
Figure 16.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of cone reconstructed by different numbers of grid cells.
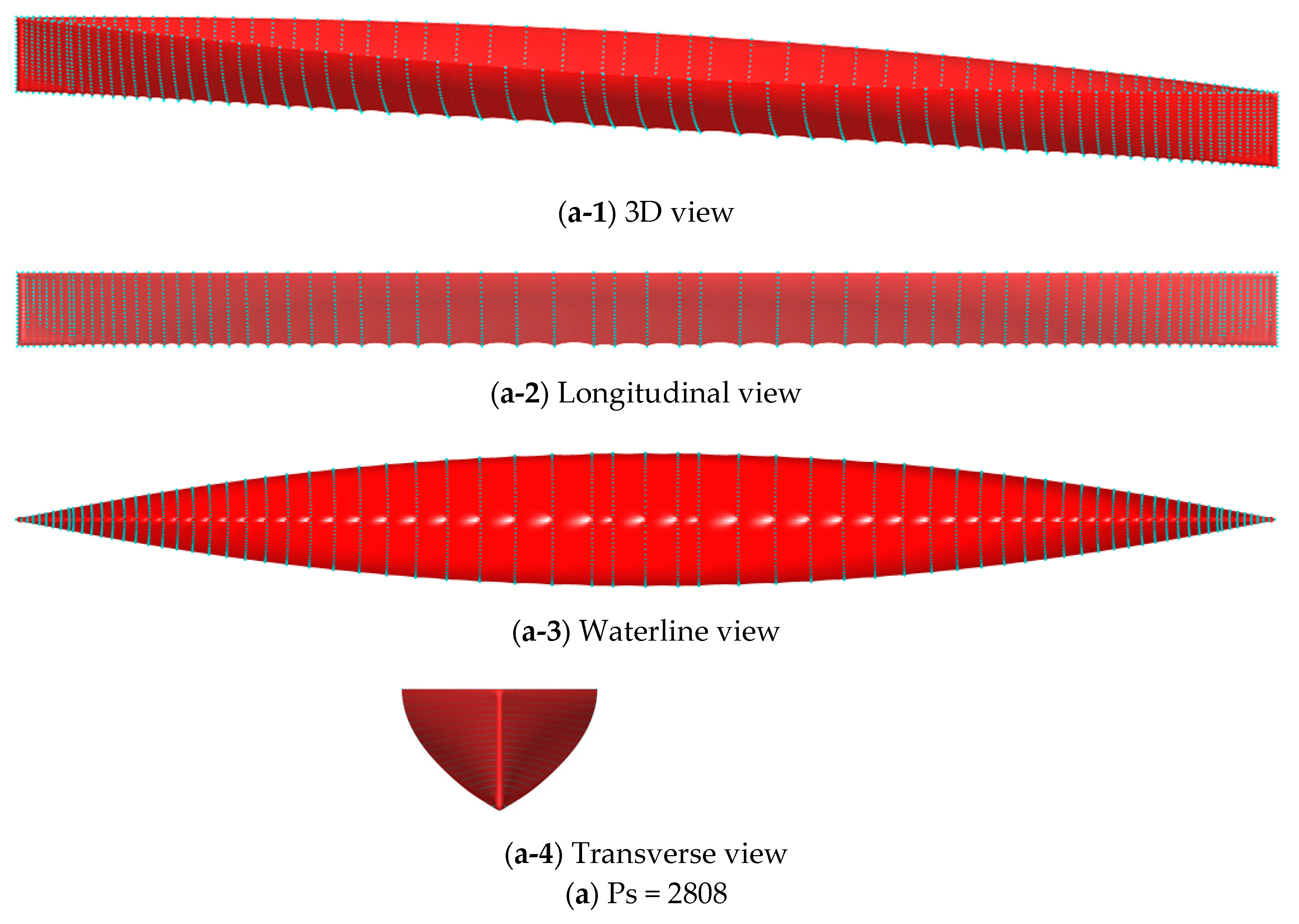
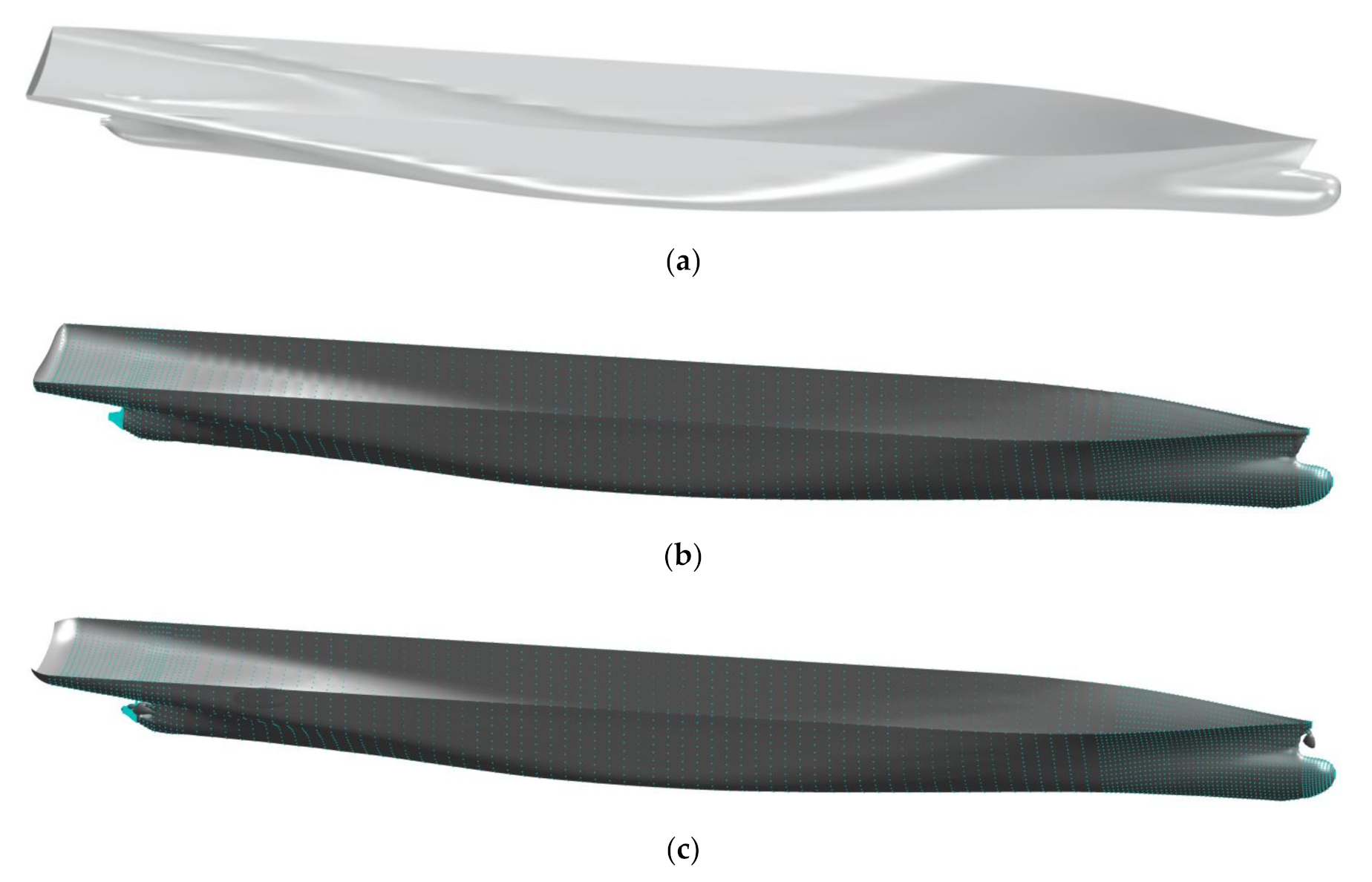
Figure 17.
3D view, longitudinal view, waterline view and transverse view of Wigley hull implicit model (red) with sample points (cyan); (a) Ps = 2808; (b) Ps = 5422.
Figure 17.
3D view, longitudinal view, waterline view and transverse view of Wigley hull implicit model (red) with sample points (cyan); (a) Ps = 2808; (b) Ps = 5422.
Figure 18.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of the Wigley hull by two sets of sample points; (a) Ps = 2808; (b) Ps = 5422.
Figure 18.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of the Wigley hull by two sets of sample points; (a) Ps = 2808; (b) Ps = 5422.
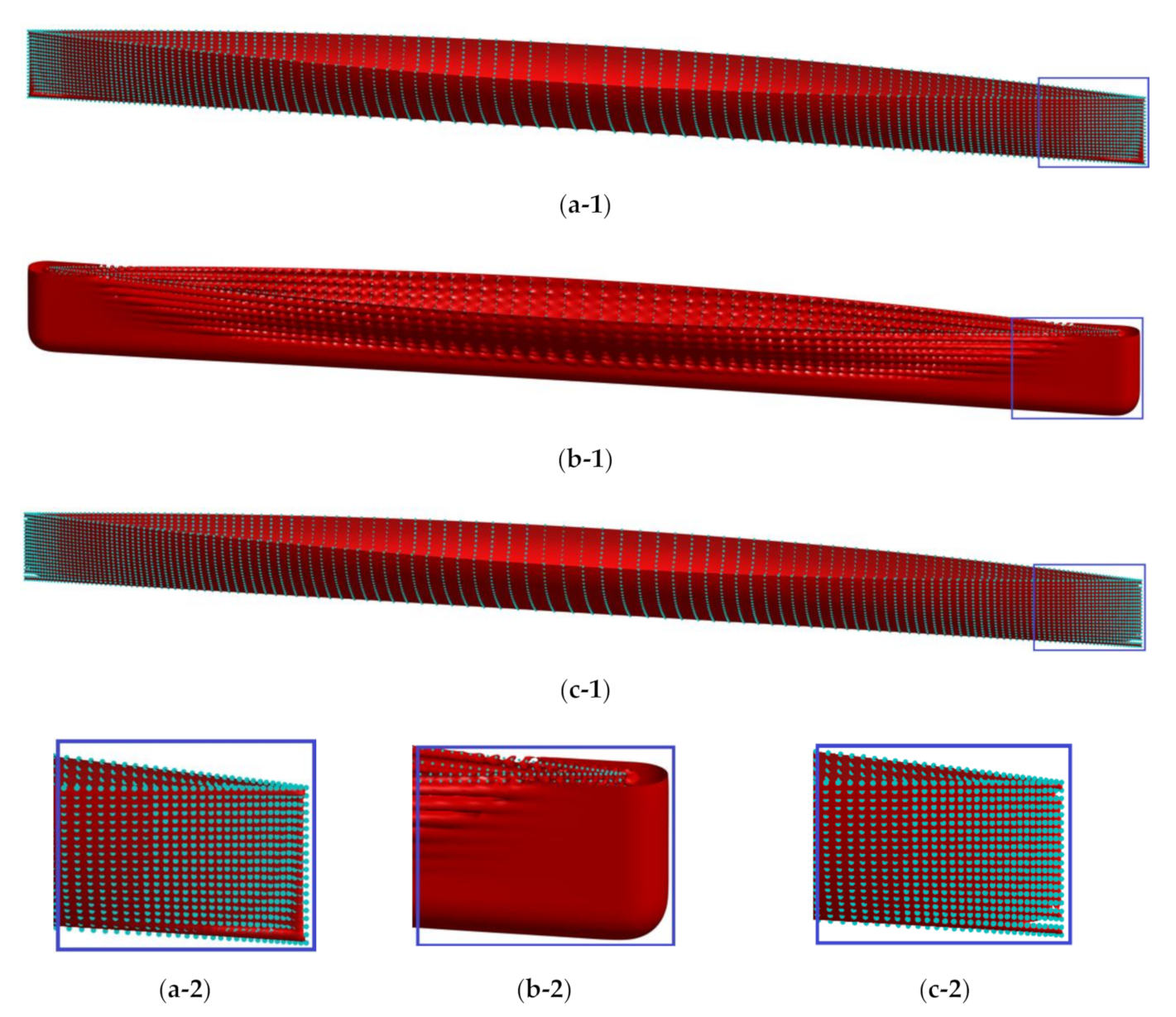
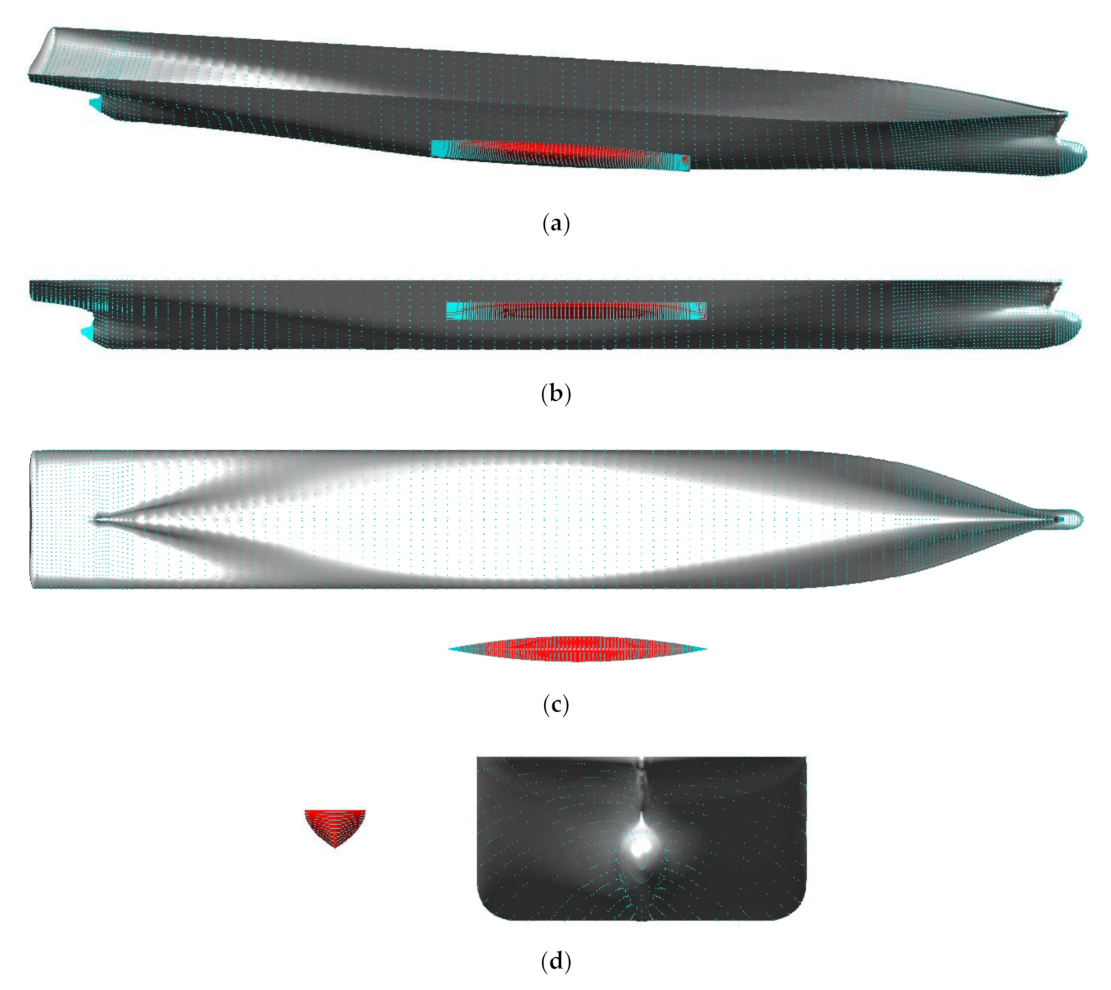
Figure 19.
Comparison RBF isosurfaces (red) and sample points (cyan) of Wigley hull by three RBFs (up) and their enlarged bow surface (down); (a) MQ-RBF of c = 0; (b) MQ-RBF of Franke’s c; (c) CSRBF of = 1.0.
Figure 19.
Comparison RBF isosurfaces (red) and sample points (cyan) of Wigley hull by three RBFs (up) and their enlarged bow surface (down); (a) MQ-RBF of c = 0; (b) MQ-RBF of Franke’s c; (c) CSRBF of = 1.0.
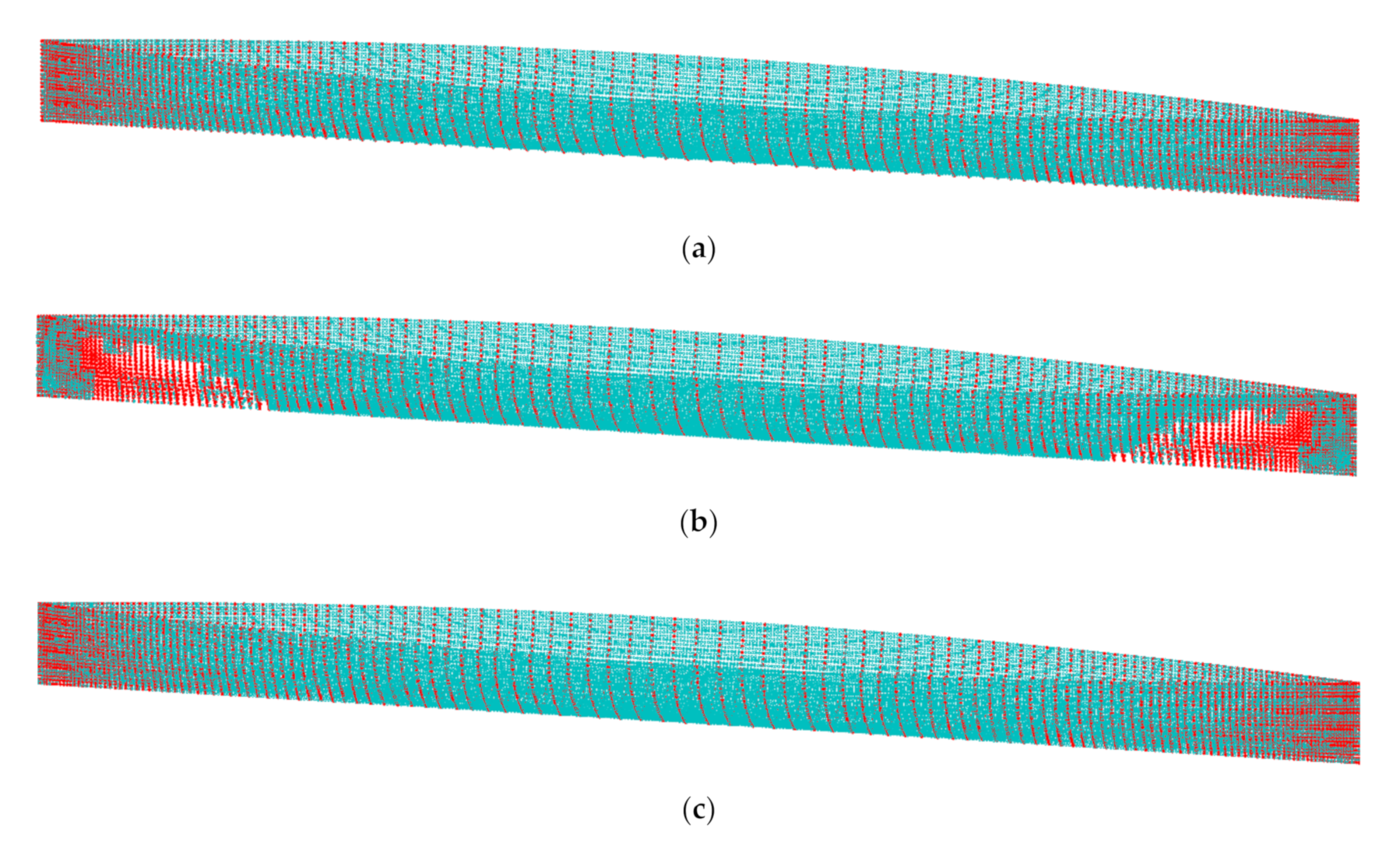
Figure 20.
Intersections (cyan) and sample points (red) of Wigley hull reconstructed by three RBFs; (a) MQ-RBF of c = 0; (b) MQ-RBF of Franke’s c; (c) CSRBF of = 1.0.
Figure 20.
Intersections (cyan) and sample points (red) of Wigley hull reconstructed by three RBFs; (a) MQ-RBF of c = 0; (b) MQ-RBF of Franke’s c; (c) CSRBF of = 1.0.
Figure 21.
Comparison of KCS hull models (a) Standard explicit model; (b) Implicit model by MQ-RBF of c = 0; (c) Implicit model by C2- CSRBF of = 1.0.
Figure 21.
Comparison of KCS hull models (a) Standard explicit model; (b) Implicit model by MQ-RBF of c = 0; (c) Implicit model by C2- CSRBF of = 1.0.
Figure 22.
Implicit multi-body hull models with sample points (cyan): Wigley hull (red) and KCS hull (grey); (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
Figure 22.
Implicit multi-body hull models with sample points (cyan): Wigley hull (red) and KCS hull (grey); (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
Figure 23.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of multi-body hull system; (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
Figure 23.
Geometric h-AMR grids (grid blocks: heavy lines; grid cells: light lines) of multi-body hull system; (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
Figure 24.
Intersection points of the multi-body hulls with sample points (red): Wigley hull (cyan) and KCS hull (green); (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
Figure 24.
Intersection points of the multi-body hulls with sample points (red): Wigley hull (cyan) and KCS hull (green); (a) 3D view; (b) Longitudinal view; (c) Waterline view; (d) Transverse view.
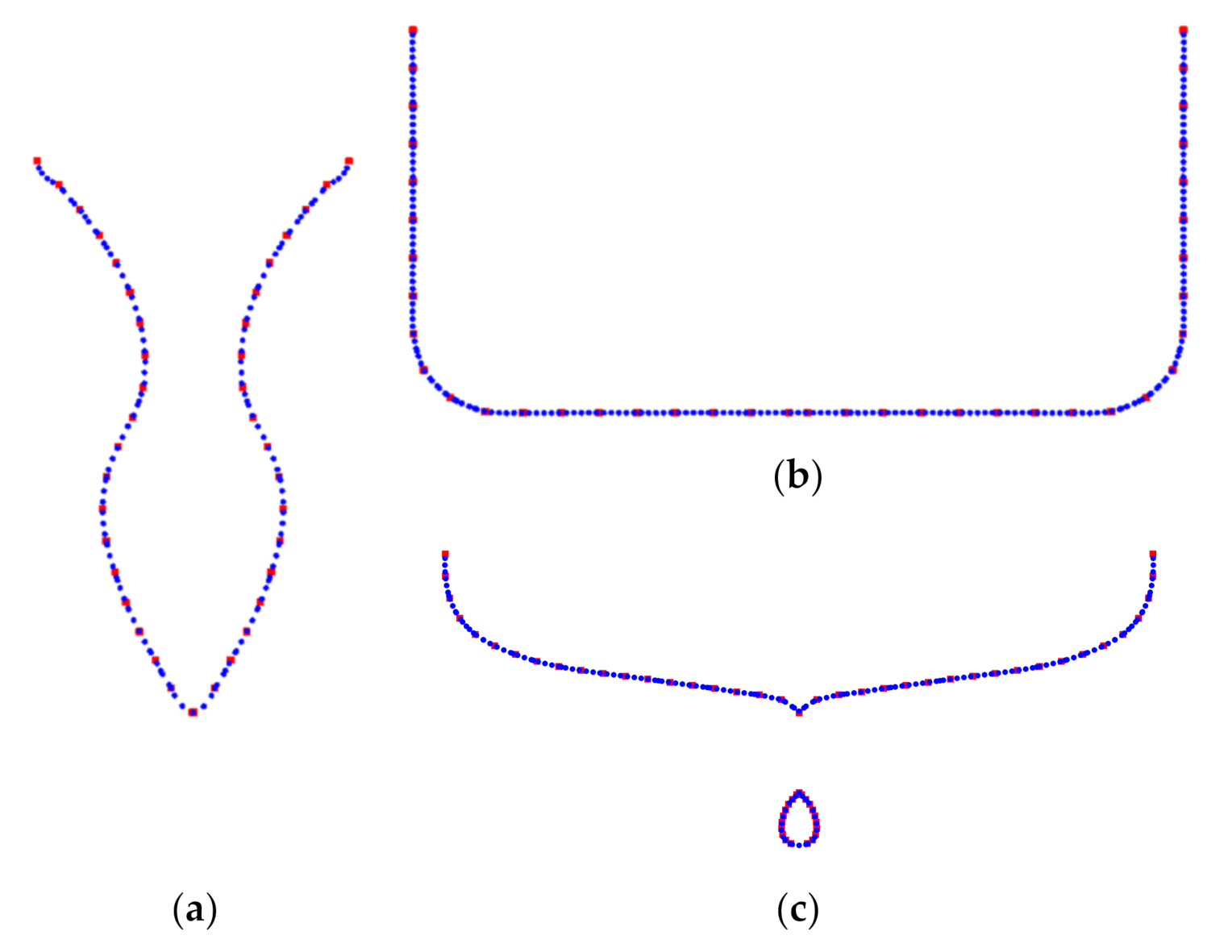
Figure 25.
Comparison of the reconstructed typical cross-sections (blue) of KCS hull in multi-body system with sample points (red); (a) Bow; (b) Midship; (c) Stern.
Figure 25.
Comparison of the reconstructed typical cross-sections (blue) of KCS hull in multi-body system with sample points (red); (a) Bow; (b) Midship; (c) Stern.
Table 1.
Some shape parameters
c in multiquadric (MQ)-RBF [
10,
34].
Table 1.
Some shape parameters
c in multiquadric (MQ)-RBF [
10,
34].
| Name | Definition | Type |
|---|
| | Constant value |
| Franke’s empirical c | |
| Exponential c | | Variable value |
| Random c | |
| Trigonometric c | |
Table 2.
Wendland’s compactly supported RBF (CSRBF) of different parameter continuities.
Table 2.
Wendland’s compactly supported RBF (CSRBF) of different parameter continuities.
| Order of Continuity | Definition |
|---|
| |
| |
| |
| |
Table 3.
Parameters of ellipsoid and h-AMR background grids.
Table 3.
Parameters of ellipsoid and h-AMR background grids.
| Name | Value |
|---|
| a, b, c | 3, 5, 2 |
| Domain length () | 20 |
| Number of grid cells () | 4 |
| Initial grid level () | 3 |
| AMR level () | 3 |
| Cut cell size (dx = dy = dz) | /27 |
Table 4.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF of c = 0 and different numbers of sample points.
Table 4.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF of c = 0 and different numbers of sample points.
| PS | PI | RMSE | meanE | maxE | TM × 10−6 | TR | TAVG × 10−6 |
|---|
| 26 | 7350 | 0.0368 | 0.0322 | 0.0718 | 418 | 0.194 | 26 |
| 62 | 7858 | 0.0128 | 0.0107 | 0.0301 | 511 | 0.266 | 34 |
| 114 | 7885 | 0.0058 | 0.0047 | 0.0157 | 1290 | 0.378 | 48 |
| 182 | 7930 | 0.0031 | 0.0024 | 0.0091 | 3459 | 0.516 | 65 |
Table 5.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF of c = 0 and different distance d.
Table 5.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF of c = 0 and different distance d.
| d | PI | RMSE | meanE | maxE | TM × 10−6 | TR | TAVG × 10−6 | RMSE% |
|---|
| 1 | 7858 | 0.0128 | 0.0107 | 0.0301 | 511 | 0.266 | 34 | 100.00% |
| 0.001 | 8294 | 0.0128 | 0.0106 | 0.0336 | 544 | 0.265 | 32 | 99.42% |
| 0.01 | 8747 | 0.0127 | 0.0107 | 0.0301 | 629 | 0.270 | 31 | 99.17% |
| 0.1 | 8166 | 0.0128 | 0.0107 | 0.0301 | 546 | 0.264 | 32 | 99.88% |
| 10 | 7846 | 0.0128 | 0.0107 | 0.0301 | 656 | 0.267 | 34 | 100.03% |
| 100 | 7846 | 0.0128 | 0.0107 | 0.0301 | 557 | 0.272 | 35 | 100.03% |
| 1000 | 7846 | 0.0128 | 0.0107 | 0.0301 | 587 | 0.281 | 36 | 100.03% |
Table 6.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF with different shape parameters c.
Table 6.
Comparison of errors and time of ellipsoidal surface reconstructed by MQ-RBF with different shape parameters c.
| c | PI | RMSE | meanE | maxE | TM × 10−6 | TR | TAVG × 10−6 | RMSE% |
|---|
| c = 0 | 7858 | 0.0128 | 0.0107 | 0.0301 | 511 | 0.266 | 34 | 100.00% |
| Franke’s c | 7939 | 0.0014 | 0.0009 | 0.0057 | 513 | 0.270 | 34 | 10.87% |
| Exponential c | 7866 | 0.0123 | 0.0102 | 0.0296 | 616 | 0.271 | 34 | 95.48% |
| Random c | 8725 | 0.0002 | 0.0001 | 0.0010 | 665 | 0.267 | 31 | 1.35% |
| Trigonometric c | 7871 | 0.0096 | 0.0077 | 0.0275 | 595 | 0.273 | 35 | 74.97% |
Table 7.
Comparison of errors and time of ellipsoidal surface reconstructed by CSRBF with different continuities and support coefficients .
Table 7.
Comparison of errors and time of ellipsoidal surface reconstructed by CSRBF with different continuities and support coefficients .
| Continuity | | PI | RMSE | meanE | maxE | TM × 10−6 | TR | TAVG × 10−6 | RMSE% |
|---|
| C0 | 0.75 | 7769 | 0.0143 | 0.0102 | 0.0405 | 514 | 0.305 | 39 | 111.40% |
| 1.0 | 7792 | 0.0132 | 0.0099 | 0.0365 | 563 | 0.299 | 38 | 102.70% |
| 1.5 | 7821 | 0.0128 | 0.0101 | 0.0337 | 567 | 0.308 | 39 | 99.53% |
| 2.0 | 7832 | 0.0127 | 0.0103 | 0.0326 | 571 | 0.330 | 42 | 99.20% |
| C2 | 0.75 | 7912 | 0.0013 | 0.0008 | 0.0057 | 565 | 0.327 | 41 | 10.43% |
| 1.0 | 7930 | 0.0009 | 0.0006 | 0.0040 | 567 | 0.321 | 40 | 7.21% |
| 1.5 | 7930 | 0.0007 | 0.0005 | 0.0030 | 589 | 0.329 | 42 | 5.58% |
| 2.0 | 7944 | 0.0007 | 0.0005 | 0.0027 | 598 | 0.337 | 42 | 5.09% |
| C4 | 0.75 | 7937 | 0.0008 | 0.0006 | 0.0031 | 591 | 0.357 | 45 | 6.36% |
| 1.0 | 7936 | 0.0006 | 0.0004 | 0.0023 | 597 | 0.344 | 43 | 4.45% |
| 1.5 | 7937 | 0.0004 | 0.0003 | 0.0017 | 602 | 0.349 | 44 | 3.31% |
| 2.0 | 7954 | 0.0003 | 0.0002 | 0.0014 | 610 | 0.350 | 44 | 2.68% |
| C6 | 0.75 | 7934 | 0.0008 | 0.0006 | 0.0031 | 616 | 0.355 | 45 | 6.46% |
| 1.0 | 7936 | 0.0005 | 0.0003 | 0.0018 | 628 | 0.353 | 44 | 3.70% |
| 1.5 | 7934 | 0.0002 | 0.0002 | 0.0009 | 681 | 0.356 | 45 | 1.89% |
| 2.0 | 7945 | 0.0002 | 0.0001 | 0.0006 | 674 | 0.367 | 46 | 1.19% |
Table 8.
Parameters of cone and h-AMR background grids.
Table 8.
Parameters of cone and h-AMR background grids.
| Name | Value |
|---|
| a, b, c | 0.5, 0.5, 0.5 |
| Domain length () | 10 |
| Number of grid cells () | 4 |
| Initial grid level () | 3 |
| AMR level () | 3 |
| Cut cell size (dx = dy = dz) | /27 |
Table 9.
Comparison of errors and time of cone reconstructed by MQ-RBF with different shape parameters and C2-CSRBF with different support coefficients .
Table 9.
Comparison of errors and time of cone reconstructed by MQ-RBF with different shape parameters and C2-CSRBF with different support coefficients .
| PI | RMSE | meanE | maxE | TM | TR | TAVG × 10−6 | RMSE% |
|---|
| c = 0 | 11,719 | 0.0059 | 0.0032 | 0.0376 | 0.038 | 1.598 | 136 | 100.00% |
| Franke’s c | 11,497 | 0.0043 | 0.0008 | 0.0687 | 0.039 | 1.590 | 138 | 72.02% |
| Exponential c | 11,680 | 0.0057 | 0.0030 | 0.0352 | 0.039 | 1.624 | 139 | 95.86% |
| Random c | 28,594 | 1.6269 | 0.0874 | 0.5531 | 0.040 | 0.313 | 11 | 27491.31% |
| Trigonometric c | 11,223 | 0.0052 | 0.0023 | 0.0572 | 0.041 | 1.574 | 140 | 87.63% |
| C2 = 1.0 | 10,546 | 0.0034 | 0.0006 | 0.0590 | 0.039 | 2.031 | 177 | 57.30% |
| C2 = 1.5 | 11,409 | 0.0033 | 0.0005 | 0.0601 | 0.042 | 2.190 | 192 | 56.15% |
| C2 = 2.0 | 11,391 | 0.0034 | 0.0005 | 0.0607 | 0.041 | 2.204 | 194 | 56.98% |
Table 10.
Comparison of errors and time of cone reconstructed by C2- CSRBF of = 1.0 and different cut cell sizes.
Table 10.
Comparison of errors and time of cone reconstructed by C2- CSRBF of = 1.0 and different cut cell sizes.
| Total Level | | | Block Number | Size | PI | RMSE | meanE | maxE | TR |
|---|
| 5 | 3 | 2 | 873 | 0.15625 | 2657 | 0.0031 | 0.0006 | 0.0497 | 0.604 |
| 6 | 3 | 3 | 3337 | 0.078125 | 10,546 | 0.0034 | 0.0006 | 0.0590 | 2.031 |
| 7 | 3 | 4 | 12,617 | 0.0390625 | 45,778 | 0.0034 | 0.0006 | 0.0590 | 7.786 |
Table 11.
Comparison of errors and time of cone reconstructed by C2- CSRBF = 1.0 and different AMR levels.
Table 11.
Comparison of errors and time of cone reconstructed by C2- CSRBF = 1.0 and different AMR levels.
| | Block Number | RMSE | meanE | maxE | TR | TIG | Ttotal |
|---|
| 6 | 0 | 37,449 | 0.0033 | 0.0006 | 0.0590 | 2.448 | 194.150 | 204.895 |
| 5 | 1 | 6697 | 0.0033 | 0.0006 | 0.0590 | 2.332 | 4.775 | 18.661 |
| 4 | 2 | 4041 | 0.0034 | 0.0006 | 0.0590 | 2.318 | 0.602 | 10.327 |
| 3 | 3 | 3337 | 0.0034 | 0.0006 | 0.0590 | 2.031 | 0.333 | 8.120 |
| 2 | 4 | 3337 | 0.0034 | 0.0006 | 0.0590 | 2.134 | 0.297 | 8.472 |
Table 12.
Comparison of errors and time of cone reconstructed by C2- CSRBF = 1.0 and different number of grid cells.
Table 12.
Comparison of errors and time of cone reconstructed by C2- CSRBF = 1.0 and different number of grid cells.
| | | Block Number | RMSE | meanE | maxE | TR |
|---|
| 1 | 0 | 128 | 1 | 0.0033 | 0.0006 | 0.0590 | 41.143 |
| 3 | 0 | 32 | 73 | 0.0034 | 0.0006 | 0.0590 | 5.839 |
| 3 | 1 | 16 | 233 | 0.0034 | 0.0006 | 0.0590 | 4.341 |
| 3 | 2 | 8 | 873 | 0.0034 | 0.0006 | 0.0590 | 3.025 |
| 3 | 3 | 4 | 3337 | 0.0033 | 0.0005 | 0.0601 | 2.031 |
| 3 | 4 | 2 | 11145 | 0.0034 | 0.0005 | 0.0601 | 2.319 |
Table 13.
Main dimensions of Wigley hull and parameters of h-AMR background grids.
Table 13.
Main dimensions of Wigley hull and parameters of h-AMR background grids.
| Name | Value |
|---|
| Length of waterline () | 60 |
| Maximum beam of waterline (B) | 6 |
| Draft (T) | 3.75 |
| Domain length () | 160 |
| Number of grid cells () | 4 |
| Initial grid level () | 5 |
| AMR level () | 4 |
Table 14.
Comparison errors and time of Wigley hull reconstructed by two sets of sample points.
Table 14.
Comparison errors and time of Wigley hull reconstructed by two sets of sample points.
| PS | PI | RMSE | meanE | maxE | TM | TR | TAVG × 10−3 |
|---|
| 2808 | 31,653 | 0.0281 | 0.0146 | 0.2968 | 12.302 | 22.737 | 0.718 |
| 5422 | 31,188 | 0.0118 | 0.0050 | 0.1754 | 80.815 | 42.773 | 1.371 |
Table 15.
Comparison of errors and time of Wigley hull reconstructed by three RBFs.
Table 15.
Comparison of errors and time of Wigley hull reconstructed by three RBFs.
| PI | RMSE | meanE | maxE | TM | TR | TAVG × 10−6 | RMSE% |
|---|
| c = 0 | 31,188 | 0.0118 | 0.0050 | 0.1754 | 80.815 | 42.773 | 1.371 | 100.00% |
| Franke’s c | 32,297 | 0.1856 | 0.0581 | 1.3624 | 85.002 | 46.123 | 1.428 | 1576.89% |
| C2 = 1.0 | 31,416 | 0.0045 | 0.0021 | 0.0421 | 95.154 | 61.520 | 1.958 | 37.94% |
Table 16.
Main dimensions of KCS hull and parameters of multi-body h-AMR background grids.
Table 16.
Main dimensions of KCS hull and parameters of multi-body h-AMR background grids.
| Name | Value |
|---|
| Length of waterline () | 232.5 |
| Maximum beam of waterline (B) | 32.2 |
| Draft (T) | 10.8 |
| Domain length () | 320 |
| Number of grid cells () | 4 |
| Initial grid level () | 6 |
| AMR level () of Wigley hull | 4 |
| AMR level () of KCS hull | 2 |
| Cut cell size (dx = dy = dz) of Wigley hull | /211 |
| Cut cell size (dx = dy = dz) of KCS hull | /29 |
Table 17.
Comparison of errors and time of single hull and multi-body hulls.
Table 17.
Comparison of errors and time of single hull and multi-body hulls.
| Name | PI | RMSE | meanE | maxE | TR | TAVG × 10−3 |
|---|
| Single Wigley | 31,188 | 0.0118 | 0.0050 | 0.1754 | 42.773 | 1.371 |
| Wigley of multi-body | 31,188 | 0.0117 | 0.0047 | 0.1737 | 42.305 | 1.356 |
| Single KCS | 43,150 | - | - | - | 59.757 | 1.385 |
| KCS of multi-body | 43,150 | - | - | - | 58.933 | 1.366 |