Exact and Numerical Solitary Wave Structures to the Variant Boussinesq System
Abstract
1. Introduction
2. Analysis of the Improved -Expansion Approach
3. Exact Solutions of the Variant Boussinesq System
- Case 1
- Case 2
- Case 3
- Case 4
- Case 5
- Case 6
- Case 7
- Case 8
4. Numerical Solutions of the Variant Boussinesq System
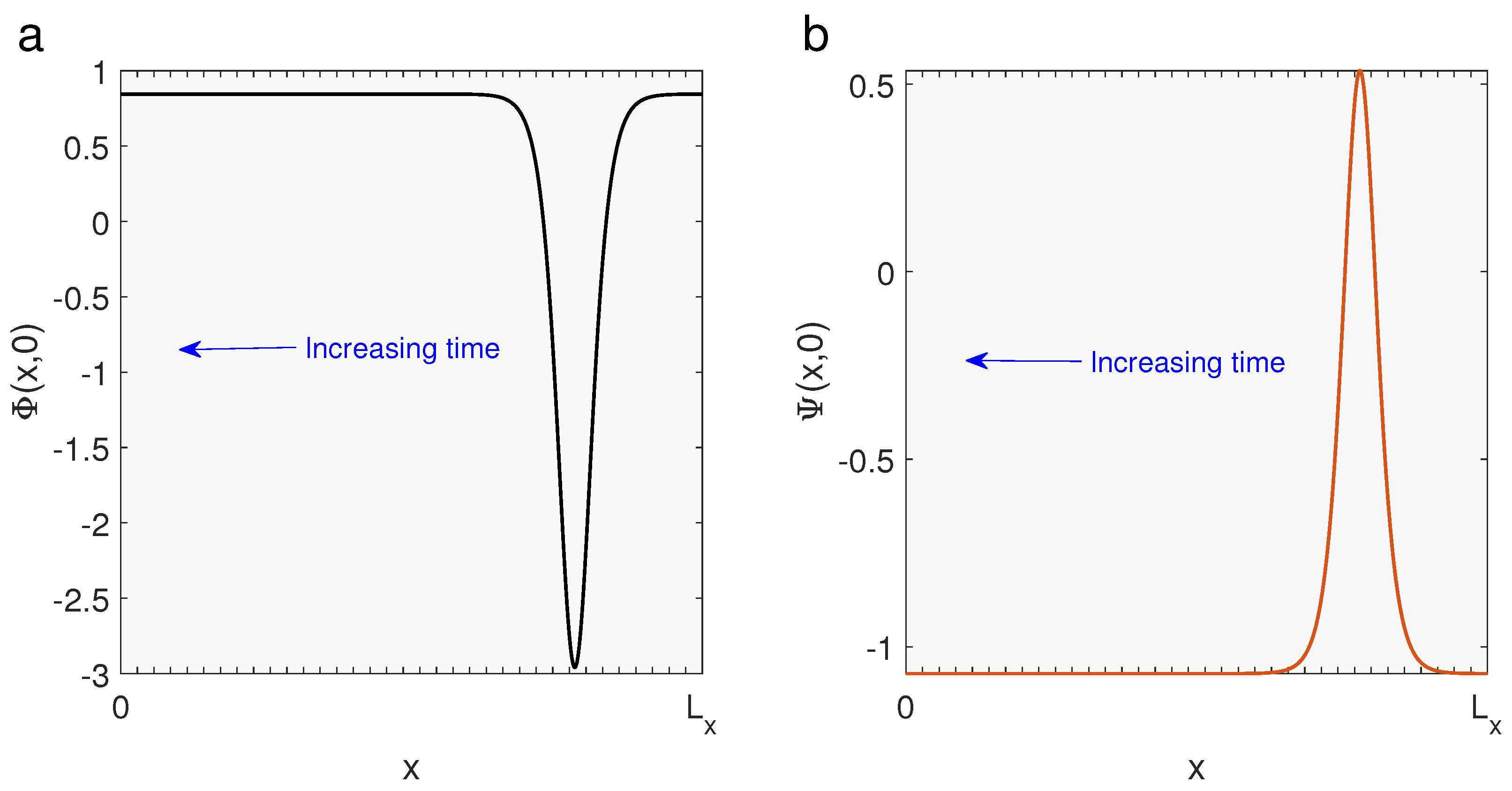
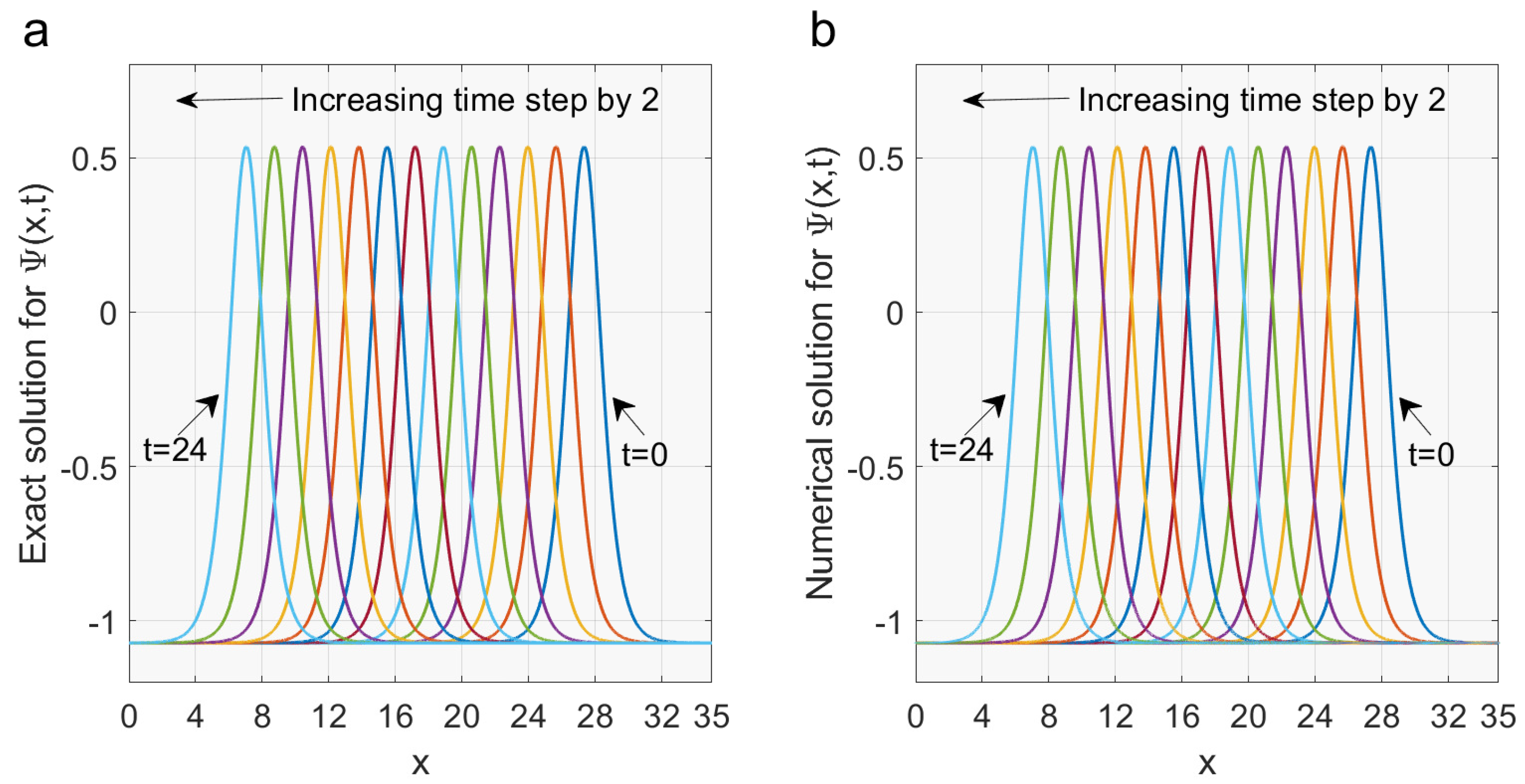
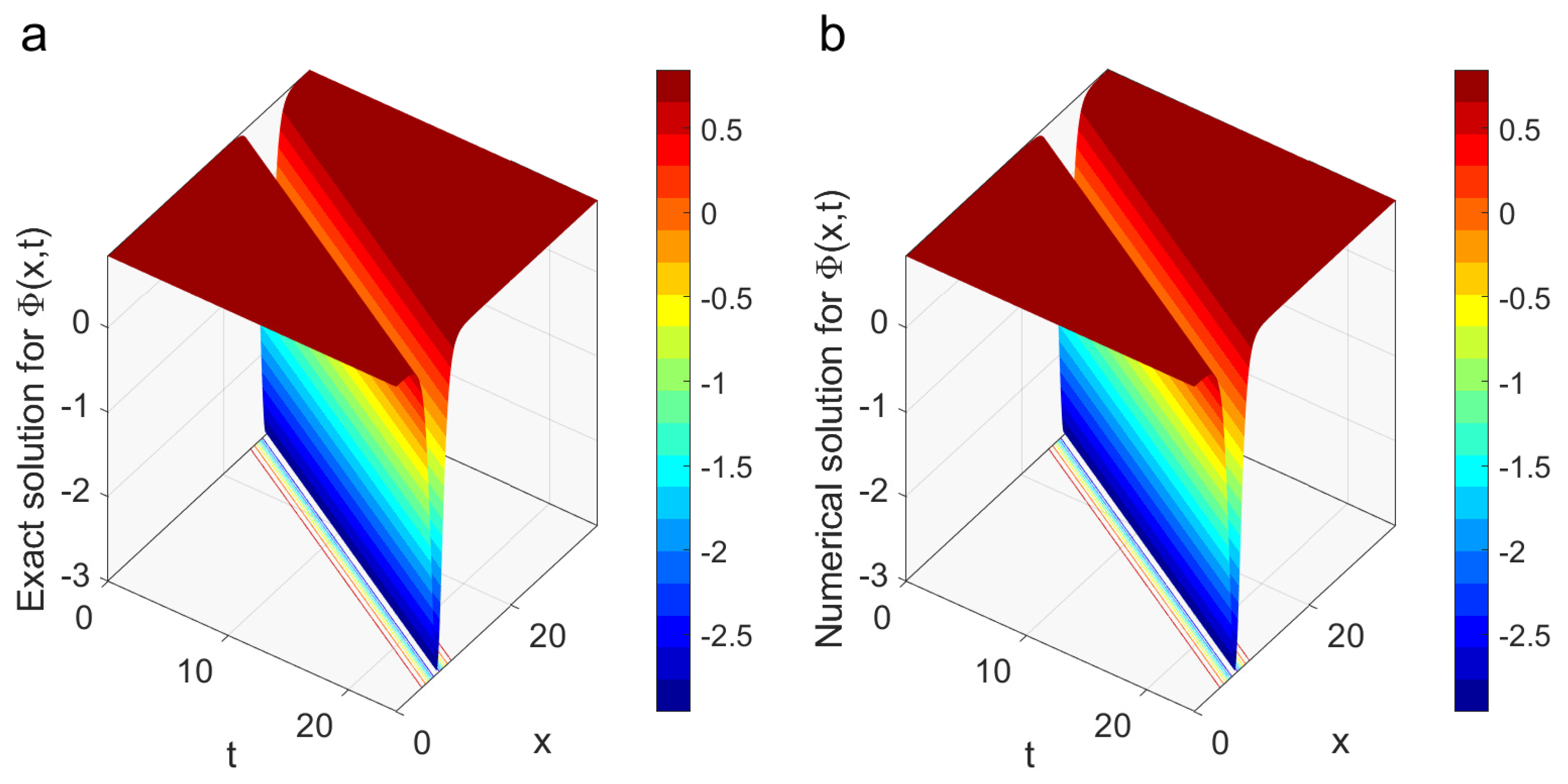
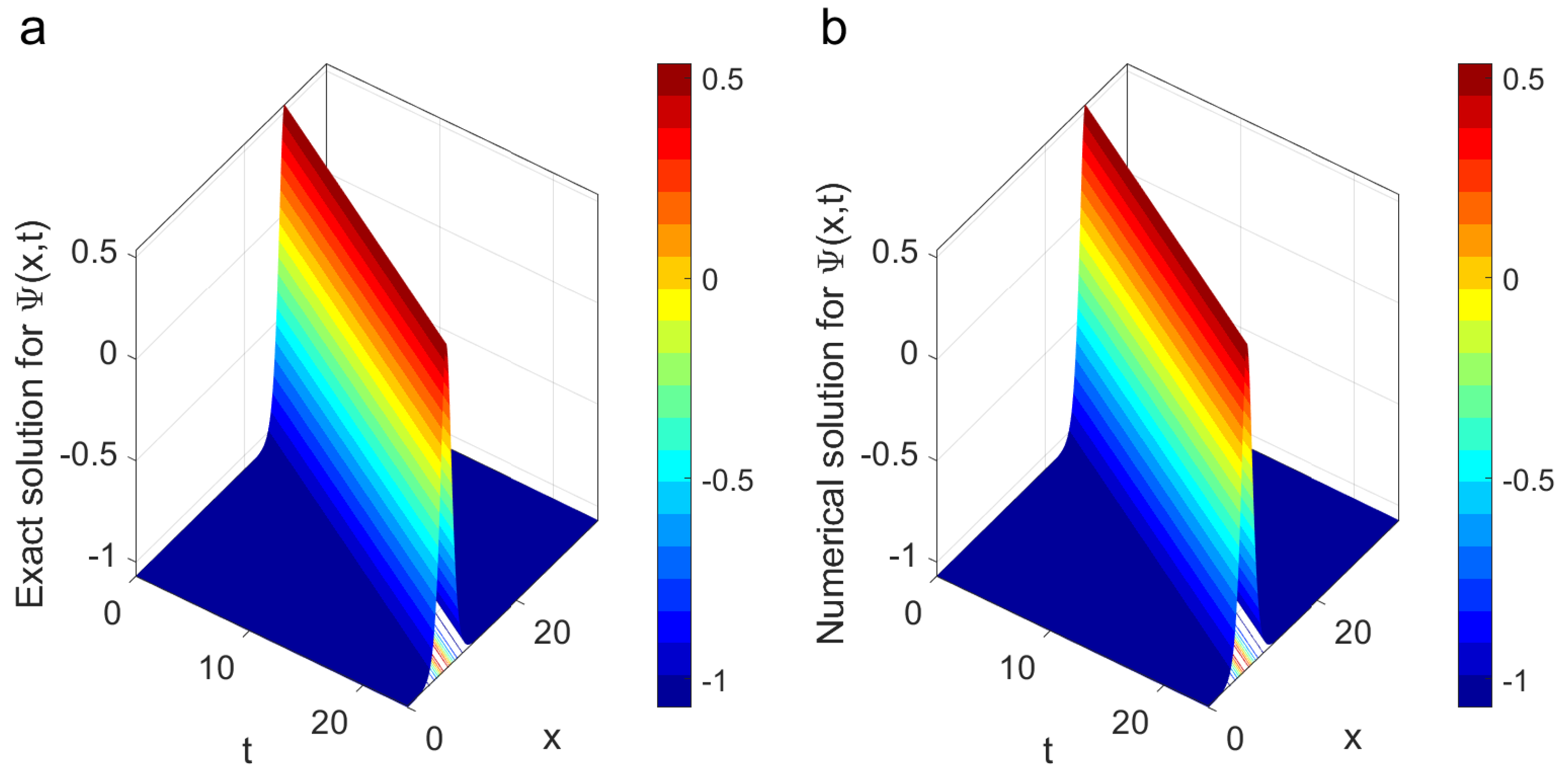
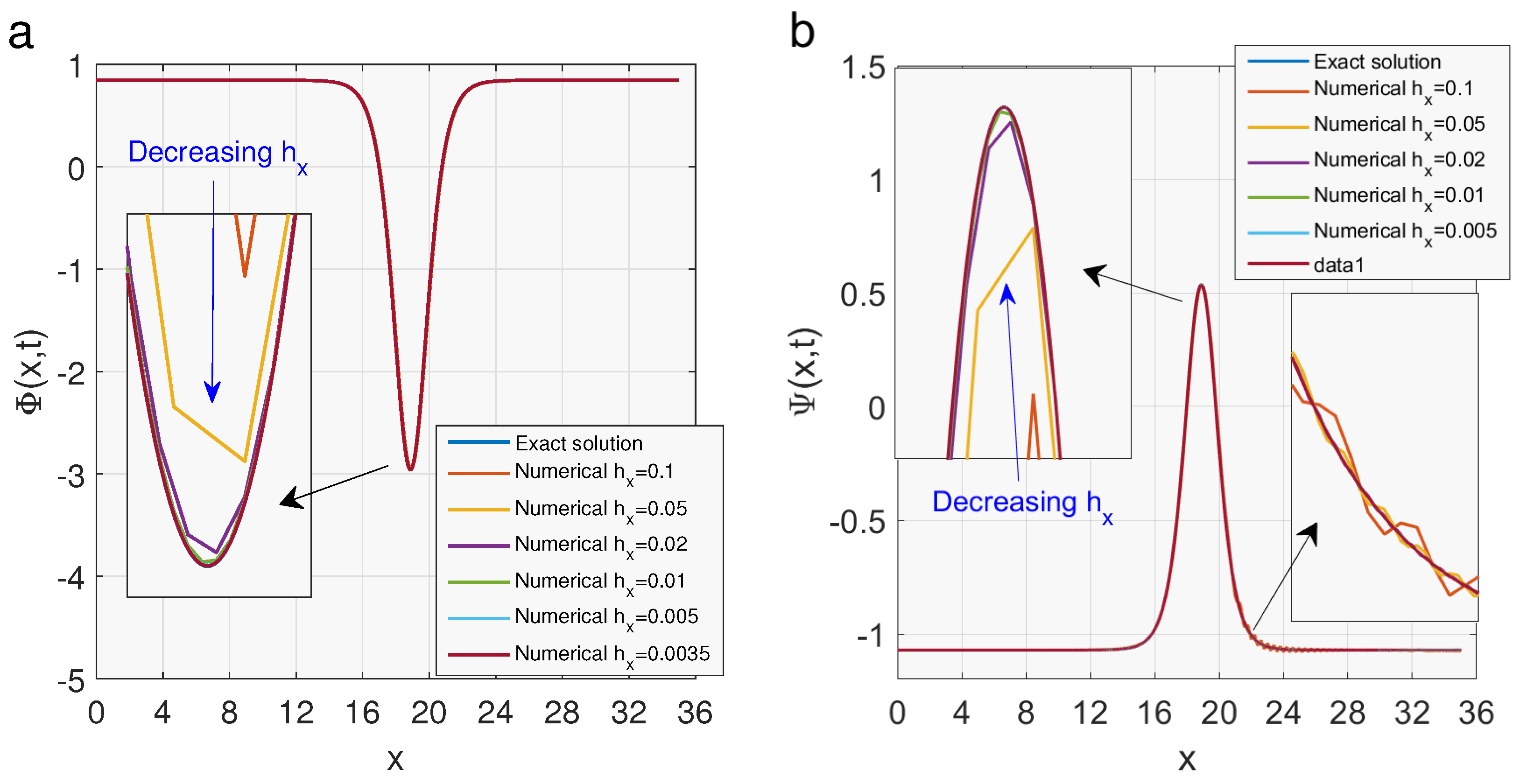
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Jacobi Elliptic Function Solutions
- If and Then,
- If and Then,
- If and Then,
- If and Then,
- If and Then,
- If and Then,
The Modulus of the Jacobi Elliptic Function
Appendix B. Space Discretization
References
- Wang, M.L.; Li, X.Z. Extended F-expansion and periodic wave solutions for the generalized Zakharov equations. Phys. Lett. A 2005, 343, 48–54. [Google Scholar] [CrossRef]
- Chen, G.; Xin, X.; Liu, H. The improved exp(-ϕ(η))-expansion method and new exact solutions of nonlinear evolution equations in mathematical physics. Adv. Math. Phys. 2019, 2019. [Google Scholar] [CrossRef]
- Conte, R.; Musette, M. Link between solitary waves and projective Riccati equations. J. Phys. A Math. 1992, 25, 5609–5623. [Google Scholar] [CrossRef]
- Chow, K.W. A class of exact periodic solutions of nonlinear envelope equation. J. Math. Phys. 1995, 36, 4125–4137. [Google Scholar] [CrossRef]
- Fan, E. Extended tanh-function method and its applications to nonlinear equations. Phys. Lett. A 2000, 277, 212–218. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The extended tanh method for abundant solitary wave solutions of nonlinear wave equations. Appl. Math. Comput. 2007, 187, 1131–1142. [Google Scholar] [CrossRef]
- Alam, M.N.; Tunc, C. An analytical method for solving exact solutions of the nonlinear Bogoyavlenskii equation and the nonlinear diffusive predator-prey system. Alex Eng. J. 2016, 55, 1855–1865. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A. Application of exp(-φ(ζ))-expansion method to find the exact solutions of modified Benjamin-Bona-Mahony equation. World Appl. Sci. J. 2013, 24, 1373–1377. [Google Scholar]
- Khan, K.; Akbar, M.A. Exact traveling wave solutions of Kadomtsev-Petviashvili equation. J. Egypt Math. Soc. 2015, 23, 278–281. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Exact solutions to the double sinh-Gordon equation by the tanh method and a variable separated ODE. method. Comput. Math. Appl. 2005, 50, 1685–1696. [Google Scholar] [CrossRef]
- Wazwaz, A.M. A sine–cosine method for handling nonlinear wave equations. Math. Comput. Model. 2004, 40, 499–508. [Google Scholar] [CrossRef]
- Adomain, G. Solving Frontier Problems of Physics: The Decomposition Method; Kluwer Academic Publishers: Boston, MA, USA, 1994. [Google Scholar]
- Wazwaz, A.M. Partial Differential Equations: Method and Applications; Taylor and Francis: Abingdon, UK, 2002. [Google Scholar]
- Hirota, R. Exact envelope soliton solutions of a nonlinear wave equation. J. Math. Phys. 1973, 14, 805–810. [Google Scholar] [CrossRef]
- Hirota, R.; Satsuma, J. Soliton solution of a coupled KdV equation. Phys. Lett. A 1981, 85, 407–408. [Google Scholar] [CrossRef]
- Ablowitz, M.J.; Clarkson, P.A. Solitons, Non-Linear Evolution Equations and Inverse Scattering Transform; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Huang, W.; Russell, R.D. The Adaptive Moving Mesh Methods; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Budd, C.J.; Huang, W. Russell, R.D. Adaptivity with moving grids. Acta Numer. 2009, 18, 111–241. [Google Scholar] [CrossRef]
- Alharbi, A.R.; Almatrafi, M.B.; Abdelrahman, M.A.E. The Extended Jacobian Elliptic Function Expansion Approach to the Generalized Fifth Order KdV Equation. J. Phy. Math. 2019, 10, 310. [Google Scholar]
- Alharbi, A.R.; Almatrafi, M.B. Numerical investigation of the Dispersive Long Wave Equation using an adaptive moving mesh method and its stability. Results Phys. 2020, 16, 102870. [Google Scholar] [CrossRef]
- Alharbi, A.R.; Almatrafi, M.B. Riccati-Bernoulli Sub-ODE approach on the partial differential equations and applications. Int. J. Math. Comput. Sci. 2020, 15, 367–388. [Google Scholar]
- Abdelrahman, M.A.E.; Almatrafi, M.B.; Alharbi, A. Fundamental solutions for the coupled KdV system and its stability. Symmetry 2020, 12, 429. [Google Scholar] [CrossRef]
- Alharbi, A.; Abdelrahman, M.A.E.; Almatrafi, M.B. Analytical and numerical investigation for the DMBBM equation. Comput. Model. Eng. Sci. 2020, 122, 743–756. [Google Scholar] [CrossRef]
- Alam, M.N.; Tunç, C. Constructions of the optical solitons and other solitons to the conformable fractional Zakharov-Kuznetsov equation with power law nonlinearity. J. Taibah Univ. Sci. 2019, 14, 94–100. [Google Scholar] [CrossRef]
- Shahida, N. Tunç, C. Resolution of coincident factors in altering the flow dynamics of an MHD elastoviscous fluid past an unbounded upright channel. J. Taibah Univ. Sci. 2019, 13, 1022–1034. [Google Scholar] [CrossRef]
- Zhang, H.Q. Extended Jacobi elliptic function expansion method and its applications. Commun. Nonlinear Sci. Numer. Simul. 2007, 12, 627–635. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Al-Joudi, S. An Improved (G′/G)-expansion Method for Solving Nonlinear PDEs in Mathematical Physics. ICNAAM AIP Conf. Proc. 2010, 1281, 2220–2224. [Google Scholar]
- Ayub, K.; Saeed, M.; Ashraf, M.; Yaqub, M.; Hassan, M. Soliton solutions of Variant Boussinesq equations through Exp-function method. UW J. Sci. Technol. 2017, 1, 24–30. [Google Scholar]
- Alharbi, A.R.; Almatrafi, M.B. Analytical and numerical solutions for the variant Boussinseq equations. J. Taibah Univ. Sci. 2020, 14, 454–462. [Google Scholar] [CrossRef]
- Zheng, B. Application Of A generalized Bernoulli Sub-ODE method for finding traveling solutions of some nonlinear equations. Wseas Trans. Math. 2012, 11, 618–626. [Google Scholar]
- Patel, P.; Kumar, P.; Rajni. The Numerical Solution of Boussinesq Equation for Shallow Water Waves. AIP Conf. Proc. 2020, 2214, 020019. [Google Scholar] [CrossRef]

| Error for | Error for | CPU | |
|---|---|---|---|
| s | |||
| s | |||
| s | |||
| s | |||
| s | |||
| s |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alharbi, A.; Almatrafi, M.B. Exact and Numerical Solitary Wave Structures to the Variant Boussinesq System. Symmetry 2020, 12, 1473. https://doi.org/10.3390/sym12091473
Alharbi A, Almatrafi MB. Exact and Numerical Solitary Wave Structures to the Variant Boussinesq System. Symmetry. 2020; 12(9):1473. https://doi.org/10.3390/sym12091473
Chicago/Turabian StyleAlharbi, Abdulghani, and Mohammed B. Almatrafi. 2020. "Exact and Numerical Solitary Wave Structures to the Variant Boussinesq System" Symmetry 12, no. 9: 1473. https://doi.org/10.3390/sym12091473
APA StyleAlharbi, A., & Almatrafi, M. B. (2020). Exact and Numerical Solitary Wave Structures to the Variant Boussinesq System. Symmetry, 12(9), 1473. https://doi.org/10.3390/sym12091473






