Analysis of the Graovac–Pisanski Index of Some Polyhedral Graphs Based on Their Symmetry Group
Abstract
1. Introduction
2. Main Results
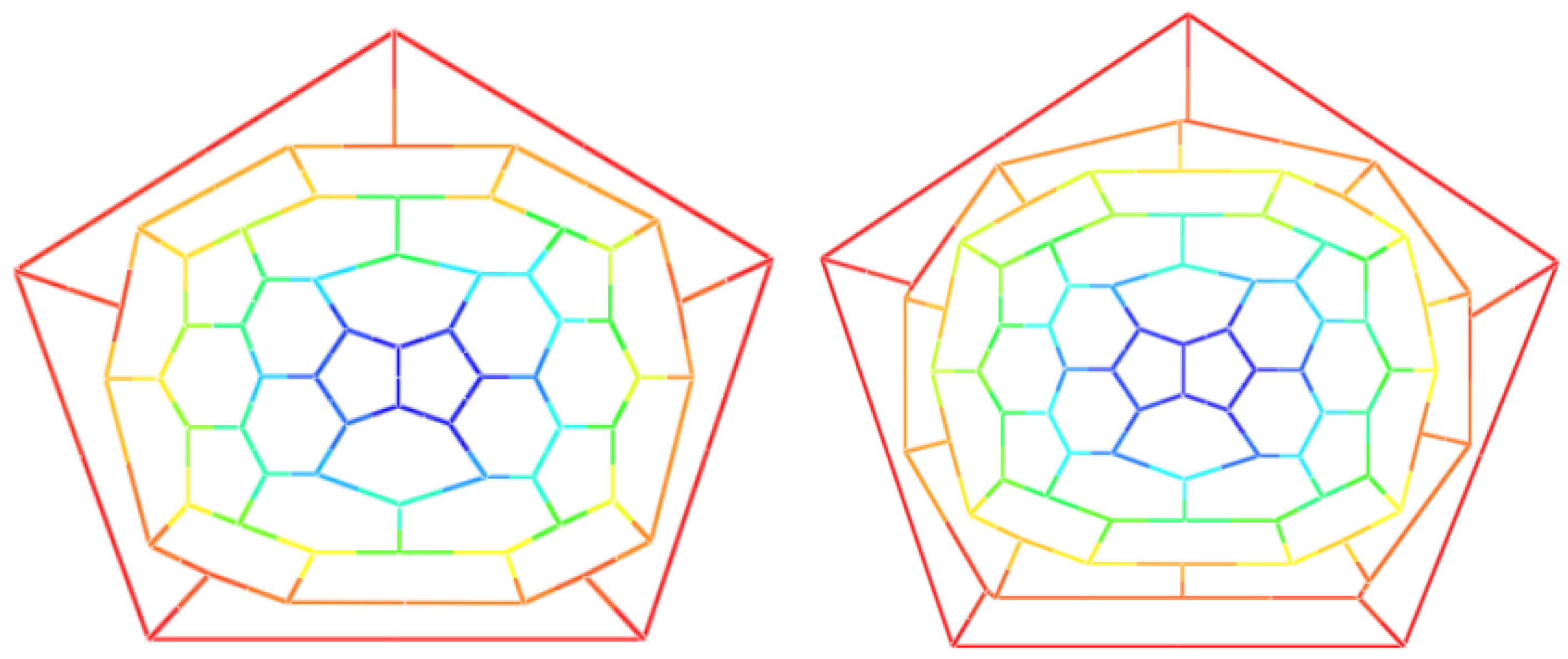
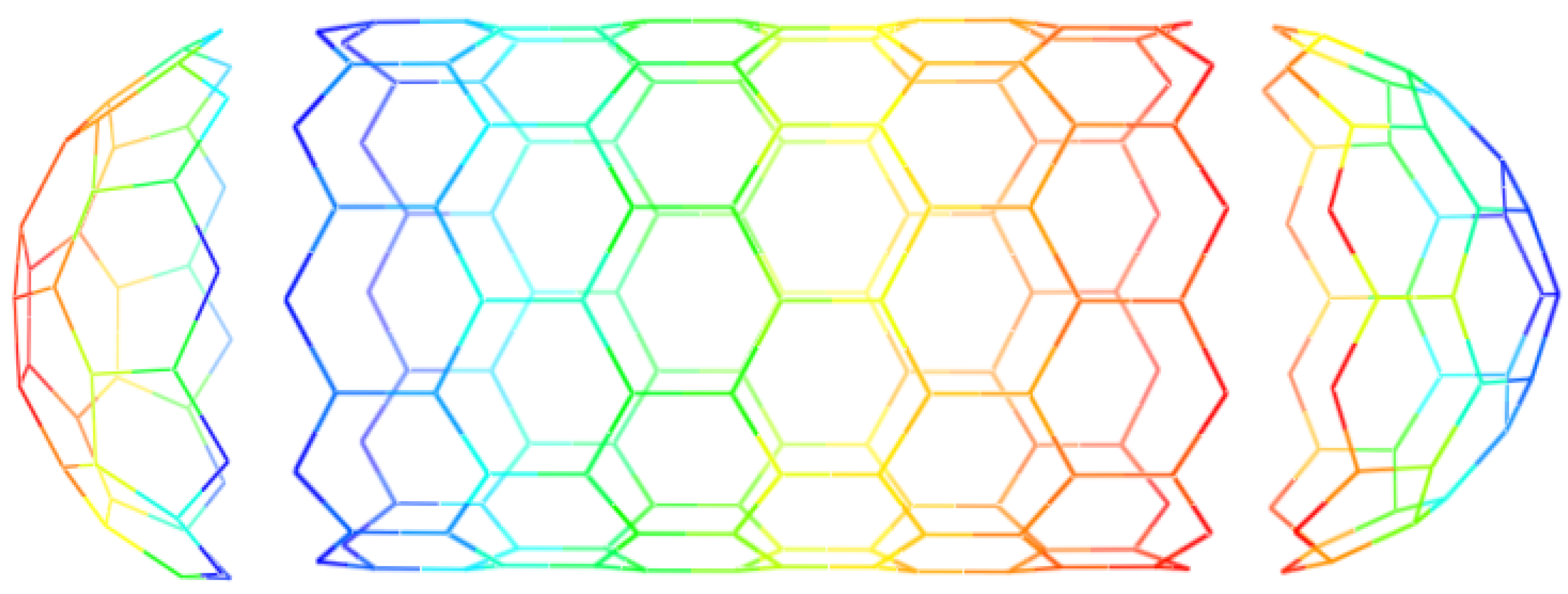
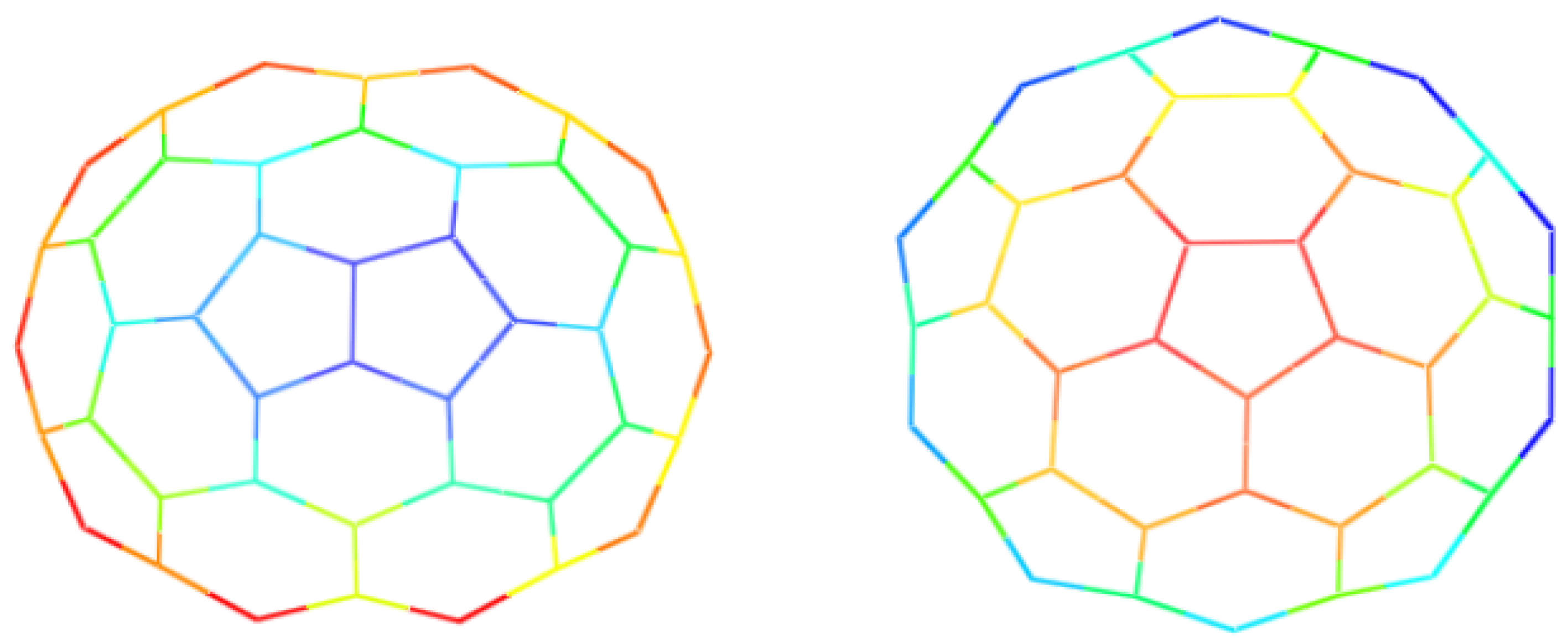
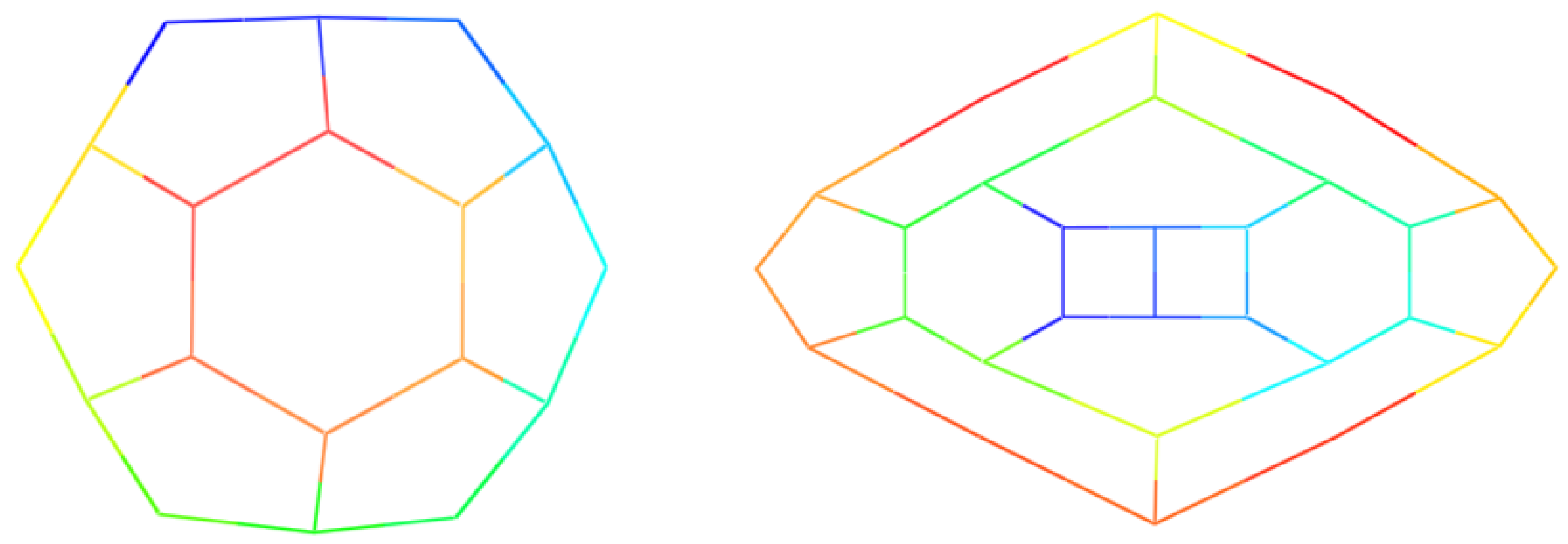
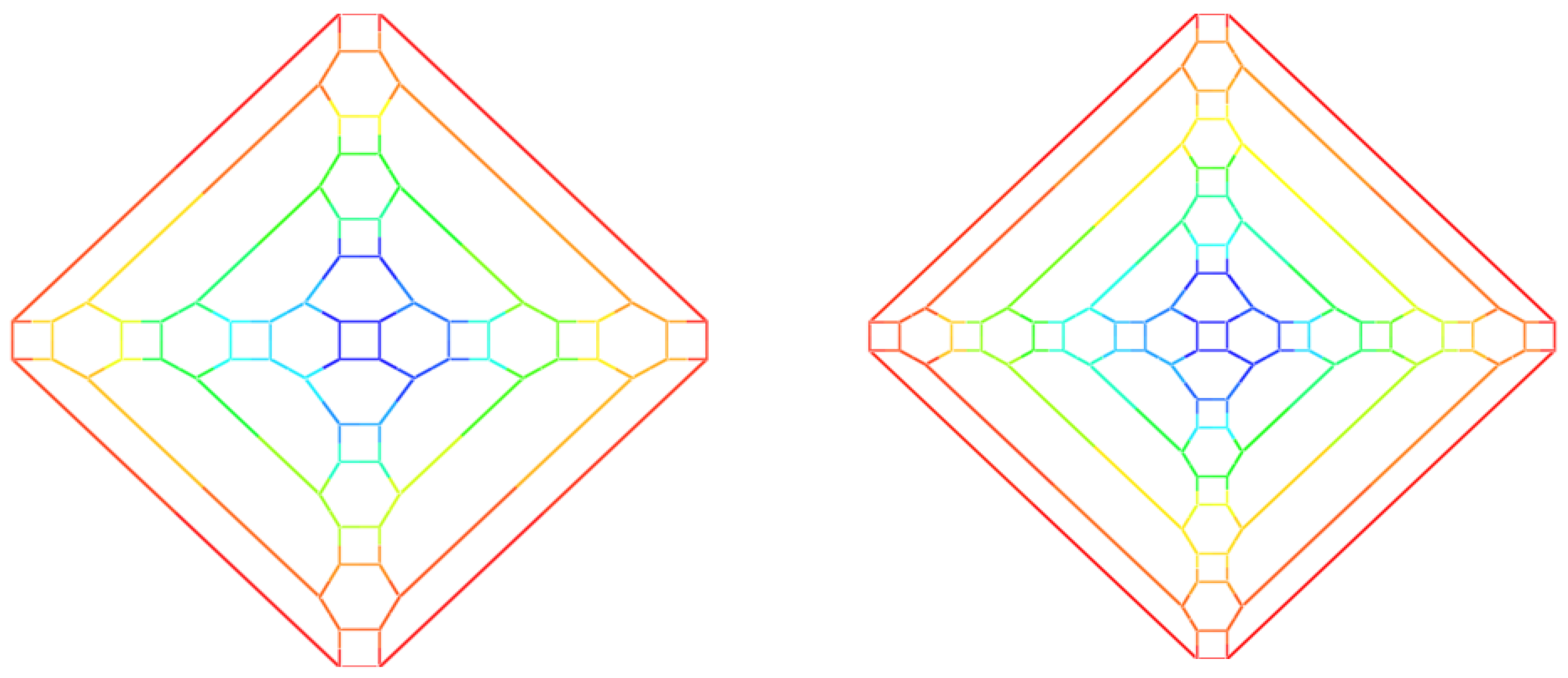
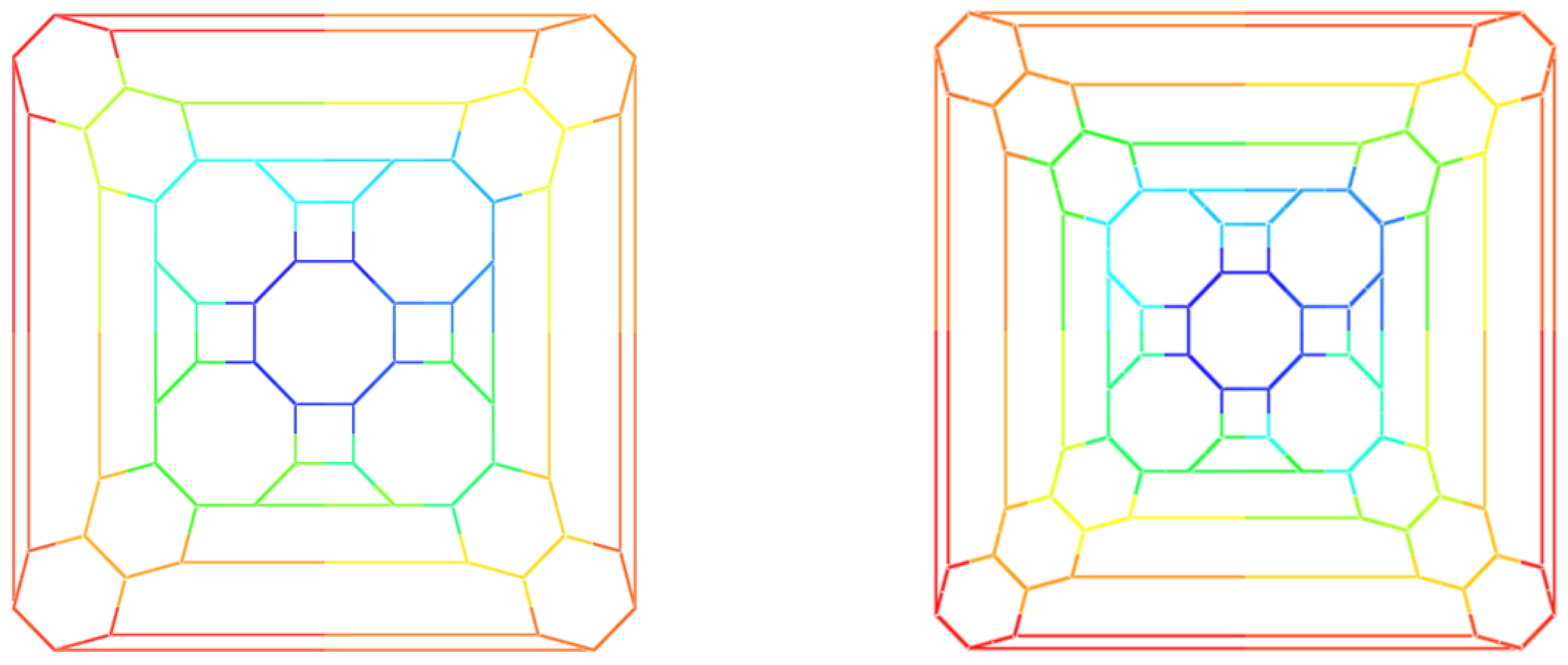
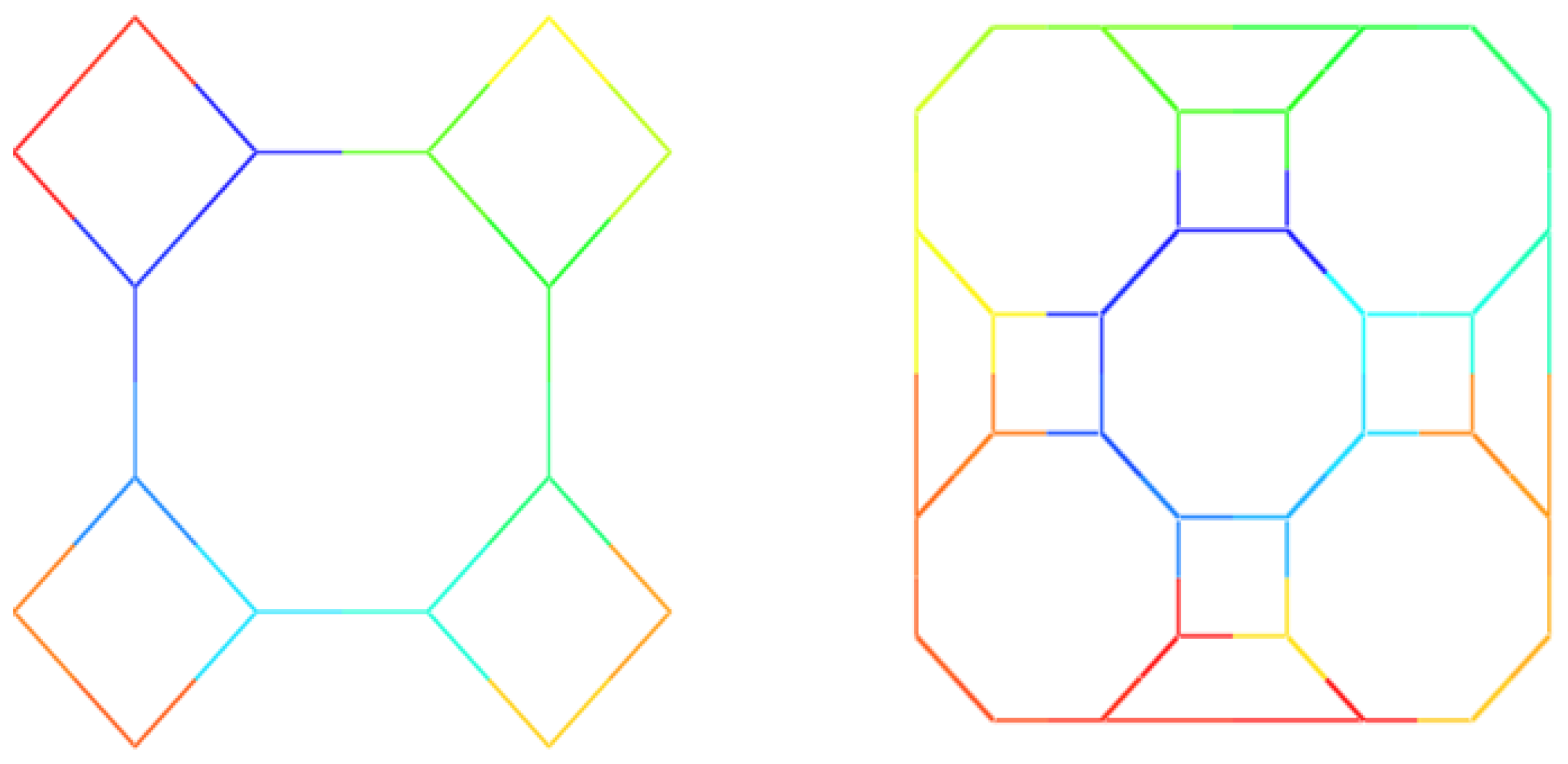
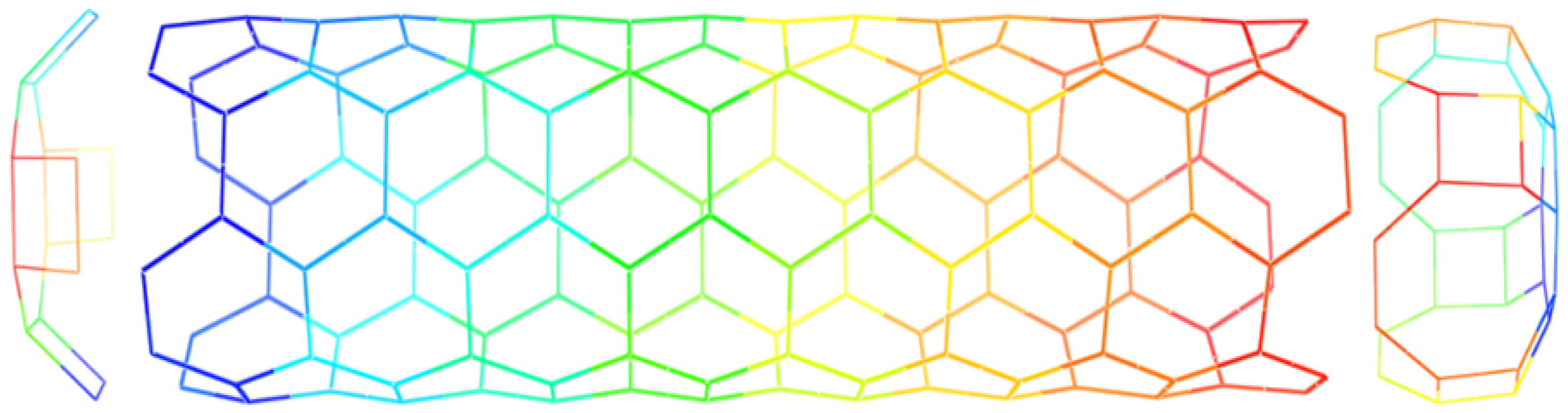
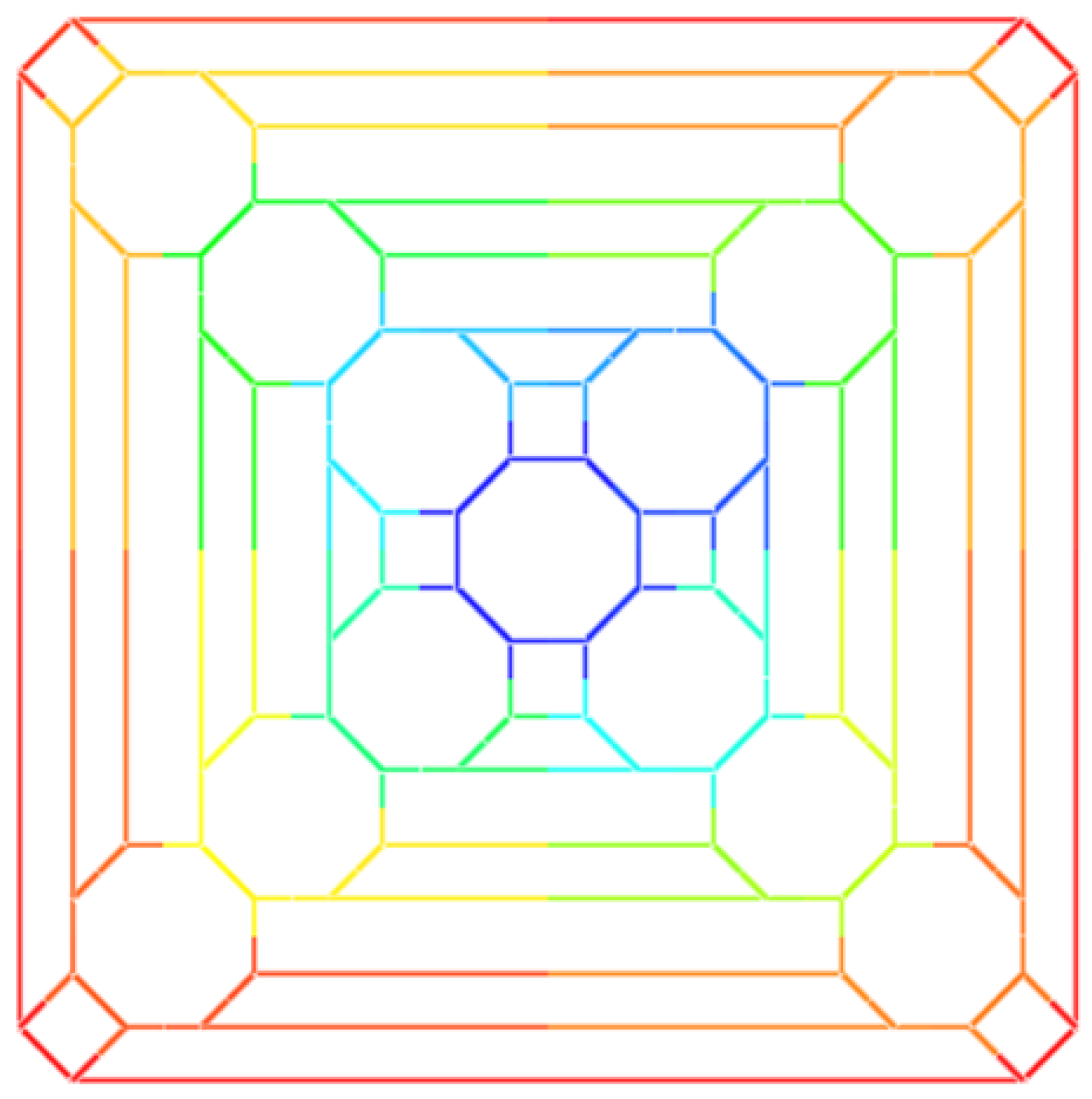
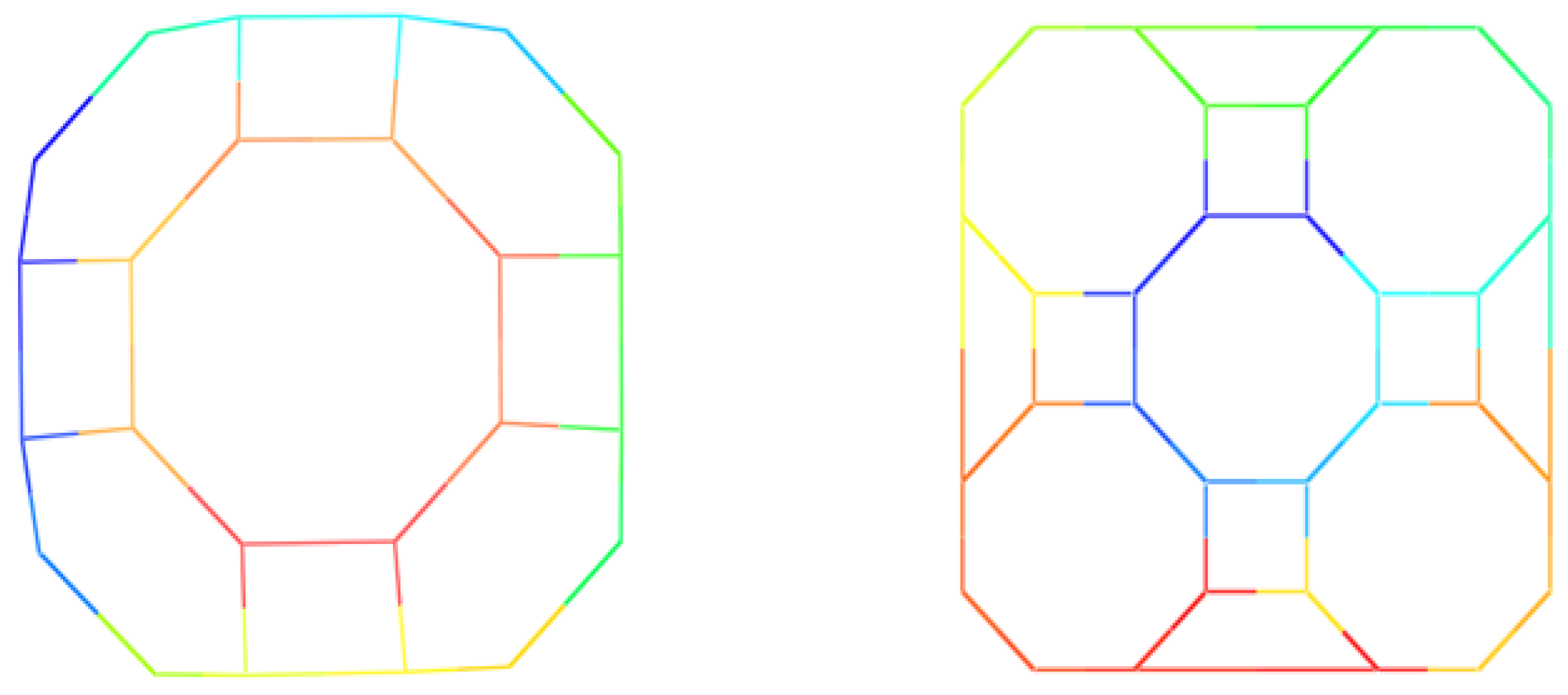
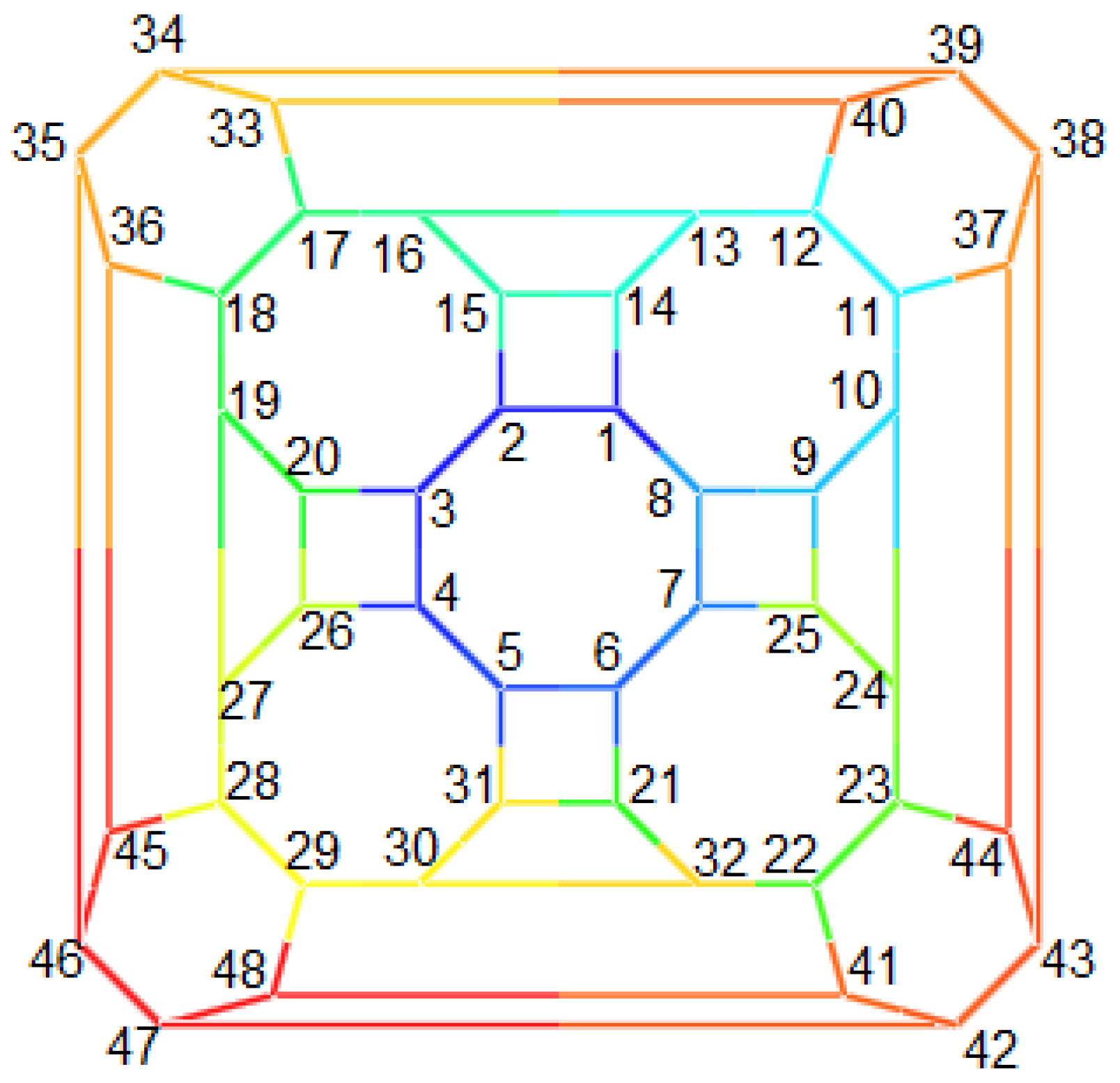
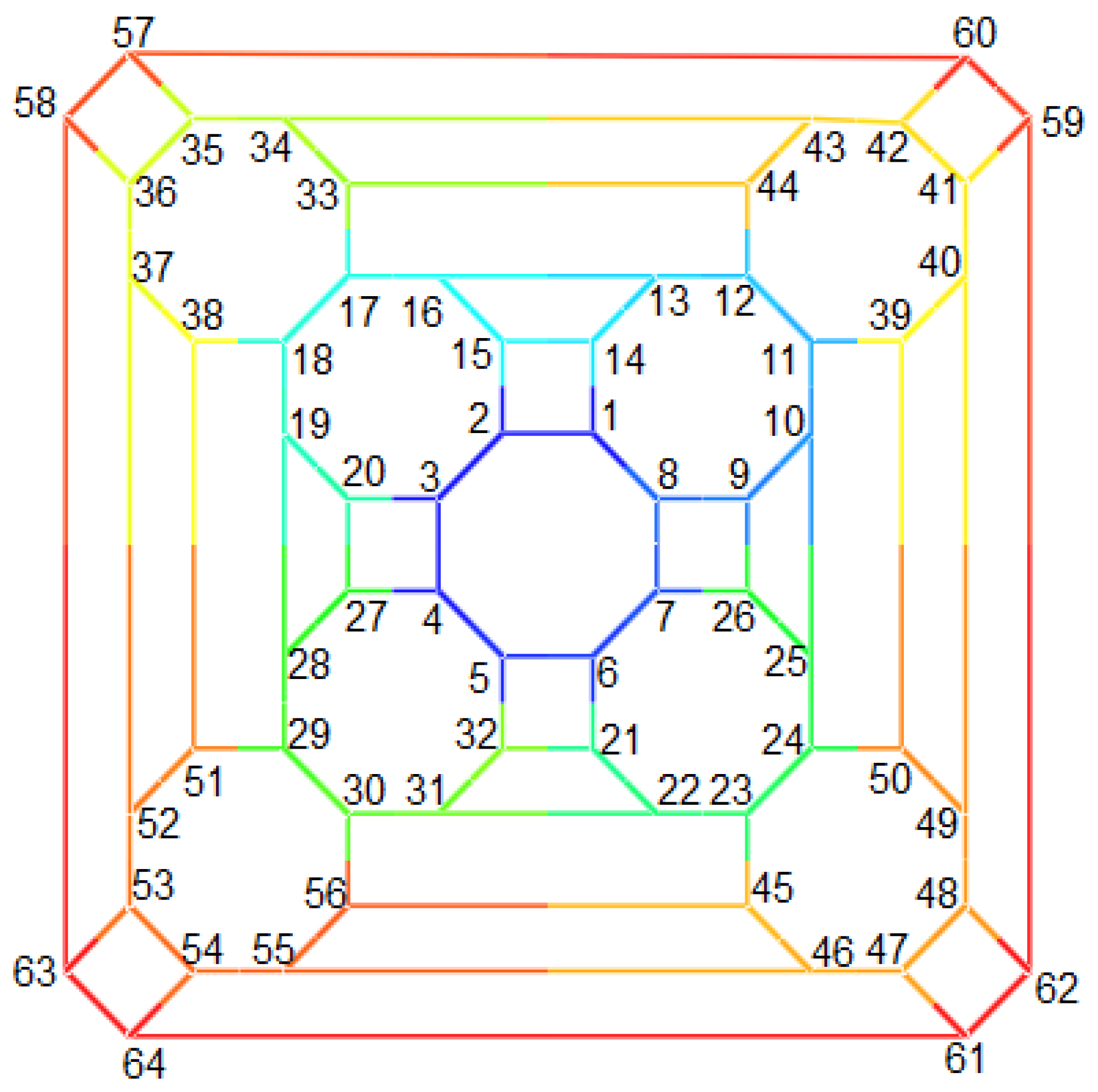
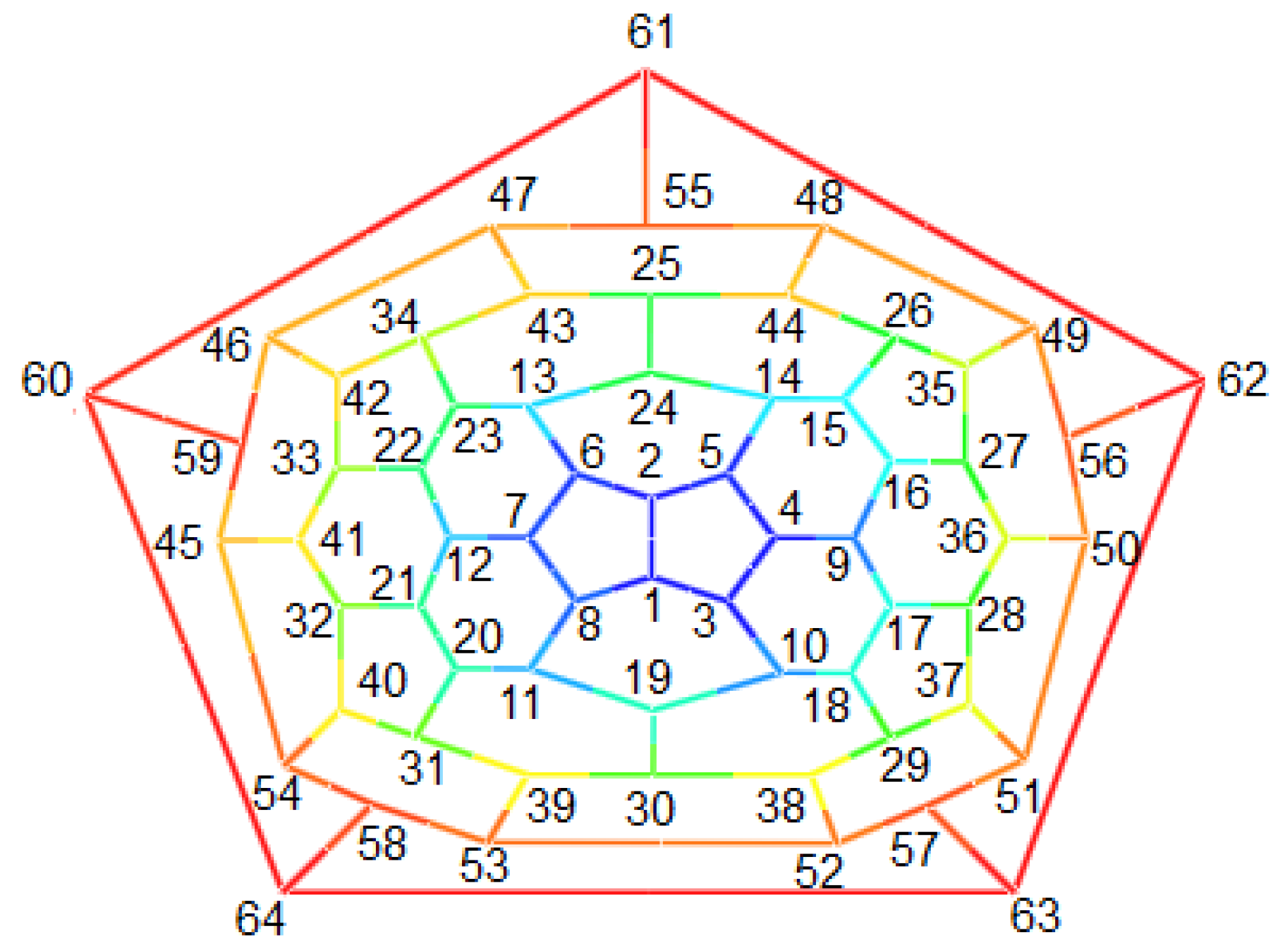
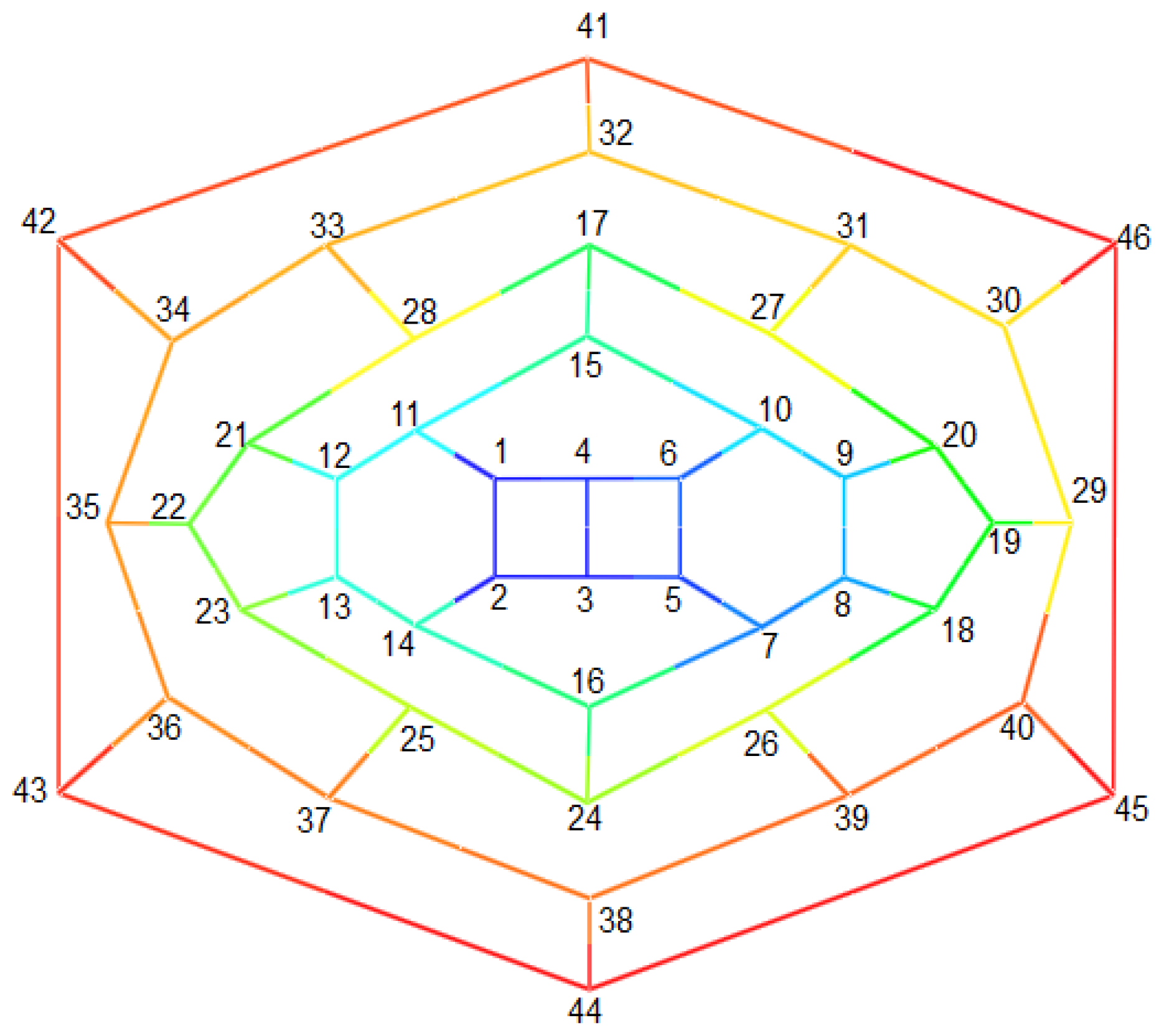
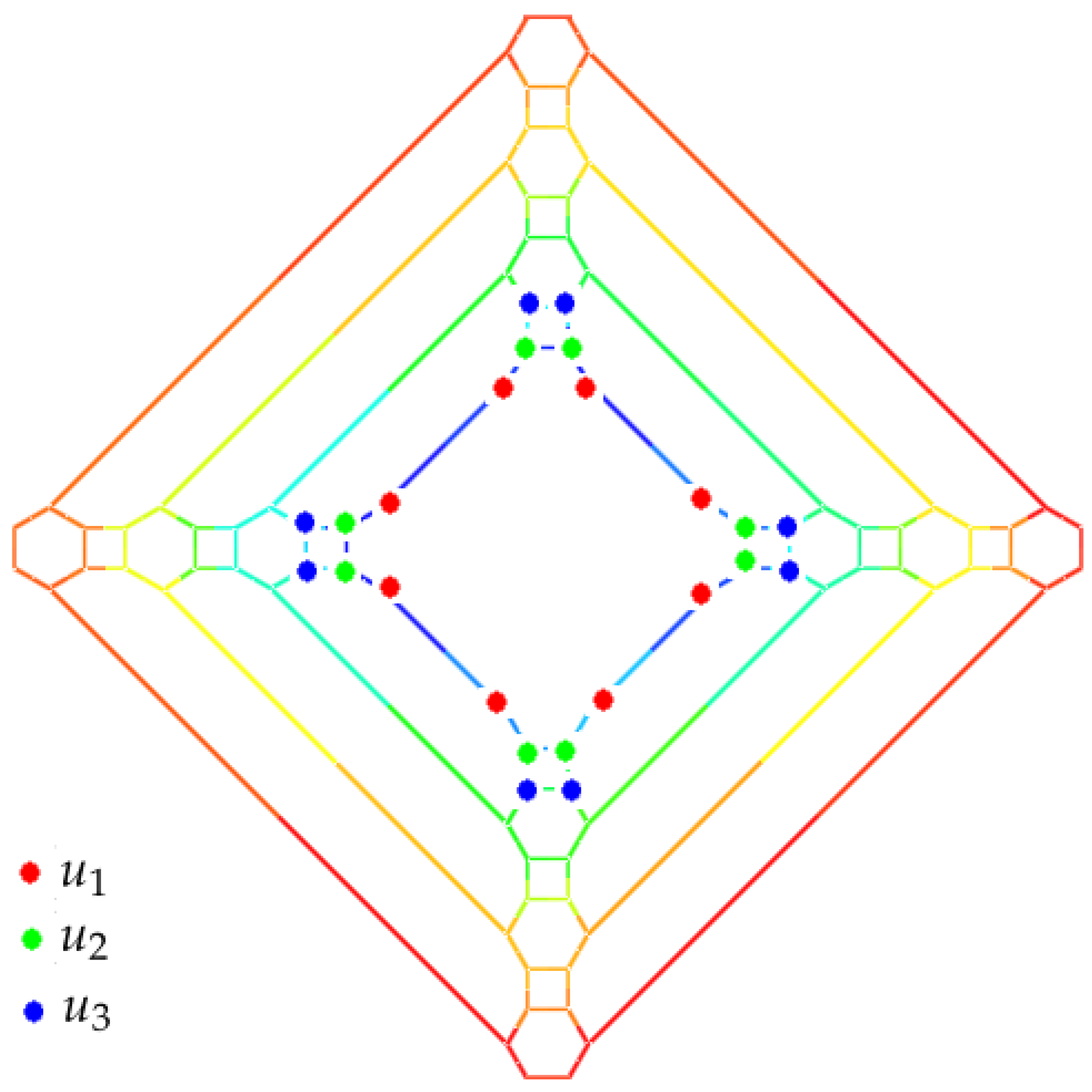
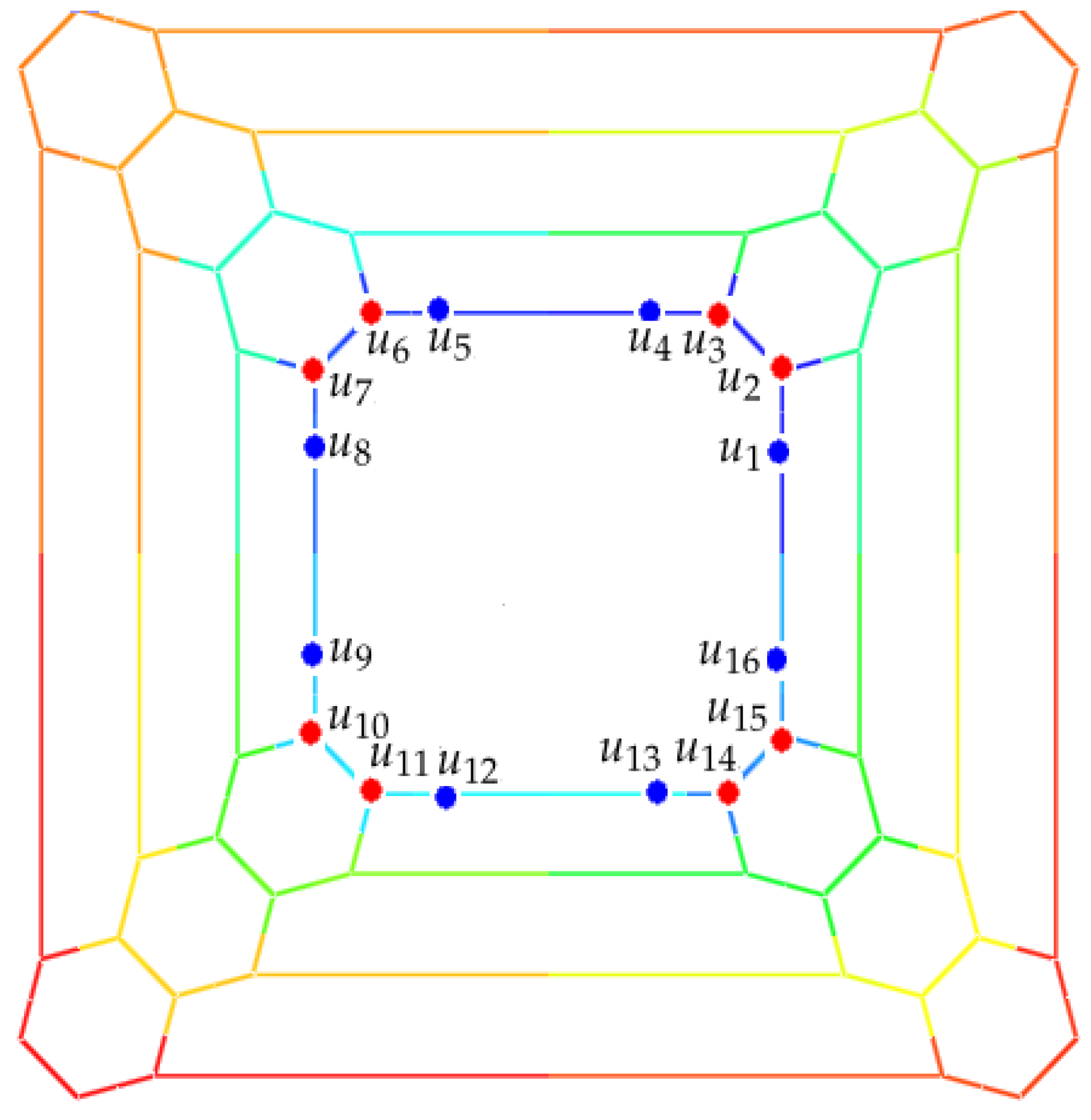
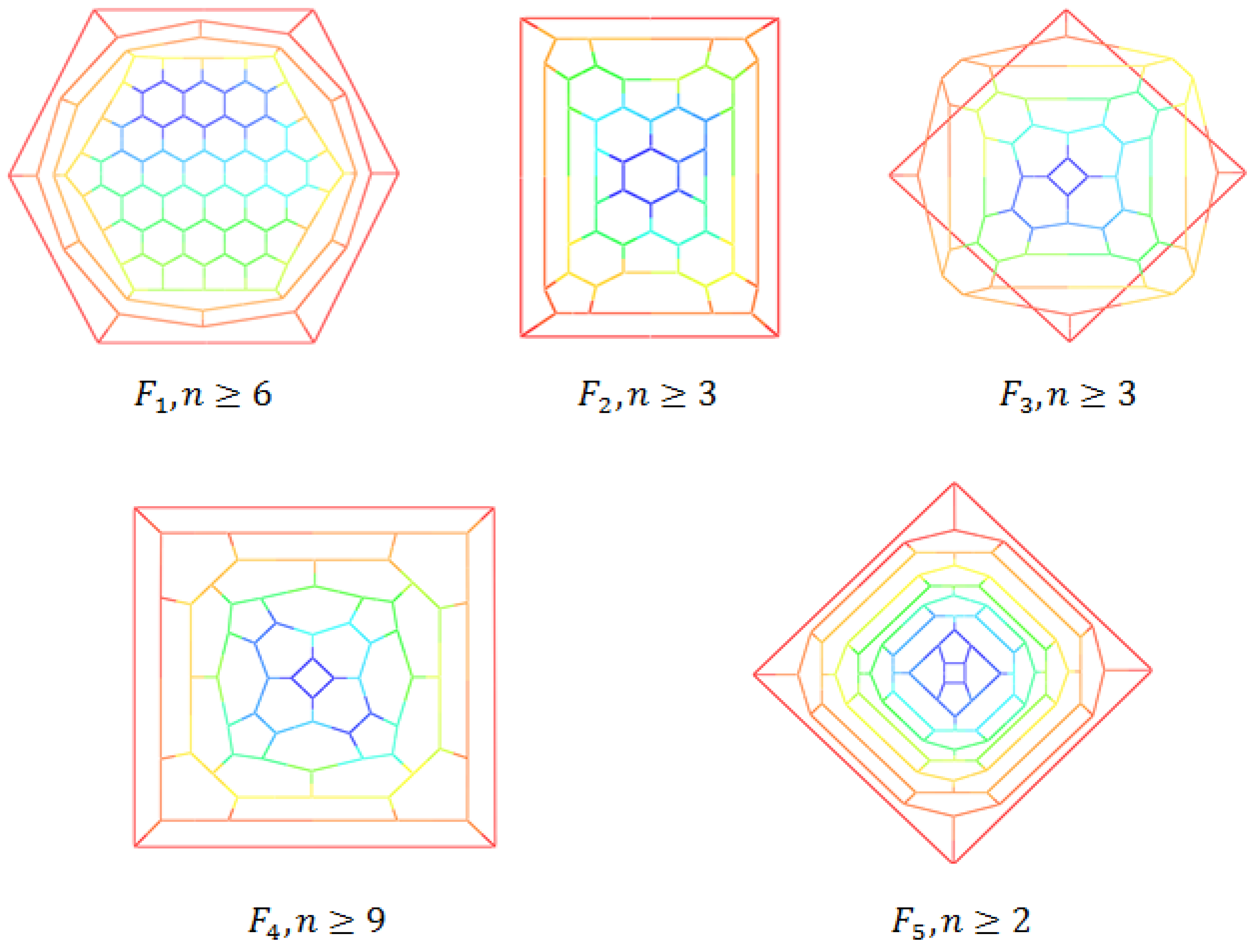
2.1. (4,5,6)-Cubic Polyhedral Graphs
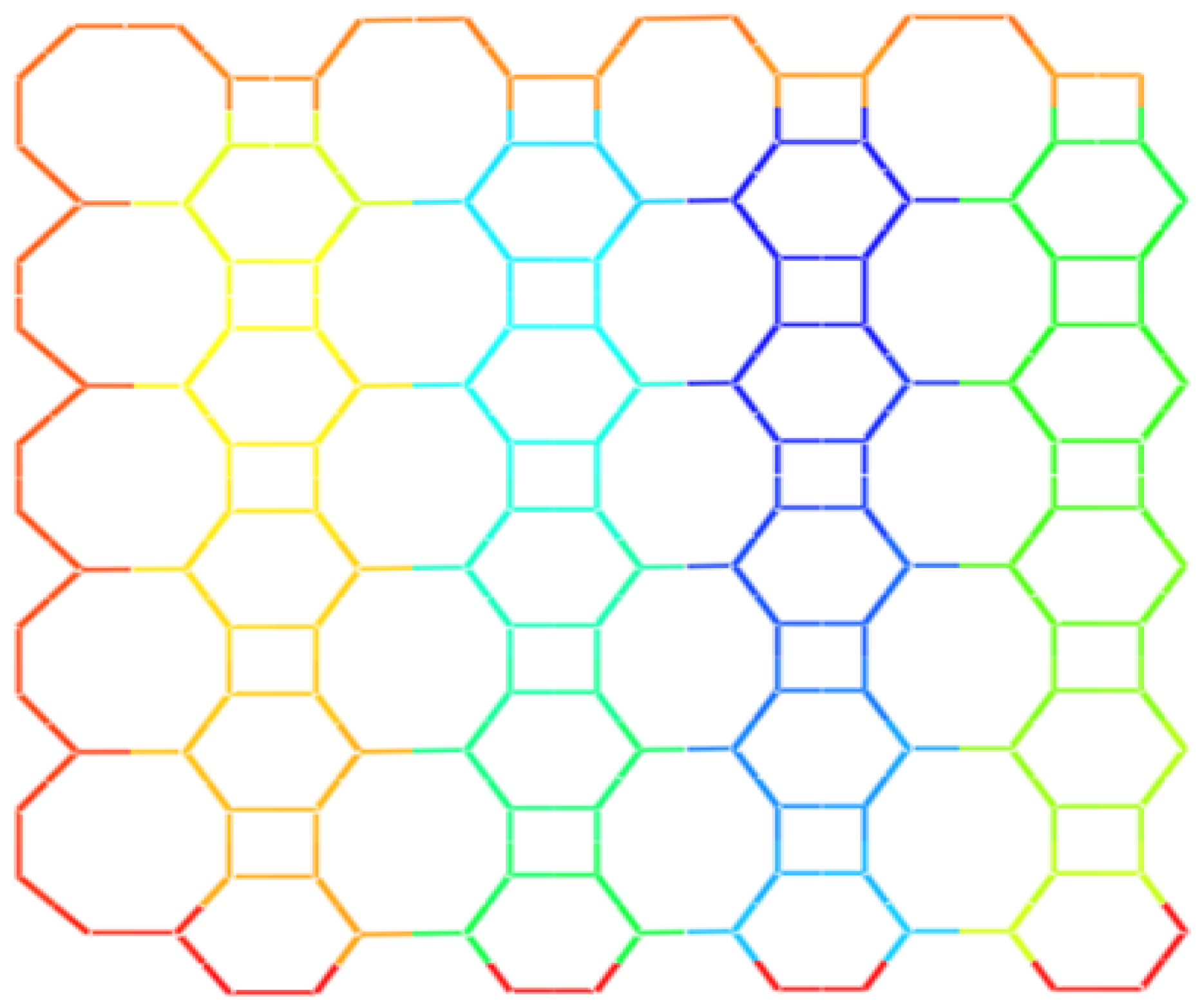
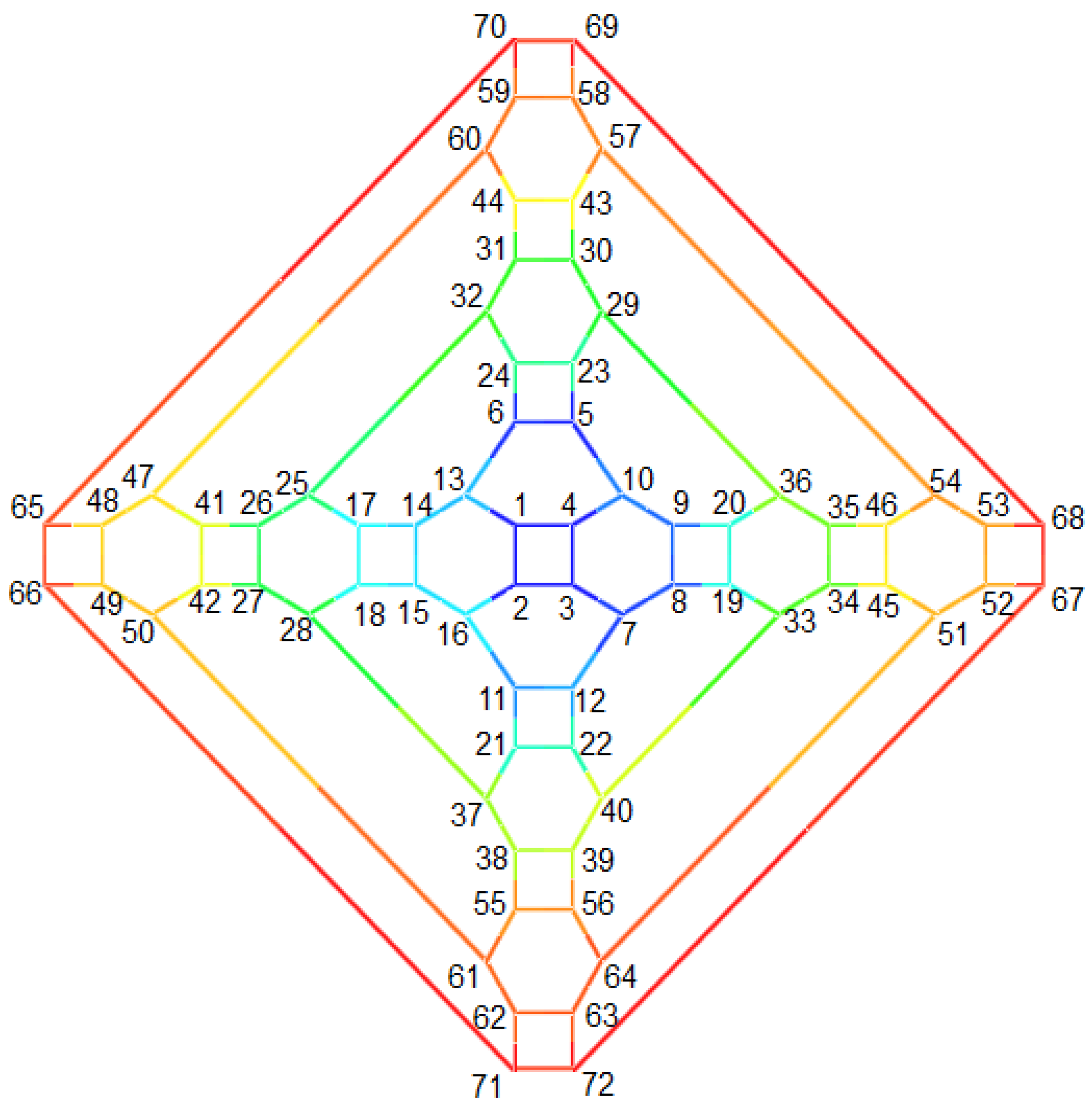
2.2. (4,6,7,8)-Cubic Polyhedral Graphs
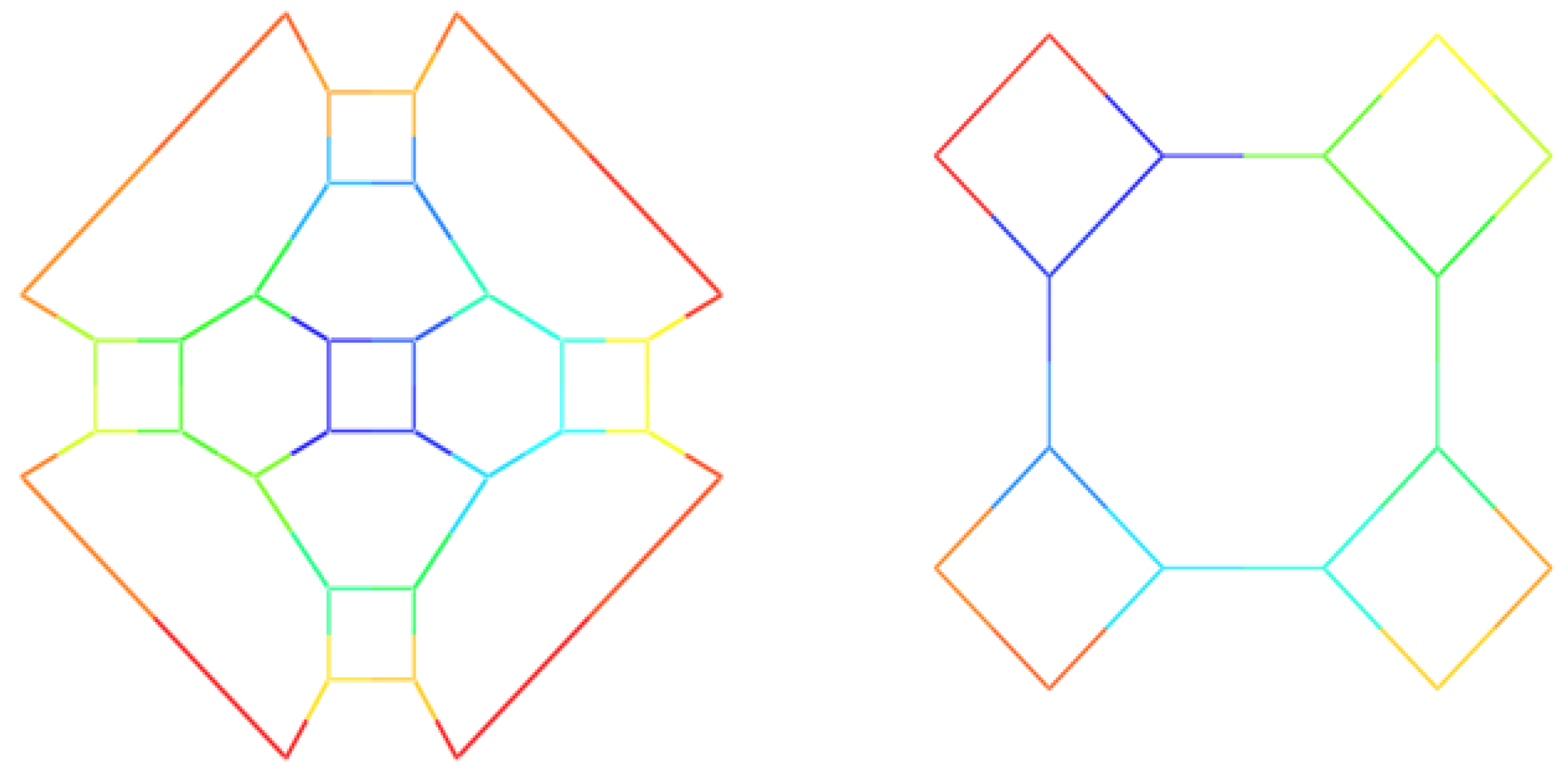
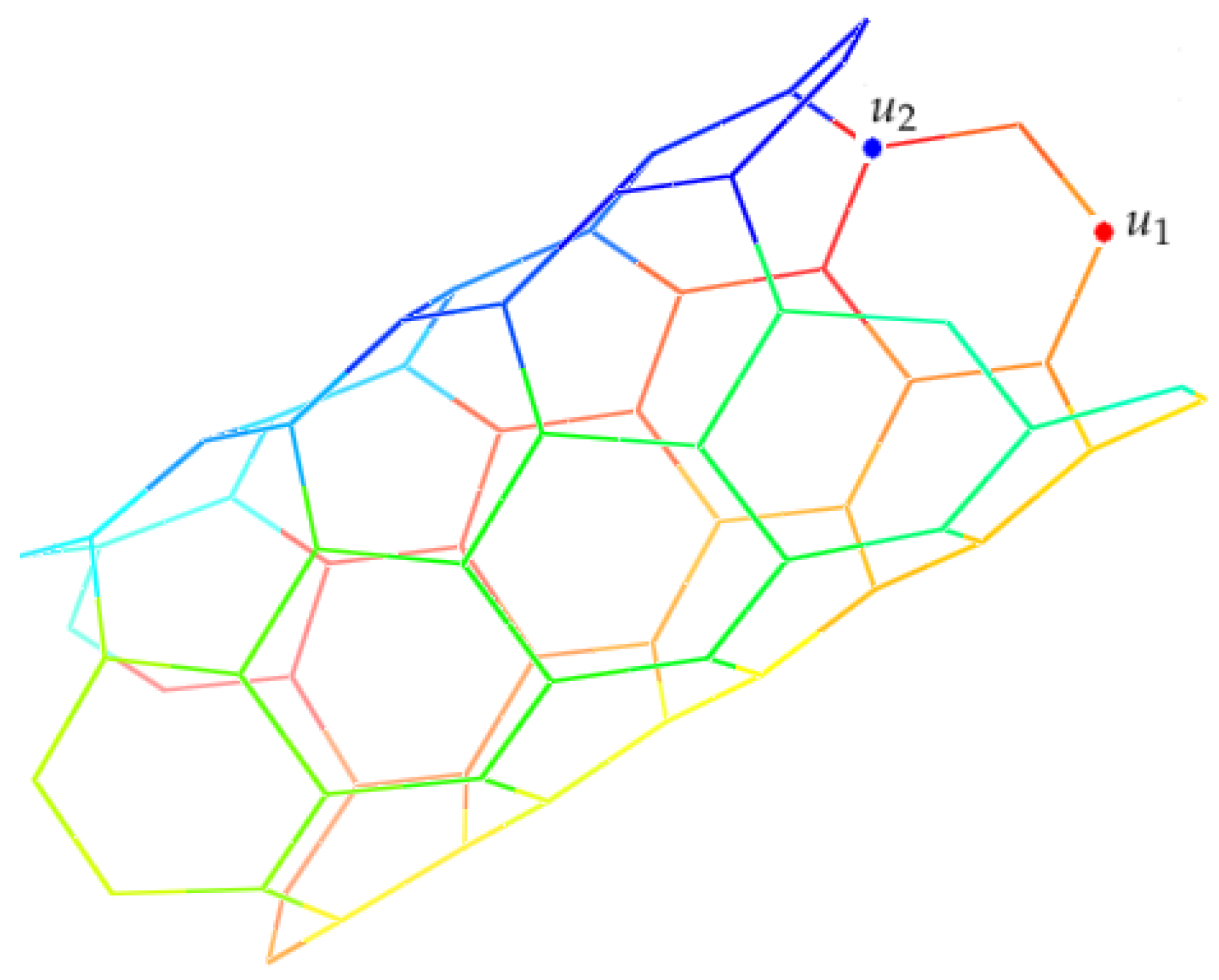
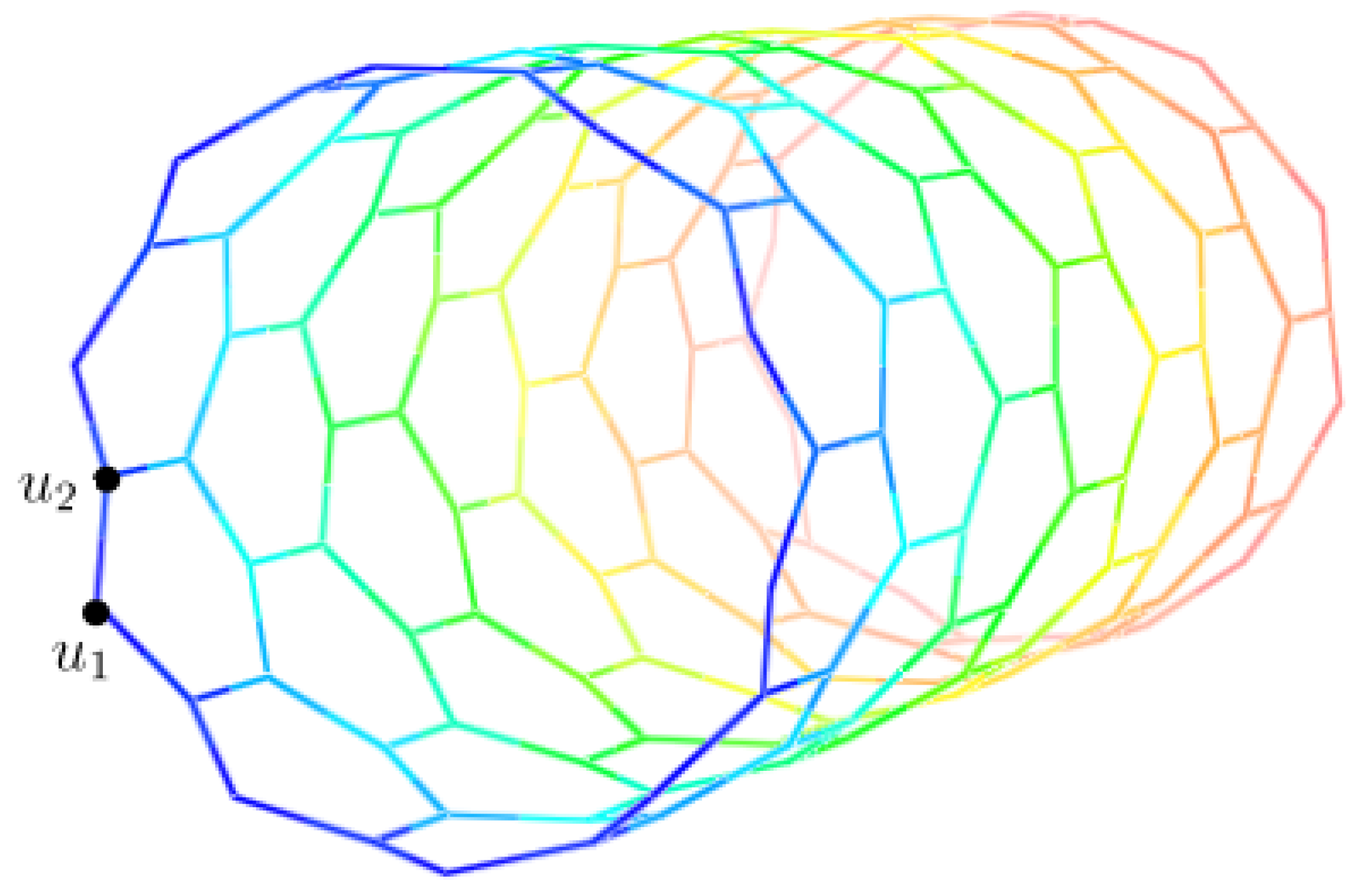
2.3. (4,6,8)-Cubic Polyhedral Graph
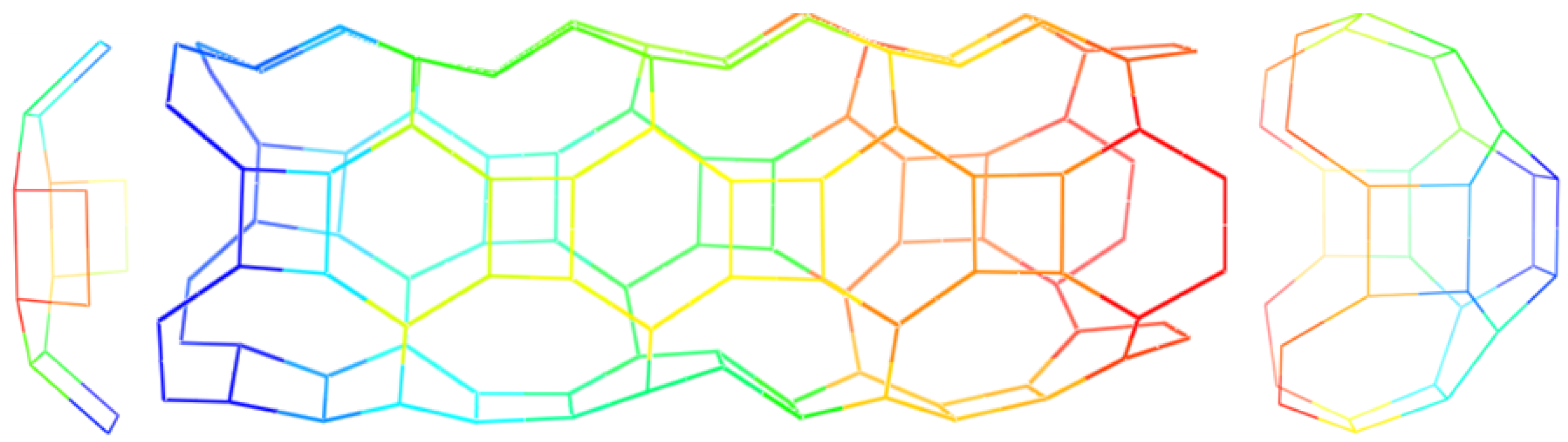
3. Symmetry Group of Polyhedral Graphs
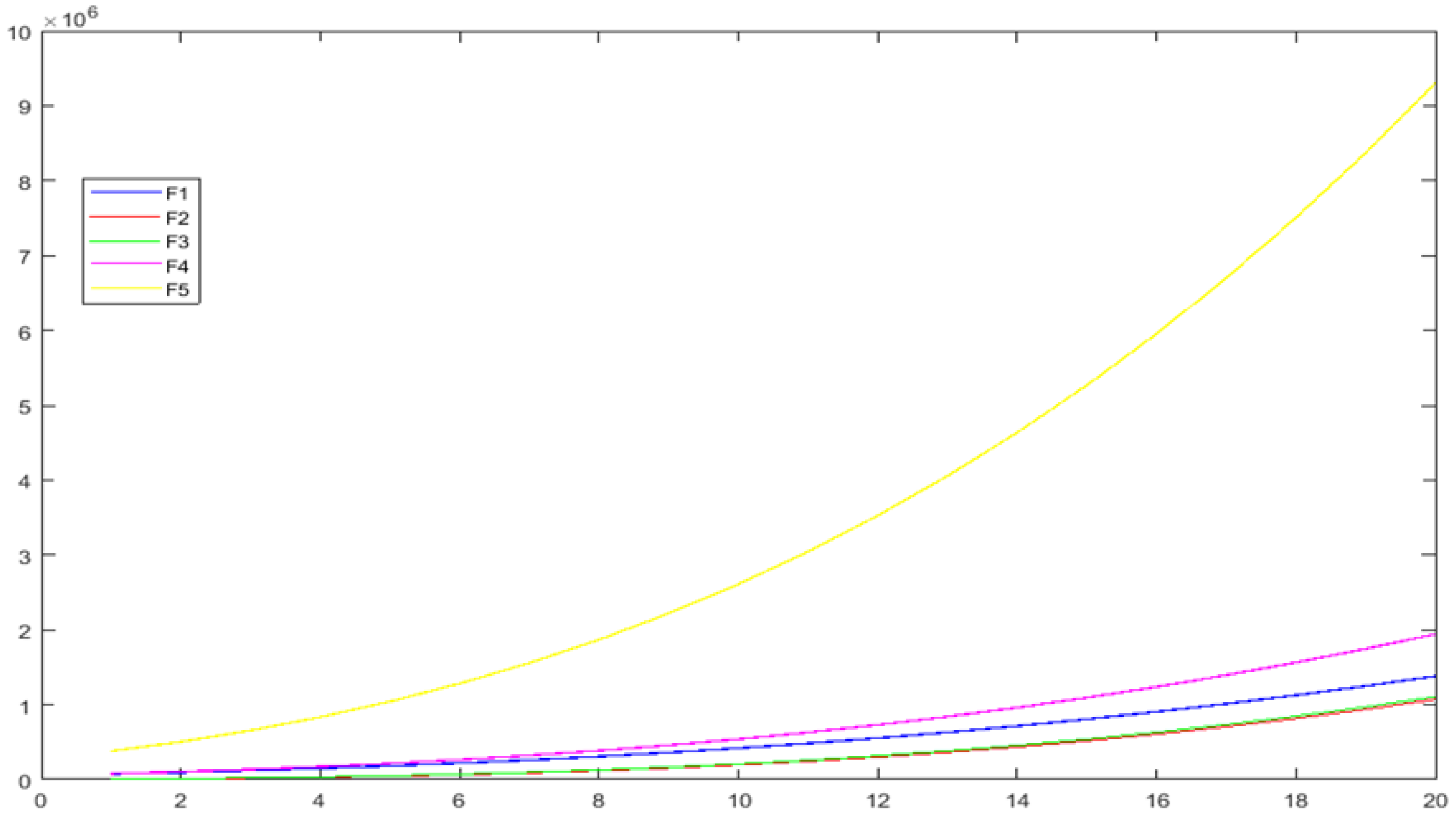
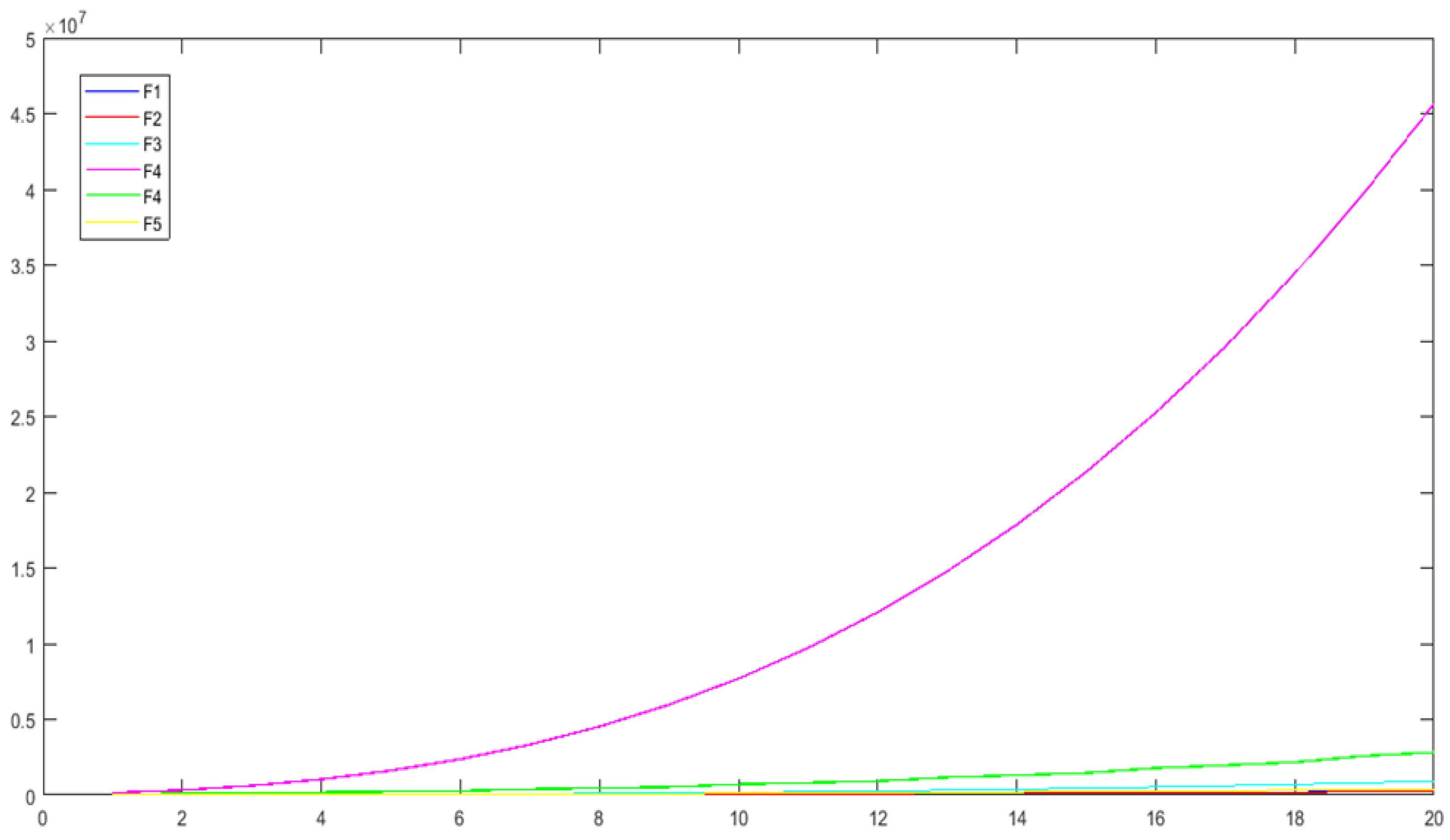
4. The Wiener Index and GP-Index of Polyhedral Graphs
| , | |
5. Summary and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wiener, H.J. Structural Determination of Paraffin Boiling Points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef] [PubMed]
- Harary, F. Graph Theory; Addison-Wesley: Reading, MA, USA, 1969. [Google Scholar]
- Dobrynin, A.A.; Entringer, R.; Gutman, I. Wiener index of trees: Theory and applications. Acta Appl. Math. 2001, 66, 211–249. [Google Scholar] [CrossRef]
- Guo, H.; Zhou, B.; Lin, H. The Wiener index of uniform hypergraphs. MATCH Commun. Math. Comput. Chem. 2017, 78, 133–152. [Google Scholar]
- Knor, M.; Škrekovski, R. Wiener index of line graphs. In Quantitative Graph Theory: Mathematical Foundations and Applications; Dehmer, M., Emmert-Streib, F., Eds.; CRC Press: Boca Raton, FL, USA, 2014; pp. 279–301. [Google Scholar]
- Knor, M.; Škrekovski, R.; Tepeh, A. Mathematical aspects of wiener index. Ars Math. Contemp. 2016, 11, 327–352. [Google Scholar] [CrossRef]
- Xu, K.; Liu, M.; Das, K.C.; Gutman, I.; Furtula, B. A survey on graphs extremal with respect to distance-based topolgical indices. MATCH Commun. Math. Comput. Chem. 2014, 71, 461–508. [Google Scholar]
- Graovac, A.; Pisanski, T. On the Wiener index of a graph. J. Math. Chem. 1991, 8, 53–62. [Google Scholar] [CrossRef]
- Črepnjak, M.; Tratnik, N.; Pleteršek, P.Ž. Predicting melting points of hydrocarbons by the Graovac-Pisanski index. Fuller. Nanotub. Carbon Nanostruct. 2016, 26, 239–245. [Google Scholar] [CrossRef]
- Gutman, I.; Šoltés, L. The range of the Wiener index and its mean isomer degeneracy. Z. Naturforschung 1991, 46, 865–868. [Google Scholar] [CrossRef]
- Ashrafi, A.R.; Shabani, H. The modified Wiener index of some graph operations. Ars Math. Contemp. 2016, 11, 277–284. [Google Scholar]
- Koorepazan-Moftakhar, F.; Ashrafi, A.R. Distance under symmetry. MATCH Commun. Math. Comput. Chem. 2015, 74, 259–272. [Google Scholar]
- Ghorbani, M.; Klavžar, S. Modified Wiener index via canonical metric representation, and some fullerene patches. Ars Math. Contemp. 2016, 11, 247–254. [Google Scholar] [CrossRef][Green Version]
- Tratnik, N. The Graovac-Pisanski index of zig-zag tubulenes and the generalized cut method. J. Math. Chem. 2017, 55, 1622–1637. [Google Scholar] [CrossRef]
- Knor, M.; Škrekovski, R.; Tepeh, A. Trees with the maximum value of Graovac– Pisanski index. Appl. Math. Comput. 2019, 358, 287–292. [Google Scholar]
- Knor, M.; Komorník, J.; Škrekovski, R.; Tepeh, A. Unicyclic graphs with the maximal value of Graovac-Pisanski index. Ars Math. Contemp. 2019, 17, 455–466. [Google Scholar] [CrossRef]
- Knor, M.; Škrekovski, R.; Tepeh, A. On the Graovac-Pisanski index of a graph. Acta Math. Univ. Comen. 2019, 88, 867–870. [Google Scholar]
- Črepnjak, M.; Knor, M.; Tratnika, N.; Pleteršek, P.Ž. The Graovac-Pisanski index of a connected bipartite graph is an integer number. arXiv 2017, arXiv:1709.04189v1. [Google Scholar]
- Hakimi-Nezhaad, M.; Ghorbani, M. Differences between Wiener and modified Wiener indices. J. Math. NanoSci. 2014, 4, 19–25. [Google Scholar]
- Ghorbani, M.; Hakimi-Nezhaad, M. An algebraic study of non-classical fullerenes. Fuller. Nanotub. Carbon Nanostruct. 2016, 24, 385–390. [Google Scholar] [CrossRef]
- Knor, M.; Škrekovski, R.; Tepeh, A. On the difference between Wiener index and Graovac–Pisanski index. MATCH Commun. Math. Comput. Chem. 2020, 83, 109–120. [Google Scholar]
- Ghorbani, M. Fullerene graphs with pentagons and heptagons. J. Math. Nanosci. 2013, 3, 33–37. [Google Scholar]
- Ghorbani, M.; Hakimi-Nezhaad, M.; Abbasi Barfaraz, F. An algebraic approach to Wiener number. J. Appl. Math. Comput. 2016, 55, 629–643. [Google Scholar] [CrossRef]
- Ghorbani, M.; Hakimi-Nezhaad, M. Polyhedral graphs under automorphism groups. Stud. Ubb Chem. 2016, LXI, 261–272. [Google Scholar]
- Hakimi-Nezhaad, M.; Ghorbani, M. On the Graovac-Pisanski index. Kragujev. J. Sci. 2017, 39, 91–98. [Google Scholar] [CrossRef]
- Ghorbani, M.; Hakimi-Nezhaad, M. Study of fullerenes via their symmetry groups. Fuller. Nanotub. Carbon Nanostruct. 2017, 25, 613–623. [Google Scholar] [CrossRef]
- Khaksari, A.; Hakimi-Nezhaad, M.; Ori, O.; Ghorbani, M. A survey of the automorphism groups of some fulleroids. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 80–86. [Google Scholar] [CrossRef]
- Koorepazan-Moftakhar, F.; Ashrafi, A.R.; Ori, O. Symmetry groups and Graovac—Pisanski index of some linear polymers. Quasigroups Relat. Sys. 2018, 26, 87–102. [Google Scholar]
- Ashrafi, A.R.; Koorepazan-Moftakhar, F.; Diudea, V.M.; Ori, O. Graovac–Pisanski index of fullerenes and fullerene—like molecules. Fuller. Nanotub. Carbon Nanostruct. 2016, 24, 779–785. [Google Scholar] [CrossRef]
- Madani, S.; Ashrafi, A.R. Symmetry and two symmetry measures for the web and spider web graphs. J. Appl. Math. Comput. 2020, 1–12. [Google Scholar] [CrossRef]
- Tratnik, N.; Pleteršek, P.Ž. The Graovac-Pisanski index of armchair nanotubes. J. Math. Chem. 2018, 56, 1103–1116. [Google Scholar] [CrossRef]
- Dixon, J.D.; Mortimer, B. Permutation Groups; Springer: Berlin/Heidelberg, Germany, 1966. [Google Scholar]
- Deza, M.; Dutour Sikirić, M.; Fowler, P.W. Zigzags, railroads, and knots in fullerenes. J. Chem. Inf. Comp. Sci. 2004, 44, 1282–1293. [Google Scholar] [CrossRef]
- Dutour Sikirić, M.; Delgado-Friedrichs, O.; Deza, M. Space fullerenes: Computer search for new Frank-Kasper structures. Acta Crystallogr. A 2010, 66, 602–615. [Google Scholar] [CrossRef] [PubMed]
- Fowler, P.W.; Manolopoulos, D.E. An Atlas of Fullerenes; Oxford University Press: New York, NY, USA, 1995. [Google Scholar]
- Zhang, H.; Ye, D. An upper bound for the Clar number of fullerene graphs. J. Math. Chem. 2007, 41, 123–133. [Google Scholar] [CrossRef]
- Ghorbani, M.; Dehmer, M.; Emmert-Streib, F. Properties of entropy-based topological measures of fullerenes. Mathematics 2020, 8, 740. [Google Scholar] [CrossRef]
- Ghorbani, M.; Dehmer, M.; Mowshowitz, A.; Tao, J.; Emmert-Streib, F. The Hosoya entropy of graphs revisited. Symmetry 2019, 11, 1013. [Google Scholar] [CrossRef]
- Ghorbani, M.; Dehmer, M.; Rahmani, S.; Rajabi-Parsa, M. A Survey on symmetry group of polyhedral graphs. Symmetry 2020, 12, 370. [Google Scholar] [CrossRef]




| W | ||
|---|---|---|
| n | Correlation |
|---|---|
| 5 | 0 |
| 6 | −0.04 |
| 7 | −0.03 |
| 8 | −0.06 |
| 9 | −0.20 |
| 10 | −0.19 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghorbani, M.; Hakimi-Nezhaad, M.; Dehmer, M.; Li, X. Analysis of the Graovac–Pisanski Index of Some Polyhedral Graphs Based on Their Symmetry Group. Symmetry 2020, 12, 1411. https://doi.org/10.3390/sym12091411
Ghorbani M, Hakimi-Nezhaad M, Dehmer M, Li X. Analysis of the Graovac–Pisanski Index of Some Polyhedral Graphs Based on Their Symmetry Group. Symmetry. 2020; 12(9):1411. https://doi.org/10.3390/sym12091411
Chicago/Turabian StyleGhorbani, Modjtaba, Mardjan Hakimi-Nezhaad, Matthias Dehmer, and Xueliang Li. 2020. "Analysis of the Graovac–Pisanski Index of Some Polyhedral Graphs Based on Their Symmetry Group" Symmetry 12, no. 9: 1411. https://doi.org/10.3390/sym12091411
APA StyleGhorbani, M., Hakimi-Nezhaad, M., Dehmer, M., & Li, X. (2020). Analysis of the Graovac–Pisanski Index of Some Polyhedral Graphs Based on Their Symmetry Group. Symmetry, 12(9), 1411. https://doi.org/10.3390/sym12091411







