Influence of Plastic Anisotropy on the Limit Load of an Overmatched Cracked Tension Specimen
Abstract
1. Introduction
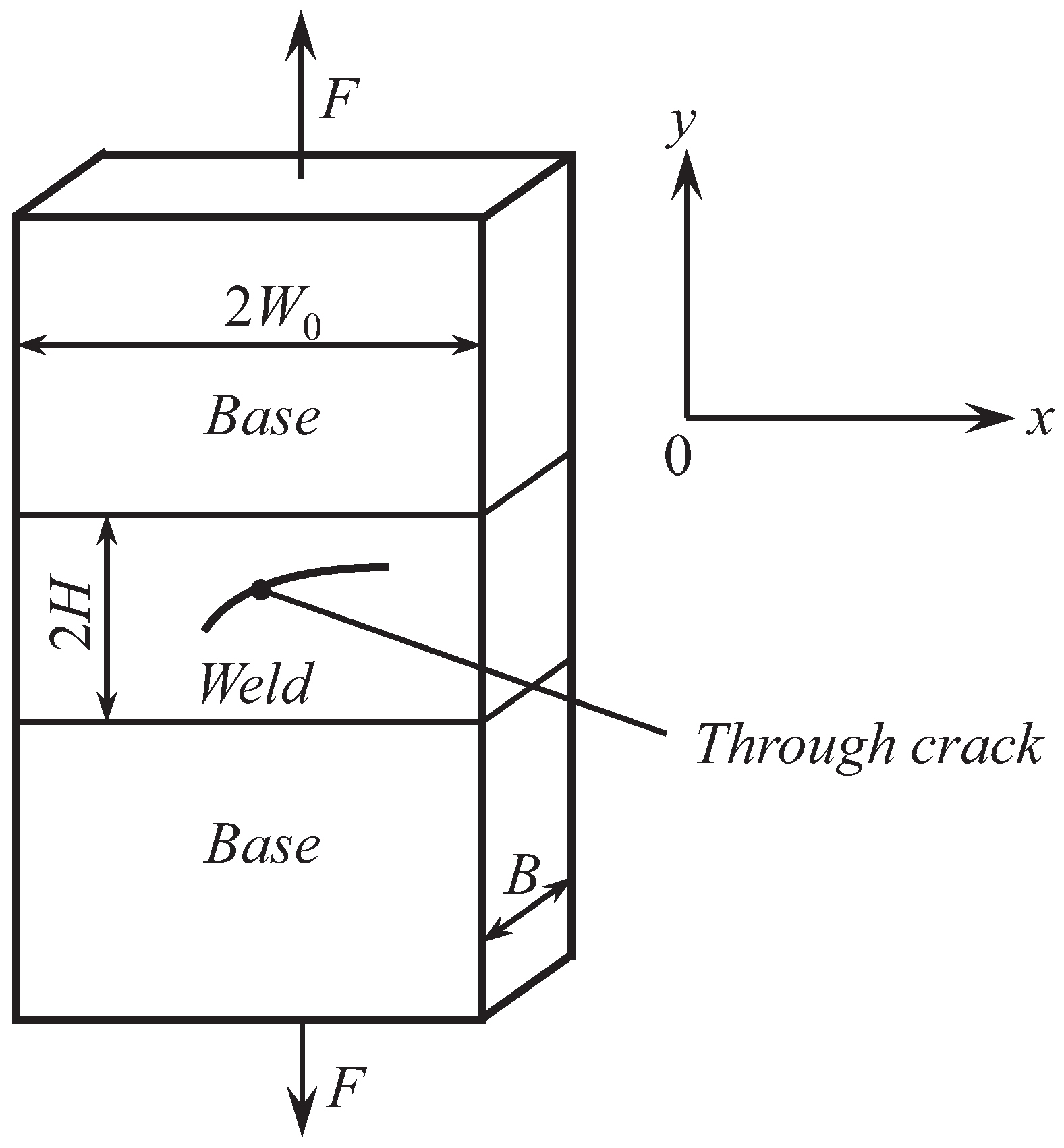
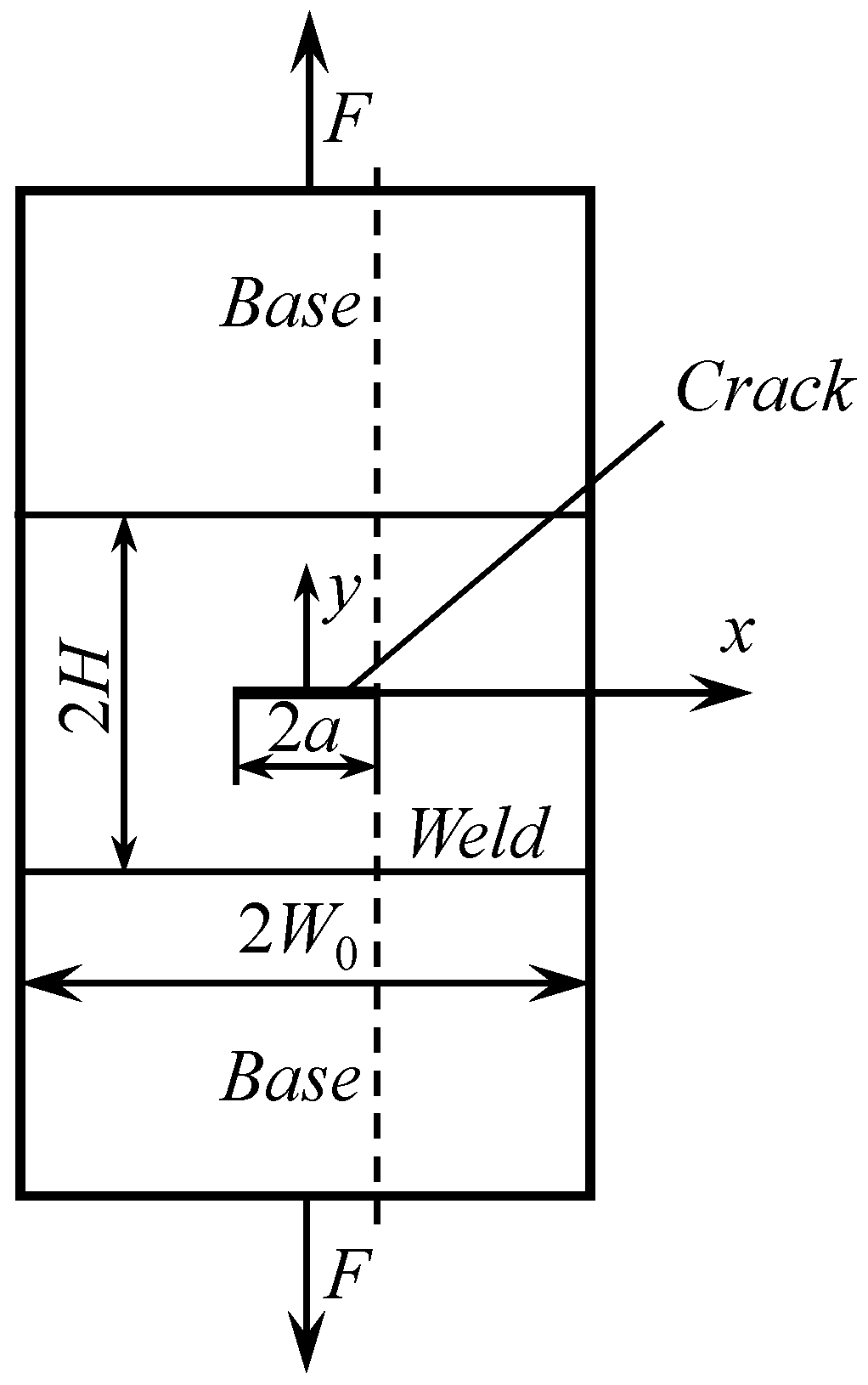
2. Statement of the Problem
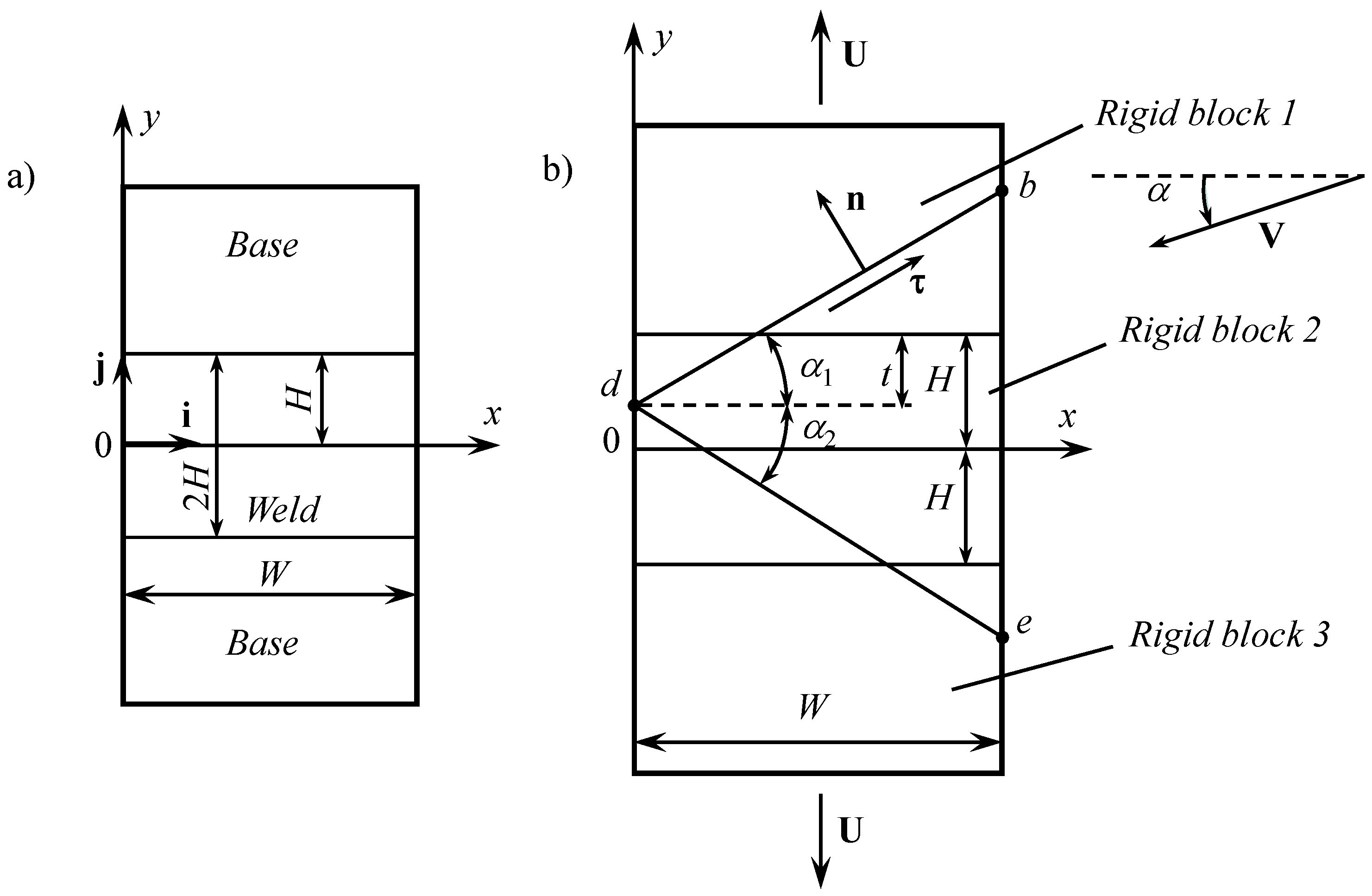
3. Solution for an Auxiliary Problem
3.1. Kinematically Admissible Velocity Field
3.2. Plastic Work Rate
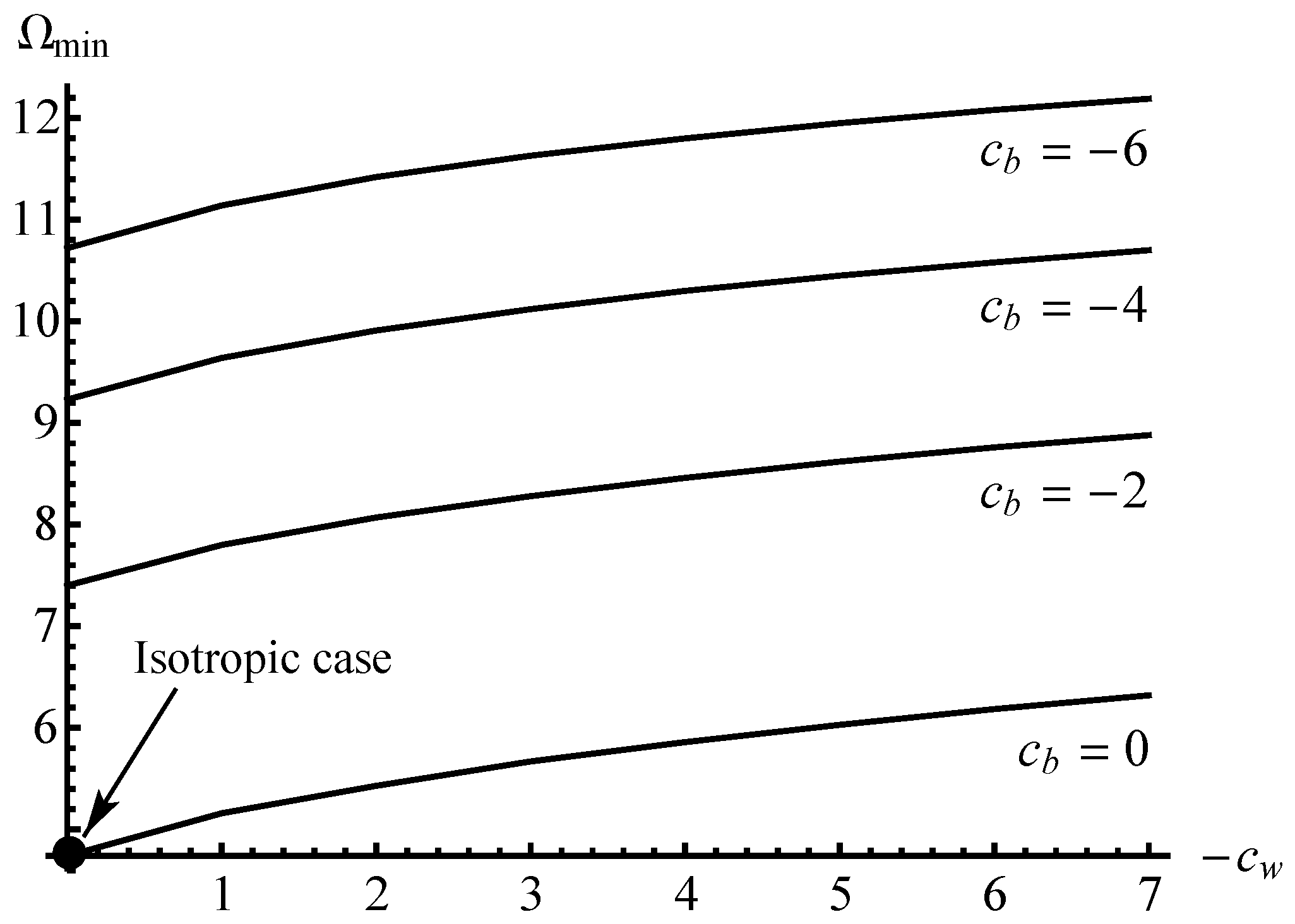
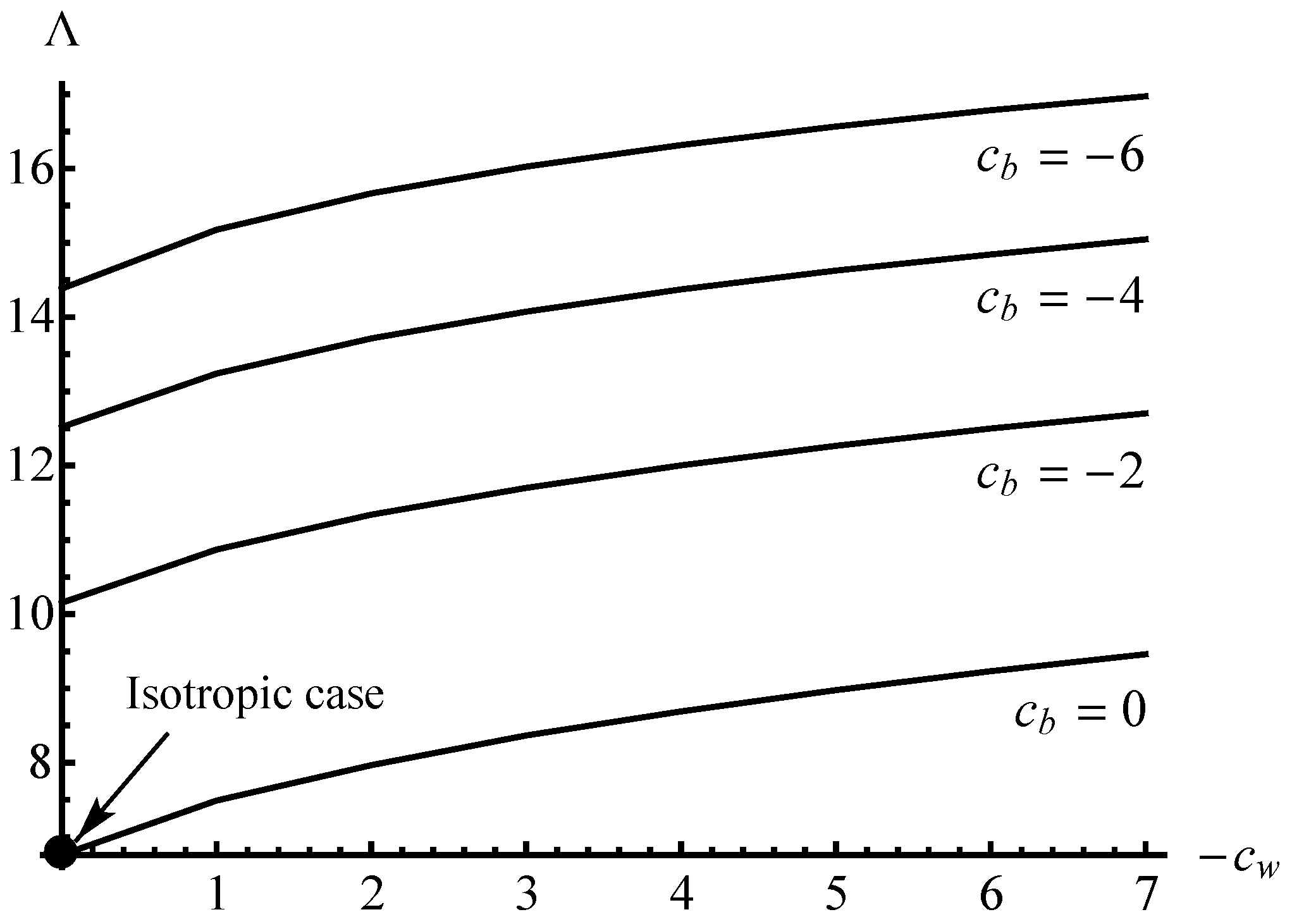
4. Limit Load of Cracked Specimens
4.1. Middle Cracked Specimen
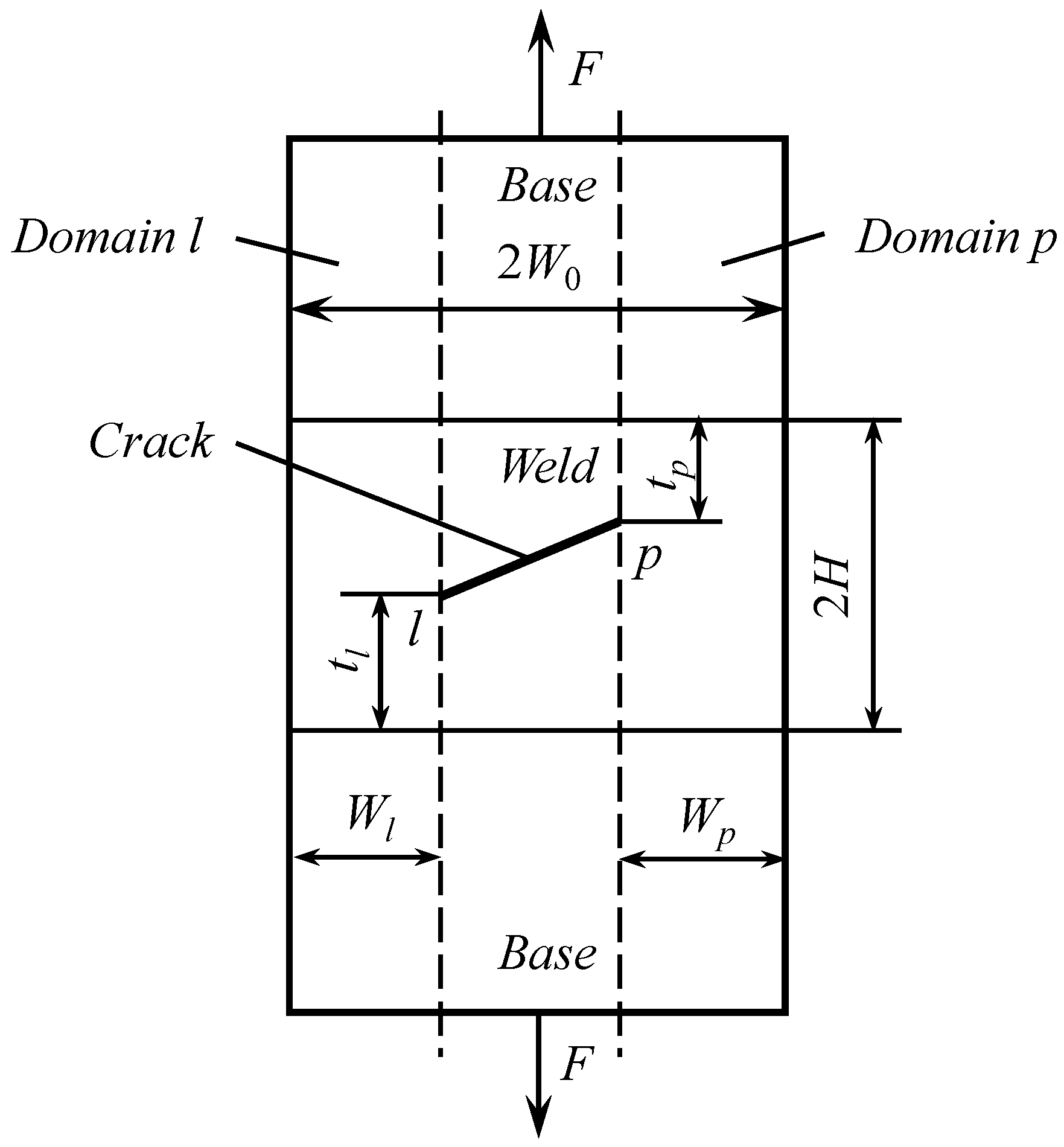
4.2. Specimen Containing an Arbitrary Straight Crack inside the Weld
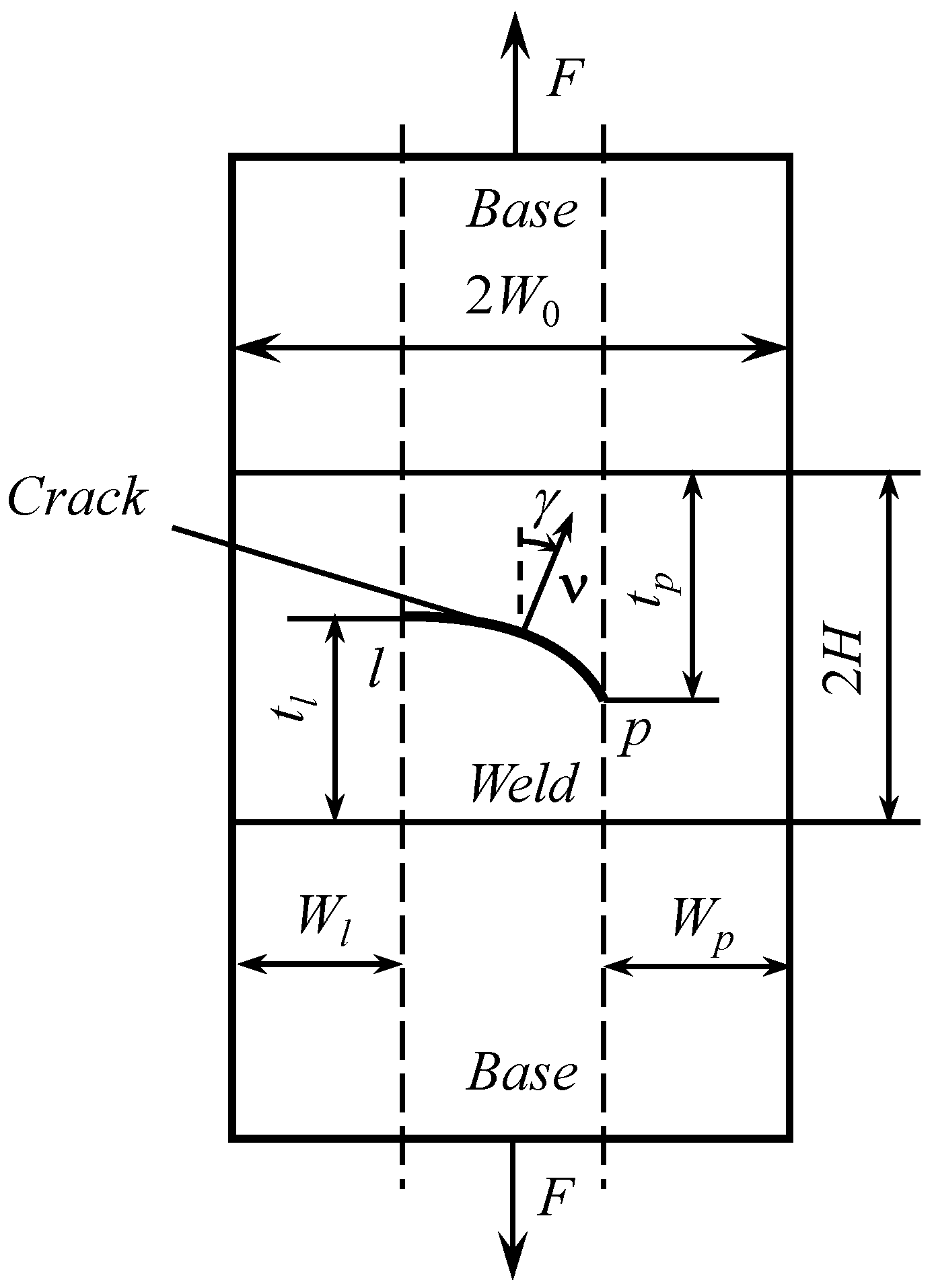
4.3. Specimen with an Arbitrary Crack inside the Weld
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zerbst, U.; Ainsworth, R.A.; Schwalbe, K.-H. Basic principles of analytical flaw assessment methods. Int. J. Press. Vessel. Pip. 2000, 77, 855–867. [Google Scholar] [CrossRef]
- Miller, A.G. Review of limit loads of structures containing defects. Int. J. Press. Vessel. Pip. 1988, 32, 197–327. [Google Scholar] [CrossRef]
- Kim, Y.-J.; Schwalbe, K.-H. Compendium of yield load solutions for strength mis-matched DE(T), SE(B) and C(T) specimens. Eng. Fract. Mech. 2001, 68, 1137–1151. [Google Scholar] [CrossRef]
- Alexandrov, S. Upper Bound Limit Load Solutions for Welded Joints with Cracks; Springer: New York, NY, USA, 2012. [Google Scholar] [CrossRef]
- Alexandrov, S.; Gracio, J. Influence of anisotropy on a limit load of welded strength overmatched middle cracked tension specimens. Fat. Fract. Eng. Mater. Struct. 2003, 26, 399–403. [Google Scholar] [CrossRef]
- Alexandrov, S.; Kontchakova, N. Influence of anisotropy on limit load of weld-strength overmatched cracked plates in pure bending. Mater. Sci. Eng. A 2004, 387–389, 395–398. [Google Scholar] [CrossRef]
- Hill, R. The Mathematical Theory of Plasticity; Clarendon Press: Oxford, UK, 1950. [Google Scholar]
- Alexandrov, S.; Chung, K.-H.; Chung, K. Effect of plastic anisotropy of weld on limit load of undermatched middle cracked tension specimens. Fat. Fract. Eng. Mater. Struct. 2007, 30, 333–341. [Google Scholar] [CrossRef]
- Alexandrov, S.; Tzou, G.-Y.; Hsia, S.-Y. Effect of plastic anisotropy on the limit load of highly undermatched welded specimens in bending. Eng. Fract. Mech. 2008, 75, 3131–3140. [Google Scholar] [CrossRef]
- Alexandrov, S.; Mustafa, Y. Influence of plastic anisotropy on the limit load of highly under-matched scarf joints with a crack subject to tension. Eng. Fract. Mech. 2014, 131, 616–626. [Google Scholar] [CrossRef]
- Alexandrov, S.; Jeng, Y.-R. Singular rigid/plastic solutions in anisotropic plasticity under plane strain conditions. Cont. Mech. Therm. 2013, 25, 685–689. [Google Scholar] [CrossRef]
- Joch, J.; Ainsworth, R.A.; Hyde, T.H. Limit load and J-estimates for idealized problems of deeply cracked welded joints in plane-strain bending and tension. Fat. Fract. Eng. Mater. Struct. 1993, 16, 1061–1079. [Google Scholar] [CrossRef]
- Hao, S.; Cornec, A.; Schwalbe, K.-H. Plastic stress-strain fields and limit loads of a plane strain cracked tensile panel with a mismatched welded joint. Int. J. Solids Struct. 1997, 34, 297–326. [Google Scholar] [CrossRef]
- Alexandrov, S.; Chikanova, N.; Kocak, M. Analytic yield load solution for overmatched center cracked tension specimen. Eng. Fract. Mech. 1999, 64, 383–399. [Google Scholar] [CrossRef]
- Kim, Y.-J.; Schwalbe, K.-H. Mismatch effect on plastic yield loads in idealized weldments I. Weld centre cracks. Eng. Fract. Mech. 2001, 68, 163–182. [Google Scholar] [CrossRef]
- Alexandrov, S. A limit load solution for a highly weld strength undermatched tensile panel with an arbitrary crack. Eng. Fract. Mech. 2010, 77, 3368–3371. [Google Scholar] [CrossRef]
- Sattari-Far, I. Finite element analysis of limit loads for surface cracks in plates. Int. J. Press. Vessel. Pip. 1994, 57, 237–243. [Google Scholar] [CrossRef]
- Kozak, D.; Gubeljak, N.; Konjatić, P.; Sertić, J. Yield load solutions of heterogeneous welded joints. Int. J. Press. Vessel. Pip. 2009, 86, 807–812. [Google Scholar] [CrossRef][Green Version]
- Naib, S.; De Waele, W.; Štefane, P.; Gubeljak, N.; Hertelé, S. Analytical limit load predictions in heterogeneous welded single edge notched tension specimens. Procedia Struct. Integr. 2018, 13, 1725–1730. [Google Scholar] [CrossRef]
- Legarth, B.N.; Tvergaard, V.; Kuroda, M. Effects of plastic anisotropy on crack-tip behaviour. Int. J. Fract. 2002, 117, 297–312. [Google Scholar] [CrossRef]
- Schwalbe, K.H.; Cornec, A. The engineering treatment model (ETM) and its practical application. Fat. Fract. Eng. Mater. Struct. 1991, 14, 405–412. [Google Scholar] [CrossRef]
- Benzerga, A.; Besson, J.; Pineau, A. Anisotropic ductile fracture: Part II: Theory. Acta Mater. 2004, 52, 4639–4650. [Google Scholar] [CrossRef]
- Tanguy, B.; Luu, T.T.; Perrin, G.; Pineau, A.; Besson, J. Plastic and damage behaviour of a high strength X100 pipeline steel: Experiments and modelling. Int. J. Press. Vessel. Pip. 2008, 85, 322–335. [Google Scholar] [CrossRef]
- Frodal, B.H.; Morin, D.; Børvik, T.; Hopperstad, O. On the effect of plastic anisotropy, strength and work hardening on the tensile ductility of aluminium alloys. Int. J. Solids Struct. 2020, 188–189, 118–132. [Google Scholar] [CrossRef]
- Schwalbe, K.-H. On the beauty of analytical models for fatigue crack propagation and fracture—A personal historical review. J. ASTM Int. 2010, 7, 1–53. [Google Scholar] [CrossRef]
- Zerbst, U.; Madia, M. Analytical flaw assessment. Eng. Fract. Mech. 2017, 187, 316–367. [Google Scholar] [CrossRef]
- Habraken, A.M. Modelling the plastic anisotropy of metals. Arch. Comput. Methods Eng. 2004, 11, 3–96. [Google Scholar] [CrossRef]
- Rice, J.R. Plane strain slip line theory for anisotropic rigid/plastic materials. J. Mech. Phys. Solids 1973, 21, 63–74. [Google Scholar] [CrossRef]
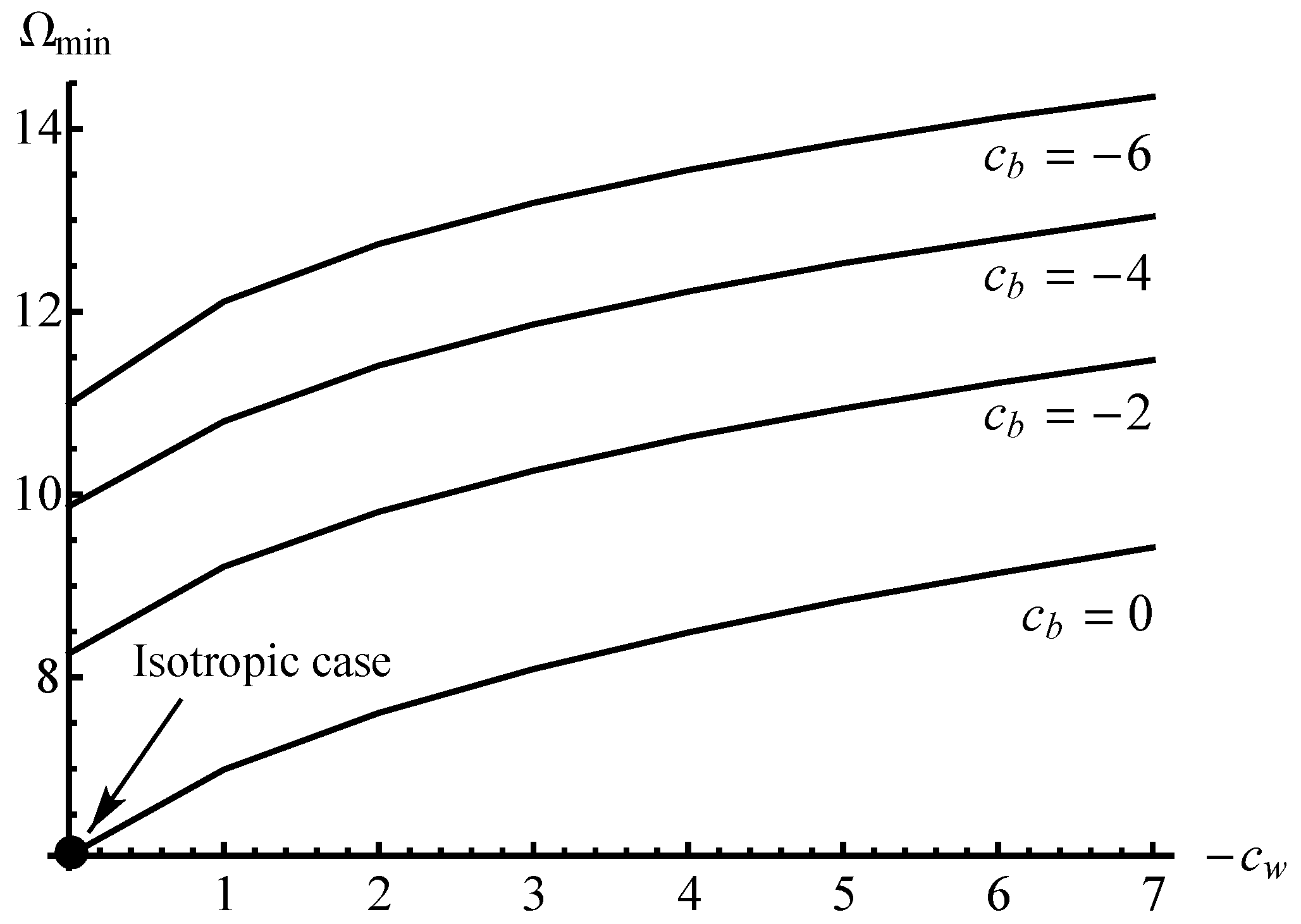
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyamina, E.; Kalenova, N.; Nguyen, D.K. Influence of Plastic Anisotropy on the Limit Load of an Overmatched Cracked Tension Specimen. Symmetry 2020, 12, 1079. https://doi.org/10.3390/sym12071079
Lyamina E, Kalenova N, Nguyen DK. Influence of Plastic Anisotropy on the Limit Load of an Overmatched Cracked Tension Specimen. Symmetry. 2020; 12(7):1079. https://doi.org/10.3390/sym12071079
Chicago/Turabian StyleLyamina, Elena, Nataliya Kalenova, and Dinh Kien Nguyen. 2020. "Influence of Plastic Anisotropy on the Limit Load of an Overmatched Cracked Tension Specimen" Symmetry 12, no. 7: 1079. https://doi.org/10.3390/sym12071079
APA StyleLyamina, E., Kalenova, N., & Nguyen, D. K. (2020). Influence of Plastic Anisotropy on the Limit Load of an Overmatched Cracked Tension Specimen. Symmetry, 12(7), 1079. https://doi.org/10.3390/sym12071079



