Development and Research on the Vertical Center Diaphragm Method Applied in Shallow Tunnel Construction
Abstract
1. Introduction
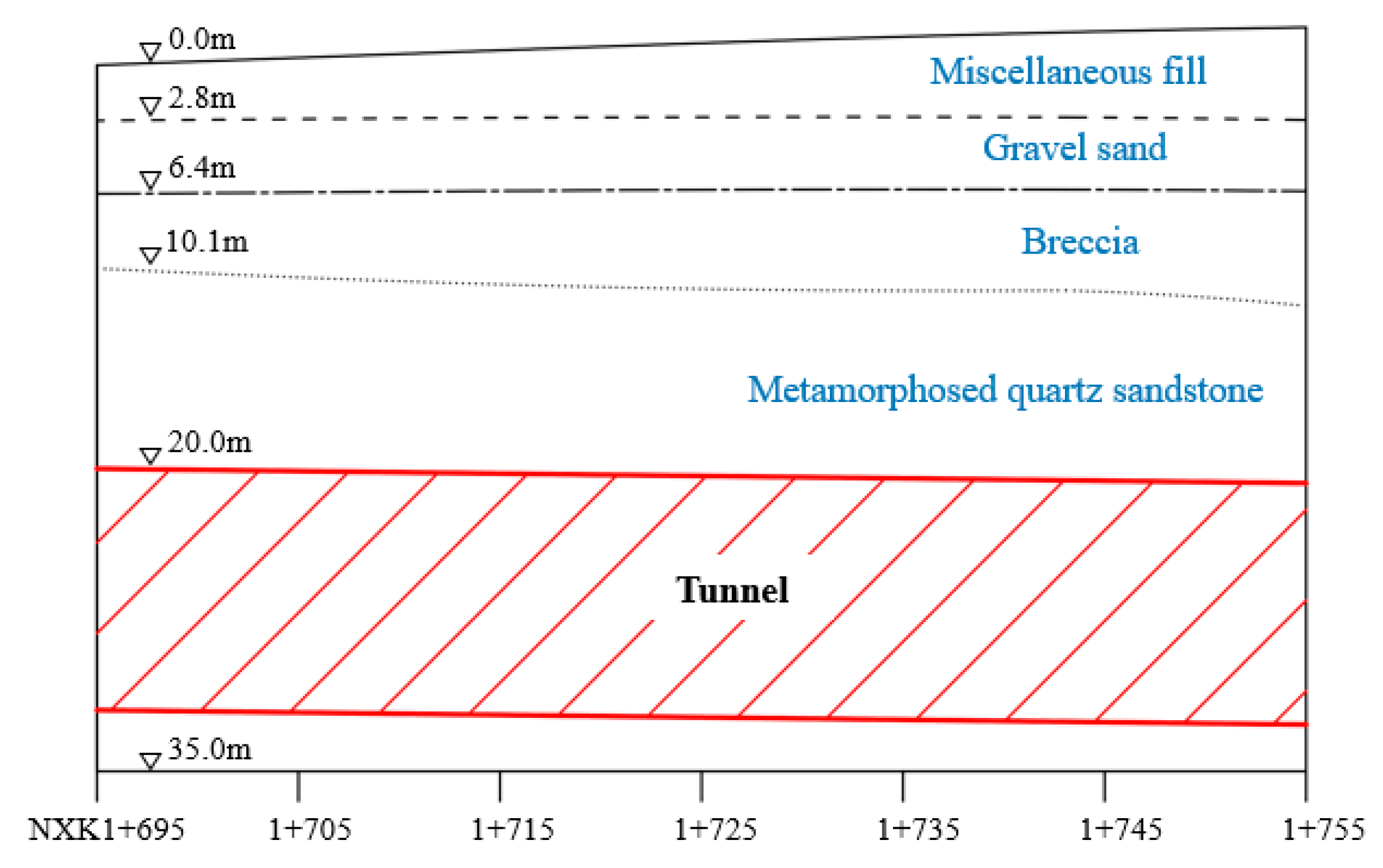
2. Project Overview
3. Applicable Conditions
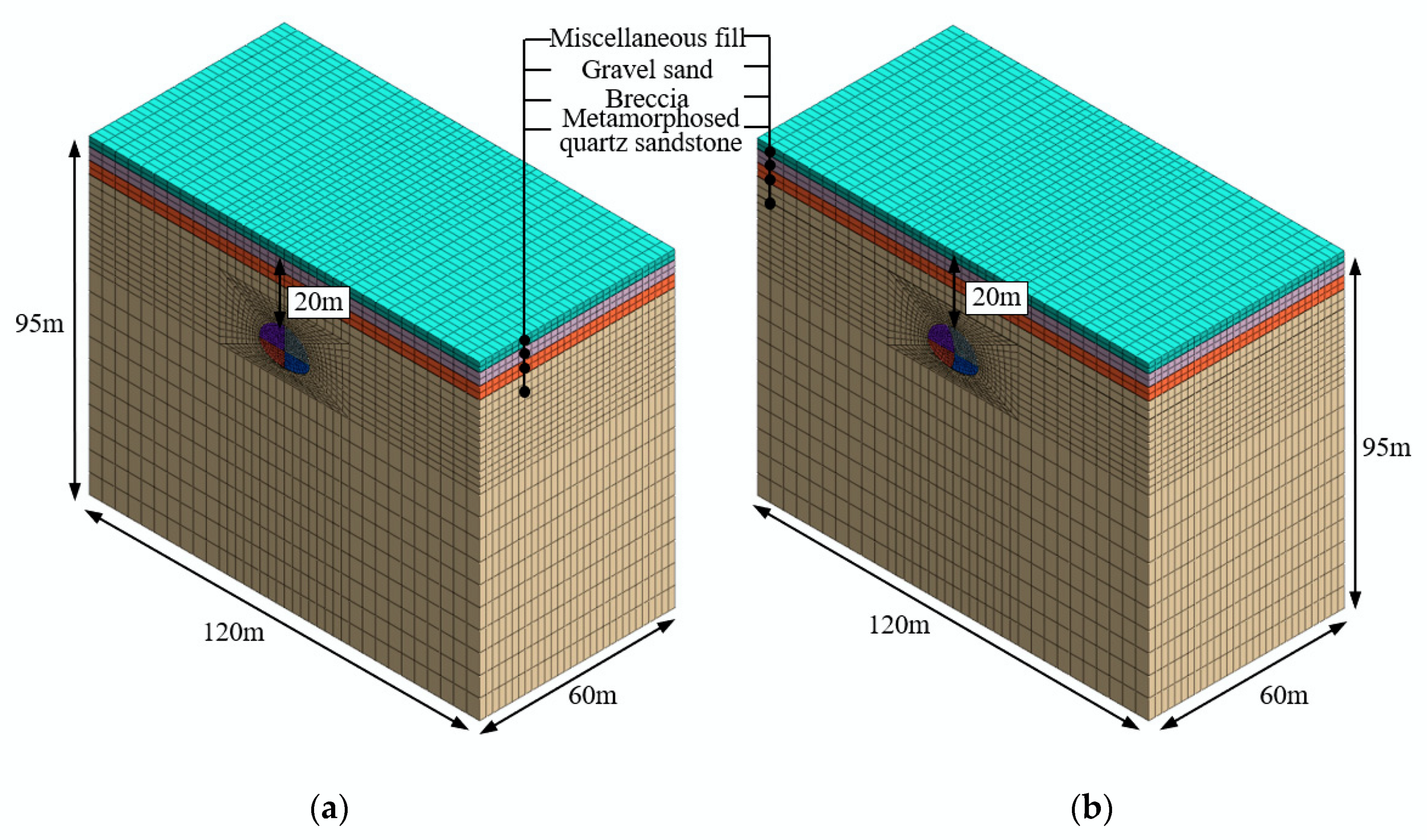
3.1. Finite-Difference Models
3.2. Calculation Conditions and Selection of Parameters
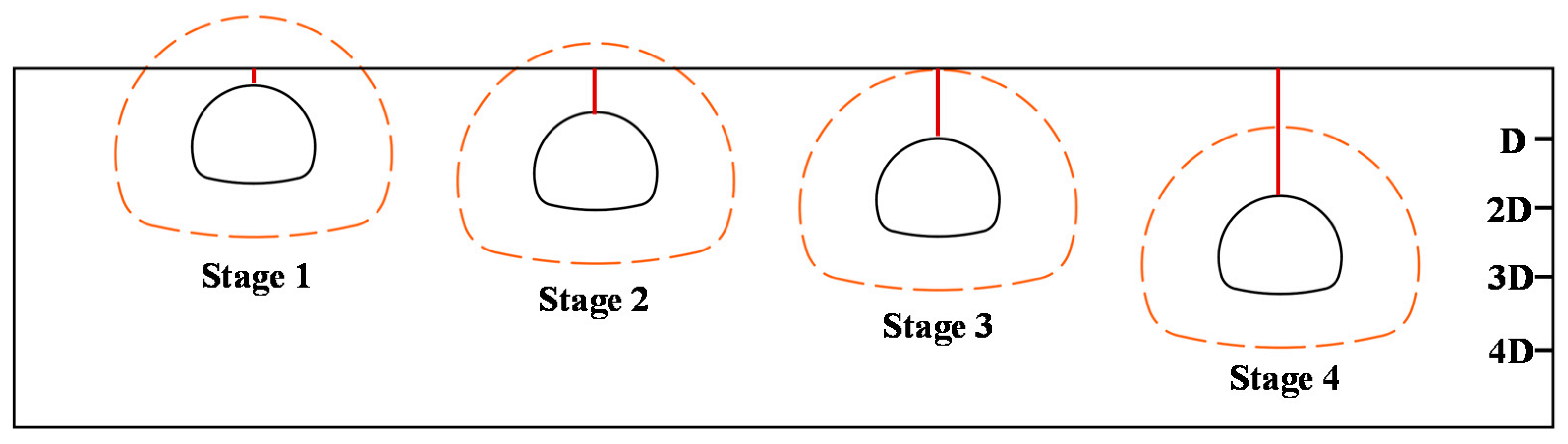
3.3. Comparative Analysis of Deformation in Different Buried Depth
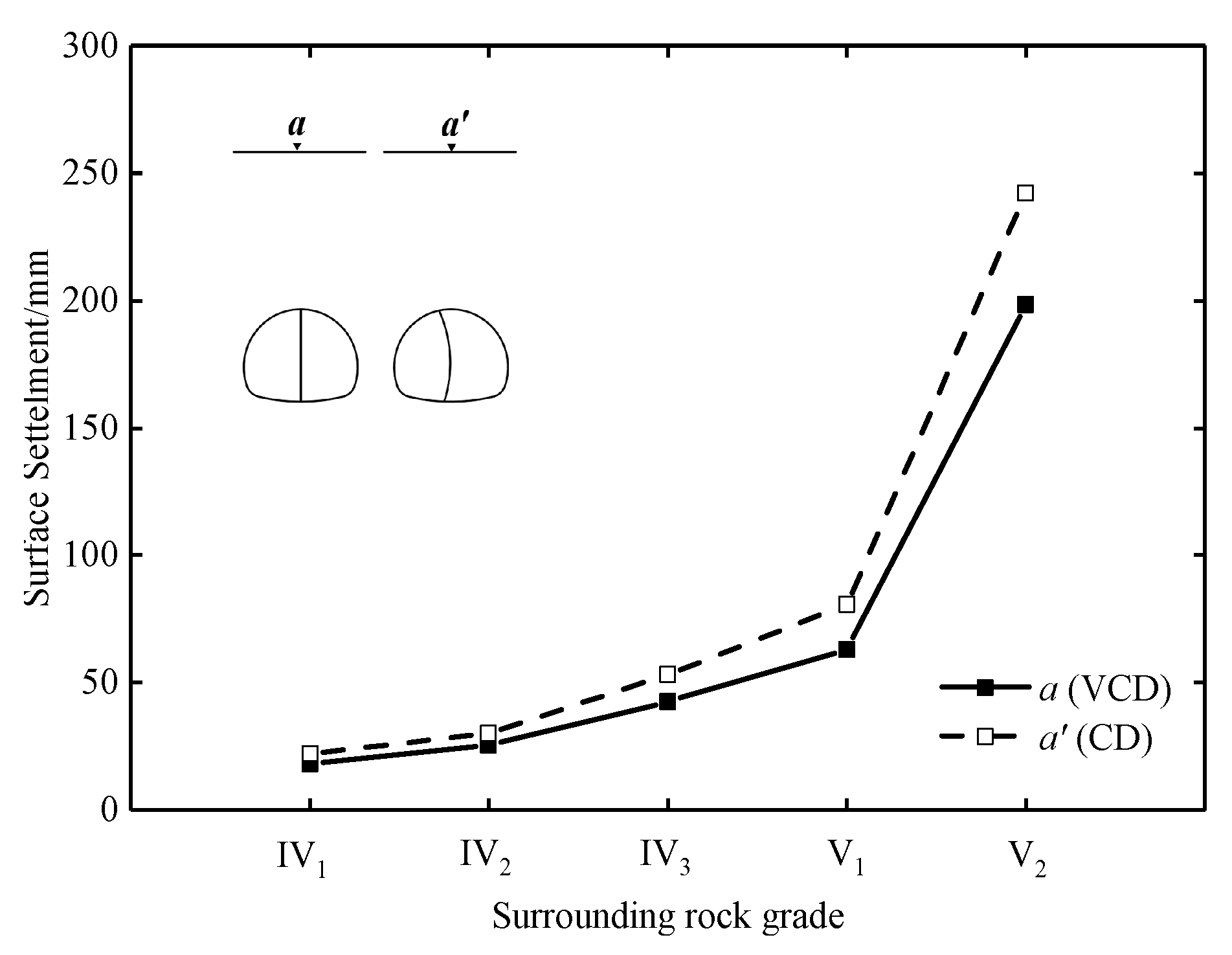
3.4. Comparative Analysis of Deformation in Different Surrounding Rock Grades
4. Deformation Process and Mechanical Properties
4.1. Finite-Difference Models and Parameters
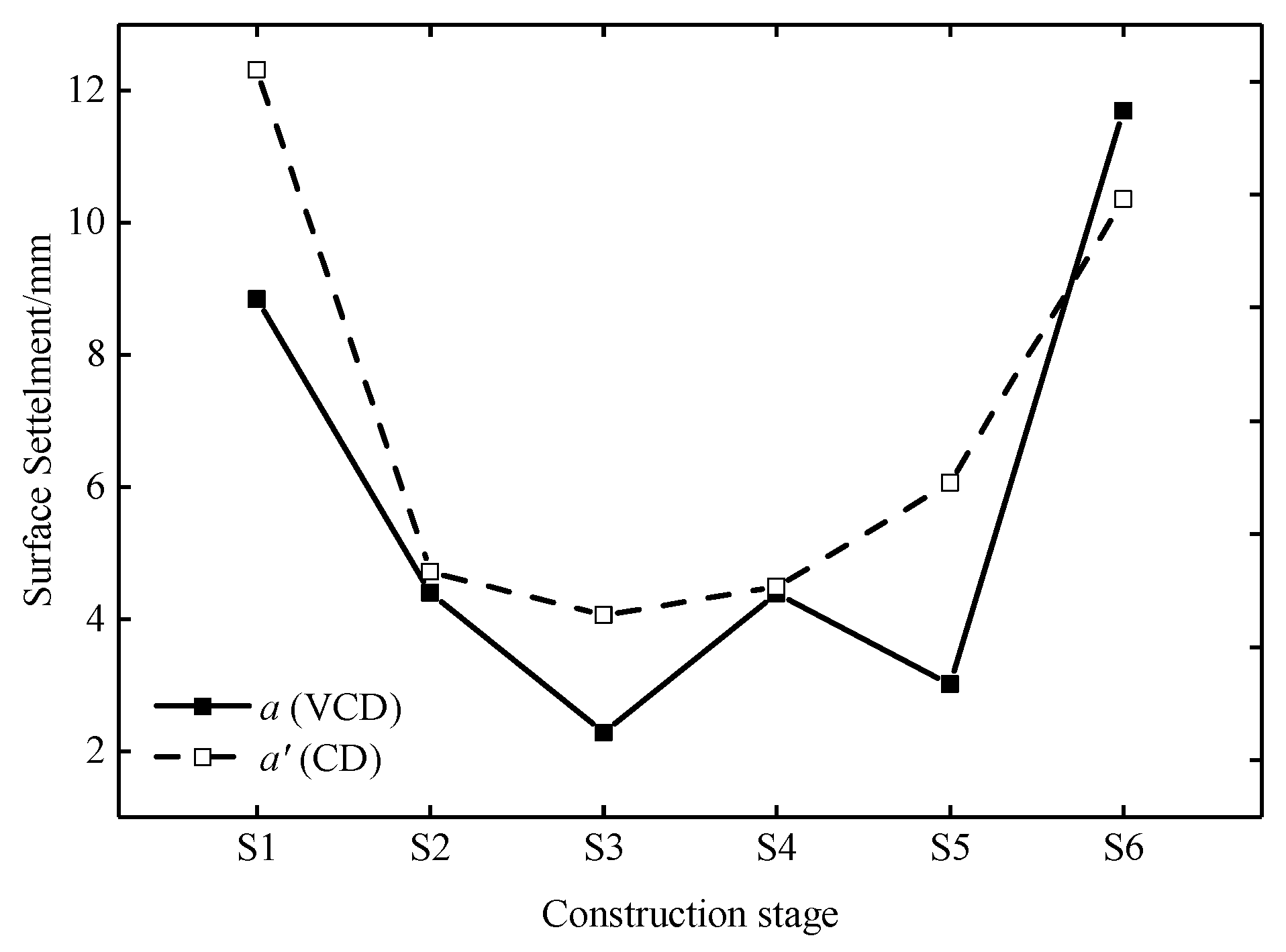
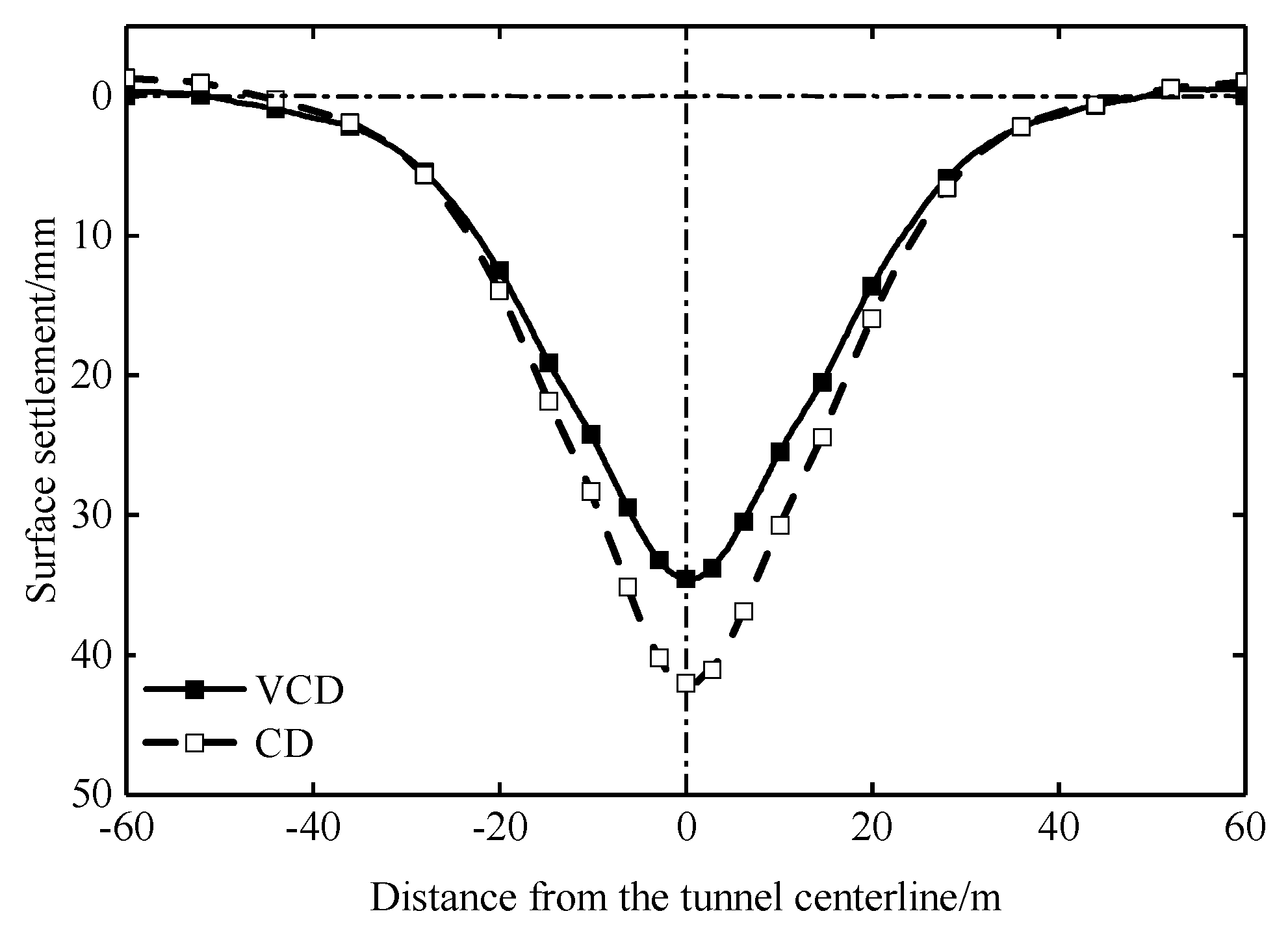
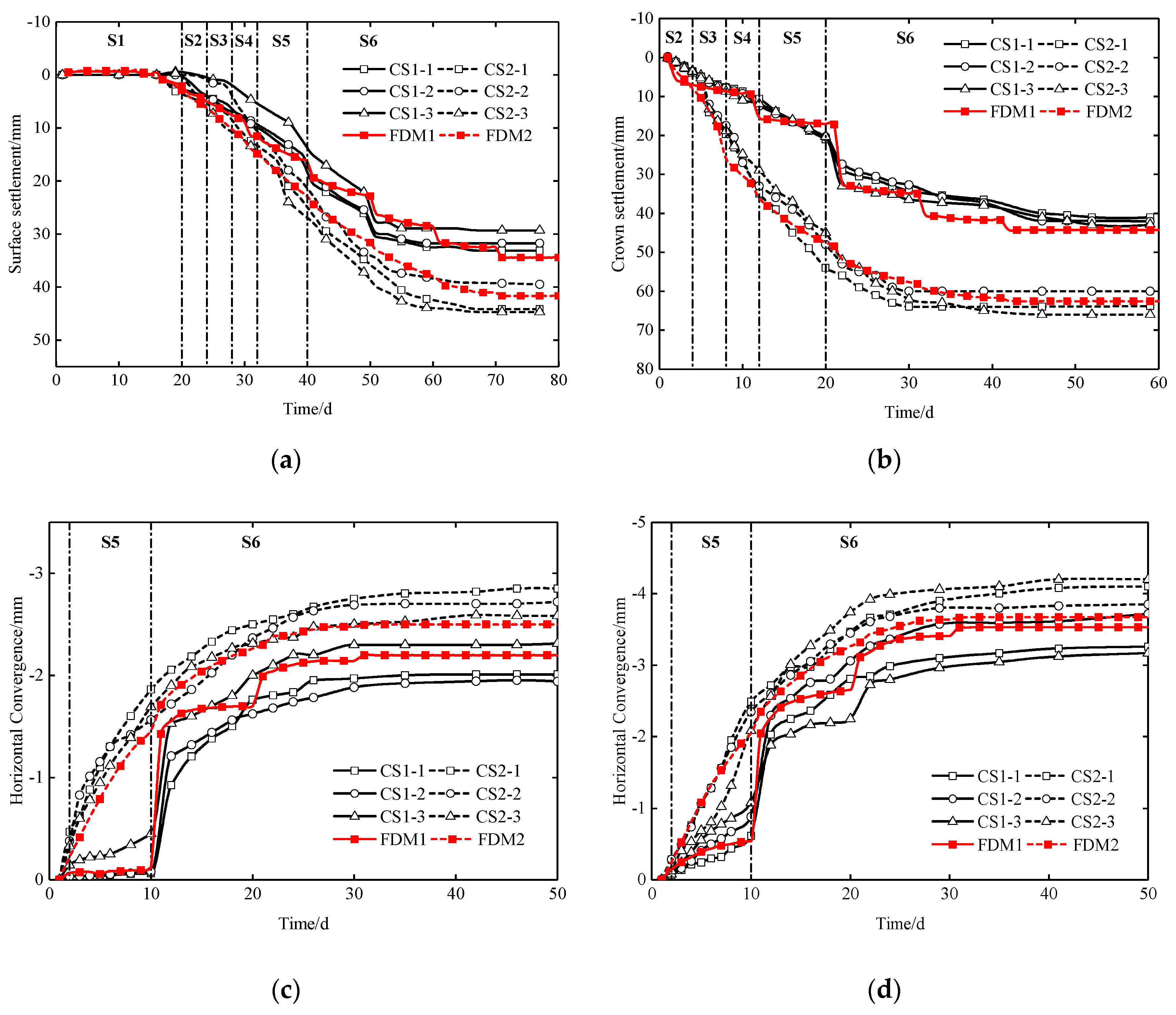
4.2. Comparison of Surface Settlement
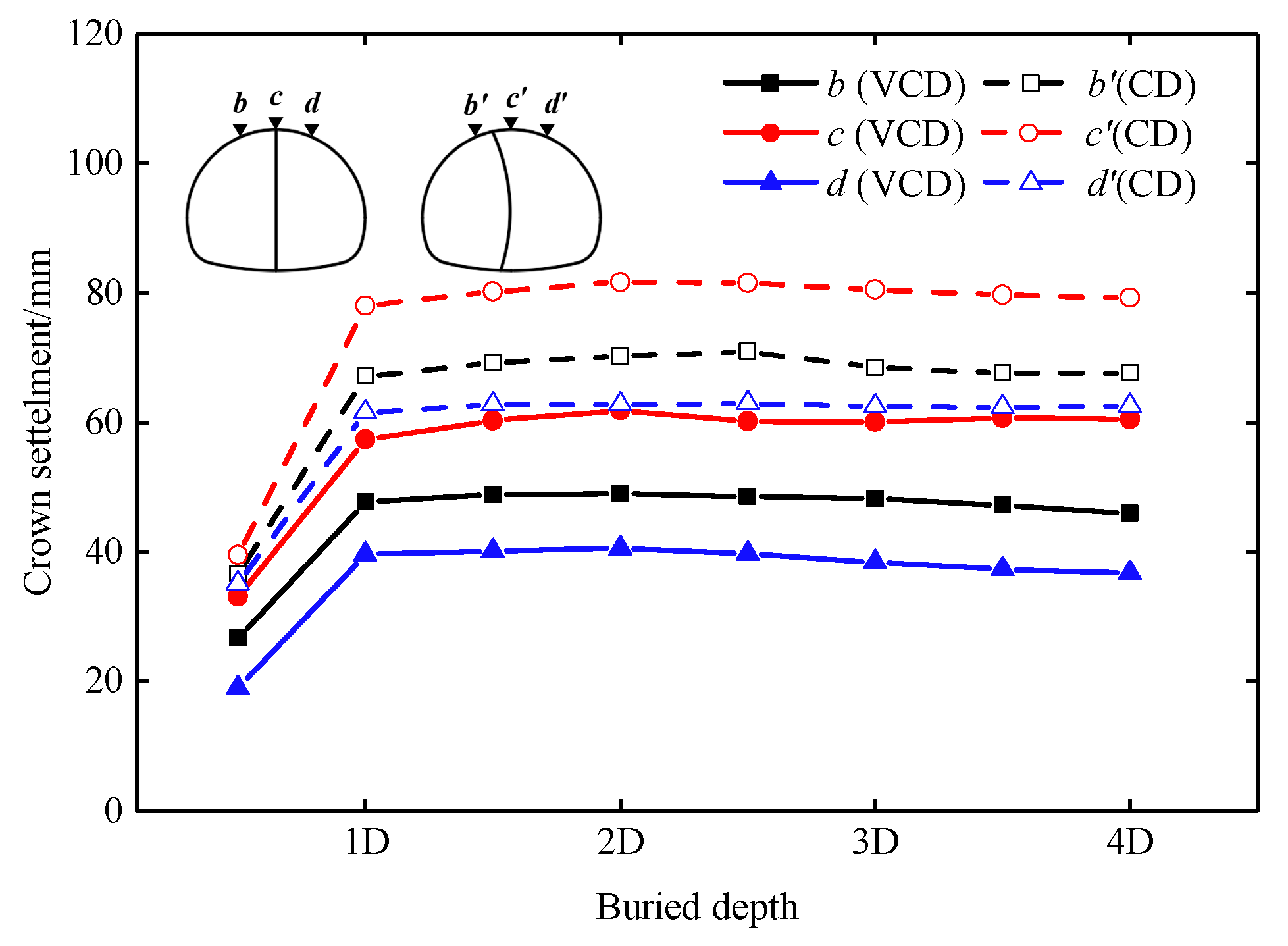
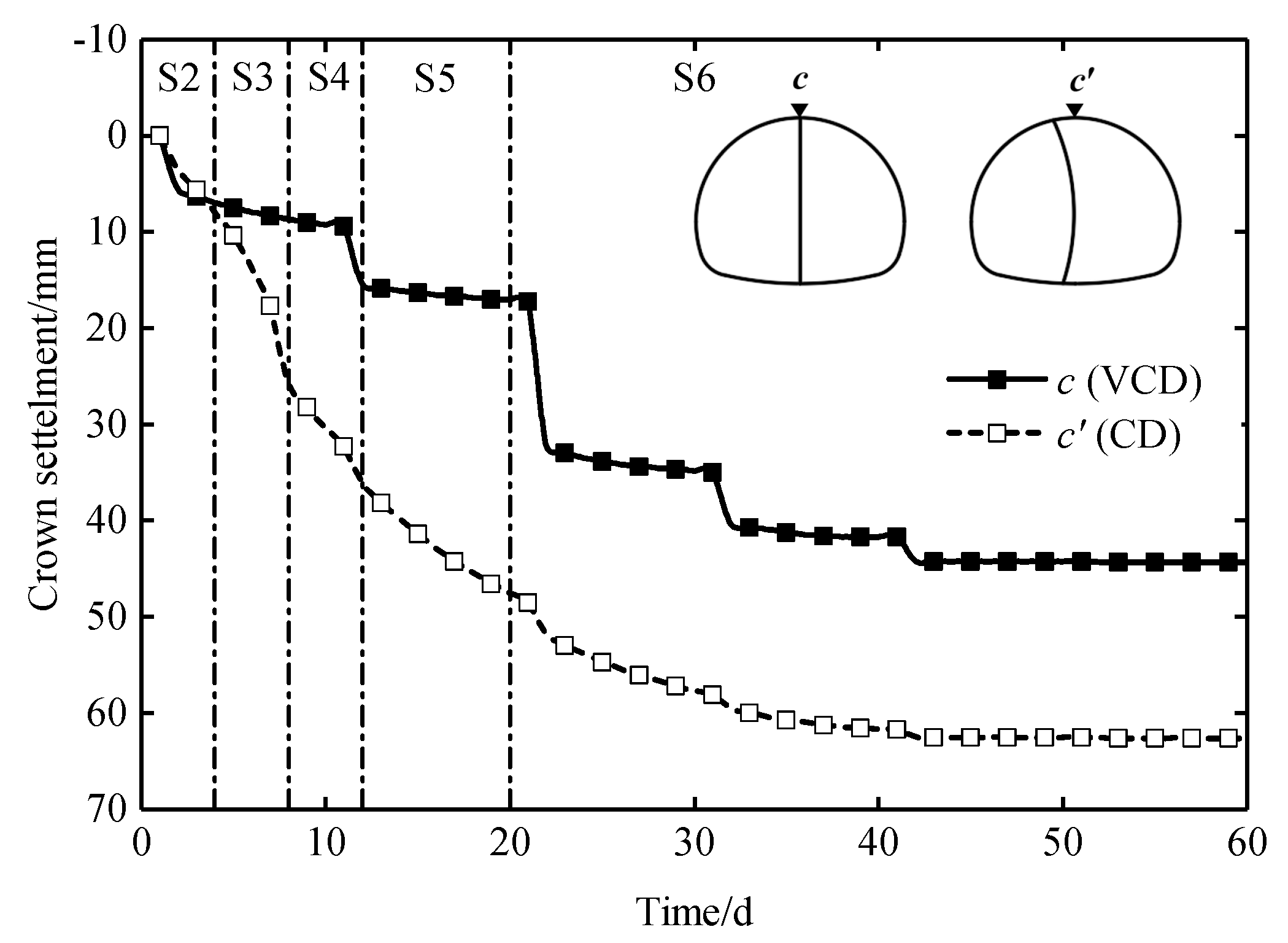
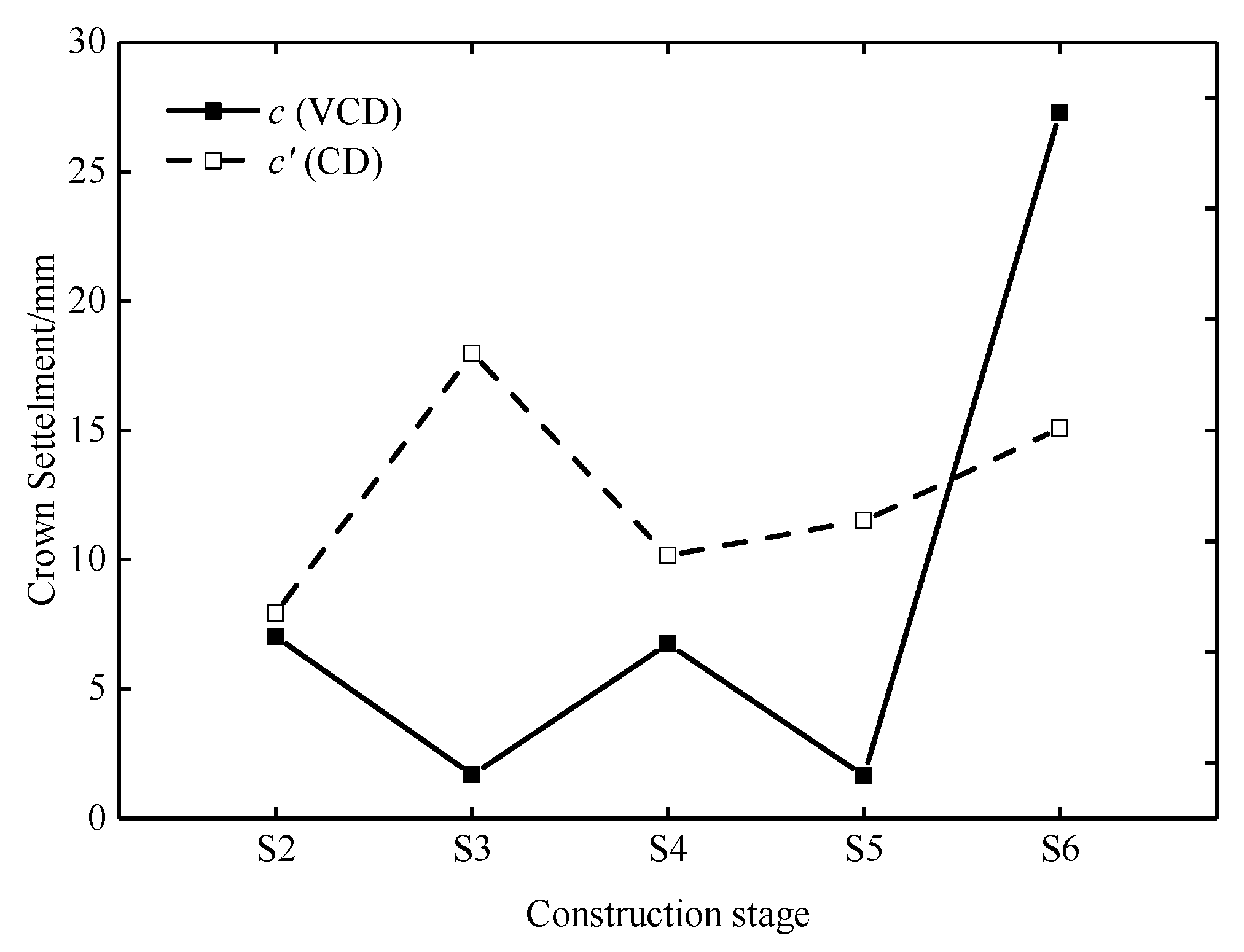
4.3. Comparison of Crown Settlement
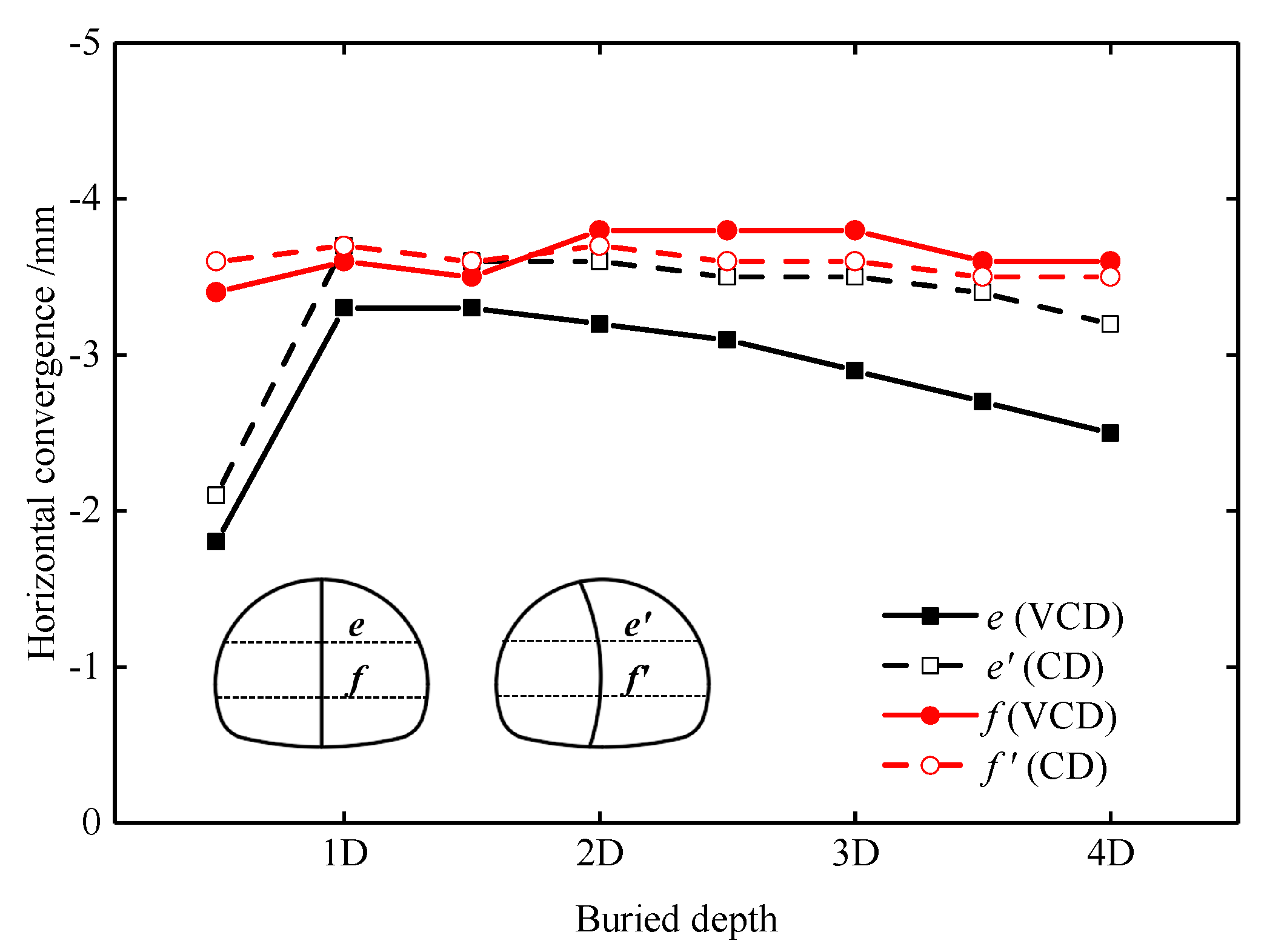
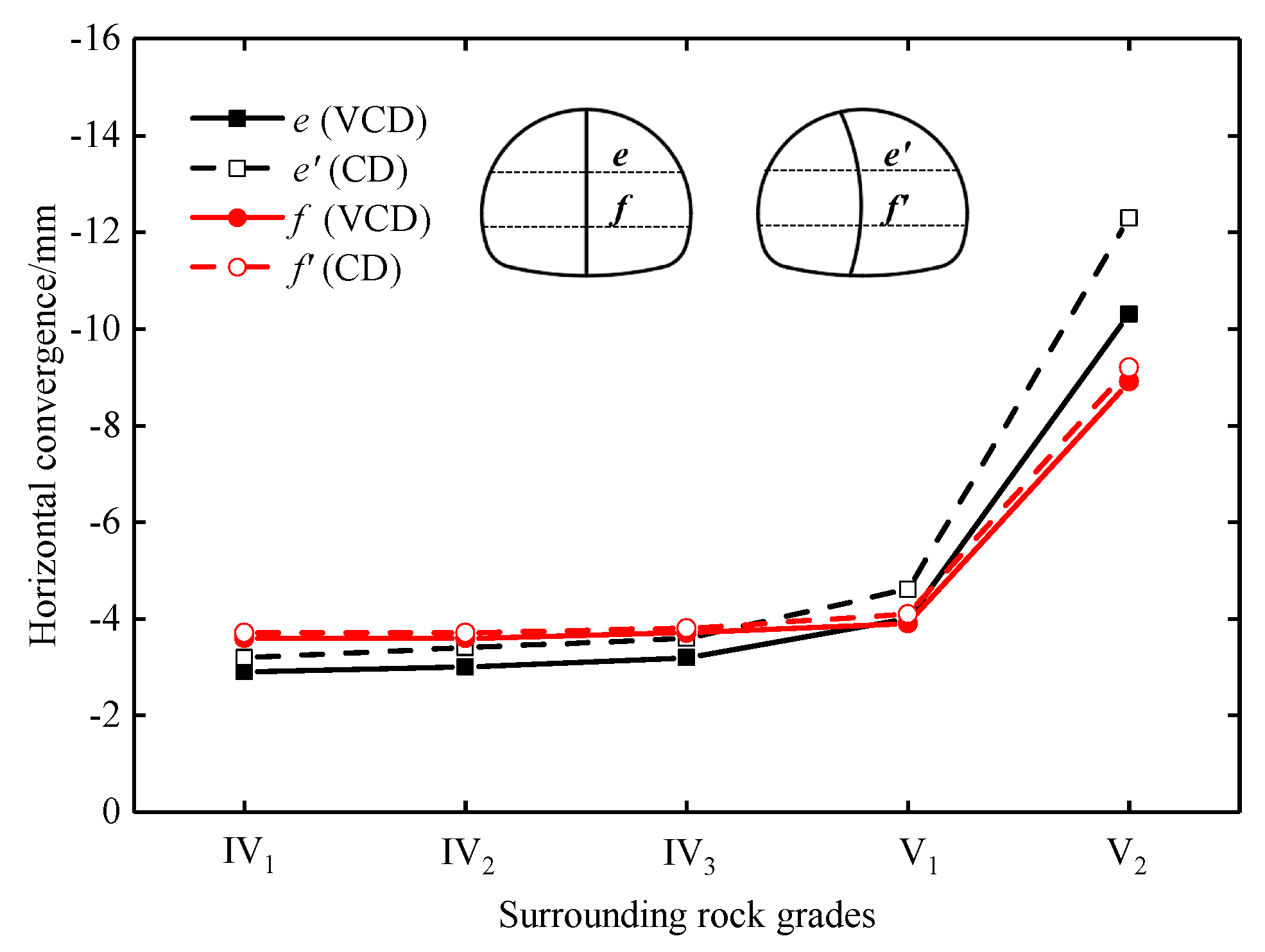
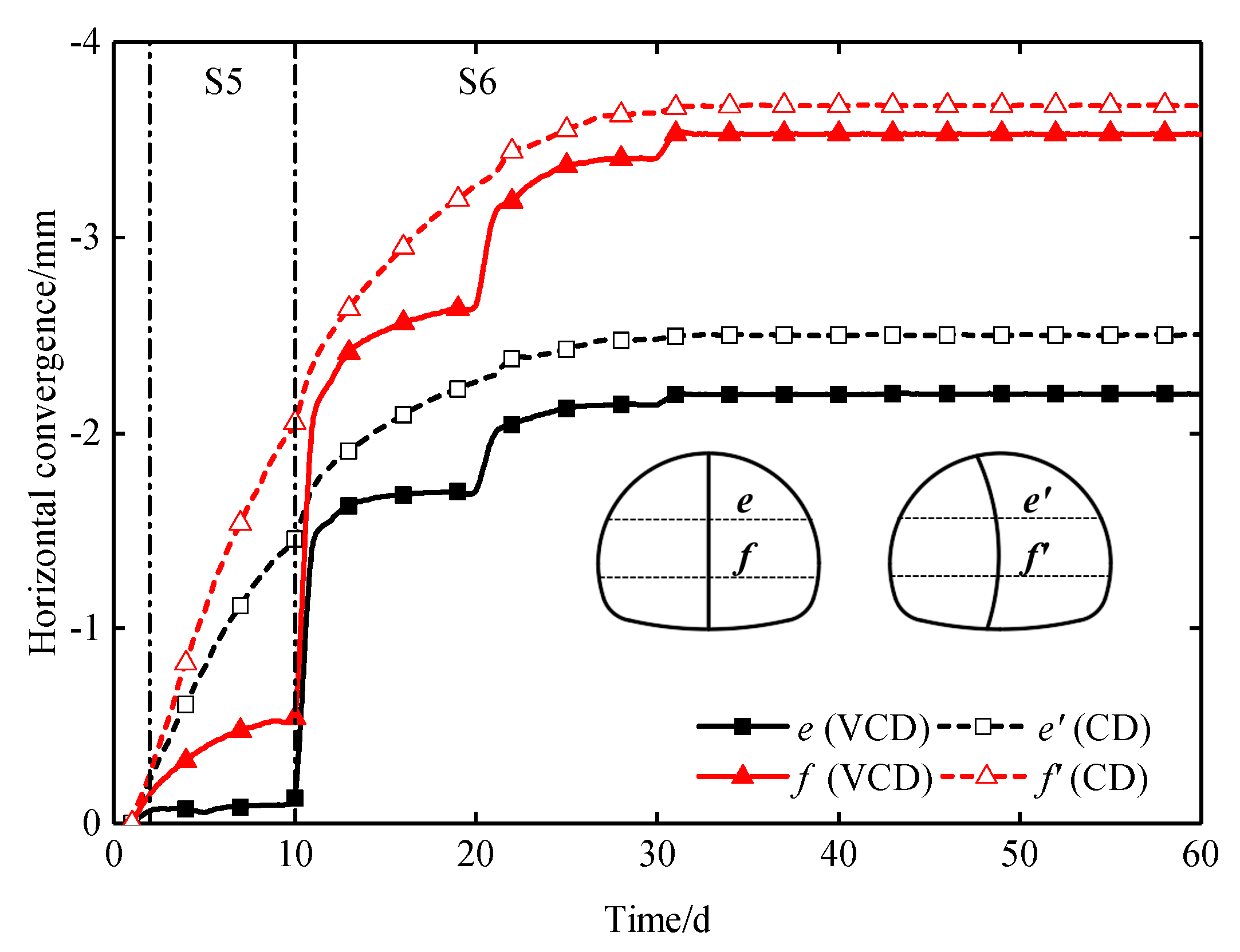
4.4. Comparison of Horizontal Convergence
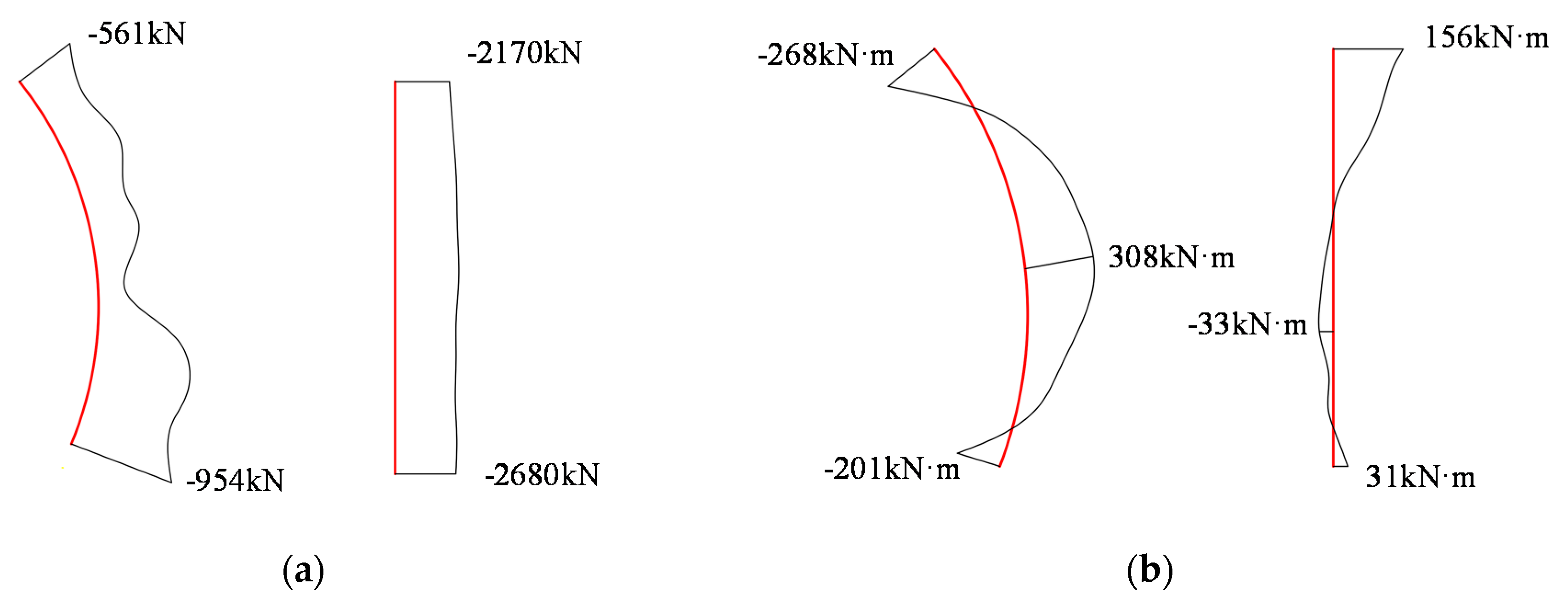
4.5. Comparison of Internal Forces on Center Diaphragm
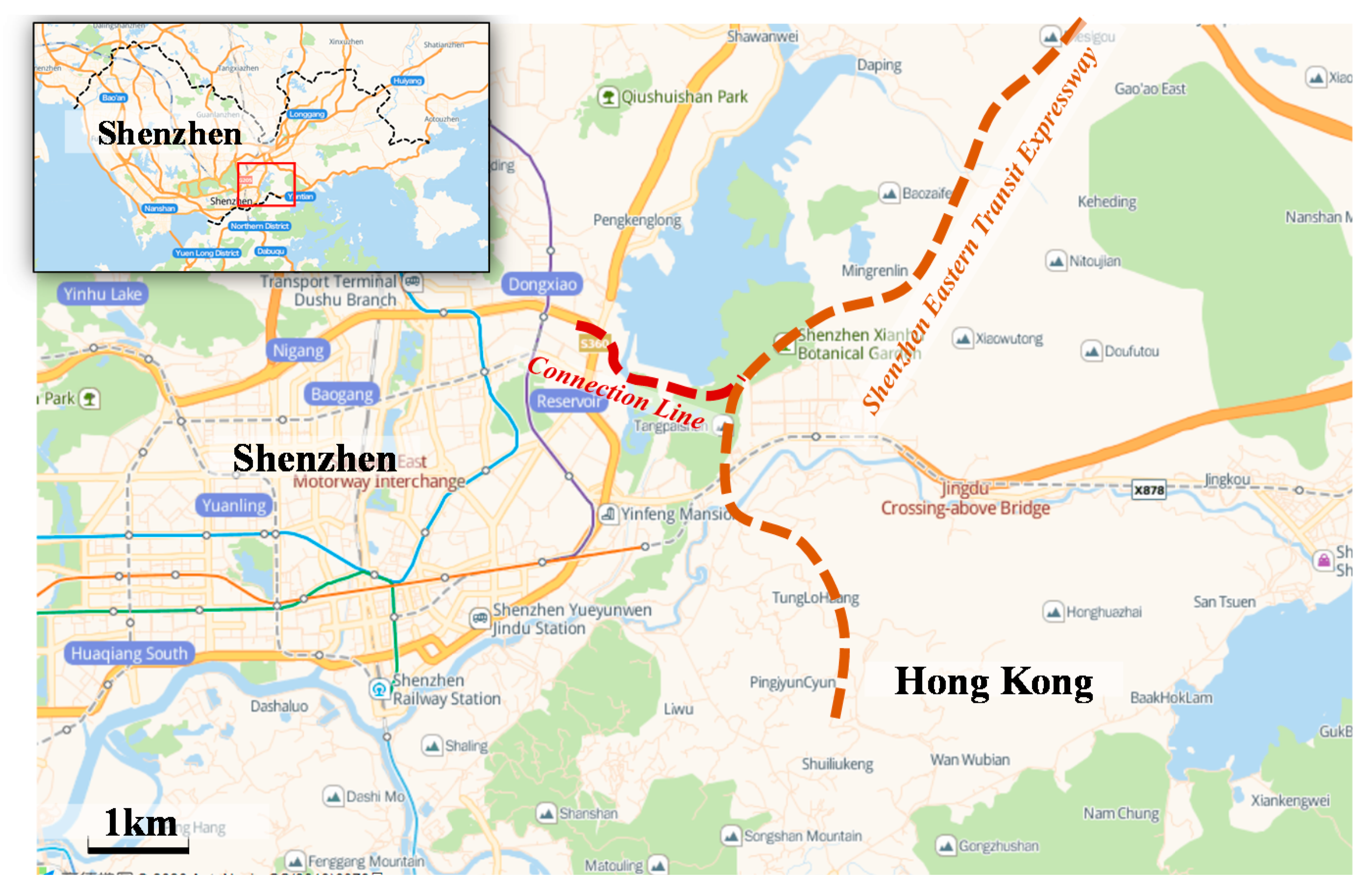
5. Field Test at Shenzhen Eastern Transit Expressway
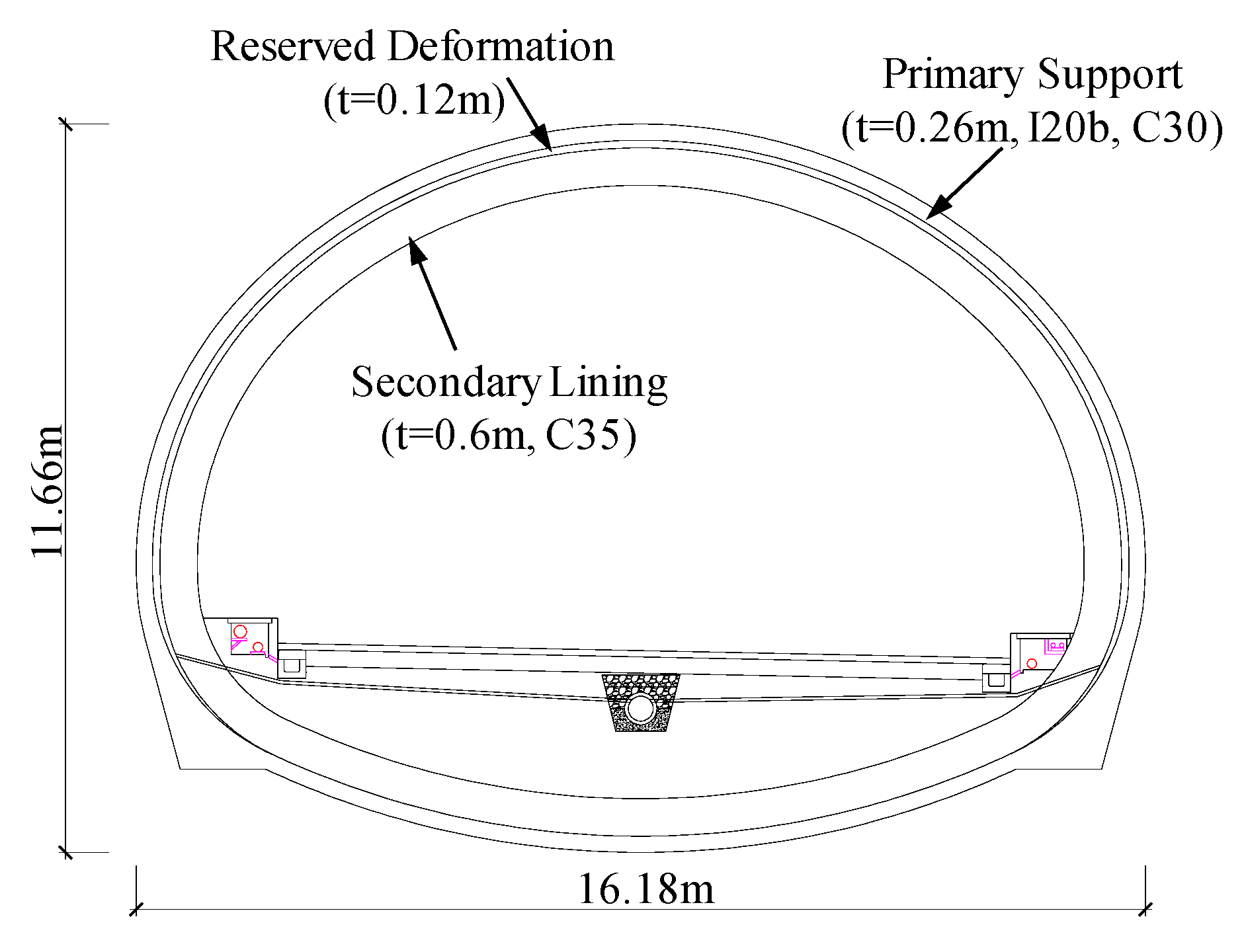
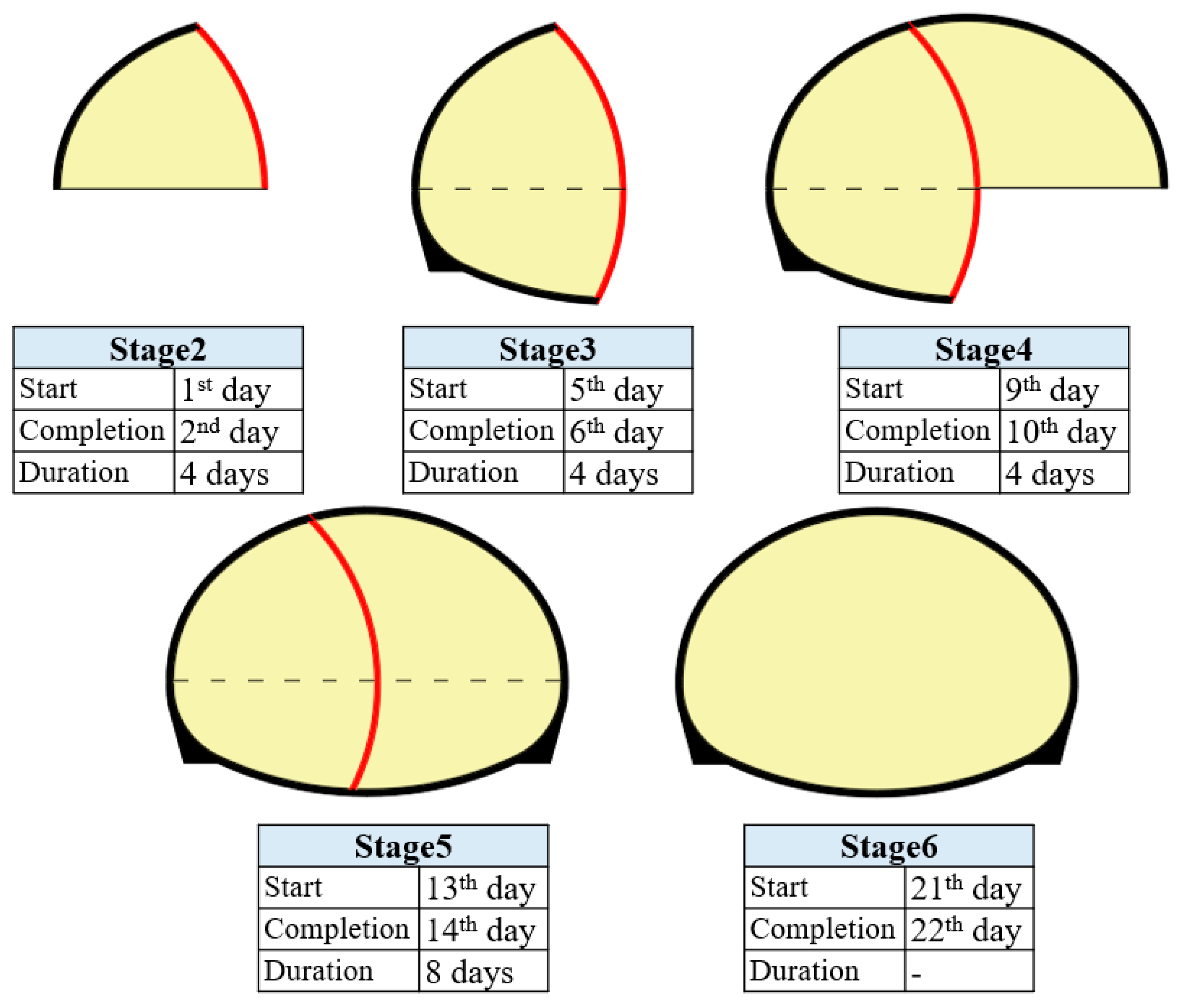
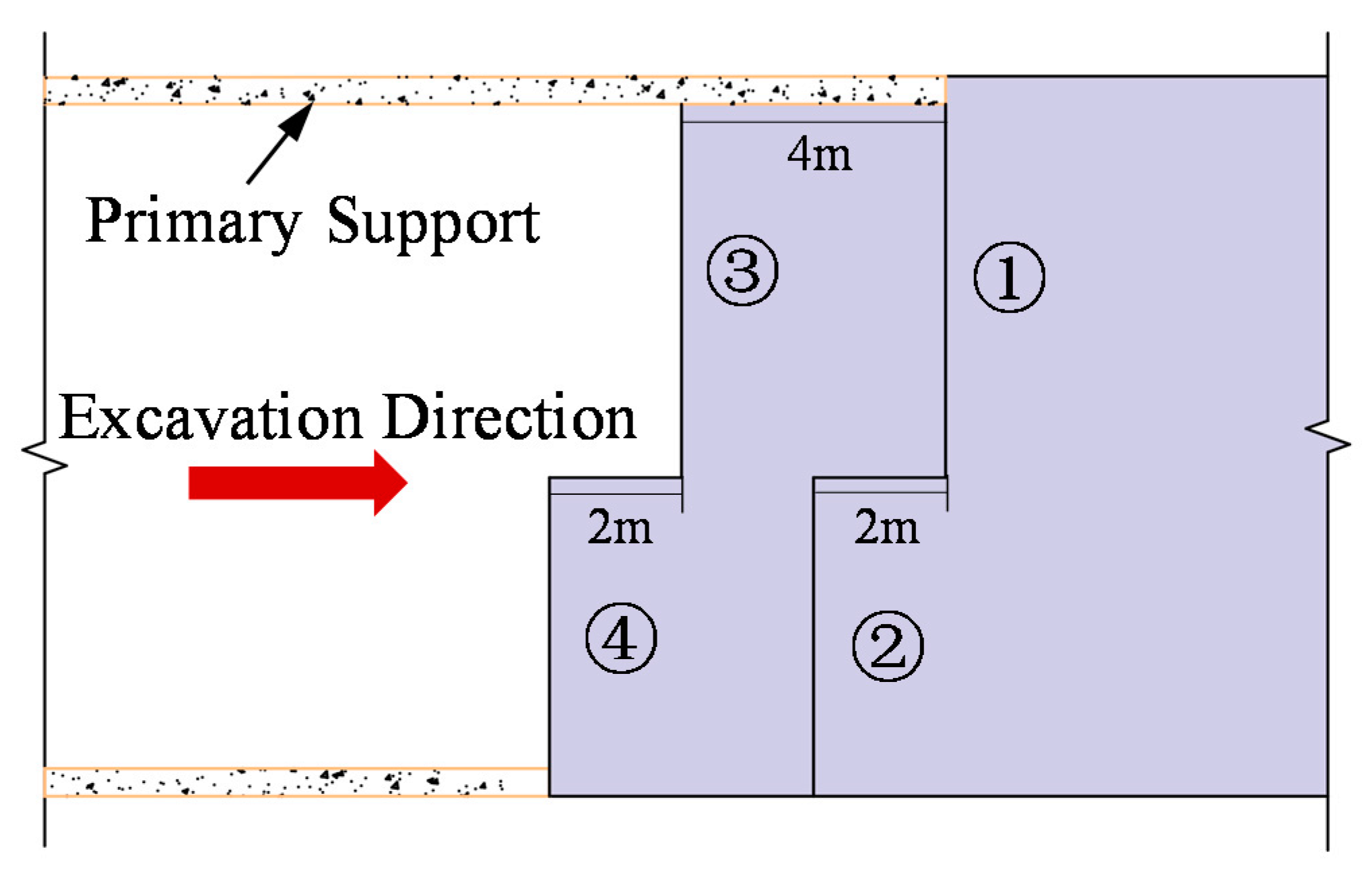
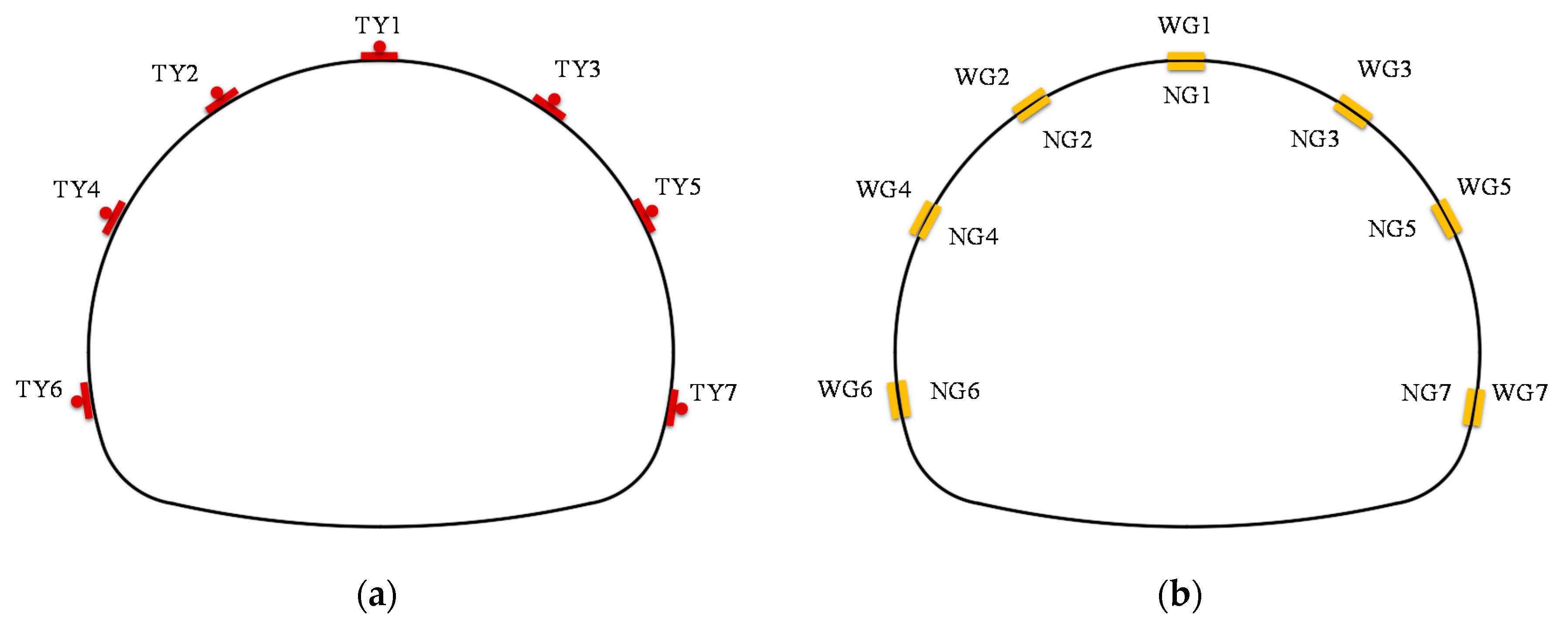
5.1. Scheme of Field Test
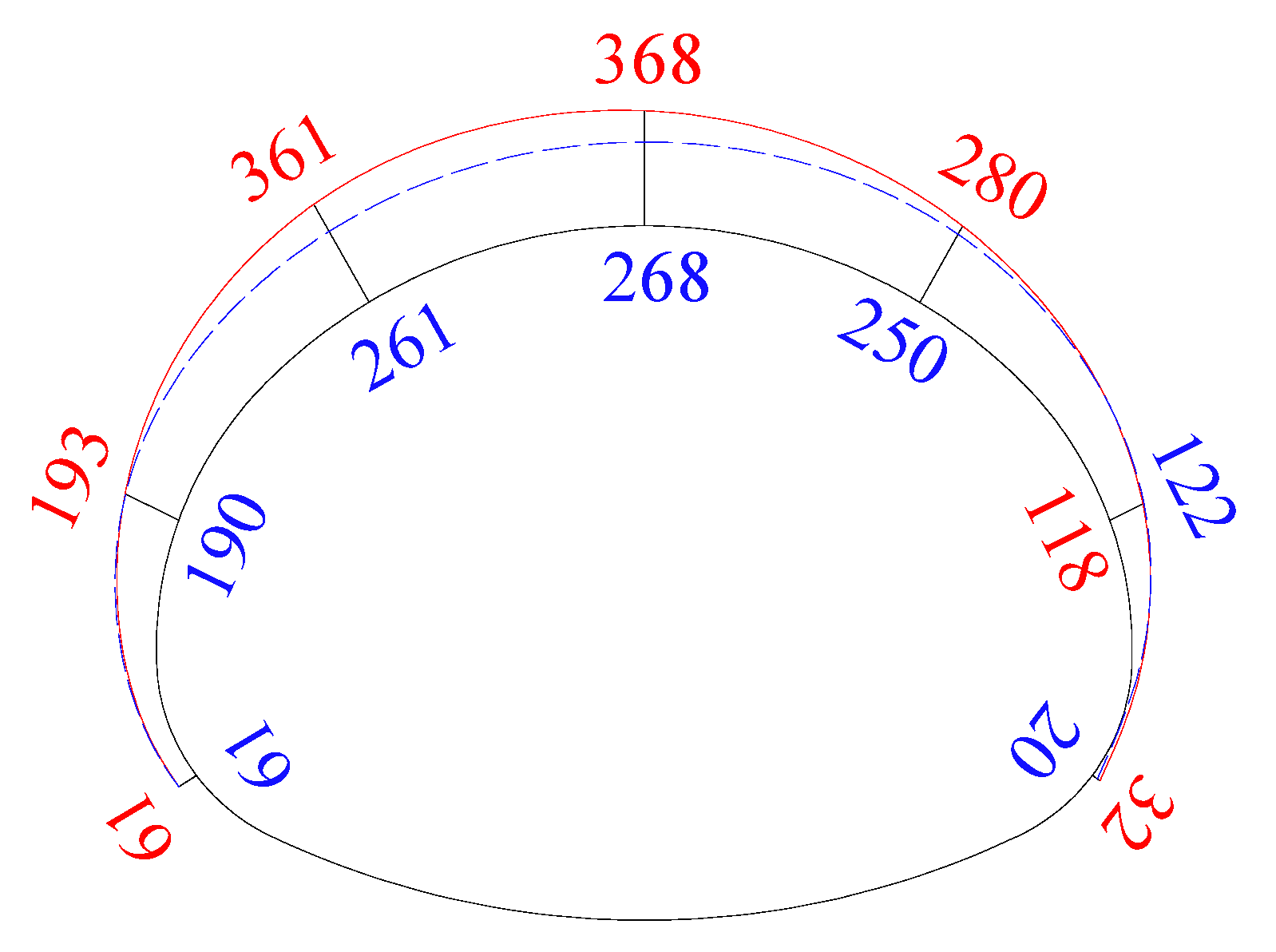
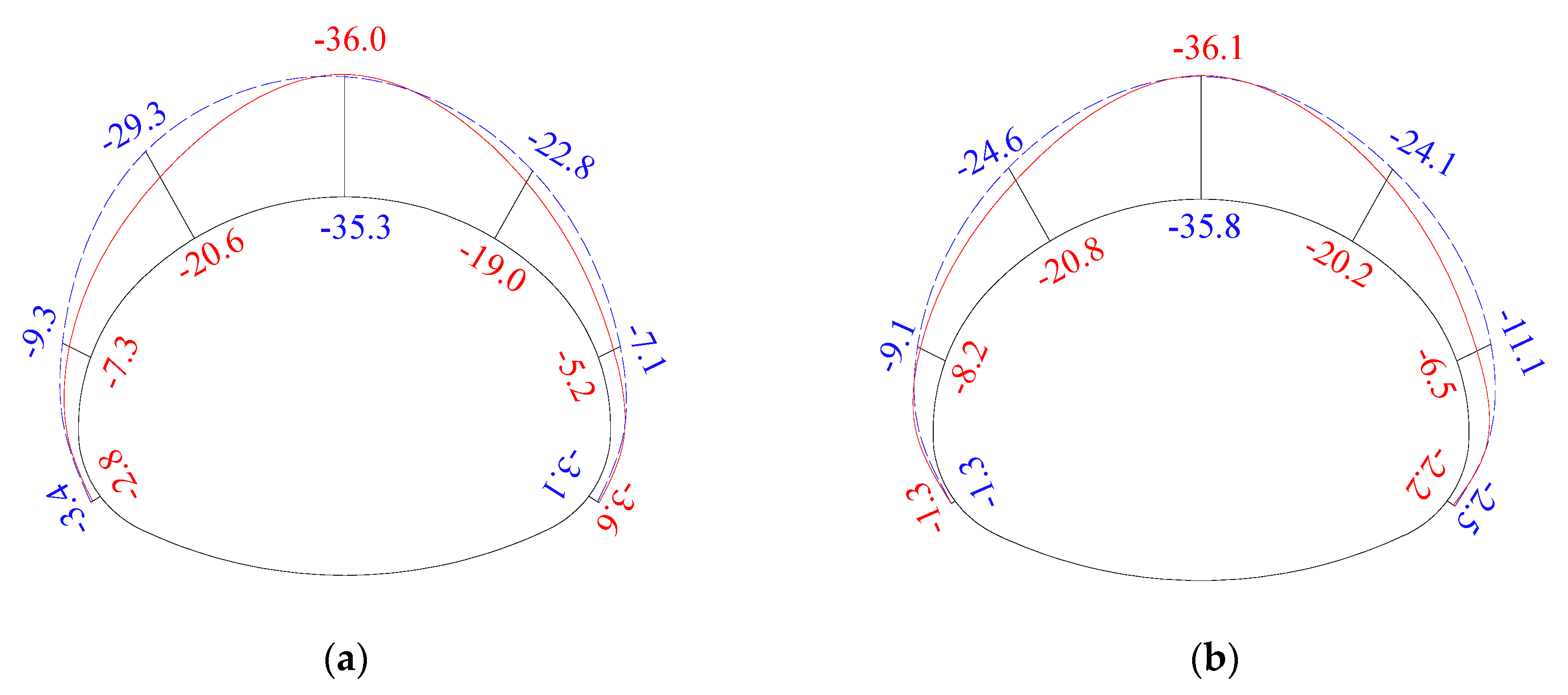
5.2. Settlement and Convergence
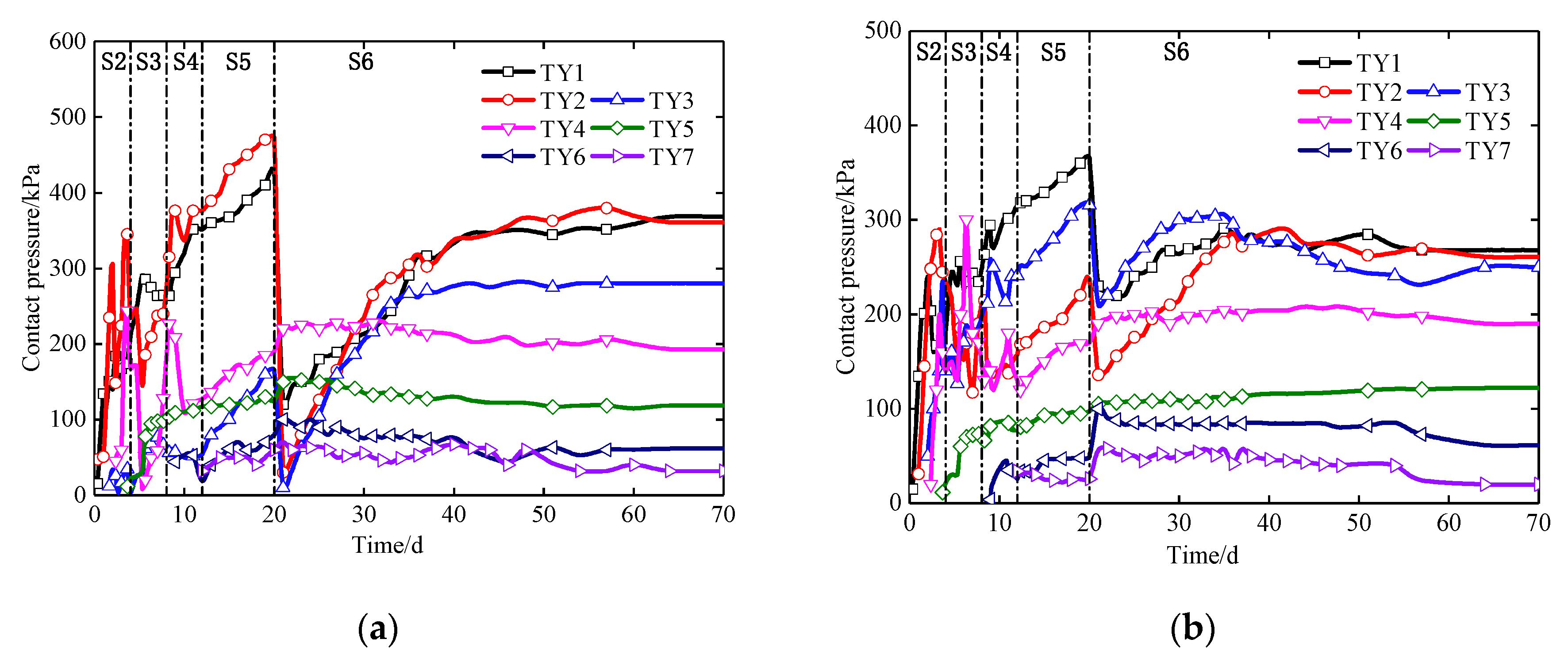
5.3. Surrounding Rock Pressure
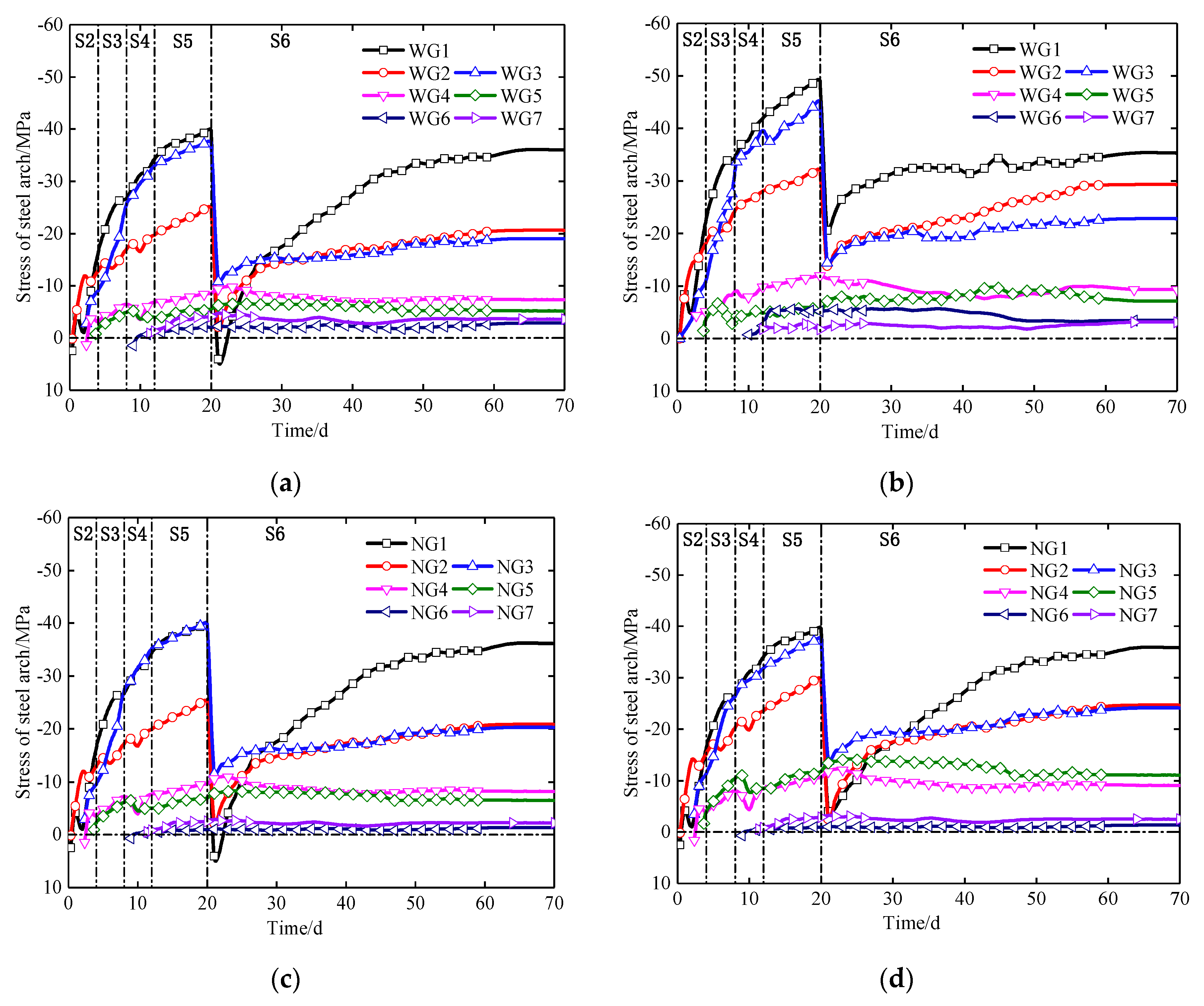
5.4. Stress on Steel Arch
5.5. Construction Efficiency and Cost
6. Conclusions
- In different buried depths, the deformation depends on the location of the disturbance area. For both the VCD and CD methods, if the buried depth changes from 0.5D to 4D, the surface settlement will increase first and then decrease, the crown settlement will increase first and then remain constant, the arch springing convergence will increase first and then decrease, and the side-wall convergence will remain constant;
- In different surrounding rock, the deformation depends on the scale of the disturbance area. For both the VCD and CD methods, if the surrounding rock changes from Grade IV1 to Grade V1, the surface settlement, the crown settlement, and the horizontal convergence will increase slowly. However, for Grade V2, the surface settlement, crown settlement, and horizontal convergence will increase rapidly;
- The VCD method could be an appropriate choice for tunnel construction when the buried depth is less than 4D and the surrounding rock is Grade IV1–V1. The numerical calculation results indicate that if the VCD method is adopted instead of the CD method, the surface settlement will be reduced by 14–29%, the crown settlement will be reduced by 16.2–46.0%, and the horizontal convergence will have no significant change. The surface settlement will be significantly reduced with the VCD method in the pre-excavation stage and the excavation stage, and the crown settlement will be significantly controlled in the excavation stage;
- If the VCD method is adopted in construction, the demolition of the center diaphragm may cause sudden changes in the surface settlement, the crown settlement, the horizontal convergence, the surrounding rock pressure, and the steel arch stress. It is recommended to eliminate potential safety hazards by demolishing the center diaphragm in stages and sections, taking appropriate auxiliary measures, and increasing the monitoring frequency;
- If the VCD method is adopted instead of the CD method, the erection time and material cost will be reduced by 10% and 5%, respectively. The manufacturing process of the vertical center diaphragm is simple, the connection quality is easier to be guaranteed, and the demolition is more convenient, which show significant economic and social benefits.
7. Patents
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, Z.G.; Huang, M.S. Geotechnical influence on existing subway tunnels induced by multiline tunneling in Shanghai soft soil. Comput. Geotech. 2014, 56, 121–132. [Google Scholar] [CrossRef]
- Jiang, M.; Yin, Z.Y. Influence of soil conditioning on ground deformation during longitudinal tunneling. Comptes Rendus Mécanique 2014, 342, 189–197. [Google Scholar] [CrossRef]
- Chen, R.P.; Meng, F.Y.; Li, Z.C.; Ye, Y.H.; Ye, J.N. Investigation of response of metro tunnels due to adjacent large excavation and protective measures in soft soils. Tunn. Undergr. Space Technol. 2016, 58, 224–235. [Google Scholar] [CrossRef]
- Wang, M.S. A summary and prospect of construction technologies for metro in China. Undergr. Space. 1998, 18, 98–103. (In Chinese) [Google Scholar]
- Sharifzadeh, M.; Kolivand, F.; Ghorbani, M.; Yasrobi, S. Design of sequential excavation method for large span urban tunnels in soft ground–Niayesh tunnel. Tunn. Undergr. Space Technol. 2013, 35, 178–188. [Google Scholar] [CrossRef]
- Hoek, E. Big tunnels in bad rock. J. Geotech. Geoenviron. Eng. 2001, 127, 726–740. [Google Scholar] [CrossRef]
- Daraei, A.; Zare, S.; Ghorbani, M. A new multi-graph approach for selecting the sequential excavation method of civil tunnels. Tunn. Undergr. Space Technol. 2019, 91, 102999. [Google Scholar] [CrossRef]
- Wang, L.C.; Hu, H.B.; Wang, S.B.; Jia, R.H. Study on construction methods for tunnels of large cross-section excavated in weak rocks. In Recent Advancement in Soil Behavior, in Situ Test Methods, Pile Foundations, and Tunneling; Ali, L., Correia, A.C., Yang, J.Y., Tao, M.J., Eds.; ASCE: Changsha, China, 2009; pp. 225–231. [Google Scholar] [CrossRef]
- Wu, K.; Zheng, Q.W.; Shao, Z.S.; Zhang, Z.L. Research into the effect of different tunnel excavation methods on rail track settlement. Indian Geotech. J. 2018, 48, 677–685. [Google Scholar] [CrossRef]
- Zhang, J.G. Study on construction method of double-track tunnel on passenger dedicated line by numerical simulation. Appl. Mech. Mater. 2012, 204–208, 1527–1531. [Google Scholar] [CrossRef]
- Bolghonabai, R.; Hossaini, M.F.; Mohammadi, M.; Nazem, A. On the selection of an appropriate excavation pattern for urban tunnels with big cross-section: A case study. Int. J. Min. Geo-Eng. 2015, 49, 297–307. [Google Scholar]
- Zhou, X.J.; Yang, C.Y. Study on Structural Design and Driving Process of a Subway Station with Large Transection by Tunneling Method. Appl. Mech. Mater. 2013, 353–356, 1325–1328. [Google Scholar] [CrossRef]
- Zhang, L. The analysis of lining stress and strain with working method from CRD to CD method. Geol. Prospect. 2007, 43, 93–98. (In Chinese) [Google Scholar]
- Hou, M.Z.; Zhang, Y.L.; Ding, C.L.; Shu, J. On construction parameters for a highway tunnel with large section. Mod. Tunn. Technol. 2014, 51, 36. (In Chinese) [Google Scholar] [CrossRef]
- Gao, H.J.; He, P.; Zheng, C.; Li, X.Y. A novel calculation method of process load for extra-large section tunnels. Symmetry 2019, 11, 1228. [Google Scholar] [CrossRef]
- Zhang, X.L. Research on the Stress Behavior of Surrounding Rock During Construction Adopting CD Method of Large Cross-Section Tunnel. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2005. (In Chinese). [Google Scholar]
- Meng, D.X. Research on Spatial Deformation Characteristics and Supporting Mechanism of Shallow Loess Tunnels with Large Section in High-Speed Railway. Ph.D. Thesis, Beijing Jiaotong University, Beijing, China, 2016. (In Chinese). [Google Scholar]
- Tan, Z.S.; Meng, D.X.; Shi, X.D.; Ji, T.G.; He, L.C. Research on support system and construction method for large-section small spacing tunnel in loess. China J. Highw. Transp. 2015, 28, 82–97. (In Chinese) [Google Scholar]
- Gao, H.J.; He, P.; Chen, Z.; Li, X.Y. Study on a surrounding rock pressure calculation method for super-large section highway tunnels. Symmetry 2019, 11, 1133. [Google Scholar] [CrossRef]
- Brinkgreve, R.B.J. Selection of soil models and parameters for geotechnical engineering. In Soil Constitutive Models: Evaluation, Selection, and Calibration; Yamanuro, J.A., Kaliakin, V.N., Eds.; ASCE: New York, NY, USA, 2005; pp. 69–98. [Google Scholar] [CrossRef]
- Roscoe, K.H.; Burland, J.B. On the generalized stress-strain behaviour of ‘wet’ clay. In Engineering Plasticity; Heyman, J., Lekie, F.A., Eds.; Cambridge University Press: London, UK, 1968; pp. 535–609. [Google Scholar]
- JTG/T D70-2010 Guidelines for Design of Highway Tunnel; People’s Communications Publishing House: Beijing, China, 2010.
- JTG D70-2010 Code for Design of Road Tunnels; People’s Communications Publishing House: Beijing, China, 2010.
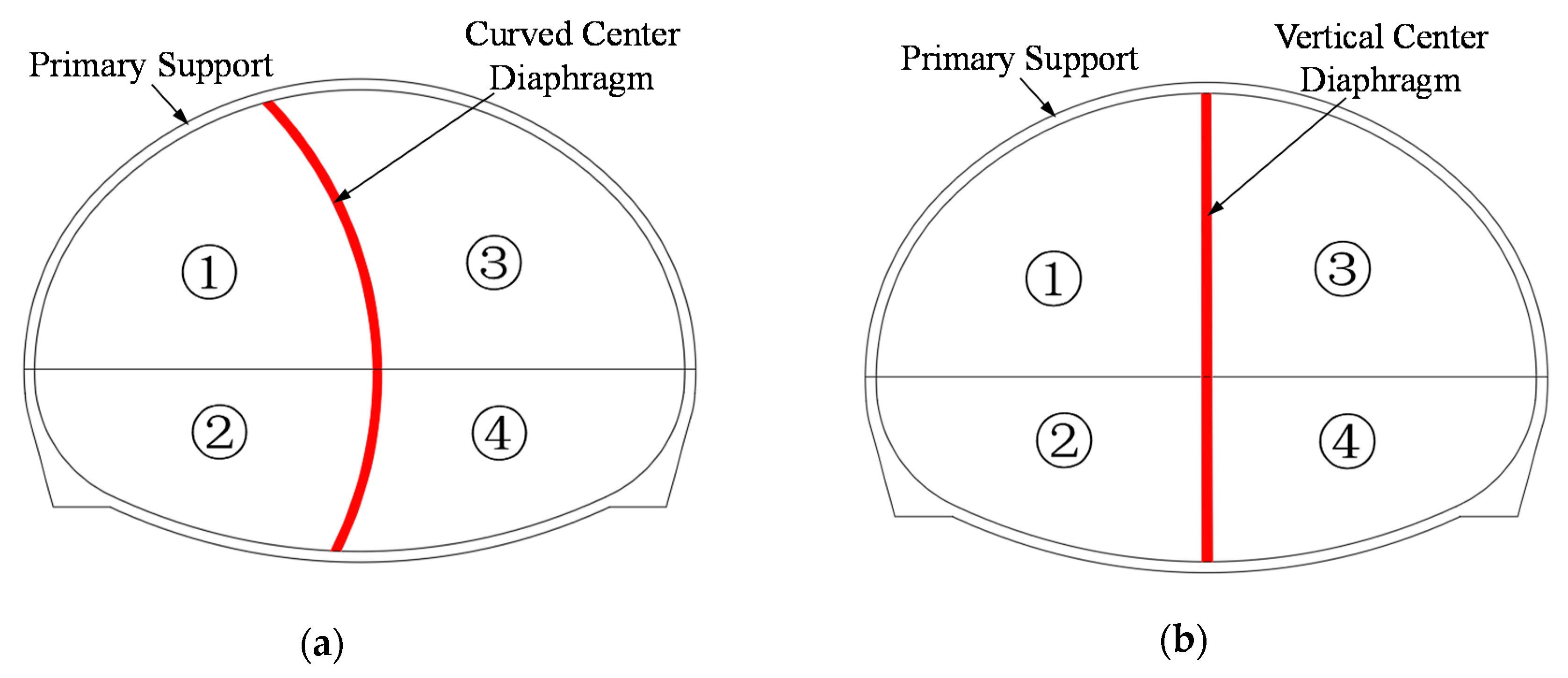
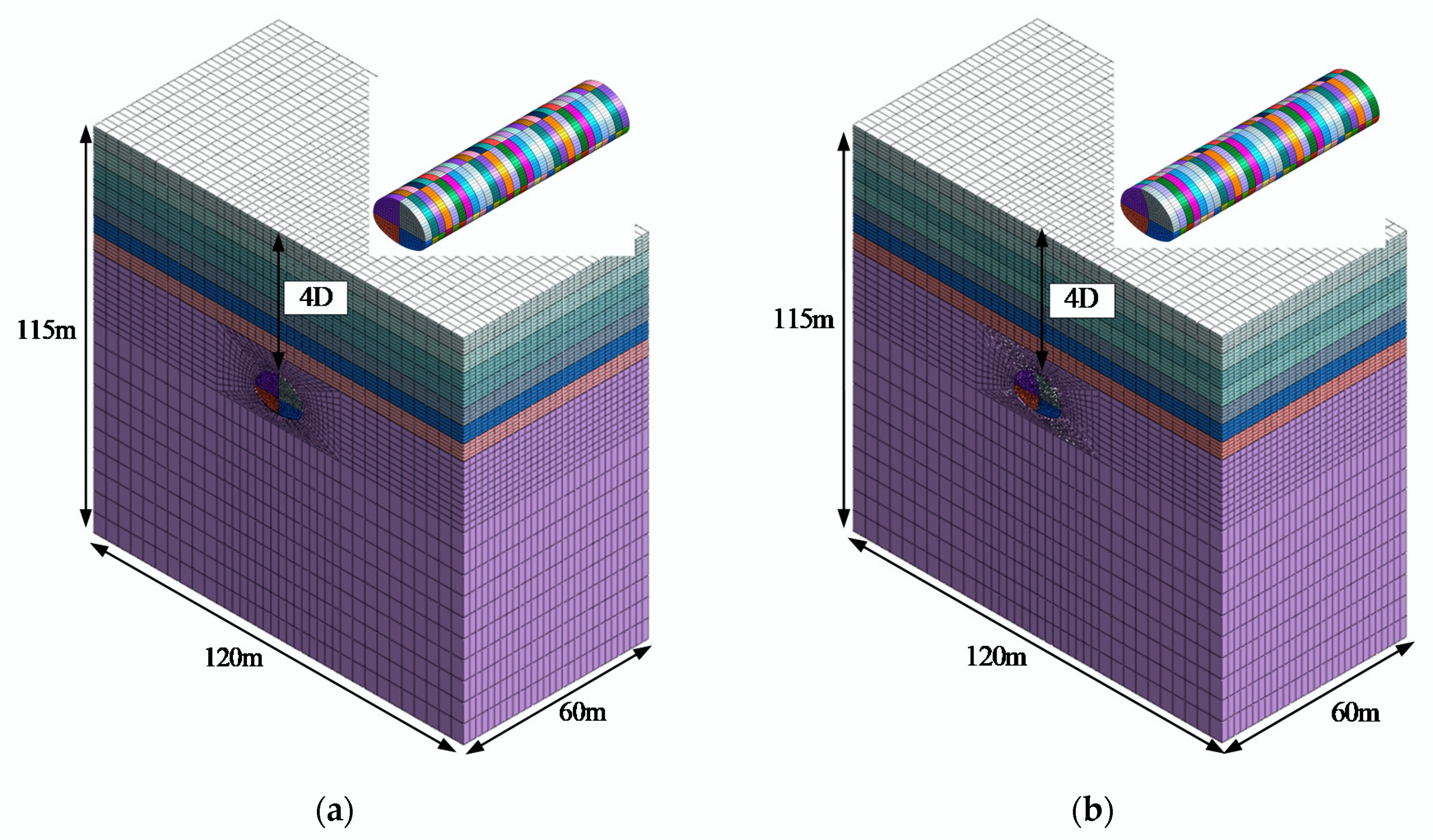
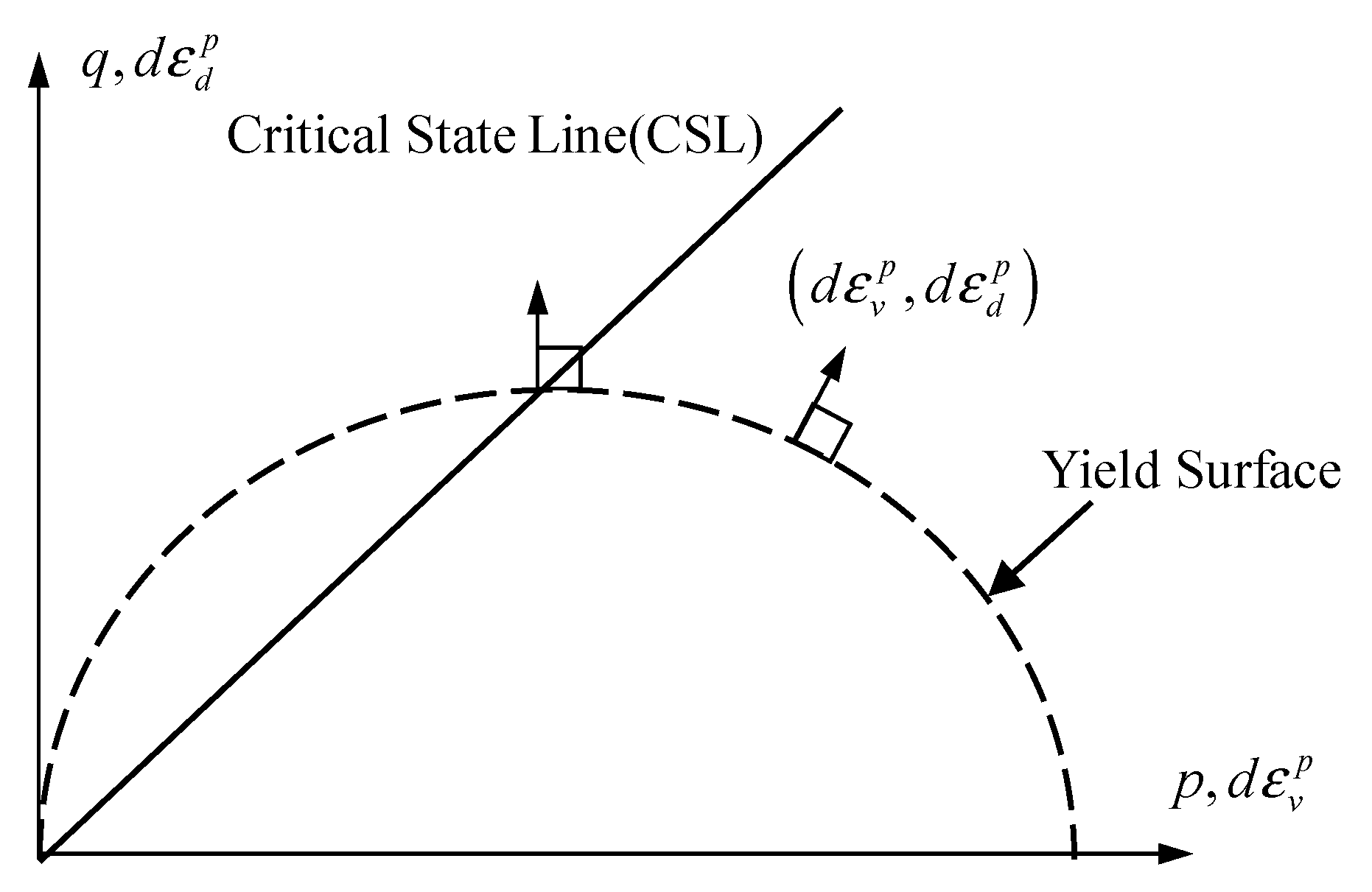
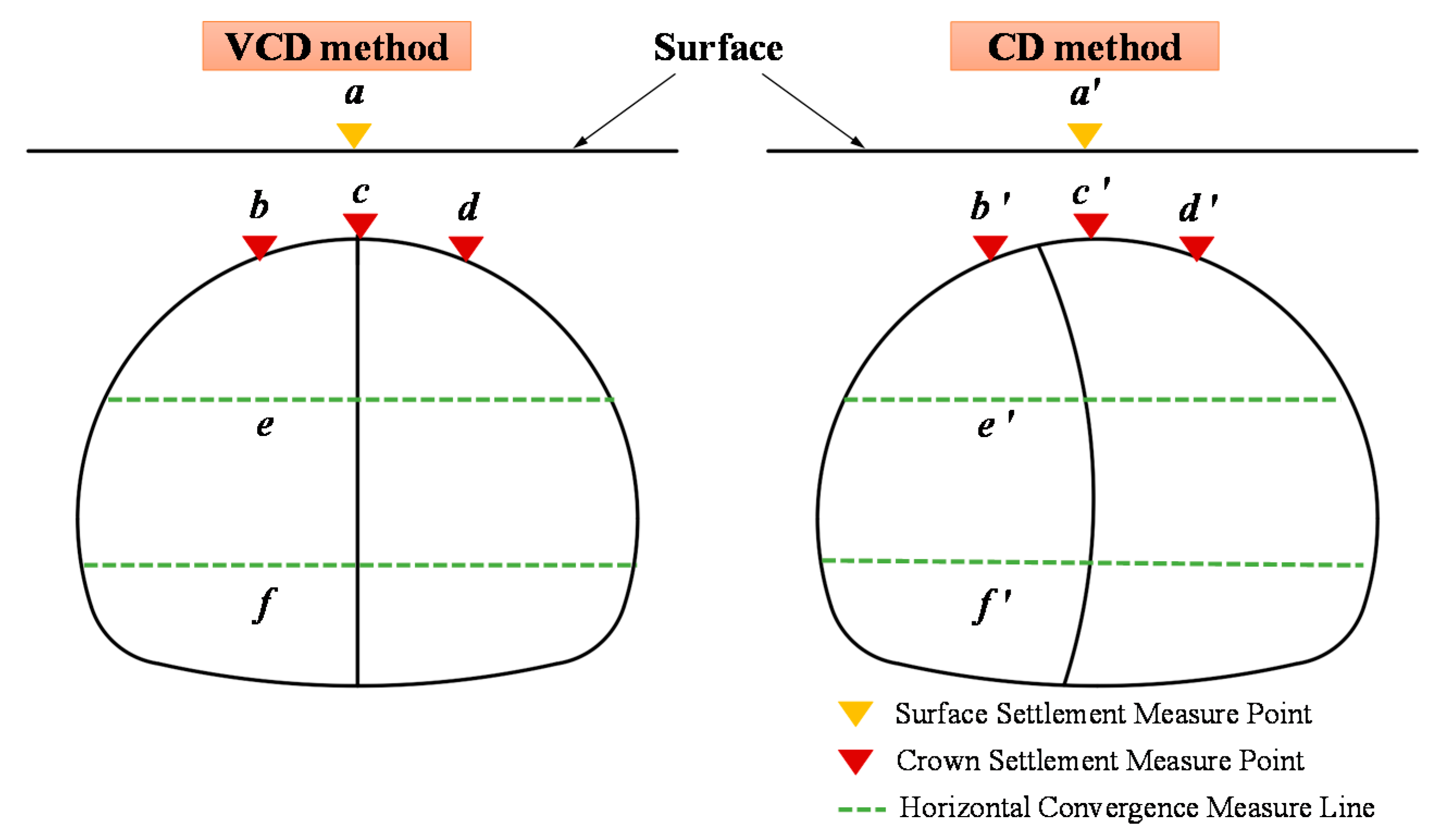




| Dimension of Z (m) | 80 | 85 | 90 | 95 | 100 | 105 | 110 | 115 | |
|---|---|---|---|---|---|---|---|---|---|
| VCD method | Element | 40710 | 46110 | 51510 | 56910 | 62310 | 67710 | 73110 | 78510 |
| Node | 43524 | 49259 | 54994 | 60729 | 66464 | 72199 | 77934 | 83669 | |
| CD method | Element | 41220 | 46620 | 52020 | 57420 | 62820 | 68220 | 73620 | 79020 |
| Node | 44051 | 49786 | 55521 | 61256 | 66991 | 72726 | 78461 | 84196 | |
| Grades | I | II | III | IV | V | ||||
|---|---|---|---|---|---|---|---|---|---|
| III1 | III2 | IV1 | IV2 | IV3 | V1 | V2 | |||
| [BQ]min | 551 | 451 | 401 | 351 | 316 | 285 | 251 | 211 | 150 |
| [BQ]max | - | 550 | 450 | 400 | 350 | 315 | 284 | 250 | 210 |
| Number | Surrounding Rock Grades | Buried Depth | |
|---|---|---|---|
| Group 1 | 1-1 | IV3 | 5m/0.5D |
| 1-2 | IV3 | 10m/1.0D | |
| 1-3 | IV3 | 15m/1.5D | |
| 1-4 | IV3 | 20m/2.0D | |
| 1-5 | IV3 | 25m/2.5D | |
| 1-6 | IV3 | 30m/3.0D | |
| 1-7 | IV3 | 35m/3.5D | |
| 1-8 | IV3 | 40m/4.0D | |
| Group 2 | 2-1 | IV1 | 20m/2.0D |
| 2-2 | IV2 | 20m/2.0D | |
| 2-3 | IV3 | 20m/2.0D | |
| 2-4 | V1 | 20m/2.0D | |
| 2-5 | V2 | 20m/2.0D |
| Parameter | Description (Unit) | Grade | ||||
|---|---|---|---|---|---|---|
| IV1 | IV2 | IV3 | V1 | V2 | ||
| γ | Unit weight (kN/m3) | 22.5 | 21.5 | 20.5 | 19.0 | 17.5 |
| M | Stress ratio at the characteristic state point | 0.80 | 0.70 | 0.60 | 0.45 | 0.35 |
| μ | Poisson’s ratio | 0.31 | 0.32 | 0.34 | 0.37 | 0.42 |
| λ | Slope of normal consolidation line | 0.080 | 0.083 | 0.086 | 0.090 | 0.093 |
| κ | Slope of elastic swelling line | 0.008 | 0.008 | 0.008 | 0.009 | 0.009 |
| Nr | Reference specific volume | 1.84 | 1.87 | 1.90 | 1.93 | 1.96 |
| Parameter | Description (Unit) | Center Diaphragm | Primary Support |
|---|---|---|---|
| E | Young’s modulus (MPa) | 25E3 | 25E3 |
| ν | Poisson’s ratio | 0.2 | 0.2 |
| γ | Unit weight (kN/m3) | 22 | 22 |
| Parameter(Unit) | Miscellaneous Fill | Gravel Sand | Breccia | Metamorphosed Quartz Sandstone |
|---|---|---|---|---|
| γ (kN/m3) | 22.5 | 21.5 | 20.5 | 19.0 |
| M | 0.80 | 0.70 | 0.60 | 0.45 |
| μ | 0.31 | 0.32 | 0.34 | 0.37 |
| λ | 0.080 | 0.083 | 0.086 | 0.090 |
| κ | 0.008 | 0.008 | 0.008 | 0.009 |
| Nr | 1.84 | 1.87 | 1.90 | 1.93 |
| No. | Vertical Center Diaphragm | Curved Center Diaphragm | ||
|---|---|---|---|---|
| Upper Part | Lower Part | Upper Part | Lower Part | |
| 1 | 149 | 102 | 154 | 97 |
| 2 | 120 | 96 | 165 | 107 |
| 3 | 149 | 84 | 152 | 105 |
| 4 | 149 | 99 | 156 | 115 |
| 5 | 132 | 101 | 152 | 115 |
| 6 | 143 | 96 | 153 | 101 |
| 7 | 119 | 98 | 167 | 108 |
| 8 | 129 | 97 | 165 | 107 |
| 9 | 147 | 91 | 157 | 112 |
| 10 | 143 | 96 | 170 | 113 |
| Average time | 138 | 96 | 159 | 108 |
| Standard deviation | 11.38 | 4.94 | 6.55 | 5.66 |
| Type | Length (m) | Steel Consumption (kg) | Price of Steel (yuan/kg) | Unit Price (yuan) |
|---|---|---|---|---|
| Vertical center diaphragm | 11.25 | 349.5 | 4.5 | 1573 |
| Curved center diaphragm | 11.81 | 366.9 | 4.5 | 1651 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z.; Tan, Z.; Zhao, J.; Liang, H. Development and Research on the Vertical Center Diaphragm Method Applied in Shallow Tunnel Construction. Symmetry 2020, 12, 855. https://doi.org/10.3390/sym12050855
Zhou Z, Tan Z, Zhao J, Liang H. Development and Research on the Vertical Center Diaphragm Method Applied in Shallow Tunnel Construction. Symmetry. 2020; 12(5):855. https://doi.org/10.3390/sym12050855
Chicago/Turabian StyleZhou, Zhenliang, Zhongsheng Tan, Jinpeng Zhao, and Han Liang. 2020. "Development and Research on the Vertical Center Diaphragm Method Applied in Shallow Tunnel Construction" Symmetry 12, no. 5: 855. https://doi.org/10.3390/sym12050855
APA StyleZhou, Z., Tan, Z., Zhao, J., & Liang, H. (2020). Development and Research on the Vertical Center Diaphragm Method Applied in Shallow Tunnel Construction. Symmetry, 12(5), 855. https://doi.org/10.3390/sym12050855







