A Brief Review of Chiral Chemical Potential and Its Physical Effects
Abstract
1. Introduction
1.1. The Problem, Chiral Anomaly, and Instantons
1.2. -Vacuum and Strong CP Problem
1.3. Event by Event P and CP Violation and Chiral Chemical Potential
2. The Effects of Chiral Imbalance
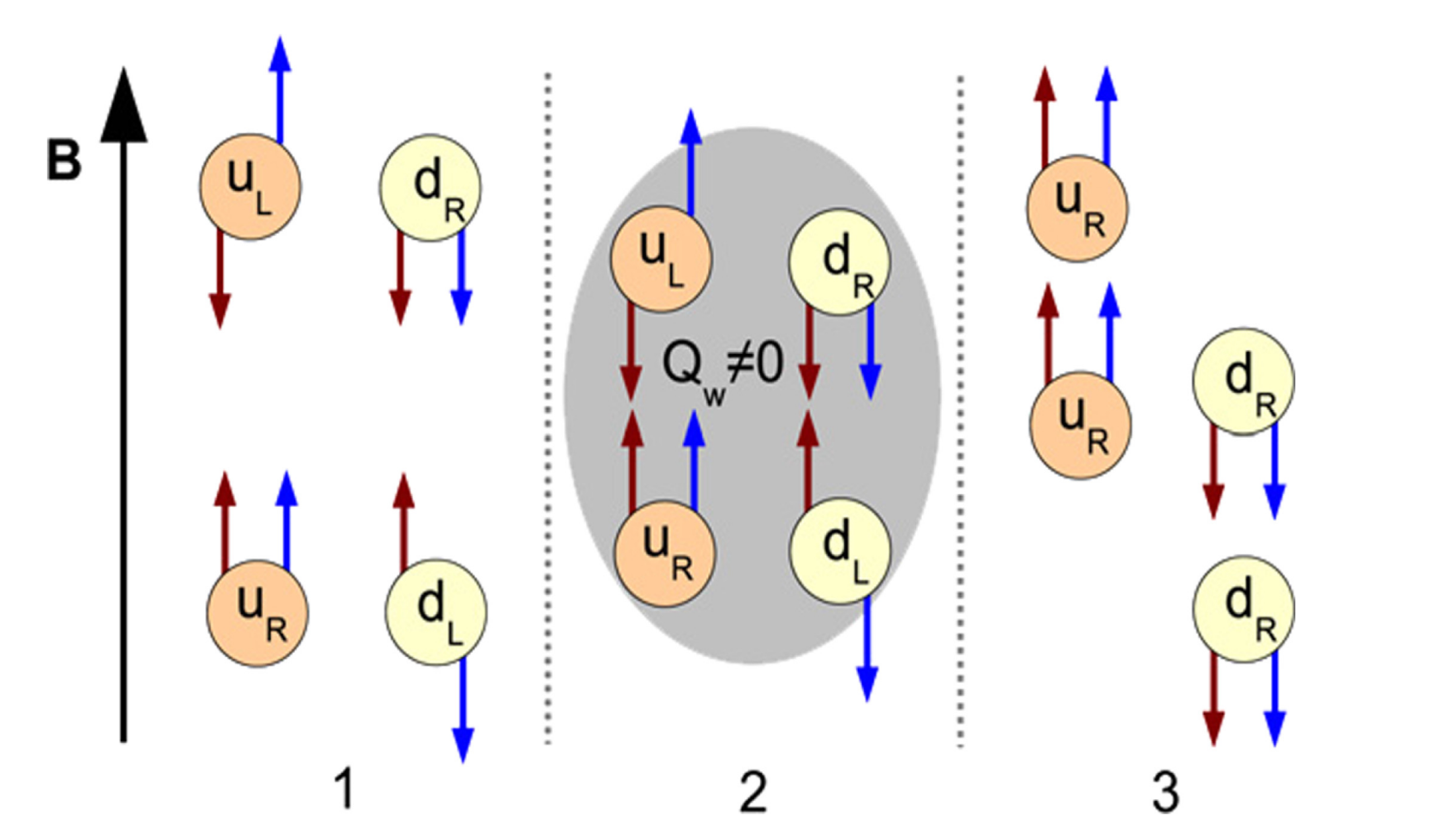
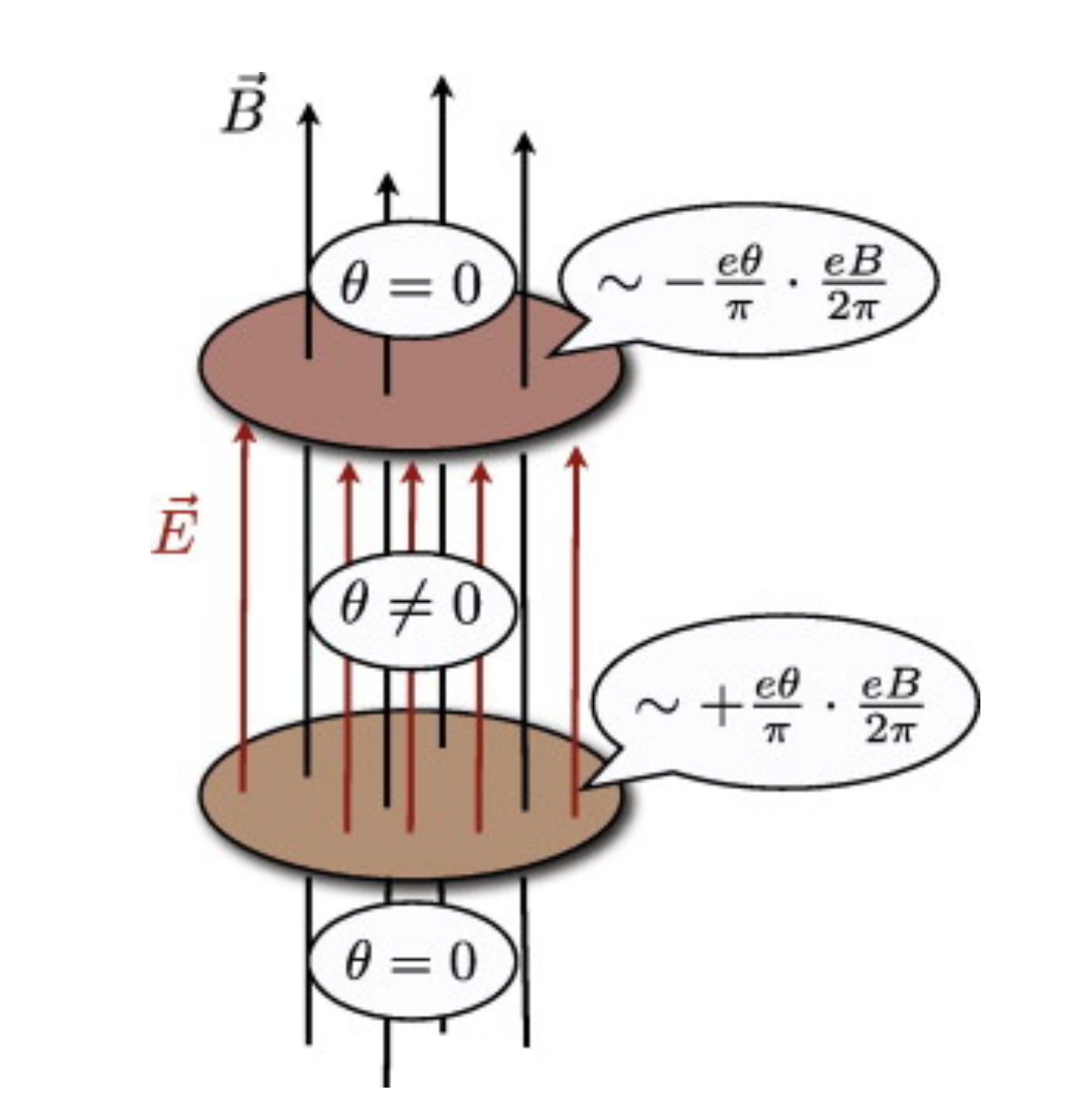
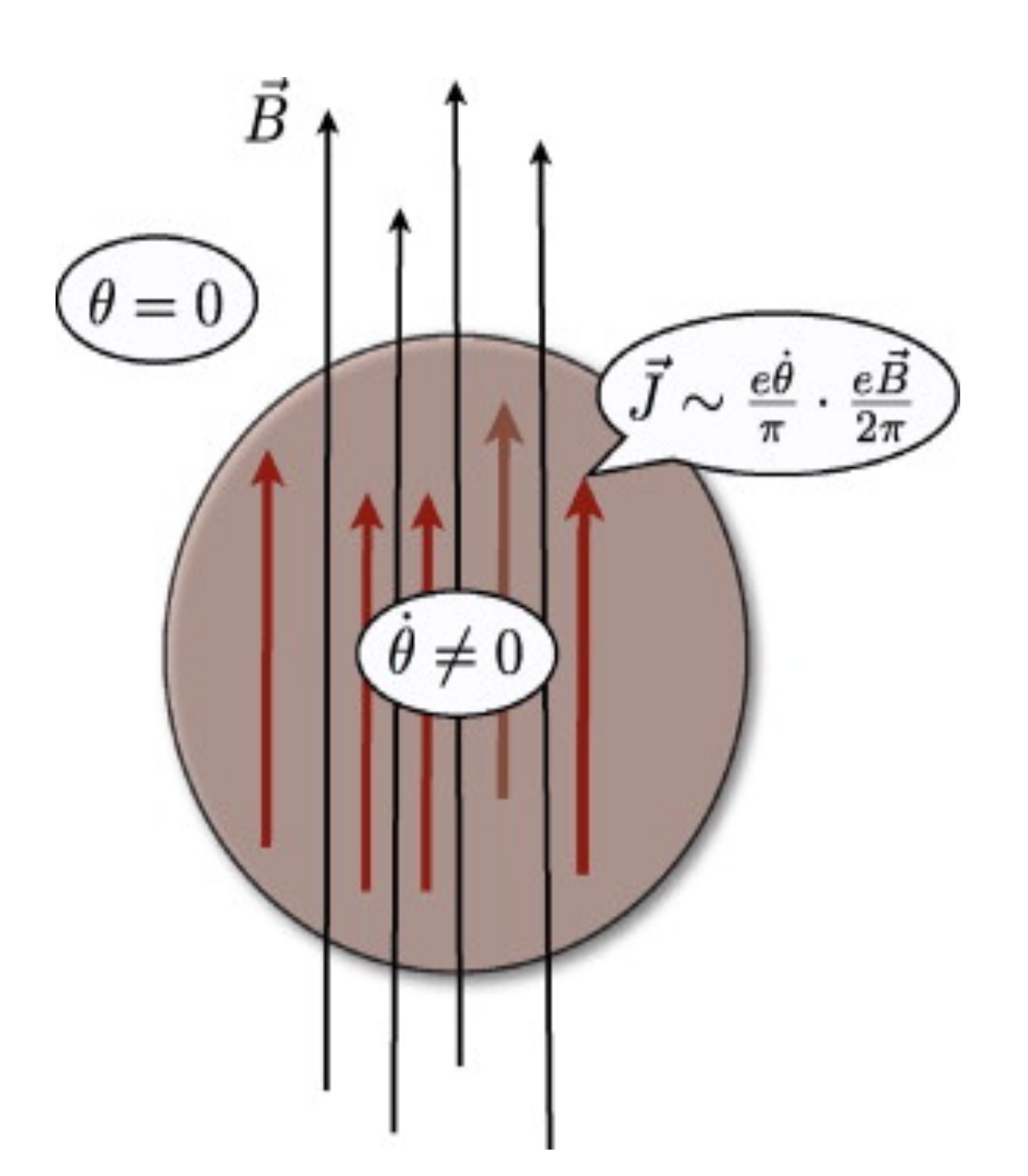
2.1. The Charge Separation Effect (CSE) and Chiral Magnetic Effect (CME)
2.2. The Effects of Chiral Chemical Potential on QCD Phase Structure
2.3. The Effects of Chiral Chemical Potential on Quark Stars
3. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hooft, G.T. Symmetry Breaking through Bell-Jackiw Anomalies. Phys. Rev. Lett. Phys. 1976, 37, 8–11. [Google Scholar] [CrossRef]
- Hooft, G.T. Computation of the quantum effects due to a four-dimensional pseudoparticle. Phys. Rev. D 1976, 14, 3432–3450. [Google Scholar] [CrossRef]
- Adler, S.L. Axial-Vector Vertex in Spinor Electrodynamics. Phys. Rev. 1969, 177, 2426–2438. [Google Scholar] [CrossRef]
- Bell, J.S.; Jackiw, R. A PCAC puzzle: π0γγ in the σ-model. Nuovo Cimento A Ser. 1969, 60, 47–61. [Google Scholar] [CrossRef]
- Fujikawa, K. Path-Integral Measure for Gauge-Invariant Fermion Theories. Phys. Rev. Lett. 1979, 42, 1195–1198. [Google Scholar] [CrossRef]
- Crewther, R.J. Effects of Topological Charge in Gauge Theories. Act. Phys. Austriaca Suppl. 1978, 19, 47–153. [Google Scholar]
- Bogomolny, E.B. Stability of Classical Solutions. Sov. J. Nucl. Phys. 1976, 24, 449. [Google Scholar]
- Callan, C.G.; Dashen, R.F.; Gross, D.J. The structure of the gauge theory vacuum. Phys. Lett. B 1976, 63, 334–340. [Google Scholar] [CrossRef]
- Jackiw, R.; Rebbi, C. Vacuum Periodicity in a Yang-Mills Quantum Theory. Phys. Rev. Lett. 1976, 37, 172–175. [Google Scholar] [CrossRef]
- Weinberg, S. The Quantum Theory of Fields; Volume 2: Modern Applications; Cambridge University Press: Cambridge, UK, 2013; pp. 455–461. [Google Scholar]
- Baker, C.A.; Doyle, D.D.; Geltenbort, P.; Green, K.; Van der Grinten, M.G.D.; Harris, P.G.; Iaydjiev, P.; Ivanov, S.N.; May, D.J.R.; Pendlebury, J.M.; et al. Improved Experimental Limit on the Electric Dipole Moment of the Neutron. Phys. Rev. Lett. 2006, 97, 131801. [Google Scholar] [CrossRef]
- Baluni, V. CP-nonconserving effects in quantum chromodynamics. Phys. Rev. D 1979, 19, 2227–2230. [Google Scholar] [CrossRef]
- Crewther, R.; Vecchia, P.D.; Veneziano, G.; Witten, E. Chiral estimate of the electric dipole moment of the neutron in quantum chromodynamics. Phys. Lett. B 1979, 88, 123–127. [Google Scholar] [CrossRef]
- Peccei, R.D.; Quinn, H.R. CP Conservation in the Presence of Pseudoparticles. Phys. Rev. Lett. 1977, 38, 1440–1443. [Google Scholar] [CrossRef]
- Peccei, R.D.; Quinn, H.R. Constraints imposed by CP conservation in the presence of pseudoparticles. Phys. Rev. D 1977, 16, 1791–1797. [Google Scholar] [CrossRef]
- Barr, S.M. Solving the Strong CP Problem without the Peccei–Quinn Symmetry. Phys. Rev. Lett. 1984, 53, 329–332. [Google Scholar] [CrossRef]
- Nelson, A. Naturally weak CP violation. Phys. Lett. B 1984, 136, 387–391. [Google Scholar] [CrossRef]
- Kim, J.E.; Carosi, G. Axions and the Strong CP Problem. Rev. Mod. Phys. 2010, 82, 557–602. [Google Scholar] [CrossRef]
- Wilczek, F. Problem of Strong P and T Invariance in the Presence of Instantons. Phys. Rev. Lett. 1978, 40, 279–282. [Google Scholar] [CrossRef]
- Weinberg, S. A New Light Boson? Phys. Rev. Lett. 1978, 40, 223–226. [Google Scholar] [CrossRef]
- Peccei, R.D. The Strong CP Problem and Axions. In Axions: Theory, Cosmology, and Experimental Searches; Kuster, M., Raffelt, G., Beltrán, B., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 3–17. [Google Scholar]
- Cheng, H.Y. The strong CP problem revisited. Phys. Rep. 1988, 158, 1–89. [Google Scholar] [CrossRef]
- Schwartz, M.D. Quantum Field Theory and the Standard Model; Cambridge University Press: Cambridge, UK, 2014; pp. 609–613. [Google Scholar]
- Bernard, C. Gauge zero modes, instanton determinants, and quantum-chromodynamic calculations. Phys. Rev. D 1979, 19, 3013–3019. [Google Scholar] [CrossRef]
- Pisarski, R.D.; Yaffe, L.G. The density of instantons at finite temperature. Phys. Lett. B 1980, 97, 110–112. [Google Scholar] [CrossRef]
- McLerran, L.; Mottola, E.; Shaposhnikov, M.E. Sphalerons and axion dynamics in high-temperature QCD. Phys. Rev. D 1991, 43, 2027–2035. [Google Scholar] [CrossRef] [PubMed]
- Moore, G.D.; Tassler, M. The sphaleron rate in SU(N) gauge theory. J. High Energy Phys. 2011, 2, 105. [Google Scholar] [CrossRef]
- Kharzeev, D.E.; McLerran, L.D.; Warringa, H.J. The Effects of topological charge change in heavy ion collisions: ‘Event by event P and CP violation’. Nucl. Phys. A 2008, 803, 227–253. [Google Scholar] [CrossRef]
- Kharzeev, D.; Pisarski, R.D.; Tytgat, M.H. Possibility of Spontaneous Parity Violation in Hot QCD. Phys. Rev. Lett. 1998, 81, 512–515. [Google Scholar] [CrossRef]
- Kharzeev, D. Parity violation in hot QCD: Why it can happen, and how to look for it. Phys. Lett. B 2006, 633, 260–264. [Google Scholar] [CrossRef]
- Kharzeev, D.; Krasnitz, A.; Venugopalan, R. Anomalous chirality fluctuations in the initial stage of heavy ion collisions and parity odd bubbles. Phys. Lett. B 2002, 545, 298–306. [Google Scholar] [CrossRef]
- Kharzeev, D.E.; Pisarski, R.D.; Tytgat, M.H. Aspects of parity, CP, and time reversal violation in hot QCD. arXiv 2001, arXiv:2000.12012. [Google Scholar]
- Buckley, K.; Fugleberg, T.; Zhitnitsky, A. Can Induced Θ Vacua be Created in Heavy-Ion Collisions? Phys. Rev. Lett. 2000, 84, 4814–4817. [Google Scholar] [CrossRef]
- Buckley, K.; Fugleberg, T.; Zhitnitsky, A. Induced θ-vacuum states in heavy ion collisions: A possible signature. Phys. Rev. C 2001, 63, 034602. [Google Scholar] [CrossRef]
- Kharzeev, D.E. Chern–Simons current and local parity violation in hot QCD matter. Nucl. Phys. A 2009, 830, 543c–546c. [Google Scholar] [CrossRef]
- Kharzeev, D.E. Topologically induced local P and CP violation in QCD × QED. Ann. Phys. 2010, 325, 205–218. [Google Scholar] [CrossRef]
- Fukushima, K.; Kharzeev, D.E.; Warringa, H.J. Chiral magnetic effect. Phys. Rev. D 2008, 78, 074033. [Google Scholar] [CrossRef]
- Jacobs, P. Measurements of high density matter at RHIC. eConf 2002, C020805, TTH05. [Google Scholar]
- Arsene, I.; Bearden, I.G.; Beavis, D.; Besliu, C.; Budick, B.; Bøggild, H.; Chasman, C.; Christensen, C.H.; Christiansen, P.; Cibor, J.; et al. Quark gluon plasma and color glass condensate at RHIC? The Perspective from the BRAHMS experiment. Nucl. Phys. A 2005, 757, 1–27. [Google Scholar] [CrossRef]
- Adams, J.; Aggarwal, M.M.; Ahammed, Z.; Amonett, J.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Badyal, S.K.; Bai, Y.; Balewski, J.; et al. Experimental and theoretical challenges in the search for the quark gluon plasma: The STAR Collaboration’s critical assessment of the evidence from RHIC collisions. Nucl. Phys. A 2005, 757, 102–183. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, F. Experimental searches for the chiral magnetic effect in heavy-ion collisions. Prog. Part. Nucl. Phys. 2019, 107, 200–236. [Google Scholar] [CrossRef]
- Wilczek, F. Two applications of axion electrodynamics. Phys. Rev. Lett. 1987, 58, 1799–1802. [Google Scholar] [CrossRef]
- Kharzeev, D.; Zhitnitsky, A. Charge separation induced by P-odd bubbles in QCD matter. Nucl. Phys. A 2007, 797, 67–79. [Google Scholar] [CrossRef]
- Collins, J.C.; Perry, M.J. Superdense Matter: Neutrons or Asymptotically Free Quarks? Phys. Rev. Lett. 1975, 34, 1353–1356. [Google Scholar] [CrossRef]
- Meyer-Ortmanns, H. Phase transitions in quantum chromodynamics. Rev. Mod. Phys. 1996, 68, 473–598. [Google Scholar] [CrossRef]
- Ruggieri, M.; Peng, G.X.; Chernodub, M. Chiral relaxation time at the crossover of quantum chromodynamics. Phys. Rev. D 2016, 94, 054011. [Google Scholar] [CrossRef]
- Ruggieri, M.; Peng, G.X. Quark matter in a parallel electric and magnetic field background: Chiral phase transition and equilibration of chiral density. Phys. Rev. D 2016, 93, 094021. [Google Scholar] [CrossRef]
- Ruggieri, M.; Chernodub, M.N.; Lu, Z.-Y. Topological susceptibility, divergent chiral density, and phase diagram of chirally imbalanced QCD medium at finite temperature. Phys. Rev. D 2020, 102, 014031. [Google Scholar] [CrossRef]
- Yamamoto, A. Chiral Magnetic Effect in Lattice QCD with a Chiral Chemical. Phys. Rev. Lett. 2011, 107, 031601. [Google Scholar] [CrossRef] [PubMed]
- Braguta, V.V.; Goy, V.A.; Ilgenfritz, E.M.; Kotov, A.Y.; Molochkov, A.V.; Müller-Preussker, M.; Petersson, B. Two-Color QCD with Chiral Chemical Potential. J. High Energy Phys. 2015, 2015, 94. [Google Scholar] [CrossRef]
- Braguta, V.V.; Ilgenfritz, E.-M.; Kotov, A.Y.; Petersson, B.; Skinderev, S.A. Study of the QCD phase diagram with a nonzero chiral chemical potential. Phys. Rev. D 2016, 93, 034509. [Google Scholar] [CrossRef]
- Braguta, V.V.; Kotov, A.Y. Catalysis of dynamical chiral symmetry breaking by chiral chemical potential. Phys. Rev. D 2016, 93, 105025. [Google Scholar] [CrossRef]
- Fukushima, K.; Ruggieri, M.; Gatto, R. Chiral magnetic effect in the Polyakov–Nambu–Jona–Lasinio model. Phys. Rev. D 2010, 81, 114031. [Google Scholar] [CrossRef]
- Yang, L.K.; Luo, X.; Zong, H.S. QCD phase diagram in chiral imbalance with self-consistent mean field approximation. Phys. Rev. D 2019, 100, 094012. [Google Scholar] [CrossRef]
- Shi, C.; He, X.-T.; Jia, W.-B.; Wang, Q.-W.; Xu, S.-S.; Zong, H.-S. Chiral transition and the chiral charge density of the hot and dense QCD matter. J. High Energy Phys. 2020, 2020, 122. [Google Scholar] [CrossRef]
- Shi, C.; He, X.-T.; Jia, W.-B.; Wang, Q.-W.; Xu, S.-S.; Zong, H.-S. QCD phase diagram with a chiral chemical potential. Phys. Rev. D 2016, 93, 074037. [Google Scholar]
- Yu, L.; Liu, H.; Huang, M. Effect of the chiral chemical potential on the chiral phase transition in the NJL model with different regularization schemes. Phys. Rev. D 2016, 94, 014026. [Google Scholar] [CrossRef]
- Wang, F.; Cao, Y.; Zong, H. Novel self-consistent mean field approximation and its application in strong interaction phase transitions. Chin. Phys. C 2019, 43, 084102. [Google Scholar] [CrossRef]
- Zhao, T.; Zheng, W.; Wang, F.; Li, C.M.; Yan, Y.; Huang, Y.F.; Zong, H.S. Do current astronomical observations exclude the existence of nonstrange quark stars? Phys. Rev. D 2019, 100, 043018. [Google Scholar] [CrossRef]
- Su, L.-Q.; Shi, C.; Xia, Y.-H.; Zong, H. Color superconductivity in a self-consistent NJL-type model. Phys. Rev. D 2020, 102, 054028. [Google Scholar] [CrossRef]
- Wu, Z.-Q.; Shi, C.; Ping, J.-L.; Zong, H.-S. Contributions of the vector-channel at finite isospin chemical potential with the self-consistent mean field approximation. Phys. Rev. D 2020, 101, 074008. [Google Scholar] [CrossRef]
- Wang, Q.; Zhao, T.; Zong, H. On the stability of two-flavor and three-flavor quark matter in quark stars within the framework of NJL model. Mod. Phys. Lett. A 2020, 2020, 2050321. [Google Scholar] [CrossRef]
- Klevansky, S.P. The Nambu—Jona–Lasinio model of quantum chromodynamics. Rev. Mod. Phys. 1992, 64, 649–708. [Google Scholar] [CrossRef]
- Adamczyk, L.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Alford, J.; Anson, C.D.; Aparin, A.; Arkhipkin, D.; et al. Beam energy dependence of moments of the net-charge multiplicity distributions in Au+Au collisions at RHIC. Phys. Rev. Lett. 2014, 113, 092301. [Google Scholar] [CrossRef] [PubMed]
- Aggarwal, M.M.; Ahammed, Z.; Alakhverdyants, A.V.; Alekseev, I.; Alford, J.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Balewski, J.; Barnby, L.S.; et al. Higher Moments of Net-proton Multiplicity Distributions at RHIC. Phys. Rev. Lett. 2010, 105, 022302. [Google Scholar] [CrossRef] [PubMed]
- Adamczyk, L.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Alford, J.; Anson, C.D.; Aparin, A.; Arkhipkin, D.; et al. Energy Dependence of Moments of Net-proton Multiplicity Distributions at RHIC. Phys. Rev. Lett. 2014, 112, 032302. [Google Scholar] [CrossRef] [PubMed]
- Luo, X. Exploring the QCD Phase Structure with Beam Energy Scan in Heavy-ion Collisions. Nucl. Phys. A 2016, 956, 75–82. [Google Scholar] [CrossRef]
- Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Ajitan, N.N.; Alekseev, I.; Anderson, D.M.; Aoyama, R.; et al. Collision Energy Dependence of Moments of Net-Kaon Multiplicity Distributions at RHIC. Phys. Lett. B 2018, 785, 551–560. [Google Scholar] [CrossRef]
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Anderson, D.M.; Aparin, A.; et al. Net-proton number fluctuations and the Quantum Chromodynamics critical point. arXiv 2020, arXiv:2001.02852. [Google Scholar]
- Gatto, R.; Ruggieri, M. Hot quark matter with an axial chemical potential. Phys. Rev. D 2012, 85, 054013. [Google Scholar] [CrossRef]
- Ruggieri, M. Critical end point of quantum chromodynamics detected by chirally imbalanced quark matter. Phys. Rev. D 2011, 84, 014011. [Google Scholar] [CrossRef]
- Cui, Z.-F.; Cloët, I.C.; Lu, Y.; Roberts, C.D.; Schmidt, S.M.; Xu, S.-S.; Zong, H.-S. Critical end point in the presence of a chiral chemical potential. Phys. Rev. D 2016, 94, 071503. [Google Scholar] [CrossRef]
- Pan, Z.; Cui, Z.-F.; Chang, C.-H.; Zong, H.-S. Finite-volume effects on phase transition in the Polyakov-loop extended Nambu–Jona–Lasinio model with a chiral chemical potential. Int. J. Mod. Phys. A 2017, 32, 1750067. [Google Scholar] [CrossRef]
- Liu, R.-L.; Lai, M.-Y.; Shi, C.; Zong, H.-S. Finite volume effects on QCD susceptibilities with a chiral chemical potential. Phys. Rev. D 2020, 102, 014014. [Google Scholar] [CrossRef]
- Chernodub, M.N.; Nedelin, A.S. Phase diagram of chirally imbalanced QCD matter. Phys. Rev. D 2011, 83, 105008. [Google Scholar] [CrossRef]
- Xu, S.-S.; Cui, Z.-F.; Wang, B.; Shi, Y.-M.; Yang, Y.-C.; Zong, H.-S. Chiral phase transition with a chiral chemical potential in the framework of Dyson-Schwinger equations. Phys. Rev. D 2015, 91, 056003. [Google Scholar] [CrossRef]
- Wang, B.; Wang, Y.-L.; Cui, Z.-F.; Zong, H.-S. Effect of the chiral chemical potential on the position of the critical endpoint. Phys. Rev. D 2015, 91, 034017. [Google Scholar] [CrossRef]
- Tian, Y.-L.; Cui, Z.-F.; Wang, B.; Shi, Y.-M.; Yang, Y.-C.; Zong, H.-S. Dyson–Schwinger Equations of Chiral Chemical Potential. Chin. Phys. Lett 2015, 32, 081101. [Google Scholar] [CrossRef]
- Witten, E. Cosmic separation of phases. Phys. Rev. D 1984, 30, 272–285. [Google Scholar] [CrossRef]
- Holdom, B.; Ren, J.; Zhang, C. Quark Matter May Not Be Strange. Phys. Rev. Lett. 2018, 120, 222001. [Google Scholar] [CrossRef]
- Wang, Q.; Shi, C.; Zong, H.-S. Nonstrange quark stars from an NJL model with proper-time regularization. Phys. Rev. D 2019, 100, 123003. [Google Scholar] [CrossRef]
- Zhang, C. Probing up-down quark matter via gravitational waves. Phys. Rev. D 2020, 101, 043003. [Google Scholar] [CrossRef]
- Zhang, C.; Mann, R.B. Unified Interacting Quark Matter and its Astrophysical Implications. arXiv 2020, arXiv:2009.07182. [Google Scholar]
- Cao, Z.; Chen, L.W.; Chu, P.C.; Zhou, Y. GW190814: Circumstantial Evidence for Up-Down Quark Star. arXiv 2020, arXiv:2009.00942. [Google Scholar]
- Li, C.-M.; Zuo, S.-Y.; Yan, Y.; Zhao, Y.-P.; Wang, F.; Huang, Y.-F.; Zong, H.-S. Strange quark stars within proper time regularized (2 + 1)-flavor NJL model. Phys. Rev. D 2020, 101, 063023. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [PubMed]
- Hinderer, T.; Lackey, B.D.; Lang, R.N.; Read, J.S. Tidal deformability of neutron stars with realistic equations of state and their gravitational wave signatures in binary inspiral. Phys. Rev. D 2010, 81, 123016. [Google Scholar] [CrossRef]
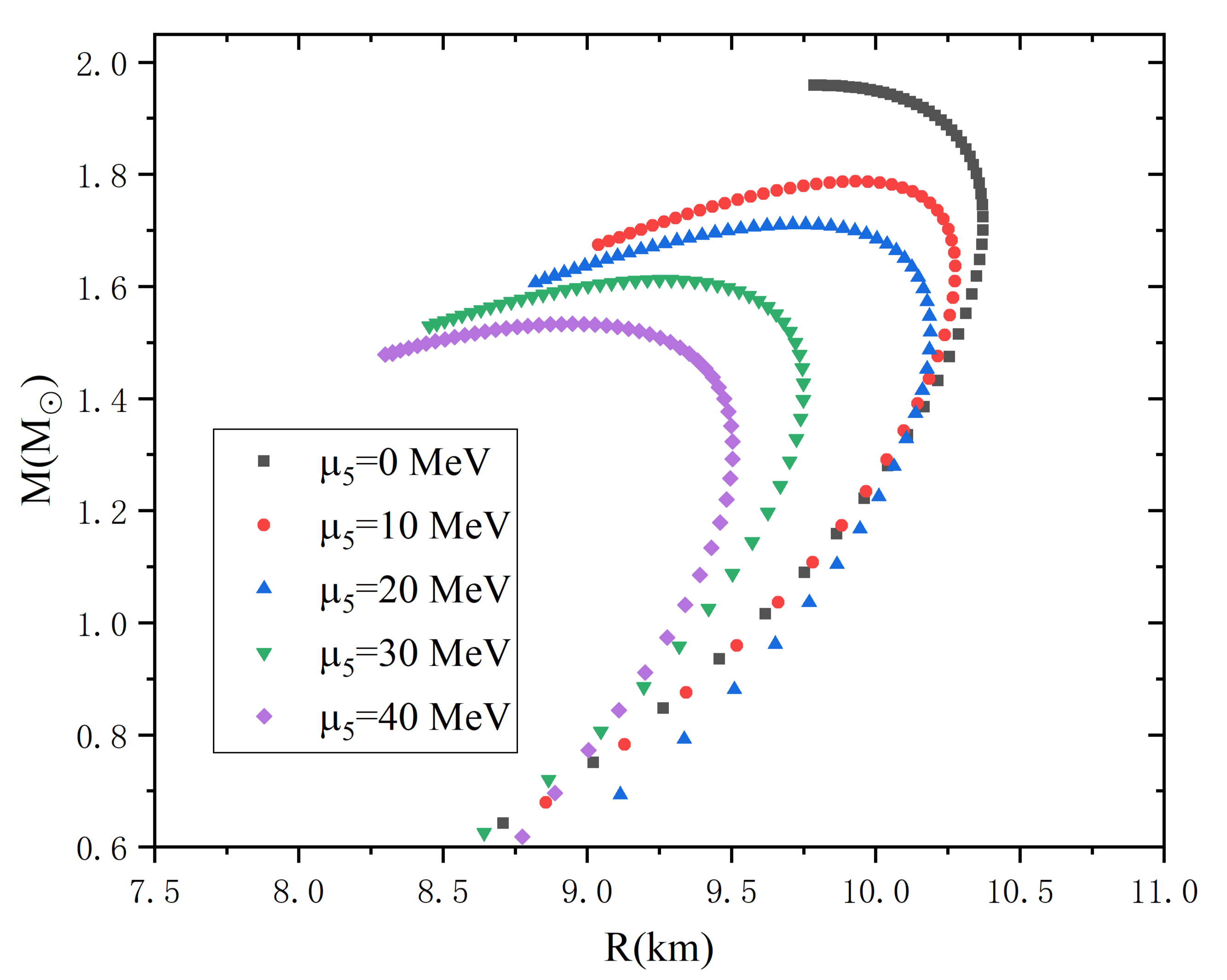
| (MeV) | ||
|---|---|---|
| 0 | 1.96 | 236 |
| 10 | 1.79 | 221 |
| 20 | 1.71 | 204 |
| 30 | 1.61 | 150 |
| 40 | 1.53 | 104 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, L.-K.; Luo, X.-F.; Segovia, J.; Zong, H.-S. A Brief Review of Chiral Chemical Potential and Its Physical Effects. Symmetry 2020, 12, 2095. https://doi.org/10.3390/sym12122095
Yang L-K, Luo X-F, Segovia J, Zong H-S. A Brief Review of Chiral Chemical Potential and Its Physical Effects. Symmetry. 2020; 12(12):2095. https://doi.org/10.3390/sym12122095
Chicago/Turabian StyleYang, Li-Kang, Xiao-Feng Luo, Jorge Segovia, and Hong-Shi Zong. 2020. "A Brief Review of Chiral Chemical Potential and Its Physical Effects" Symmetry 12, no. 12: 2095. https://doi.org/10.3390/sym12122095
APA StyleYang, L.-K., Luo, X.-F., Segovia, J., & Zong, H.-S. (2020). A Brief Review of Chiral Chemical Potential and Its Physical Effects. Symmetry, 12(12), 2095. https://doi.org/10.3390/sym12122095







