1. Introduction
In this paper, we are concerned with the finite, undirected, and simple graphs. Let and denote the sets of vertices and edges of , respectively. Many situations in various practically motivated problems and in mathematics and theoretical computer science can be captured by a graph. This simple structure has very widespread applications. It has several useful applications in operational research, such as, minimum cost path, and scheduling problems. It is also used in sociology. For example, to explore rumor spreading using social network analysis software.
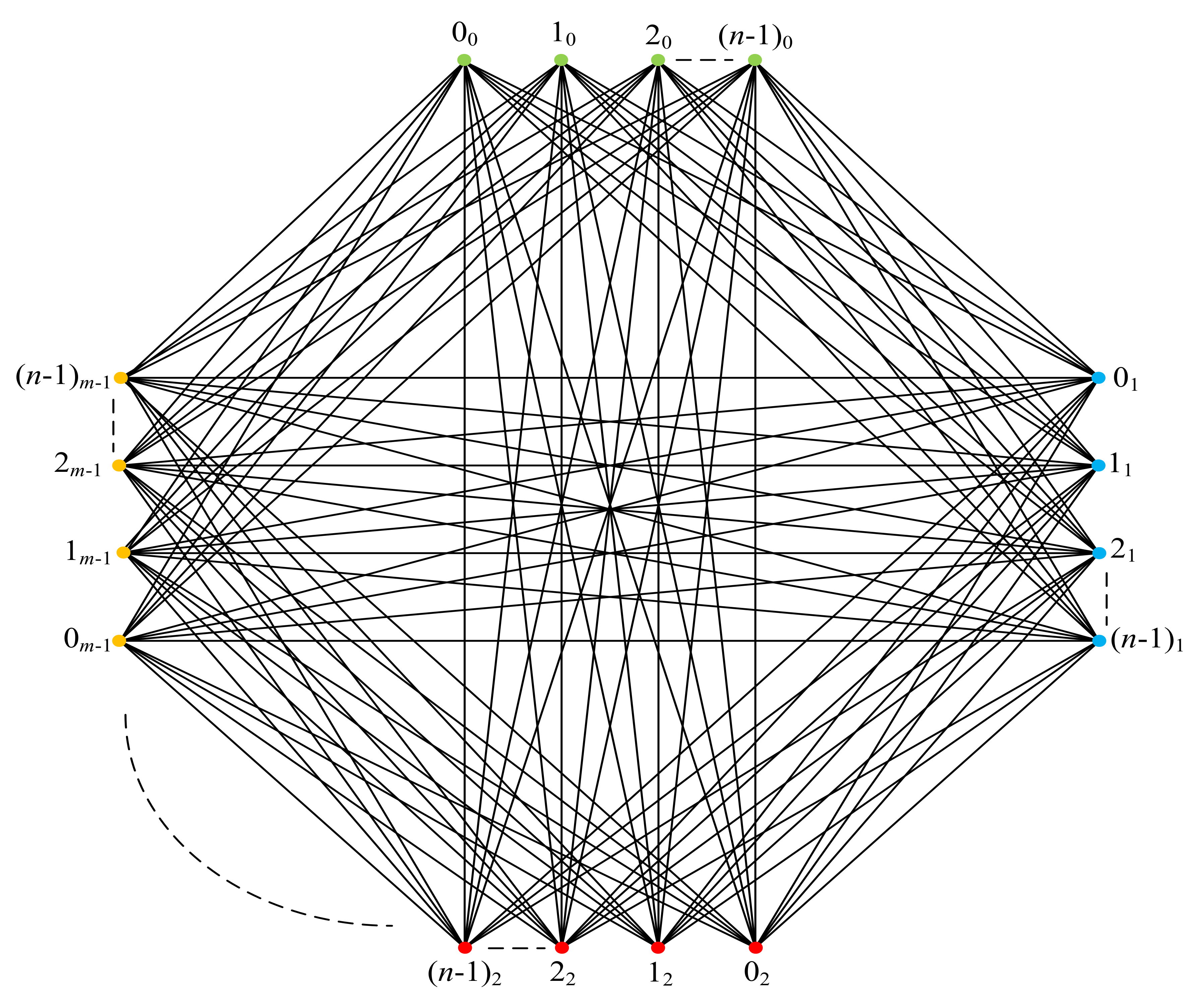
An orthogonal double covers (ODC) of is a collection of spanning isomorphic subgraphs (called pages) of such that:
- (i)
double cover property: Every edge of is in exactly one page of and in exactly one page of
- (ii)
orthogonality property: For and and for all
Some problems in statistical design theory [
1] and the theory of Armstrong databases [
2] are the motivation of studying the ODCs. ODCs have been investigated for more than 40 years. There is an extensive literature on the ODCs. The authors in [
2,
3] introduced the motivation and an overview of results and problems concerned with the ODCs. In [
4,
5], the authors have generalized the notion of an ODC to orthogonal decompositions of complete digraphs. Also, the ODC has been generalized to the suborthogonal double covers [
5,
6], and symmetric graph designs [
7,
8,
9,
10].
A technique to construct ODCs for Cayley graphs has been introduced by Scapellato et al. [
11]. It has been shown that for all
where
is a
-regular Cayley graph on an abelian group there is an ODC, a few well known exceptions apart. Sampathkumar et al. [
12] have constructed the cyclic ODCs of
-regular circulant graphs. El-Shanawany and El-Mesady [
13] have introduced a technique to construct the CODCs of circulant graphs by several graph classes such as tripartite graphs, complete bipartite graphs, and disjoint union of
and butterfly. In [
14], a technique for orthogonal labeling is produced for the corona product of two finite or infinite graph classes such as path, cycle, and star graphs. In addition, the nonexistence of the orthogonal
-labeling is proved for the corona product of
and an infinite cycle.
For many years, the researchers have interested in the decompositions of graphs into Hamilton paths, or into Hamilton cycles. Bryant et al., [
15] proved that a complete multipartite graph
with
vertices and
edges can be decomposed into edge-disjoint Hamilton paths if and only if
is an integer and the maximum degree of
is at most
. In [
16], surveys results on cycle decompositions of complete multipartite graphs were introduced. The authors in [
17] reduced the problem of finding an edge-decomposition of a balanced
-partite graph of large minimum degree into
-cliques to the problem of finding a fractional
-clique decomposition or an approximate one. All the previous results motivate us to the results of this paper. In this paper, we generalize the ODC of
to the circular intensely orthogonal double cover design (CIODCD) of
. Since, the ODCs are very important in solving many problems in the statistical design and Armstrong databases, then the generalization of the ODCs to the CIODCD has a very important role in the statistical design theory and the relational databases. Now, the ODC can be considered as a special case of our generalization. Then the CIODCD can be utilized to model more general relational databases.
The paper is organized as follows.
Section 2 introduces the basic definitions and terminologies that will be used throughout.
Section 3 and
Section 4 deal with the half and symmetric symmetric starters matrices, respectively.
3. CIODCDs by Half Starters Matrices
In this section, we will use two half starters matrices to construct CIODCDs of by two given graphs These two graphs allow us to introduce later two matrices represent them. That is, we often consider these two matrices instead of and respectively.
Definition 2. Letbe a spanning subgraph ofandThen the graph with
is called the -translate of Note that sums and differences are calculated in (i.e., sums and differences are calculated modulo ).
Definition 3. Letbe a spanning subgraph ofThe length of an edgeis defined byfor allNote thathasclasses of edge lengths; one different class for each part of.
Definition 4. A spanning subgraphofis called a half starter graph with respect toif
- (i)
- (ii)
The lengths of all edges inare mutually different,
As an immediate consequence of the Definition 2 and the Definition 3, the following result can be introduced.
Lemma 1. Ifis a half starter, then the union of all translates offorms an edge decomposition of Proof. Using contradiction method, let
Assume
is an edge with length
belongs to the intersection graph
Then
are two distinct edges in
, both of them have length
. This leads to contradiction because
is half starter. □
In what follows, we will represent a half starter
by
matrix
whose rows are arranged as follows,
The half starter
can be decomposed into
bipartite graphs, each one can be generated by a row in the matrix
where the bipartite graph between the two sets
and
is generated by the row
the edge set of the bipartite graph between the two sets
and
is
Note that the lengths of the edges of the bipartite graph between the two sets and are indexed by
The CIODCD of
in
Figure 2 can be represented by the following matrices.
Definition 5. Two half starter matricesandare said to be intensely orthogonal if the elements of any row in the difference matrixare all distinct and equal to.
Theorem 1. If the two half starter matricesandare intensely orthogonal, thenwithis a CIODCDs of
Proof. Take into account the relation between the ith row of the half starter matrix and the order that denotes the bipartite subgraph of between part and part Firstly, from Lemma 1, all edges appear exactly in two subgraphs and thus the double cover property is done for all edges of Now, let , and Since the ith row in represents the bipartite subgraph of between part and part Then the intensely orthogonality property will be satisfied if we prove that if and , and if
First case is satisfied directly from the definition of the construction. But if
let
and
then for the first row of
and
we can find exactly one element
where
Thus
but this means that there is exactly one edge
where
Also
this is verified for the other rows of
and
, then the intensely orthogonality property is satisfied and
for more illustration, see (1), (2). □
4. CIODCDs by Symmetric Starters Matrices
In this section, we will study symmetric starter matrix of CIODCDs of by a given graph . That is, we shall reduce two half starters matrices to one half starter matrix under certain conditions to construct a symmetric starter matrix.
Definition 6. Letbe a spanning subgraph ofthe subgraphofwith is called symmetric graph of
Remark 1. Ifis a half starter, then is also a half starter.
Definition 7. A half starteris called a symmetric starter with respect toifandare intensely orthogonal.
Theorem 2. Letbe a positive integer and letbe a half starter ofrepresented by the matrixThenis a symmetric starter if and only if, for each rowof Proof. We know that is a half starter and represented by Since is an edge in we have is an edge in of length Therefore, and thus Consequently, and are intensely orthogonal if and only if Hence, the double cover and the intensely orthogonality properties are verified, and the CIODCD of is constructed. □
For all the following results, the value of
Theorem 3. Letbe integers. Then the matrixis a symmetric starter matrix of a CIODCD ofby Proof. Since
then for each row of
we have
and hence,
is a symmetric starter matrix. The edge set of
is
where
□
Theorem 4. Letbe an integer andorThen the matrixis a symmetric starter matrix of a CIODCD ofby
Proof. Since
then for each row of
, we have
where
and hence,
is a symmetric starter matrix. The edge set of
is
where
□
Theorem 5. Letbe an integer andbe a positive integer. Then there is a CIODCD ofby Proof. Let the matrix
then for each row of
we have
Hence, for
we have
but these elements are mutually different and equal to
This leads to that
is a symmetric starter matrix. The edge set of
is
□
For more illustration, let
and
then there is a CIODCD of
by
See
Figure 3, where
Theorem 6. Letbe a prime number. Then there is a CIODCD ofby Proof. Let the matrix
then for each row of
we have
and hence,
is a symmetric starter matrix. The edge set of
is
□
For more illustration, let
and
, then there is a CIODCD of
by
See
Figure 4, where