Extremal Trees with Respect to the Difference between Atom-Bond Connectivity Index and Randić Index
Abstract
1. Introduction
2. Preliminary Results
- (1)
- Suppose that .
- (1.1)
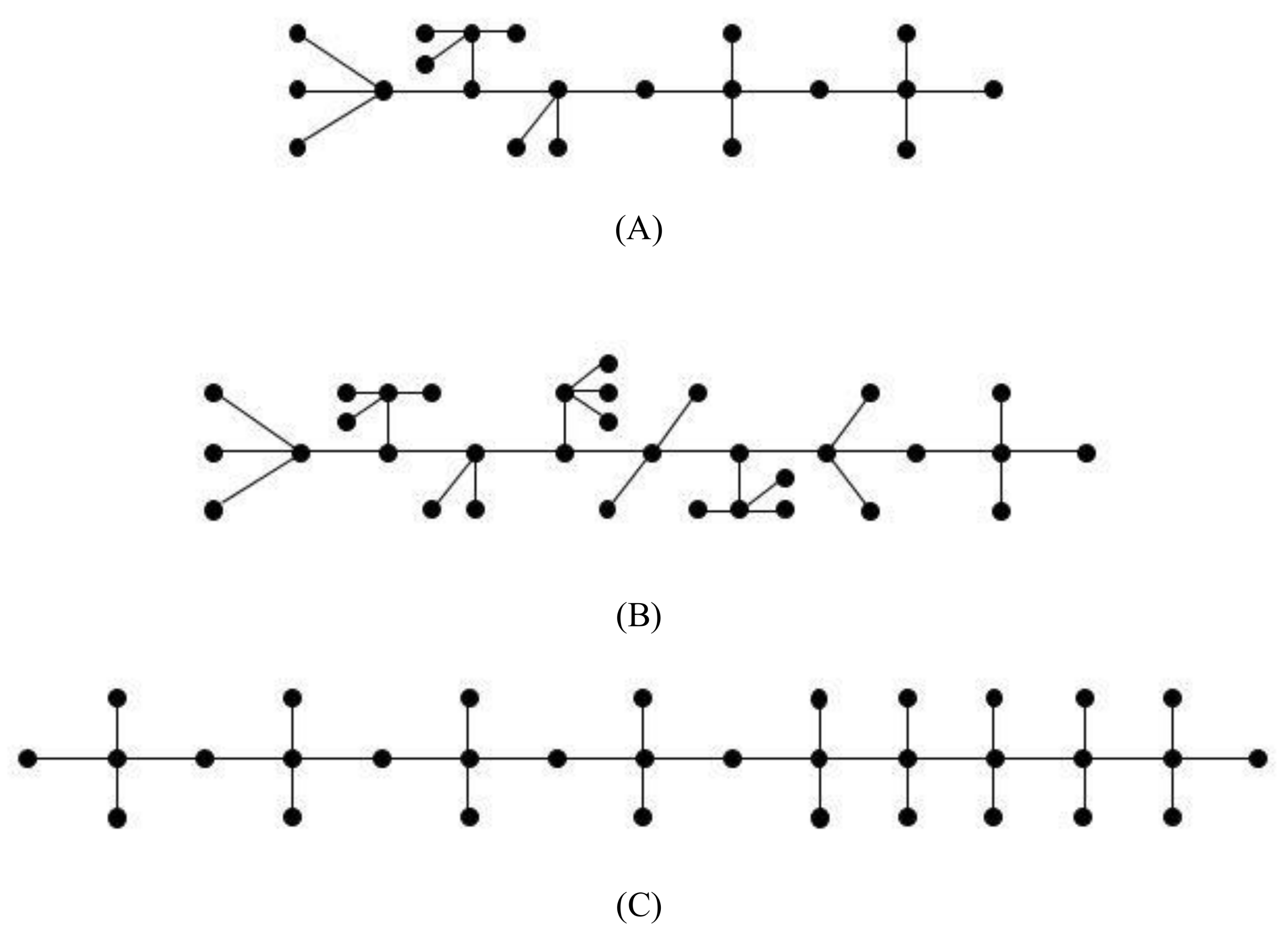
- For , the maximum value iswhich is uniquely attained by those trees that contain a unique vertex of degree 2 and no vertex of degree 3, that is, and , such that the unique vertex of degree 2 is adjacent to two vertices of degree 4, that is, and .
- (1.2)
- For , the second maximum value iswhich is uniquely attained by those trees that contain no vertex of degree 2 and exactly two vertices of degree 3, that is, and , such that each vertex of degree 3 is adjacent to three vertices of degree 4, that is, and .
- (1.3)
- For , the third maximum value iswhich is uniquely attained by those trees that contain no vertex of degree 2 and exactly two vertices of degree 3, which are adjacent, that is, , , and such that each vertex of degree 3 is adjacent to exactly two vertices of degree 4, that is, and .
- (2)
- Suppose that .
- (2.1)
- For , the maximum value isand the equality holds if and only if and such that and .
- (2.2)
- For , the second maximum value iswhich is uniquely attained by those trees that contain exactly two vertices of degree 2 and no vertex of degree 3, that is, and , such that either vertex of degree 2 is adjacent to two vertices of degree 4, that is, and .
- (2.3)
- For , the third maximum value iswhich is uniquely attained by those trees that contain a unique vertex of degree 2 and exactly two vertices of degree 3, that is, and , such that each vertex of degree 2 and 3 is adjacent to only vertices of degree 4, that is, , , and .
- (3)
- Suppose that .
- (3.1)
- For , the maximum value iswhich is uniquely attained by those trees that contain no vertex of degree 2 or 3, that is, .
- (3.2)
- For , the second maximum value iswhich is uniquely attained by those trees that contain a unique vertex of degree 2 and a unique vertex of degree 3, that is, , such that each vertex of degree 2 and 3 is adjacent to only vertices of degree 4, that is, , , and .
- (3.3)
- For , the third maximum value iswhich is uniquely attained by those trees that contain no vertex of degree 2 and exactly three vertices of degree 3, that is, and , such that each vertex of degree 3 is adjacent to three vertices of degree 4, that is, , and .
3. Maximum Index for Chemical Trees
- (1)
- Suppose that .
- (1.1)
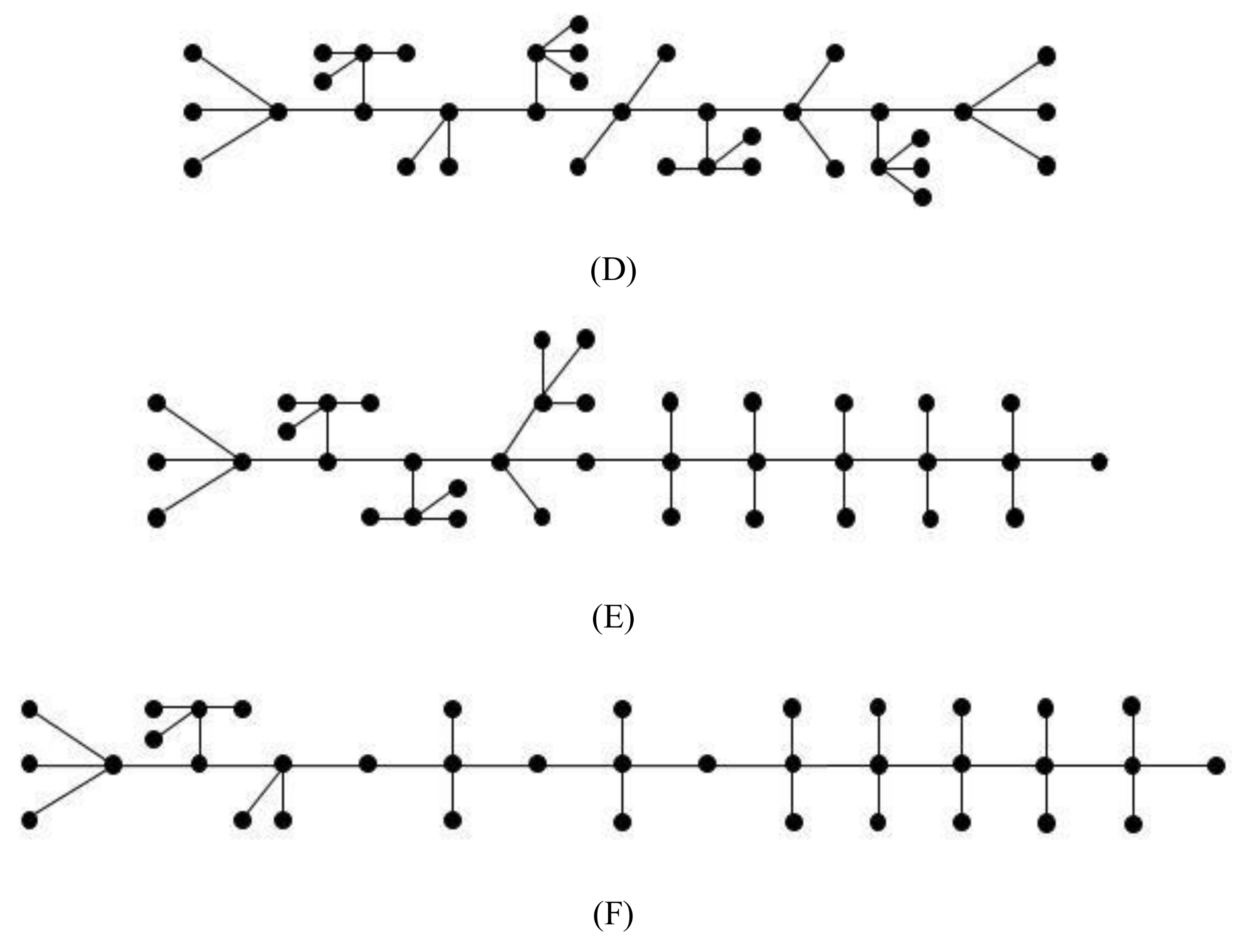
- For , the fourth maximum value isand the equality holds if and only if and such that , and .
- (1.2)
- For , the fifth maximum value isand the equality holds if and only if and such that , and .
- (1.3)
- For , the sixth maximum value isand the equality holds if and only if , such that and .
- (2)
- Suppose that .
- (2.1)
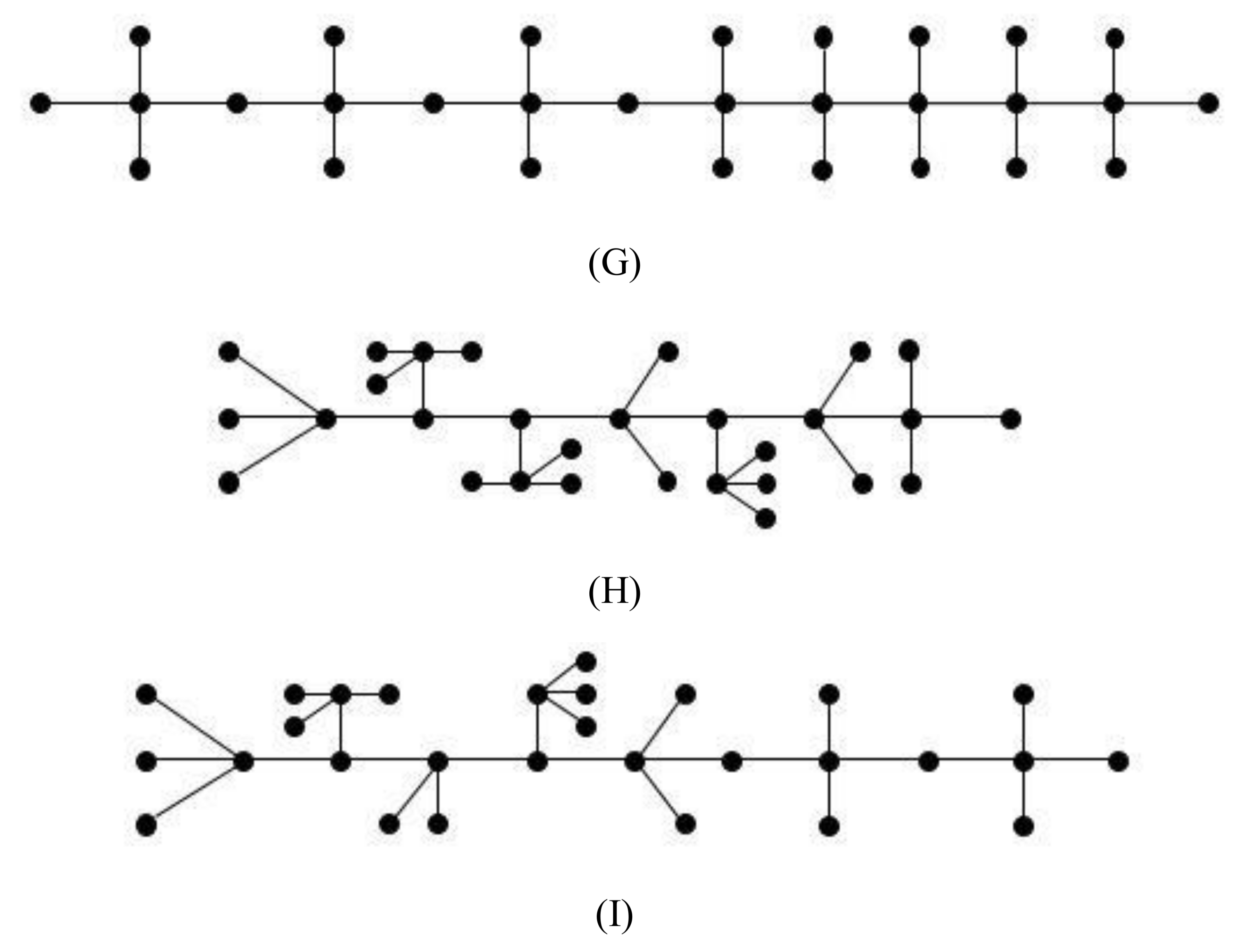
- For , the fourth maximum value isand the equality holds if and only if and such that and .
- (2.2)
- For , the fifth maximum value isand the equality holds if and only if , such that , , and .
- (2.3)
- For , the sixth maximum value isand the equality holds if and only if and such that , , and .
- (3)
- Suppose that .
- (3.1)
- For , the fourth maximum value isand the equality holds if and only if and such that and .
- (3.2)
- For , the fifth maximum value isand the equality holds if and only if and such that , and .
- (3.3)
- For , the sixth maximum value isand the equality holds if and only if and such that , and .
- when ,
- when ,
- when ,
- when ,
- (i)
- If , then the fourth, fifth and sixth minimum values are 0.0580155, 0.0736795 and 0.0802936, respectively.
- (ii)
- If , then the fourth, fifth and sixth minimum values are 0.0714748, 0.0737492 and 0.0780889, respectively.
- (iii)
- If , then the fourth, fifth and sixth minimum values are 0.0602202, 0.0715445 and 0.07588419, respectively.
4. Upper Bound for Index of Molecular Trees
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- West, D.B. Introduction to Graph Theory, 2nd ed.; Prentice Hall, Inc.: Upper Saddle River, NJ, USA, 2001. [Google Scholar]
- Devillers, J.; Balaban, A.T. (Eds.) Topological Indices and Related Descriptors in QSAR and QSPR; Gordon and Breach: Amsterdam, The Netherlands, 1999. [Google Scholar]
- Todeschini, R.; Consonni, V. Handbook of Molecular Descriptors; Wiley-VCH: Weinheim, Germany, 2000. [Google Scholar]
- Randić, M. On characterization of molecular branching. J. Am. Chem. Soc. 1975, 97, 6609–6615. [Google Scholar] [CrossRef]
- Gutman, I.; Furtula, B. Recent results in the theory of Randić index. In Mathematical Chemistry Monograph. 6; University of Kragujevac: Kragujevac, Serbia, 2008. [Google Scholar]
- Li, X.; Gutman, I. Mathematical aspects of Randić-type molecular structure descriptors. In Mathematical Chemistry Monograph. 1; University of Kragujevac: Kragujevac, Serbia, 2006. [Google Scholar]
- Li, X.; Shi, Y. A survey on the Randić index. MATCH Commun. Math. Comput. Chem. 2008, 59, 127–156. [Google Scholar]
- Randić, M. On the history of the Randić index and emerging hostility towards chemical graph theory. MATCH Commun. Math. Comput. Chem. 2008, 59, 5–124. [Google Scholar]
- Husin, N.M.; Hasni, R.; Du, Z.; Ali, A. More results on extremum Randić indices of (molecular) trees. Filomat 2018, 32, 3581–3590. [Google Scholar] [CrossRef]
- Li, J.; Balachandran, S.; Ayyaswamy, S.K.; Venkatakrishnan, Y.B. The Randić indices of trees, unicyclic graphs and bicyclic graphs. Ars Combin. 2016, 127, 409–419. [Google Scholar]
- Estrada, E.; Torres, L.; Rodríguez, L.; Gutman, I. An atom-bond connectivity index: Modelling the enthalpy of formation of alkanes. Indian J. Chem. Sect. A 1998, 37, 849–855. [Google Scholar]
- Estrada, E. Atom-bond connectivity and energetic of branched alkanes. Chem. Phys. Lett. 2008, 463, 422–425. [Google Scholar] [CrossRef]
- Gutman, I.; Furtula, B.; Ahmadi, M.B.; Hosseini, S.A.; Nowbandegani, P.S.; Zarrinderakht, M. The ABC index conundrum. Filomat 2013, 27, 1075–1083. [Google Scholar] [CrossRef][Green Version]
- Cui, Q.; Qian, Q.; Zhong, L. The maximum atom-bond connectivity index for graphs with edge-connectivity one. Discrete Appl. Math. 2017, 220, 170–173. [Google Scholar] [CrossRef]
- Dimitrov, D. On the structural properties of trees with minimal atom-bond connectivity index III: Bounds on B1- and B2-branches. Discrete Appl. Math. 2016, 204, 90–116. [Google Scholar] [CrossRef]
- Gao, Y.; Shao, Y. The smallest ABC index of trees with n pendant vertices. MATCH Commun. Math. Comput. Chem. 2016, 76, 141–158. [Google Scholar]
- Shao, Z.; Wu, P.; Gao, Y.; Gutman, I.; Zhang, Z. On the maximum ABC index of graphs without pendent vertices. Discrete Appl. Math. 2017, 315, 298–312. [Google Scholar] [CrossRef]
- Xing, R.; Zhou, B.; Dong, F. On the atom-bond connectivity index of connected graphs. Discrete Appl. Math. 2011, 159, 1617–1630. [Google Scholar] [CrossRef]
- Xing, R.; Zhou, B.; Du, Z. Further results on atom-bond connectivity index of trees. Discrete Appl. Math. 2010, 158, 1536–1545. [Google Scholar] [CrossRef]
- Das, K.C.; Das, S.; Zhou, B. Sum-connectivity index. Front. Math. China 2016, 11, 47–54. [Google Scholar] [CrossRef]
- Das, K.C.; Trinajstić, N. Comparison between the first geometric-arithmetic index and atom-bond connectivity index. Chem. Phys. Lett. 2010, 497, 149–151. [Google Scholar] [CrossRef]
- Raza, Z.; Bhatti, A.A.; Ali, A. More comparison between the first geometric-arithmetic index and atom-bond connectivity index. Miskolc Math. Notes 2016, 17, 561–570. [Google Scholar] [CrossRef]
- Zhong, L.; Cui, Q. On a relation between the atom-bond connectivity and the first geometric-arithmetic indices. Discrete Appl. Maths 2015, 185, 249–253. [Google Scholar] [CrossRef]
- Ali, A.; Du, Z. On the difference between atom-bond connectivity index and Randić index of binary and chemical trees. Int. J. Quantum Chem. 2017, 117, e25446. [Google Scholar] [CrossRef]
- Riaz, A.; Ellahi, R.; Bhatti, M.M.; Marin, M. Study of heat and mass transfer in the Erying-Powell model of fluid propagating peristaltically through a rectangular compliant channel. Heat Transfer Res. 2019, 50, 1539–1560. [Google Scholar] [CrossRef]
- Gutman, I.; Miljkovic, O.; Caporossi, G.; Hansen, P. Alkanes with small and large Randić connectivity indices. Chem. Phys. Lett. 1999, 306, 366–372. [Google Scholar] [CrossRef]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zuki, W.N.N.N.W.; Du, Z.; Kamran Jamil, M.; Hasni, R. Extremal Trees with Respect to the Difference between Atom-Bond Connectivity Index and Randić Index. Symmetry 2020, 12, 1591. https://doi.org/10.3390/sym12101591
Zuki WNNNW, Du Z, Kamran Jamil M, Hasni R. Extremal Trees with Respect to the Difference between Atom-Bond Connectivity Index and Randić Index. Symmetry. 2020; 12(10):1591. https://doi.org/10.3390/sym12101591
Chicago/Turabian StyleZuki, Wan Nor Nabila Nadia Wan, Zhibin Du, Muhammad Kamran Jamil, and Roslan Hasni. 2020. "Extremal Trees with Respect to the Difference between Atom-Bond Connectivity Index and Randić Index" Symmetry 12, no. 10: 1591. https://doi.org/10.3390/sym12101591
APA StyleZuki, W. N. N. N. W., Du, Z., Kamran Jamil, M., & Hasni, R. (2020). Extremal Trees with Respect to the Difference between Atom-Bond Connectivity Index and Randić Index. Symmetry, 12(10), 1591. https://doi.org/10.3390/sym12101591




