Calculation of Limit Support Pressure for EPB Shield Tunnel Face in Water-Rich Sand
Abstract
1. Introduction
2. Numerical Simulation of the Distribution Rules of the Seepage Field Near the Tunnel Face
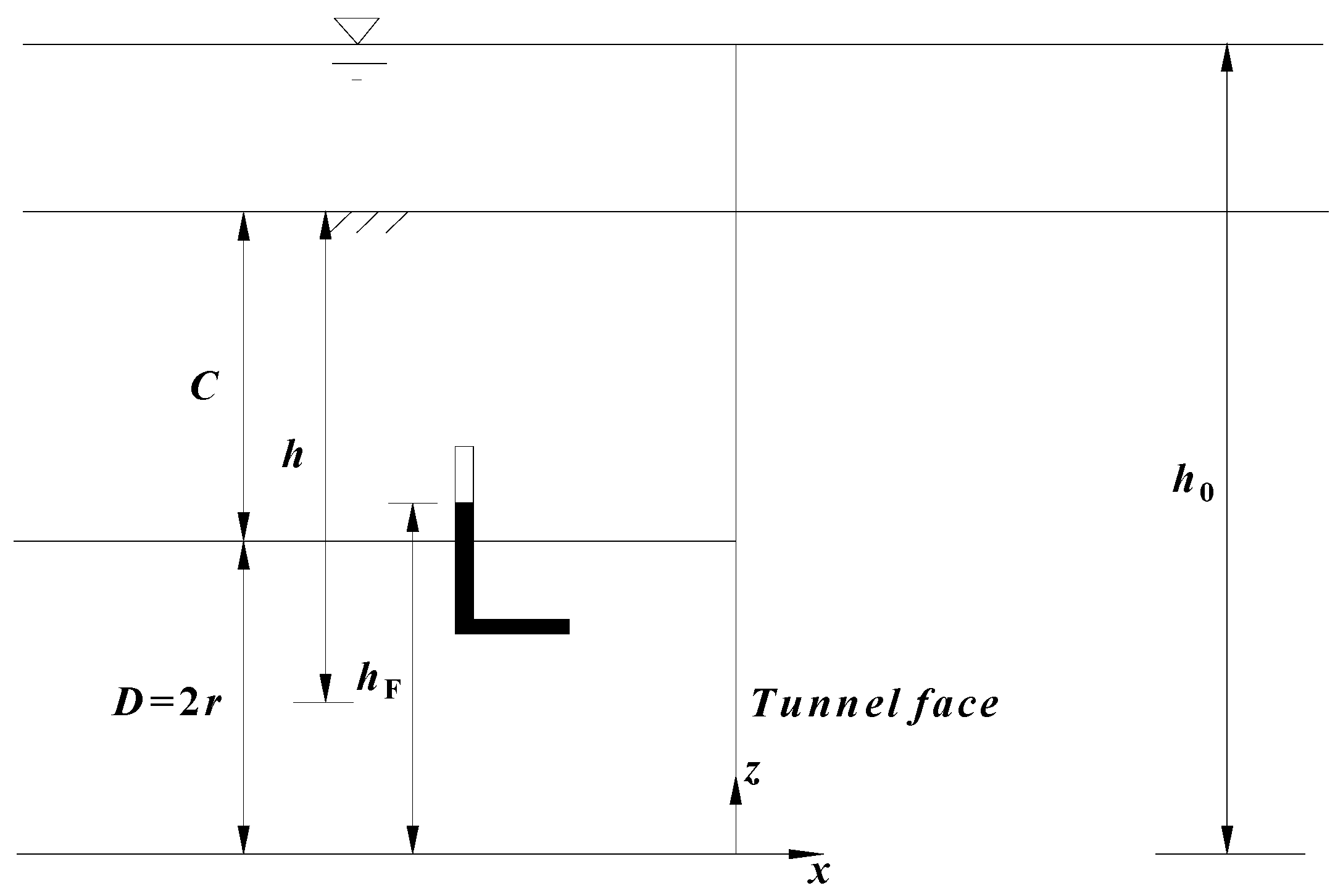
2.1. Overview of Calculation of a Hydraulic Gradient around the Tunnel Face
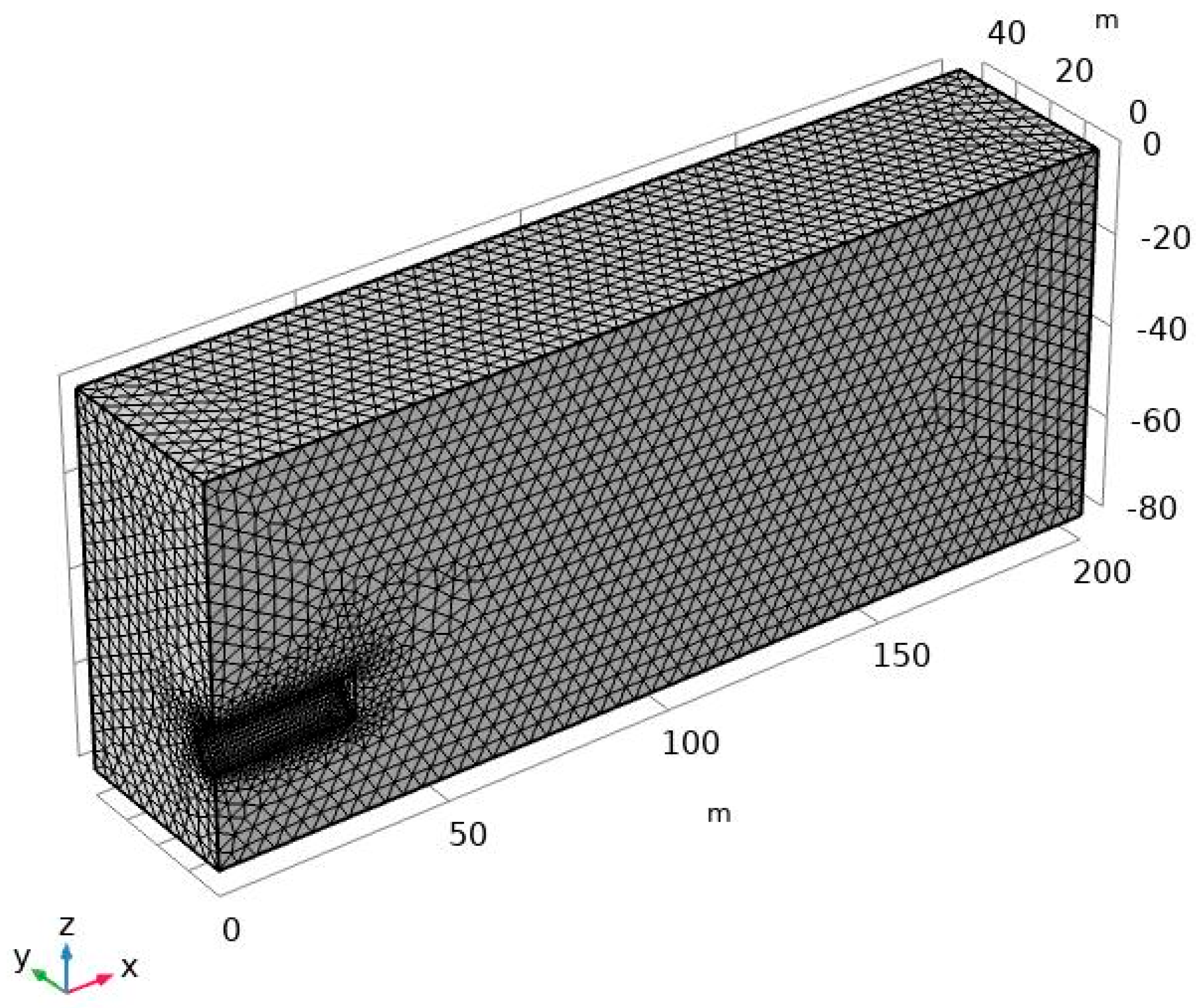
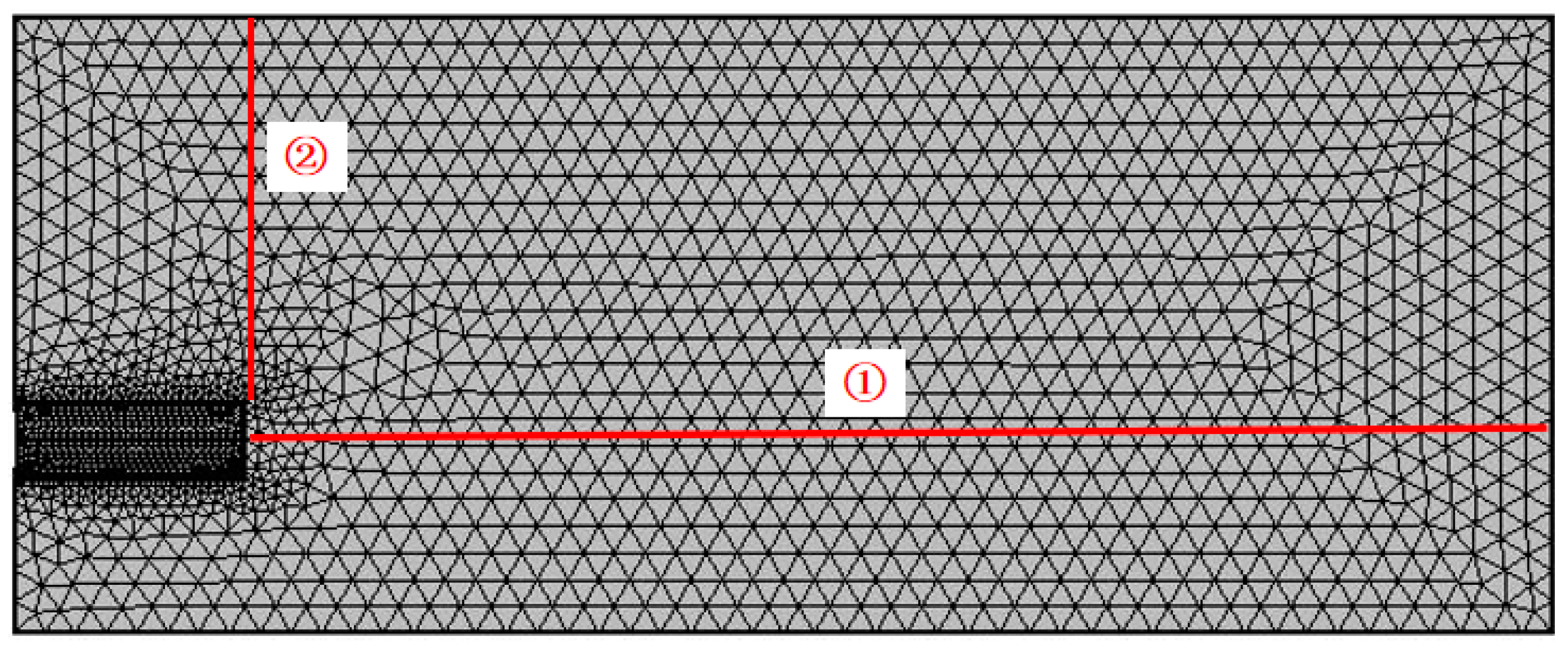
2.2. Finite Element Modeling
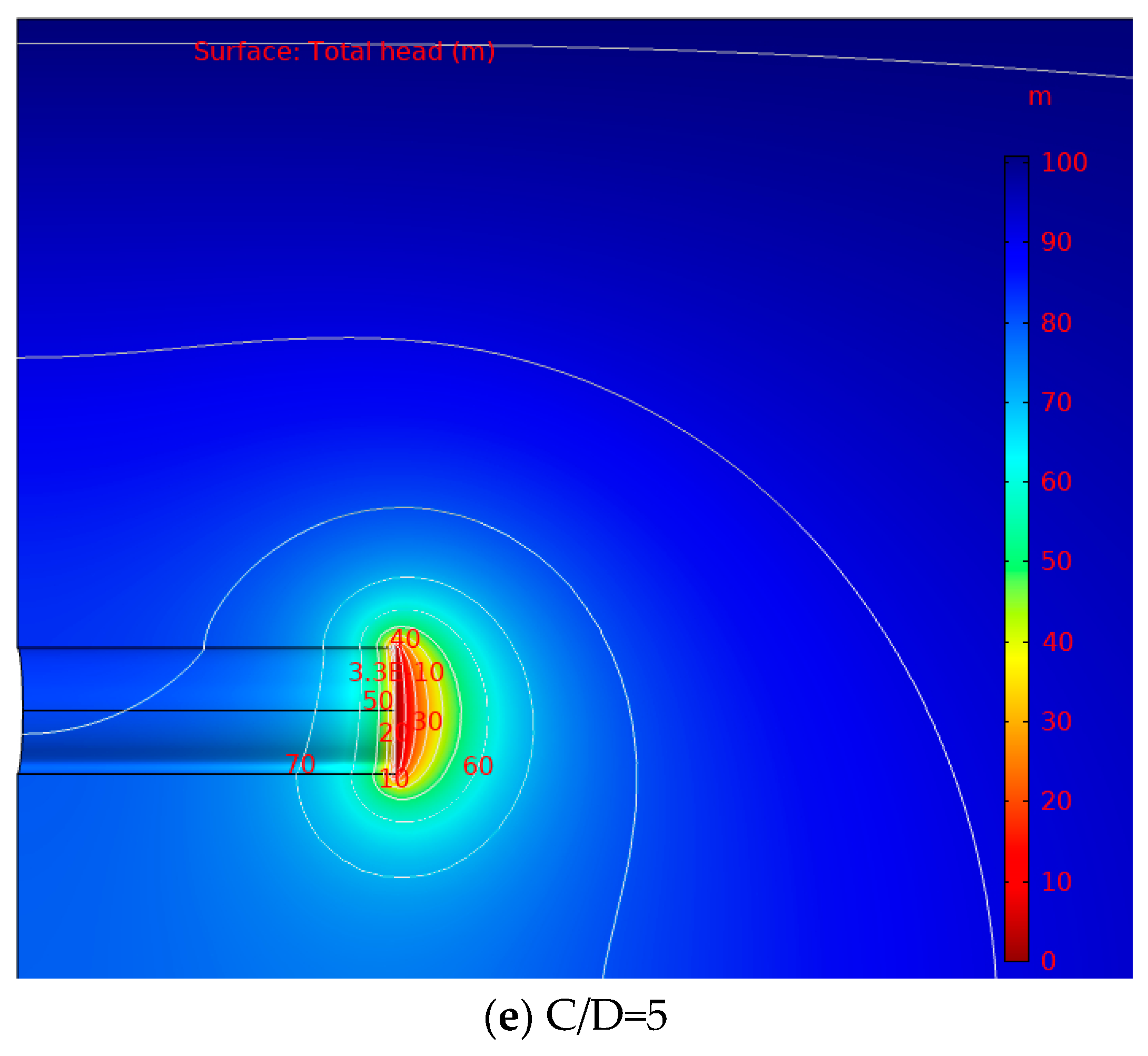
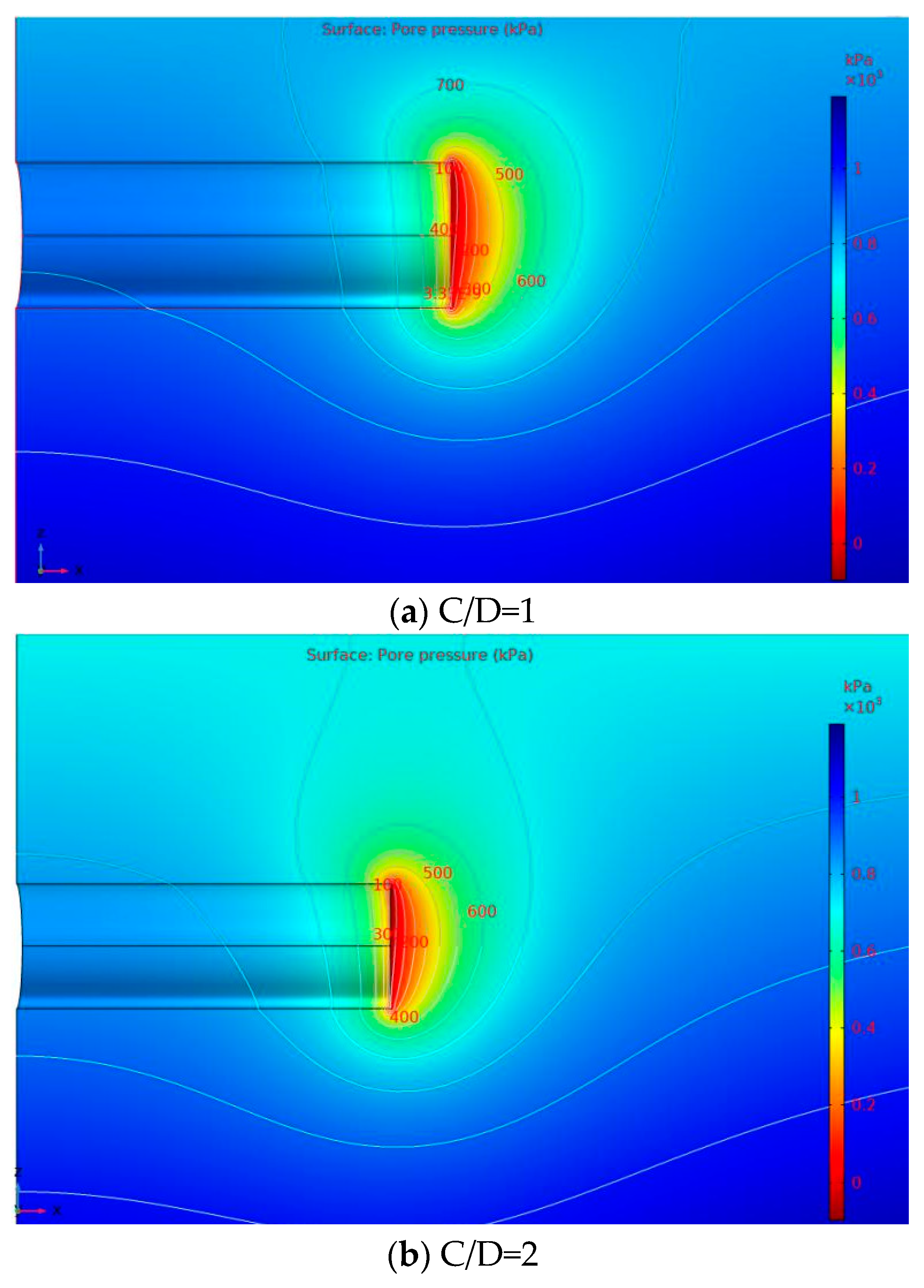
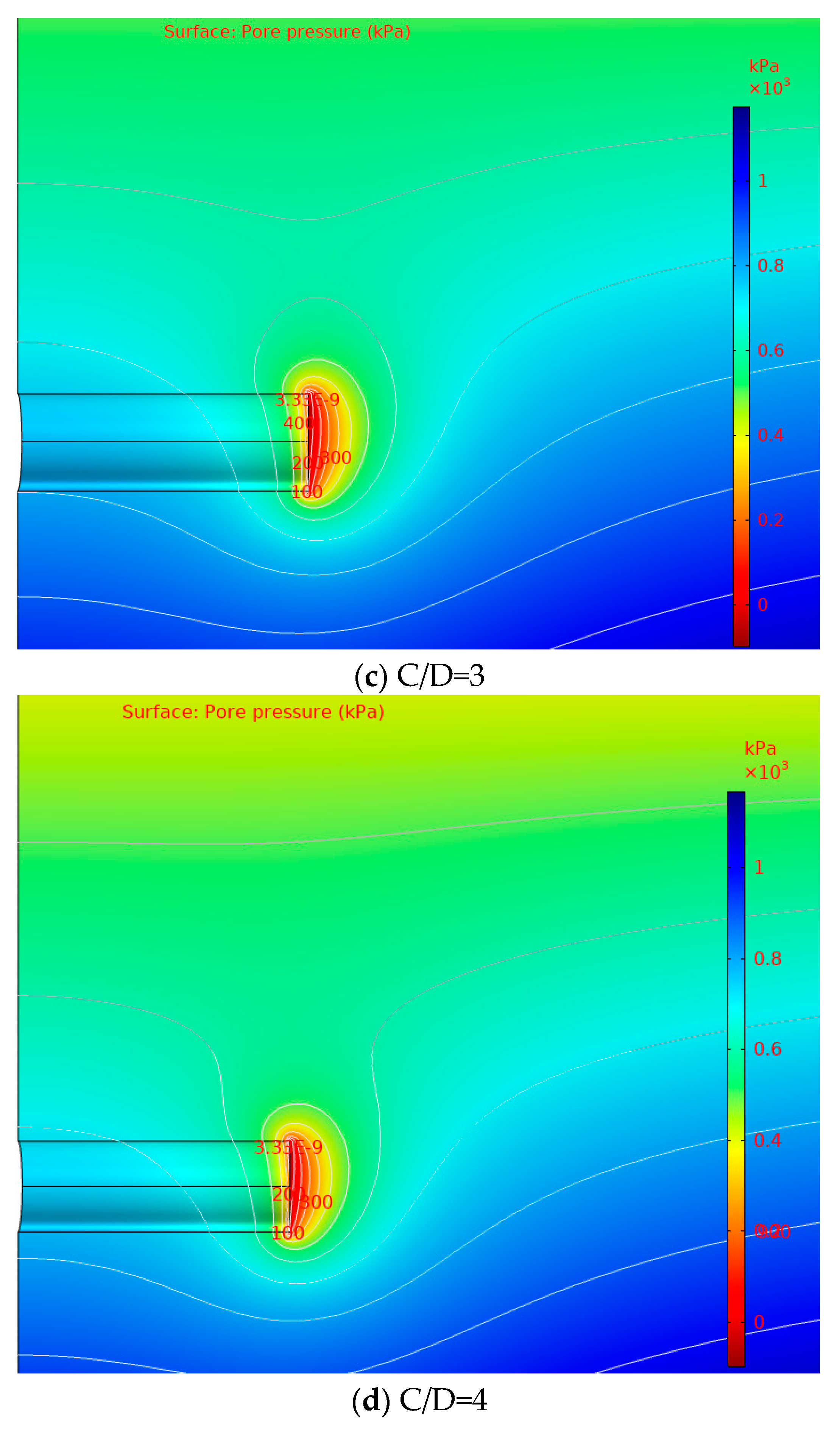
2.3. Seepage Field Analysis
2.4. New Approximate Analytical Solutions of Total Hydraulic Head Ahead of the Tunnel Face for the Circular Tunnel
3. Limit Equilibrium Model of the Tunnel Face Stability Considering the Effect of Seepage Forces
3.1. Outline
3.2. Mechanical Analysis of the Prism
3.3. Mechanical Analysis of the Wedge
3.4. Calculation of Limit Support Pressure
4. Comparison of the Limit Support Pressures with the Existing Approaches
5. Sensitivity Analysis of Model Parameters on the Limit Support Pressures
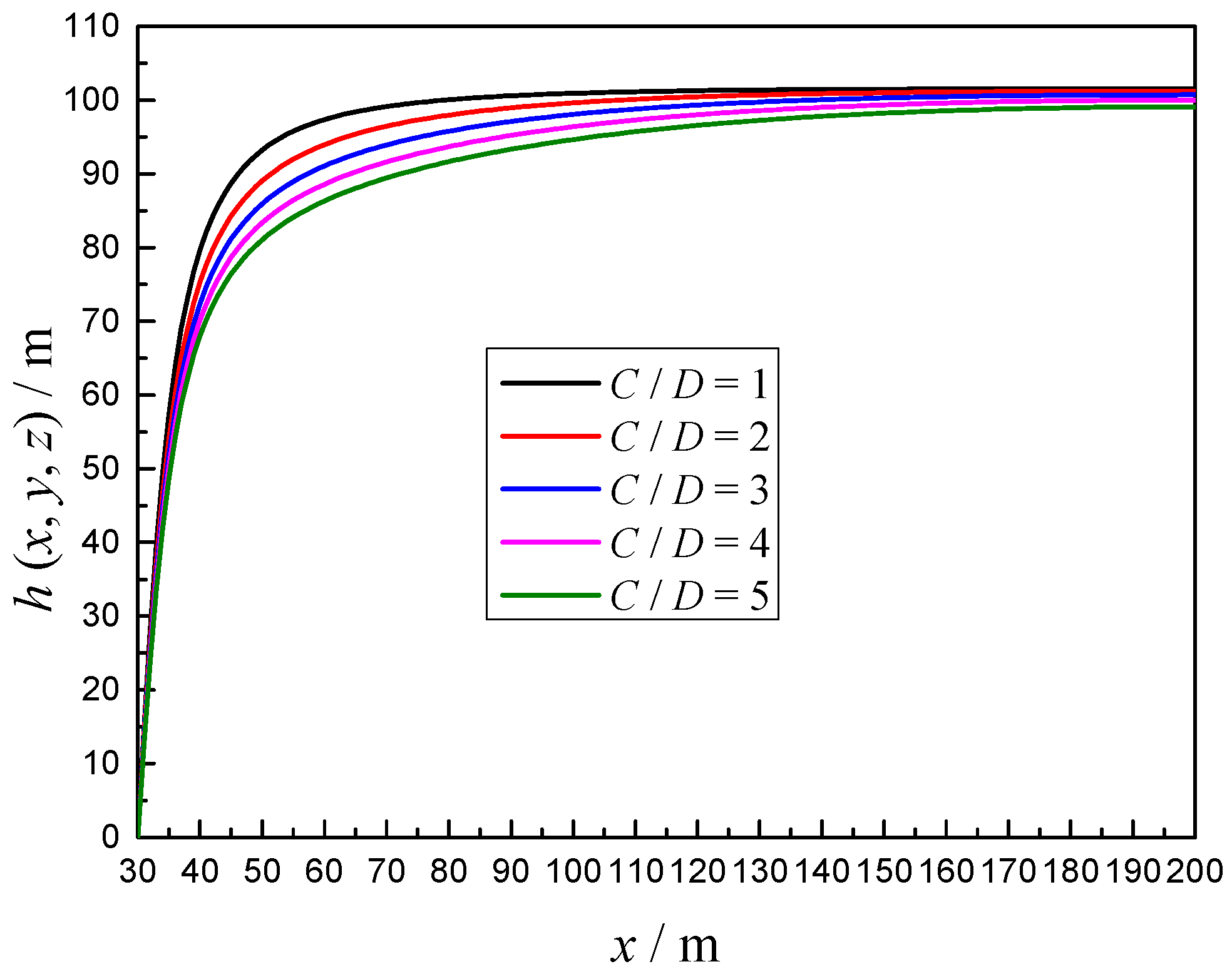
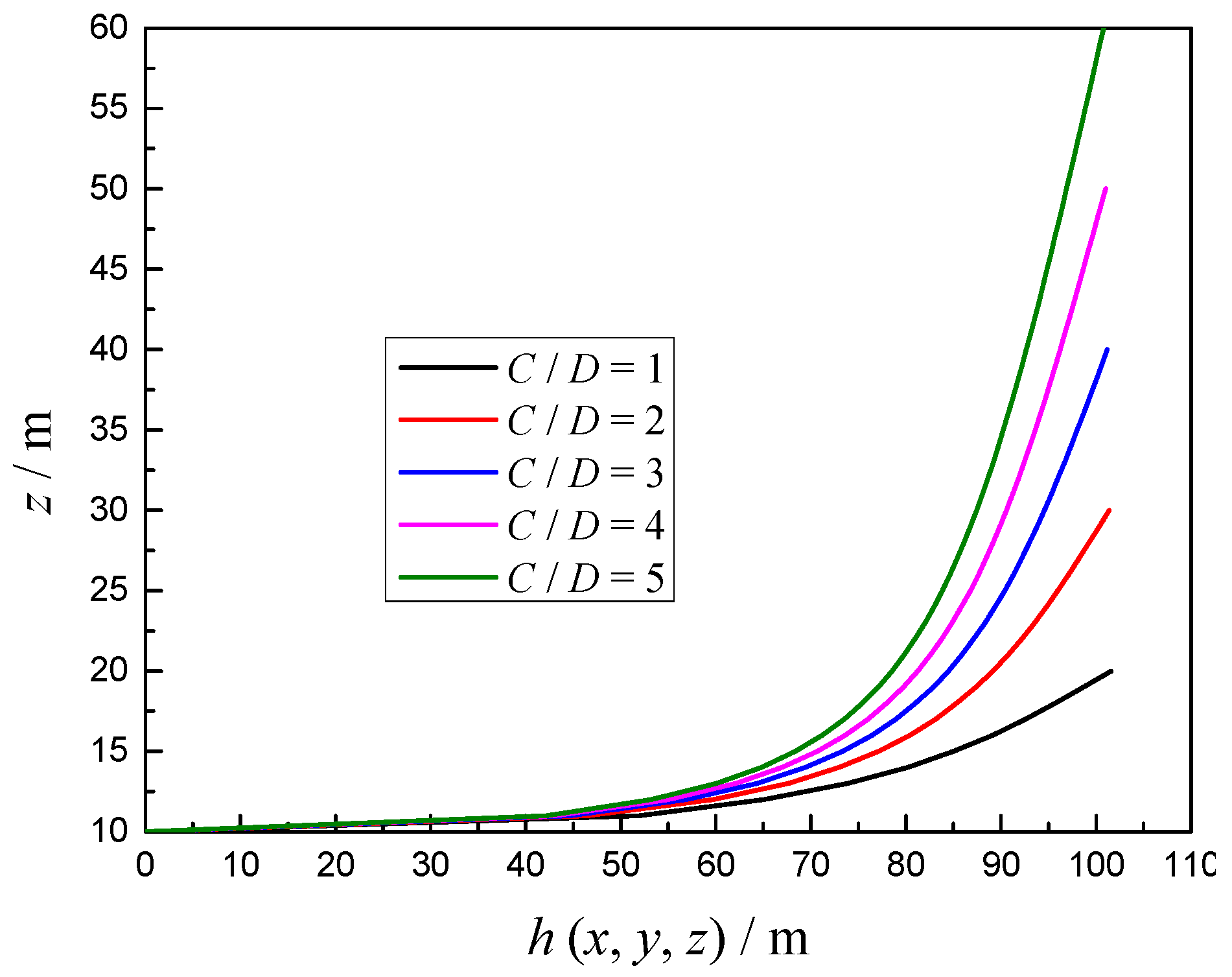
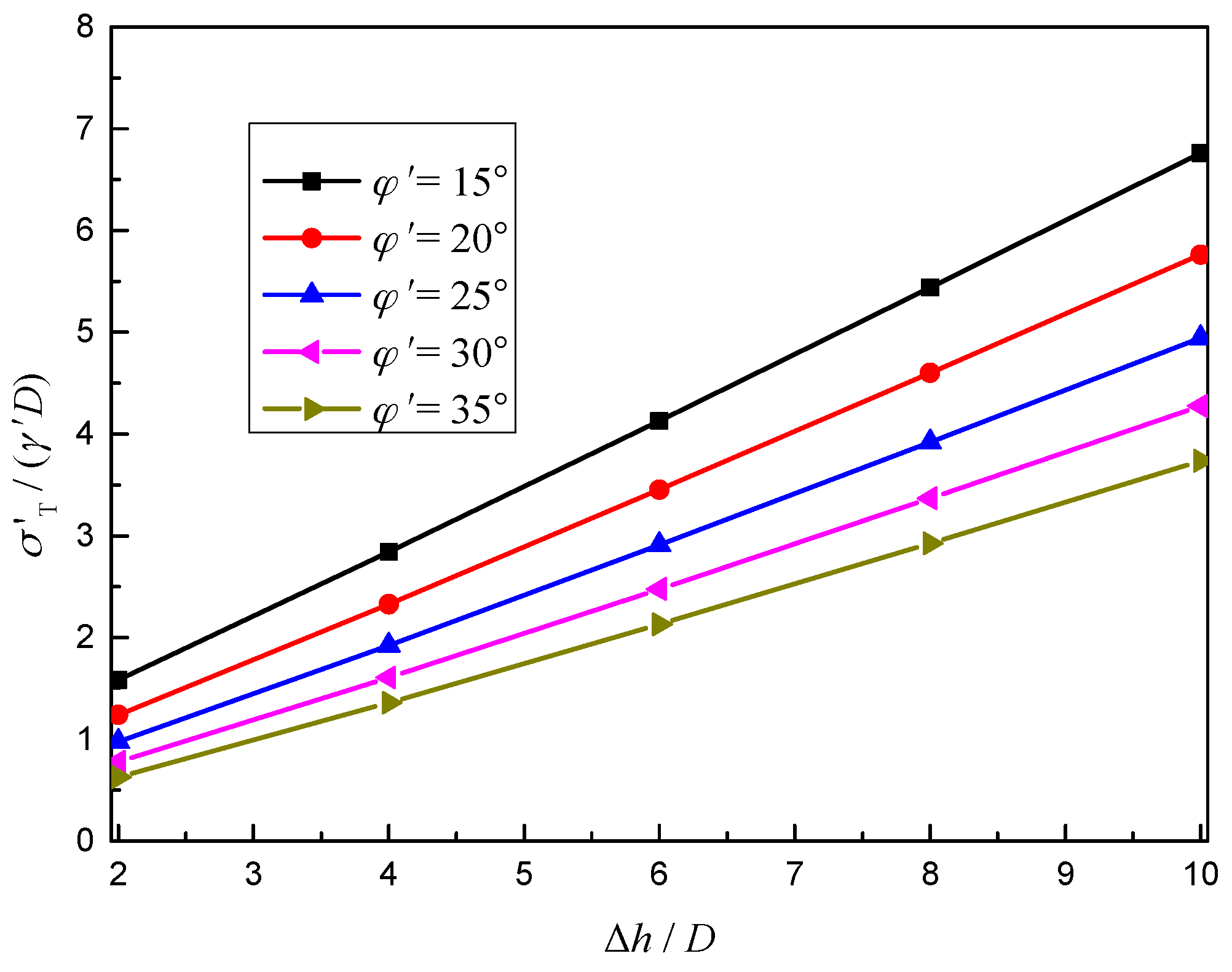
5.1. Influence of the Variables of the Hydraulic Head on Limit Support Pressures
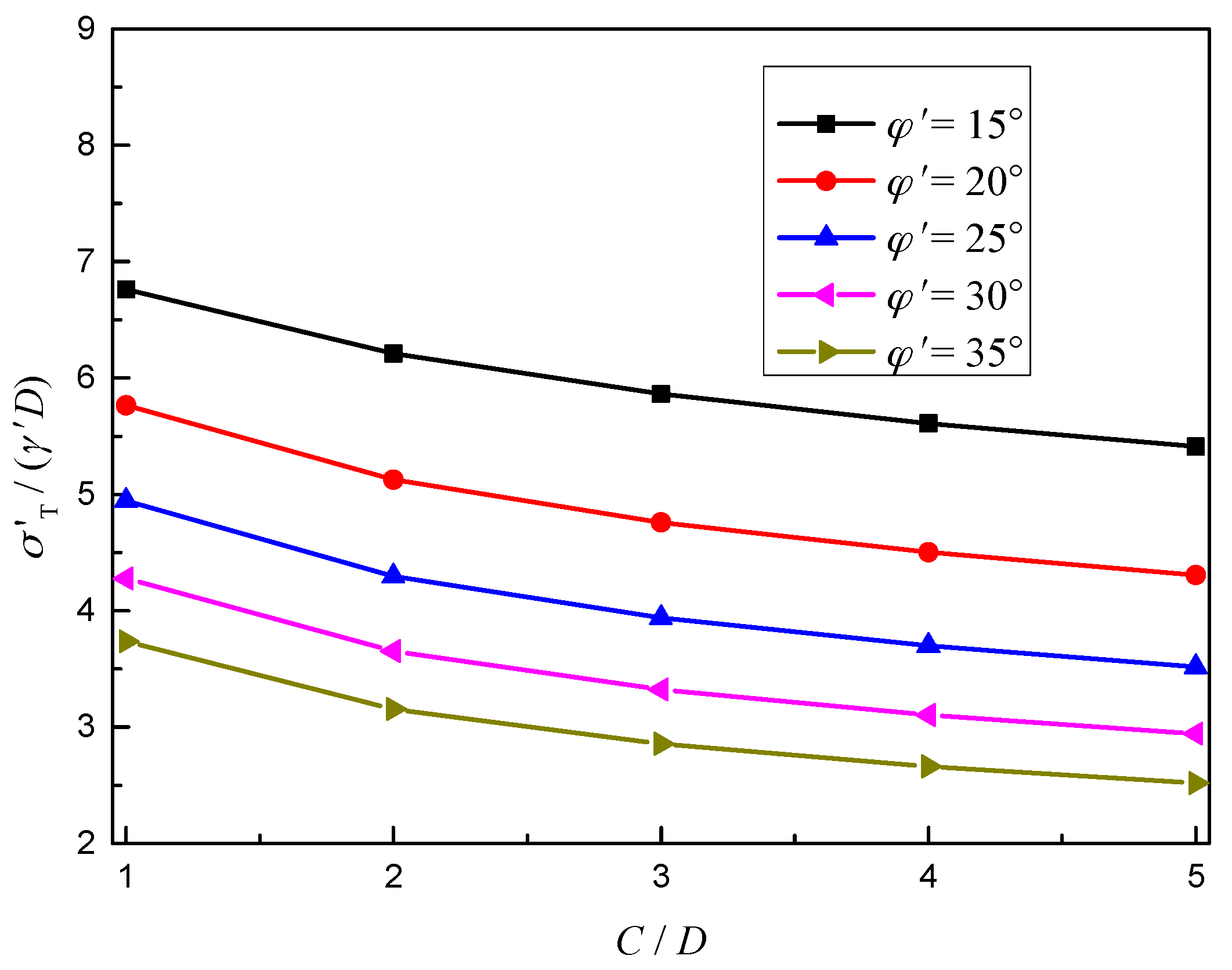
5.2. Influence of the Variables of the C/D on Limit Support Pressures
6. Conclusions
- (1)
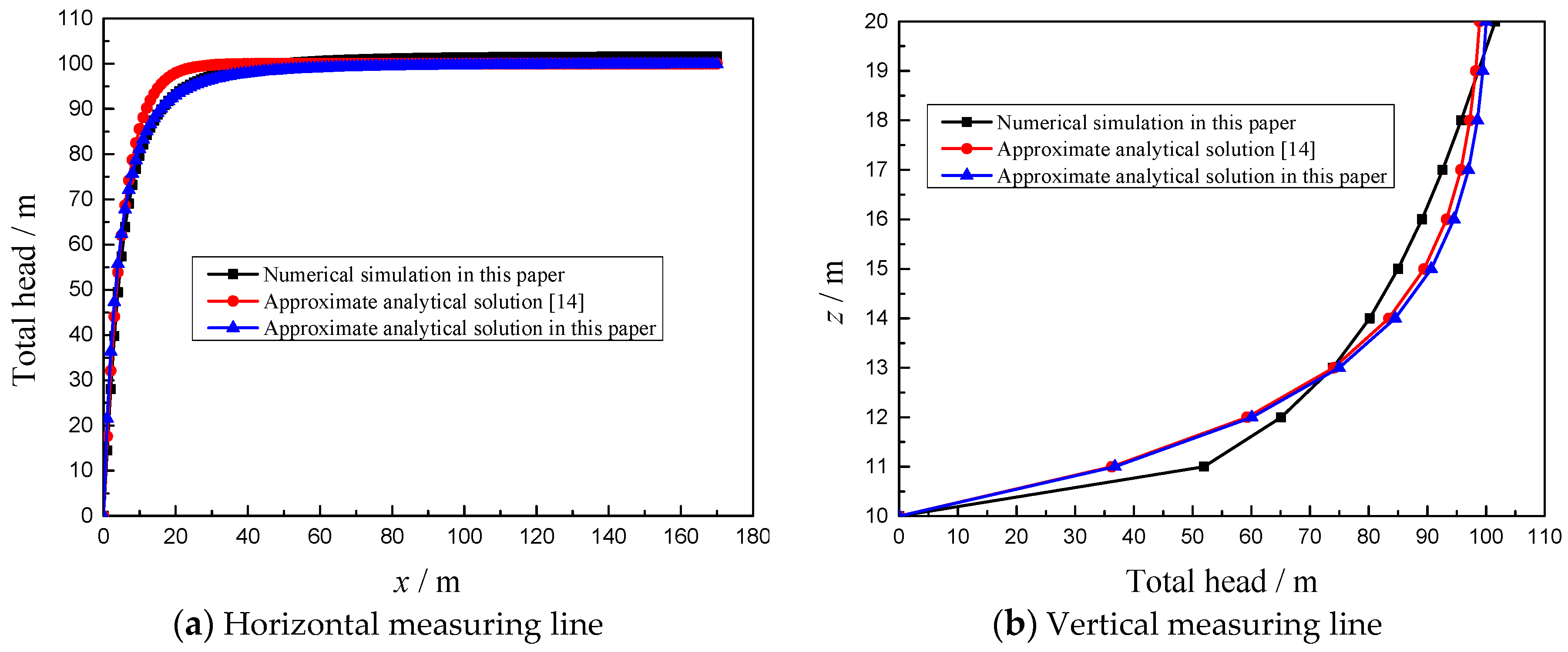
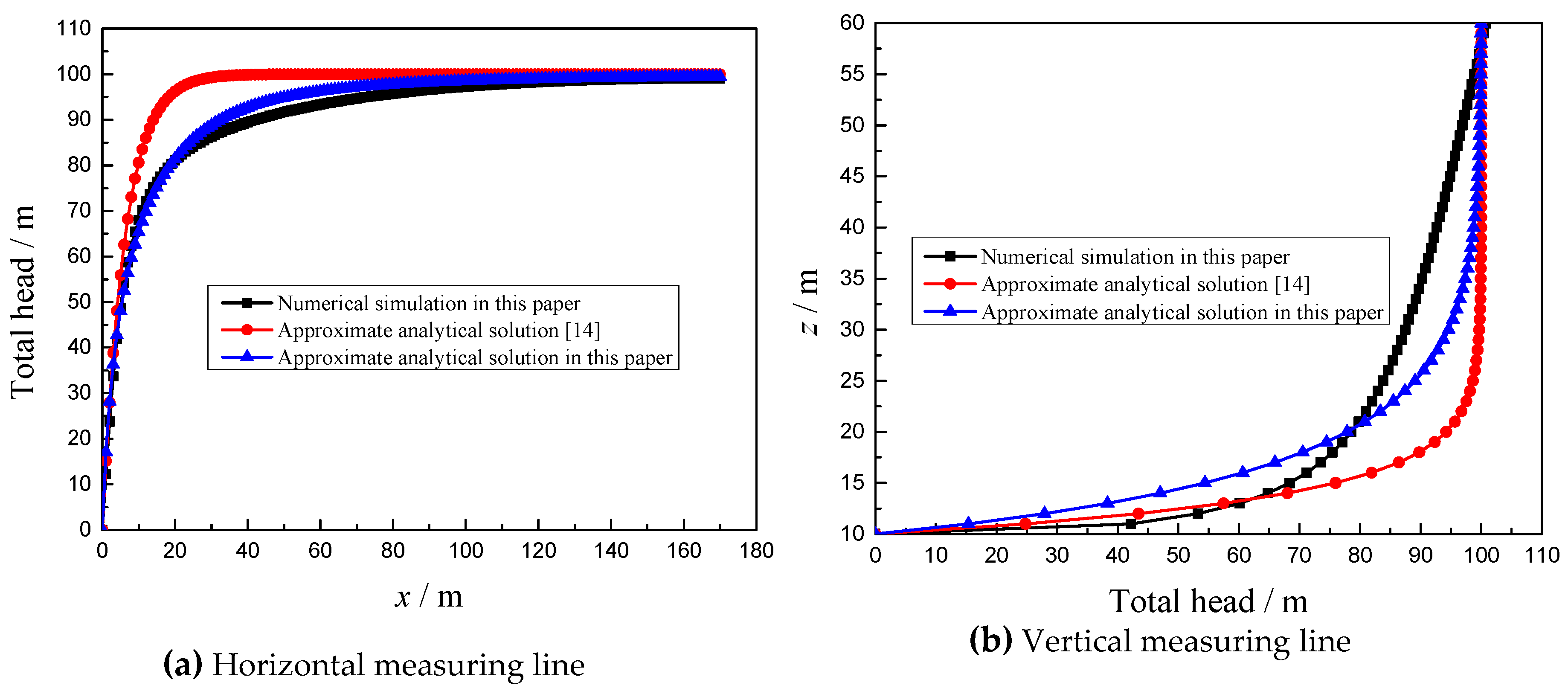
- The distribution law of total hydraulic head field along the horizontal distance at the axis of the shield tunnel is analyzed. The results show that the distribution of total hydraulic head at the axis of the shield tunnel face along the horizontal distance is a “negative exponential” function. The distribution law of total hydraulic head along the depth direction of the shield tunnel in front of the tunnel face on the vertical symmetrical surface is analyzed. The results indicate that the distribution of total hydraulic head along the depth is nonlinear when the tunnel is close to the tunnel face.
- (2)
- The comparative results of the horizontal and vertical hydraulic head field with the numerical simulations in this paper and existing approximate analytical solutions demonstrate accuracy of the formula proposed in this paper.
- (3)
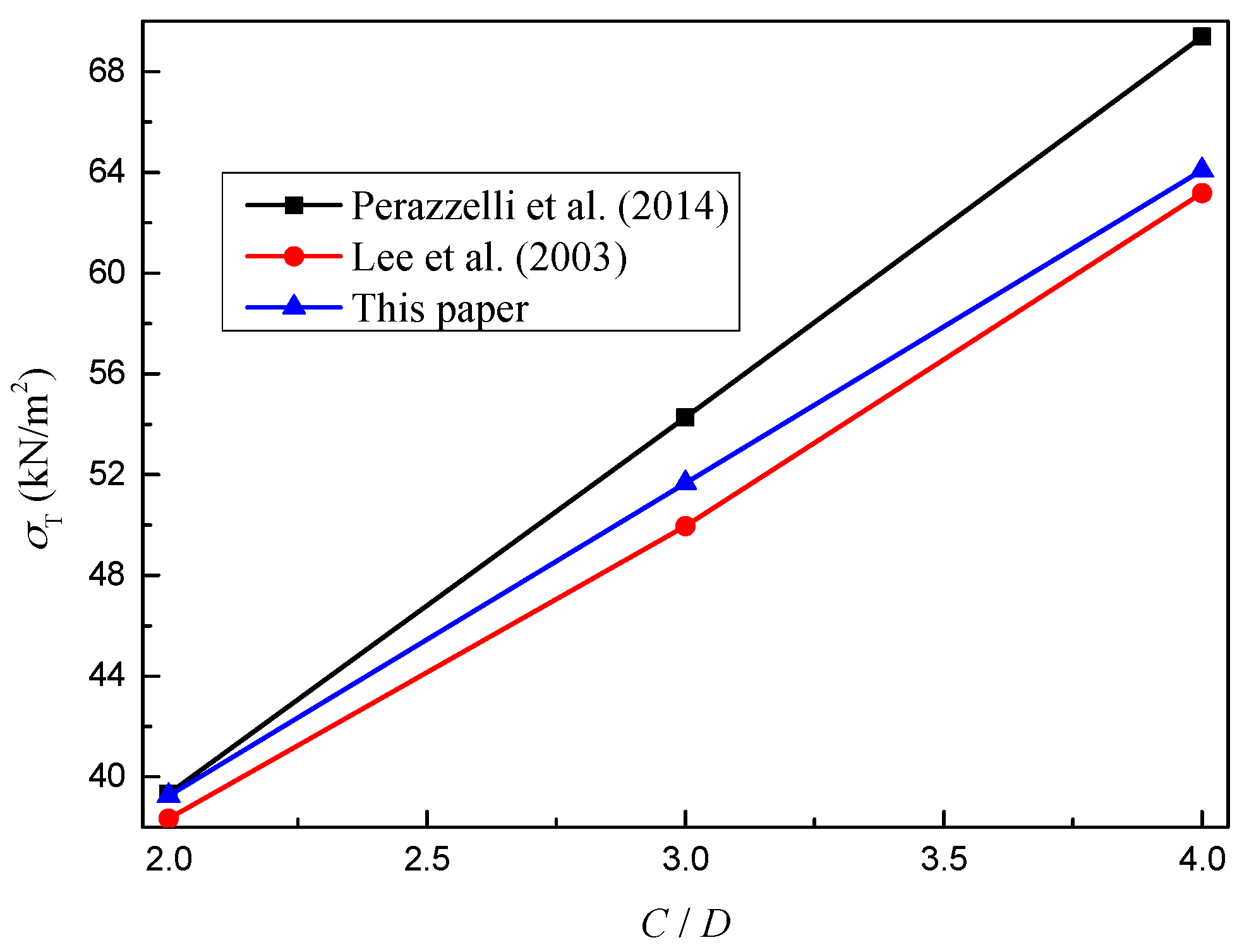
- Comparisons of the results of limit support pressure obtained from the theoretical analysis in this paper and the existing approaches show that the failure mechanism proposed in this paper could provide relatively satisfactory results for the limit support pressures applied to the tunnel face.
- (4)
- When the buried depth ratio is the same, the normalized effective limit support pressures of the shield tunnel face increases linearly with the rise of the hydraulic head. When the hydraulic head is the same, the normalized effective limit support pressures of the shield tunnel face decreases nonlinearly with the increase of the buried depth ratio. Moreover, the higher the effective friction angle of the soil, the lower the normalized effective limit support pressures will be.
Author Contributions
Funding
Conflicts of Interest
References
- Lee, I.M.; Nam, S.W.; Ahn, J.H. Effect of seepage forces on tunnel face stability. Can. Geotech. J. 2003, 40, 342–350. [Google Scholar] [CrossRef]
- Tang, L.J. Numerical Investigations and Centrifugal Model Tests on Face Stability of Shield Tunnel in Dry and Saturated Sandy Soils. Ph.D. Thesis, Zhejiang University, Hangzhou, China, 2014. (In Chinese). [Google Scholar]
- Lü, X.; Zhou, Y.; Huang, M.; Zeng, S. Experimental study of the face stability of shield tunnel in sands under seepage condition. Tunn. Undergr. Space Technol. 2018, 74, 195–205. [Google Scholar] [CrossRef]
- Chen, R.P.; Yin, X.S.; Tang, L.J.; Chen, Y.M. Centrifugal model tests on face failure of earth pressure balance shield induced by steady state seepage in saturated sandy silt ground. Tunn. Undergr. Space Technol. 2018, 81, 315–325. [Google Scholar] [CrossRef]
- DE Buhan, P.; Cuvillier, A.; Dormieux, L.; Maghous, S. Face stability of shallow circular tunnels driven under the water table: A numerical analysis. Int. J. Numer. Anal. Met. 1999, 23, 79–95. [Google Scholar] [CrossRef]
- Ströhle, P.M.; Vermeer, P.A. Tunnel face stability with groundwater flow. In Proceedings of the 7th European Conference on Numerical Methods in Geotechnical Engineering, Trondheim, Norway, 2–4 June 2010; CRC Press (Taylor & Francis): London, UK, 2010; pp. 813–818. [Google Scholar]
- Li, C.L.; Miao, L.C. Finite element simulation of seepage field and analysis of soil deformation around tunnel due to shield tunneling. J. Southeast Univ. (Nat. Sci. Ed.) 2010, 40, 1066–1072. (In Chinese) [Google Scholar]
- Lu, X.; Zhou, Y.; Huang, M.; Li, F. Computation of the minimum limit support pressure for the shield tunnel face stability under seepage condition. Int. J. Civ. Eng. 2017, 15, 849–863. [Google Scholar] [CrossRef]
- Calista, M.; Pasculli, A.; Sciarra, N. Reconstruction of the geotechnical model considering random parameters distributions. In Engineering Geology for Society and Territory; Springer International Publishing: Heidelberg, Germany, 2015; Volume 2, pp. 1347–1351. [Google Scholar]
- Vu-Bac, N.; Lahmer, T.; Zhuang, X.; Nguyen-Thoi, T.; Rabczuk, T. A software framework for probabilistic sensitivity analysis for computationally expensive models. Adv. Eng. Softw. 2016, 100, 19–31. [Google Scholar] [CrossRef]
- Pasculli, A.; Calista, M.; Sciarra, N. Variability of local stress states resulting from the application of Monte Carlo and finite difference methods to the stability study of a selected slope. Eng. Geol. 2018, 245, 370–389. [Google Scholar] [CrossRef]
- Anagnostou, G.; Kovári, K. The face stability of slurry-shield-driven tunnels. Tunn. Undergr. Space Technol. 1994, 9, 165–174. [Google Scholar] [CrossRef]
- Anagnostou, G.; Kovari, K. Face stability conditions with earth-pressure-balanced shields. Tunn. Undergr. Space Technol. 1996, 11, 165–173. [Google Scholar] [CrossRef]
- Perazzelli, P.; Leone, T.; Anagnostou, G. Tunnel face stability under seepage flow conditions. Tunn. Undergr. Space Technol. 2014, 43, 459–469. [Google Scholar] [CrossRef]
- Perazzelli, P.; Cimbali, G.; Anagnostou, G. Stability under seepage flow conditions of a tunnel face reinforced by bolts. Procedia Eng. 2017, 191, 215–224. [Google Scholar] [CrossRef]
- Lee, I.M.; Nam, S.W. The study of seepage forces acting on the tunnel lining and tunnel face in shallow tunnels. Tunn. Undergr. Space Technol. 2001, 16, 31–40. [Google Scholar] [CrossRef]
- Leca, E.; Dormieux, L. Upper and lower bound solutions for the face stability of shallow circular tunnels in frictional material. Géotechnique 1990, 40, 581–606. [Google Scholar] [CrossRef]
- Park, J.K.; Blackburn, J.T.; Ahn, J.H. Upper bound solutions for tunnel face stability considering seepage and strength increase with depth. In Proceedings of the Underground Space the 4th Dimension of Metropolises, Prague, Czech Republic, 5–10 May 2007; pp. 1217–1222. [Google Scholar]
- Lu, X.L.; Wang, H.R.; Huang, M.S. Upper bound solution for the face stability of shield tunnel below the water table. Math. Probl. Eng. 2014, 727964. [Google Scholar] [CrossRef]
- Liu, W.; Albers, B.; Zhao, Y.; Tang, X.W. Upper bound analysis for estimation of the influence of seepage on tunnel face stability in layered soils. J. Zhejiang Univ.-Sci. A 2016, 17, 886–902. [Google Scholar] [CrossRef]
- Huangfu, M.; Wang, M.S.; Tan, Z.S.; Wang, X.Y. Analytical solutions for steady seepage into an underwater circular tunnel. Tunn. Undergr. Space Technol. 2010, 25, 391–396. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, X.J.; Tang, X.W.; Chen, R.P. Supporting pressure for earth pressure balance tunnel face stability when tunneling is implemented in saturated sandy soil. J. Zhejiang Univ. (Eng. Sci.) 2012, 46, 664–704. (In Chinese) [Google Scholar]
- Lei, H.B. Numerical Simulation and Approximate Analytical Solution for Groundwater Flow in Vicinity of the Face of Shield Tunnel Excavations. Master’s Thesis, Beijing Jiaotong University, Beijing, China, 2016. (In Chinese). [Google Scholar]


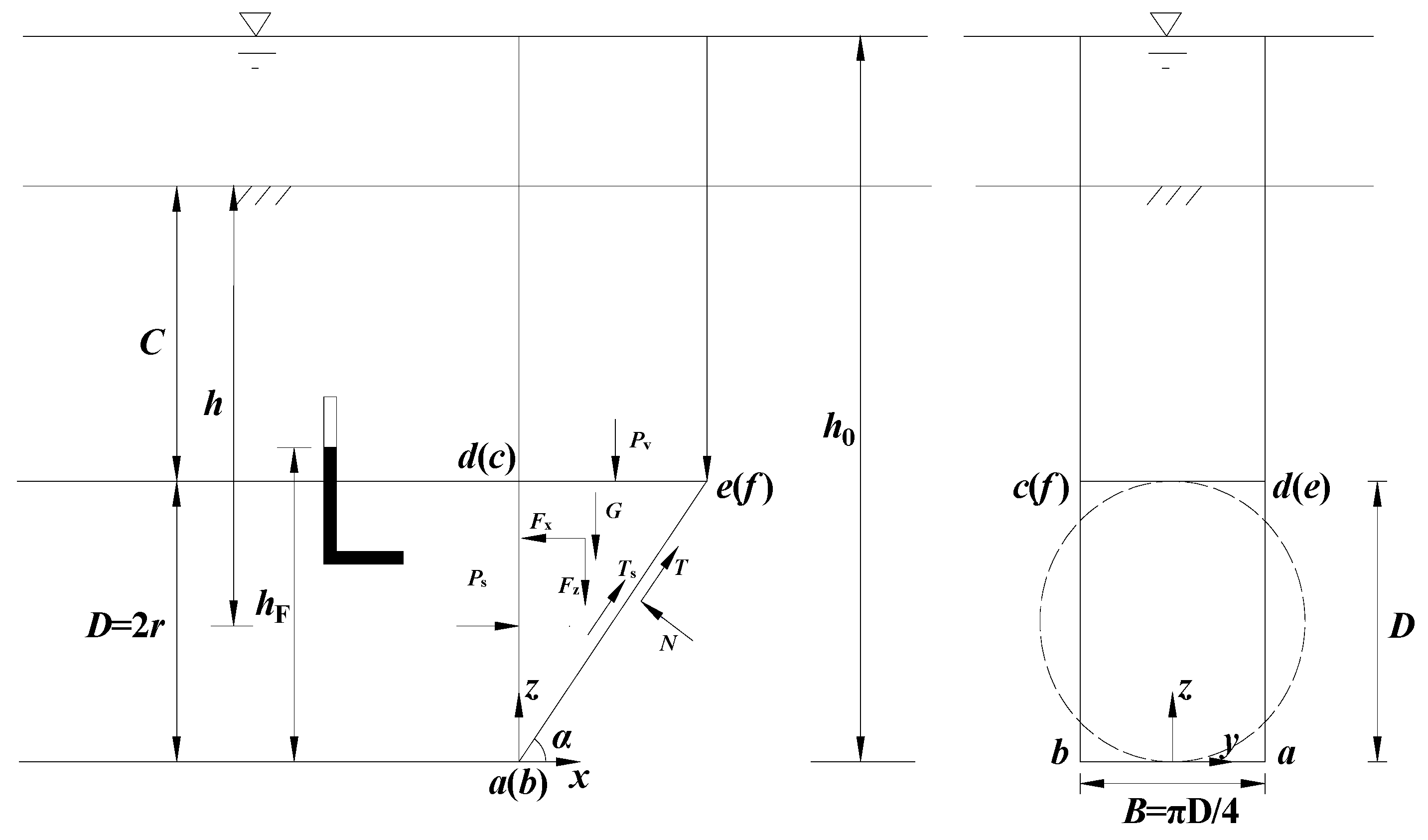
| References | Hydraulic Gradient | Parameter Specification |
|---|---|---|
| Liu et al. [22] | h0—undisturbed hydraulic head hF—hydraulic head on the tunnel face C—depth of cover D—diameter of the tunnel face | |
| Perazzelli et al. [14] | a,b—constants determined by curve fitting to the numerical results H—height of the tunnel face x,z—vertical and horizontal coordinates | |
| Lei [23] | a,b,c—constants determined by curve fitting to the numerical results r—radius of the tunnel face | |
| Liu et al. [20] | d1,d2—the thicknesses of the cover layers k0, k1—the permeabilities in the crossed layer and cover layer |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Han, K.; Xie, T.; Luo, J. Calculation of Limit Support Pressure for EPB Shield Tunnel Face in Water-Rich Sand. Symmetry 2019, 11, 1102. https://doi.org/10.3390/sym11091102
Wang L, Han K, Xie T, Luo J. Calculation of Limit Support Pressure for EPB Shield Tunnel Face in Water-Rich Sand. Symmetry. 2019; 11(9):1102. https://doi.org/10.3390/sym11091102
Chicago/Turabian StyleWang, Lin, Kaihang Han, Tingwei Xie, and Jianjun Luo. 2019. "Calculation of Limit Support Pressure for EPB Shield Tunnel Face in Water-Rich Sand" Symmetry 11, no. 9: 1102. https://doi.org/10.3390/sym11091102
APA StyleWang, L., Han, K., Xie, T., & Luo, J. (2019). Calculation of Limit Support Pressure for EPB Shield Tunnel Face in Water-Rich Sand. Symmetry, 11(9), 1102. https://doi.org/10.3390/sym11091102






