Quantum Behavior of a
PT
-Symmetric Two-Mode System with Cross-Kerr Nonlinearity
Abstract
1. Introduction
2. Quantum Hamiltonian and Dynamical Equations
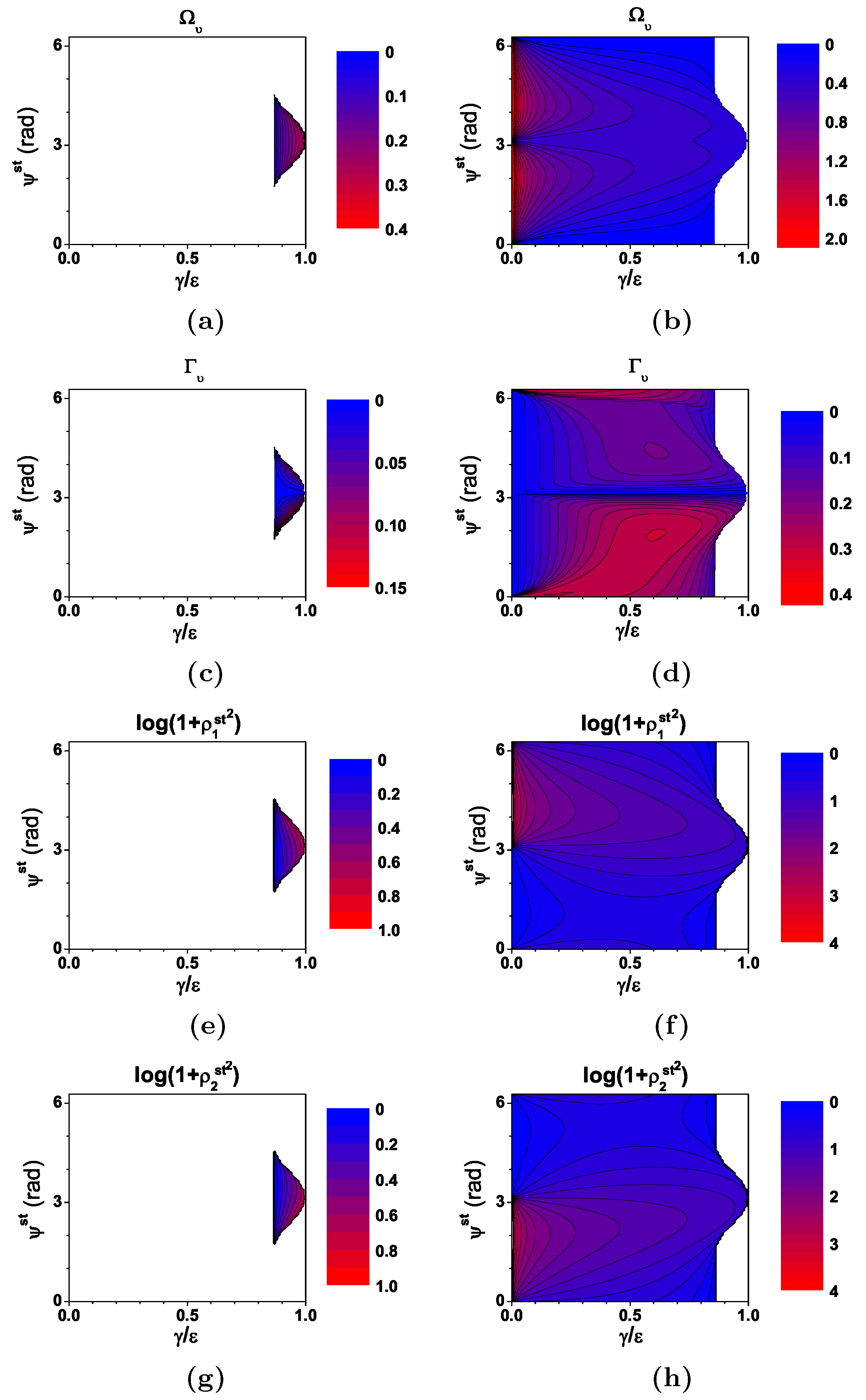
3. Stationary States and Their Stability
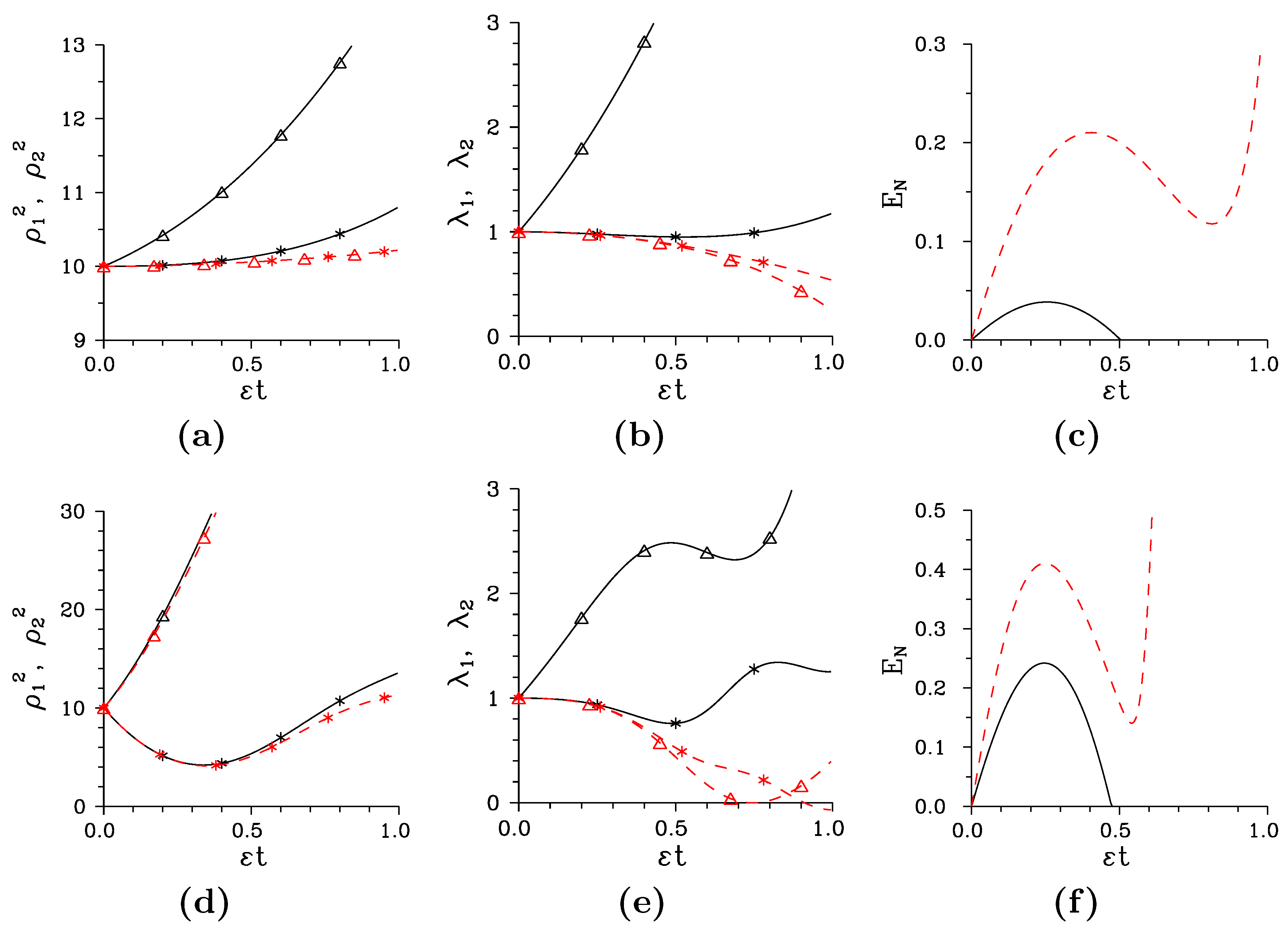
4. Quantum Properties of the Evolving States
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bender, C.M.; Boettcher, S. Real Spectra in non-Hermitian Hamiltonians Having Symmetry. Phys. Rev. Lett. 1998, 80, 5243–5246. [Google Scholar] [CrossRef]
- Bender, C.M.; Boettcher, S.; Meisinger, P.N. -Symmetric quantum mechanics. J. Math. Phys. 1999, 40, 2201–2229. [Google Scholar] [CrossRef]
- Bender, C.M.; Brody, D.C.; Jones, H.F. Must a Hamiltonian be Hermitian? Am. J. Phys. 2003, 71, 1095–1102. [Google Scholar] [CrossRef]
- Morales, J.D.H.; Guerrero, J.; López-Aguayo, S.; Rodríguez-Lara, B.M. Revisiting the Optical -Symmetric Dimer. Symmetry 2016, 8, 83. [Google Scholar] [CrossRef]
- Meystre, P.; Sargent, M., III. Elements of Quantum Optics, 4th ed.; Springer: Berlin, Germany, 2007. [Google Scholar]
- Sargent, M.; Scully, M.O.; Lamb, W.E. Laser Physics; Addison-Wesley: Boston, MA, USA, 1974. [Google Scholar]
- El-Ganainy, R.; Makris, K.G.; Christodoulides, D.N.; Musslimani, Z.H. Theory of coupled optical -Symmetric structures. Opt. Lett. 2007, 32, 2632–2634. [Google Scholar] [CrossRef] [PubMed]
- Ramezani, H.; Kottos, T.; El-Ganainy, R.; Christodoulides, D.N. Unidirectional nonlinear -Symmetric optical structures. Phys. Rev. A 2010, 82, 043803. [Google Scholar] [CrossRef]
- Zyablovsky, A.A.; Vinogradov, A.P.; Pukhov, A.A.; Dorofeenko, A.V.; Lisyansky, A.A. -symmetry in optics. Phys.-Uspekhi 2014, 57, 1063–1082. [Google Scholar] [CrossRef]
- Ögren, M.; Abdullaev, F.K.; Konotop, V.V. Solitons in a -Symmetric χ(2) coupler. Opt. Lett. 2017, 42, 4079–4082. [Google Scholar] [CrossRef]
- Turitsyna, E.G.; Shadrivov, I.V.; Kivshar, Y.S. Guided modes in non-Hermitian optical waveguides. Phys. Rev. A 2017, 96, 033824. [Google Scholar] [CrossRef]
- Xu, X.; Shi, L.; Ren, L.; Zhang, X. Optical gradient forces in -Symmetric coupled-waveguide structures. Opt. Express 2018, 26, 10220–10229. [Google Scholar] [CrossRef]
- Liu, Z.P.; Zhang, J.; Özdemir, S.K.; Peng, B.; Jing, H.; Lü, X.Y.; Li, C.W.; Yang, L.; Nori, F.; Liu, Y.X. Metrology with -Symmetric Cavities: Enhanced Sensitivity near the -Phase Transition. Phys. Rev. Lett. 2016, 117, 110802. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Chong, Y.D. symmetry breaking and nonlinear optical isolation in coupled microcavities. Opt. Express 2016, 24, 6916–6930. [Google Scholar] [CrossRef] [PubMed]
- Arkhipov, I.I.; Miranowicz, A.; Di Stefano, O.; Stassi, R.; Savasta, S.; Nori, F.; Özdemir, S.K. Scully-Lamb quantum laser model for parity-time-symmetric whispering-gallery microcavities: Gain saturation effects and nonreciprocity. Phys. Rev. A 2019, 99, 053806. [Google Scholar] [CrossRef]
- Graefe, E.M.; Jones, H.F. -Symmetric sinusoidal optical lattices at the symmetry-breaking threshold. Phys. Rev. A 2011, 84, 013818. [Google Scholar] [CrossRef]
- Miri, M.A.; Regensburger, A.; Peschel, U.; Christodoulides, D.N. Optical mesh lattices with symmetry. Phys. Rev. A 2012, 86, 023807. [Google Scholar] [CrossRef]
- Ornigotti, M.; Szameit, A. Quasi -symmetry in passive photonic lattices. J. Opt. 2014, 16, 065501. [Google Scholar] [CrossRef]
- Shui, T.; Yang, W.X.; Li, L.; Wang, X. Lop-sided Raman-Nath diffraction in -antisymmetric atomic lattices. Opt. Lett. 2019, 44, 2089–2092. [Google Scholar] [CrossRef]
- Tchodimou, C.; Djorwe, P.; Nana Engo, S.G. Distant entanglement enhanced in -Symmetric optomechanics. Phys. Rev. A 2017, 96, 033856. [Google Scholar] [CrossRef]
- Wang, D.Y.; Bai, C.H.; Liu, S.; Zhang, S.; Wang, H.F. Distinguishing photon blockade in a -Symmetric optomechanical system. Phys. Rev. A 2019, 99, 043818. [Google Scholar] [CrossRef]
- Peřina, J. Quantum Statistics of Linear and Nonlinear Optical Phenomena; Kluwer: Dordrecht, The Netherlands, 1991. [Google Scholar]
- Agarwal, G.S.; Qu, K. Spontaneous generation of photons in transmission of quantum fields in -Symmetric optical systems. Phys. Rev. A 2012, 85, 31802(R). [Google Scholar] [CrossRef]
- Scheel, S.; Szameit, A. -Symmetric photonic quantum systems with gain and loss do not exist. Eur. Phys. Lett. 2018, 122, 34001. [Google Scholar] [CrossRef]
- Peřinová, V.; Lukš, A.; Křepelka, J. Quantum description of a -Symmetric nonlinear directional coupler. J. Opt. Soc. Am. B 2019, 36, 855–861. [Google Scholar] [CrossRef]
- Antonosyan, D.A.; Solntsev, A.S.; Sukhorukov, A.A. Photon-pair generation in a quadratically nonlinear parity-time symmetric coupler. Phot. Res. 2018, 6, A6–A9. [Google Scholar] [CrossRef]
- Naikoo, J.; Thapliyal, K.; Banerjee, S.; Pathak, A. Quantum Zeno effect and nonclassicality in a -Symmetric system of coupled cavities. Phys. Rev. A 2019, 99, 023820. [Google Scholar] [CrossRef]
- Miranowicz, A.; Leoński, W. Two-mode optical state truncation and generation of maximally entangled states in pumped nonlinear couplers. J. Phys. B At. Mol. Opt. Phys. 2006, 39, 1683–1700. [Google Scholar] [CrossRef]
- He, B.; Yan, S.B.; Wang, J.; Xiao, M. Quantum noise effects with Kerr-nonlinearity enhancement in coupled gain-loss waveguides. Phys. Rev. A 2015, 91, 053832. [Google Scholar] [CrossRef]
- Kalaga, J.K.; Kowalewska-Kudłaszyk, A.; Leoński, W.; Barasinski, A. Quantum correlations and entanglement in a model comprised of a short chain of nonlinear oscillators. Phys. Rev. A 2016, 94, 032304. [Google Scholar] [CrossRef]
- Vashahri-Ghamsari, S.; He, B.; Xiao, M. Continuous-variable entanglement generation using a hybrid -Symmetric system. Phys. Rev. A 2017, 96, 033806. [Google Scholar] [CrossRef]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Boyd, R.W. Nonlinear Optics, 2nd ed.; Academic Press: New York, NY, USA, 2003. [Google Scholar]
- Peřina, J., Jr. Coherent light in intense spatiospectral twin beams. Phys. Rev. A 2016, 93, 063857. [Google Scholar] [CrossRef]
- Peřina, J., Jr.; Peřina, J. Quantum statistics of nonlinear optical couplers. In Progress in Optics; Wolf, E., Ed.; Elsevier: Amsterdam, The Netherlands, 2000; Volume 41, pp. 361–419. [Google Scholar]
- Thapliyal, K.; Pathak, A.; Sen, B.; Peřina, J. Higher-order nonclassicalities in a codirectional nonlinear optical coupler: Quantum entanglement, squeezing, and antibunching. Phys. Rev. A 2014, 90, 013808. [Google Scholar] [CrossRef]
- Sanders, B.C.; Milburn, G.J. Complementarity in a quantum nondemolition measurement. Phys. Rev. A 1989, 39, 694–702. [Google Scholar] [CrossRef] [PubMed]
- Leoński, W.; Kowalewska-Kudlaszyk, A. Quantum Scissors and Finite-Dimensional States Engineering. In Progress in Optics; Wolf, E., Ed.; Elsevier: Amsterdam, The Netherlands, 2011; Volume 56, pp. 131–185. [Google Scholar]
- Kalaga, J.K.; Jarosik, M.W.; Szczȩśniak, R.; Nguen, T.D.; Leoński, W. Pulsed Nonlinear Coupler as an Effective Tool for the Bell-Like States Generation. Acta Phys. Polon. A 2019, 135, 273–275. [Google Scholar] [CrossRef]
- Kalaga, J.K.; Kowalewska-Kudłaszyk, A.; Jarosik, M.W.; Szczȩśniak, R.; Leoński, W. Enhancement of the entanglement generation via randomly perturbed series of external pulses in a nonlinear Bose-Hubbard dimer. Nonlinear Dyn. 2019. [Google Scholar] [CrossRef]
- Korolkova, N.; Peřina, J. Kerr nonlinear coupler with varying linear coupling coefficient. J. Mod. Opt. 1997, 44, 1525–1534. [Google Scholar] [CrossRef]
- Fiurášek, J.; Křepelka, J.; Peřina, J. Quantum-phase properties of the Kerr couplers. Opt. Commun. 1999, 167, 115–124. [Google Scholar] [CrossRef]
- Ariunbold, G.; Peřina, J. Non-classical behaviour and switching in Kerr nonlinear couplers. J. Mod. Opt. 2001, 48, 1005–1019. [Google Scholar] [CrossRef]
- Bartkowiak, M.; Wu, L.A.; Miranowicz, A. Quantum circuits for amplification of Kerr nonlinearity via quadrature squeezing. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 145501. [Google Scholar] [CrossRef]
- Peřina, J., Jr.; Lukš, A.; Kalaga, J.; Leoński, W.; Miranowicz, A. Nonclassical light in quantum -Symmetric two-mode systems. To be published.
- Sukhorukov, A.A.; Xu, Z.; Kivshar, Y.S. Nonlinear suppression of time reversals in -Symmetric optical couplers. Phys. Rev. A 2010, 83, 043818. [Google Scholar] [CrossRef]
- Callen, H.B.; Welton, T.A. Irreversibility and Generalized Noise. Phys. Rev. 1951, 83, 34–40. [Google Scholar] [CrossRef]
- Kubo, R. The fluctuation-dissipation theorem. Rep. Prog. Phys. 1966, 29, 255–284. [Google Scholar] [CrossRef]
- Lukš, A.; Peřinová, V.; Peřina, J. Principal squeezing of vacuum fluctuations. Opt. Commun. 1988, 67, 149–151. [Google Scholar] [CrossRef]
- Hill, S.; Wootters, W.K. Computable entanglement. Phys. Rev. Lett. 1997, 78, 5022. [Google Scholar] [CrossRef]
- Adesso, G.; Illuminati, F. Entanglement in continuous variable systems: Recent advances and current perspectives. J. Phys. A Math. Theor. 2007, 40, 7821–7880. [Google Scholar] [CrossRef]
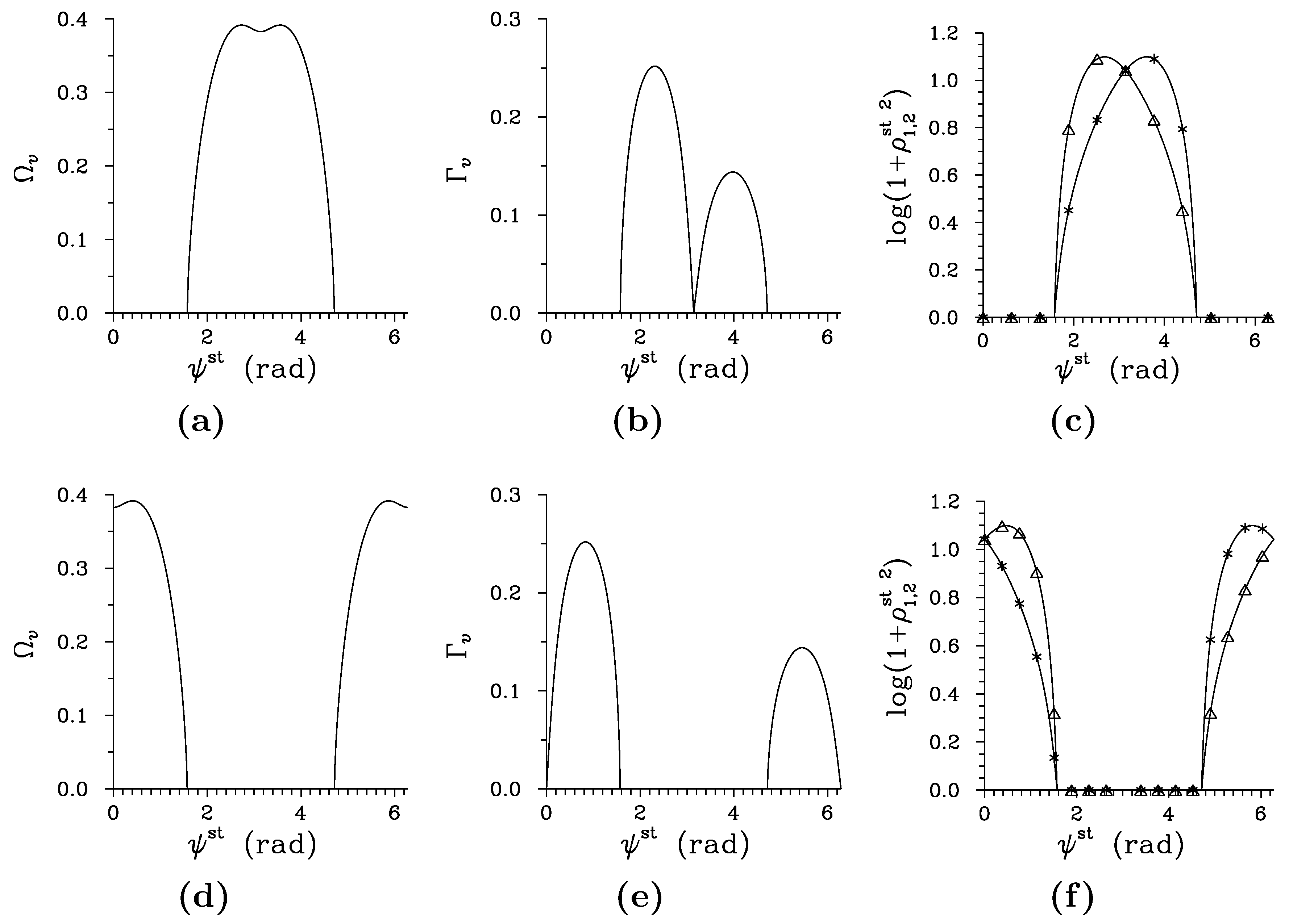
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peřina, J., Jr.; Lukš, A.
Quantum Behavior of a
Peřina J Jr., Lukš A.
Quantum Behavior of a
Peřina, Jan, Jr., and Antonín Lukš.
2019. "Quantum Behavior of a
Peřina, J., Jr., & Lukš, A.
(2019). Quantum Behavior of a





