Correlation Dynamics of Dipolar Bosons in 1D Triple Well Optical Lattice
Abstract
1. Introduction
2. Methodology
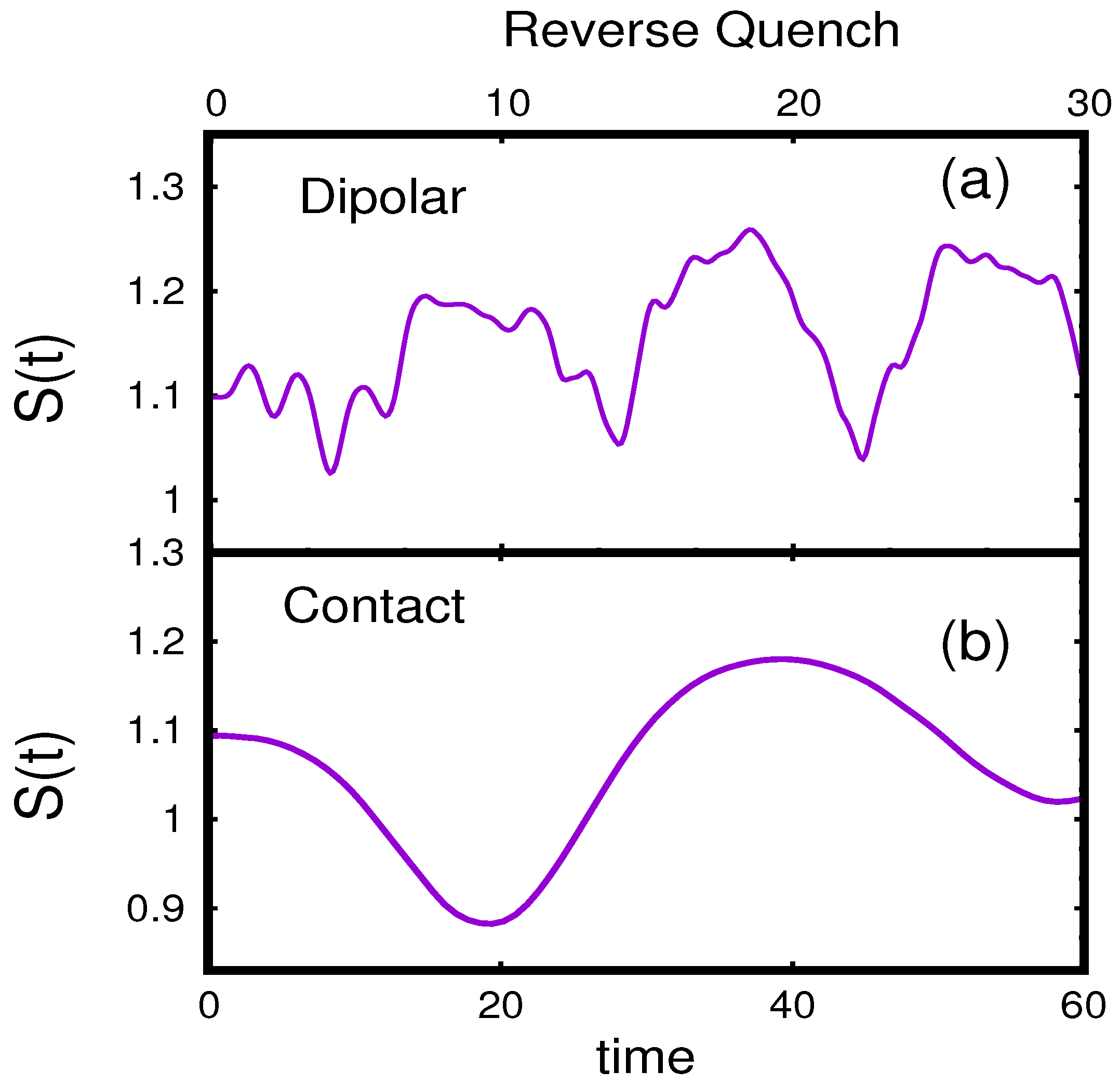
3. Quantities of Interest
4. Results for Quench Dynamics
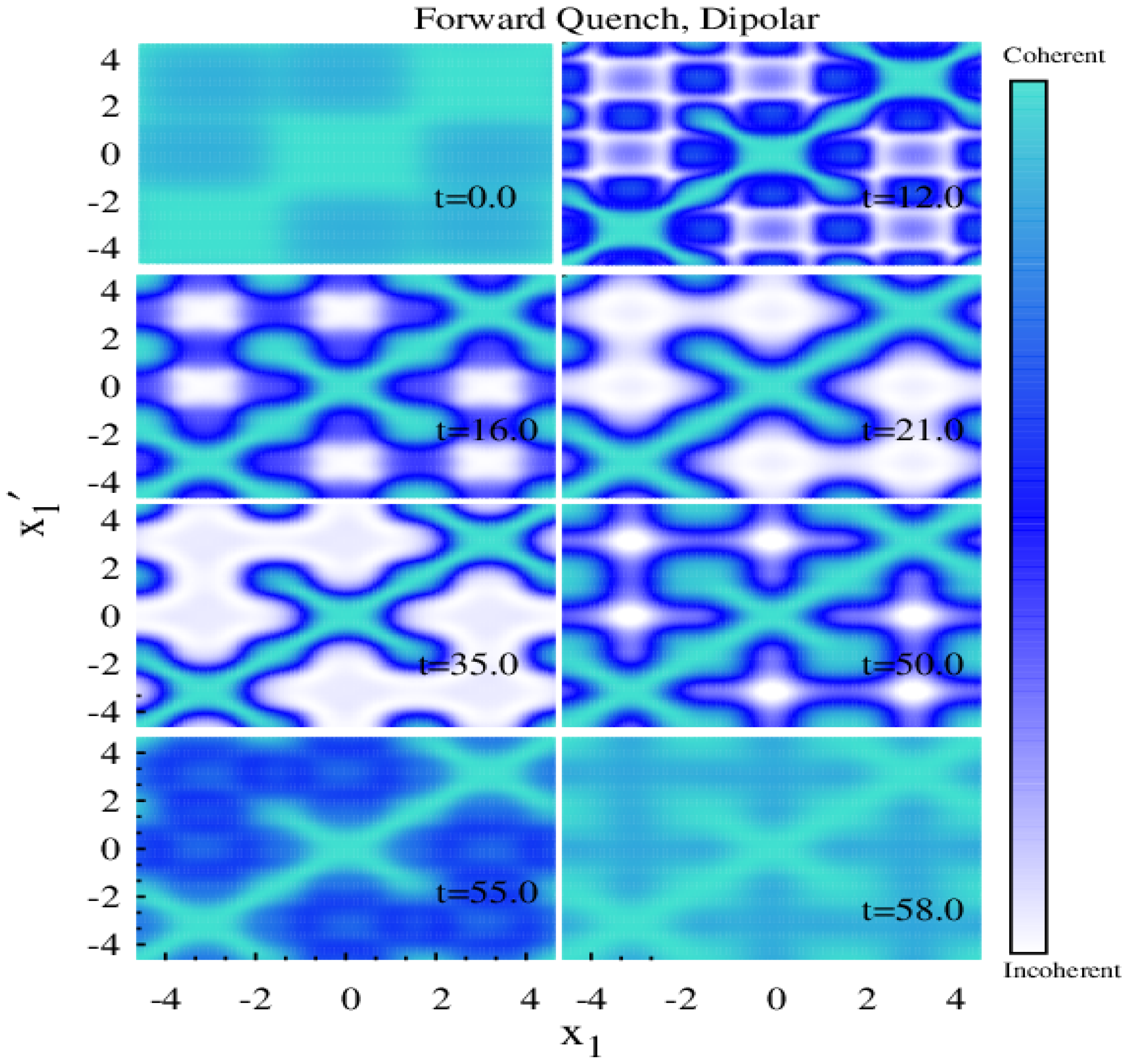
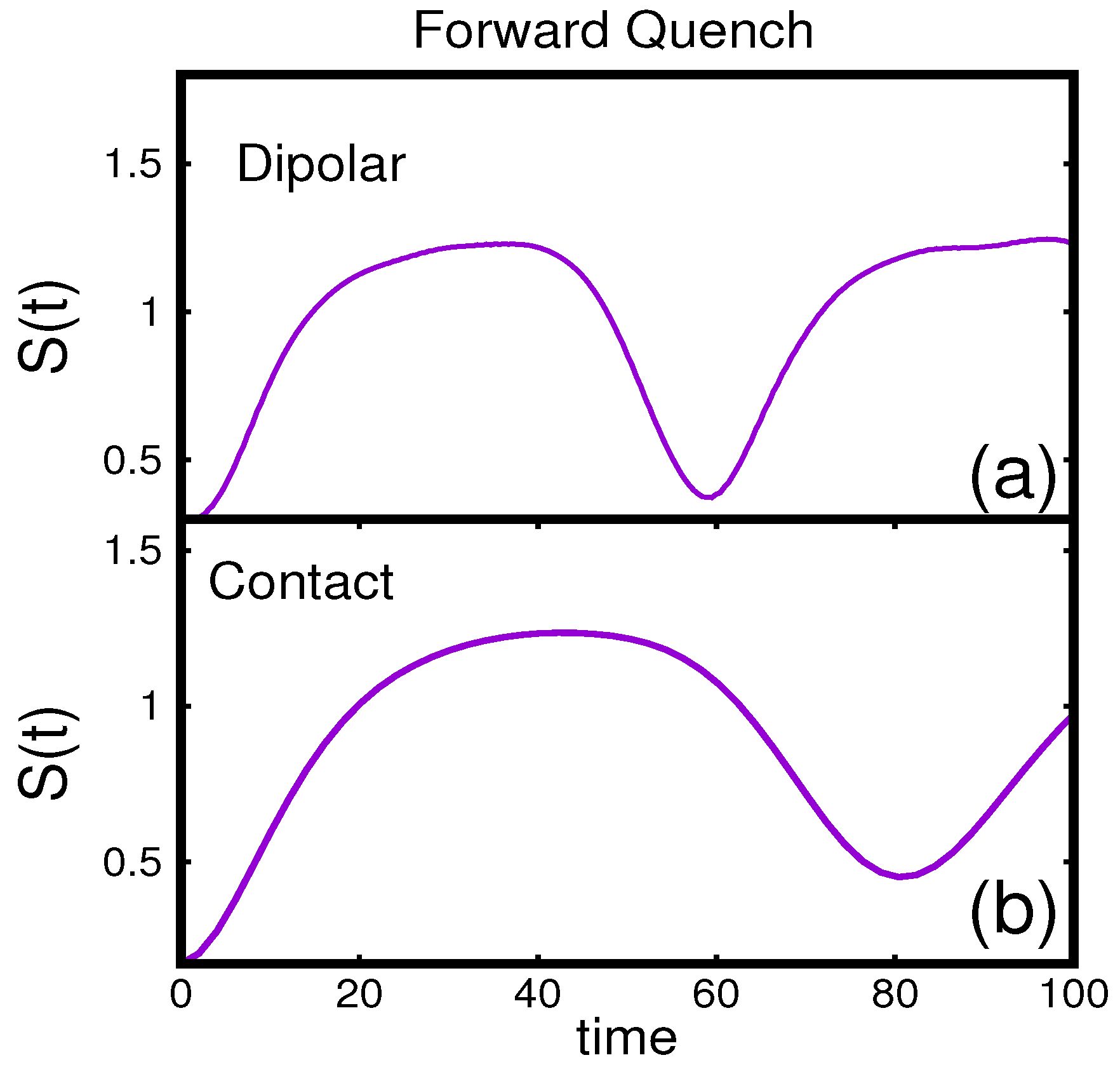
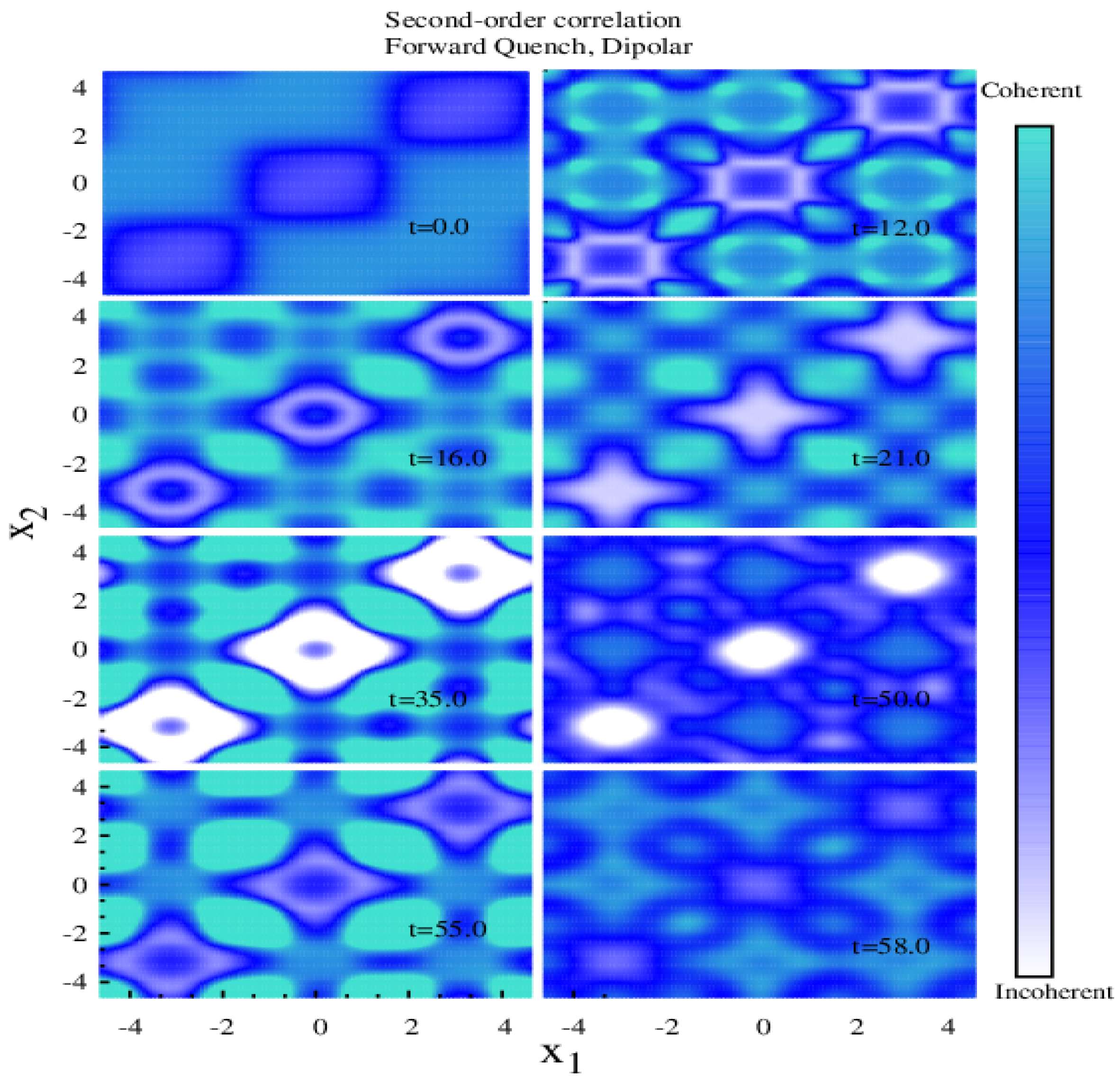
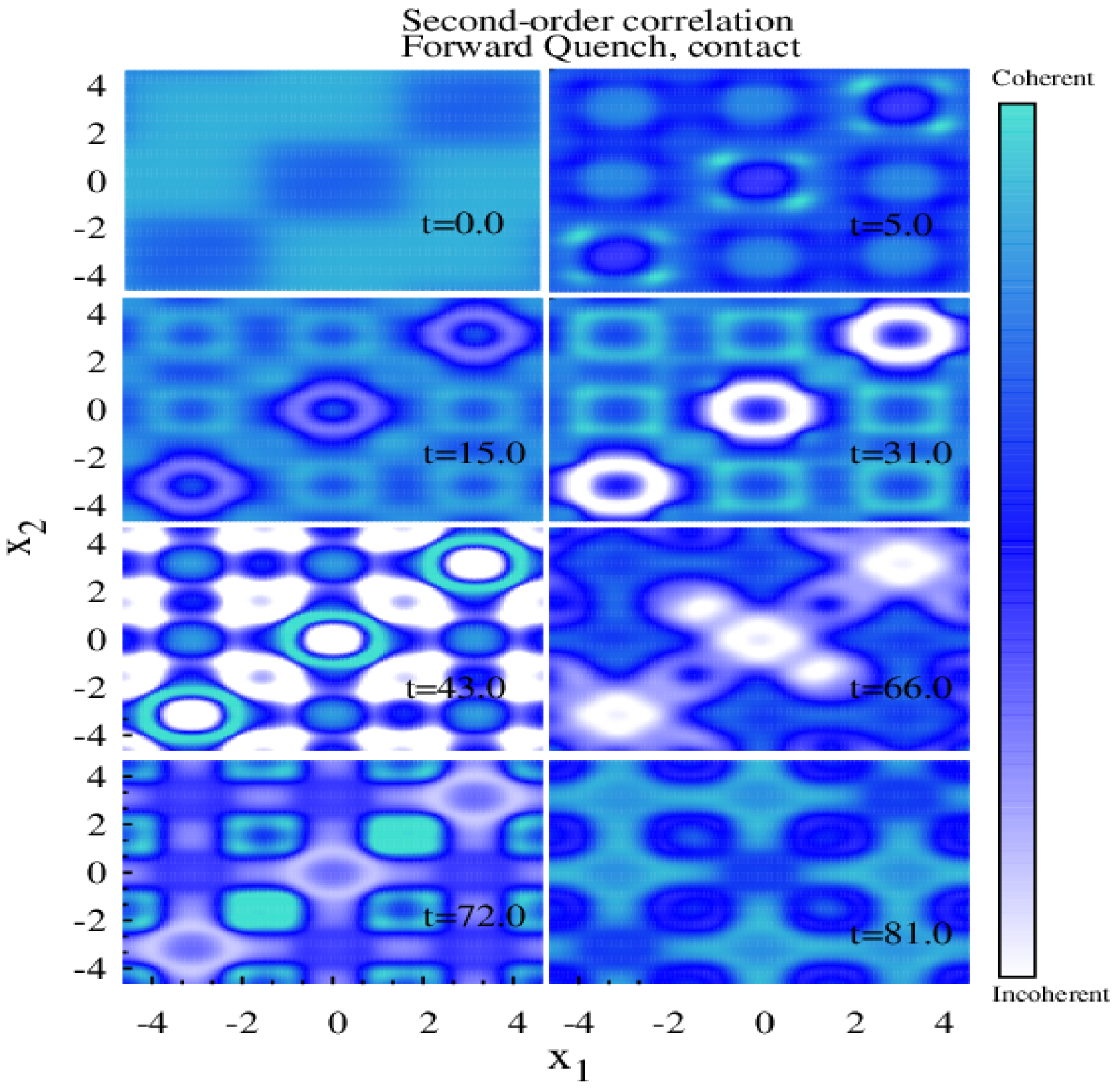
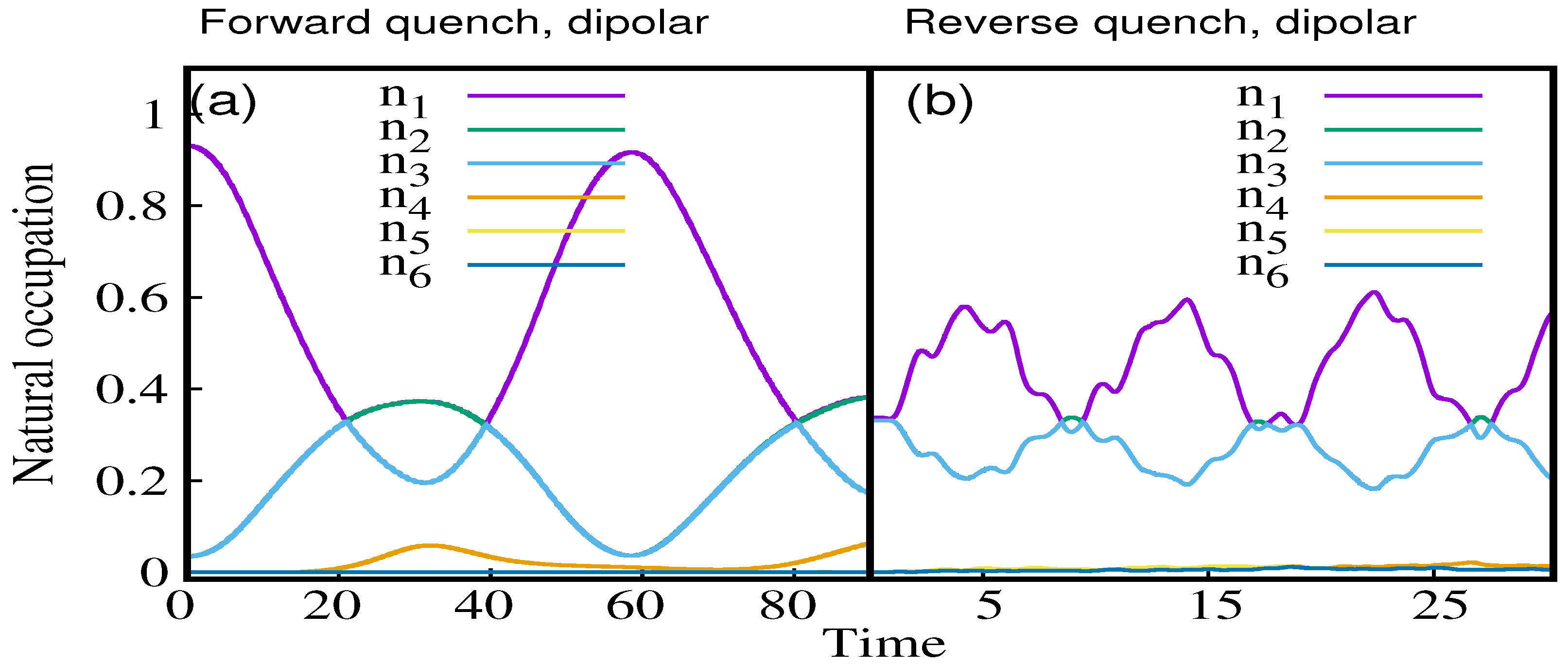
4.1. Forward Quench (, )
- (a)
- The system enters completely fragmented phase.
- (b)
- The Shannon information entropy becomes maximum.
- (c)
- The off-diagonal correlation is completely lost.
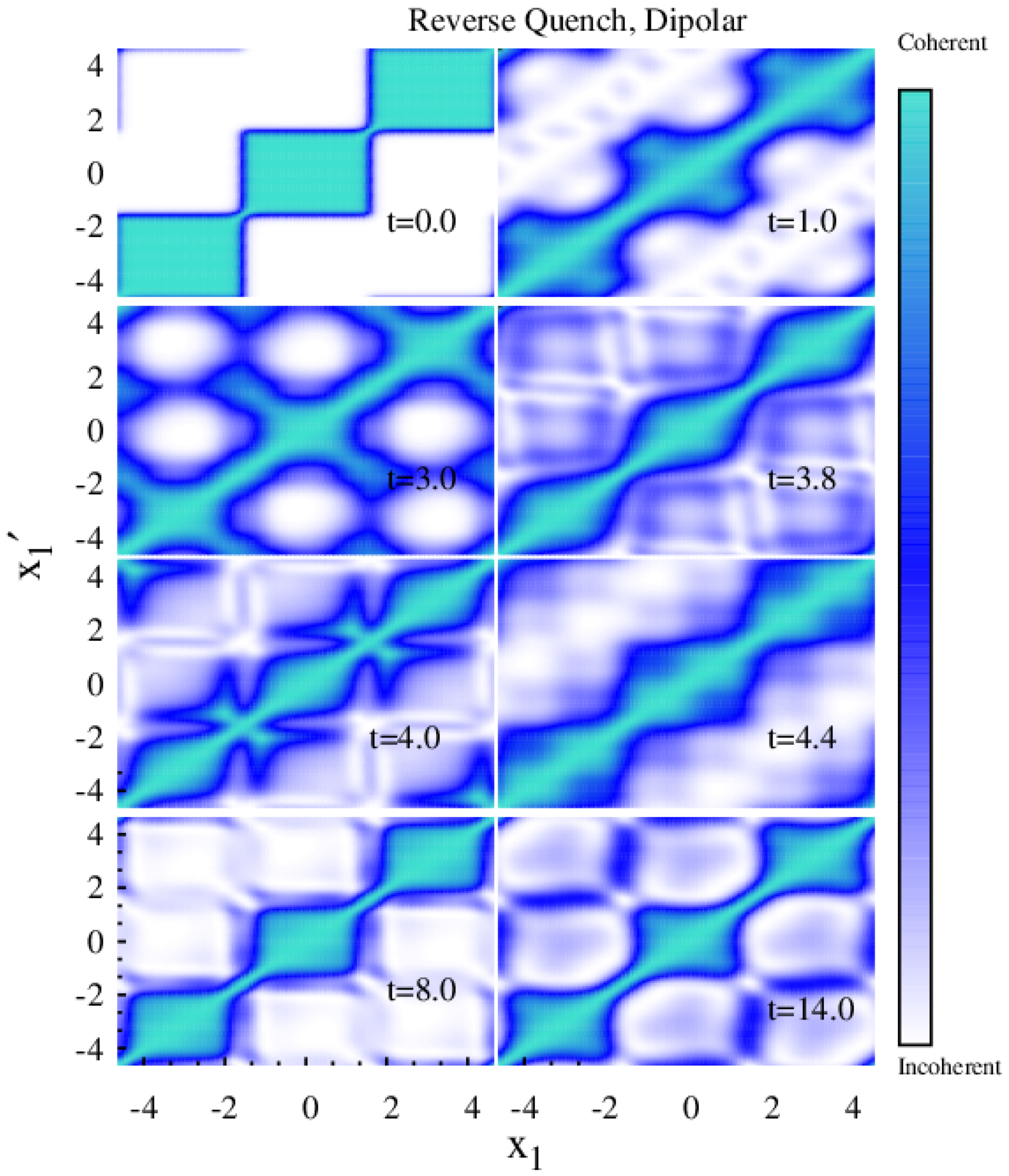
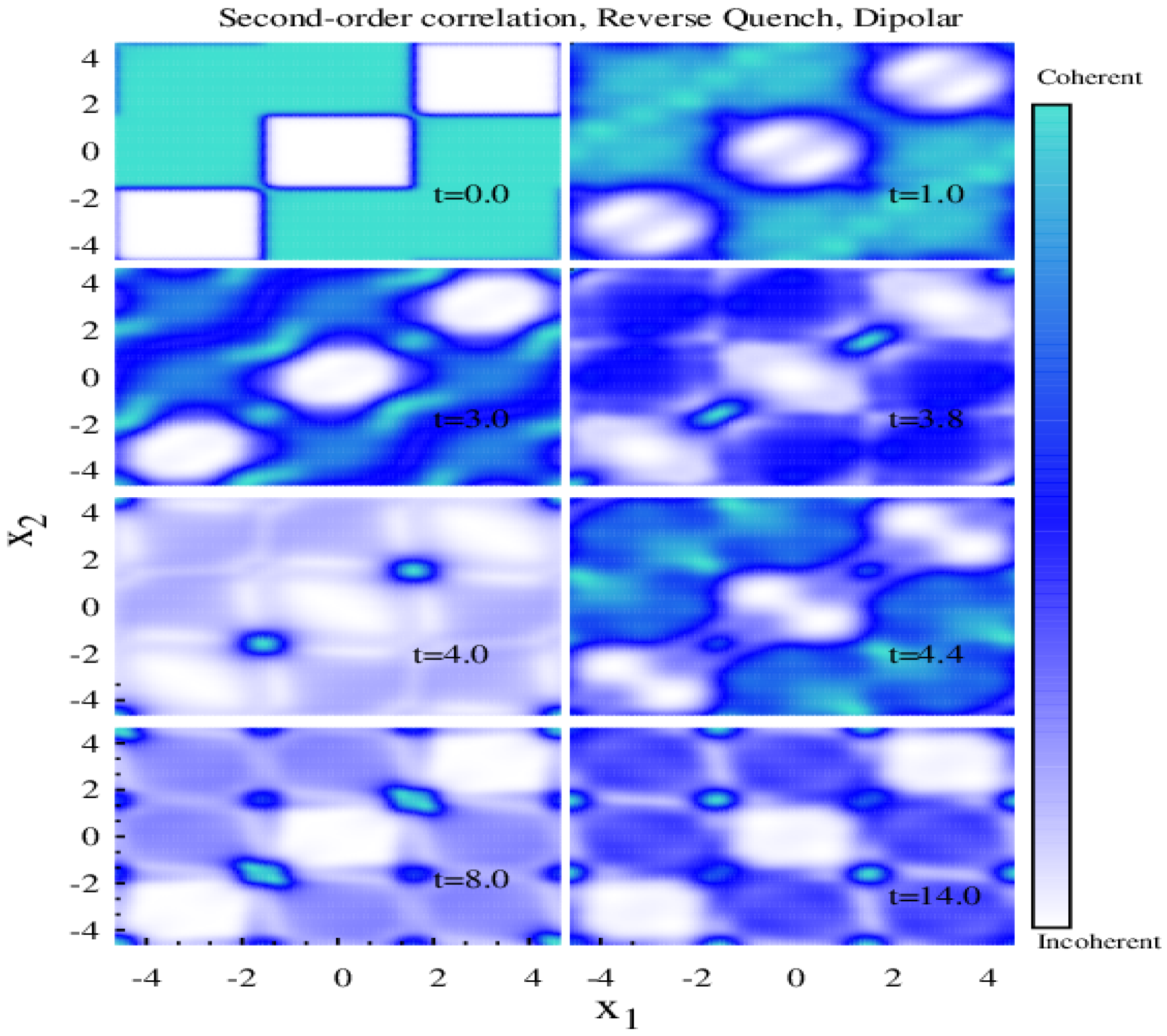
- (a)
- The system revives to Phase.
- (b)
- Information entropy reaches its minimum value.
- (c)
- The system becomes fully coherent.
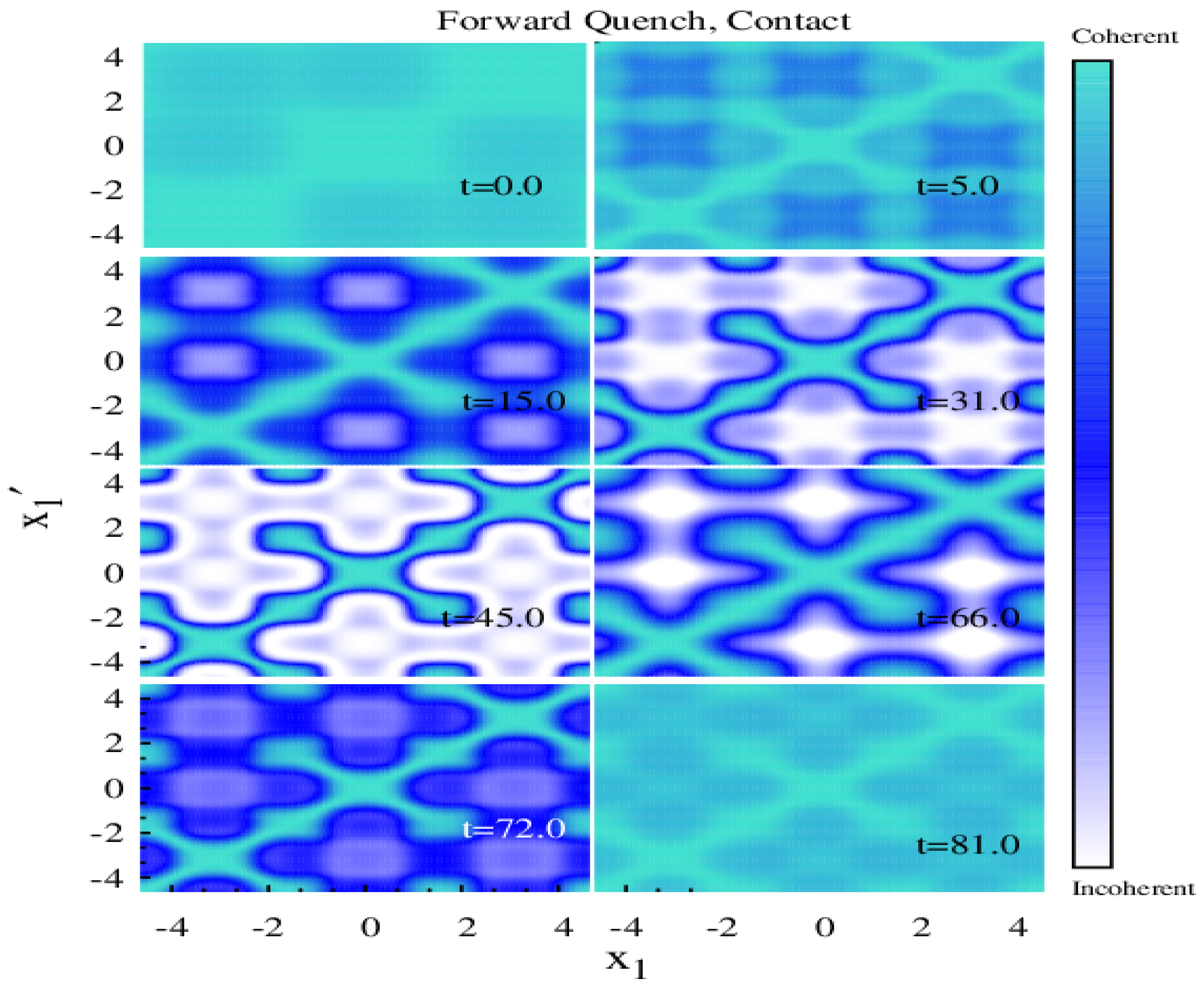
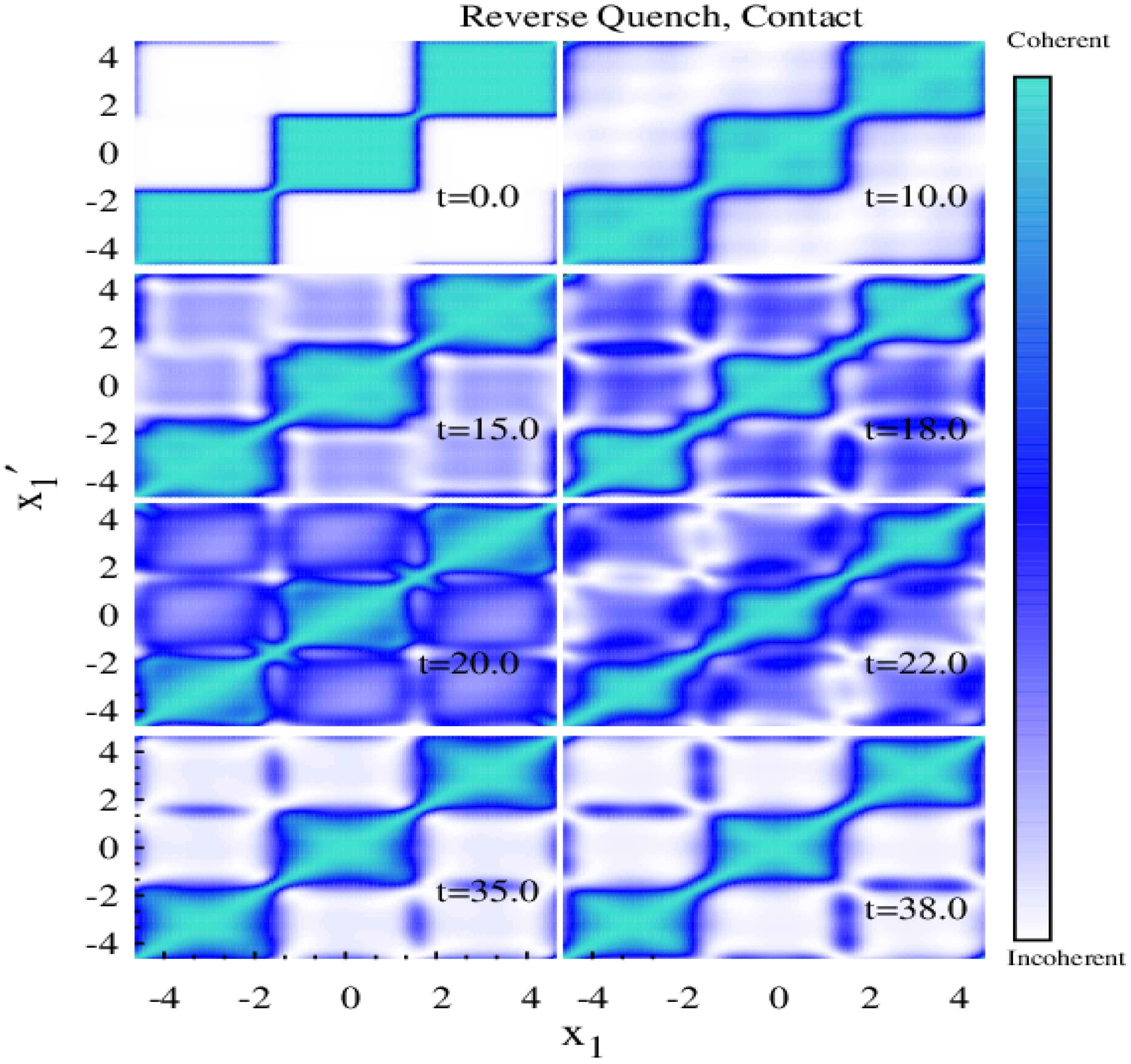
4.2. Reverse Quench ( to
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bakr, W.S.; Peng, A.; Tai, M.E.; Ma, R.; Simon, J.; Gillen, J.; Foelling, S.; Pollet, L.; Greiner, M. Probing the Superfluid to Mott Insulator Transition at the Single Atom Level. Science 2010, 329, 547. [Google Scholar] [CrossRef] [PubMed]
- Hung, C.-L.; Zhang, X.; Ha, L.-C.; Tung, S.-K.; Gemelke, N.; Chin, C. Extracting density-density correlations from in situ images of atomic quantum gases. New. J. Phys. 2011, 13, 075019. [Google Scholar] [CrossRef]
- Trotzky, S.; Chen, Y.-A.; Flesch, A.; McCulloch, I.P.; Schollwöck, U.; Eisert, J.; Bloch, I. Probing the relaxation towards equilibrium in an isolated strongly correlated one-dimensional Bose gas. Nat. Phys. 2012, 8, 325. [Google Scholar] [CrossRef]
- Cheneau, M.; Barmettler, P.; Poletti, D.; Endres, M.; Schaua, P.; Fukuhara, T.; Gross, C.; Bloch, I.; Kollath, C.; Kuhr, S. Light-cone-like spreading of correlations in a quantum many-body system. Nature 2012, 481, 484. [Google Scholar] [CrossRef] [PubMed]
- Kinoshita, T.; Wenger, T.; Weiss, D.S. A quantum Newton’s cradle. Nature 2006, 440, 900. [Google Scholar] [CrossRef] [PubMed]
- Greiner, M.; Mandel, O.; Esslinger, T.; Hänsch, T.W.; Bloch, I. Quantum phase transition from a superfluid to a Mott insulator in a gas of ultracold atoms. Nature 2002, 415, 39. [Google Scholar] [CrossRef] [PubMed]
- Greiner, M.; Mandel, O.; Hänsch, T.W.; Bloch, I. Collapse and revival of the matter wave field of a Bose Einstein condensate. Nature 2002, 419, 51. [Google Scholar] [CrossRef] [PubMed]
- Will, S.; Best, T.; Schneider, U.; Hackermuller, L.; Luhmann, D.; Bloch, I. Time-resolved observation of coherent multi-body interactions in quantum phase revivals. Nature 2010, 465, 197. [Google Scholar] [CrossRef]
- Langen, T.; Geiger, R.; Kuhnert, M.; Rauer, B.; Schmiedmayer, J. Local emergence of thermal correlations in an isolated quantum many-body system. Nat. Phys. 2013, 9, 640. [Google Scholar] [CrossRef]
- Nagao, K.; Kunimi, M.; Takasu, Y.; Takahashi, Y.; Danshita, I. Semiclassical quench dynamics of Bose gases in optical lattices. Phys. Rev. A 2019, 99, 023622. [Google Scholar] [CrossRef]
- Lacki, M.; Heyl, M. Dynamical quantum phase transitions in collapse and revival oscillations of a quenched superfluid. Phys. Rev. B 2019, 99, 121107. [Google Scholar] [CrossRef]
- Miyake, H.; Siviloglou, G.A.; Puentes, G.; Pritchard, D.E.; Ketterle, W.; Weld, D.M. Bragg Scattering as a Probe of Atomic Wave Functions and Quantum Phase Transitions in Optical Lattices. Phys. Rev. Lett. 2011, 107, 175302. [Google Scholar] [CrossRef] [PubMed]
- Natu, S.S.; McKay, D.C.; DeMarco, B.; Mueller, E.J. Evolution of condensate fraction during rapid lattice ramps. Phys. Rev. A 2012, 85, 061601. [Google Scholar] [CrossRef]
- Hung, C.-L.; Gurarie, V.; Chin, C. From Cosmology to Cold Atoms: Observation of Sakharov Oscillations in a Quenched Atomic Superfluid. Science 2013, 341, 1213. [Google Scholar] [CrossRef] [PubMed]
- Gring, M.; Kuhnert, M.; Langen, T.; Kitagawa, T.; Rauer, B.; Schreitl, M.; Mazets, I.; Smith, D.A.; Demler, E.; Schmiedmayer, J. Relaxation and Prethermalization in an Isolated Quantum System. Science 2012, 337, 1318. [Google Scholar] [CrossRef] [PubMed]
- Fischer, U.R.; Schutzhold, R.; Uhlmann, M. Bogoliubov theory of quantum correlations in the time-dependent Bose-Hubbard model. Phys. Rev. A 2008, 77, 043615. [Google Scholar] [CrossRef]
- Lahaye, T.; Menotti, C.; Santos, L.; Lewenstein, M.; Pfau, T. The physics of dipolar bosonic quantum gases. Rep. Prog. Phys. 2009, 72, 126401. [Google Scholar] [CrossRef]
- Cevolani, L.; Carleo, G.; Sanchez-Palencia, L. Protected quasilocality in quantum systems with long-range interactions. Phys. Rev. A 2015, 92, 041603. [Google Scholar] [CrossRef]
- Baranov, M.A. Theoretical progress in many-body physics with ultracold dipolar gases. Phys. Rep. 2008, 464, 71. [Google Scholar] [CrossRef]
- Cazalilla, M.; Citro, R.; Giamarchi, T.; Orignac, E.; Rigol, M. One dimensional bosons: From condensed matter systems to ultracold gases. Rev. Mod. Phys. 2011, 83. [Google Scholar] [CrossRef]
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885. [Google Scholar] [CrossRef]
- Langen, T. Non-Equilibrium Dynamics of One-Dimensional Bose Gases. Ph.D. Thesis, Vienna University of Technology, Vienna, Austria, 2013. [Google Scholar]
- Zöllner, S.; Bruun, G.M.; Pethick, C.J.; Reimann, S.M. Bosonic and Fermionic Dipoles on a Ring. Phys. Rev. Lett. 2011, 107, 035301. [Google Scholar] [CrossRef] [PubMed]
- Astrakharchik, G.E.; Morigi, G.E.; de Chiara, G.; Boronat, J. Ground state of low-dimensional dipolar gases: Linear and zigzag chains. Phys. Rev. A 2008, 78, 063622. [Google Scholar] [CrossRef]
- Astrakharchik, G.E.; Lozovik, Y.E. Super-Tonks-Girardeau regime in trapped one-dimensional dipolar gases. Phys. Rev. A 2008, 77, 013404. [Google Scholar] [CrossRef]
- Deuretzbacher, F.; Cremon, J.C.; Reimann, S.M. Ground-state properties of few dipolar bosons in a quasi-one-dimensional harmonic trap. Phys. Rev. A 2010, 81, 063616. [Google Scholar] [CrossRef]
- Arkhipov, A.S.; Astrakharchik, G.E.; Belikov, A.V.; Lozovik, Y.E. Ground-state properties of a one-dimensional system of dipoles. JETP Lett. 2005, 82, 39. [Google Scholar] [CrossRef]
- Imambekov, A.; Mazets, I.E.; Petrov, D.S.; Gritsev, V.; Manz, S.; Hofferberth, S.; Schumm, T.; Demler, E.; Schmiedmayer, J. Density ripples in expanding low-dimensional gases as a probe of correlations. Phys. Rev. A 2009, 80, 033604. [Google Scholar] [CrossRef]
- Peter, D.; Pawlowski, K.; Pfau, T.; Rza̧żewski, K. Mean-field description of dipolar bosons in triple-well potentials. J. Phys. B 2012, 45, 225302. [Google Scholar] [CrossRef][Green Version]
- Lahaye, T.; Pfau, T.; Santos, L. Mesoscopic Ensembles of Polar Bosons in Triple-Well Potentials. Phys. Rev. Lett. 2010, 104, 170404. [Google Scholar] [CrossRef] [PubMed]
- Dell’Anna, L.; Mazzarella, G.; Penna, V.; Salasnich, L. Entanglement entropy and macroscopic quantum states with dipolar bosons in a triple-well potential. Phys. Rev. A 2013, 87, 053620. [Google Scholar] [CrossRef]
- Xiong, B.; Fischer, U.R. Interaction-induced coherence among polar bosons stored in triple-well potentials. Phys. Rev. A 2013, 88, 063608. [Google Scholar] [CrossRef]
- Gallemi, A.; Guilleumas, M.; Mayol, R.; Sanpera, A. Role of anisotropy in dipolar bosons in triple-well potentials. Phys. Rev. A 2013, 88, 063645. [Google Scholar] [CrossRef]
- Gallemi, A.; Queralto, G.; Guilleumas, M.; Mayol, R.; Sanpera, A. Quantum spin models with mesoscopic Bose-Einstein condensates. Phys. Rev. A 2016, 94, 063626. [Google Scholar] [CrossRef]
- Biedroń, K.; Lacki, M.; Zakrzewski, J. Extended Bose-Hubbard model with dipolar and contact interactions. Phys. Rev. B 2018, 97, 245102. [Google Scholar] [CrossRef]
- Chatterjee, B.; Lode, A.U.J. Order parameter and detection for a finite ensemble of crystallized one-dimensional dipolar bosons in optical lattices. Phys. Rev. A 2018, 98, 053624. [Google Scholar] [CrossRef]
- Alon, O.E.; Streltsov, A.I.; Cederbaum, L.S. Multiconfigurational time-dependent Hartree method for bosons: Many-body dynamics of bosonic systems. Phys. Rev. A 2008, 77, 033613. [Google Scholar] [CrossRef]
- Alon, O.E.; Streltsov, A.I.; Cederbaum, L.S. Unified view on multiconfigurational time propagation for systems consisting of identical particles. J. Chem. Phys. 2007, 127, 154103. [Google Scholar] [CrossRef] [PubMed]
- Streltsov, A.I.; Alon, O.E.; Cederbaum, L.S. Role of Excited States in the Splitting of a Trapped Interacting Bose-Einstein Condensate by a Time-Dependent Barrier. Phys. Rev. Lett. 2007, 99, 030402. [Google Scholar] [CrossRef]
- Fasshauer, E.; Lode, A.U.J. Multiconfigurational time-dependent Hartree method for fermions: Implementation, exactness, and few-fermion tunneling to open space. Phys. Rev. A 2016, 93, 033635. [Google Scholar] [CrossRef]
- Lode, A.U.J. Multiconfigurational time-dependent Hartree method for bosons with internal degrees of freedom: Theory and composite fragmentation of multicomponent Bose-Einstein condensates. Phys. Rev. A 2016, 93, 063601. [Google Scholar] [CrossRef]
- Lode, A.U.J.; Tsatsos, M.C.; Fasshauer, E.; Lin, R.; Papariello, L.; Molignini, P.; Lévêque, C. MCTDH-X: The Time-Dependent Multiconfigurational Hartree for Indistinguishable Particles Software. 2018. Available online: http://ultracold.org (accessed on 1 June 2019).
- Neuhaus-Steinmetz, J.; Mistakidis, S.I.; Schmelcher, P. Quantum dynamical response of ultracold few-boson ensembles in finite optical lattices to multiple interaction quenches. Phys. Rev. A 2017, 95, 053610. [Google Scholar] [CrossRef]
- Koutentakis, G.M.; Mistakidis, S.I.; Schmelcher, P. Quench-induced resonant tunneling mechanisms of bosons in an optical lattice with harmonic confinement. Phys. Rev. A 2017, 95, 013617. [Google Scholar] [CrossRef]
- Mistakidis, S.I.; Schmelcher, P. Mode coupling of interaction quenched ultracold few-boson ensembles in periodically driven lattices. Phys. Rev. A 2017, 95, 013625. [Google Scholar] [CrossRef]
- Mistakidis, S.I.; Cao, L.; Schmelcher, P. Interaction quench induced multimode dynamics of finite atomic ensembles. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 225303. [Google Scholar] [CrossRef]
- Mistakidis, S.I.; Wulf, T.; Negretti, A.; Schmelcher, P. Resonant quantum dynamics of few ultracold bosons in periodically driven finite lattices. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 244004. [Google Scholar] [CrossRef]
- Alon, O.E.; Streltsov, A.I.; Cederbaum, L.S. Multiorbital mean-field approach for bosons, spinor bosons, and Bose-Bose and Bose-Fermi mixtures in real-space optical lattices. Phys. Rev. A 2007, 76, 013611. [Google Scholar] [CrossRef]
- Plaßmann, T.; Mistakidis, S.I.; Schmelcher, P. Quench dynamics of finite bosonic ensembles in optical lattices with spatially modulated interactions. J. Phys. B At. Mol. Opt. Phys. 2018, 51, 225001. [Google Scholar] [CrossRef]
- Mistakidis, S.I.; Cao, L.; Schmelcher, P. Negative-quench-induced excitation dynamics for ultracold bosons in one-dimensional lattices. Phys. Rev. A 2015, 91, 033611. [Google Scholar] [CrossRef]
- Nguyen, H.V.; Tsatsos, M.C.; Luo, D.; Lode, A.U.J.; Telles, G.D.; Bagnato, V.S.; Hulet, R.G. Parametric Excitation of a Bose-Einstein Condensate: From Faraday Waves to Granulation. Phys. Rev. X 2019, 9, 011052. [Google Scholar] [CrossRef]
- Lode, A.U.J.; Chakrabarti, B.; Kota, V.K.B. Many-body entropies, correlations, and emergence of statistical relaxation in interaction quench dynamics of ultracold bosons. Phys. Rev. A 2015, 92, 033622. [Google Scholar] [CrossRef]
- Roy, R.; Gammal, A.; Tsatsos, M.C.; Chatterjee, B.; Chakrabarti, B.; Lode, A.U.J. Phases, many-body entropy measures, and coherence of interacting bosons in optical lattices. Phys. Rev. A 2018, 97, 043625. [Google Scholar] [CrossRef]
- Bera, S.; Chakrabarti, B.; Gammal, A.; Tsatsos, M.C.; Lekala, M.L.; Chatterjee, B.; Lévêque, C.; Lode, A.U.J. Sorting Fermionization from Crystallization in Many-Boson Wavefunctions. arXiv 2018, arXiv:1806.02539. [Google Scholar]
- Zhou, T.; Yang, K.; Zhu, Z.; Yu, X.; Yang, S.; Xiong, W.; Zhou, X.; Chen, X. Observation of atom-number fluctuations in optical lattices via quantum collapse and revival dynamics. Phys. Rev. A 2019, 99, 013602. [Google Scholar] [CrossRef]
- Sakmann, K.; Streltsov, A.I.; Alon, O.E.; Cederbaum, L.S. Reduced density matrices and coherence of trapped interacting bosons. Phys. Rev. A 2008, 78, 023615. [Google Scholar] [CrossRef]
- Glauber, R.J. The quantum theory of optical coherence. Phys. Rev. 1963, 130, 2529. [Google Scholar] [CrossRef]
- Massen, S.E.; Moustakidis, C.C.; Panos, C.P. Comparison of the information entropy in fermionic and bosonic systems. Phys. Lett. A 2002, 299, 131. [Google Scholar] [CrossRef]
- Haldar, S.K.; Chakrabarti, B.; Das, T.K.; Biswas, A. Correlated many-body calculation to study characteristics of Shannon information entropy for ultracold trapped interacting bosons. Phys. Rev. A 2013, 88, 033602. [Google Scholar] [CrossRef]
- Olshanii, M. Atomic Scattering in the Presence of an External Confinement and a Gas of Impenetrable Bosons. Phys. Rev. Lett. 1998, 81, 938. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bera, S.; Salasnich, L.; Chakrabarti, B. Correlation Dynamics of Dipolar Bosons in 1D Triple Well Optical Lattice. Symmetry 2019, 11, 909. https://doi.org/10.3390/sym11070909
Bera S, Salasnich L, Chakrabarti B. Correlation Dynamics of Dipolar Bosons in 1D Triple Well Optical Lattice. Symmetry. 2019; 11(7):909. https://doi.org/10.3390/sym11070909
Chicago/Turabian StyleBera, Sangita, Luca Salasnich, and Barnali Chakrabarti. 2019. "Correlation Dynamics of Dipolar Bosons in 1D Triple Well Optical Lattice" Symmetry 11, no. 7: 909. https://doi.org/10.3390/sym11070909
APA StyleBera, S., Salasnich, L., & Chakrabarti, B. (2019). Correlation Dynamics of Dipolar Bosons in 1D Triple Well Optical Lattice. Symmetry, 11(7), 909. https://doi.org/10.3390/sym11070909




