Charged Pion Condensation in Dense Quark Matter: Nambu–Jona-Lasinio Model Study
Abstract
1. Introduction
2. Charged Pion Condensation in the Framework of NJL Model
2.1. The Model and Its Thermodynamic Potential
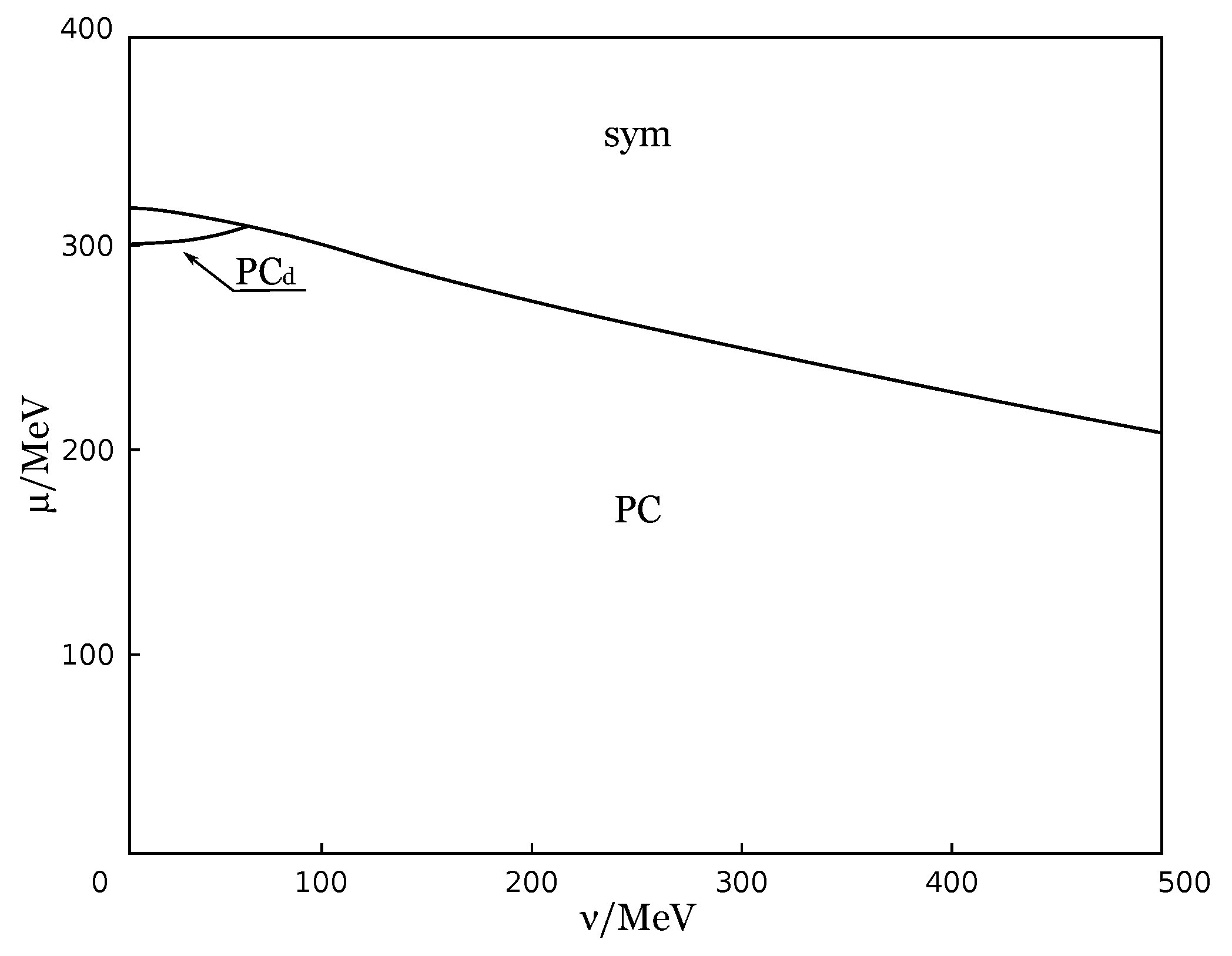
2.2. Phase Diagram at and in the Chiral Limit
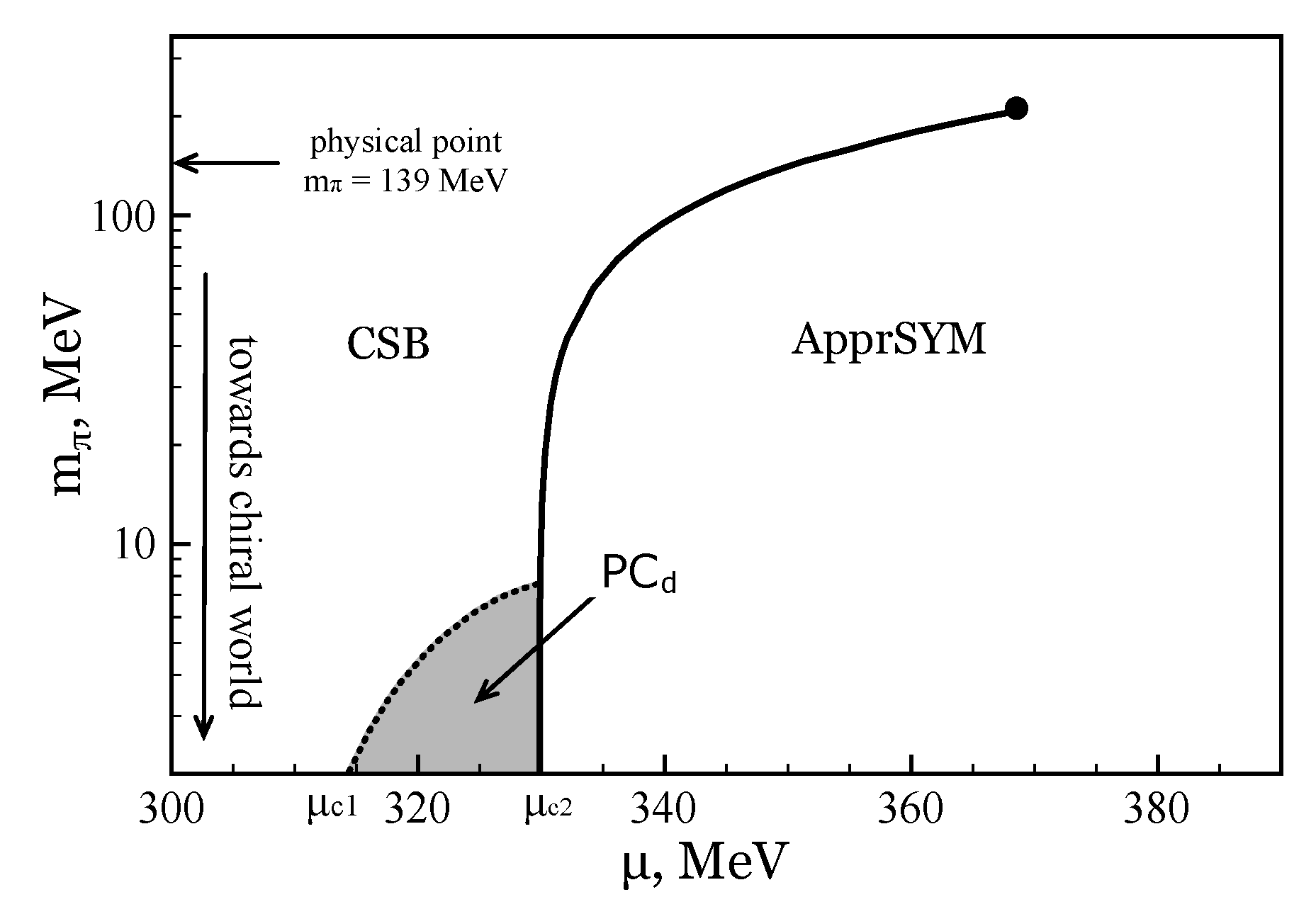
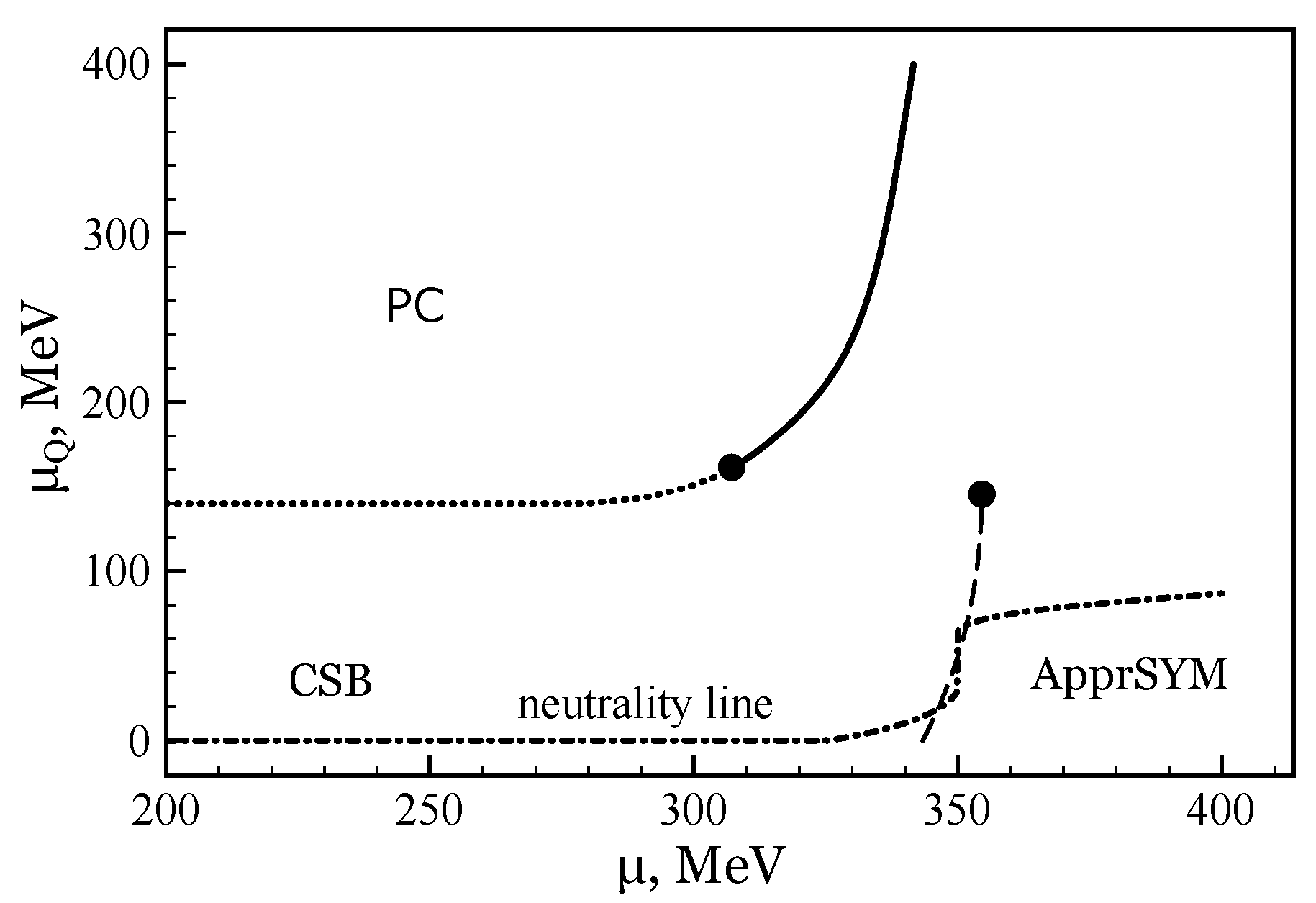
2.3. Account of Electric Neutrality and -Equilibrium Conditions in the Chiral Limit
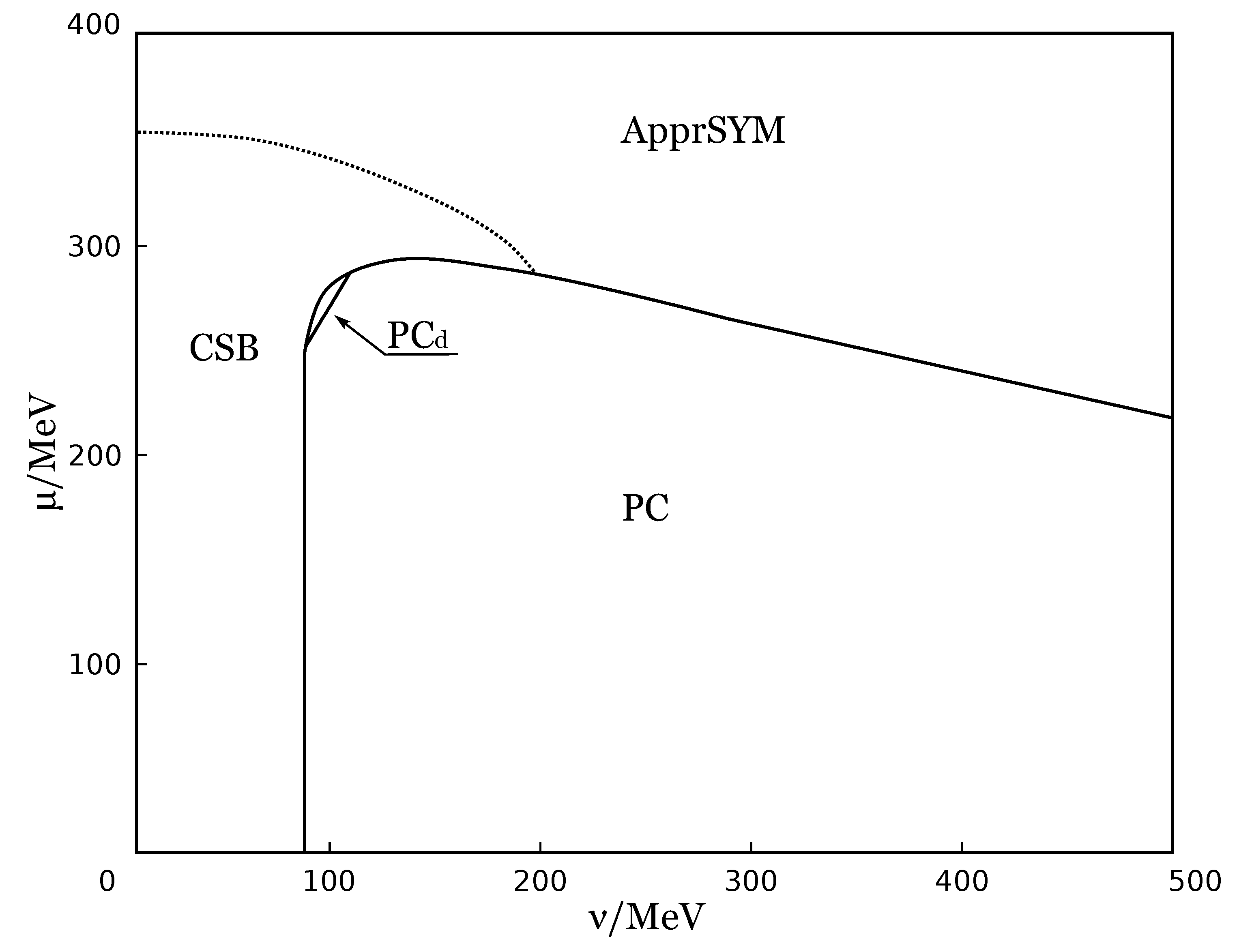
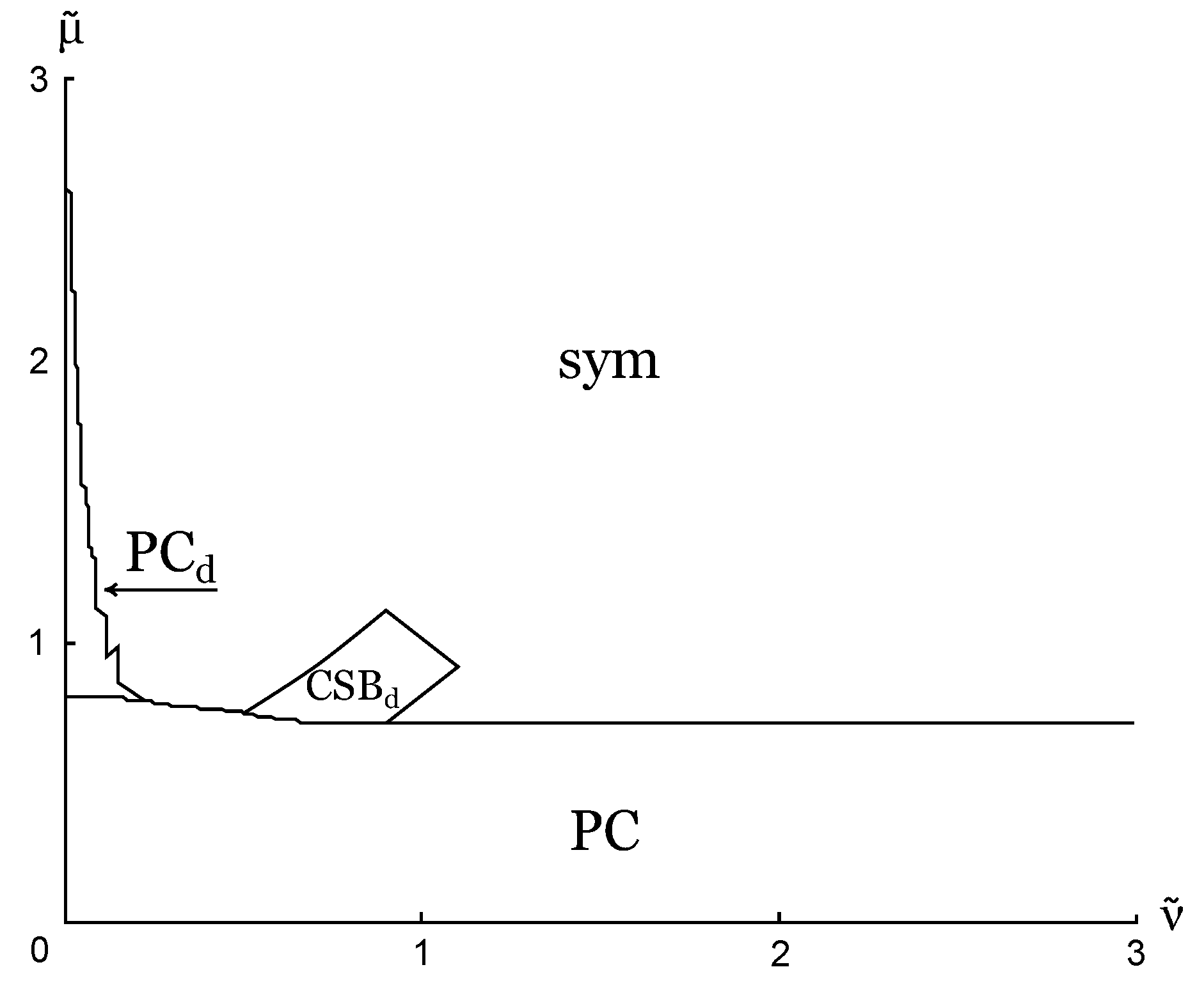
2.4. Phase Diagram at and at the Physical Point ()
3. Conditions Promoting the Appearance of Charged Pion Condensation in Dense Quark Matter
3.1. The NJL2 Model and Its Phase Structure
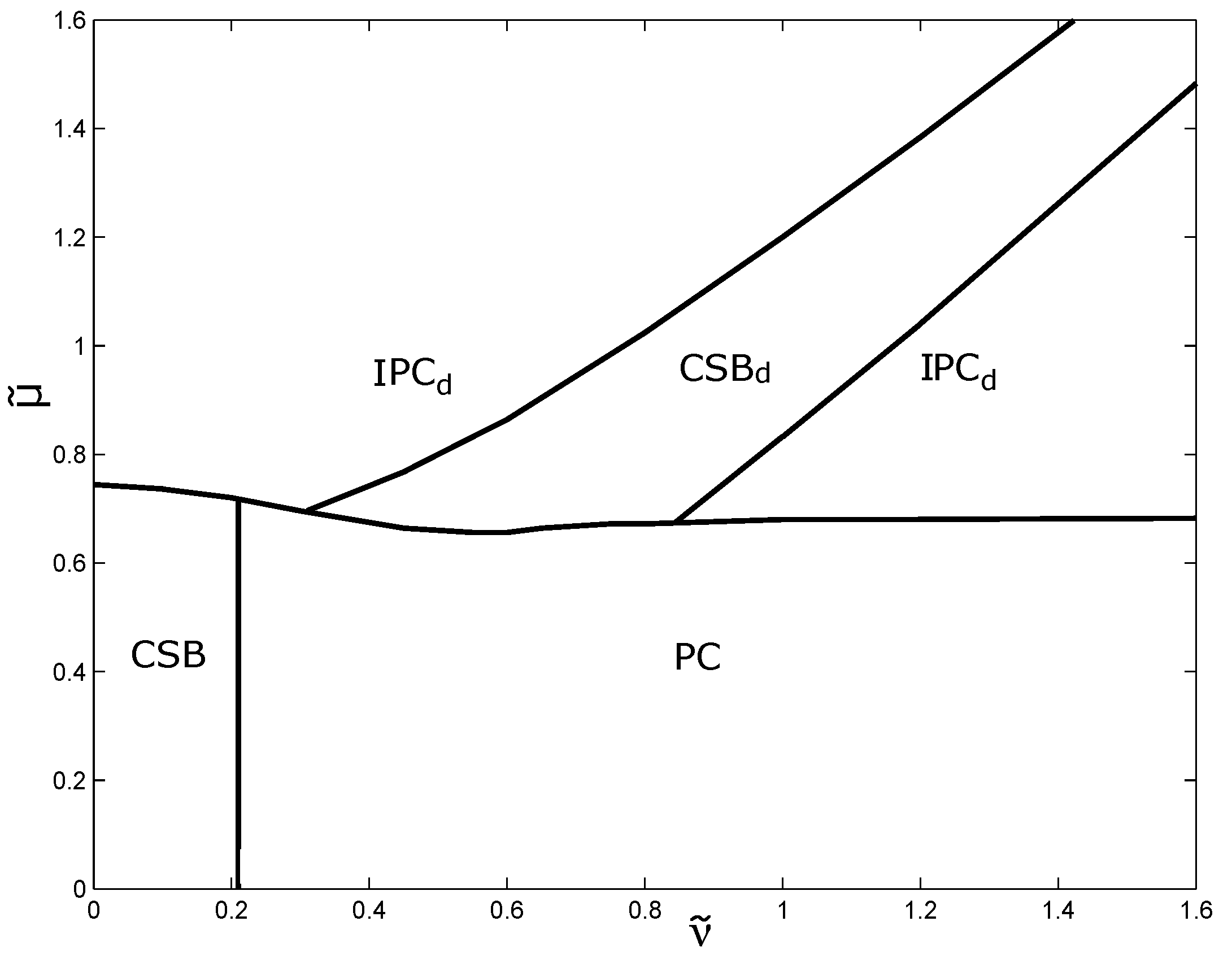
3.2. Finite Size Effect and Nontrivial Topology
3.3. Inhomogeneous Pion Condensation in Dense Baryonic Matter
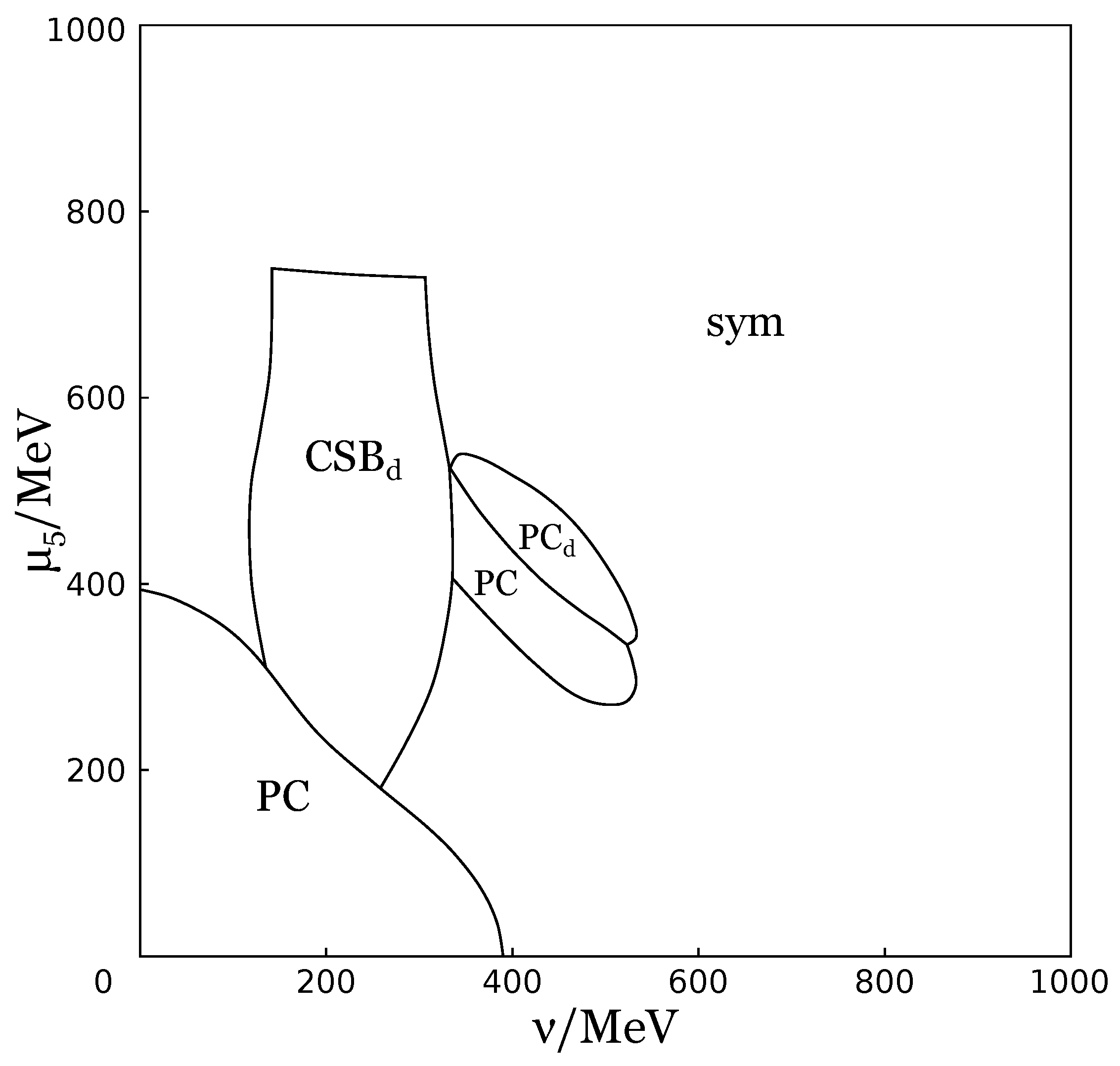
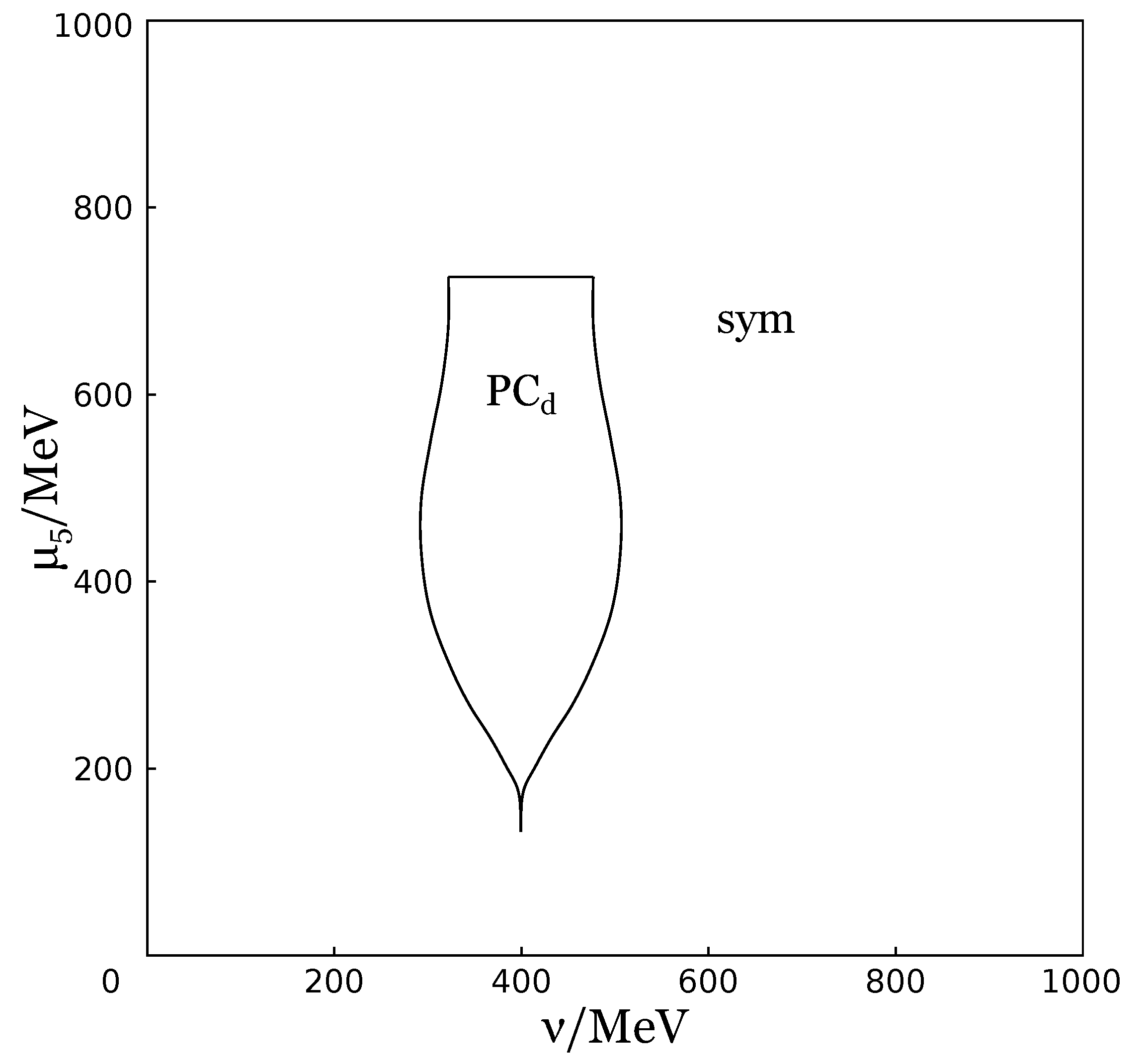
3.4. Chiral Imbalanced Dense Quark Matter
4. Summary and Conclusions
- (1)
- finite size effects and nontrivial topology,
- (2)
- possibility of inhomogeneous charged pion condensates,
- (3)
- chiral imbalance.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Shuryak, E.V. Quantum Chromodynamics and the Theory of Superdense Matter. Phys. Rept. 1980, 61, 71–158. [Google Scholar] [CrossRef]
- Schäfer, T. Quark matter. arXiv 2003, arXiv:hep-ph/0304281. [Google Scholar]
- Stone, J.R. High Density Matter. EPJ Web Conf. 2013, 63, 03009. [Google Scholar] [CrossRef]
- Fukushima, K.; Sasaki, C. The phase diagram of nuclear and quark matter at high baryon density. Prog. Part. Nucl. Phys. 2013, 72, 99–154. [Google Scholar] [CrossRef]
- Shuryak, E.V. The QCD vacuum, hadrons and the superdense matter. World Sci. Lect. Notes Phys. 2004, 71, 1–618. [Google Scholar]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I. Phys. Rev. 1961, 112, 345. [Google Scholar] [CrossRef]
- Hatsuda, T.; Kunihiro, T. QCD phenomenology based on a chiral effective Lagrangian. Phys. Rept. 1994, 247, 221–367. [Google Scholar] [CrossRef]
- Klevansky, S.P. The Nambu-Jona-Lasinio model of quantum chromodynamics. Rev. Mod. Phys. 1992, 64, 649. [Google Scholar] [CrossRef]
- Buballa, M. NJL model analysis of quark matter at large density. Phys. Rept. 2005, 407, 205–376. [Google Scholar] [CrossRef]
- Ebert, D.; Kalinovsky, Y.L.; Münchow, L.; Volkov, M.K. Mesons and diquarks in a NJL model at finite temperature and chemical potential. Int. J. Mod. Phys. 1993, A8, 1295. [Google Scholar] [CrossRef]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455. [Google Scholar] [CrossRef]
- Shovkovy, I.A. Two lectures on color superconductivity. Found. Phys. 2005, 35, 1309–1358. [Google Scholar] [CrossRef]
- Ebert, D.; Klimenko, K.G. Gapless pion condensation in quark matter with finite baryon density. J. Phys. G 2006, 32, 599. [Google Scholar] [CrossRef][Green Version]
- Ebert, D.; Klimenko, K.G. Pion condensation in electrically neutral cold matter with finite baryon density. Eur. Phys. J. C 2006, 46, 771–776. [Google Scholar] [CrossRef][Green Version]
- He, L.; Jin, M.; Zhuang, P. Effect of U(A)(1) breaking on chiral phase structure and pion superfluidity at finite isospin chemical potential. Mod. Phys. Lett. A 2007, 22, 637–643. [Google Scholar] [CrossRef]
- He, L.; Jin, M.; Zhuang, P. Pion superfluidity and meson properties at finite isospin density. Phys. Rev. D 2005, 71, 116001. [Google Scholar] [CrossRef]
- He, L.; Zhuang, P. Phase structure of Nambu-Jona-Lasinio model at finite isospin density. Phys. Lett. B 2005, 615, 93–101. [Google Scholar] [CrossRef]
- Barducci, A.; Casalbuoni, R.; Pettini, G.; Ravagli, L. A Calculation of the QCD phase diagram at finite temperature, and baryon and isospin chemical potentials. Phys. Rev. D 2004, 69, 096004. [Google Scholar] [CrossRef]
- Loewe, M.; Villavicencio, C. Two-flavor condensates in chiral dynamics: Temperature and isospin density effects. Phys. Rev. D 2005, 71, 094001. [Google Scholar] [CrossRef]
- Splittorff, K.; Son, D.T.; Stephanov, M.A. QCD—Like theories at finite baryon and isospin density. Phys. Rev. D 2001, 64, 016003. [Google Scholar] [CrossRef]
- Son, D.T.; Stephanov, M.A. QCD at finite isospin density. Phys. Rev. Lett. 2001, 86, 592. [Google Scholar] [CrossRef] [PubMed]
- Andersen, J.O.; Kyllingstad, L.T. Pion Condensation in a two-flavor NJL model: The role of charge neutrality. J. Phys. G 2009, 37, 015003. [Google Scholar] [CrossRef]
- Kogut, J.B.; Sinclair, D.K. Quenched lattice QCD at finite isospin density and related theories. Phys. Rev. D 2002, 66, 014508. [Google Scholar] [CrossRef]
- Gupta, S. Critical behavior in QCD at finite isovector chemical potential. arXiv 2002, arXiv:hep-lat/0202005. [Google Scholar]
- Abuki, H.; Anglani, R.; Gatto, R.; Pellicoro, M.; Ruggieri, M. The Fate of pion condensation in quark matter: From the chiral to the real world. Phys. Rev. D 2009, 79, 034032. [Google Scholar] [CrossRef]
- Anglani, R. A Microscopic study of pion condensation within Nambu-Jona-Lasinio model. Acta Phys. Pol. Suppl. 2010, 3, 735. [Google Scholar]
- Abuki, H.; Ciminale, M.; Gatto, R.; Ippolito, N.D.; Nardulli, G.; Ruggieri, M. Electrical neutrality and pion modes in the two flavor PNJL model. Phys. Rev. D 2008, 78, 014002. [Google Scholar] [CrossRef]
- Abuki, H.; Anglani, R.; Gatto, R.; Nardulli, G.; Ruggieri, M. Chiral crossover, deconfinement and quarkyonic matter within a Nambu-Jona Lasinio model with the Polyakov loop. Phys. Rev. D 2008, 78, 034034. [Google Scholar] [CrossRef]
- Ebert, D.; Klimenko, K.G. Properties of the massive Gross-Neveu model with nonzero baryon and isospin chemical potentials. Phys. Rev. D 2009, 80, 125013. [Google Scholar] [CrossRef]
- Migdal, A.B. Stability of Vacuum and Limiting Fields. Zh. Eksp. Teor. Fiz. 1971, 61, 2210. [Google Scholar] [CrossRef]
- Migdal, A.B. Phase Transition in Nuclear Matter and Non-pair Nuclear Forces. Sov. Phys. JETP 1973, 36, 1052. [Google Scholar]
- Migdal, A.B.; Saperstein, E.E.; Troitsky, M.A.; Voskresensky, D.N. Pion degrees of freedom in nuclear matter. Phys. Rept. 1990, 192, 179. [Google Scholar] [CrossRef]
- Yakovlev, D.G.; Levenfish, K.P.; Shibanov, Y.A. Cooling neutron stars and superfluidity in their interiors. Phys. Usp. 1999, 42, 737. [Google Scholar] [CrossRef]
- Tatsumi, T. Alternating Layer Spin Structure Realized In The Sigma Model. Prog. Theor. Phys. 1980, 63, 1252–1267. [Google Scholar] [CrossRef]
- Tatsumi, T. A Realistic Approach To Charged Pion Condensation In Neutron Matter. Prog. Theor. Phys. 1982, 68, 1231–1247. [Google Scholar] [CrossRef]
- Tatsumi, T. A Realistic Calculation of the Cooling Rate of Neutron Stars with the Charged Pion Condensate. Prog. Theor. Phys. 1983, 69, 1137–1145. [Google Scholar] [CrossRef][Green Version]
- Takatsuka, T.; Tamagaki, R. Nucleon superfluidity under pion condensation in neutron star matter. Prog. Theor. Phys. Suppl. 1993, 112, 107–122. [Google Scholar] [CrossRef]
- Takatsuka, T.; Tamagaki, R. Effects of charged-pion condensation on neutron 3P2 superfluidity. Prog. Theor. Phys. 1997, 97, 263–281. [Google Scholar] [CrossRef]
- Takatsuka, T.; Tamagaki, R.; Tatsumi, T. Characteristic aspects of pion condensed phases. Prog. Theor. Phys. Suppl. 1993, 112, 67–106. [Google Scholar] [CrossRef]
- Suzuki, T.; Tatsumi, T.; Sakai, H. The Landau-Migdal Parameters, G-prime(N N) and G-prime(N Delta) and Pion Condensations. arXiv 1999, arXiv:nucl-Th/9901097. [Google Scholar]
- Ohnishi, A.; Jido, D.; Sekihara, T.; Tsubakihara, K. Possibility of s-wave pion condensates in neutron stars revisited. Phys. Rev. C 2009, 80, 038202. [Google Scholar] [CrossRef]
- Ericson, T.E.O.; Weise, W. Pions and Nuclei; Oxford University Press: Oxford, UK, 1988. [Google Scholar]
- Wakasa, T.; Sakai, H.; Okamura, H.; Otsu, H.; Fujita, S.; Ishida, S.; Sakamoto, N.; Uesaka, S.; Satou, N.; Greenfield, M.B.; et al. Gamow-Teller strength of Nb-90 in the continuum studied via multipole decomposition analysis of the Zr-90 (p, n) reaction at 295 MeV. Phys. Rev. C 1997, 55, 2909. [Google Scholar] [CrossRef]
- Suzuki, T.; Sakai, H. The Landau-Migdal parameters, g’(NN) and g’(N Delta). Phys. Lett. B 1999, 455, 25. [Google Scholar] [CrossRef]
- Ebert, D.; Khunjua, T.G.; Klimenko, K.G.; Zhukovsky, V.C. Charged pion condensation phenomenon of dense baryonic matter induced by finite volume: The NJL(2) model consideration. Int. J. Mod. Phys. A 2012, 27, 1250162. [Google Scholar] [CrossRef]
- Gubina, N.V.; Klimenko, K.G.; Kurbanov, S.G.; Zhukovsky, V.C. Inhomogeneous charged pion condensation phenomenon in the NJL2 model with quark number and isospin chemical potentials. Phys. Rev. D 2012, 86, 085011. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov, R.N.; Zhukovsky, V.C. Inhomogeneous charged pion condensation in chiral asymmetric dense quark matter in the framework of NJL2 model. Phys. Rev. D 2017, 95, 105010. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov-Larionov, R.N. Affinity of NJL2 and NJL4 model results on duality and pion condensation in chiral asymmetric dense quark matter. EPJ Web Conf. 2018, 191, 05016. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Zhukovsky, V.C.; Klimenko, K.G.; Zhokhov, R.N. Duality and Charged Pion Condensation in Chirally Asymmetric Dense Quark Matter in the Framework of an NJL2 Model. Int. J. Mod. Phys. Conf. Ser. 2018, 47, 1860093. [Google Scholar] [CrossRef]
- Ebert, D.; Khunjua, T.G.; Klimenko, K.G. Duality between chiral symmetry breaking and charged pion condensation at large Nc: Consideration of an NJL2 model with baryon, isospin, and chiral isospin chemical potentials. Phys. Rev. D 2016, 94, 116016. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov, R.N. Dense baryon matter with isospin and chiral imbalance in the framework of NJL4 model at large Nc: Duality between chiral symmetry breaking and charged pion condensation. Phys. Rev. D 2018, 97, 054036. [Google Scholar] [CrossRef]
- Khunjua, T.; Klimenko, K.; Zhokhov, R. Dense quark matter with chiral and isospin imbalance: NJL-model consideration. EPJ Web Conf. 2018, 191, 05015. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov, R.N. Dualities in dense quark matter with isospin, chiral, and chiral isospin imbalance in the framework of the large-Nc limit of the NJL4 model. Phys. Rev. D 2018, 98, 054030. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov, R.N. Chiral imbalanced hot and dense quark matter: NJL analysis at the physical point and comparison with lattice QCD. Eur. Phys. J. C 2019, 79, 151. [Google Scholar] [CrossRef]
- Fukushima, K.; Kharzeev, D.E.; Warringa, H.J. The Chiral Magnetic Effect. Phys. Rev. D 2008, 78, 074033. [Google Scholar] [CrossRef]
- Kharzeev, D.E.; McLerran, L.D.; Warringa, H.J. The Effects of topological charge change in heavy ion collisions: ‘Event by event P and CP violation’. Nucl. Phys. A 2008, 803, 227. [Google Scholar] [CrossRef]
- Li, Q.; Kharzeev, D.E.; Zhang, C.; Huang, Y.; Pletikosic, I.; Fedorov, A.V.; Zhong, R.D.; Schneeloch, J.A.; Gu, G.D.; Valla, T. Observation of the chiral magnetic effect in ZrTe5. Nat. Phys. 2016, 12, 550–554. [Google Scholar] [CrossRef]
- Braguta, V.V.; Katsnelson, M.I.; Kotov, A.Y.; Trunin, A.M. Catalysis of Dynamical Chiral Symmetry Breaking by Chiral Chemical Potential in Dirac semimetals. arXiv 2019, arXiv:1904.07003. [Google Scholar]
- Boyda, D.L.; Braguta, V.V.; Katsnelson, M.I.; Kotov, A.Y. Lattice Quantum Monte Carlo Study of Chiral Magnetic Effect in Dirac Semimetals. Ann. Phys. 2018, 396, 78–86. [Google Scholar] [CrossRef]
- Ebert, D.; Klimenko, K.G.; Zhukovsky, V.C.; Fedotov, A.M. Quark and pion condensation in a chromomagnetic background field. Eur. Phys. J. C 2007, 49, 709–719. [Google Scholar] [CrossRef]
- Barducci, A.; Casalbuoni, R.; Gatto, R.; Modugno, M.; Pettini, G. Thermodynamics of the massive Gross-Neveu model. Phys. Rev. D 1995, 51, 3042. [Google Scholar] [CrossRef]
- Gross, D.J.; Neveu, A. Dynamical symmetry breaking in asymptotically free field theories. Phys. Rev. D 1974, 10, 3235. [Google Scholar] [CrossRef]
- Ebert, D.; Klimenko, K.G. Pion condensation in the Gross-Neveu model with nonzero baryon and isospin chemical potentials. arXiv 2009, arXiv:0902.1861. [Google Scholar]
- Wolff, U. The phase diagram of the infinite-N Gross-Neveu model at finite temperature and chemical potential. Phys. Lett. B 1985, 157, 303. [Google Scholar] [CrossRef]
- Inagaki, T.; Kouno, T.; Muta, T. Phase Structure of Four-Fermion Theories at Finite Temperature and Chemical Potential in Arbitrary Dimensions. Int. J. Mod. Phys. A 1995, 10, 2241–2268. [Google Scholar] [CrossRef]
- Kanemura, S.; Sato, H.-T. Phase Diagram of Gross-Neveu Model at Finite Temperature, Density and Constant Curvature. Mod. Phys. Lett. A 1995, 10, 1777–1786. [Google Scholar] [CrossRef]
- Klimenko, K.G. Massive Gross-Neveu model in the leading order of the 1/N expansion. Allowance for the temperature and the chemical potential. Theor. Math. Phys. 1988, 75, 487–493. [Google Scholar] [CrossRef]
- Chodos, A.; Minakata, H.; Cooper, F.; Singh, A.; Mao, W. Two-dimensional model with chiral condensates and Cooper pairs having a QCD-like phase structure. Phys. Rev. D 2000, 61, 045011. [Google Scholar] [CrossRef]
- Ohwa, K. Crystalline ground state in chiral Gross-Neveu and Cooper pair models at finite densities. Phys. Rev. D 2002, 65, 085040. [Google Scholar] [CrossRef]
- Mermin, N.D.; Wagner, H. Absence of Ferromagnetism or Antiferromagnetism in One- or Two-Dimensional Isotropic Heisenberg Models. Phys. Rev. Lett. 1966, 17, 1133. [Google Scholar] [CrossRef]
- Coleman, S. There are no Goldstone bosons in two dimensions. Commun. Math. Phys. 1973, 31, 259. [Google Scholar] [CrossRef]
- Schon, V.; Thies, M. 2d Model Field Theories at Finite Temperature and Density. Phys. Rev. D 2000, 62, 096002. [Google Scholar]
- Brzoska, A.; Thies, M. No first-order phase transition in the Gross-Neveu model? Phys. Rev. D 2002, 65, 125001. [Google Scholar] [CrossRef]
- Wang, Q.W.; Xia, Y.; Zong, H.S. Finite volume effects with stationary wave solution from Nambu–Jona-Lasinio model. arXiv 2018, arXiv:1802.00258. [Google Scholar]
- Wang, Q.W.; Xia, Y.; Zong, H.S. Nambu-Jona-Lasinio model with proper time regularization in a finite volume. Mod. Phys. Lett. A 2018, 33, 1850232. [Google Scholar] [CrossRef]
- Palhares, L.F.; Fraga, E.S.; Kodama, T. Chiral transition in a finite system and possible use of finite size scaling in relativistic heavy ion collisions. J. Phys. G 2011, 38, 085101. [Google Scholar] [CrossRef]
- Xu, K.; Huang, M. Quantized first-order phase transition and two sets of critical end point in droplet quark matter. arXiv 2019, arXiv:1904.01154. [Google Scholar]
- Xu, K.; Huang, M. Zero-mode contribution and quantized first order phase transition in a droplet quark matter. arXiv 2019, arXiv:1903.08416. [Google Scholar]
- Inagaki, T.; Matsuo, Y.; Shimoji, H. Four-Fermion Interaction Model on MD−1⊗S1. Symmetry 2019, 11, 451. [Google Scholar] [CrossRef]
- Inagaki, T.; Muta, T.; Odintsov, S.D. Dynamical symmetry breaking in curved space-time: Four fermion interactions. Prog. Theor. Phys. Suppl. 1997, 127, 93–193. [Google Scholar] [CrossRef]
- Malbouisson, A.P.C.; Malbouisson, J.M.C.; Pereira, R.C. Boundary effects on the mass and coupling constant in the compactified Ginzburg–Landau model: The boundary dependent critical temperature. J. Math. Phys. 2009, 50, 083304. [Google Scholar] [CrossRef]
- Abreu, L.M.; Khanna, F.C.; Malbouisson, A.P.; Malbouisson, J.M.; Santana, A.E. Finite-size effects on the phase transition in a four- and six-fermion interaction model. Phys. Lett. A 2014, 378, 2597–2602. [Google Scholar] [CrossRef]
- Corrêa, E.B.; Linhares, C.A.; Malbouisson, A.P.; Malbouisson, J.M.; Santana, A.E. Finite-size, chemical-potential and magnetic effects on the phase transition in a four-fermion interacting model. Eur. Phys. J. C 2017, 77, 261. [Google Scholar] [CrossRef]
- Khanna, F.C. Thermal Quantum Field Theory—Algebraic Aspects and Applications; World Scientific: Hackensack, NJ, USA, 2009. [Google Scholar]
- Khanna, F.C.; Malbouisson, A.P.; Malbouisson, J.M.; Santana, A.E. Quantum field theory on toroidal topology: Algebraic structure and applications. Phys. Rept. 2014, 539, 135–224. [Google Scholar] [CrossRef]
- Kiriyama, O. Colour-superconducting strangelets in the Nambu-Jona-Lasinio model. Phys. Rev. D 2005, 72, 054009. [Google Scholar] [CrossRef]
- Kiriyama, O.; Hosaka, A. Chiral phase properties of finite size quark droplets in the Nambu-Jona-Lasinio model. Phys. Rev. D 2003, 67, 085010. [Google Scholar] [CrossRef]
- Grunfeld, A.G.; Lugones, G. Finite size effects in strongly interacting matter at zero chemical potential from Polyakov loop Nambu-Jona-Lasinio model in the light of lattice data. Eur. Phys. J. C 2018, 78, 640. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Ray, R.; Sur, S. Fluctuation of strongly interacting matter in the Polyakov–Nambu–Jona-Lasinio model in a finite volume. Phys. Rev. D 2015, 91, 051501. [Google Scholar] [CrossRef]
- Colangelo, G. Finite volume effects in chiral perturbation theory. Nucl. Phys. Proc. Suppl. 2005, 140, 120–126. [Google Scholar] [CrossRef]
- Sachrajda, C.T.; Villadoro, G. Twisted boundary conditions in lattice simulations. Phys. Lett. B 2005, 609, 73–85. [Google Scholar] [CrossRef]
- Bijnens, J.; Relefors, J. Chiral Perturbation Theory at Finite Volume and/or with Twisted Boundary Conditions. arXiv 2016, arXiv:1611.06068. [Google Scholar] [CrossRef][Green Version]
- Samanta, S.; Ghosh, S.; Mohanty, B. Finite size effect of hadronic matter on its transport coefficients. J. Phys. G 2018, 45, 075101. [Google Scholar] [CrossRef]
- Sarkar, N.; Deb, P.; Ghosh, P. Incomplete equilibration in high-multiplicity pp and pPb events at LHC. arXiv 2019, arXiv:1905.06532. [Google Scholar]
- Abreu, L.M.; Nery, E.S. Finite-size effects on the phase structure of the Walecka model. Phys. Rev. C 2017, 96, 055204. [Google Scholar] [CrossRef]
- Ishikawa, T.; Nakayama, K.; Suzuki, K. Casimir effect for nucleon parity doublets. Phys. Rev. D 2019, 99, 054010. [Google Scholar] [CrossRef]
- De, J.N.; Agrawal, B.K.; Samaddar, S.K. Equation of state of finite nuclei and liquid gas phase transition. Phys. Rev. C 1999, 59, 1. [Google Scholar] [CrossRef]
- Moretto, L.G.; Bugaev, K.A.; Elliott, J.B.; Ghetti, R.; Helgesson, J.; Phair, L. The Complement: A Solution to liquid drop finite size effects in phase transitions. Phys. Rev. Lett. 2005, 94, 202701. [Google Scholar] [CrossRef] [PubMed]
- Lombardo, M.P. Lattice QCD at finite density: Imaginary chemical potential. PoS CPOD 2006, 2006, 003. [Google Scholar] [CrossRef]
- Kashiwa, K. Imaginary Chemical Potential, NJL-Type Model and Confinement–Deconfinement Transition. Symmetry 2019, 11, 562. [Google Scholar] [CrossRef]
- Ebert, D.; Klimenko, K.G. Cooper pairing and finite-size effects in a NJL-type four-fermion model. Phys. Rev. D 2010, 82, 025018. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Espriu, D.; Planells, X. An effective QCD Lagrangian in the presence of an axial chemical potential. Eur. Phys. J. C 2013, 73, 2294. [Google Scholar] [CrossRef]
- Andrianov, A.A.; Espriu, D.; Planells, X. Chemical potentials and parity breaking: the Nambu–Jona-Lasinio model. Eur. Phys. J. C 2014, 74, 2776. [Google Scholar] [CrossRef]
- Gatto, R.; Ruggieri, M. Hot Quark Matter with an Axial Chemical Potential. Phys. Rev. D 2012, 85, 054013. [Google Scholar] [CrossRef]
- Yu, L.; Liu, H.; Huang, M. Spontaneous generation of local CP violation and inverse magnetic catalysis. Phys. Rev. D 2014, 90, 074009. [Google Scholar] [CrossRef]
- Yu, L.; Liu, H.; Huang, M. Effect of the chiral chemical potential on the chiral phase transition in the NJL model with different regularization schemes. Phys. Rev. D 2016, 94, 014026. [Google Scholar] [CrossRef]
- Braguta, V.V.; Kotov, A.Y. Catalysis of Dynamical Chiral Symmetry Breaking by Chiral Chemical Potential. Phys. Rev. D 2016, 93, 105025. [Google Scholar] [CrossRef]
- Cao, G.; Zhuang, P. Effects of chiral imbalance and magnetic field on pion superfluidity and color superconductivity. Phys. Rev. D 2015, 92, 105030. [Google Scholar] [CrossRef]
- Khunjua, T.G.; Klimenko, K.G.; Zhokhov, R.N. Dualities and inhomogeneous phases in dense quark matter with chiral and isospin imbalances in framework of effective model. arXiv 2019, arXiv:1901.02855 [hep-ph]. JHEP 06 (2019) 006. [Google Scholar]
- Ruggieri, M.; Peng, G.X. Critical Temperature of Chiral Symmetry Restoration for Quark Matter with a Chiral Chemical Potential. J. Phys. G 2016, 43, 125101. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khunjua, T.; Klimenko, K.; Zhokhov, R. Charged Pion Condensation in Dense Quark Matter: Nambu–Jona-Lasinio Model Study. Symmetry 2019, 11, 778. https://doi.org/10.3390/sym11060778
Khunjua T, Klimenko K, Zhokhov R. Charged Pion Condensation in Dense Quark Matter: Nambu–Jona-Lasinio Model Study. Symmetry. 2019; 11(6):778. https://doi.org/10.3390/sym11060778
Chicago/Turabian StyleKhunjua, Tamaz, Konstantin Klimenko, and Roman Zhokhov. 2019. "Charged Pion Condensation in Dense Quark Matter: Nambu–Jona-Lasinio Model Study" Symmetry 11, no. 6: 778. https://doi.org/10.3390/sym11060778
APA StyleKhunjua, T., Klimenko, K., & Zhokhov, R. (2019). Charged Pion Condensation in Dense Quark Matter: Nambu–Jona-Lasinio Model Study. Symmetry, 11(6), 778. https://doi.org/10.3390/sym11060778






