Overview of Low-Temperature Heat Capacity Data for Zn₂(C₈H₄O₄)₂^.C₆H₁₂N₂ and the Salam Hypothesis

Abstract

The review presents the progress in the analysis of low-temperature heat capacity of the metal-organic framework Zn₂(C₈H₄O₄)₂^.C₆H₁₂N₂ (Zn-DMOF). In Zn-DMOF, left-twisted D₃(S) and right-twisted D₃(R) DABCO molecules (C₆H₁₂N₂) can transform into each other by tunneling to form a racemate. Termination of tunneling leads to a phase transition in the subsystem of twisted molecules. It is suggested that Zn-DMOF may be considered a model system to study the mechanisms of phase transitions belonging to the same type as hypothetical Salam phase transitions.

1. Introduction

According to the Salam hypothesis, a small parity-violating energy difference (PVED) between amino acid molecules along with the Bose-Einstein (BE) condensation, makes the less stable right enantiomers tunnel into the more stable left enantiomers, by changing their structural forms. This process was described as a second-order phase transition, which is an analog of the Bardeen-Cooper-Schrieffer (BCS) phase transition; therefore, physical properties such as heat capacity and magnetic susceptibility should change during this phase transition according to the BCS laws [1,2]. Even though no such phase transitions have been found in the crystals of known amino acids, the building material of living organisms, the systems demonstrating the BE condensation of chiral molecules are still of interest. A model of BE condensation was developed for a gas of non-interacting chiral molecules to determine the PVED contribution from low-temperature heat capacity data [3]. The present review summarizes low-temperature heat capacity data, which indicate that the BE condensation may work in a subsystem of triethylenediamine (DABCO) molecules (C₆H₁₂N₂) in the metal-organic framework Zn₂(C₈H₄O₄)₂^.C₆H₁₂N₂ (Zn-DMOF) and that the mechanism of Salam phase transitions remains possible. In Zn-DMOF, the enantiomers are represented by left- and right-twisted DABCO molecules, which transform into each other as a result of tunneling.

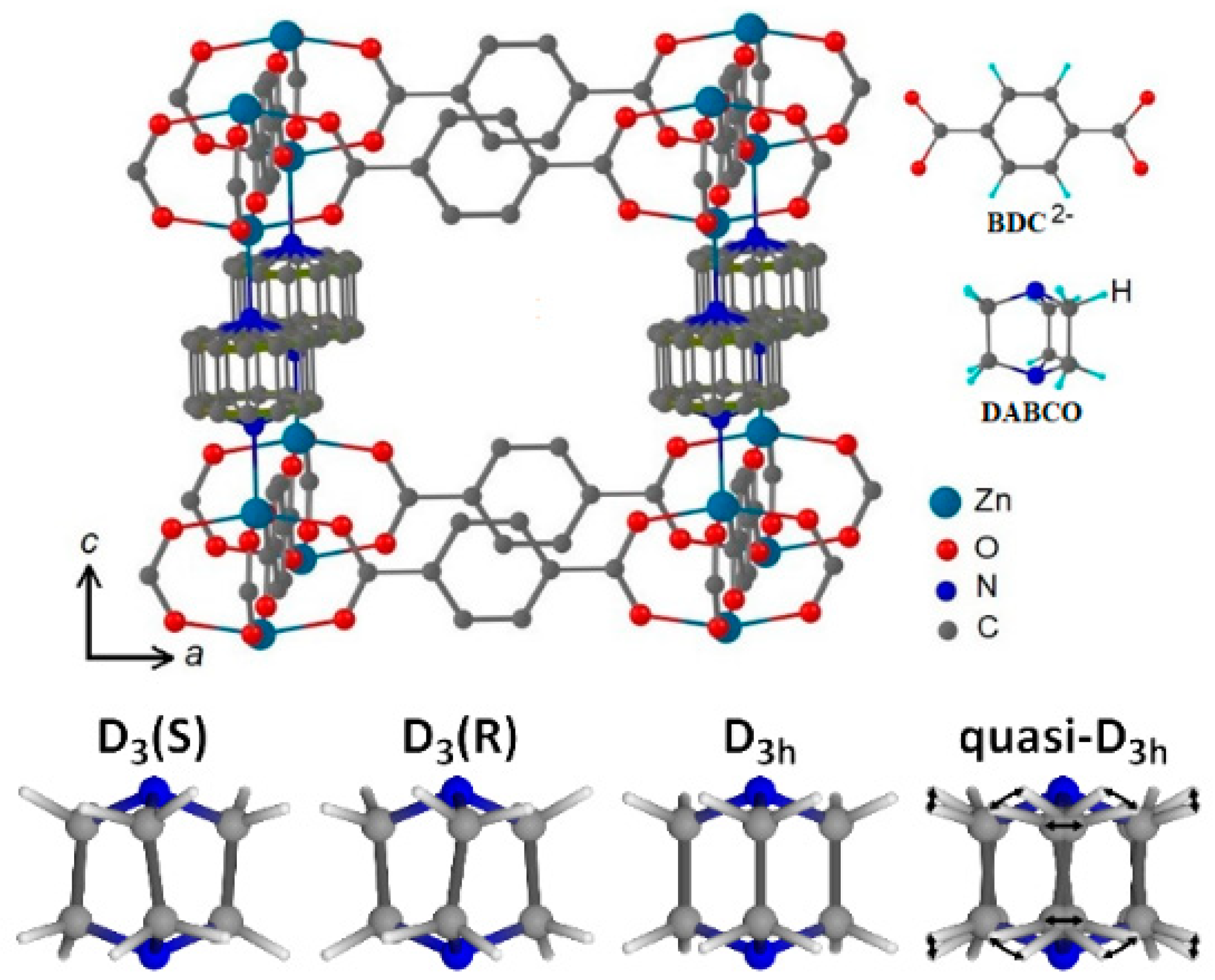
2. Structure of DABCO Molecule in Zn₂(C₈H₄O₄)₂·C₆H₁₂N₂

Triethylenediamine (DABCO) appears in the form of two conformational isomers with D_3h and D₃ point group symmetries, depending on intermolecular interactions. Also, a quasi-D_3h form of DABCO is possible due to strong vibrations of the molecule around the C₃ axis. The molecules with the D₃ symmetry, which can be left-twisted D₃(S) or right-twisted D₃(R), are considered to be chiral isomers (enantiomers) [4].

Above 223 K, the crystal structure of the metal-organic framework Zn-DMOF is tetragonal, with space group P4/mmm [5]. The horizontal planes are formed by terephthalate anions [C₈H₄O₄]²⁻ (BDC²⁻) which are linked to {Zn₂} pairs by carboxylate anions. The vertical edges are formed by DABCO molecules (linkers), the point symmetry of which does not contain a 4-fold rotational symmetry axis (Figure 1). This is the reason why DABCO molecules are orientationally disordered; moreover, D₃(S) and D₃(R) forms can transform into each other (by activation or tunneling) [4,6]. Calorimetry, nuclear magnetic resonance, and X-ray structural analysis data provide evidence of the presence of phase transitions in Zn-DMOF at ~14, ~60, and ~130 K [7,8,9,10,11,12].

3. Mobility of DABCO Molecules in Zn₂(C₈H₄O₄)₂·C₆H₁₂N₂

In Zn-DMOF, BDC²⁻ anions and DABCO molecules are involved in activation mobility. According to the nuclear magnetic resonance (NMR) studies of the activation mobility of BDC²⁻ anions, the [C₆H₄] groups of BDC²⁻ anions rotate about the C₂ axis through an angle of 180° (flipping) [13,14,15]. No effect of BDC²⁻ flipping on the mobility of DABCO in Zn-DMOF was discovered [13,16,17].

According to the detailed analysis of the temperature behavior of the spin-lattice relaxation times of hydrogen nuclei (¹H NMR T₁(T)), D₃(S) and D₃(R) forms of DABCO can make up a racemic mixture, and their mirror symmetry may be broken during the phase transition at ~60 K [6,11,12]. The time decay of nuclear magnetic moments (M) of hydrogen atoms in DABCO was analyzed to find the distribution of DABCO molecules over different states. Above ~165 K, the time decay of M is a single exponential function characterized by a single value T₁. In this case, DABCO molecules with D₃ and D_3h symmetries reorient similarly, their proton spins constitute a single spin system, the activation barrier is equal to ~4 kJ/mol. Between 165 and 60 K, the time decay of M is a biexponential function containing two values T₁, each corresponding to a certain fraction of nuclear spins in M. The ratio of these fractions is estimated to be ⅓:⅔. The ⅓·M fraction corresponds to ¹H spins of DABCO molecules of the D_3h symmetry, the mobility of which is characterized by a short value T_1SH. The ⅔·M fraction corresponds to ¹H spins of the sum of S- and R-forms of DABCO. In this case, these forms are indistinguishable due to tunneling transitions, so the above fraction (⅔·M) represents the racemic state of DABCO molecules and is characterized by a single value T_1L of a larger magnitude. During the phase transition at 60 K and down to 25 K, the behavior of T_1L is interpreted as the termination of tunneling between energy degenerate quantum states of R- and S- forms of DABCO, and their fractions in M remain equal to each other (⅓:⅓). Below 25 K, the decay M is nonexponential and can be conventionally characterized by three values T₁. So, the phase transition at ~14 K is associated with the redistribution of DABCO molecules over different energy states characterized by contributions ¼·M, ¼·M, and ½·M and the appearance of a chiral polarized state [11].

Note that the racemate state was also reported for 1,4-bis(carboxyethynyl)bicyclo[2.2.2]octane (BABCO) molecules, which are analogs of DABCO. It was shown that the ratio of left- and right- twisted forms of BABCO can be controlled by light [18,19]. The disorder of DABCO and its analogs, which causes phase transitions, is also observed in other systems [20,21,22].

Thus, the ¹H NMR T₁(T) data testify that phase transitions are associated with the mobility of DABCO molecules. The analysis of the function M provides quantitative data on the distribution of DABCO molecules over different states at various temperatures. However, it is still unclear how these states are structurally realized in Zn-DMOF. Low-temperature heat capacity data for Zn-DMOF may be used to clarify this problem.

4. Low-Temperature Heat Capacity in Zn₂(C₈H₄O₄)₂·C₆H₁₂N₂

All phase transitions in Zn-DMOF (at ~14, ~60, and ~130 K) are second-order phase transitions [10].

Table 1. ΔC_p (J/mol/K) values at the phase transitions in Zn-DMOF under various pressures of the heat-exchange gas ⁴He (P·10⁵,Pa).

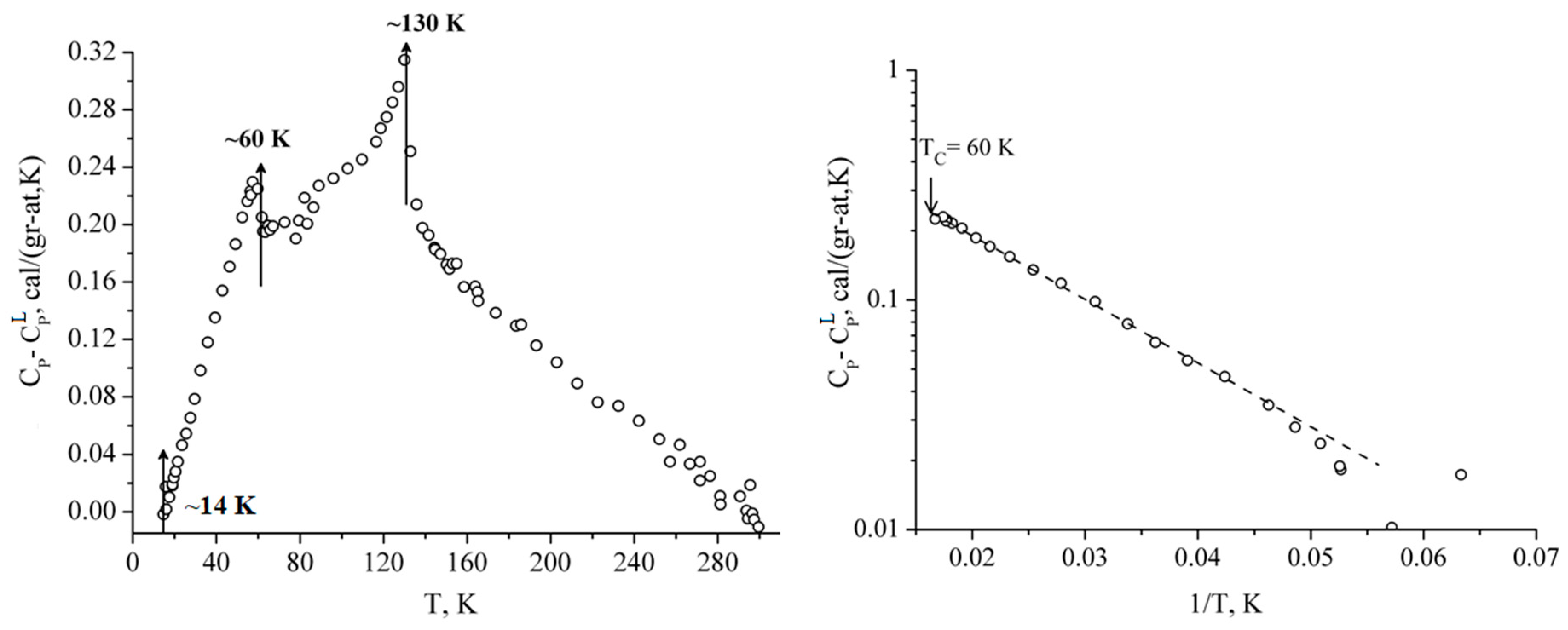
P	~14 K	~60 K	~130 K
0.51	6.0 ± 0.4	8.0 ± 0.2	23.0 ± 0.3
1.52	5.0 ± 0.4	11.0 ± 0.2	23.0 ± 0.3

shows the maximum values of the anomalous parts of heat capacity ΔC_p = C_p − C_p^L, where C_p is the heat capacity of the substance and C_p^L is the regular part of heat capacity "in the absence of phase transitions". The entropy of the phase transitions is shown in

Table 2. Entropies ΔS/R of the phase transitions in the region of critical temperatures (T_c, K) under various pressures of the heat-exchange gas ⁴He (P·10⁵,Pa) in Zn-DMOF. R is the universal gas constant.

Tc	~14	~60	~130
P	ΔS/R	ΔS/R	ΔS/R
0.51	0.42 ± 0.05	0.14 ± 0.02	0.30 ± 0.04
1.52	0.28 ± 0.04	0.23 ± 0.02	0.30 ± 0.04

.

The obtained data indicate that the absorbed atoms of ⁴He affect the states of D₃(S) and D₃(R) forms of DABCO (phase transitions ~14 and ~60 K) and do not affect the ordering and disordering of BDC²⁻ anions during the phase transition at 130 K. This result can be explained by the fact that the structure of DABCO is flexible [23,24] as compared to that of BDC²⁻ anions and can therefore be deformed in the presence of adjoining ⁴He atoms, whereas the structure of BDC²⁻ anions remains unchanged.

The temperature dependence of heat capacity of Zn-DMOF is almost linear between the phase transitions at ~14, ~60, and ~130 K and above 130 K [8,10,11] to indicate the presence of a one-dimensional elastic continuum [8,12]. Figure 2 shows the comparison of experimental [8] and tabulated (Tarasov model) [25,26] heat capacity values using the fitting parameters obtained in [11].

According to the XRD data, the crystal lattice of Zn-DMOF expands along the a and b axes (dL/LdT(a,b) = −9.59·10⁻⁶ K⁻¹) and shrinks along the c axis (dL/LdT(c) = 12.2·10⁻⁶ K⁻¹) as the temperature decreases to ~130 K and below ~130 K |dL/LdT(c)| > |dL/LdT(a,b)|(dL/LdT is the coefficient of thermal expansion) [9]. Hence, the interactions in the -Zn-DABCO-Zn- chain directed along the c axis are assumingly stronger than BDC²⁻-[Zn₂]⁴⁺-BDC²⁻- interactions in the ab plane, which determines a one-dimensional elastic continuum for the behavior of the heat capacity. The phase transition at ~130 K was interpreted as an order-disorder phase transition associated with a change in the relative spatial arrangement of BDC²⁻ anions, while the DABCO molecules preserve their activation mobility and remain disordered [9].

Linear regions of heat capacity in Zn-DMOF were analyzed using the Stockmayer-Hecht model for the heat capacity of chain crystalline polymers [26,27]. The model assumes that molecular groups in a chain vibrate as single units connected by strong intrachain bonds, while interchain interactions are neglected. The temperature dependence of the volumetric heat capacity C_v is expressed in terms of two relationships, C_v/(3Nk) and T/T_m, where N is the number of repeated vibrating units, T_m = hν_m/k, h is the Planck constant, k is the Boltzmann constant, and ν_m is the maximum frequency of stretching vibrations in the chain. The repeated vibrating unit along the c axis in Zn-DMOF consists of two Zn atoms and one DABCO molecule ({Zn₂DABCO}) [5].

Experimental smoothed values C_p obtained as functions of temperature in [8] were represented on a log-log plot and fitted by best tabulated values C_v/(3Nk) for each T/T_m assuming that C_p−C_v is small [27] (Figure 3). As a result, it was found that the vibrating chain is formed by ~38–39 {Zn₂DABCO} units above 130 K, by ~30 units at 60–130 K, and by ~12 units at 14–60 K (

Table 3. Calculated parameters for Zn-DMOF. $\stackrel{·}{\mathbf{M}}$ is the nuclear magnetic moment, $\stackrel{·}{\mathbf{N}}$ is the number of {Zn₂DABCO} units normalized with respect to corresponding values above 130 K.

Region of Fit, K	299.6–141.6	130.1–72.6	57.4–14.7
νm, cm−1	1250	765	285
N	~38.5	~28.9	~12.0
$\stackrel{·}{\mathbf{N}}$	1	~0.75	~0.31
$\stackrel{·}{\mathbf{M}}$	1	~0.67	~0.33

). Below 14 K, the heat capacity obeys the ~T³ law (Figure 3) to indicate that interchain interactions become stronger and the lattice vibrational modes become three-dimensional [8,25].

The values ν_m ~ 1250 cm⁻¹ and ν_m ~ 765 cm⁻¹ fall into the region of stretching vibrations of DABCO, and ν_m ~ 285 cm⁻¹ fall into the region of Zn-N and Zn-Zn stretchings (Table 3) [28]. Thus, the obtained values ν_m correspond to the stretchings in the chains, in accordance with the model [27].

As can be seen, the values obtained from the analysis of C_p for N {Zn₂DABCO} units correlate with fractions (M) in different phases of Zn-DMOF, if N and M values above 130 K are taken as a unit (Table 3). The obtained quantitative agreement between NMR data and the analysis of heat capacity suggests the following conclusions. Above 130 K, the chains consisting of ~39 {Zn₂DABCO} units contain DABCO molecules with D₃(S), D₃(R), and D_3h symmetries. At 60-130 K, the longest chains (~29 {Zn₂DABCO} units) contain only D₃ forms in the racemic state. The vibrations of these chains make the largest contribution to the heat capacity, while the vibrations of the chains consisting of D_3h forms make no contribution practically, due to their shorter size. Finally, below ~60 K there are three types of chains (~12 {Zn₂DABCO} units) of the same length but containing three different DABCO forms (D₃(S), D₃(R), and D_3h). The size of the chains below 14 K cannot be estimated, since the heat capacity is no more linear at these temperatures.

5. Heat Capacity Behavior during the Phase Transition at 60 K and the Salam Hypothesis

The PVED values for the DABCO molecule and the [Zn₂DABCO]⁴⁺ cation were obtained in [29]. The difference between the energies of mirror isomers is as small as ~5·10⁻¹⁶ kJ/mol (~5.2·10⁻¹⁸ eV) for DABCO and an order of magnitude higher (~5·10⁻¹⁵ kJ/mol or ~5.2·10⁻¹⁷ eV) for the [Zn₂DABCO]⁴⁺ cation. Therefore, the contribution of PVED increases in the presence of Zn²⁺ cations and is determined mainly by the contribution of zinc cations. This contribution increases if Zn²⁺ cations are replaced by heavier cations Cd²⁺ and Hg²⁺ [30]. Hence, it can be assumed that the PVED breaking of mirror symmetry between D₃(S) and D₃(R) forms of DABCO may be caused by their external environment in the Zn-DMOF structure.

If the symmetry breaking during the phase transition at ~60 K takes place in the chains containing only D₃(S) and D₃(R) forms of DABCO, then, according to the Salam hypothesis, the behavior of heat capacity must correspond to the behavior of heat capacity during the superconducting phase transition [1,2].

In fact, it was discovered that the temperature behavior of heat capacity of Zn-DMOF is an exponential function below ~60 K (Figure 4) [31]. The behavior of heat capacity in the region of second-order phase transitions was studied using the values of the anomalous part of heat capacity ΔC_p = C_p − C_p^L, and the behavior of C_p^L was described using the Tarasov model [25]. Figure 3 shows the obtained ΔC_p values.

The region below ~60 K is of particular interest, since it is associated with the termination of tunneling between D₃(S) and D₃(R) forms of DABCO as the temperature decreases [11,12]. Based on the hypothesis suggested in [1,2], a study was carried out to verify the compliance of heat capacity ΔC_p to the exponential dependence ~ exp(−Δ/T), гдe Δ = 1.76·T_c (Δ is the energy gap at 0 K). Figure 4 shows ΔC_p as a function of 1/T in the temperature region 15 K < T < 60 K. As can be seen, a good agreement with the exponential law is achieved for the parameter Δ equal to ~56 K (or ~5 ·10⁻³ eV). [31]. The obtained value Δ turned out to be almost twice as small as expected (~106 K for T_c = 60 K). There is probably some inaccuracy with the parameters determining function C_p^L, which may cause the error of determining the Δ value. However, the detected exponential behavior of ΔC_p below 60 K signifies the presence of a BE condensation. The amplitude of ΔC_p during the phase transition at is 60 K ≈ 10 J/mol/K (Table 1), which corresponds to the thermal energy jump (ΔC_p·T_c) ≈ 600 J/mol (or 6·10⁻³ eV), which agrees well with Δ. The value of (ΔC_p·T_c) is 10¹⁵ times bigger than the PVED (~5.2·10⁻¹⁸ eV) of one DABCO molecule, but it can be explained by the phenomenon of BE condensation [1,2].

However, neither C_p data nor ¹H NMR T₁(T) data show any energy difference between D₃(S) and D₃(R) forms of DABCO below 60 K (according to the Salam hypothesis, the ratio between D₃(S) and D₃(R) forms of DABCO should change). Apparently, the energy difference between D₃(S) and D₃(R) forms remains negligible and can be observed only at lower temperatures, when the thermal energy of the crystal approaches zero [6]. Indeed, according to ¹H NMR T₁(T) data, the decay M as a function of time shows anomalous behavior below 25 K [12], but it is not manifested in the C_p behavior until the phase transition at ~14 K.

6. Conclusions

The Salam hypothesis is considered impossible during the phase transitions in amino acid crystals, since the barriers between L- and D-forms of alanine involve intramolecular bond breaking and are as high as ~200 kJ/mol [32]. In Zn-DMOF, the activation barrier between D₃(S) and D₃(R) forms of DABCO is estimated to be ~4 kJ/mol [7] and 5 kJ/mol [27] according to NMR data and quantum chemical calculations, respectively. Thus, this barrier is ~40 times smaller than the barrier between L- and D-forms of alanine [30]. The NMR data indicate the presence of tunneling between D₃(S) and D₃(R) forms of DABCO. The tunneling splitting for the DABCO molecule in the free state is estimated to be ~6 cm¹⁻ (~8.6 K) [24], which is comparable to the temperature range of observed phase transitions in Zn-DMOF. The behavior of heat capacity below 60 K corresponds to the heat capacity during the BE condensation. According to the NMR data, still lower temperatures are associated with a redistribution of DABCO with different symmetries over energy states to form a chiral polarized state. In the model system [Zn₂DABCO]⁴⁺, the R-form is most favorable due to the PVED [29,30], but it is currently unclear which symmetry of the chains built of {Zn₂DABCO} units corresponds to the most energetically favorable state. The method of resonant X-ray diffraction with circularly polarized X-rays [33] or optical methods seem to be most preferable for use at low and extra-low temperatures.

We believe that metal-organic frameworks or related compounds containing enantiomers in the racemic state (not necessarily amino acid molecules) may be considered as model systems to study Salam phase transitions. Our studies were aimed at revealing the effects of chirality stabilization in isomeric molecules in solids at low temperatures with the goal of exploring the idea of the cold scenario of life origin on the Earth.